Degradation of Mechanical Properties of Pine Wood Under Symmetric Axial Cyclic Loading Parallel to Grain
Abstract
:1. Introduction
2. Materials and Methods
2.1. Preliminary Tests
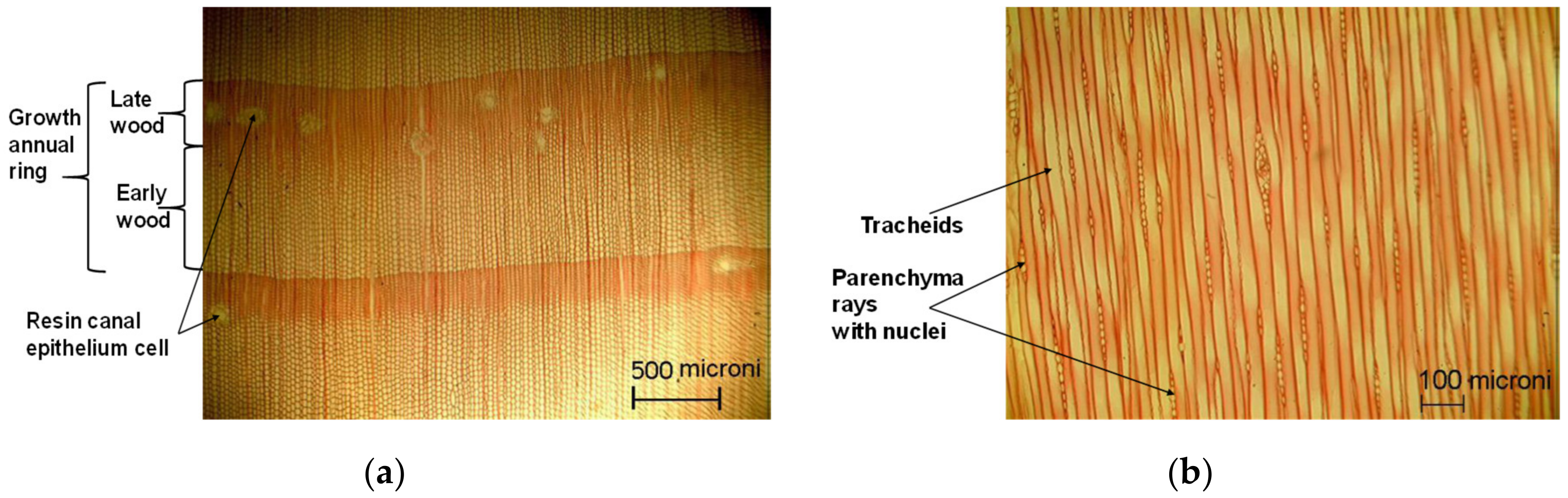
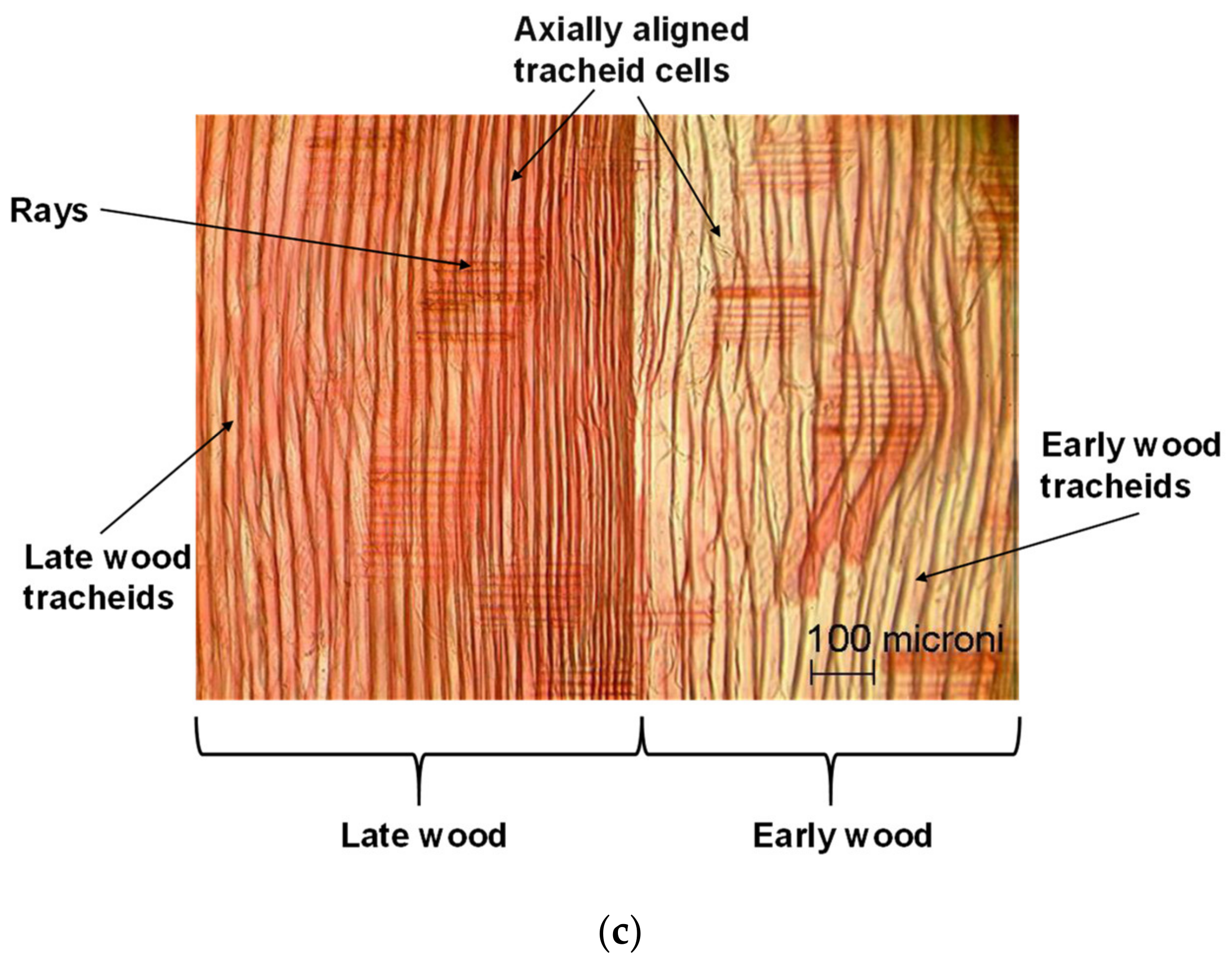
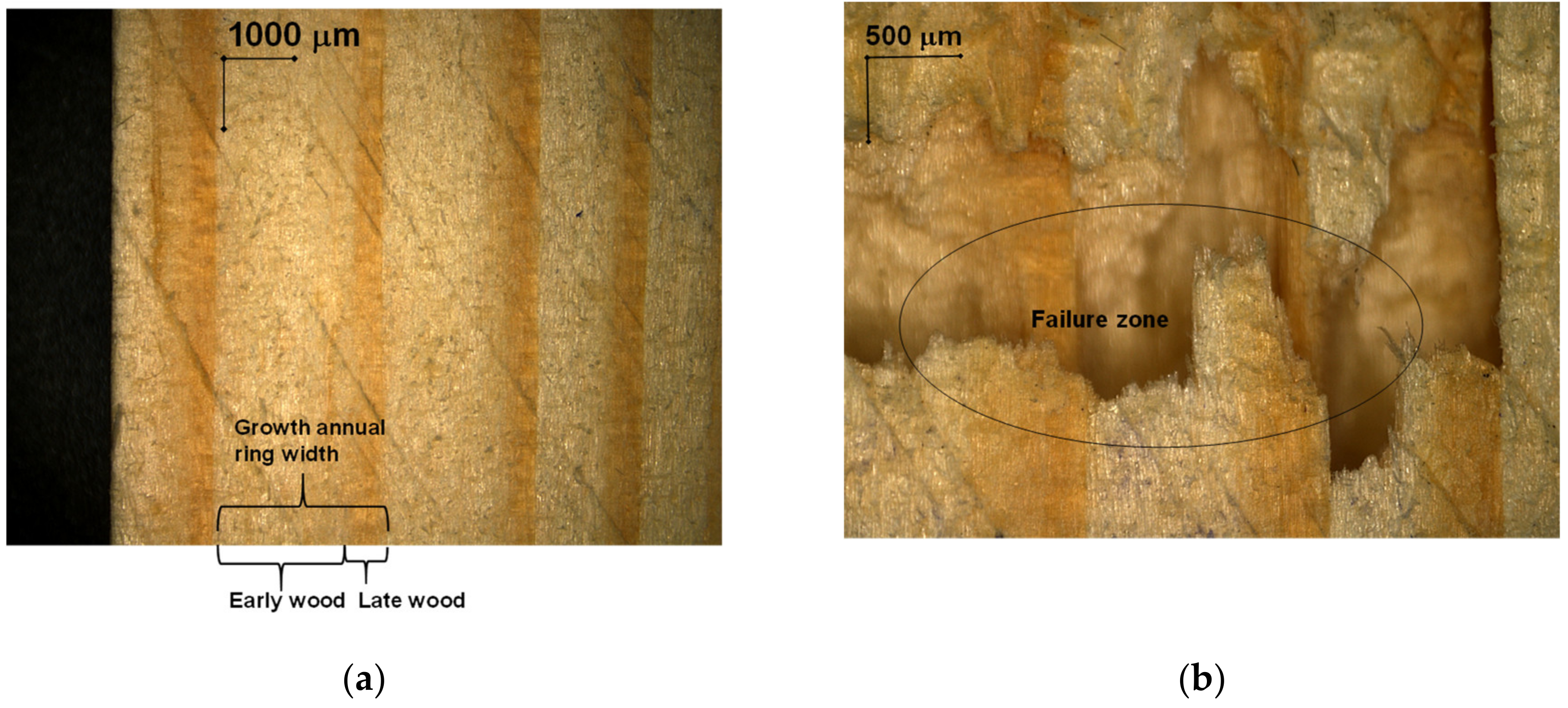
2.2. Materials
2.3. Methods
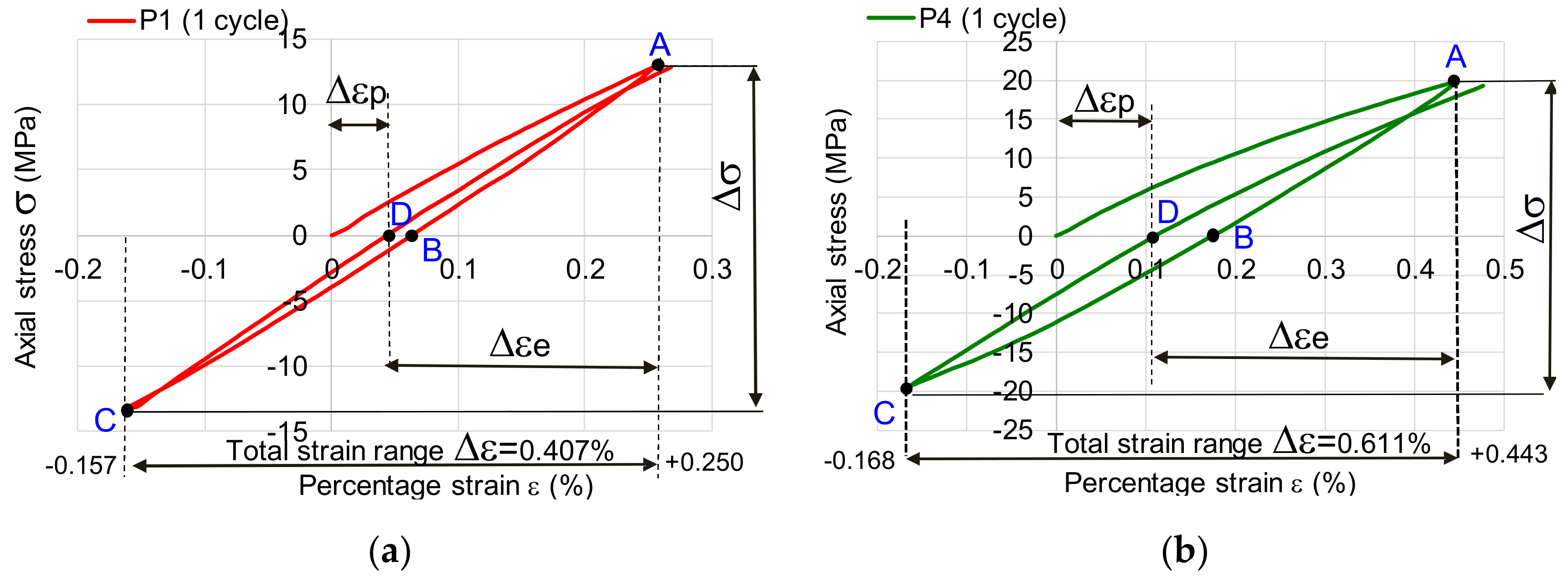
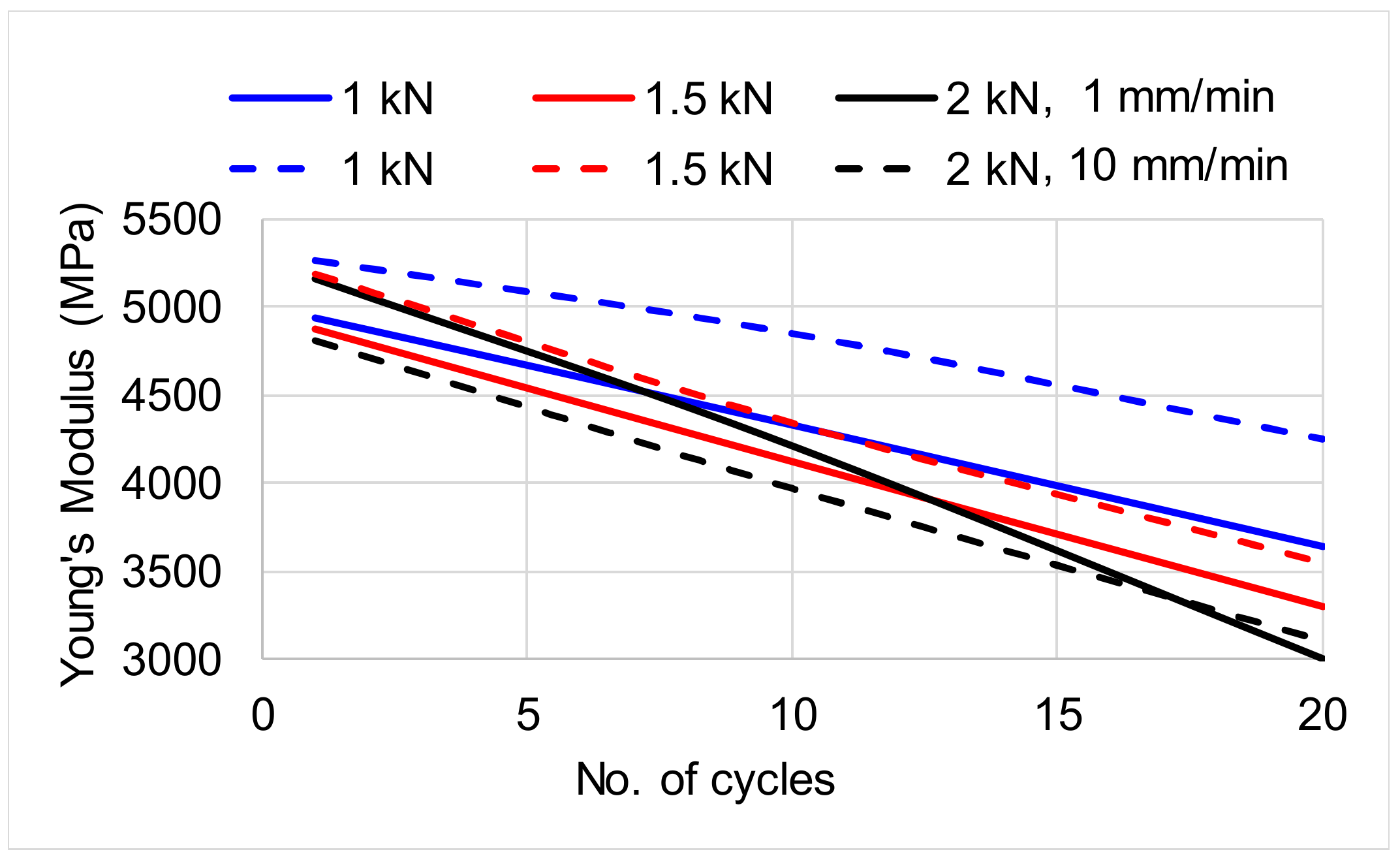
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Gong, M.; Smith, I. Short-term mechanical behaviour of softwood in high-stress-level compression parallel to grain. In Proceedings of the Fourth International Conference on the Development of Wood Science, Wood Technology and Forestry, Missenden Abbey, UK, 14–16 July 1999; pp. 25–34. [Google Scholar]
- Ion, F.; Taranu, N.; Secu, A.; Entuc, I.S.; Scutaru, M.C.; Ungureanu, D. Evaluation of the wood strength class using the experimental approach. Bull. Polytech. Inst. IasiConstr. Archit. Sect. 2017, 63, 121–132. [Google Scholar]
- Silvestru Grigore, C.V.; Dinulică, F.; Spârchez, G.; Hălălișan, A.F.; Dincă, L.C.; Enescu, R.E.; Crișan, V.E. Radial Growth Behavior of Pines on Romanian Degraded Lands. Forests 2018, 9, 213. [Google Scholar] [CrossRef] [Green Version]
- Christoforo, A.L.; Panzera, T.H.; Lahr, F.A.R. Estimation of tensile strength parallel to grain of wood species. Eng. Agric. 2019, 39, 533–536. [Google Scholar] [CrossRef] [Green Version]
- Dai, D.; Fan, M. Wood fibres as reinforcements in natural fibre composites: Structure, properties, processing and applications. In Natural Fibre Reinforcements. Materials, Processes and Applications; Woodhead Publishing: Sawston, UK, 2014; pp. 3–65. [Google Scholar] [CrossRef]
- Feio, A.; Machado, J.; Lourenco, P. Parellel to the grain behavior and NDT correlations for chestnut wood (Castanea sativa Mill). In Proceedings of the International Conference “Conservation of Historic Wooden Structures”, Florence, Italy, 22–27 February 2005. [Google Scholar]
- Fakoor, M.; Sabour, M.H.; Khansari, N.M. A new approach for investigation of damage zone properties in orthotropic materials. Eng. Solid Mech. 2014, 2, 283–292. [Google Scholar] [CrossRef]
- Gaff, M.; Gašparìk, M. Effect of cyclic loading on modulus of elasticity of Aspen wood. Bioresources 2015, 10, 290–298. [Google Scholar] [CrossRef] [Green Version]
- Konstantinov, A.Y.; Lomunov, A.K.; Iuzhina, T.N.; Gray, G.T., III. Investigation of wood anisotropy under dynamic loading. Strength Ductility Probl. 2018, 80, 555–565. [Google Scholar] [CrossRef]
- Pečnik, J.G.; Kutnar, A.; Militz, H.; Schwarzkopf, M.; Schwager, H. Fatigue behavior of beech and pine wood modified with low molecular weight phenol-formaldehyde resin. Holzforschung 2020. published online. [Google Scholar] [CrossRef]
- Sharapov, E.; Mahnert, K.C.; Militz, H. Residual strength of thermally modified Scots pine after fatigue testing in flexure. Eur. J. Wood Wood Prod. 2016, 74, 875–884. [Google Scholar] [CrossRef]
- Pinho, S.T.; Iannucci, L.; Robinson, P. Physically based failure models and criteria for laminated fibre-reinforced composites with emphasis on fibre kinking. Part II: FE implementation. Compos. Part A 2006, 37, 766–777. [Google Scholar] [CrossRef]
- Yildirim, M.N.; Uysal, B.; Ozcifci, A.; Ertas, A.H. Determination of fatigue and static strength of scots pine and beech wood. Wood Res. 2015, 60, 679–686. [Google Scholar]
- Hassani, M.M.; Wittel, F.K.; Hering, S.; Herrmann, H.J. Rheological Model for Wood. Comp. Method Appl. M 2014, 283, 1032–1060. [Google Scholar] [CrossRef]
- Andrianopoulos, N.; Manolopoulos, V. Can Coulomb criterion be generalized in case of ductile materials? An application to Bridgman experiments. Int. J. Mech. Sci. 2012, 54, 241–248. [Google Scholar] [CrossRef]
- Stanciu, M.D.; Șova, D.; Savin, A.; Iliaș, N.; Gorbacheva, G. Physical and Mechanical Properties of Ammonia- Treated Black Locust Wood. Polymers 2020, 12, 377. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Stanciu, M.D.; Teodorescu, D.H.; Tămaș, F.; Terciu, O.M. Mechanical and Rheological Behaviour of Composites Reinforced with Natural Fibres. Polymers 2020, 12, 1402. [Google Scholar] [CrossRef] [PubMed]
- EN 384. Structural Timber. In Determination of Characteristic Values of Mechanical Properties and Density; CEN European Committee for Standardization: Brussels, Belgium, 2004. [Google Scholar]
- ASTM D143-14 Standard Test Method for Small Clear Specimen of Timber; American Society for Testing Material Standard: West Conshohocken, PA, USA, 2014.
- EN 408. Timber Structures-Structural Timber and Glued Laminated Timber -Determination of Some Physical and Mechanical Properties; British Standards Institution: London, UK, 2003. [Google Scholar]
- Varenik, K.A.; Varenik, A.S.; Sanzharovskij, R.C. Boltzmann principle of superposition in the theory of wood creep for deformations in time. In IOP Conf. Series: Materials Science and Engineering; IOP Publishing: Bristol, UK, 2018; Volume 441, p. 012057. [Google Scholar] [CrossRef]
- Metri, V.; Briels, W.J. Brownian dynamics investigation of the Boltzmann superposition principle for orthogonal superposition rheology. J. Chem. Phys. 2019, 150, 014903. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Ferreira, D.; Fonseca, E.; Pinto, C.; Borges, P. Tensile strength of pine and ash woods–experimental and numerical study. In Proceedings of the 6th International Conference on Mechanics and Materials in Design, Ponta Delgada, Azores, 26–30 July 2015. [Google Scholar]
- Kloiber, M.; Drdácky´, M.; Machado, J.S.; Piazza, M.; Yamaguchi, N. Prediction of mechanical properties by means of semi-destructive methods: A review. Constr. Build. Mater. 2015, 101, 1215–1234. [Google Scholar] [CrossRef] [Green Version]
- Strömbro, J.; Gudmunson, P. Mechano-sorptive creep under compressive loading–A micromechanical model. Int. J. Solids Struct. 2008, 45, 2420–2450. [Google Scholar] [CrossRef] [Green Version]
- Gutkin, R.; Pinho, S.T. Combining damage and friction to model compressive damage growth in fibre-reinforced composites. J. Compos. Mater. 2014, 49, 2483–2495. [Google Scholar] [CrossRef] [Green Version]
- Sirumbal-Zapata, L.; Málaga-Chuquitaype, C.; Elghazouli, A. A three-dimensional plasticity-damage constitutive model for timber under cyclic loads. Comput. Struct. 2018, 195, 47–63. [Google Scholar] [CrossRef]
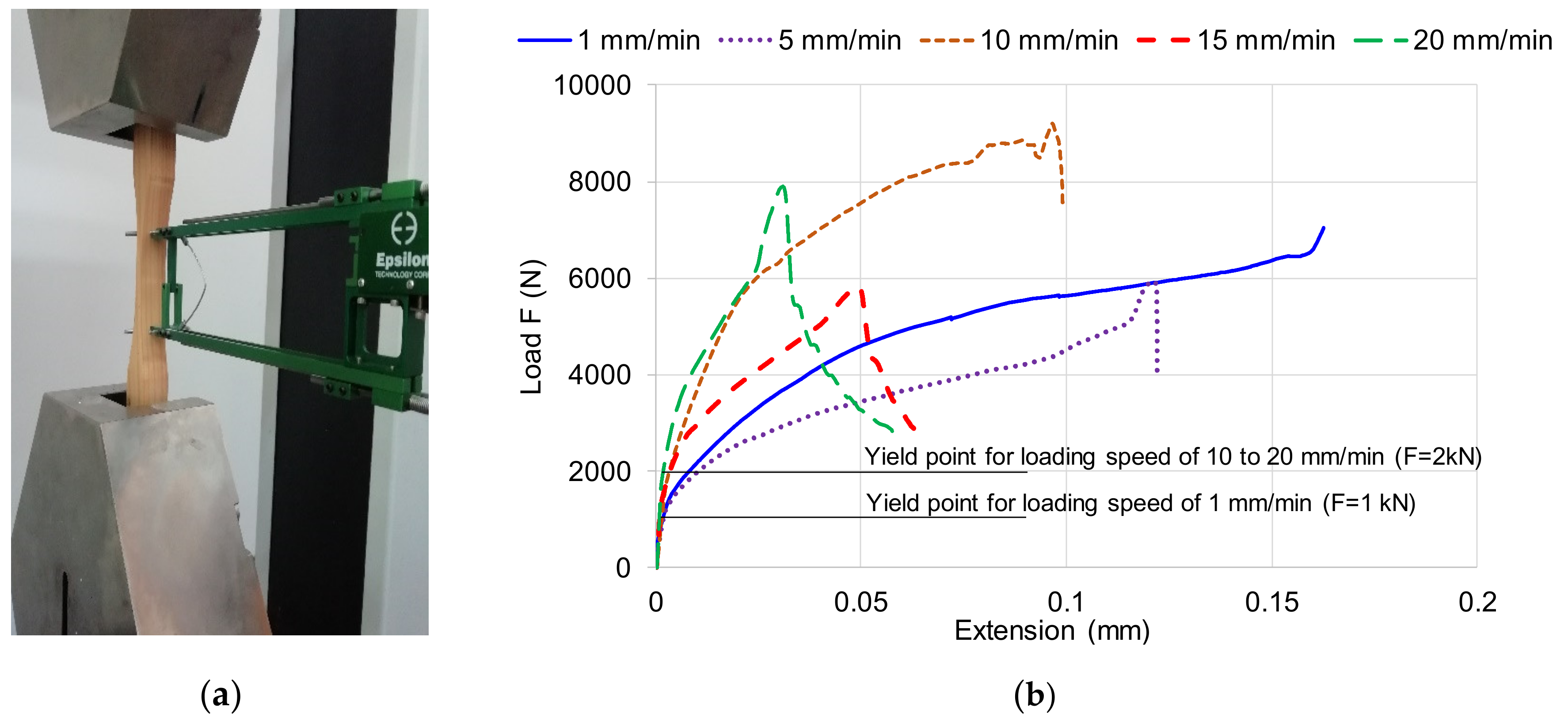
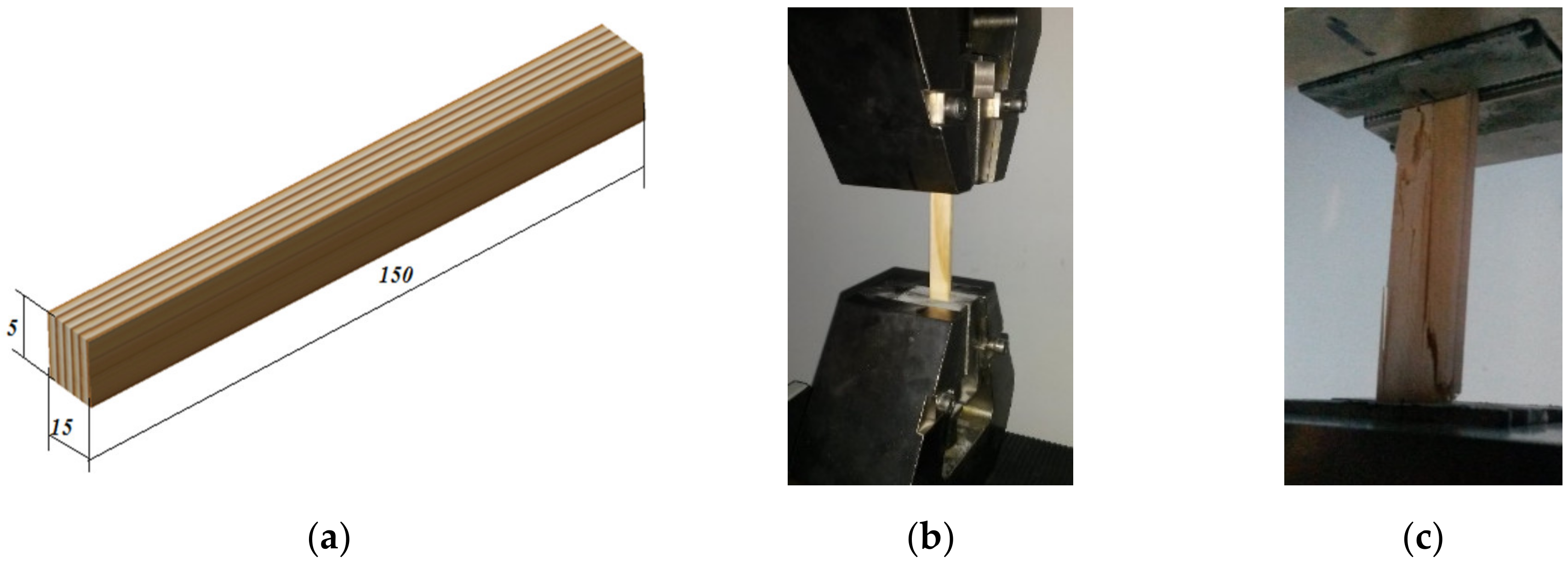
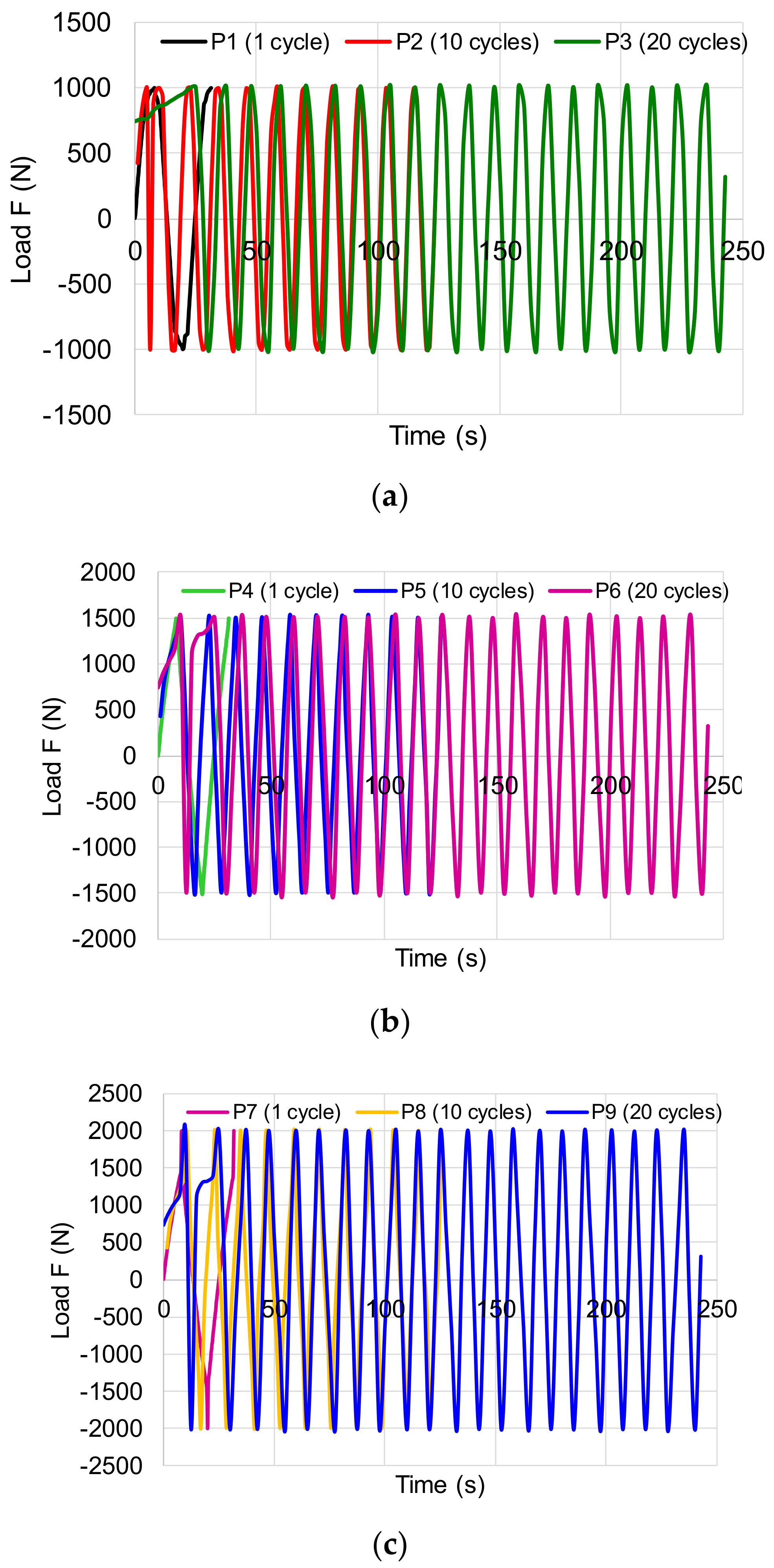






| Set of Tests | Sample | No of Cycles | Gauge Length (mm) | Speed (mm/min) | Width (mm) | Thickness (mm) | Area (mm2) | Limit of Tensile/ Compression Loading (kN) |
|---|---|---|---|---|---|---|---|---|
| A1 | P1 | 1 | 60 | 1 | 15 | 5 | 75 | ±1 |
| P2 | 10 | 60 | 1 | 15 | 5 | 75 | ±1 | |
| P3 | 20 | 60 | 1 | 15 | 5 | 75 | ±1 | |
| B1 | P4 | 1 | 60 | 1 | 5 | 5 | 75 | ±1.5 |
| P5 | 10 | 60 | 1 | 15 | 5 | 75 | ±1.5 | |
| P6 | 20 | 60 | 1 | 15 | 5 | 75 | ±1.5 | |
| C1 | P7 | 1 | 60 | 1 | 15 | 5 | 75 | ±2 |
| P8 | 10 | 60 | 1 | 15 | 5 | 75 | ±2 | |
| P9 | 20 | 60 | 1 | 15 | 5 | 75 | ±2 | |
| A10 | P10 | 1 | 60 | 10 | 15 | 5 | 75 | ±1 |
| P11 | 10 | 60 | 10 | 15 | 5 | 75 | ±1 | |
| P12 | 20 | 60 | 10 | 15 | 5 | 75 | ±1 | |
| B10 | P13 | 1 | 60 | 10 | 15 | 5 | 75 | ±1.5 |
| P14 | 10 | 60 | 10 | 15 | 5 | 75 | ±1.5 | |
| P15 | 20 | 60 | 10 | 15 | 5 | 75 | ±1.5 | |
| C10 | P16 | 1 | 60 | 10 | 15 | 5 | 75 | ±2 |
| P17 | 10 | 60 | 10 | 15 | 5 | 75 | ±2 | |
| P18 | 20 | 60 | 10 | 15 | 5 | 75 | ±2 |
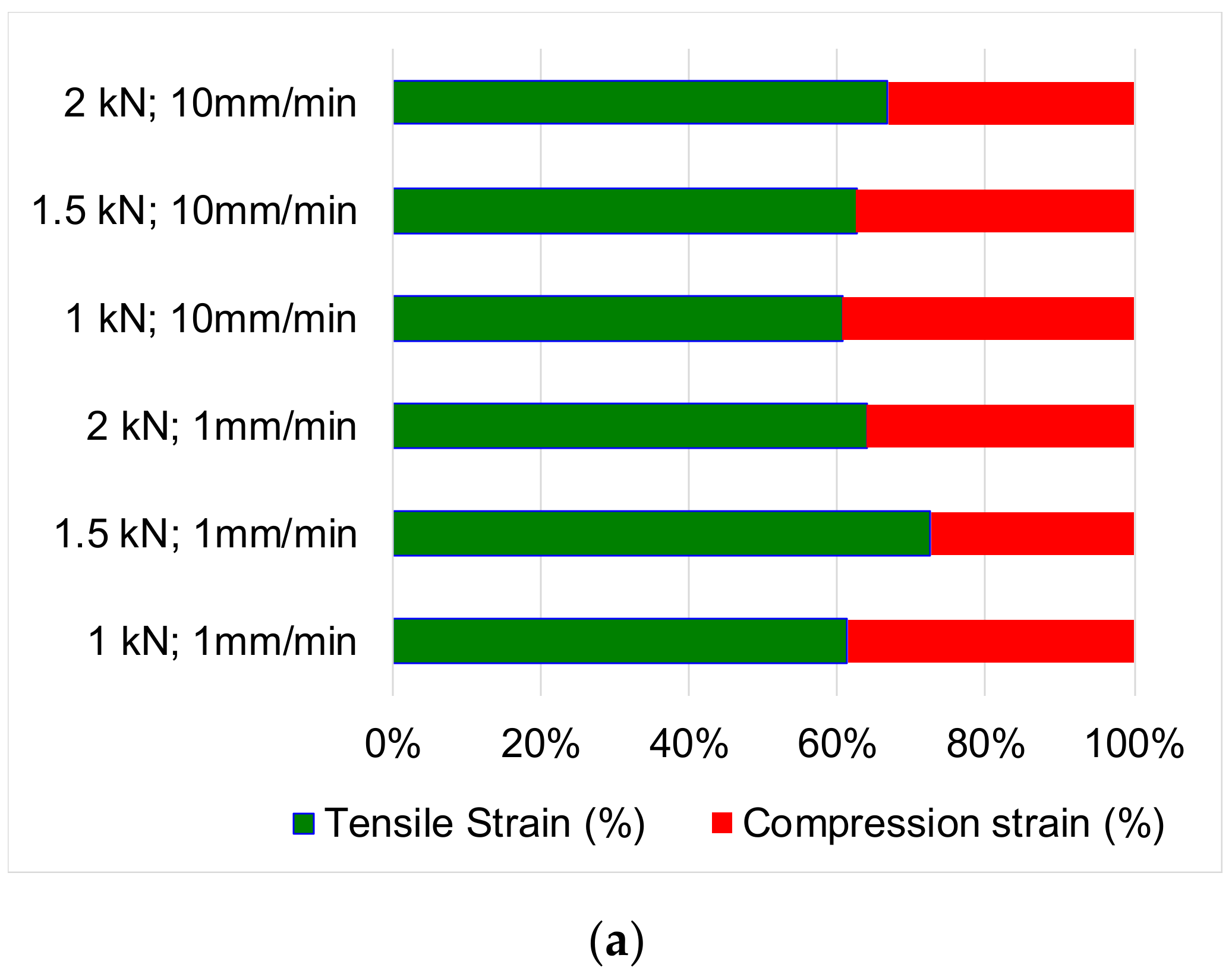
| Set of Tests | Δε = εt +εc (%) | Δεe (%) | Δεp (%) | εt (%) | εc (%) |
|---|---|---|---|---|---|
| A1 | 0.407 | 0.2042 | 0.0458 | 0.250 | 0.157 |
| B1 | 0.611 | 0.3422 | 0.1008 | 0.443 | 0.168 |
| C1 | 0.969 | 0.5368 | 0.0832 | 0.620 | 0.349 |
| A10 | 0.402 | 0.1982 | 0.0458 | 0.244 | 0.158 |
| B10 | 0.639 | 0.3212 | 0.0788 | 0.400 | 0.239 |
| C10 | 1.036 | 0.5566 | 0.1354 | 0.692 | 0.344 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Stanciu, M.D.; Teodorescu, H.D.; Vlase, S. Degradation of Mechanical Properties of Pine Wood Under Symmetric Axial Cyclic Loading Parallel to Grain. Polymers 2020, 12, 2176. https://doi.org/10.3390/polym12102176
Stanciu MD, Teodorescu HD, Vlase S. Degradation of Mechanical Properties of Pine Wood Under Symmetric Axial Cyclic Loading Parallel to Grain. Polymers. 2020; 12(10):2176. https://doi.org/10.3390/polym12102176
Chicago/Turabian StyleStanciu, Mariana D., Horațiu D. Teodorescu, and Sorin Vlase. 2020. "Degradation of Mechanical Properties of Pine Wood Under Symmetric Axial Cyclic Loading Parallel to Grain" Polymers 12, no. 10: 2176. https://doi.org/10.3390/polym12102176
APA StyleStanciu, M. D., Teodorescu, H. D., & Vlase, S. (2020). Degradation of Mechanical Properties of Pine Wood Under Symmetric Axial Cyclic Loading Parallel to Grain. Polymers, 12(10), 2176. https://doi.org/10.3390/polym12102176







