Fused Deposition Modeling of Polyamides: Crystallization and Weld Formation
Abstract
1. Introduction
2. Materials and Methods
3. Results and Discussion
3.1. Thermal Analysis
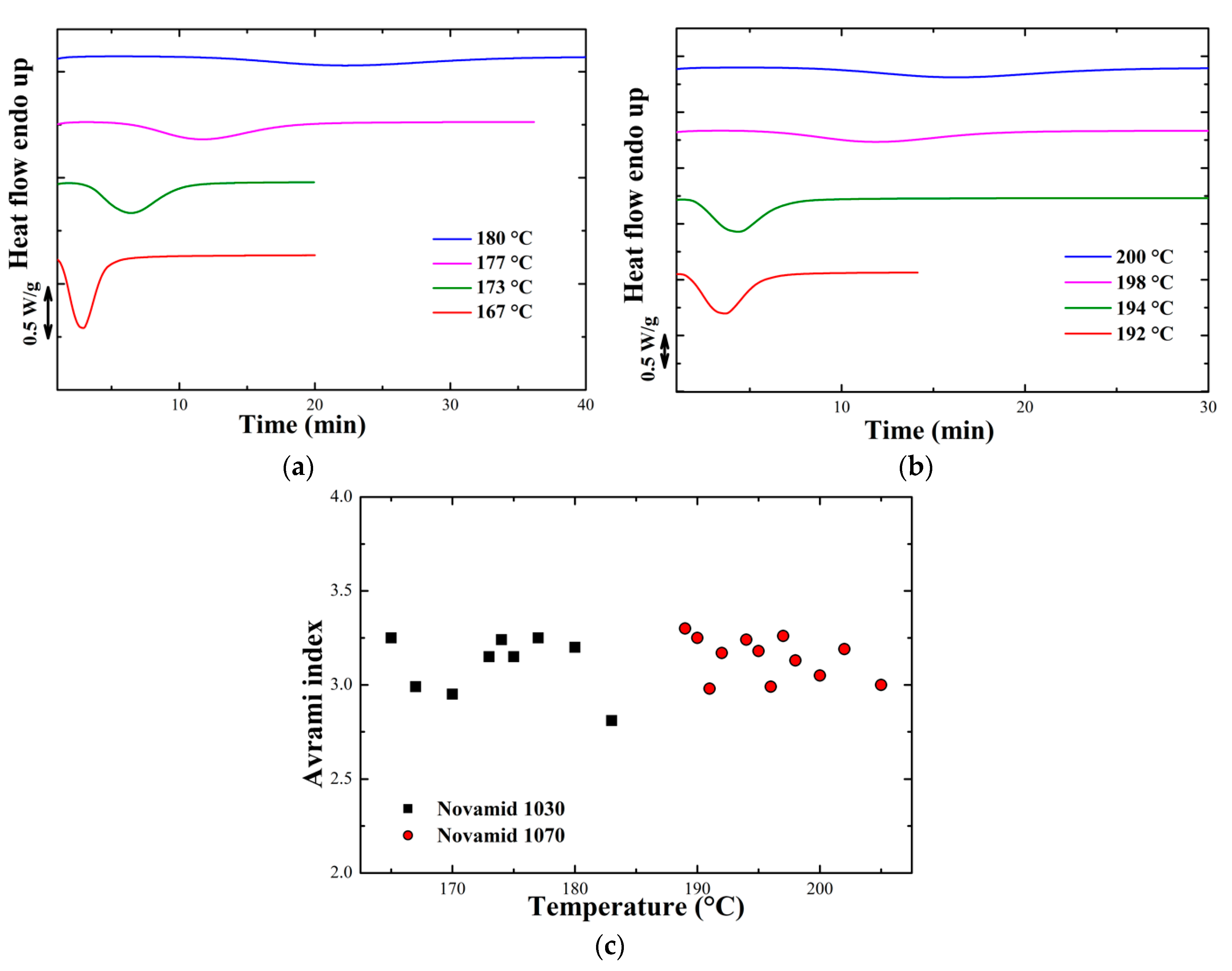
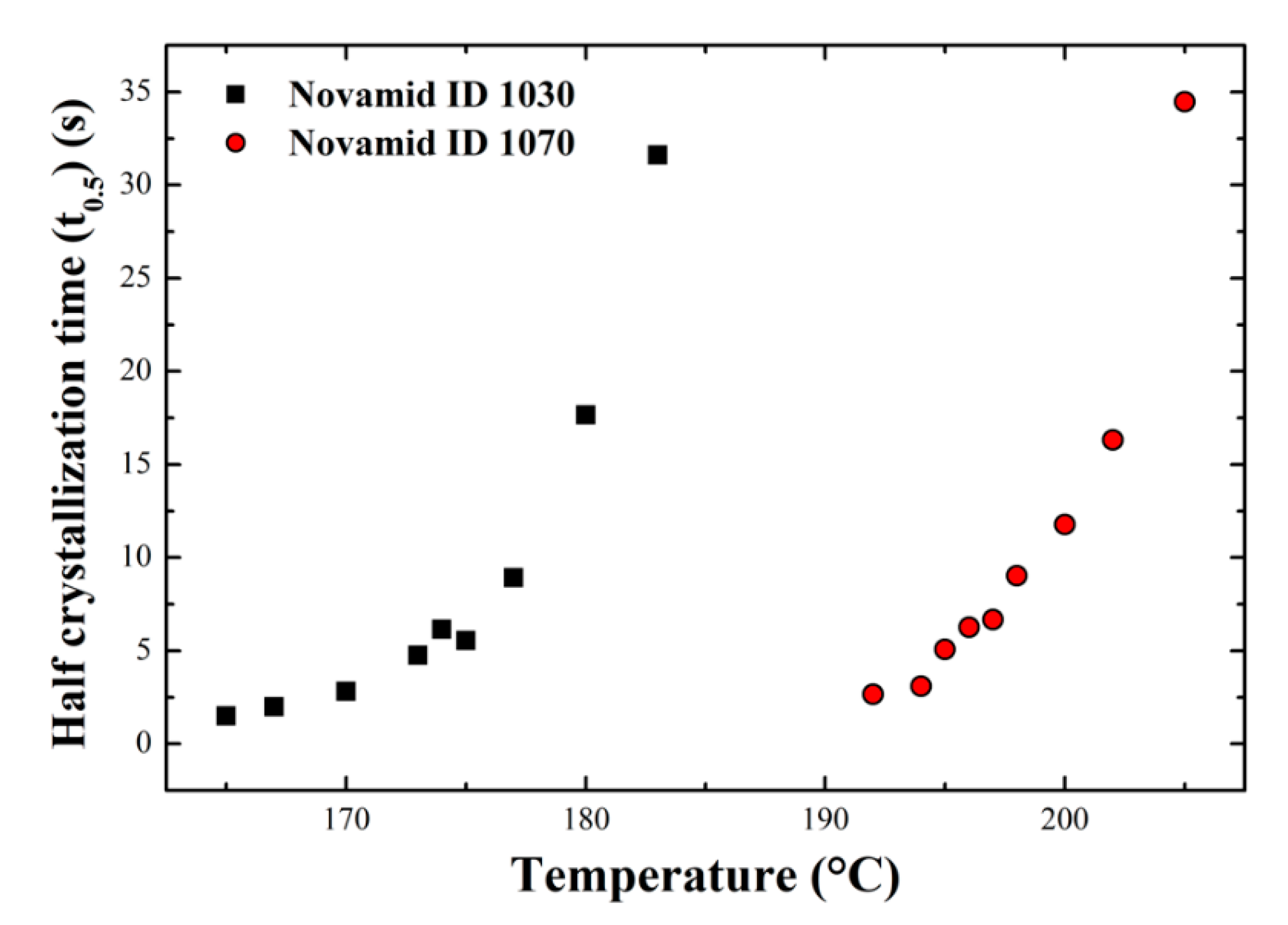
3.1.1. Isothermal Characterization
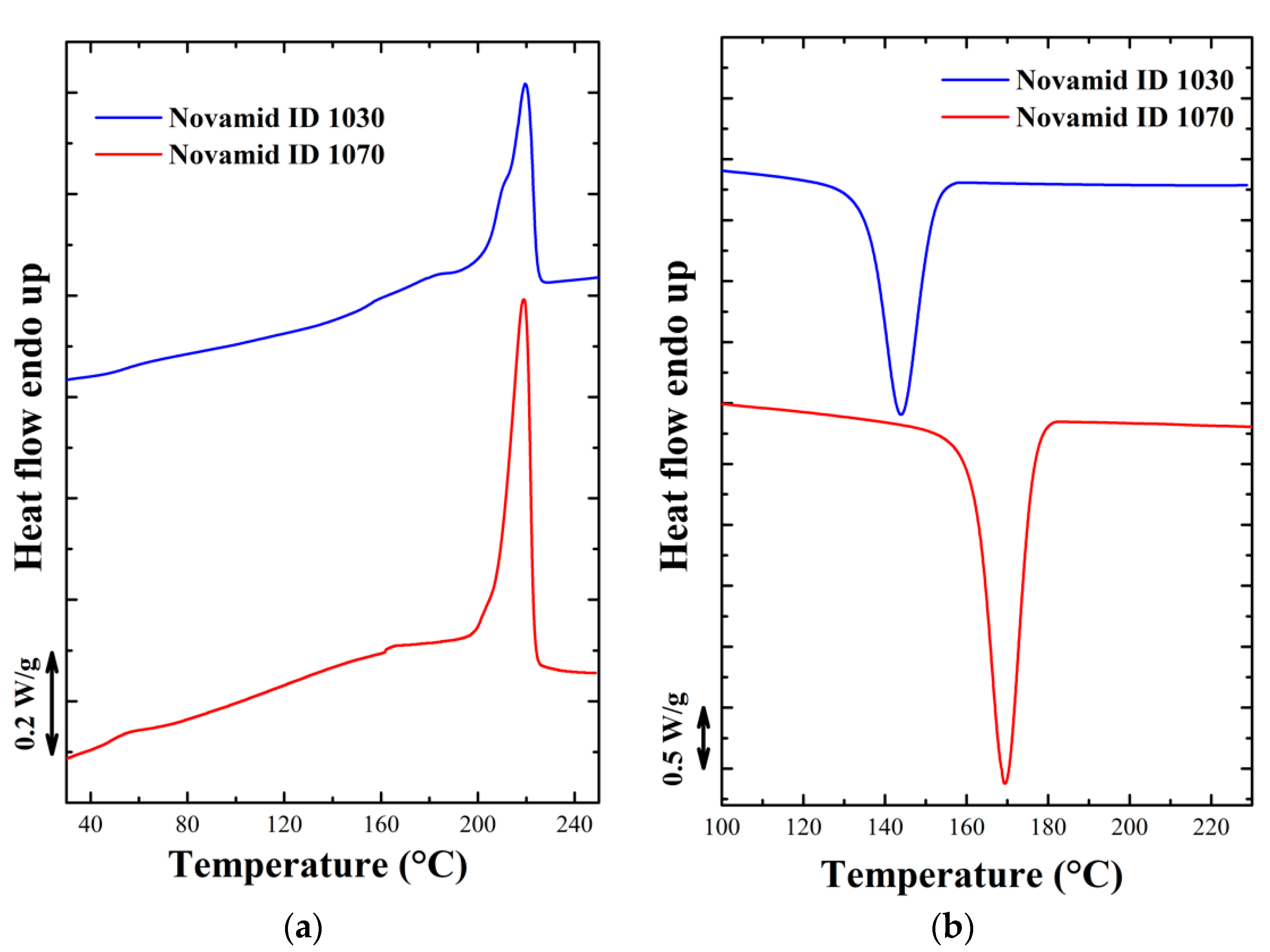
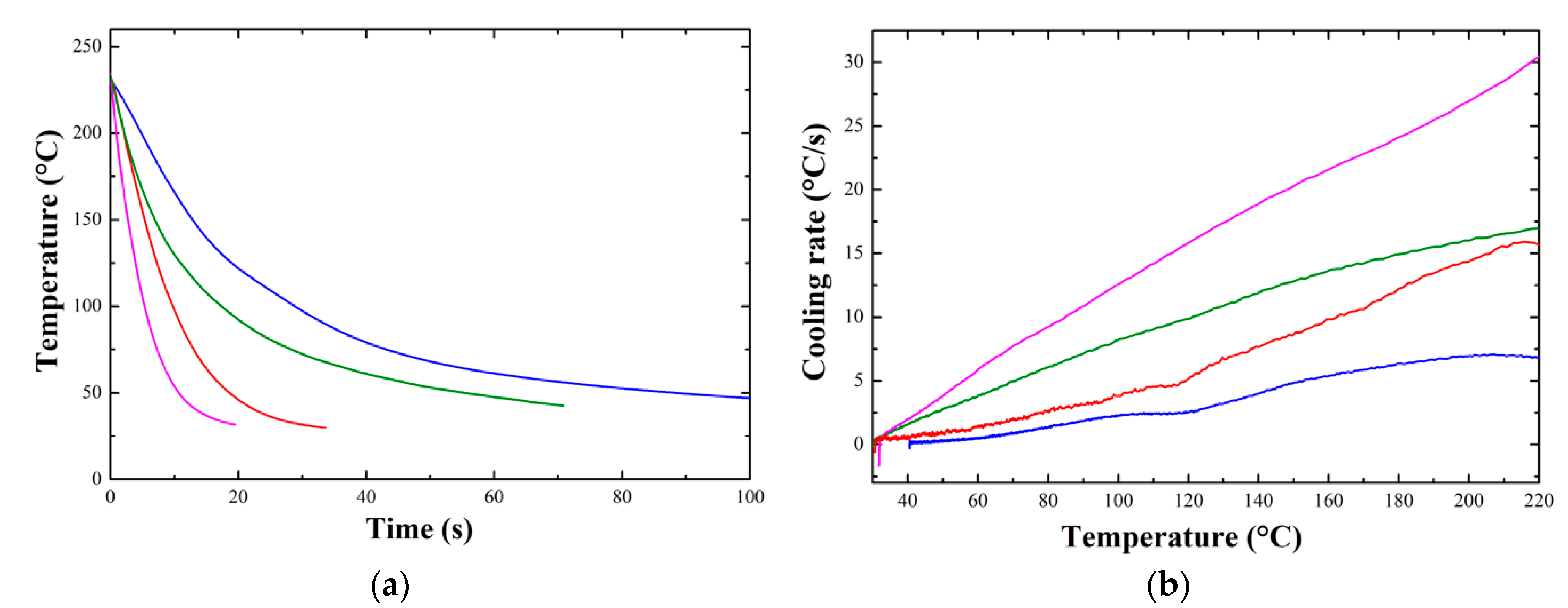
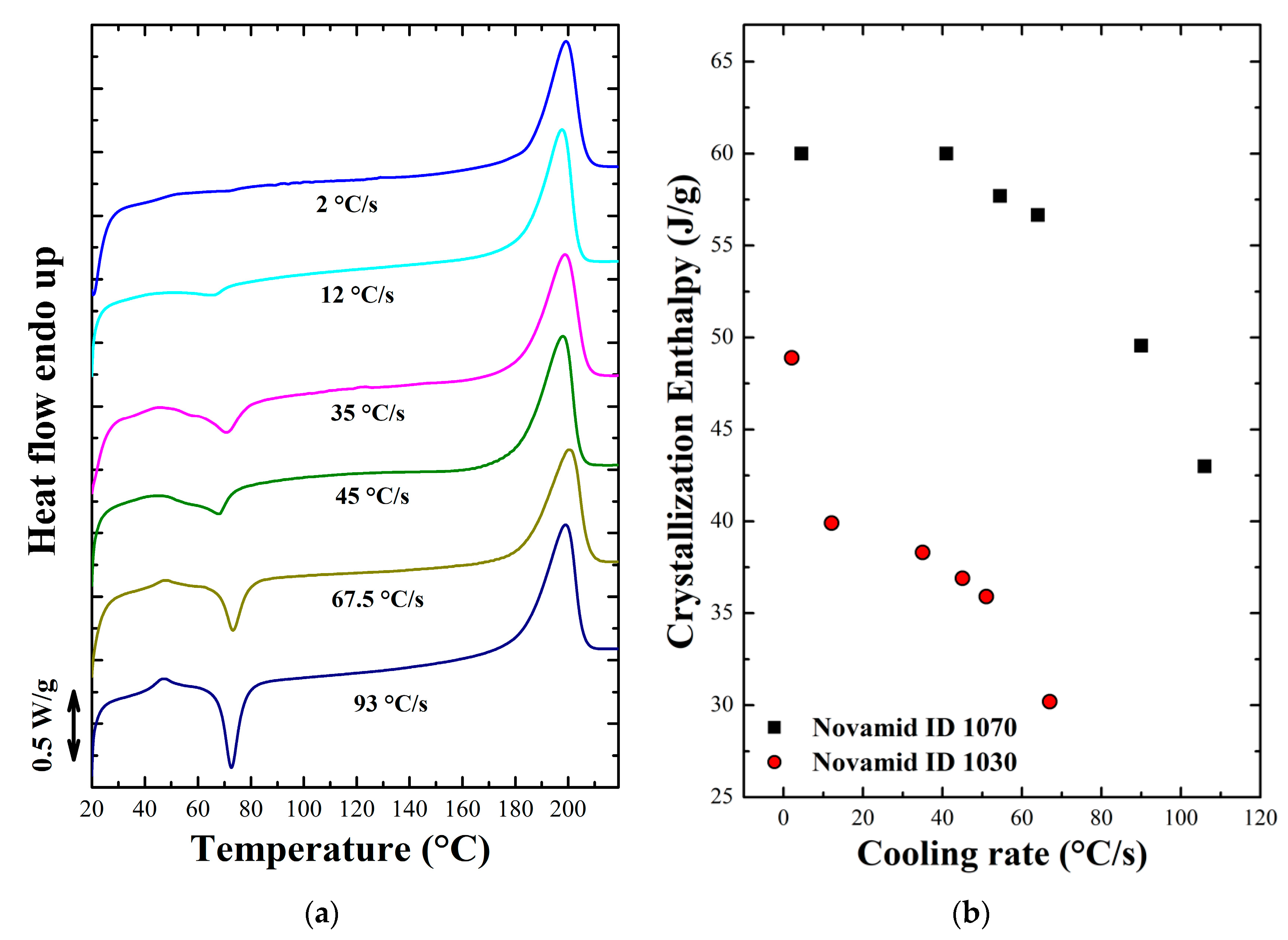
3.1.2. Non Isothermal Characterization
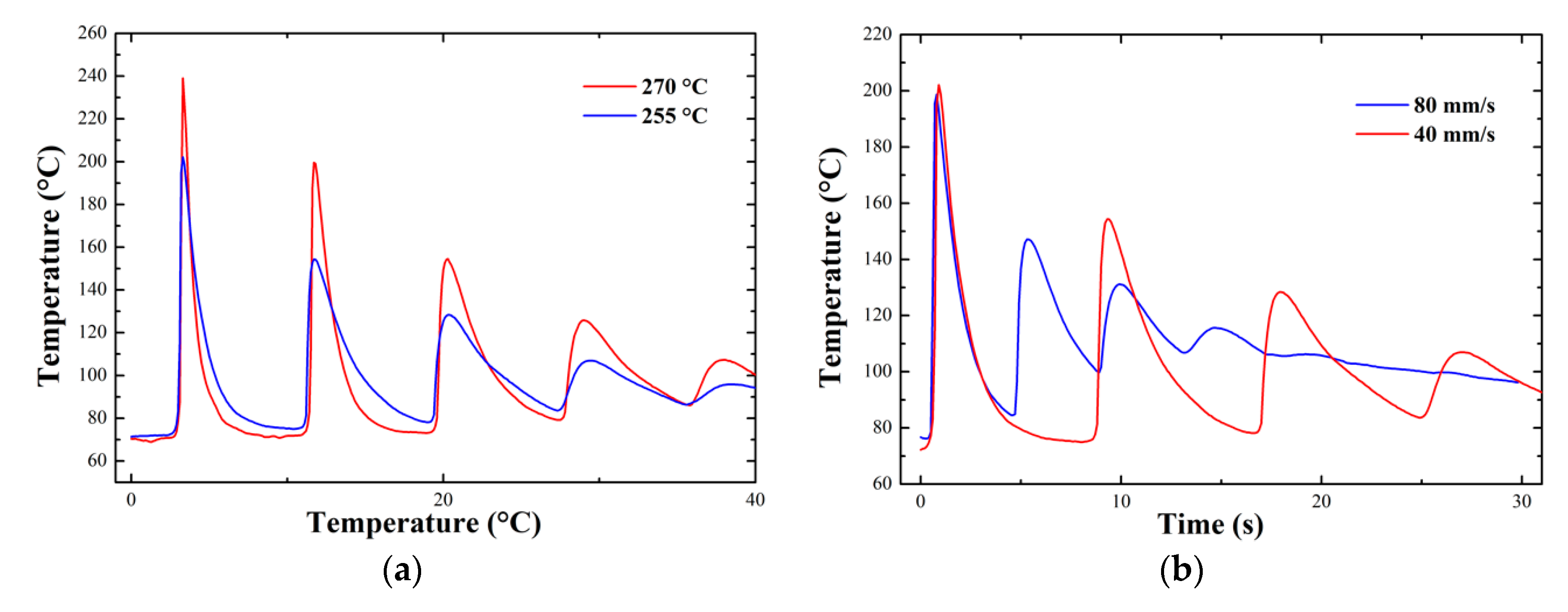
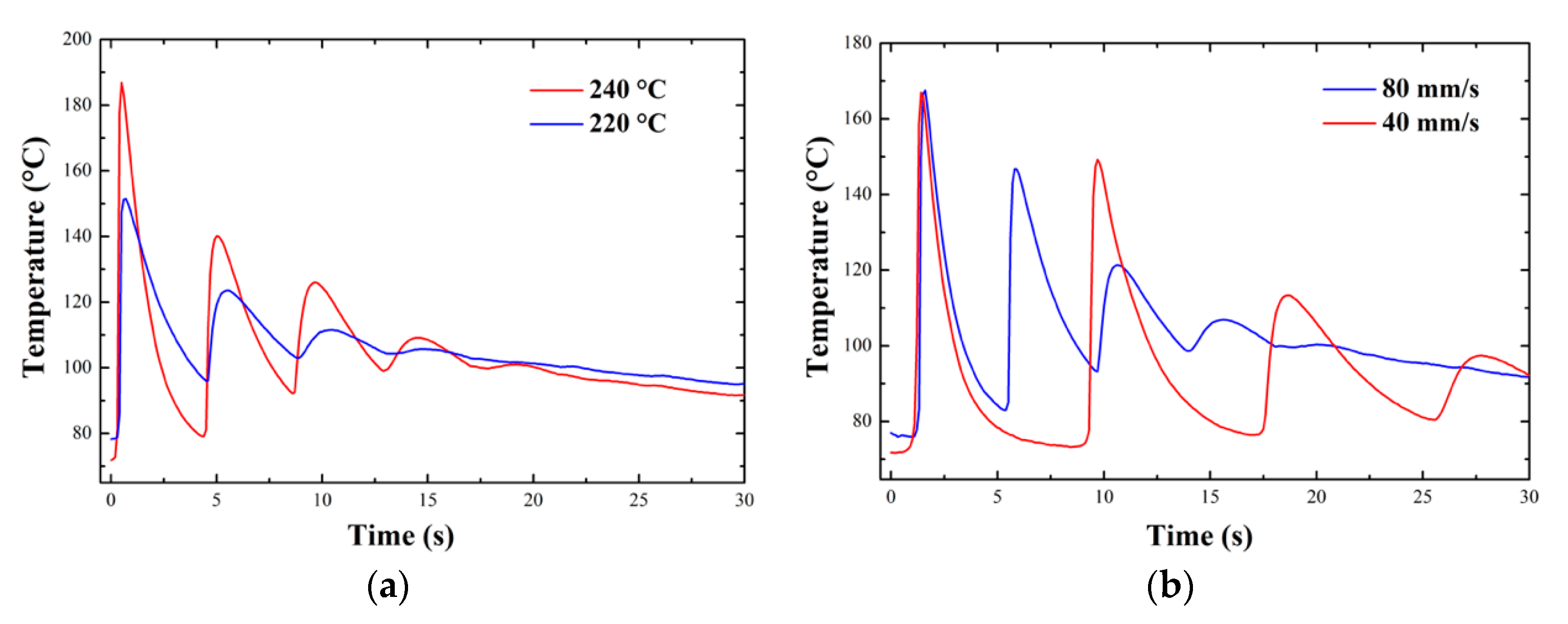
3.1.3. In-Situ Temperature Measurements
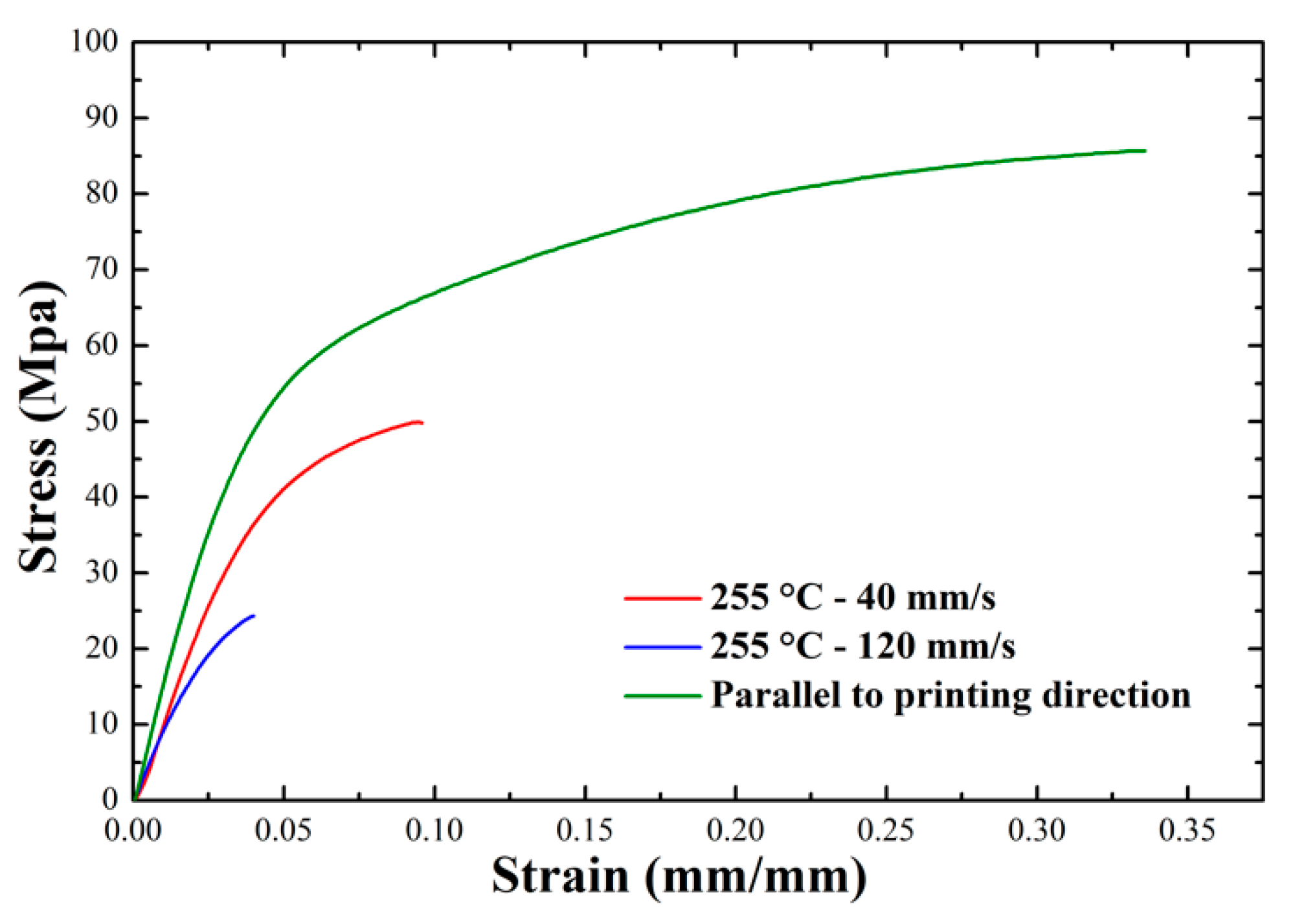
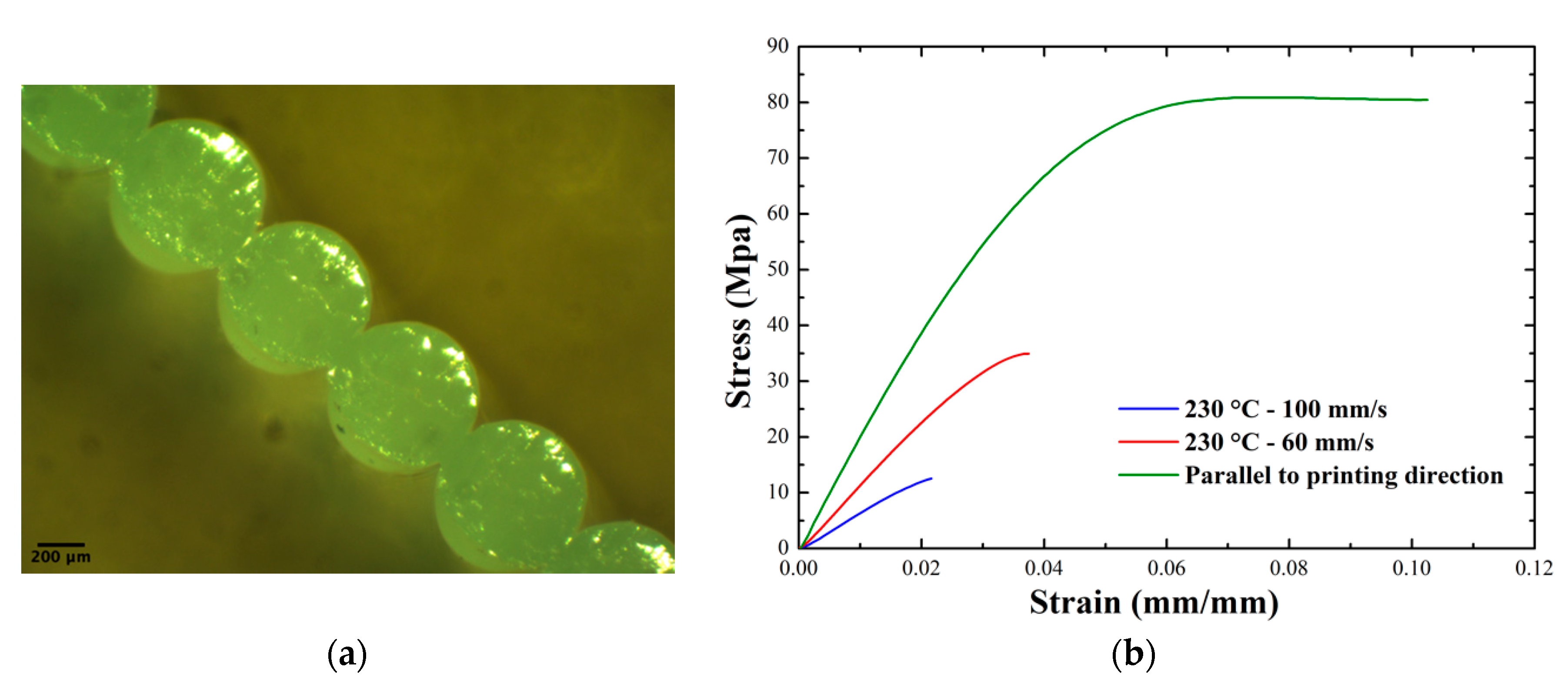
3.1.4. Weld Strength Measurements
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
Appendix A. Isothermal Analysis
Appendix B. Non Isothermal Analysis

Appendix C. DSC Quenched Samples

Appendix D. In-Situ Temperature Measurements
Appendix E. Betha Novamid ID 1070

Appendix F. Weld Strength Tests
References
- Gold, A.; Strong, S.R.; Turner, B.N. A review of melt extrusion additive manufacturing, processes: I. Process design and modelling. Rap. Prot. J. 2014, 20, 192. [Google Scholar]
- Ligon, S.C.; Liska, R.; Stampfl, J.; Gurr, M.; Mülhaupt, R. Polymers for 3D Printing and Customized Additive Manufacturing. Chem. Rev. 2017, 117, 10212–10290. [Google Scholar] [CrossRef] [PubMed]
- Wendel, B.; Rietzel, D.; Kühnlein, F.; Feulner, R.; Hülder, G.; Schmachtenberg, E. Additive Processing of Polymers. Macromol. Mater. Eng. 2008, 293, 799. [Google Scholar] [CrossRef]
- Kreiger, M.; Pearce, J.M. Environmental Life Cycle Analysis of Distributed Three-Dimensional Printing and Conventional Manufacturing of Polymer Products. ACS Sustain. Chem. Eng. 2013, 1, 1511. [Google Scholar] [CrossRef]
- Bikas, H.; Stavropoulos, P.; Chryssolouris, G. Additive manufacturing methods and modelling approaches: A critical review. Int. J. Adv. Manuf. Technol. 2016, 83, 389. [Google Scholar] [CrossRef]
- Ahn, S.; Montero, M.; Odell, D.; Roundy, S.; Wright, P.K. Anisotropic material properties of fused deposition modeling ABS. Rapid Prototyp. J. 2002, 8, 248. [Google Scholar] [CrossRef]
- Srinivas, V.; van Hooy-Corstjens, C.S.; Rastogi, S.; Harings, J.A.W. Promotion of molecular diffusion and/or crystallization in fused deposition modeled poly(lactide) welds. Polymer 2020, 202, 122637. [Google Scholar] [CrossRef]
- Seppala, J.E.; Migler, K.B. Infrared thermography of welding zones produced by polymer extrusion additive manufacturing. Addit. Manuf. 2016, 12, 71. [Google Scholar] [CrossRef]
- Bellehumeur, C.; Li, L.; Sun, Q.; Gu, P. Modeling of Bond Formation Between Polymer Filaments in the Fused Deposition Modeling Process. J. Manuf. Process. 2004, 6, 170. [Google Scholar] [CrossRef]
- Croccolo, D.; De Agostinis, M.; Olmi, G. Experimental characterization and analytical modelling of the mechanical behaviour of fused deposition processed parts made of ABS-M30. Comput. Mater. Sci. 2013, 79, 506. [Google Scholar] [CrossRef]
- Kausch, H.-H. Polymers/Properties and Applications; Springer: Berlin/Heidelberg, Germany, 1987; Volume 2, p. 456. [Google Scholar]
- Seppala, J.E.; Han, S.H.; Hillgartner, K.E.; Davis, C.S.; Migler, K.B. Weld formation during material extrusion additive manufacturing. Soft Matter 2017, 13, 6761. [Google Scholar] [CrossRef] [PubMed]
- Zhou, Y.-G.; Zou, J.-R.; Wu, H.-H.; Xu, B.-P. Balance between bonding and deposition during fused deposition modeling of polycarbonate and acrylonitrile-butadiene-styrene composites. Polym. Compos. 2020, 41, 60–72. [Google Scholar] [CrossRef]
- Chacón, J.M.; Caminero, M.A.; García-Plaza, E.; Núñez, P.J. Additive manufacturing of PLA structures using fused deposition modelling: Effect of process parameters on mechanical properties and their optimal selection. Mater. Des. 2017, 124, 143. [Google Scholar] [CrossRef]
- Mark, J.E. (Ed.) Physical Properties of Polymers Handbook; Springer: Berlin/Heidelberg, Germany, 2007. [Google Scholar]
- Yang, C.; Tian, X.; Li, D.; Cao, Y.; Zhao, F.; Shi, C. Influence of thermal processing conditions in 3D printing on the crystallinity and mechanical properties of PEEK material. J. Mater. Process. Technol. 2017, 248, 1. [Google Scholar] [CrossRef]
- Geng, P.; Zhao, J.; Wu, W.; Wang, Y.; Wang, B.; Wang, S.; Li, G. Effect of Thermal Processing and Heat Treatment Condition on 3D Printing PPS Properties. Polymer 2018, 10, 875. [Google Scholar] [CrossRef]
- Vaes, D.; Coppens, M.; Goderis, B.; Zoetelief, W.; Van Puyvelde, P. Assessment of Crystallinity Development during Fused Filament Fabrication through Fast Scanning Chip Calorimetry. Appl. Sci. 2019, 9, 2676. [Google Scholar] [CrossRef]
- Northcutt, L.A.; Orski, S.V.; Migler, K.B.; Kotula, A.P. Effect of processing conditions on crystallization kinetics during materials extrusion additive manufacturing. Polymer 2018, 154, 182. [Google Scholar] [CrossRef]
- Srinivas, V.; Van Hooy-Corstjens, C.S.; Harings, J.A. Correlating molecular and crystallization dynamics to macroscopic fusion and thermodynamic stability in fused deposition modeling; a model study on polylactides. Polymer 2018, 142, 348. [Google Scholar] [CrossRef]
- DSM develops new 3D printing materials. Reinf. Plast. 2019, 63, 15. [CrossRef]
- Cavallo, D.; Portale, G.; Balzano, L.; Azzurri, F.; Bras, W.; Peters, G.W.; Alfonso, G.C. Real-Time WAXD Detection of Mesophase Development during Quenching of Propene/Ethylene Copolymers. Macromolecules 2010, 43, 10208–10212. [Google Scholar] [CrossRef]
- Cavallo, D.; Gardella, L.; Alfonso, G.C.; Portale, G.; Balzano, L.; Androsch, R. Effect of cooling rate on the crystal/mesophase polymorphism of polyamide 6. Colloid Polym. Sci. 2011, 289, 1073. [Google Scholar] [CrossRef]
- Jin, M.; Giesa, R.; Neuber, C.; Schmidt, H. Filament Materials Screening for FDM 3D Printing by Means of Injection-Molded Short Rods. Macromol. Mater. Eng. 2018, 303, 1800507. [Google Scholar] [CrossRef]
- Cheng, G.Z.; Estepar, R.S.J.; Folch, E.; Onieva, J.; Gangadharan, S.; Majid, A. Three-dimensional Printing and 3D Slicer: Powerful Tools in Understanding and Treating Structural Lung Disease. Chest 2016, 149, 1136. [Google Scholar] [CrossRef] [PubMed]
- Massey, L.K. Plastics Design Library, Film Properties of Plastics and Elastomers, 2nd ed.; William Andrew Publishing: Norwich, NY, USA, 2004; Volume 45, p. 173. [Google Scholar]
- Hubbes, S.-S.; Danzl, W.; Foerst, P. Crystallization kinetics of palm oil of different geographic origins and blends thereof by the application of the Avrami model. LWT 2018, 93, 189. [Google Scholar] [CrossRef]
- Lorenzo, A.T.; Arnal, M.L.; Albuerne, J.; Müller, A.J. DSC isothermal polymer crystallization kinetics measurements and the use of the Avrami equation to fit the data: Guidelines to avoid common problems. Polym. Test. 2007, 26, 222. [Google Scholar] [CrossRef]
- Rhoades, A.M.; Williams, J.L.; Androsch, R. Crystallization kinetics of polyamide 66 at processing-relevant cooling conditions and high supercooling. Thermochim. Acta 2015, 603, 103. [Google Scholar] [CrossRef]
- Sun, Q.; Rizvi, G.M.; Bellehumeur, C.T.; Gu, P. Effect of processing conditions on the bonding quality of FDM polymer filaments. Rapid Prototyp. J. 2008, 14, 72. [Google Scholar] [CrossRef]
- McIlroy, C.; Seppala, J.E.; Kotula, A.P. Combining Modeling and Measurements To Predict Crystal Morphology in Material Extrusion. ACS Symp. Ser. 2019, 85–113. [Google Scholar] [CrossRef]
- Zhang, X.; Wang, J. Controllable interfacial adhesion behaviors of polymer-on-polymer surfaces during fused deposition modeling 3D printing process. Chem. Phys. Lett. 2020, 739, 136959. [Google Scholar] [CrossRef]
- Cruz, P.; Shoemake, E.D.; Adam, P.; Leachman, J. Tensile strengths of polyamide based 3D printed polymers in liquid nitrogen. IOP Conf. Series: Mater. Sci. Eng. 2015, 102, 012020. [Google Scholar] [CrossRef]
- Ghodbane, S.A.; Murthy, N.S.; Dunn, M.G.; Kohn, J. Achieving molecular orientation in thermally extruded 3D printed objects. Biofabrication 2019, 11, 045004. [Google Scholar] [CrossRef] [PubMed]
- Costanzo, A.; Spotorno, R.; Candal, M.V.; Fernández, M.M.; Müller, A.J.; Graham, R.S.; Cavallo, D.; McIlroy, C. Residual alignment and its effect on weld strength in material-extrusion 3D-printing of polylactic acid. Addit. Manuf. 2020, 36, 101415. [Google Scholar] [CrossRef]
- Mileva, D.; Kolesov, I.; Androsch, R. Morphology of cold-crystallized polyamide 6. Colloid Polym. Sci. 2012, 290, 971. [Google Scholar] [CrossRef]
- Levenhagen, N.P.; Dadmun, M. Bimodal molecular weight samples improve the isotropy of 3D printed polymeric samples. Polymer 2017, 122, 232. [Google Scholar] [CrossRef]


| Parameter | Novamid ID 1030 | Novamid ID 1070 |
|---|---|---|
| Nozzle temperature | 220–240 °C | 255–270 °C |
| Print speed | 20–120 mm/S | 20–120 mm/s |
| Buildplate temperature | 80 °C | 80 °C |
| Chamber temperature | 50 °C | 50 °C |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Costanzo, A.; Croce, U.; Spotorno, R.; Fenni, S.E.; Cavallo, D. Fused Deposition Modeling of Polyamides: Crystallization and Weld Formation. Polymers 2020, 12, 2980. https://doi.org/10.3390/polym12122980
Costanzo A, Croce U, Spotorno R, Fenni SE, Cavallo D. Fused Deposition Modeling of Polyamides: Crystallization and Weld Formation. Polymers. 2020; 12(12):2980. https://doi.org/10.3390/polym12122980
Chicago/Turabian StyleCostanzo, Andrea, Umberto Croce, Roberto Spotorno, Seif Eddine Fenni, and Dario Cavallo. 2020. "Fused Deposition Modeling of Polyamides: Crystallization and Weld Formation" Polymers 12, no. 12: 2980. https://doi.org/10.3390/polym12122980
APA StyleCostanzo, A., Croce, U., Spotorno, R., Fenni, S. E., & Cavallo, D. (2020). Fused Deposition Modeling of Polyamides: Crystallization and Weld Formation. Polymers, 12(12), 2980. https://doi.org/10.3390/polym12122980








