Effect of the Shape of Styrene–Acrylonitrile Water-Filter Housings on the Destructive Pressure, Crack-Initiation, Propagation Conditions and Fracture Toughness of Styrene–Acrylonitrile
Abstract
:1. Introduction
2. Experimental
2.1. Material
2.2. Testing
2.3. Types and Geometrical Characteristics of the Water-Filter Housings
2.4. Samples for the Destructive Pressure Test
- -
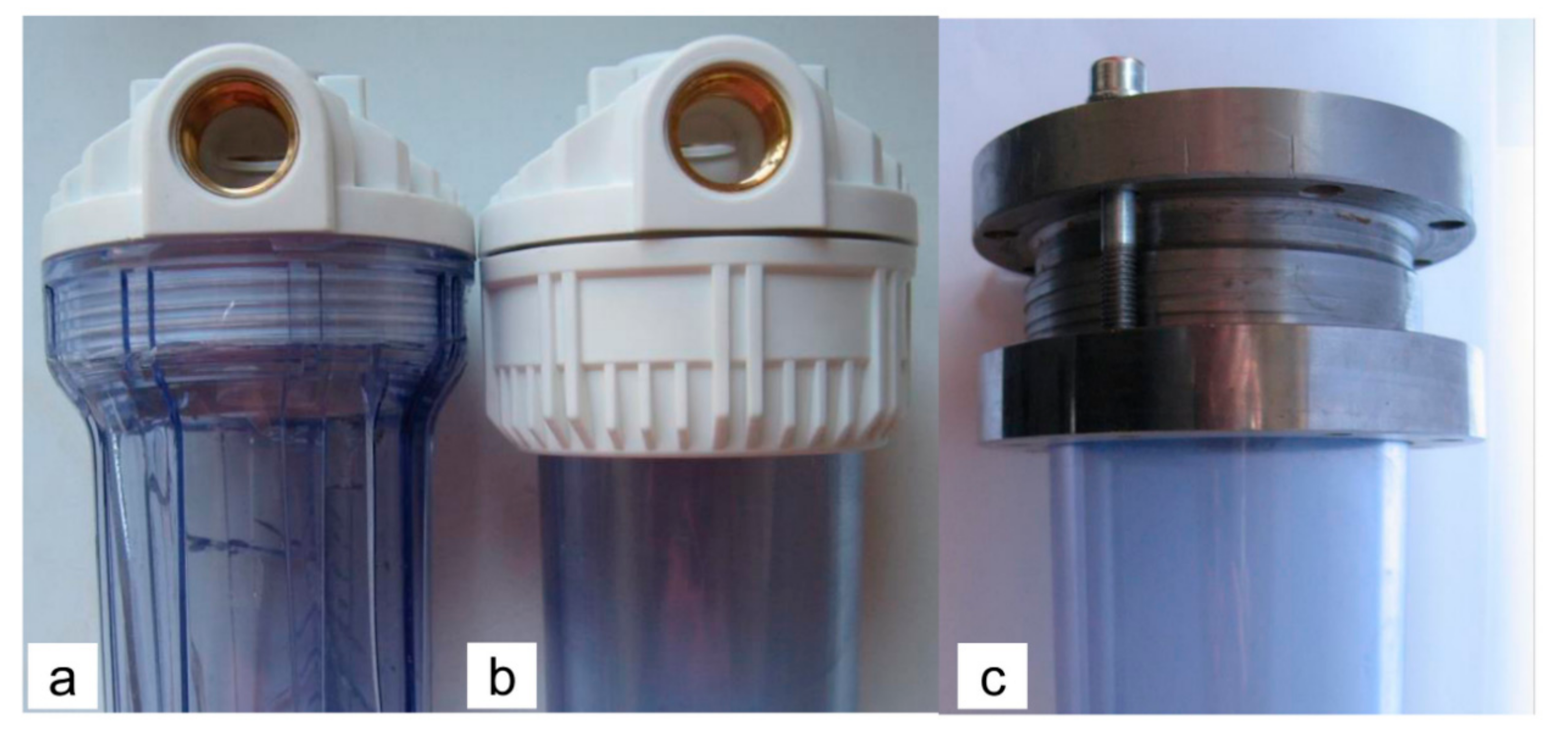
- A: the housing with the external ribs is directly threaded to the head of PP (Figure 2a),
- -
- B: the housing without the ribs is fixed to the PP head with a PP nut (Figure 2b),
- -
- C: the housing without the ribs is fixed to the steel head with a steel ring (Figure 2c),
- -
- D: the housing without the ribs but a thicker base is fixed to the steel head with a steel ring (Figure 2c).
3. Results and Discussion
3.1. Destructive Pressure Test
- -
- the housing broke at the pressure of pd = 34 bar,
- -
- B: the PP nut broke at the pressure pd = 48 bar, the housing remained undamaged,
- -
- C: the housing broke at the pressure of pd = 50 bar,
- -
- D: the housing broke at the pressure of pd = 71 bar.
3.2. Analysis of the Fractures
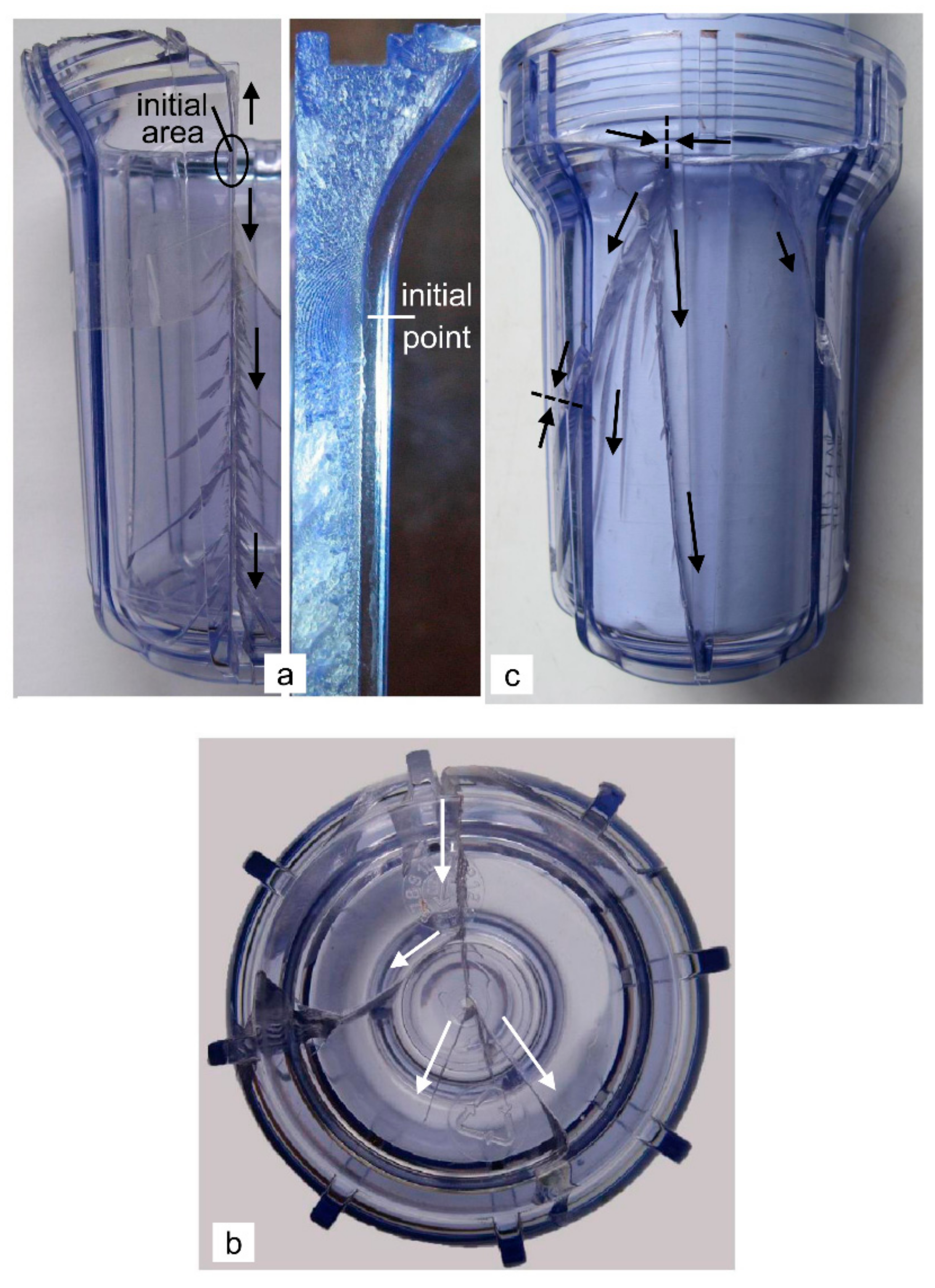
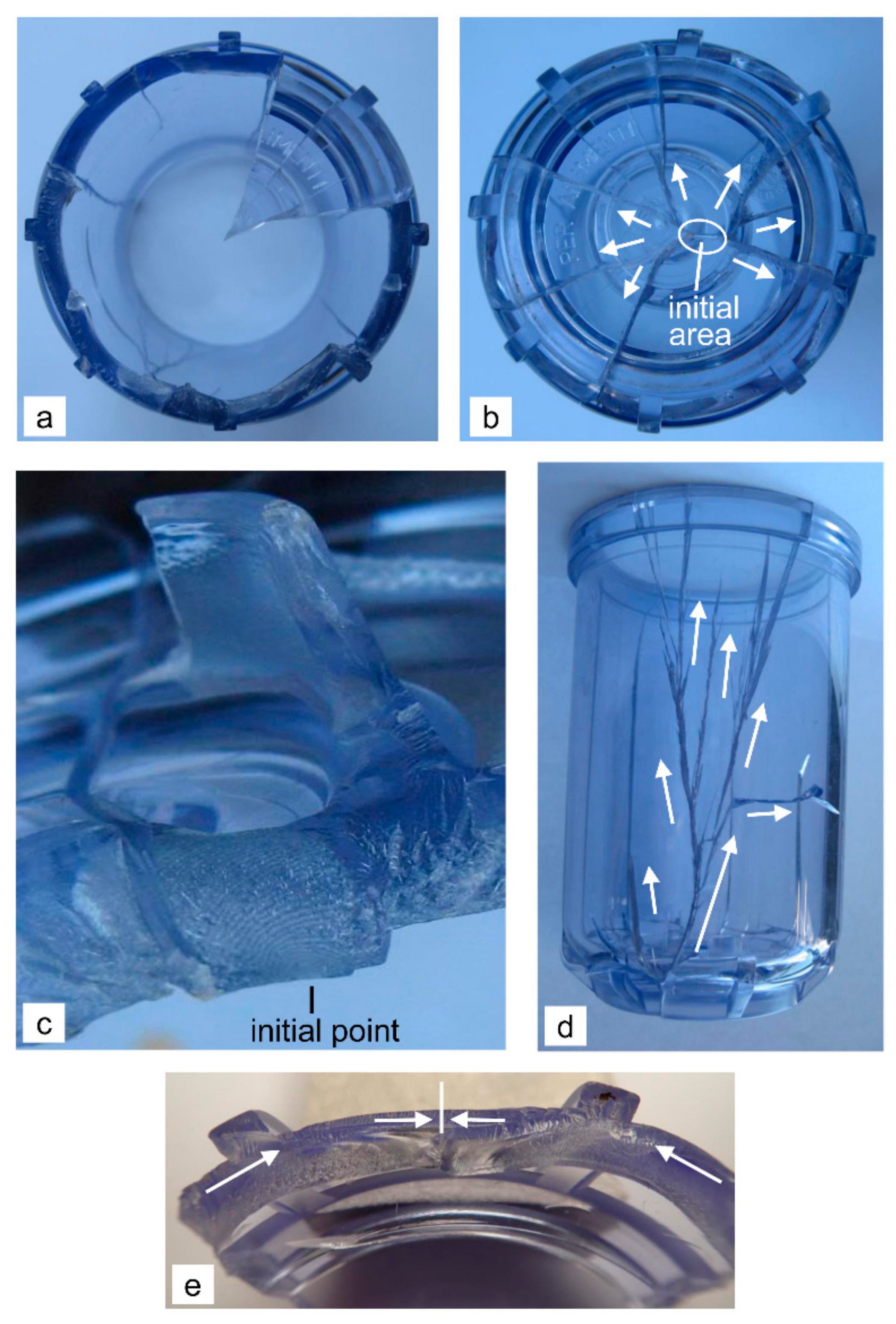
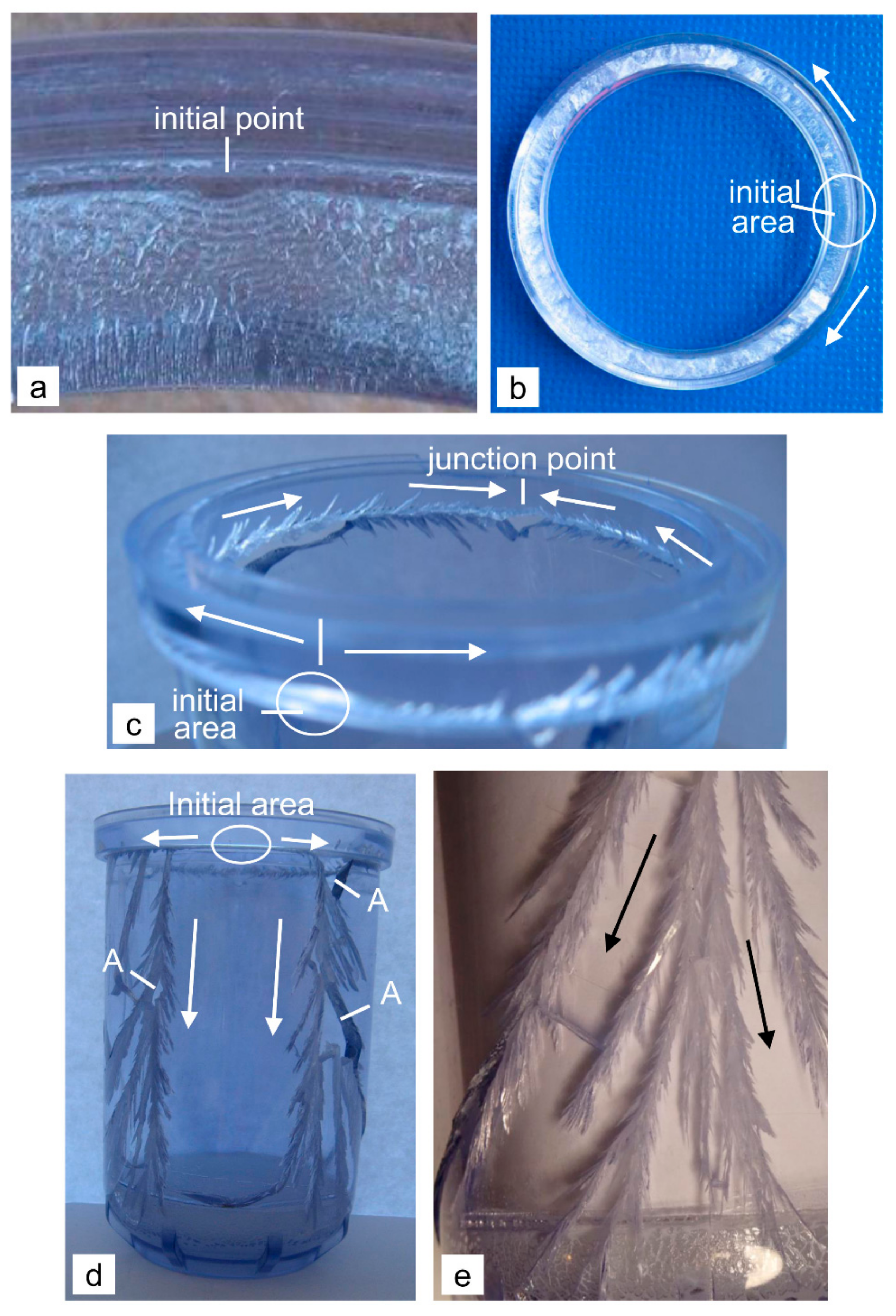
3.2.1. Visual Analysis of the Cracks
(a) Sample of Series A
(b) Sample of Series B
(c) Samples of Series C
(d) Sample of Series D
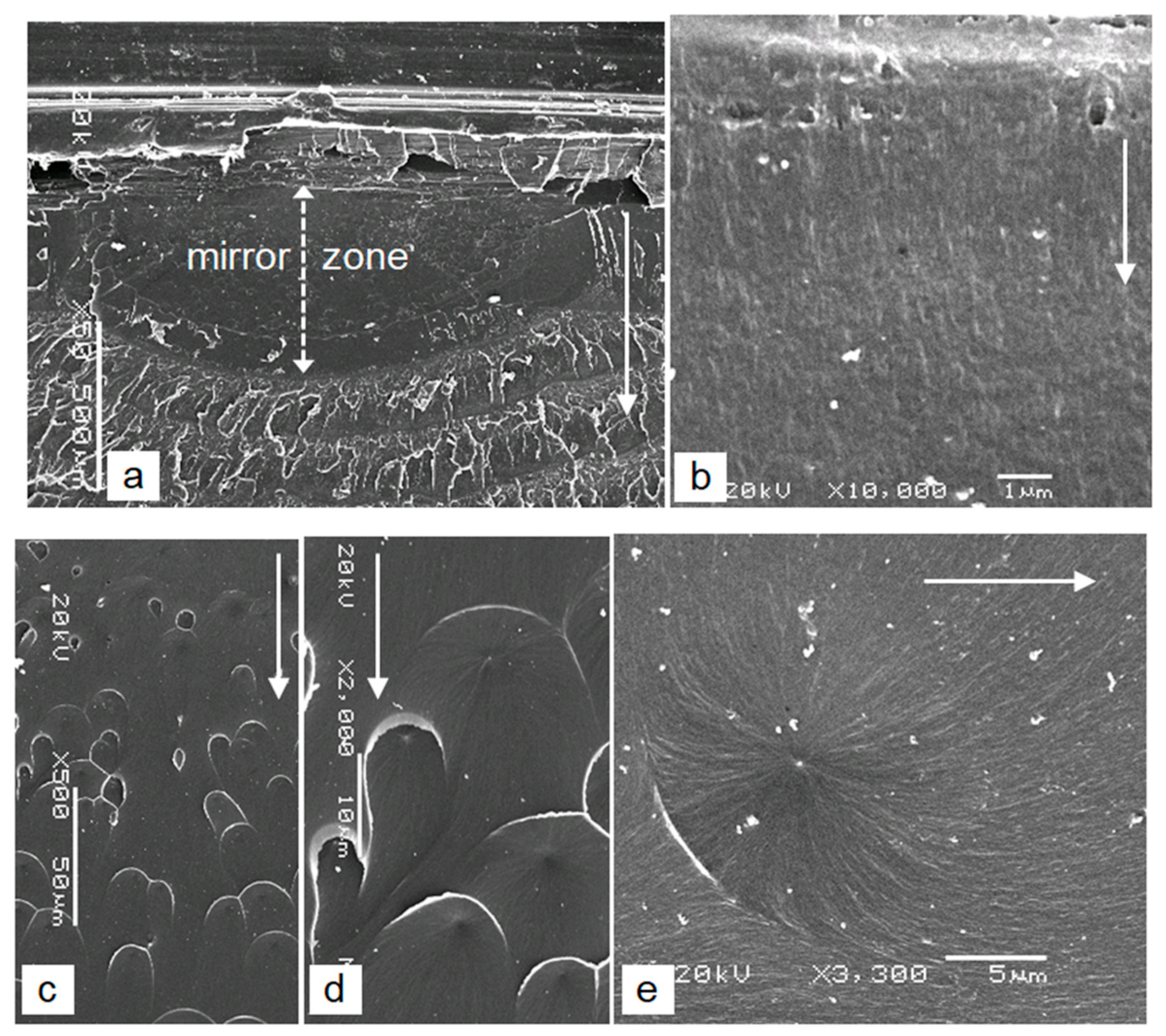
3.2.2. Electron Microscopy of Fracture Surfaces
3.3. Assessment of the Conditions for Crack Initiation
3.3.1. (a) Sample of Series A
3.3.2. (b) Sample of Series C
3.3.3. (c) Sample of Series D
3.4. Estimation of the Crack-Growth Conditions
3.5. Summary of the Results
4. Conclusions
- -
- The tensile strength of the SAN of the water-filter housings is Rm = 73 N/mm2, which is in the range of the literature data. In spite of the same tensile strength, the shape of the housings with its details strongly affects their critical area and the value of the destructive pressure. The least destructive pressure pd = 34 bar was for the housing with external vertical ribs. The crack initiated at a sharp transition between the rib and the wall with Kt ≈ 3.4, as a result of the tangential stress σt. The largest destructive pressure pd = 71 bar was for the housing without the ribs with the thickened base. Here, the crack initiated at a sharp transition between the clamping ring and the wall with Kt ≈ 2.75, as a result of the axial stress σa. Between these two examples is a housing without the ribs with a uniform thick wall and a destructive pressure of pd = 50 bar. Here, the crack initiated in the middle of the thickened base without a stress concentrator as a result of the stress σr = σt.
- -
- The different shape of the housings with associated various critical sites and the various destructive pressures strongly affects the initiation areas, the direction, the kinetics, the mode of growth and the splitting of the cracks during their propagation. They represent unique and repeatable parameters for each type of housing individually.
- -
- The critical depth of the cracks, the fracture toughness of the SAN, and the critical thickness of the wall for the formation of a brittle fracture are very dependent on the stress-increasing rate or the velocity of the crack propagation. The lower the destructive pressure is, the faster is the stress-increasing rate and the smaller is the critical length of the crack amz required for the formation of uncontrolled crack growth. Furthermore, the crack growth is also faster. Consequently, at a lower destructive pressure, the fracture toughness of the SAN is lower as well as the critical thickness of the wall for the formation of a brittle fracture.
- -
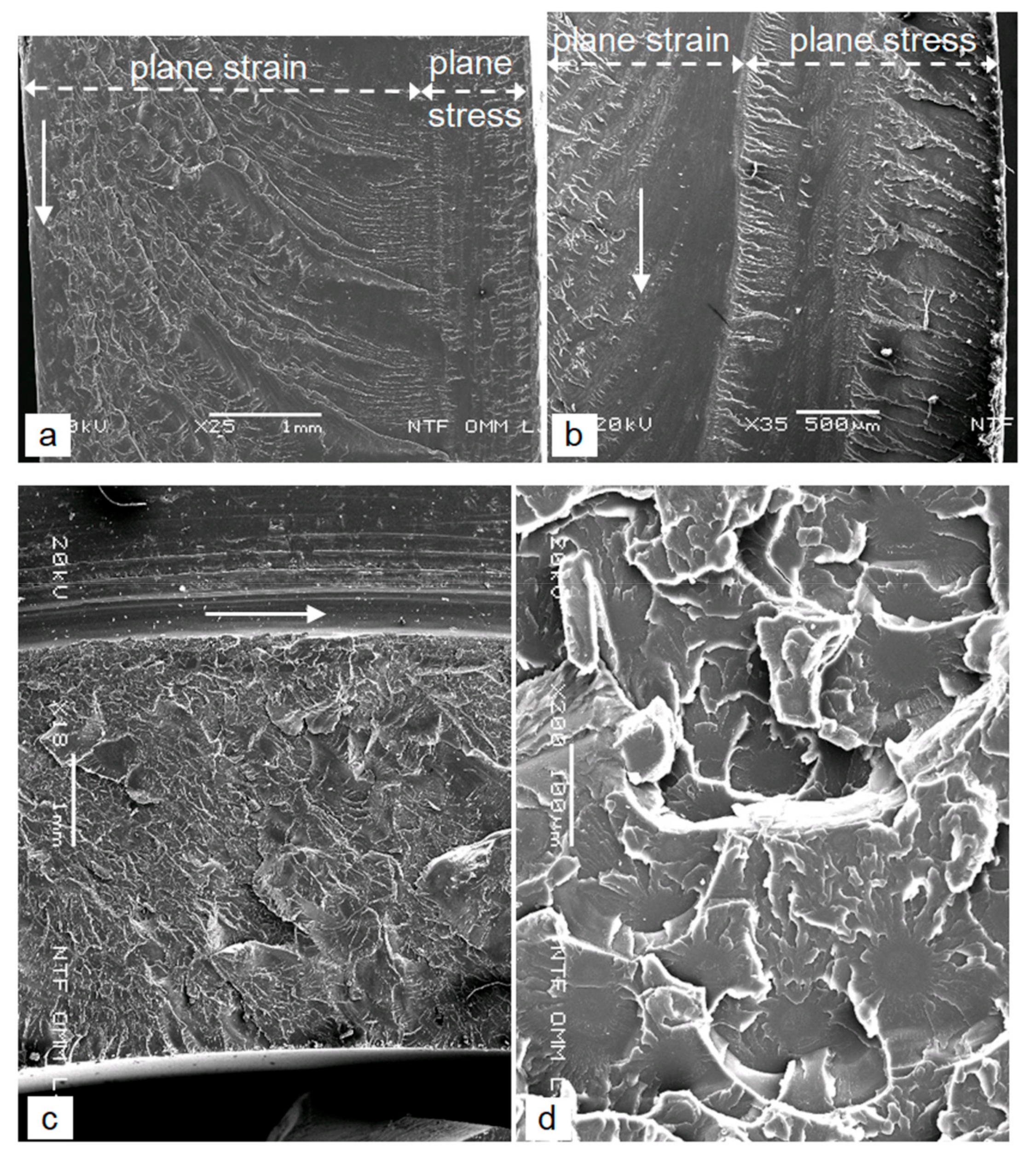
- The SAN from which the water-filter housings were made has the following fracture-mechanical properties at destructive pressures pd = 34, 50 and 71 bar, respectively: fracture toughness KIC ≈ 1.45, 1.77 and 3.55 MPa∙m1/2; depth of the mirror zone amz ≈ 100, 150 and 600 μm; the critical thickness for the formation of a brittle fracture t ≈ 1.0, 1.5 and 6.0 mm or t = 10amz. Therefore, the crack in the wall of the samples of series A and C grows predominantly in the plane-strain state, while the crack in the sample of series D grows in the plane-stress state.
- -
- The crack-growth velocity affects the surface texture of the mirror zone. In all cases, regardless of the growth velocity of the sub-critical cracks, the mirror zone is microscopically very finely rough as a result of the formation and tearing of the crazes. On the surface of the mirror zone of the slowest-growing sub-critical crack, different shape of contours also exist, and the most typical of this is a large number of parabolic markings. These are oriented with an open side in the growing direction of the main crack. All the observed contours are the result of the initiation of secondary micro-cracks at micro-unhomogeneities sites in the SAN, which intersect their growth fronts with the main crack. This is only the case for a sufficiently small growth rate of the main crack, when the micro-unhomogeneities can act as a local stress micro-concentrator ahead of the growing front of the main crack. If the crack-growth velocity of the main crack is greater than the critical cracking rate for this phenomenon, the surface of the mirror zone is finely rough without the mentioned contours.
- -
- The texture of all the analyzed over-critical surface cracks in the vicinity of the mirror zone is same due to the initial small growth rate, regardless of the destructive pressure. Macroscopic and microscopic semi-elliptical Wallner lines and from the initial area divergent-oriented hackle lines are visible. The Wallner lines generally show the front of the growth crack, and the hackle lines are directed towards the direction of the main crack growth.
- -
- The crack-growth velocity affects the surface texture of the over-critical unstable cracks. In the subsequent rapid-growing over-critical cracks in the plane-strain state, the Wallner lines and hackle lines are strongly curved, indicating a different cracking velocity through the wall thickness. The Wallner lines and hackle lines together form a group of intersecting curves that are mathematically described as a system of a single-parametric family of curves with a family of orthogonal trajectories. On the surface of the slowly growing over-critical crack in the plane-stress state at some distance from the initiation area a strong plastic shear tearing of the material with a lot of secondary micro-cracks, initiated at micro-unhomogeneities in the styrene–acrylonitrile are visible. Macroscopically, such cracks appear in the form of a pine twig or feathers, and microscopically on the fracture surface they have a lot of rosette-like forms. On a fracture surface, far enough away from the initial area, only the hackle lines exist. This is a consequence of a very rough and split crack-growing front that causes a strong, diffuse reflection of the returned shock waves, and which prevents the formation of the Wallner lines. The proof of this is the beginning slow-growing phase of the over-critical crack near the initial area. There the Wallner lines are clearly visible, despite the low crack-propagation velocity due to the smooth crack surface, which is a consequence of the non-split crack-growing front.
Author Contributions
Funding
Conflicts of Interest
References
- Zorc, B.; Nagode, A. Analyses of a Leaking Styrene–Acrylonitrile Water-Filter Housing. Eng. Fail. Anal. 2015, 57, 156–163. [Google Scholar] [CrossRef]
- Zorc, B.; Nagode, A. Determination of the Crack-Initiation and Propagation Conditions in a Styrene–Acrylonitrile Water-Filter Housing with External Ribs Based on Destructive Pressure Tests. Eng. Fail. Anal. 2017, 79, 491–503. [Google Scholar] [CrossRef]
- Shigley, J.E. Mechanical Engineering Design; McGraw-Hill Book Company: New York, NY, USA, 1986. [Google Scholar]
- Collins, J.A. Failure of Materials in Mechanical Design, 2nd ed.; John Wiley & Sons: New York, NY, USA, 1993. [Google Scholar]
- Pilkey, W.D.; Pilkey, D.F. Peterson’s Stress Concentration Factors, 3rd ed.; John Wiley & Sons: Hoboken, NJ, USA, 2008. [Google Scholar]
- Schijve, J. Fatigue of Structures and Materials, 2nd ed.; Springer Science + Business Media: New York, NY, USA, 2009. [Google Scholar]
- Liu, A.F. Mechanics and Mechanisms of Fracture: An Introdution; ASM International®, Materials Park: Cleveland, OH, USA, 2005. [Google Scholar]
- Hlebanja, J. Zvarjeni spoji, tlačne posode in prirobnice (Welded Joints, Pressure Vesseles and Flanges); Univerza v Ljubljani-Fakulteta za strojništvo: Ljubljana, Slovenia, 1974. [Google Scholar]
- Ruge, J. Handbuch der Schweiβtechnik, Band IV-Berechnung der Verbindungen; Springer: Berlin, Germany, 1988. [Google Scholar]
- Janssen, M.; Zuidema, J.; Wanhill, R.J.H. Fracture Mechanics, 2nd ed.; VSSD: Delft, The Netherlands, 2002. [Google Scholar]
- Lampman, S. (Ed.) Characterization and Failure Analysis of Plastics: Fracture and Fractography; ASM International®, Materials Park: Cleveland, OH, USA, 2003; pp. 404–416. [Google Scholar]
- McCrum, N.G.; Buckley, C.P.; Bucknall, C.B. Principles of Polymer Engineering, 2nd ed.; Oxford University Press: Oxford, UK, 2011. [Google Scholar]
- Alger, M.S.M. Polymer Science Dictionary, 2nd ed.; Chapman & Hall: London, UK, 1997; p. 106. [Google Scholar]
- Young, R.J.; Lowell, P.A. Introduction to Polymers, 3rd ed.; CRC Press Taylor & Francis Group: London, UK, 2011. [Google Scholar]
- Gusler, G.M.; McKenna, G.B. The Effect of Physicalaging on the Craze Response of a Polystyrene and a Styrene–Acrylonitrile Copolymere. In SPA/ANTEC 1996 Proceedings (Print Version/3 Volumes); CRC Press Taylor & Francis Grouo: London, UK, 1996; pp. 1537–1542. [Google Scholar]
- Brizić, M.; Janović, Z.; Šmit, I.; Štefanović, D.; Požar, H. (Eds.) Tehnička Enciklopedija (Technical Encyclopedia), 10: Polimerni Materijali (Polymer Materials); Jugoslavenski leksikografski zavod “Miroslav Krleža”: Zagreb, Croatia, 1986; pp. 581–622. [Google Scholar]
- Navodnik, J. Plastik Orodjar-Priročnik (Manufacturer of Plastic Products and Toolmaker-Handbook), 3rd ed.; Navodnih-kemijski inženiring: Velenje, Slovenia, 1998. [Google Scholar]
- Lampman, S. (Ed.) Characterization and Failure Analysis of Plastics: Engineering Plastics—An Introduction; ASM International®, Materials Park: Cleveland, OH, USA, 2003; pp. 3–27. [Google Scholar]
- Budynas, R.G.; Nisbett, J.K. Shigley’s Mechanical Engineering Design, 10th ed.; McGraw-Hill Education: New York, NY, USA, 2015. [Google Scholar]
- Blodgett, O.W. Design of Weldments; The James F. Lincoln Arc Welding Foundation: Cleveland, OH, USA, 1963. [Google Scholar]
- Gooyer, L.E.; Overbeeke, J.L. The stress distributions in shouldered shafts under axisymmetric loading. J. Strain Anal. 1991, 26, 181–184. [Google Scholar] [CrossRef] [Green Version]
- Noda, N.A.; Takase, Y.; Monda, K. Stress Concentration Factors for Shoulder Fillets in Round and Flat Bars under Various Loads. Int. J. Fatigue 1997, 19, 75–84. [Google Scholar] [CrossRef]
- Kraut, B. Strojniški priročnik (Handbook of Mechanical Engineering); Strojniški vestnik: Ljubljana, Slovenia, 1981. [Google Scholar]
- Kim, H.; Keskkula, H.; Paul, D.R. The Role of Inherent Ductility in Rubber Toughening of Brittle Polymers. Polymer 1991, 32, 2372–2376. [Google Scholar] [CrossRef]
- Lampman, S. (Ed.) Characterization and Failure Analysis of Plastics: Mechanical Testing and Properties of Plastics—An Introduction; ASM International®, Materials Park: Cleveland, OH, USA, 2003; pp. 3–28. [Google Scholar]
- Landes, J.D.; Becker, W.T.; Shipley, R.S.; Raphael, J. Stress Analysis and Fracture Mechanics. In ASM Handbook®—Failure Analysis and Prevention; Becker, W.T., Shipley, R.J., Eds.; ASM International®, Materials Park: Cleveland, OH, USA, 2008; Volume 11, pp. 460–483. [Google Scholar]
- Grellmann, W.; Lach, R.; Seidler, S. Experimental Determination of Geometry-Independent Fracture Mechanics Values J, CTOD and K for Polymers. Int. J. Fract. 2002, 118, L9–L14. [Google Scholar] [CrossRef]
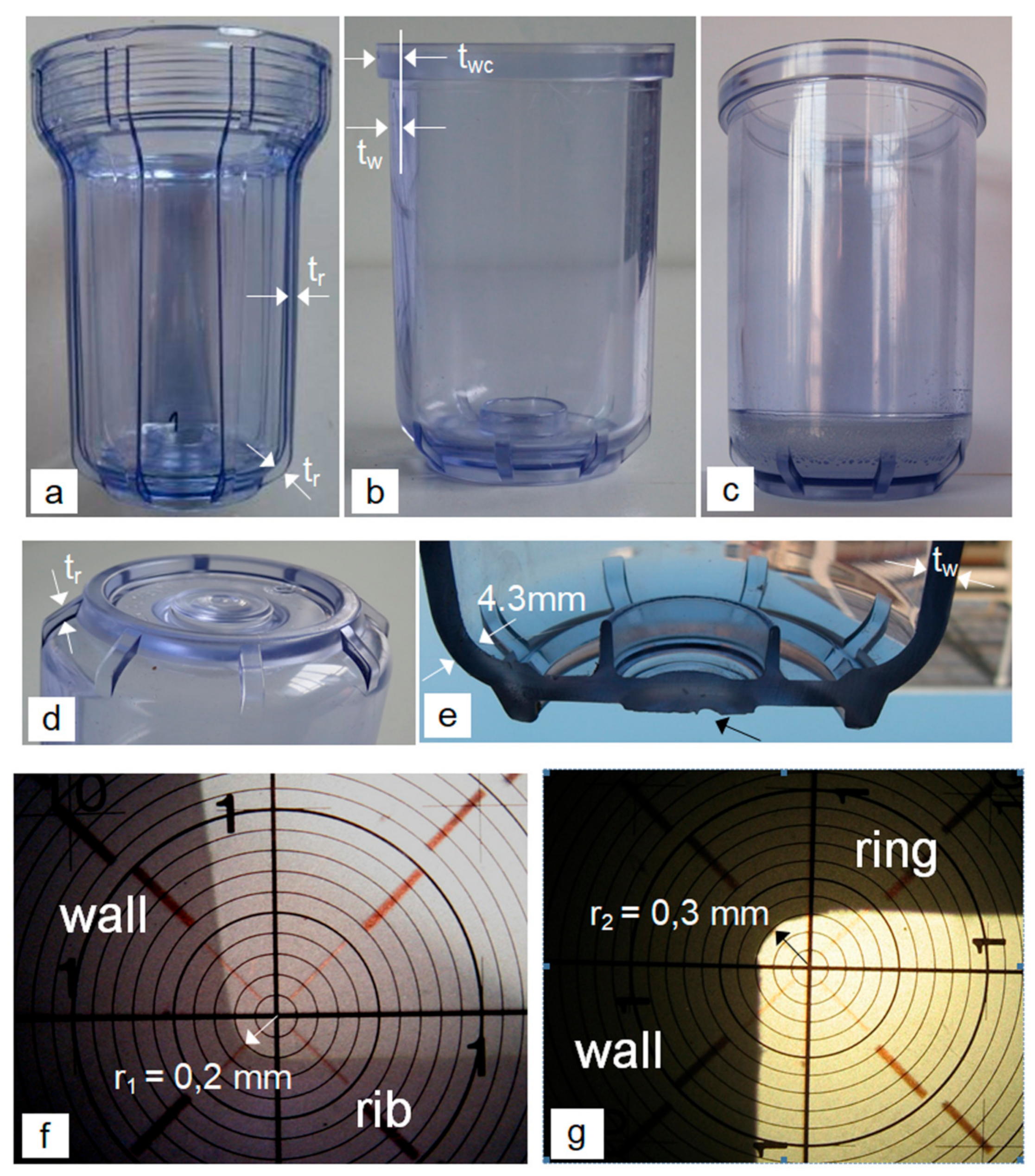


| Sample | tw (mm) | Kt | Initial Dominant Stress | pd (bar) | KIC (MPa⋅m1/2) | amz (mm) | t (mm) | Crack Growth State |
|---|---|---|---|---|---|---|---|---|
| Series A | 4.7 | 3.4 | σt | 34 | 1.45 | 0.10 | 1.0 | plane-strain |
| Series C | 4.3 | 1.0 | σt = σr | 50 | 1.77 | 0.15 | 1.5 | plane-strain |
| Series D | 4.3 | 2.75 | σa | 71 | 3.55 | 0.60 | 5.9 | plane-stress |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zorc, B.; Zorc, M.; Kosec, B.; Nagode, A. Effect of the Shape of Styrene–Acrylonitrile Water-Filter Housings on the Destructive Pressure, Crack-Initiation, Propagation Conditions and Fracture Toughness of Styrene–Acrylonitrile. Polymers 2020, 12, 280. https://doi.org/10.3390/polym12020280
Zorc B, Zorc M, Kosec B, Nagode A. Effect of the Shape of Styrene–Acrylonitrile Water-Filter Housings on the Destructive Pressure, Crack-Initiation, Propagation Conditions and Fracture Toughness of Styrene–Acrylonitrile. Polymers. 2020; 12(2):280. https://doi.org/10.3390/polym12020280
Chicago/Turabian StyleZorc, Borut, Matija Zorc, Borut Kosec, and Aleš Nagode. 2020. "Effect of the Shape of Styrene–Acrylonitrile Water-Filter Housings on the Destructive Pressure, Crack-Initiation, Propagation Conditions and Fracture Toughness of Styrene–Acrylonitrile" Polymers 12, no. 2: 280. https://doi.org/10.3390/polym12020280
APA StyleZorc, B., Zorc, M., Kosec, B., & Nagode, A. (2020). Effect of the Shape of Styrene–Acrylonitrile Water-Filter Housings on the Destructive Pressure, Crack-Initiation, Propagation Conditions and Fracture Toughness of Styrene–Acrylonitrile. Polymers, 12(2), 280. https://doi.org/10.3390/polym12020280






