A Kinetic Analysis of the Thermal Degradation Behaviours of Some Bio-Based Substrates
Abstract
1. Introduction
2. Materials and Methods
3. Results and Discussion
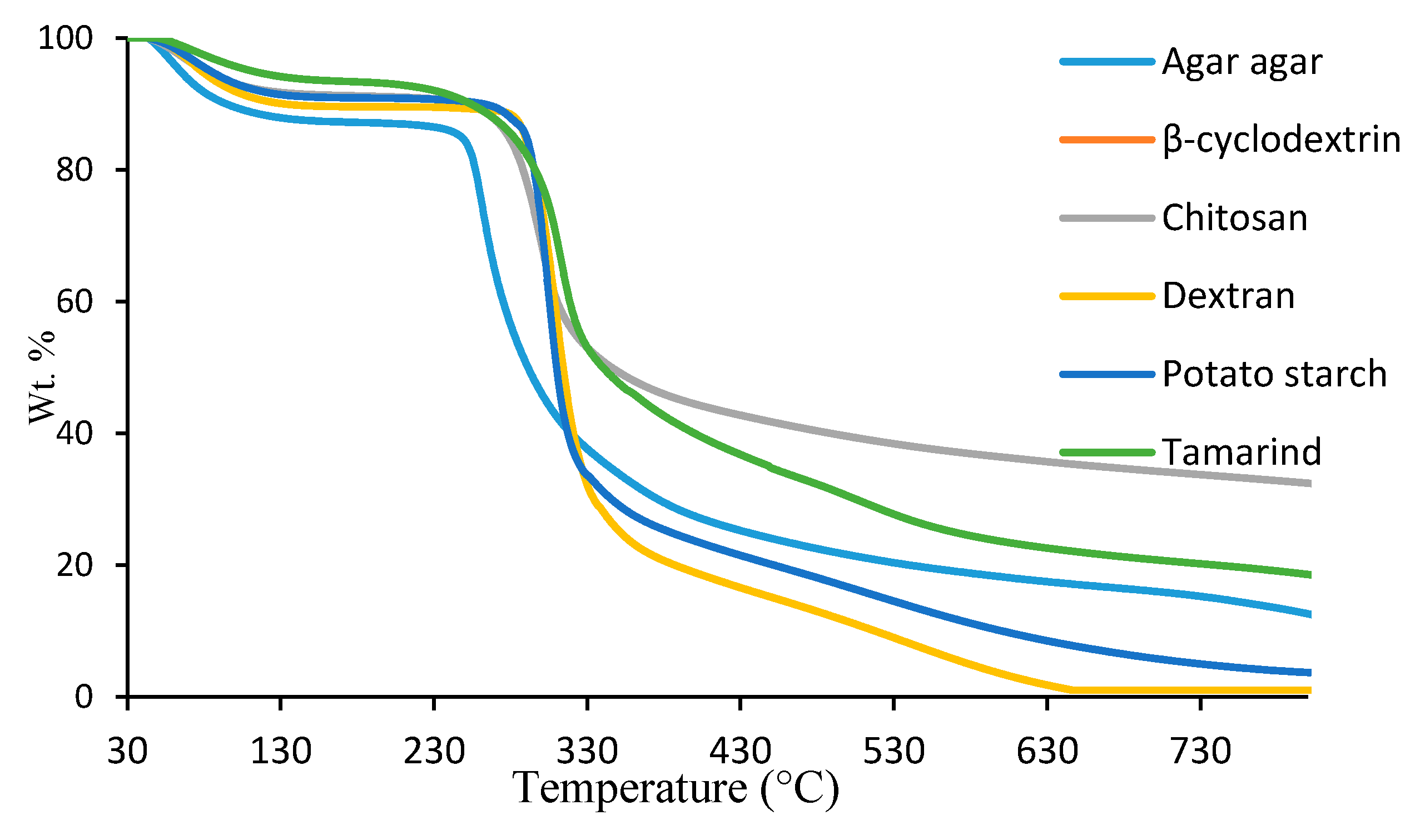
3.1. Detailed Kinetic Analysis
3.1.1. Flynn–Wall–Ozawa (FWO) Method
3.1.2. Method Using the Propriety Software
3.1.3. Correlation of Ea Values with Some Relevant Combustion Parameters
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Babu, R.P.; O’connor, K.; Seeram, R. Current progress on bio-based polymers and their future trends. Prog. Biomater. 2013, 2, 8. [Google Scholar] [CrossRef]
- Garrison, T.F.; Murawski, A.; Quirino, R.L. Bio-based polymers with potential for biodegradability. Polymers 2016, 8, 262. [Google Scholar] [CrossRef]
- Imre, B.; Pukánszky, B. Compatibilization in bio-based and biodegradable polymer blends. Eur. Polym. J. 2013, 49, 1215–1233. [Google Scholar] [CrossRef]
- Zia, K.M.; Noreen, A.; Zuber, M.; Tabasum, S.; Mujahid, M. Recent developments and future prospects on bio-based polyesters derived from renewable resources: A review. Int. J. Biol. Macromol. 2016, 82, 1028–1040. [Google Scholar] [CrossRef]
- Isikgor, F.H.; Becer, C.R. Lignocellulosic biomass: A sustainable platform for the production of bio-based chemicals and polymers. Polym. Chem. 2015, 6, 4497–4559. [Google Scholar] [CrossRef]
- Chai, M.W.; Bickerton, S.; Bhattacharyya, D.; Das, R. Influence of natural fibre reinforcements on the flammability of bio-derived composite materials. Compos. Part B Eng. 2012, 43, 2867–2874. [Google Scholar] [CrossRef]
- Kalali, E.N.; Zhang, L.; Shabestari, M.E.; Croyal, J.; Wang, D.Y. Flame-retardant wood polymer composites (WPCs) as potential fire safe bio-based materials for building products: Preparation, flammability and mechanical properties. Fire Saf. J. 2019, 107, 210–216. [Google Scholar] [CrossRef]
- Thomas, A.; Joseph, P.; Moinuddin, K.; Zhu, H.; Tretsiakova-McNally, S. Thermal and Calorimetric Evaluations of Some Chemically Modified Carbohydrate-Based Substrates with Phosphorus-Containing Groups. Polymers 2020, 12, 588. [Google Scholar] [CrossRef] [PubMed]
- Thomas, A.; Moinuddin, K.; Zhu, H.; Joseph, P. Passive fire protection of wood using some bio-derived fire retardants. Fire Saf. J. 2020, in press. [Google Scholar] [CrossRef]
- Dirion, J.L.; Reverte, C.; Cabassud, M. Kinetic parameter estimation from TGA: Optimal design of TGA experiments. Chem. Eng. Res. Des. 2008, 86, 618–625. [Google Scholar] [CrossRef]
- El-Sayed, S.A.; Mostafa, M. Pyrolysis characteristics and kinetic parameters determination of biomass fuel powders by differential thermal gravimetric analysis (TGA/DTG). Energy Convers. Manag. 2014, 85, 165–172. [Google Scholar] [CrossRef]
- Marcilla, A.; Beltran, M. Thermogravimetric kinetic study of poly (vinyl chloride) pyrolysis. Polym. Degrad. Stab. 1995, 48, 219–229. [Google Scholar] [CrossRef]
- Budrugeac, P. The evaluation of the non-isothermal kinetic parameters of the thermal and thermo-oxidative degradation of polymers and polymeric materials: Its use and abuse. Polym. Degrad. Stab. 2000, 71, 185–187. [Google Scholar] [CrossRef]
- Gai, C.; Dong, Y.; Zhang, T. The kinetic analysis of the pyrolysis of agricultural residue under non-isothermal conditions. Bioresour. Technol. 2013, 127, 298–305. [Google Scholar] [CrossRef] [PubMed]
- Harbourne, N.; Jacquier, J.C.; Morgan, D.J.; Lyng, J.G. Determination of the degradation kinetics of anthocyanins in a model juice system using isothermal and non-isothermal methods. Food Chem. 2008, 111, 204–208. [Google Scholar] [CrossRef]
- Flynn, J.H.; Wall, L.A. A quick, direct method for the determination of activation energy from thermogravimetric data. J. Polym. Sci. Part B Polym. Lett. 1966, 4, 323–328. [Google Scholar] [CrossRef]
- Ozawa, T. Estimation of activation energy by isoconversion methods. Thermochim. Acta 1992, 203, 159–165. [Google Scholar] [CrossRef]
- Moreno, R.M.; De Medeiros, E.S.; Ferreira, F.C.; Alves, N.; Gonçalves, P.D.S.; Mattoso, L.H.C. Thermogravimetric studies of decomposition kinetics of six different IAC Hevea rubber clones using Flynn–Wall–Ozawa approach. Plast. Rubber Compos. 2006, 35, 15–21. [Google Scholar] [CrossRef]
- Du, W.; Wang, G.; Wang, Y.; Liu, X. Thermal degradation of bituminous coal with both model-free and model-fitting methods. Appl. Therm. Eng. 2019, 152, 169–174. [Google Scholar] [CrossRef]
- Iqbal, M.S.; Massey, S.; Akbar, J.; Ashraf, C.M.; Masih, R. Thermal analysis of some natural polysaccharide materials by isoconversional method. Food Chem. 2013, 140, 178–182. [Google Scholar] [CrossRef]
- Opfermann, J.; Kaisersberger, E. An advantageous variant of the Ozawa-Flynn-Wall analysis. Thermochim. Acta 1992, 203, 167–175. [Google Scholar] [CrossRef]
- Vyazovkin, S.; Wight, C.A. Model-free and model-fitting approaches to kinetic analysis of isothermal and nonisothermal data. Thermochim. Acta 1999, 340, 53–68. [Google Scholar] [CrossRef]
- Howell, B.A. The utility of variable temperature techniques in the determination of kinetic parameters. Thermochim. Acta 2002, 388, 275–281. [Google Scholar] [CrossRef]
- Bigger, S.W.; Cran, M.J.; Bohn, M.A. Novel theoretical and computer-assisted modeling of isothermal and non-isothermal depolymerization kinetics. Polym. Test. 2015, 44, 1–7. [Google Scholar] [CrossRef]
- Bigger, S.W.; Cran, M.J.; Tawakkal, I.S. Two novel algorithms for the thermogravimetric assessment of polymer degradation under non-isothermal conditions. Polym. Test. 2015, 43, 139–146. [Google Scholar] [CrossRef]
- Lyon, R.E.; Walters, R.N. Pyrolysis combustion flow calorimetry. J. Anal. Appl. Pyrolysis 2004, 71, 27–46. [Google Scholar] [CrossRef]
- Tretsiakova-McNally, S.; Joseph, P. Pyrolysis combustion flow calorimetry studies on some reactively modified polymers. Polymers 2015, 7, 453–467. [Google Scholar] [CrossRef]
- Solorzano, J.A.P.; Moinuddin, K.A.M.; Tretsiakova-McNally, S.; Joseph, P. A Study of the Thermal Degradation and Combustion Characteristics of Some Materials Commonly Used in the Construction Sector. Polymers 2019, 11, 1833. [Google Scholar] [CrossRef]
- Westmoreland, P.R.; Inguilizian, T.; Rotem, K. Flammability kinetics from TGA/DSC/GCMS, microcalorimetry and computational quantum chemistry. Thermochim. Acta 2001, 367, 401–405. [Google Scholar] [CrossRef]
- Burnham, A.K. Use and misuse of logistic equations for modeling chemical kinetics. J. Therm. Anal. Calorim. 2017, 127, 1107–1116. [Google Scholar] [CrossRef]
- Wadhwani, R.; Sutherland, D.; Moinuddin, K.A.M.; Joseph, P. Kinetics of pyrolysis of litter materials from pine and eucalyptus forests. J. Therm. Anal. Calorim. 2017, 130, 2035–2046. [Google Scholar] [CrossRef]






| Sl. No. | log β (K·min−1) | Temp (°C) | Temp (T) (K) | 1/T (K−1) |
|---|---|---|---|---|
| 1 | 0.6989 | 279 | 552 | 0.00181079 |
| 2 | 1.0000 | 287 | 560 | 0.00178704 |
| 3 | 1.3010 | 285 | 558 | 0.00179163 |
| 4 | 1.4771 | 309 | 582 | 0.00171812 |
| 5 | 1.7782 | 322 | 595 | 0.00167868 |
| Sl. No. | α | Potato Starch | β-Cyclodextrin | Dextran | Agar-Agar | Tamarind | Chitosan |
|---|---|---|---|---|---|---|---|
| 1 | 0.2 | 169 | 143 | 169 | 141 | 123 | 191 |
| 2 | 0.3 | 175 | 152 | 183 | 143 | 165 | 160 |
| 3 | 0.4 | 178 | 154 | 202 | 138 | 182 | * |
| 4 | 0.5 | 187 | 161 | 220 | 140 | 213 | 168 |
| 5 | 0.6 | 236 | 169 | 249 | # | # | 167 |
| † STDEV | 27.1 | 9.78 | 31.4 | 2.08 | 37.5 | 13.5 |
| Sl. No. | Kinetic Model | Equation | Ea (kJ·mol−1) | A (s−1) | R2 |
|---|---|---|---|---|---|
| 1 | P1 Power Law | α1/n | * - | - | - |
| 2 | E1 Exponential law | ln(α) | * - | - | - |
| 3 | A2 Avrami–Erofeev Model | [−ln(1 − α)]1/2 | 43.0 | 1.029 × 103 | 0.9933 |
| 4 | A3 Avrami–Erofeev Model | [−ln(1 − α)]1/3 | 26.0 | 1.880 × 101 | 0.9931 |
| 5 | A4 Avrami–Erofeev Model | [−ln(1 − α)]1/4 | 18.0 | 2.631 × 100 | 0.9930 |
| 6 | B1 Prout–Tompkins | [−ln(α/(1 − α))] + C | * - | - | - |
| 7 | R1 Contracting area | 1 − (1 − α)1/2 | 86.0 | 1.682 × 107 | 0.9944 |
| 8 | R3 Contracting volume | 1 − (1 − α)1/3 | 89.0 | 6.174 × 106 | 0.9942 |
| 9 | D1 One dimensional | α2 | 164 | 8.417 × 1013 | 0.9952 |
| 10 | D2 Two dimensional | (1 − α)ln(1 − α) + α | 175 | 9.164 × 1014 | 0.9744 |
| 11 | D3 Three dimensional | [1 − (1 − α)1/3]2 | 187 | 1.235 × 1016 | 0.9765 |
| 12 | D4 Ginstling–Brounshtein | (1 − 2α/3) − (1 − α)2/3 | 179 | 2.181 × 1015 | 0.9751 |
| 13 | F1 First order | −ln(1 − α) | 95.0 | 2.330 × 107 | 0.9880 |
| 14 | F2 Second order | 1/(1 − α) | 32.0 | 1.487 × 101 | 0.9050 |
| 15 | F3 Third order | 1/(1 − α)2 | 72.0 | 1.400 × 105 | 0.7580 |
| Sl. No. | Substrate | Ea (FWO Method (kJ·mol−1) | * Ea (In-House Method) (kJ·mol−1) | A (s−1) | # R2 | Kinetic Model Chosen |
|---|---|---|---|---|---|---|
| 1 | β-cyclodextrin | 156 | 118 | 7.74 × 109 | 0.997 | Avrami–Erofeev |
| 2 | Dextran | 205 | 160 | 6.93 × 1013 | 0.993 | First order |
| 3 | Potato starch | 189 | 188 | 1.03 × 1016 | 0.976 | Contracting volume |
| 4 | Agar-agar | 141 | 140 | 4.78 × 1011 | 0.890 | Two-Dimensional Diffusion |
| 5 | Tamarind | 170 | 170 | 1.79 × 1013 | 0.990 | Ginstling–Brounshtein |
| 6 | Chitosan | 146 | 164 | 6.71 × 1013 | 0.995 | One dimensional diffusion |
| Sample | pHRR (W·g−1) | THR (kJ·g−1) | HRC (J·g−1·K−1) | Char Yield (wt. %) | * hc (kJ/g) |
|---|---|---|---|---|---|
| β-cyclodextrin | 453 | 11.6 | 459 | 11.11 | 13.03 |
| Dextran | 289 | 10.4 | 288 | # - | # 9 |
| Potato Starch | 363 | 10.4 | 368 | 12.50 | 11.84 |
| Agar-agar | 256 | 12.3 | 250 | 3.680 | 12.75 |
| Tamarind | 158 | 10.0 | 155 | 25.12 | 13.30 |
| Chitosan | 103 | 6.60 | 107 | # - | # - |
| Sl. No. | Sample | Ea (kJ mol−1) | THR (kJ g−1) | hc (kJ g−1) | HRC (J g−1 K−1) | pHRR (W g−1) |
|---|---|---|---|---|---|---|
| 1 | β-cyclodextrin | 156 | 11.6 | 13.03 | 459 | 453 |
| 2 | Dextran | 205 | 10.4 | --- | 288 | 289 |
| 3 | Potato Starch | 189 | 10.4 | 11.81 | 368 | 363 |
| 4 | Agar-agar | 141 | 12.3 | 12.75 | 250 | 256 |
| 5 | Tamarind | 170 | 10.0 | 13.30 | 155 | 158 |
| 6 | Chitosan | 146 | 6.60 | ---- | 107 | 103 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Thomas, A.; Moinuddin, K.; Tretsiakova-McNally, S.; Joseph, P. A Kinetic Analysis of the Thermal Degradation Behaviours of Some Bio-Based Substrates. Polymers 2020, 12, 1830. https://doi.org/10.3390/polym12081830
Thomas A, Moinuddin K, Tretsiakova-McNally S, Joseph P. A Kinetic Analysis of the Thermal Degradation Behaviours of Some Bio-Based Substrates. Polymers. 2020; 12(8):1830. https://doi.org/10.3390/polym12081830
Chicago/Turabian StyleThomas, Ananya, Khalid Moinuddin, Svetlana Tretsiakova-McNally, and Paul Joseph. 2020. "A Kinetic Analysis of the Thermal Degradation Behaviours of Some Bio-Based Substrates" Polymers 12, no. 8: 1830. https://doi.org/10.3390/polym12081830
APA StyleThomas, A., Moinuddin, K., Tretsiakova-McNally, S., & Joseph, P. (2020). A Kinetic Analysis of the Thermal Degradation Behaviours of Some Bio-Based Substrates. Polymers, 12(8), 1830. https://doi.org/10.3390/polym12081830







