System Size Dependence in the Zimm–Bragg Model: Partition Function Limits, Transition Temperature and Interval
Abstract
:1. Introduction
2. Materials and Methods
2.1. Hamiltonian Formulation of the Zimm–Bragg Model
2.2. Mapping the Potts-Like Spin Language to Zimm–Bragg Model
2.3. Eigenvalue Analysis of the Zimm–Bragg Model
3. Results
3.1. Size Limits of Partition Function
3.1.1. Infinite Chain Limit
3.1.2. Long Chain Limit
3.1.3. Short Chain Limit or Single Sequence Approximation
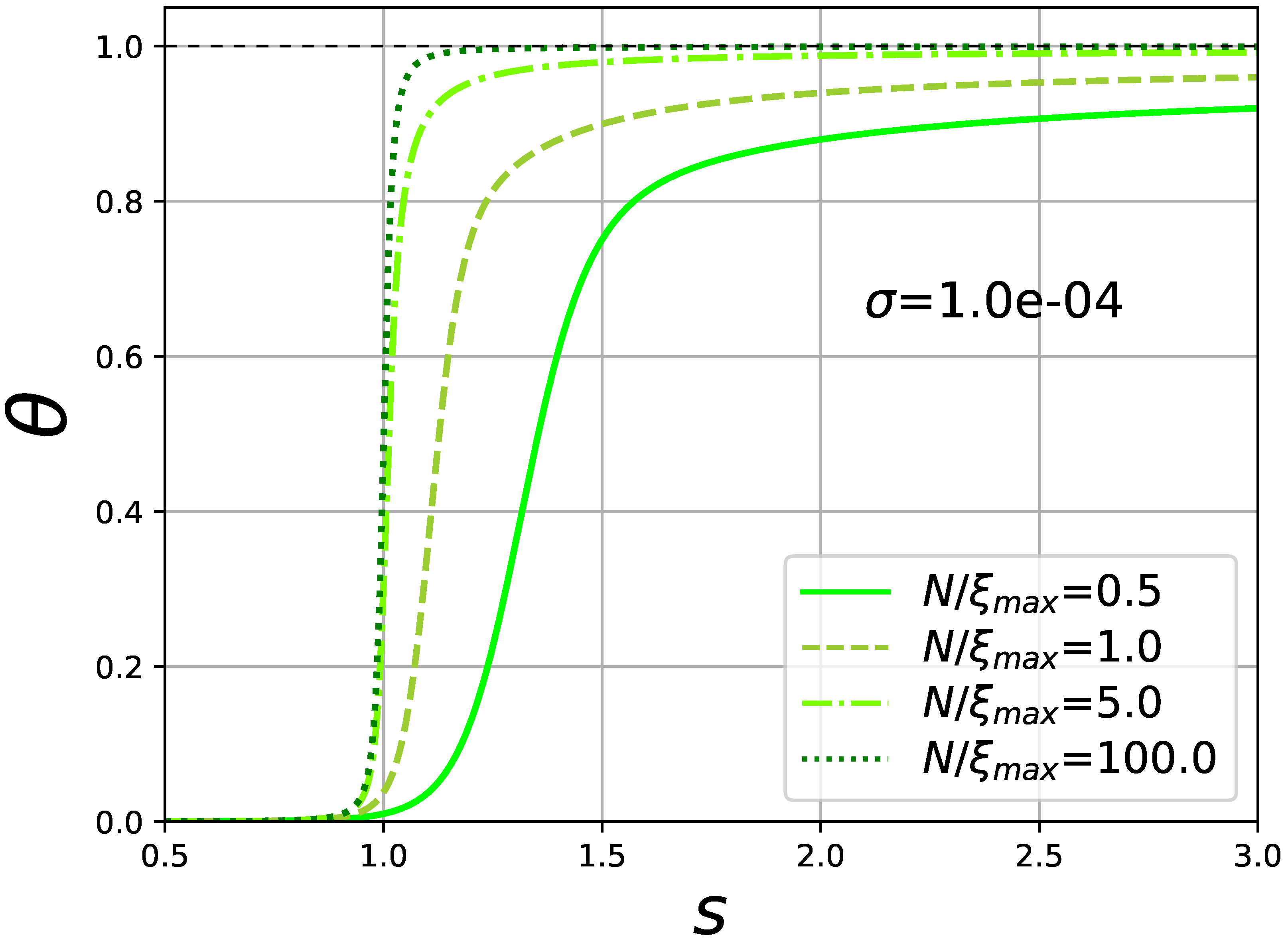
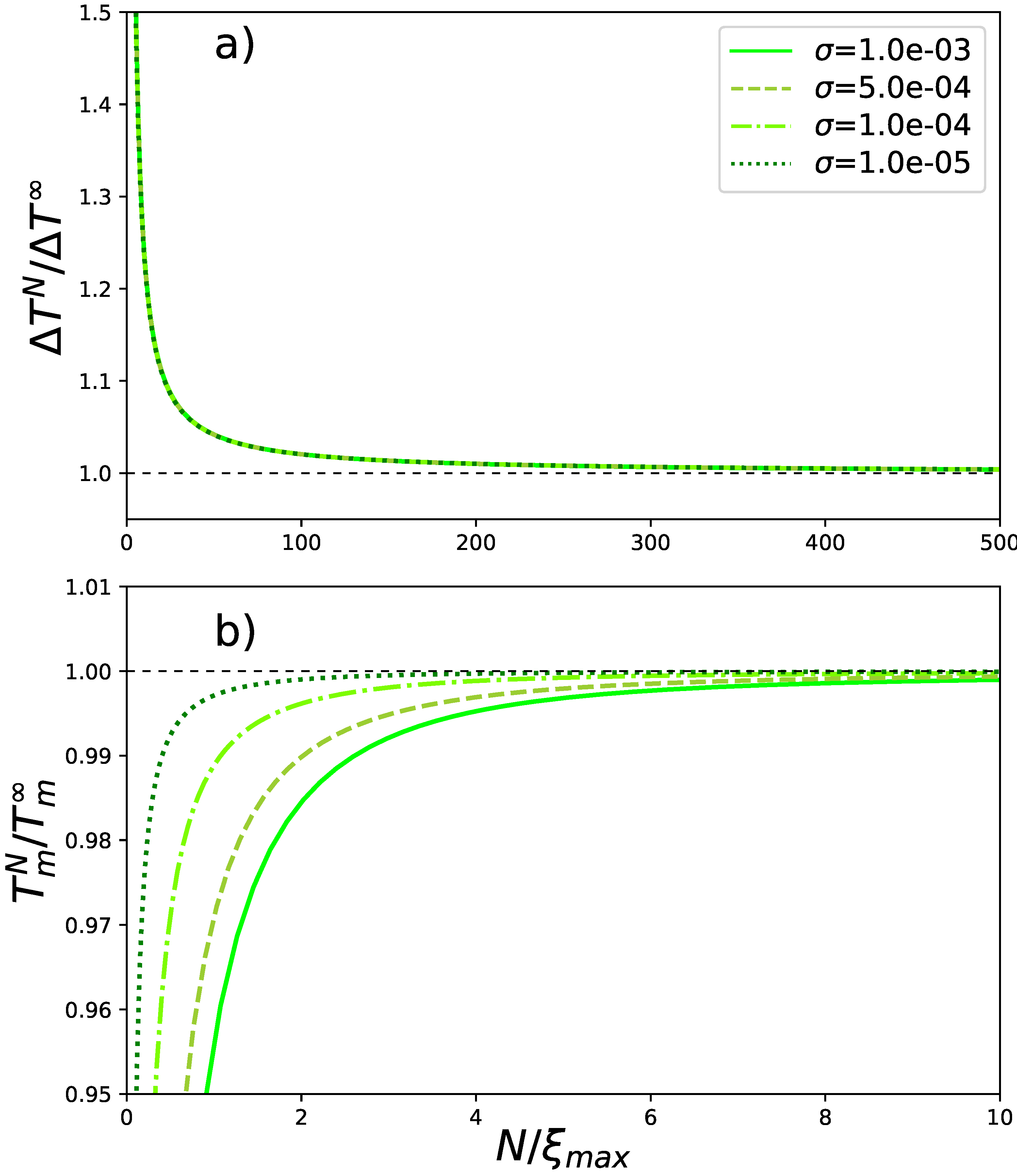
3.2. Exact Solution and Measurable Quantities
4. Discussion
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Zimm, B.H.; Bragg, J.K. Theory of the Phase Transition between Helix and Random Coil in Polypeptide Chains. J. Chem. Phys. 1959, 31, 526–535. [Google Scholar] [CrossRef] [Green Version]
- Poland, D.; Scheraga, H.A. Theory of Helix-Coil Transitions in Biopolymers; Academic Press: New York, NY, USA, 1970. [Google Scholar]
- Baxter, R.J. Exactly Solved Models in Statistical Mechanics; Academic Press: San Diego, CA, USA, 1982. [Google Scholar]
- Tonoyan, S.; Khechoyan, D.; Mamasakhlisov, Y.; Badasyan, A. Statistical mechanics of DNA-nanotube adsorption. Phys. Rev. E. 2020, 101, 062422. [Google Scholar] [CrossRef]
- Cui, X.; Xu, S.; Wang, X.; Chen, C. The nano-bio interaction and biomedical applications of carbon nanomaterials. Carbon 2018, 138, 436–450. [Google Scholar] [CrossRef]
- Zhu, Z. An Overview of Carbon Nanotubes and Graphene for Biosensing Applications. Nano-Micro Lett. 2017, 9, 25. [Google Scholar] [CrossRef] [Green Version]
- Safaee, M.M.; Gravely, M.; Rocchio, C.; Simmeth, M.; Roxbury, D. DNA Sequence Mediates Apparent Length Distribution in Single- Walled Carbon Nanotubes. ACS Appl. Mater. Interfaces 2019, 11, 2225–2233. [Google Scholar] [CrossRef] [Green Version]
- Ren, Y.; Baumgartner, R.; Fu, H.; van der Schoot, P.; Cheng, J.; Lin, Y. Revisiting the Helical Cooperativity of Synthetic Polypeptides in Solution. Biomacromolecules 2017, 18, 2324–2332. [Google Scholar] [CrossRef] [PubMed]
- Badasyan, A.V.; Giacometti, A.; Mamasakhlisov, Y.S.; Morozov, V.F.; Benight, A.S. Microscopic formulation of the Zimm–Bragg model for the helix-coil transition. Phys. Rev. E 2010, 81, 021921. [Google Scholar] [CrossRef] [Green Version]
- Ananikyan, N.S.; Hajryan, S.A.; Mamasakhlisov, E.S.; Morozov, V.F. Helix-coil transition in polypeptides: A microscopical approach. Biopolymers 1990, 30, 357–367. [Google Scholar] [CrossRef]
- Morozov, V.; Mamasakhlisov, E.; Hayryan, S.; Chin-Kun, H. Microscopical approach to the helix—coil transition in DNA. Physica A 2000, 281, 51–59. [Google Scholar] [CrossRef]
- Badasyan, A.V.; Grygoryan, A.V.; Mamasakhlisov, E.S.; Benight, A.S.; Morozov, V.F. The helix-coil transition in heterogeneous double stranded DNA: Microcanonical method. J. Chem. Phys. 2005, 123, 194701. [Google Scholar] [CrossRef] [PubMed]
- Badasyan, A.; Giacometti, A.; Podgornik, R.; Mamasakhlisov, Y.; Morozov, V. Helix-coil transition in terms of Potts-like spins. Eur. Phys. J. E 2013, 36, 1–9. [Google Scholar] [CrossRef] [PubMed]
- Kramers, H.A.; Wannier, G.H. Statistics of the two-dimensional ferromagnet. Phys. Rev. 1941, 60, 252–262. [Google Scholar] [CrossRef]
- Flory, P. Statistical Mechanics of Chain Molecules; Interscience: New York, NY, USA, 1969. [Google Scholar]
- Landau, L.; Lifshits, E. Statistical Physics; Pergamon: Oxford, UK, 1988. [Google Scholar]
- Schellman, J.A. The Factors Affecting the Stability of Hydrogen-bonded Polypeptide Structures in Solution. J. Chem. Phys. 1958, 62, 1485–1494. [Google Scholar] [CrossRef]
- Qian, H.; Schellman, J.A. Helix-Coil Theories: A Comparative Study for Finite Length Polypeptide. J. Phys. Chem. 1992, 96, 3987–3994. [Google Scholar] [CrossRef]
- Gibbs, J.H.; DiMarzio, E.A. Statistical Mechanics of Helix-Coil Transitions in Biological Macromolecules. J. Chem. Phys. 1959, 30, 271–282. [Google Scholar] [CrossRef]
- Kittel, C. Phase transition of a molecular zipper. Am. J. Phys. 1969, 37, 917–920. [Google Scholar] [CrossRef]
- Cantor, C.; Schimmel, T. Biophysical Chemistry; Freeman and Co.: San-Francisco, CA, USA, 1980. [Google Scholar]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Badasyan, A. System Size Dependence in the Zimm–Bragg Model: Partition Function Limits, Transition Temperature and Interval. Polymers 2021, 13, 1985. https://doi.org/10.3390/polym13121985
Badasyan A. System Size Dependence in the Zimm–Bragg Model: Partition Function Limits, Transition Temperature and Interval. Polymers. 2021; 13(12):1985. https://doi.org/10.3390/polym13121985
Chicago/Turabian StyleBadasyan, Artem. 2021. "System Size Dependence in the Zimm–Bragg Model: Partition Function Limits, Transition Temperature and Interval" Polymers 13, no. 12: 1985. https://doi.org/10.3390/polym13121985





