Effect of Natural Aging on Oak Wood Fire Resistance
Abstract
:1. Introduction
2. Experimental
2.1. Material
2.2. Methods
2.2.1. Chemical Composition of Wood
2.2.2. Flame Ignition Temperature and Spontaneous Ignition Temperatures
- τ—induction time of spontaneous ignition (s);
- A—pre-exponential (frequency) factor (-);
- E—activation energy of spontaneous ignition (J·mol−1);
- R—gas constant (8.314 J·K−1·mol−1);
- T—ignition thermodynamic temperature (K).
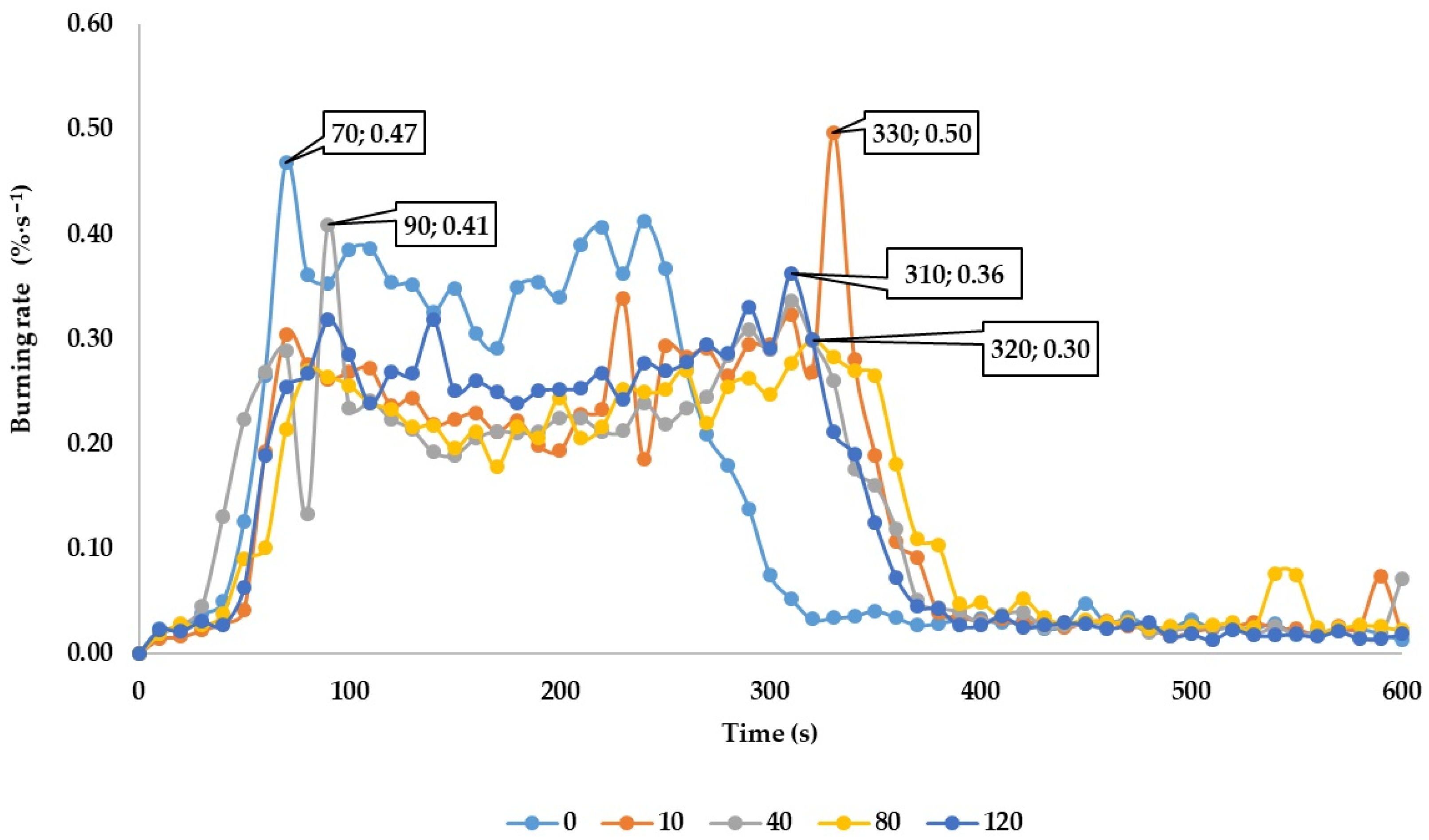
2.2.3. The Mass Burning Rate
- —absolute burning rate (%·s−1);
- δ (τ)—specimen mass in the time (τ) (%);
- δ (τ + Δτ)—specimen mass in the time (τ + Δτ) (%);
- Δτ—time interval in which the mass values are recorded (s).
3. Results and Discussion
3.1. Wood Chemical Composition
3.2. Fire-Technical Properties
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- EN 1995-1-1 + A1. Eurocode 5. Design of Wooden Structures. Depending on the Required Fire Resistance of the Building Structure, the Minimum Cross-Sectional Dimensions of Load-Bearing Wooden Elements in Wooden Structures Must Be Designed; European Committee for Standardization: Brussels, Belgium, 2008. [Google Scholar]
- Kačíková, D.; Majlingová, A.; Veľková, V.; Zachar, M. Modelling of Internal Fires Using the Results of Progressive Methods of Fire Engineering, 1st ed.; Technical University: Zvolen, Slovakia, 2017; p. 147. [Google Scholar]
- Friquin, K.L. Material properties and external factors influencing the charring rate of solid wood and glue-laminated timber. Fire Mater. 2010, 35, 303–327. [Google Scholar] [CrossRef]
- Cachim, P.B.; Franssen, J.-M. Assessment of Eurocode 5 Charring Rate Calculation Methods. Fire Technol. 2010, 46, 169–181. [Google Scholar] [CrossRef]
- White, R.; Dietenberger, M. Wood Products: Thermal Degradation and Fire. In Encyclopedia of Materials: Science and Technology; Elsevier BV: Amsterdam, The Netherlands, 2001; pp. 9712–9716. [Google Scholar]
- Bartlett, A.I.; Hadden, R.M.; Bisby, L.A. A Review of Factors Affecting the Burning Behaviour of Wood for Application to Tall Timber Construction. Fire Technol. 2019, 55, 1–49. [Google Scholar] [CrossRef] [Green Version]
- Lau, P.W.C.; White, R.; Van Zeeland, I. Modelling the charring behaviour of structural lumber. Fire Mater. 1999, 23, 209–216. [Google Scholar] [CrossRef]
- Očkajová, A.; Kučerka, M.; Kminiak, R.; Krišťák, Ľ.; Igaz, R.; Réh, R. Occupational exposure to dust produced when milling thermally modified wood. Int. J. Environ. Res. Public Health 2020, 17, 1478. [Google Scholar] [CrossRef] [Green Version]
- Aristri, M.; Lubis, M.; Yadav, S.; Antov, P.; Papadopoulos, A.; Pizzi, A.; Fatriasari, W.; Ismayati, M.; Iswanto, A. Recent Developments in Lignin- and Tannin-Based Non-Isocyanate Polyurethane Resins for Wood Adhesives—A Review. Appl. Sci. 2021, 11, 4242. [Google Scholar] [CrossRef]
- Kačíková, D.; Kubovský, I.; Ulbriková, N.; Kačík, F. the impact of thermal treatment on structural changes of teak and iroko wood lignins. Appl. Sci. 2020, 10, 5021. [Google Scholar] [CrossRef]
- Frangi, A.; Fontana, M. Charring rates and temperature profiles of wood sections. Fire Mater. 2003, 27, 91–102. [Google Scholar] [CrossRef]
- Njankouo, J.M.; Dotreppe, J.-C.; Franssen, J.-M. Experimental study of the charring rate of tropical hardwoods. Fire Mater. 2004, 28, 15–24. [Google Scholar] [CrossRef]
- Schmid, J.; Just, A.; Klippel, M.; Fragiacomo, M. The Reduced Cross-Section Method for Evaluation of the Fire Resistance of Timber Members: Discussion and Determination of the Zero-Strength Layer. Fire Technol. 2015, 51, 1285–1309. [Google Scholar] [CrossRef]
- Sonderegger, W.; Kránitz, K.; Bues, C.-T.; Niemz, P. Aging effects on physical and mechanical properties of spruce, fir and oak wood. J. Cult. Herit. 2015, 16, 883–889. [Google Scholar] [CrossRef]
- Kránitz, K.; Sonderegger, W.; Bues, C.-T.; Niemz, P. Effects of aging on wood: A literature review. Wood Sci. Technol. 2016, 50, 7–22. [Google Scholar] [CrossRef]
- Topaloglu, E.; Ustaomer, D.; Ozturk, M.; Pesman, E. Changes in wood properties of chestnut wood structural elements with natural aging. Maderas Cienc. Tecnol. 2021, 23, 23. [Google Scholar] [CrossRef]
- Reinprecht, L. Wood Deterioration, Protection and Maintenance, 1st ed.; Wiley & Sons, Ltd.: Chichester, UK, 2016; pp. 145–217. [Google Scholar]
- Majlingová, A.; Zachar, M.; Lieskovský, M.; Mitterová, I. The analysis of mass loss and activation energy of selected fast-growing tree species and energy crops using the Arrhenius equation. Acta Fac. Xylologiae Zvolen 2018, 60, 175–186. [Google Scholar]
- Martinka, J.; Mózer, V.; Hroncová, E.; Ladomerský, J. Influence of spruce wood form on ignition activation energy. Wood Res. 2015, 60, 815–822. [Google Scholar]
- Rantuch, P.; Wachter, I.; Hrušovský, I.; Balog, K. Ignition Activation Energy of Materials based on Polyamide 6. Trans. VSB Tech. Univ. Ostrav. Saf. Eng. Ser. 2016, 11, 27–31. [Google Scholar] [CrossRef]
- Martinka, J.; Hroncová, E.; Kačíková, D.; Rantuch, P.; Balog, K.; Ladomerský, J. Ignition parameters of poplar wood. Acta Fac. Xylologiae 2017, 59, 85–95. [Google Scholar]
- Luptáková, J.; Kačík, F.; Eštoková, A.; Kačíková, D.; Šmíra, P.; Nasswettrová, A.; Bubeníková, T. Comparison of activation energy of thermal degradation of heat sterilised silver fir wood to larval frass regarding fire safety. Acta Fac. Xylologiae Zvolen 2018, 60, 19–29. [Google Scholar]
- Zachar, M.; Majlingová, A.; Šišulák, S.; Baksa, J. Comparison of the activation energy required for spontaneous ignition and flash point of the Norway spruce wood and thermowood specimens. Acta Fac. Xylologiae Zvolen 2017, 59, 79–90. [Google Scholar]
- Karlsson, B.; Quintiere, J. Enclosure Fire Dynamics; Informa UK Limited: London, UK, 1999; p. 336. [Google Scholar]
- Shi, L.; Chew, M.Y.L. Experimental study of woods under external heat flux by autoignition. J. Therm. Anal. Calorim. 2012, 111, 1399–1407. [Google Scholar] [CrossRef]
- STN ISO 871. Plastics. Determination of Ignition Temperature Using a Hot-Air Oven; International Organization for Standardization: Geneva, Switzerland, 2010. [Google Scholar]
- STN EN ISO 291. Plastics. Standard Atmospheres for Conditioning and Testing; International Organization for Standardization: Geneva, Switzerland, 2008. [Google Scholar]
- ASTM D1107-21. Standard Test Method for Ethanol-Toluene Solubility of Wood; ASTM International: West Conshohocken, PA, USA, 2021. [Google Scholar]
- Sluiter, A.; Hames, B.; Ruiz, R.; Scarlata, C.; Sluiter, J.; Templeton, D.; Crocker, D. Determination of Structural Carbohydrates and Lignin in Biomass (NREL/TP-510-42618); National Renewable Energy Laboratory: Golden, CO, USA, 2012. [Google Scholar]
- Seifert, V.K. About a new method for rapid determination of pure cellulose. Das Pap. 1956, 10, 301–306. (In German) [Google Scholar]
- Wise, L.E.; Murphy, M.; D’addieco, A.A. Chlorite holocellulose, its fractionation and bearing on summative wood analysis and on studies on the hemicelluloses. Pap. Trade J. 1946, 122, 35–44. [Google Scholar]
- ISO 10694. Soil Quality. Determination of Organic and Total Carbon after Dry Combustion (Elementary Analysis); International Organization for Standardization: Geneva, Switzerland, 1995. [Google Scholar]
- ISO 13878. Soil Quality. Determination of Total Nitrogen Content by Dry Combustion (Elemental Analysis); International Organization for Standardization: Geneva, Switzerland, 1998. [Google Scholar]
- ISO 15178. Soil Quality. Determination of Total Sulfur by Dry Combustion; International Organization for Standardization: Geneva, Switzerland, 2000. [Google Scholar]
- ISO 11885. Water Quality. Determination of Selected Elements by Inductively Coupled Plasma Optical Emission Spectrometry (ICP-OES); International Organization for Standardization: Geneva, Switzerland, 2007. [Google Scholar]
- ISO 11925-2. Reaction to Fire Tests—Ignitability of Products Subjected to Direct Impingement of Flame—Part 2: Single-Flame Source Test; International Organization for Standardization: Geneva, Switzerland, 2020. [Google Scholar]
- Santos, R.B.; Capanema, E.A.; Balakshin, M.Y.; Chang, H.; Jameel, H. Lignin structural variation in hardwood species. J. Agric. Food Chem. 2012, 60, 4923–4930. [Google Scholar] [CrossRef] [PubMed]
- Kolář, T.; Rybníček, M. The changes in chemical composition and properties of subfossil oak deposited in holocene sediments. Wood Res. 2014, 59, 149–166. [Google Scholar]
- Cárdenas-Gutiérrez, M.A.; Pedraza-Bucio, F.E.; López-Albarrán, P.; Rutiaga-Quiñones, J.G. Chemical components of the branches of six hardwood species. Wood Res. 2018, 63, 795–808. [Google Scholar]
- Hrčka, R.; Kučerová, V.; Hýrošová, T. Correlations between oak wood properties. BioResources 2018, 4, 8885–8898. [Google Scholar] [CrossRef]
- Kačík, F.; Šmíra, P.; Kačíková, D.; Reinprecht, L.; Nasswettrova, A. Chemical changes in fir wood from old buildings due to ageing. Cellul. Chem. Technol. 2014, 48, 79–88. [Google Scholar]
- Kučerová, V.; Lagaňa, R.; Výbohová, E.; Hýrošová, T. The effect of chemical changes during heat treatment on the color and mechanical properties of fir wood. BioResources 2016, 11, 9079–9094. [Google Scholar] [CrossRef]
- Kubovský, I.; Kačíková, D.; Kačík, F. Structural Changes of Oak Wood Main Components Caused by Thermal Modification. Polymers 2020, 12, 485. [Google Scholar] [CrossRef] [Green Version]
- Čabalová, I.; Zachar, M.; Kačík, F.; Tribulová, T. Impact of thermal loading on selected chemsical and morphological properties of spruce ThermoWood. BioResources 2019, 1, 387–400. [Google Scholar]
- Popescu, C.-M.; Hill, C.A.S. The water vapour adsorption–desorption behaviour of naturally aged Tilia cordata Mill. wood. Polym. Degrad. Stab. 2013, 98, 1804–1813. [Google Scholar] [CrossRef]
- Zhao, C.; Zhang, X.; Liu, L.; Yu, Y.; Zheng, W.; Song, P. Probing Chemical Changes in Holocellulose and Lignin of Timbers in Ancient Buildings. Polymers 2019, 11, 809. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Fengel, D.; Wegener, G. Wood—Chemistry, Ultrastructure, Reactions, 2nd ed.; Walter de Gruyter: Berlin, Germany, 1989; p. 613. [Google Scholar]
- Jebrane, M.; Pockrandt, M.; Cuccui, I.; Allegretti, O.; Uetimane, E.; Terziev, N. Comparative Study of two softwood species industrially modified by Thermowood (R) and thermo-vacuum process. BioResources 2018, 13, 715–728. [Google Scholar] [CrossRef] [Green Version]
- Passialis, C.N. Physico-chemical characteristics of waterlogged archaeological wood. Holzforschung 1977, 51, 111–113. [Google Scholar] [CrossRef]
- Florian, M.L.E. Scope and history of archaeological wood. In Archaeological Wood: Properties, Chemistry, and Preservation; Rowell, R.M., Barbour, R.J., Eds.; Oxford University Press: Pxford, UK, 1990; pp. 3–32. [Google Scholar]
- Krutul, D.; Kocoń, J. Inorganic constituents and scanning electron microscopic study of fossil oak wood (Quercus sp.). Holzforsch. Holzverwendung 1982, 34, 69–77. [Google Scholar]
- Teaca, C.A.; Roşu, D.; Mustaţă, F.; Rusu, T.; Roşu, L.; Roşca, I.; Varganici, C.D. Natural bio-based products for wood coating and protection against degradation: A Review. BioResources 2019, 14, 4873–4901. [Google Scholar] [CrossRef]
- Carrión, J.S. Plant Evolution (Evolución Vegetal); Marin, D., Ed.; University of Murcia: Madrid, Spain, 2003; p. 497. [Google Scholar]
- Krutul, D.; Radomski, A.; Zawadzki, J.; Zielenkiewicz, T.; Antczak, A. Comparison of the chemical composition of the fossil and recent oak wood. Wood Res. 2010, 55, 113–120. [Google Scholar]
- Wikberg, H.; Maunu, S.L. Characterisation of thermally modified hard- and softwoods by 13C CPMAS NMR. Carbohydr. Polym. 2004, 58, 461–466. [Google Scholar] [CrossRef]
- Čabalová, I.; Kačík, F.; Lagaňa, R.; Výbohová, E.; Bubeníková, T.; Čaňová, I.; Ďurkovič, J. Effect of thermal treatment on the chemical, physical, and mechanical properties of pedunculate oak (Quercus robur L.) wood. BioResources 2018, 13, 157–170. [Google Scholar] [CrossRef]
- Čabalová, I.; Bélik, M.; Kučerová, V.; Jurczyková, T. Chemical and Morphological Composition of Norway Spruce Wood (Picea abies, L.) in the Dependence of its Storage. Polymers 2021, 13, 1619. [Google Scholar] [CrossRef]
- Tureková, I.; Balog, K. Flame ignition parameters of polyethylene and activation energy of initiation of combustion process. Res. Pap. 2001, 11, 181–186. [Google Scholar]
- Kačíková, D.; Makovická-Osvaldová, L. Wood burning rate of various tree parts from selected softwoods. Acta Fac. Xylologiae Zvolen 2009, 51, 27–32. [Google Scholar]
| Approximate Age of Oak Sample (years) | Density (g·cm−3) |
|---|---|
| recent | 0.681 ± 0.03 |
| 10 | 0.641 ± 0.11 |
| 40 | 0.660 ± 0.28 |
| 80 | 0.688 ± 0.12 |
| 120 | 0.702 ± 0.21 |
| Age of Oak Sample (years) | Extractives (%) | Lignin (%) | Cellulose (%) | Holocellulose (%) | Hemicelluloses (%) | C/H Ratio |
|---|---|---|---|---|---|---|
| 0 | 3.93 ± 0.04 | 23.04 ± 0.14 | 33.48 ± 0.06 | 73.03 ± 0.11 | 39.55 ± 0.17 | 0.84 |
| 10 | 3.97 ± 0.06 | 22.86 ± 0.04 | 33.79 ± 0.57 | 73.16 ± 0.02 | 39.37 ± 0.55 | 0.86 |
| 40 | 5.78 ± 0.04 | 22.14 ± 0.02 | 33.41 ± 0.11 | 72.08 ± 0.06 | 38.67 ± 0.23 | 0.86 |
| 80 | 6.62 ± 0.03 | 22.31 ± 0.02 | 36.22 ± 0.01 | 71.08 ± 0.04 | 34.85 ± 0.03 | 1.04 |
| 120 | 7.34 ± 0.11 | 22.91 ± 0.04 | 36.40 ± 0.08 | 69.75 ± 0.15 | 33.35 ± 0.23 | 1.09 |
| Age of Oak Sample (years) | Carbon (g·kg−2) | Nitrogen (g·kg−2) | Sulfur (mg·kg−2) | Phosphor (g·kg−2) | Calcium (g·kg−2) | Magnesium (g·kg−2) | Potassium (g·kg−2) |
|---|---|---|---|---|---|---|---|
| 0 | 489 | 1.32 | 299 | 0.151 | 0.702 | 0.044 | 0.621 |
| 10 | 491 | 1.07 | 252 | 0.155 | 0.495 | 0.134 | 0.921 |
| 40 | 486 | 1.35 | 291 | 0.145 | 0.296 | 0.029 | 0.834 |
| 80 | 493 | 1.29 | 122 | 0.14 | 0.244 | 0.008 | 0.435 |
| 120 | 501 | 0.98 | 94 | 0.118 | 0.293 | 0.022 | 0.544 |
| Age of Oak Sample (years) | Thermal Loading | Average Time τ (s) | Average Temperature t (°C) | Average Temperature T (K) | Inverse Value 1/T (K−1) |
|---|---|---|---|---|---|
| 0 | FIT | 219.6 ± 21.38 | 436.02 ± 21.05 | 709.17 | 0.0014111 |
| SIT | 336.4 ± 18.49 | 374.96 ± 23.09 | 648.11 | 0.0015448 | |
| 10 | FIT | 257.4 ± 13.33 | 434.14 ± 23.14 | 706.19 | 0.0014153 |
| SIT | 336.1 ± 19.51 | 375.43 ± 24.30 | 648.58 | 0.0015436 | |
| 40 | FIT | 248.2 ± 25.28 | 432.43 ± 17.62 | 705.58 | 0.0014182 |
| SIT | 376.1 ± 20.29 | 371.24 ± 26.72 | 644.39 | 0.0015542 | |
| 80 | FIT | 221.6 ± 17.14 | 429.52 ± 16.24 | 702.67 | 0.0014274 |
| SIT | 379.2 ± 17.58 | 361.23 ± 22.13 | 633.33 | 0.0015777 | |
| 120 | FIT | 229.5 ± 24.30 | 430.48 ± 18.12 | 703.63 | 0.0014219 |
| SIT | 391.2 ± 19.54 | 363.37 ± 21.89 | 636.52 | 0.0015727 |
| Age of Oak Sample (years) | Exponential Equation | τ (s) | A | Activation Energy (kJ·mol−1) |
|---|---|---|---|---|
| 0 | y = 0.067 × e5715.9x | 219.6 | 0.0672 | 47.710 |
| 10 | y = 0.108 × e5472x | 257.4 | 0.1080 | 45.727 |
| 40 | y = 0.044 × e6077x | 248.2 | 0.0439 | 50.684 |
| 80 | y = 0.045 × e5941x | 221.6 | 0.0450 | 49.535 |
| 120 | y = 0.162 × e5090.9x | 229.5 | 0.1621 | 42.444 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zachar, M.; Čabalová, I.; Kačíková, D.; Jurczyková, T. Effect of Natural Aging on Oak Wood Fire Resistance. Polymers 2021, 13, 2059. https://doi.org/10.3390/polym13132059
Zachar M, Čabalová I, Kačíková D, Jurczyková T. Effect of Natural Aging on Oak Wood Fire Resistance. Polymers. 2021; 13(13):2059. https://doi.org/10.3390/polym13132059
Chicago/Turabian StyleZachar, Martin, Iveta Čabalová, Danica Kačíková, and Tereza Jurczyková. 2021. "Effect of Natural Aging on Oak Wood Fire Resistance" Polymers 13, no. 13: 2059. https://doi.org/10.3390/polym13132059
APA StyleZachar, M., Čabalová, I., Kačíková, D., & Jurczyková, T. (2021). Effect of Natural Aging on Oak Wood Fire Resistance. Polymers, 13(13), 2059. https://doi.org/10.3390/polym13132059







