Effect of Extrusion Parameters on Short Fiber Alignment in Fused Filament Fabrication
Abstract
:1. Introduction
- X—along the toolpath;
- Y—in the layer plane perpendicular to the toolpath;
- Z—perpendicular to the toolpath and the layer, in most cases this is also the tool axis.
2. Materials and Methods
2.1. Materials
2.2. Method
2.2.1. Parameter Identification & Design of Experiments
- Within the nozzle there is convergent flow in a narrow channel with flow along the tool axis Z due to the inner nozzle geometry. Nozzle diameter, polymer viscosity and flow velocity are the main factors.
- Under the nozzle, the flow turns to follow the toolpath. The layer height is usually lower than the nozzle diameter, therefore the flow is convergent in X with a cold wall on the part, that should result in the aforementioned stretched layer. Layer height in relation to the nozzle diameter is added to the list of relevant parameters to describe the degree of convergence of the gap between nozzle and part into which the material is pushed.
- The extrusion line may be wider than the nozzle and therefore become divergent in Y. This occurs in parallel to phase two and only if the extrusion line width is larger than the nozzle diameter, making the material flow to the side rather than just planar as in [45,46]. The relevant parameter is the extrusion line width in relation to the nozzle diameter, again a relative factor is used to describe the degree of divergence, as a pure line width would carry no information on the divergence of flow. A 0.6 mm line printed by a 0.4 mm nozzle has a divergence, while the same line printed by a 0.6 mm nozzle is purely planar flow.
- As the nozzle moves over the deposited material the material can be stretched, as the bottom of the material is standing still, while the nozzle edge moves over it in contact with the material. The parameters are the layer height and the speed of the nozzle.
- Fiber alignment in the XY plane, the aim information of this article
- Actual extrusion width/setpoint extrusion width, this is to ensure the extrusion process achieves the desired line geometry and is stable, without filament slipping.
- Surface quality on the XZ side of the printed specimens, this is also to ensure process stability. An irregular wall indicates an unstable extrusion process.
2.2.2. Analysis
- Transformed the image colors into a grayscale.
- Applied a threshold to transform the grayscale images into a binary of white fibers and black surroundings.
- Identified objects of white pixels and applied a threshold to delete smaller objects to filter and remove reflections of scratches or particles. In this case a threshold of 21 µm2 was used.
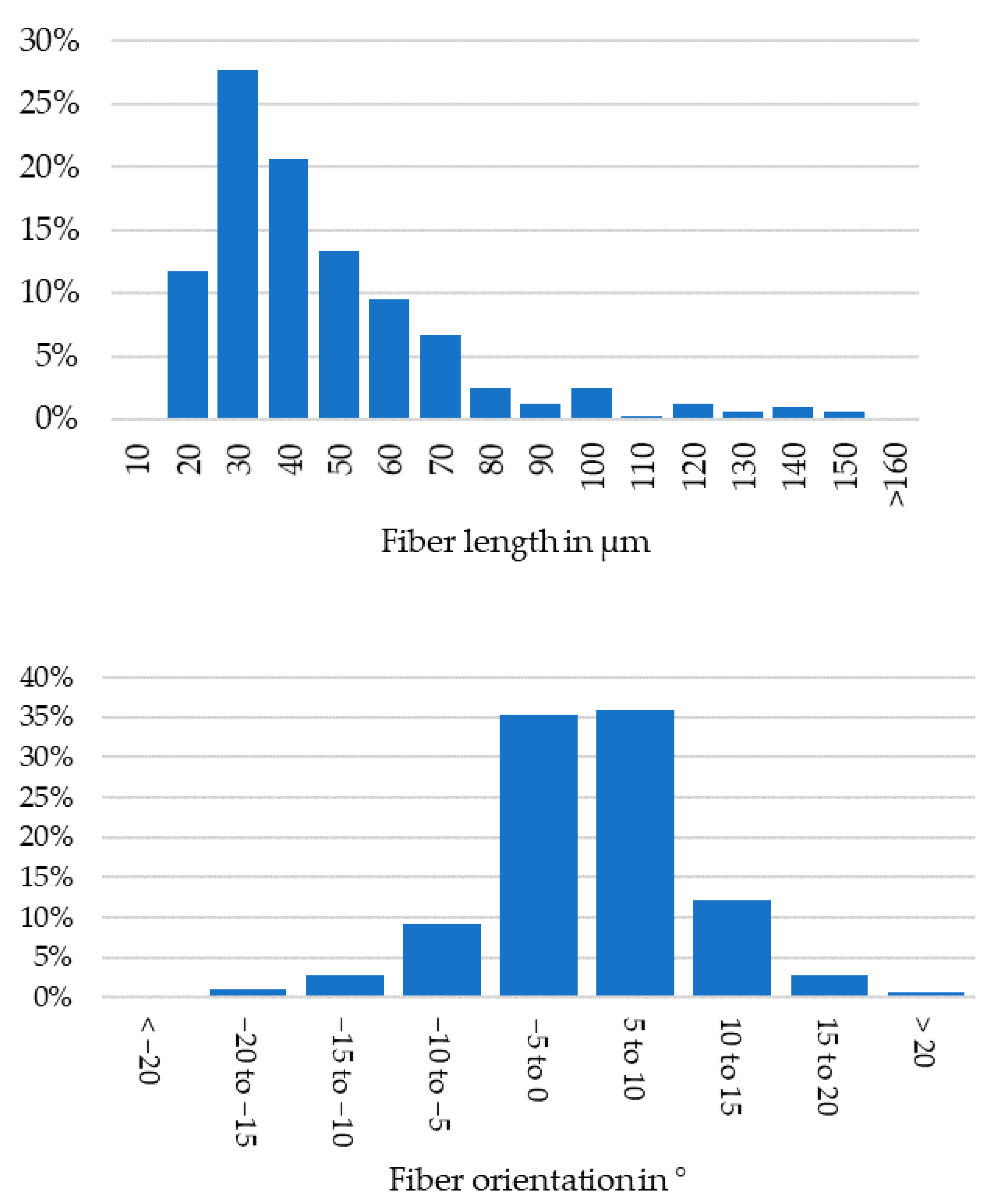
- Calculated the object location to then calculate the length of the main axis, the width perpendicular to it and orientation.
- It then classified the objects into fibers and large particles by considering objects with a length to width ratio of 3:1 a fiber and everything else a particle.
- Finally, it output the length and orientation distribution of the three classes, objects, fibers and particles to create plots of different statistical visualizations, such as histograms and cumulative curves as well as saving the data of the objects.
3. Results
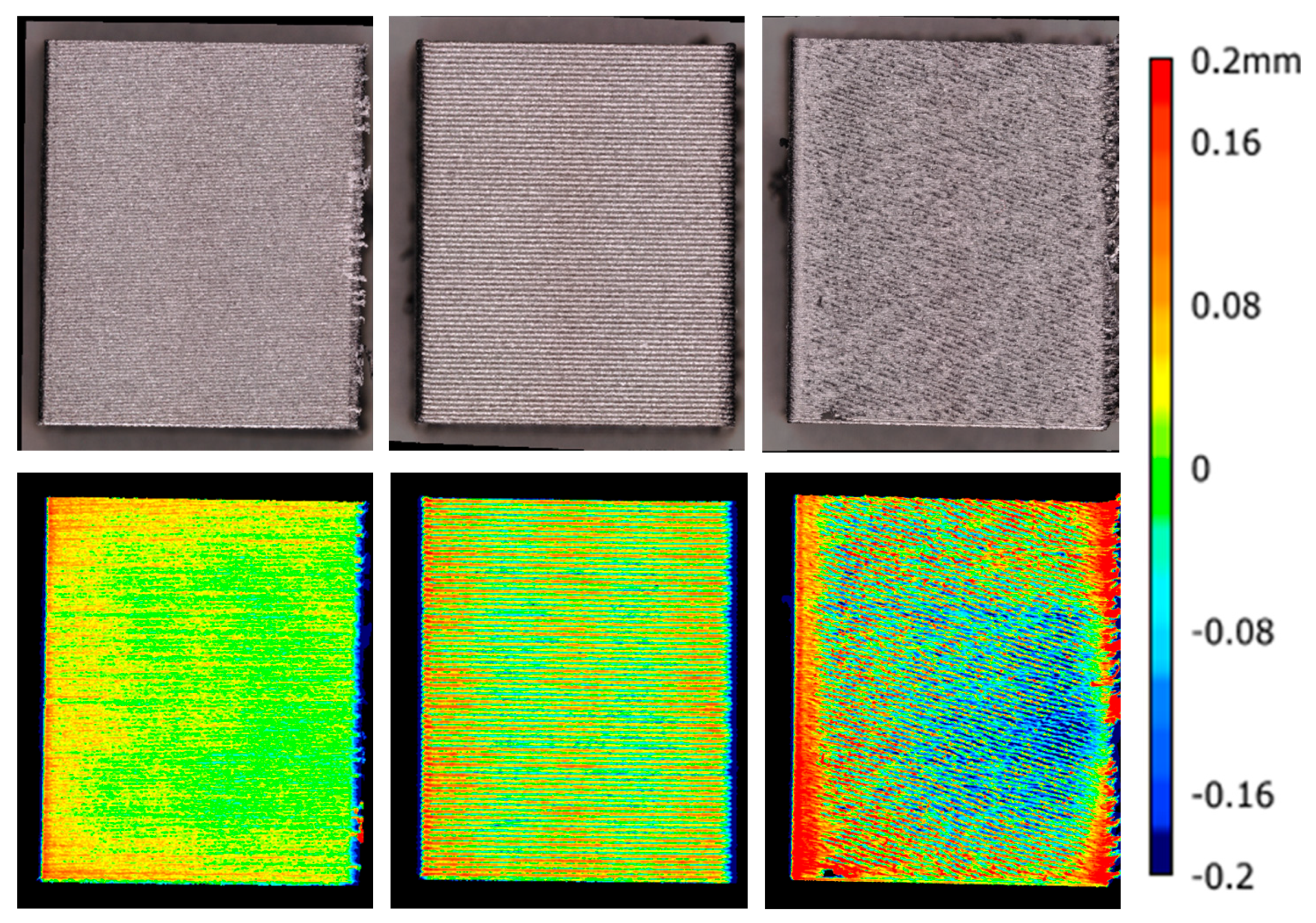
3.1. Extrusion Shape and Surface Quality by 3D Scans
3.1.1. Extrusion Line Shape and Width
3.1.2. Surface Roughness
- N11 with the lowest surface roughness, due to stable extrusion in flat extrusion lines and low layer height of 0.16 mm resulting in a homogeneous surface waviness.
- N14 with very stable but convex extrusion lines in large layers of 0.48 mm, showing a still homogeneous but larger waviness
- N23 with the roughest surface, even though the layer height of 0.12 mm is the lowest of the three examples, due to surface artefacts from the jagged extrusion line shape.
3.2. Fiber Alignment in XY
3.3. Tensile Tests on High and Low Fiber Aligned Specimens
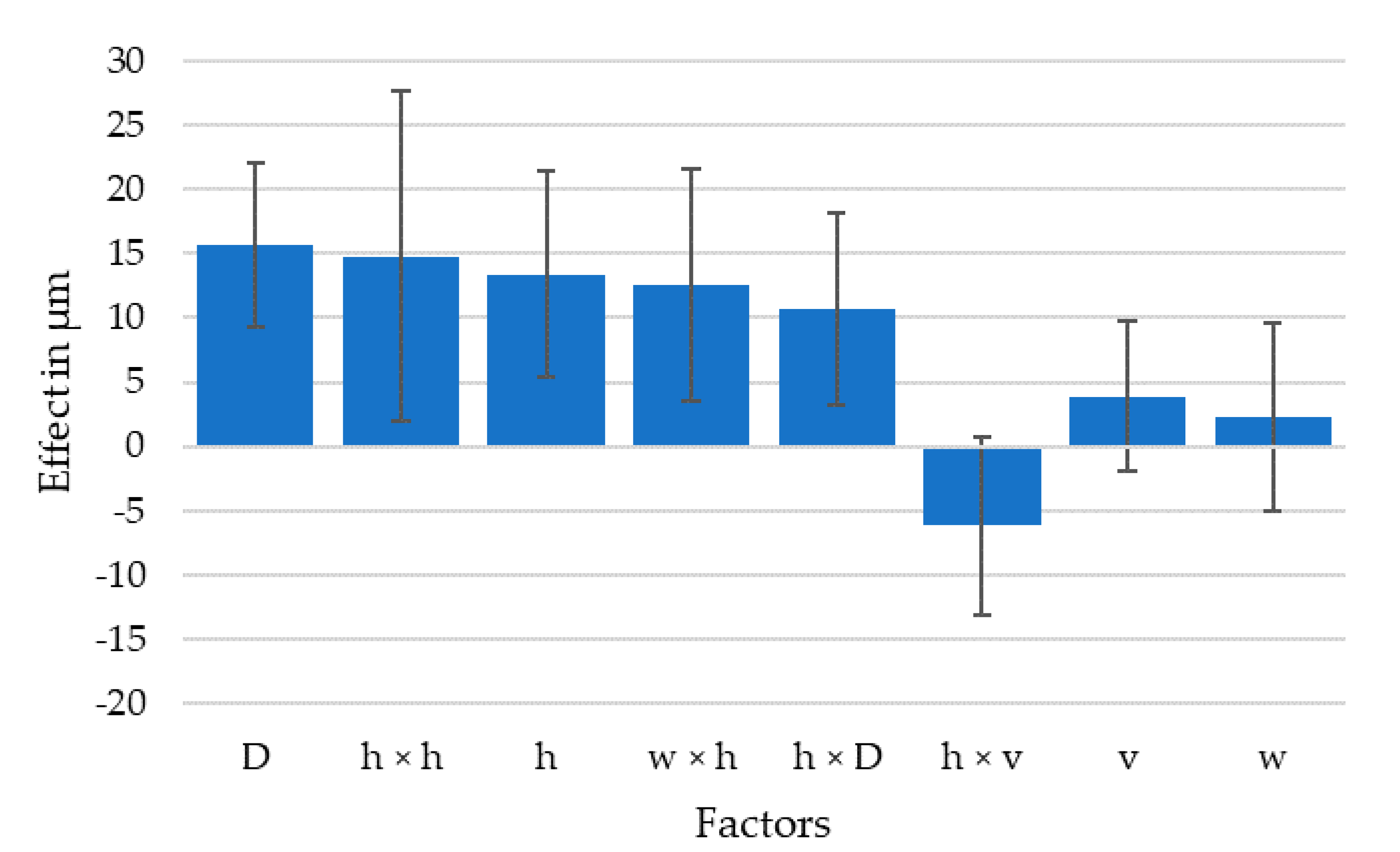
4. Discussion
- R2 shows the model fit and should be larger than 0.5 for a significant model.
- Q2 shows an estimate of prediction precision and should be larger than 0.5 for a good model.
- Model validity tests diverse model problems and should be larger than 0.25.
- Reproducibility is the variation of the replicates and should be larger than 0.5.
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Conflicts of Interest
Appendix A
| DoE Parameters | Measured Values | Printer Settings | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| Experiment Number | Line Width/Nozzle Diameter (%) | Layer Height/Nozzle Diameter (%) | Nozzle Diameter (mm) | Material Flow Velocity at Nozzle Tip (mm/s) | Standard Deviation of Fiber Orientation in XY (°) | Surface Roughness Sa in XZ (µm) | Real Width of Set Width (%) | Extrusion Line Shape | Print Speed (mm/min) | Line Width (mm) | Layer Height (mm) |
| N1 | 100 | 60 | 0.4 | 15 | 10.27 | 26.35 | 106.25 | Convex | 1500.0 | 0.4 | 0.24 |
| N2 | 200 | 60 | 0.4 | 15 | 22.13 | 29.59 | 102.71 | Convex | 750.0 | 0.8 | 0.24 |
| N3 | 100 | 20 | 0.4 | 25 | -- | -- | -- | -- | 7500.0 | 0.4 | 0.08 |
| N4 | 200 | 20 | 0.4 | 25 | 16.17 | 28.36 | 90.08 | Concave | 3750.0 | 0.8 | 0.08 |
| N5 | 200 | 60 | 0.4 | 25 | 19.93 | 34.60 | 100.38 | Convex | 1250.0 | 0.8 | 0.24 |
| N6 | 100 | 33 | 0.4 | 15 | 11.23 | 21.26 | 104.50 | Concave | 2727.3 | 0.4 | 0.132 |
| N7 | 100 | 47 | 0.4 | 25 | 8.27 | 24.57 | 101.92 | Flat | 3191.5 | 0.4 | 0.188 |
| N8 | 200 | 20 | 0.4 | 18.3 | 13.80 | 26.74 | 80.17 | Jagged | 2745.0 | 0.8 | 0.08 |
| N9 | 133 | 20 | 0.4 | 15 | 15.97 | 29.25 | 109.25 | Jagged | 3370.7 | 0.53 | 0.08 |
| N10 | 133 | 60 | 0.4 | 25 | 9.67 | 25.09 | 101.26 | Convex | 1872.6 | 0.53 | 0.24 |
| N11 | 150 | 40 | 0.4 | 20 | 12.40 | 18.06 | 82.50 | Flat | 2000.0 | 0.6 | 0.16 |
| N12 | 100 | 20 | 0.8 | 15 | 13.50 | 72.30 | 120.04 | Jagged | 4500.0 | 0.8 | 0.16 |
| N13 | 200 | 20 | 0.8 | 15 | 13.47 | 23.02 | 83.19 | Concave | 2250.0 | 1.6 | 0.16 |
| N14 | 100 | 60 | 0.8 | 25 | 9.13 | 42.71 | 104.25 | Convex | 2500.0 | 0.8 | 0.48 |
| N15 | 200 | 60 | 0.8 | 25 | 31.77 | 58.42 | 88.15 | Convex | 1250.0 | 1.6 | 0.48 |
| N16 | 100 | 47 | 0.8 | 15 | 11.73 | 36.77 | 103.92 | Flat | 1914.9 | 0.8 | 0.376 |
| N17 | 100 | 33 | 0.8 | 25 | 9.97 | 32.36 | 107.71 | Concave | 4545.5 | 0.8 | 0.264 |
| N18 | 200 | 20 | 0.8 | 21.7 | 16.23 | 26.79 | 88.40 | Concave | 3255.0 | 1.6 | 0.16 |
| N19 | 200 | 60 | 0.8 | 18.3 | 30.43 | 63.54 | 97.27 | Convex | 915.0 | 1.6 | 0.48 |
| N20 | 133 | 20 | 0.8 | 25 | 11.33 | 53.62 | 104.87 | Concave | 5617.9 | 1.06 | 0.16 |
| N21 | 167 | 20 | 0.8 | 25 | 21.53 | 25.30 | 89.33 | Flat | 4504.5 | 1.34 | 0.16 |
| N22 | 167 | 60 | 0.8 | 15 | 28.27 | 54.78 | 100.00 | Convex | 900.9 | 1.34 | 0.48 |
| N23 | 100 | 20 | 0.6 | 21.7 | 14.27 | 74.79 | 142.83 | Jagged | 6510.0 | 0.6 | 0.12 |
| N24 | 100 | 60 | 0.6 | 18.3 | 12.47 | 32.81 | 102.44 | Convex | 1830.0 | 0.6 | 0.36 |
| N25 | 200 | 47 | 0.6 | 15 | 18.50 | 36.05 | 96.47 | Concave | 957.4 | 1.2 | 0.282 |
| N26 | 200 | 33 | 0.6 | 25 | 18.43 | 35.90 | 95.61 | Flat | 2272.7 | 1.2 | 0.198 |
| N27 | 167 | 20 | 0.6 | 15 | 13.47 | 25.48 | 88.23 | Flat | 2689.2 | 1 | 0.12 |
| N28 | 150 | 40 | 0.6 | 20 | 15.17 | 28.23 | 97.78 | Concave | 2000.0 | 0.9 | 0.24 |
| N29 | 150 | 40 | 0.6 | 20 | 13.83 | 24.36 | 94.67 | Concave | 2000.0 | 0.9 | 0.24 |
| N30 | 150 | 40 | 0.6 | 20 | 18.87 | 26.43 | 98.78 | Concave | 2000.0 | 0.9 | 0.24 |
| Parameter | Value | Parameter | Value | Parameter | Value | Parameter | Value | Parameter | Value |
|---|---|---|---|---|---|---|---|---|---|
| Extrusion multiplier | 1.00 | Extrusion width | Manual | Retraction | Enabled | Retraction distance | 0.75 mm | Extra restart distance | 0 |
| Retraction Vertical lift | 0.2 mm | Retraction speed | 1800 mm/min | Coast at End | Disabled | Wipe nozzle | Disabled | Top solid layers | 0 |
| Bottom solid layers | 0 | Outline/perimeter shells | 1 for cubes; 0 for tensile Specimens | Outline Direction | Outside-In | Print islands sequentially without optimization | Enabled | Single outline corkscrew | Disabled |
| First layer height | 100% | First layer width | 100% | First layer speed | 40% | Start points | Optimize start point to for fastest printing | Additions | All disabled |
| Infill pattern | Rectilinear | ||||||||
| Interior fill percentage | 0 for cubes; 100% for tensile specimens | Outline overlap | 99% | Infill extrusion width | 100% | Minimum infill length | 5 mm | Combine infill every | 1 layer |
| Include solid diaphragm every | Disabled | Infill angle offsets | 0° for X specimens; 90° for Y specimens | Support | Disabled | Temperature extruder | 265 °C; single setpoint | Temperature heated bed | 75 °C; single setpoint |
| Fan speed | 0% at layer 1; 80% at layer 5 | Fan options and overrides | All disabled | Printing Speeds | According to DoE; Underspeeds at 100% | XY and Z Speeds (non-work moves) | 8000 mm/min | Speed overrides | Disabled |
| Bridging | Not Applicable | Horizontal size compensation | 0 | Filament Diameter | 1.75 mm | Only retract when crossing open spaces | Enabled | Force retraction between layers | enabled |
| Minimum travel for retraction | Disabled | Perform retraction during wipe movement | Disabled | Only wipe extruder for outer most perimeters | Enabled | Avoid crossing outline for travel movements | Enabled | Non-manifold Segments | Heal |
References
- Quanjin, M.; Rejab, M.; Idris, M.S.; Kumar, N.M.; Abdullah, M.H.; Reddy, G.R. Recent 3D and 4D intelligent printing technologies: A comparative review and future perspective. Procedia Comput. Sci. 2020, 167, 1210–1219. [Google Scholar] [CrossRef]
- Mathijsen, D. Bigger, stronger, faster… pushing the envelope of 3D printers to turn them into true production tools. Reinf. Plast. 2018, 62, 194–202. [Google Scholar] [CrossRef]
- Merklein, M.; Junker, D.; Schaub, A.; Neubauer, F. Hybrid additive manufacturing technologies—An analysis regarding potentials and applications. Phys. Procedia 2016, 83, 549–559. [Google Scholar] [CrossRef] [Green Version]
- Weller, C.; Kleer, R.; Piller, F.T. Economic implications of 3D printing: Market structure models in light of additive manufacturing revisited. Int. J. Prod. Econ. 2015, 164, 43–56. [Google Scholar] [CrossRef]
- Bourell, D.L. Perspectives on additive manufacturing. Annu. Rev. Mater. Res. 2016, 46, 1–18. [Google Scholar] [CrossRef]
- Thompson, M.K.; Moroni, G.; Vaneker, T.; Fadel, G.; Campbell, R.I.; Gibson, I.; Bernard, A.; Schulz, J.; Graf, P.; Ahuja, B.; et al. Design for additive manufacturing. Trends, opportunities, considerations, and constraints. CIRP Ann. 2016, 65, 737–760. [Google Scholar] [CrossRef] [Green Version]
- Oettmeier, K.; Hofmann, E. Additive manufacturing technology adoption: An empirical analysis of general and supply chain-related determinants. J. Bus. Econ. 2016, 87, 97–124. [Google Scholar] [CrossRef]
- Baumers, M.; Dickens, P.; Tuck, C.; Hague, R. The cost of additive manufacturing. Machine productivity, economies of scale and technology-push. Technol. Forecast. Soc. Chang. 2016, 102, 193–201. [Google Scholar] [CrossRef]
- Gao, W.; Zhang, Y.; Ramanujan, D.; Ramani, K.; Chen, Y.; Williams, C.B.; Wang, C.C.; Shin, Y.C.; Zhang, S.; Zavattieri, P.D. The status, challenges, and future of additive manufacturing in engineering. Comput. Des. 2015, 69, 65–89. [Google Scholar] [CrossRef]
- Zawadzki, P.; Żywicki, K. Smart product design and production control for effective mass customization in the industry 4.0 Concept. Manag. Prod. Eng. Rev. 2016, 7, 105–112. [Google Scholar] [CrossRef]
- Wulle, F.; Coupek, D.; Schäffner, F.; Verl, A.; Oberhofer, F.; Maier, T. Workpiece and machine design in additive manufacturing for multi-axis fused deposition modeling. Procedia CIRP 2017, 60, 229–234. [Google Scholar] [CrossRef]
- Wimpenny, D.I.; Pandey, P.M.; Kumar, L.J. Advances in 3D Printing & Additive Manufacturing Technologies; Springer: Singapore, 2017. [Google Scholar]
- Adamczak, S.; Bochnia, J.; Kaczmarska, B. An analysis of tensile test results to assess the innovation risk for an additive manufacturing technology. Metrol. Meas. Syst. 2015, 22, 127–138. [Google Scholar] [CrossRef]
- Hofstätter, T.; Pedersen, D.; Tosello, G.; Hansen, H. Challenges and opportunities of fibre-reinforced polymers in additive manufacturing with focus on industrial applications. In Proceedings of the Joint Special Interest Group Meeting Between Euspen and ASPE: Dimensional Accuracy and Surface Finish in Additive Manufacturing, Leuven, Belgium, 10–12 October 2017; pp. 10–11. [Google Scholar]
- Oropallo, W.; Piegl, L.A. Ten challenges in 3D printing. Eng. Comput. 2016, 32, 135–148. [Google Scholar] [CrossRef]
- Elser, A.; Königs, M.; Verl, A.; Servos, M. On achieving accuracy and efficiency in additive manufacturing: Requirements on a hybrid CAM system. Procedia CIRP 2018, 72, 1512–1517. [Google Scholar] [CrossRef]
- Turner, B.N.; Gold, S.A. A review of melt extrusion additive manufacturing processes. II. Materials, dimensional accuracy, and surface roughness. Rapid Prototyp. J. 2015, 21, 250–261. [Google Scholar] [CrossRef]
- Butler, C.A.; Mccullough, R.L.; Pitchumani, R.; Gillespie, J.W. An analysis of mechanisms governing fusion bonding of thermoplastic composites. J. Thermoplast. Compos. Mater. 1998, 11, 338–363. [Google Scholar] [CrossRef]
- Coogan, T.J.; Kazmer, D.O. Prediction of interlayer strength in material extrusion additive manufacturing. Addit. Manuf. 2020, 35, 101368. [Google Scholar] [CrossRef]
- Consul, P.; Chaplin, A.; Tagscherer, N.; Zaremba, S.; Drechsler, K. Interlaminar strength in large-scale additive manufacturing of slow crystallizing polyaryletherketone carbon composites. Polym. Int. 2020, 70, 1099–1108. [Google Scholar] [CrossRef]
- Liaw, C.-Y.; Tolbert, J.W.; Chow, L.W.; Guvendiren, M. Interlayer bonding strength of 3D printed PEEK specimens. Soft Matter 2021, 17, 4775–4789. [Google Scholar] [CrossRef]
- Bartolai, J.; Simpson, T.W.; Xie, R. Predicting strength of additively manufactured thermoplastic polymer parts produced using material extrusion. Rapid Prototyp. J. 2018, 24, 321–332. [Google Scholar] [CrossRef]
- Ahn, S.-H.; Montero, M.; Odell, D.; Roundy, S.; Wright, P.K. Anisotropic material properties of fused deposition modeling ABS. Rapid Prototyp. J. 2002, 8, 248–257. [Google Scholar] [CrossRef] [Green Version]
- Spoerk, M.; Savandaiah, C.; Arbeiter, F.; Traxler, G.; Cardon, L.; Holzer, C.; Sapkota, J. Anisotropic properties of oriented short carbon fibre filled polypropylene parts fabricated by extrusion-based additive manufacturing. Compos. Part A Appl. Sci. Manuf. 2018, 113, 95–104. [Google Scholar] [CrossRef]
- Wahab, M.A.; Karim, M.R.; Aijaz, M.O.; Salahuddin, B.; Aziz, S.; Sina, A.A.I. A study on the interfacial compatibility, microstructure and physico-chemical properties of polyimide/organically modified silica nanocomposite membrane. Polymers 2021, 13, 1328. [Google Scholar] [CrossRef]
- Ferdous, W.; Ngo, T.D.; Nguyen, K.T.; Ghazlan, A.; Mendis, P.; Manalo, A. Effect of fire-retardant ceram powder on the properties of phenolic-based GFRP composites. Compos. Part B Eng. 2018, 155, 414–424. [Google Scholar] [CrossRef]
- Stewart, R. Carbon fibre composites poised for dramatic growth. Reinf. Plast. 2009, 53, 16–21. [Google Scholar] [CrossRef]
- Ye, L.; Lu, Y.; Su, Z.; Meng, G. Functionalized composite structures for new generation airframes: A review. Compos. Sci. Technol. 2005, 65, 1436–1446. [Google Scholar] [CrossRef]
- Mohammed, A.A.; Manalo, A.C.; Ferdous, W.; Zhuge, Y.; Vijay, P.V.; Alkinani, A.Q.; Fam, A. State-of-the-art of prefabricated FRP composite jackets for structural repair. Eng. Sci. Technol. Int. J. 2020, 23, 1244–1258. [Google Scholar] [CrossRef]
- Ferdous, W.; Manalo, A.; AlAjarmeh, O.; Mohammed, A.A.; Salih, C.; Yu, P.; Khotbehsara, M.M.; Schubel, P. Static behaviour of glass fibre reinforced novel composite sleepers for mainline railway track. Eng. Struct. 2021, 229, 111627. [Google Scholar] [CrossRef]
- Adam, T.; Liao, G.; Petersen, J.; Geier, S.; Finke, B.; Wierach, P.; Kwade, A.; Wiedemann, M. multifunctional composites for future energy storage in aerospace structures. Energies 2018, 11, 335. [Google Scholar] [CrossRef] [Green Version]
- Chen, C.-H.; Cheng, C.-H. Effective elastic moduli of misoriented short-fiber composites. Int. J. Solids Struct. 1996, 33, 2519–2539. [Google Scholar] [CrossRef]
- Rezaei, F.; Yunus, R.; Ibrahim, N.A. Effect of fiber length on thermomechanical properties of short carbon fiber reinforced polypropylene composites. Mater. Des. 2009, 30, 260–263. [Google Scholar] [CrossRef]
- Tucker, C.L., III; Liang, E. Stiffness predictions for unidirectional short-fiber composites: Review and evaluation. Compos. Sci. Technol. 1999, 59, 655–671. [Google Scholar] [CrossRef]
- Unterweger, C.; Duchoslav, J.; Stifter, D.; Fürst, C. Characterization of carbon fiber surfaces and their impact on the mechanical properties of short carbon fiber reinforced polypropylene composites. Compos. Sci. Technol. 2015, 108, 41–47. [Google Scholar] [CrossRef]
- Vincent, M.; Giroud, T.; Clarke, A.; Eberhardt, C. Description and modeling of fiber orientation in injection molding of fiber reinforced thermoplastics. Polymer 2005, 46, 6719–6725. [Google Scholar] [CrossRef]
- Tseng, H.-C.; Su, T.-H. Coupled flow and fiber orientation analysis for 3D injection molding simulations of fiber composites. In Proceedings of the AIP Conference, Kelantan, Malaysia, 18–19 August 2018; AIP Publishing: Melville, NY, USA, 2019; p. 030021. [Google Scholar] [CrossRef]
- Tseng, H.-C.; Chang, R.-Y.; Hsu, C.-H. Improved fiber orientation predictions for injection molded fiber composites. Compos. Part A Appl. Sci. Manuf. 2017, 99, 65–75. [Google Scholar] [CrossRef]
- Tseng, H.-C.; Chang, R.-Y.; Hsu, C.-H. The use of shear-rate-dependent parameters to improve fiber orientation predictions for injection molded fiber composites. Compos. Part A Appl. Sci. Manuf. 2018, 104, 81–88. [Google Scholar] [CrossRef]
- Kim, E.G.; Park, J.K.; Jo, S.H. A study on fiber orientation during the injection molding of fiber-reinforced polymeric composites. J. Mater. Process. Technol. 2001, 111, 225–232. [Google Scholar] [CrossRef]
- Wang, Z.; Smith, D.E. Finite element modelling of fully-coupled flow/fiber-orientation effects in polymer composite deposition additive manufacturing nozzle-extrudate flow. Compos. Part B Eng. 2021, 219, 108811. [Google Scholar] [CrossRef]
- Das, A.; Gilmer, E.L.; Biria, S.; Bortner, M.J. Importance of polymer rheology on material extrusion additive manufacturing: Correlating process physics to print properties. ACS Appl. Polym. Mater. 2021, 3, 1218–1249. [Google Scholar] [CrossRef]
- Mackay, M.E. The importance of rheological behavior in the additive manufacturing technique material extrusion. J. Rheol. 2018, 62, 1549–1561. [Google Scholar] [CrossRef]
- Heller, B.P.; Smith, D.E.; Jack, D.A. Effects of extrudate swell and nozzle geometry on fiber orientation in Fused Filament Fabrication nozzle flow. Addit. Manuf. 2016, 12, 252–264. [Google Scholar] [CrossRef]
- Heller, B.P.; Smith, D.E.; Jack, D.A. Planar deposition flow modeling of fiber filled composites in large area additive manufacturing. Addit. Manuf. 2019, 25, 227–238. [Google Scholar] [CrossRef]
- Wang, Z.; Smith, D.E. A Fully coupled simulation of planar deposition flow and fiber orientation in polymer composites additive manufacturing. Materials 2021, 14, 2596. [Google Scholar] [CrossRef]
- Shafighfard, T.; Cender, T.A.; Demir, E. Additive manufacturing of compliance optimized variable stiffness composites through short fiber alignment along curvilinear paths. Addit. Manuf. 2021, 37, 101728. [Google Scholar] [CrossRef]
- Mulholland, T.; Goris, S.; Boxleitner, J.; Osswald, T.; Rudolph, N. Process-induced fiber orientation in fused filament fabrication. J. Compos. Sci. 2018, 2, 45. [Google Scholar] [CrossRef] [Green Version]
- Matschinski, A.; Ziegler, P.; Abstreiter, T.; Wolf, T.; Drechsler, K. Fiber formation of printed carbon fiber/poly (ether ether ketone) with different nozzle shapes. Polym. Int. 2021, 70, 1109–1117. [Google Scholar] [CrossRef]
- Armstrong, C.D.; Todd, N.; Alsharhan, A.T.; Bigio, D.I.; Sochol, R.D. A 3D printed morphing nozzle to control fiber orientation during composite additive manufacturing. Adv. Mater. Technol. 2021, 6, 2000829. [Google Scholar] [CrossRef]
- Guell, D.; Bénard, A. Flow-induced alignment in composite materials: Current applications and future prospects. In Flow-Induced Alignment in Composite Materials; Guell, D.C., Papathanasiou, T.D., Eds.; Woodhead Publishing Ltd.: Cambridge, UK, 1997; pp. 1–42. [Google Scholar]
- Coogan, T.J.; Kazmer, D.O. Modeling of interlayer contact and contact pressure during fused filament fabrication. J. Rheol. 2019, 63, 655–672. [Google Scholar] [CrossRef]
- Ehrenstein, G.W. Faserverbund-Kunststoffe. Werkstoffe—Verarbeitung—Eigenschaften, 2nd ed.; Hanser: München, Germany; Wien, Austria, 2019. [Google Scholar]


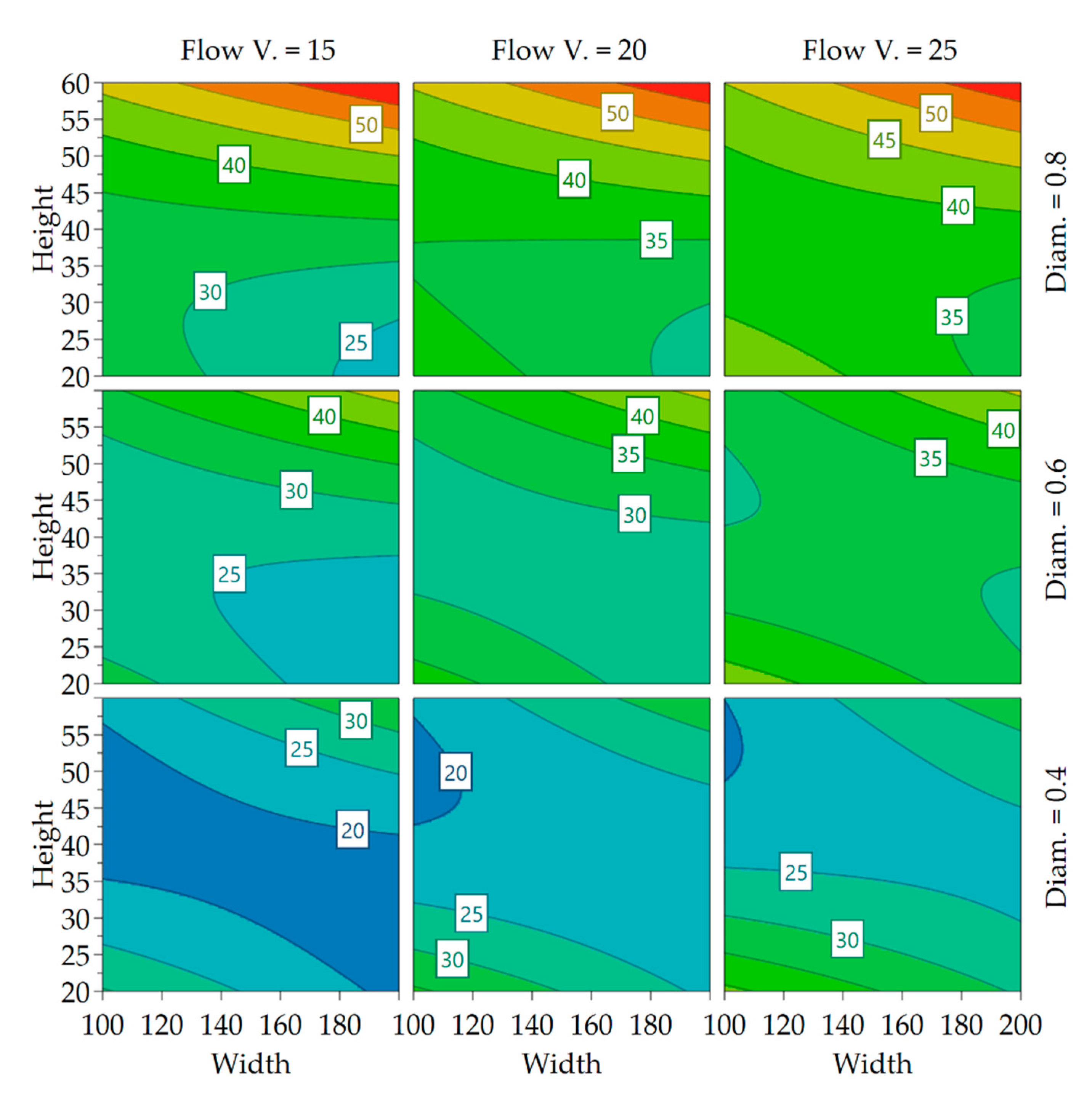
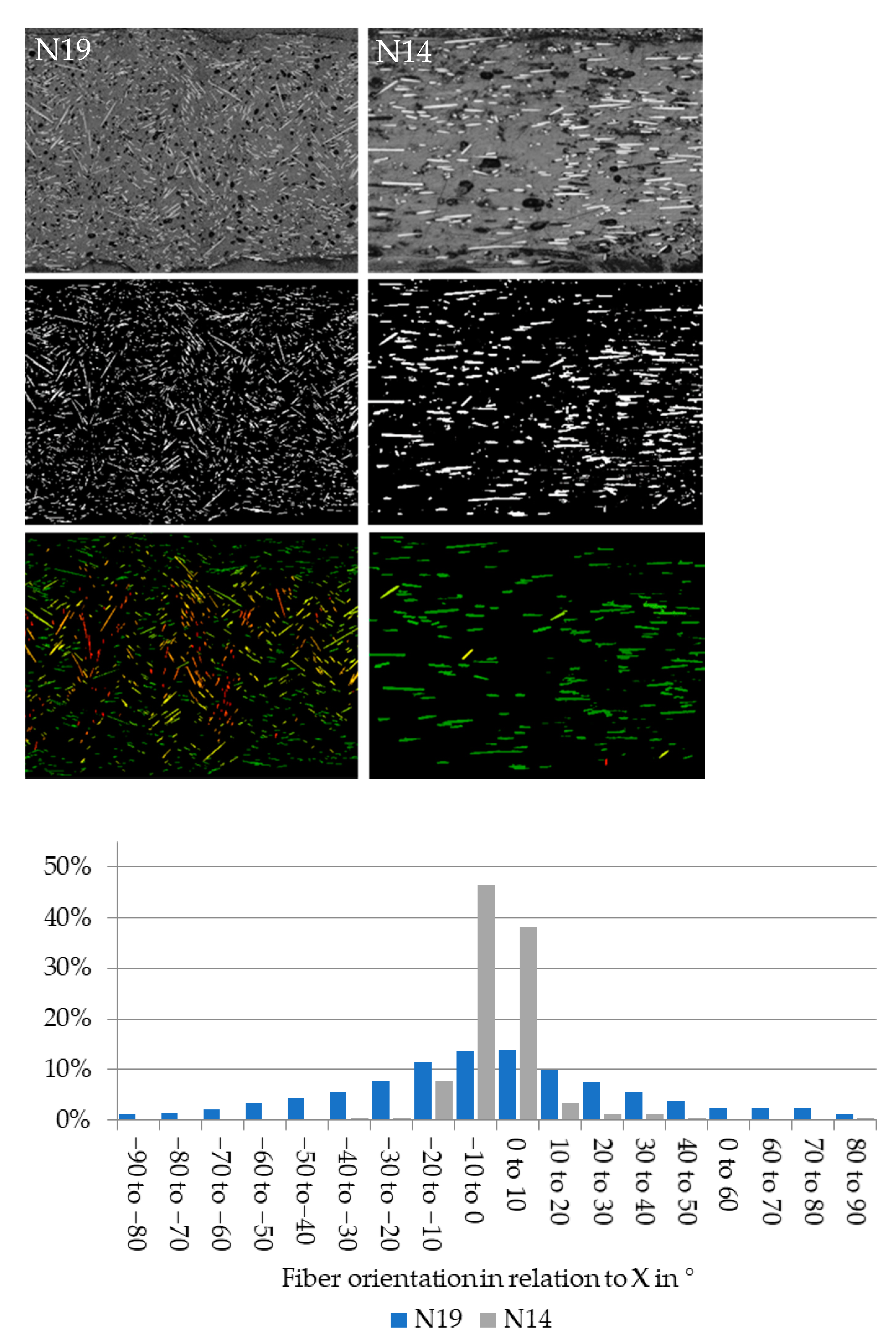
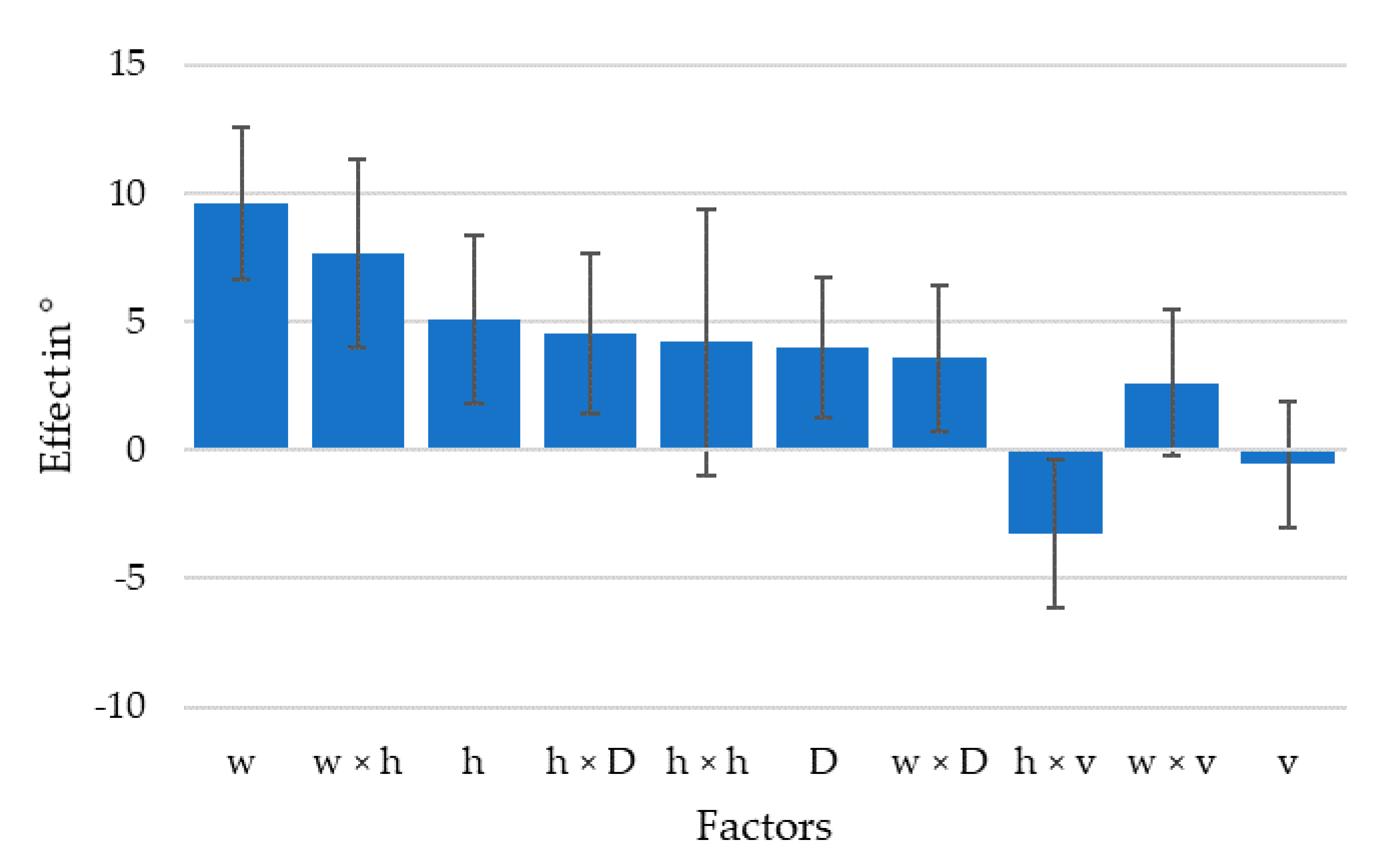



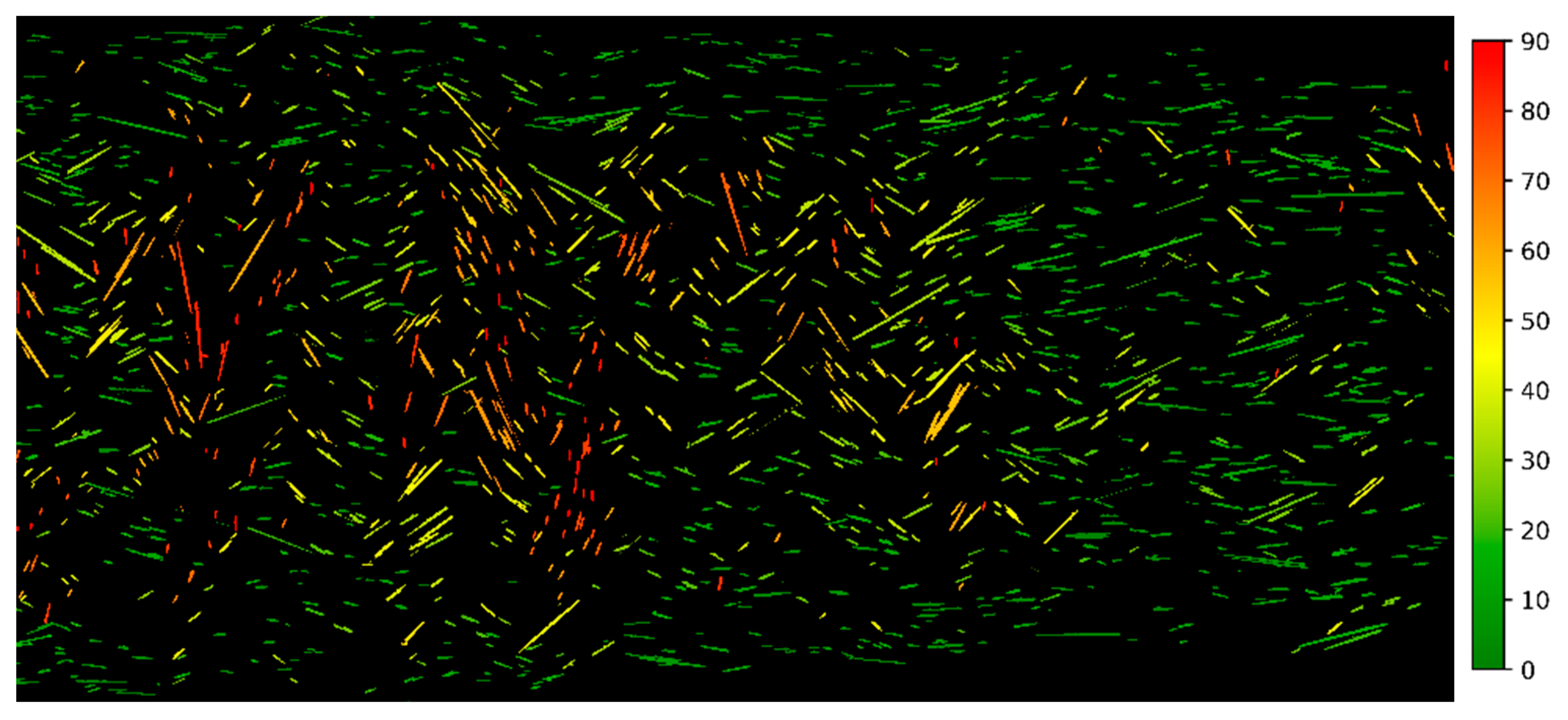
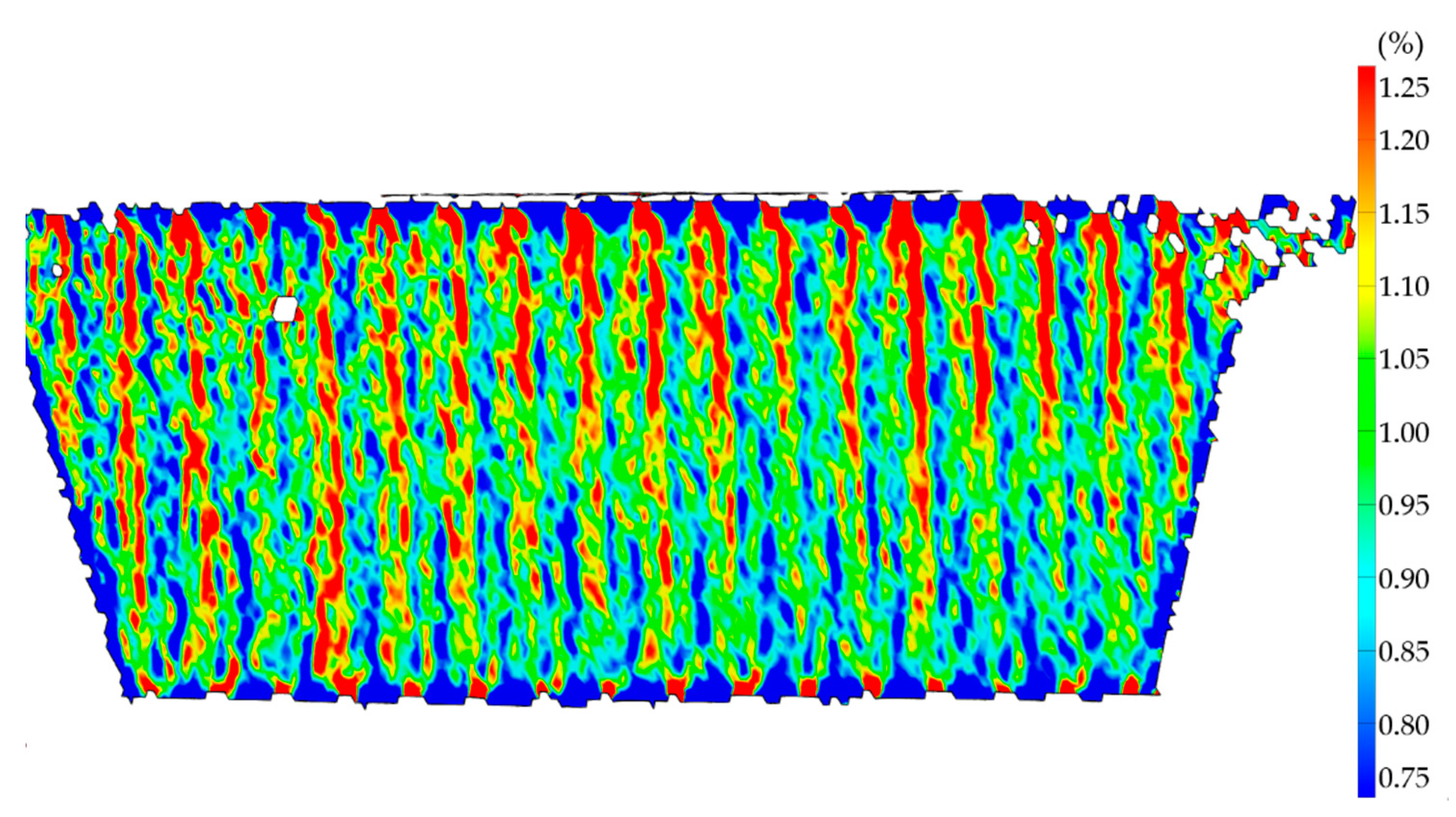
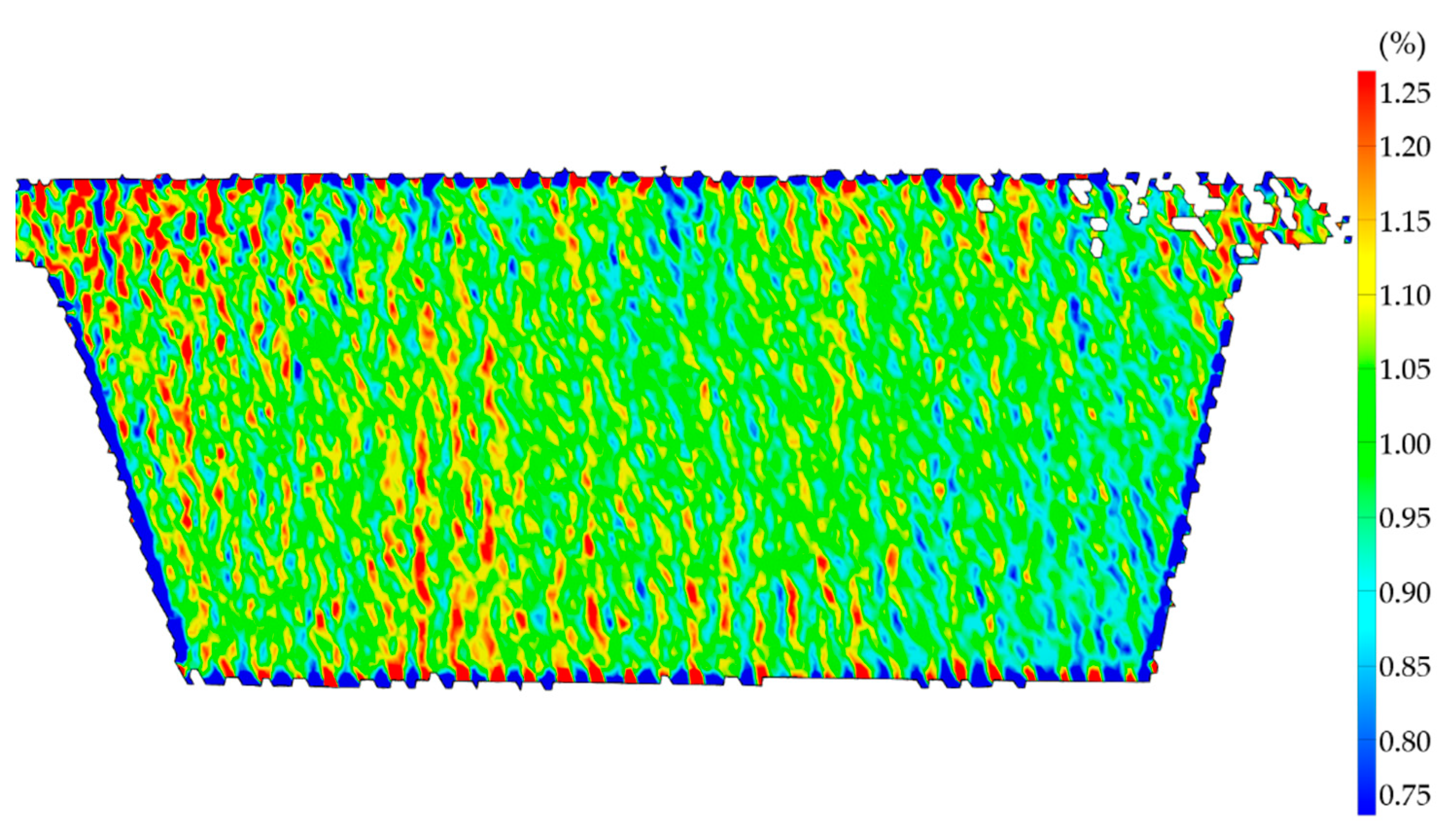
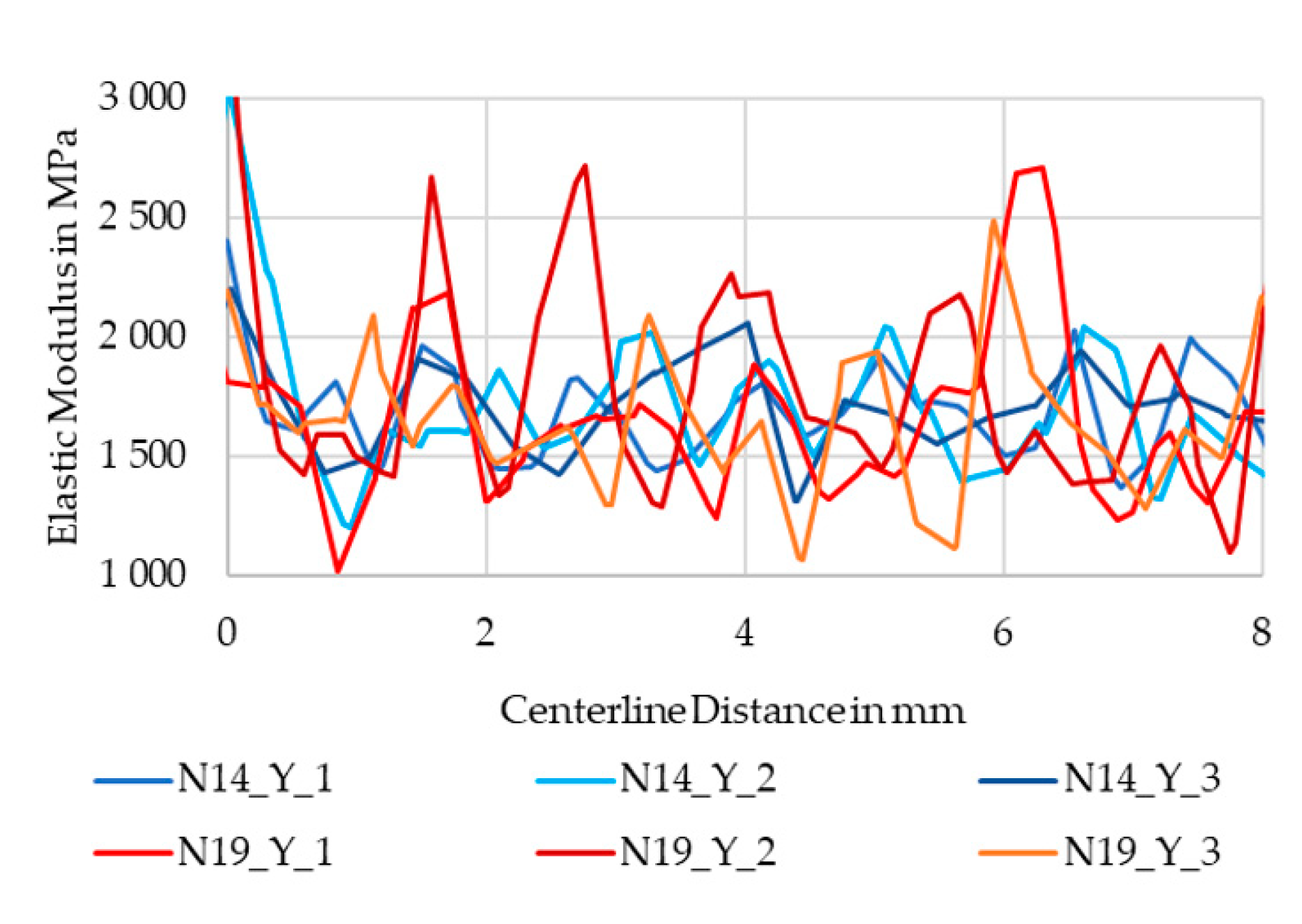
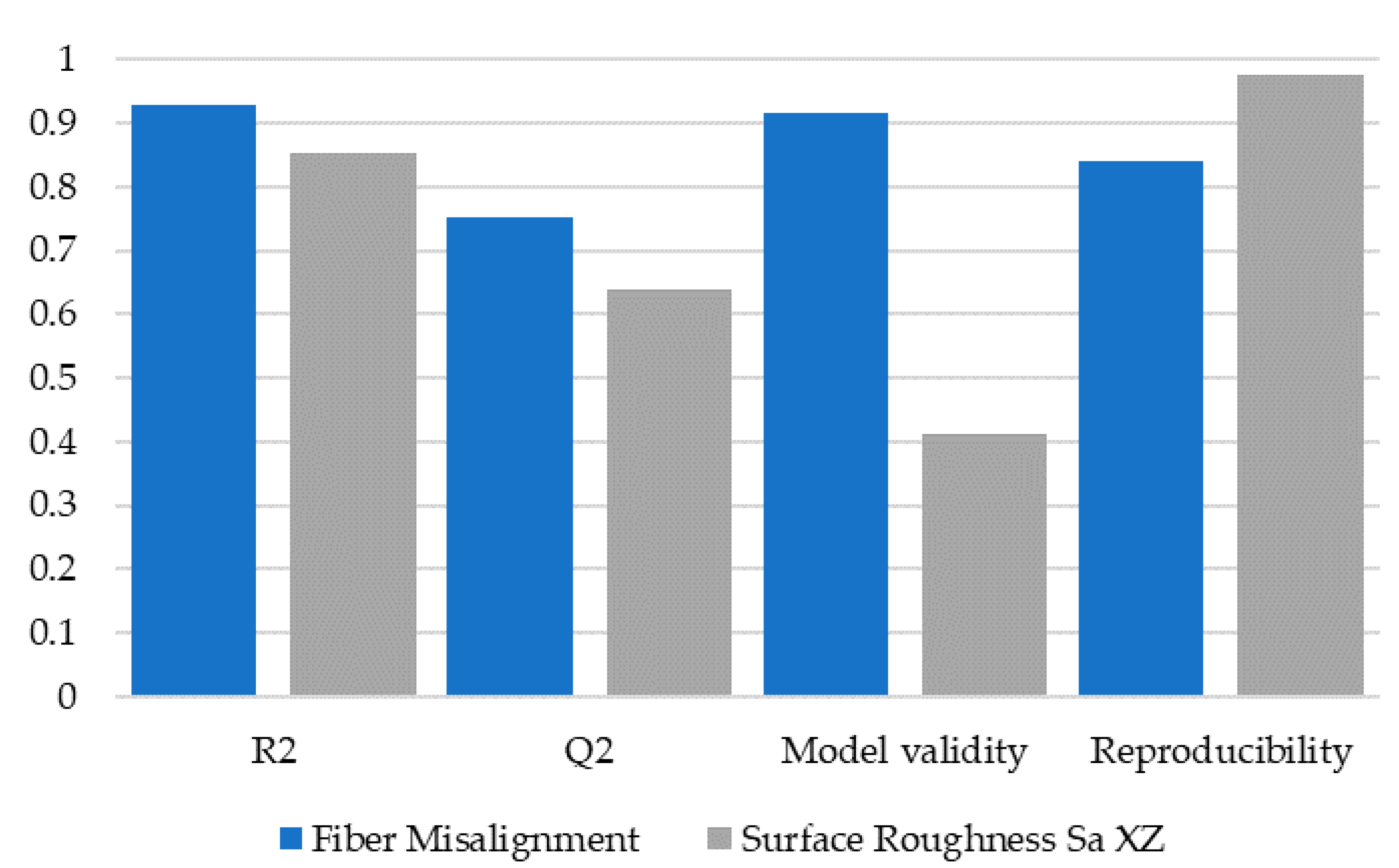
| Parameter | Lower Limit | Upper Limit | Unit |
|---|---|---|---|
| w: Extrusion width/nozzle diameter | 100 | 200 | % |
| h: Layer height/nozzle diameter | 20 | 60 | % |
| D: Nozzle diameter | 0.4 | 0.8 | mm |
| v: Flow velocity at nozzle outlet | 15 | 25 | mm/s |
| Strain in % | Strength in MPa | E-Modulus in MPa | Toughness in J/mm3 | |
|---|---|---|---|---|
| N14_X | 2.17 | 56.80 | 7328.39 | 953.85 |
| N19_X | 3.81 | 33.47 | 3375.88 | 1051.29 |
| N14_Y | 1.51 | 20.74 | 1759.86 | 180.68 |
| N19_Y | 1.95 | 19.58 | 1750.65 | 253.66 |
| Sample Group | Modulus Q25 (MPa) | Modulus Q75 (MPa) | Difference (MPa) |
|---|---|---|---|
| N14 | 1523.63 | 1813.78 | 290.15 |
| N19 | 1514.37 | 2045.30 | 530.93 |
| Experiment | Extrusion Line width (mm) | Extrusion Line Height (mm) | Flow Velocity (mm/s) | Nozzle Diameter (mm) | Fiber Misalignment (°) |
|---|---|---|---|---|---|
| N2 | 0.8 | 0.24 | 15 | 0.4 | 22.13 |
| N17 | 0.8 | 0.264 | 25 | 0.8 | 9.97 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Consul, P.; Beuerlein, K.-U.; Luzha, G.; Drechsler, K. Effect of Extrusion Parameters on Short Fiber Alignment in Fused Filament Fabrication. Polymers 2021, 13, 2443. https://doi.org/10.3390/polym13152443
Consul P, Beuerlein K-U, Luzha G, Drechsler K. Effect of Extrusion Parameters on Short Fiber Alignment in Fused Filament Fabrication. Polymers. 2021; 13(15):2443. https://doi.org/10.3390/polym13152443
Chicago/Turabian StyleConsul, Patrick, Kai-Uwe Beuerlein, Genc Luzha, and Klaus Drechsler. 2021. "Effect of Extrusion Parameters on Short Fiber Alignment in Fused Filament Fabrication" Polymers 13, no. 15: 2443. https://doi.org/10.3390/polym13152443
APA StyleConsul, P., Beuerlein, K.-U., Luzha, G., & Drechsler, K. (2021). Effect of Extrusion Parameters on Short Fiber Alignment in Fused Filament Fabrication. Polymers, 13(15), 2443. https://doi.org/10.3390/polym13152443







