Finite Element Method-Based Dynamic Response of Micropolar Polymers with Voids
Abstract
:1. Introduction
2. Notation, Formulas, and Methods
3. Kinematics, Kinetic Energy, Potential Energy, and Work
3.1. Kinematics
3.2. Kinetic Energy
3.3. Internal Energy
3.4. Work
3.5. Lagrangian
4. Euler–Lagrange Equations
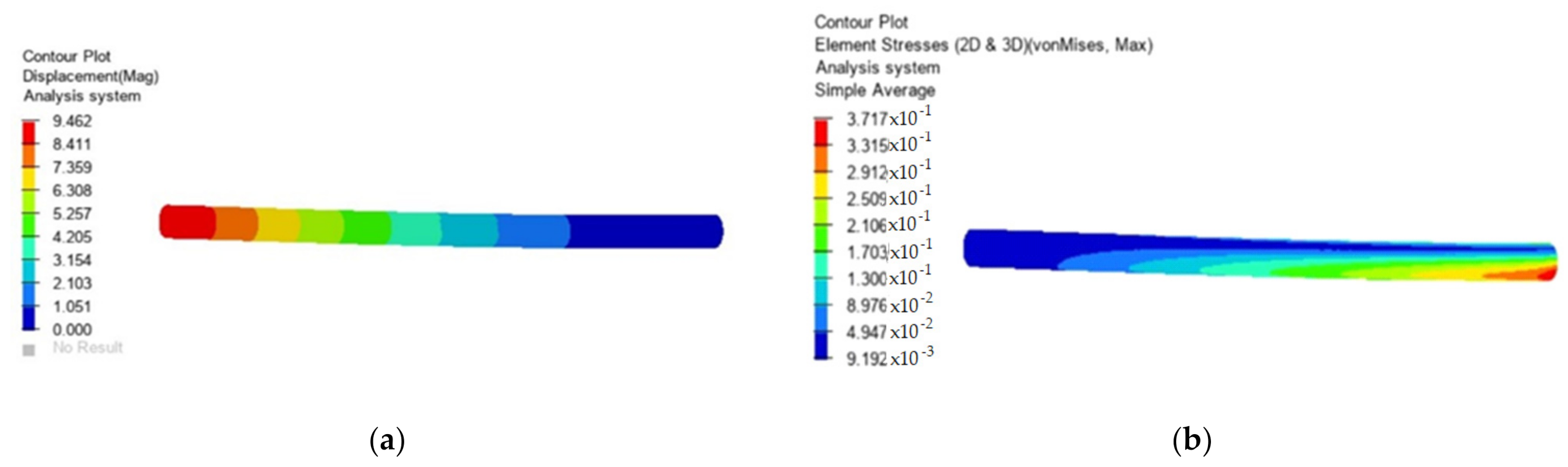
5. Conclusions and Discussion
- The main phase in approaching MBS systems with elastic elements made of void materials is the writing of equations of motion. In this stage, thorough knowledge of the elastic properties of the worn material and of the constitutive laws is necessary. Once these equations are obtained, the other steps to be followed, namely, the assembly of the equations of motion and their solution, will be calculated according to the classical procedures currently used in common FEM software. To obtain these equations, a considerable amount of effort can be required, their shape depending both on the properties of the studied material but also on the chosen shape functions.
- The method used in the paper is Lagrangian formalism, which is used in most articles that study this issue. The use of this method has the advantage of a homogeneous writing and allows the automation of intermediate stages and the use of previously obtained results by other authors for parts of these equations. The major advantage of using this formalism is the frequent previous use and the existence of a rich experience in this use. The Lagrange method has a major advantage, namely, the formal use of well-known sizes in mechanics as kinetic energy, potential energy, and mechanical work, notions with which we are very accustomed and with which we operate easily. The use of other methods, although possible, has rarely been used by researchers [27,28,29,30,31,32,33,34,35,36,37]. Consideration concerning the future development of the method can be found in [55,56,57,58,59,60,61,62,63]. Another approach would be to use the Gibbs–Appell method, which seems to have the advantage of obtaining savings during modeling and calculation itself. However, this method has the disadvantage that researchers have to use a notion they are unfamiliar with, which is the energy of accelerations. A method consisting of applying Maggi’s equations can become useful when analyzing multibody systems in which the liaisons between the elements are nonholonomic. Hamiltonian formalism, another way of studying, can be advantageous from the point of view of a numerical calculation, finally obtaining a system of first-order differential equations. However, this method also has the disadvantage of an increased complexity of intermediate calculations. The last method we mentioned, that of Kane’s equations, is equivalent to Maggi’s formalism.
- In manufacturing, if a resin that has a high viscosity is used, voids in the material will most likely appear. This is because it is difficult for a resin with a high viscosity to penetrate and clog all of the empty spaces between the fibers. If the fiber concentration is very high, removing these voids is very difficult, if not impossible. It is obvious that often, in practical applications, we cannot remove them and we must take them into account when making calculations, as their presence can significantly change the mechanical properties.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Gladysz, G.M.; Chawla, K.K. Intrinsic Voids in Polymers. In Voids in Materials: From Unavoidable Defects to Designed Cellular Materials; Elsevier: Amsterdam, The Netherlands; Oxford/Cambridge, UK, 2015; pp. 37–48. [Google Scholar]
- Sung, B.J.; Yethiraj, A. Structure of void space in polymer solutions. Phys. Rev. E 2010, 81, 237. [Google Scholar] [CrossRef]
- Liang, J.; Chen, R.; Zhang, J.F. Geometry and statistics of voids in lattice polymers. Biophys. J. 2002, 82, 471A. [Google Scholar]
- Day, D.; Gu, M. Formation of voids in a doped polymethylmethacrylate polymer. Appl. Phys. Lett. 2009, 80, 2404–2406. [Google Scholar] [CrossRef]
- Nie, L.; Lee, K.; Liao, G.L. Void control in adhesive bonding using thermosetting polymer. Sens. Actuators A-Phys. 2011, 167, 398–405. [Google Scholar] [CrossRef]
- Lee, J.W.; Wang, C.S.; Price, G.E. Void formation in coagulated rigid-rod polymer thin-films. Polymer 1995, 36, 955–958. [Google Scholar] [CrossRef]
- Gerhard-Multhaupt, R. Voided polymer electrets-New materials, new challenges, new chances. In Proceedings of the 11th International Symposium on Electrets (ISE 11), Glen Waverley, Melbourne, Australia, 1–3 October 2002; pp. 36–45. [Google Scholar]
- Murray, B.R.; Leen, S.B.; Bradaigh, C.M.O. Void distributions and permeability prediction for rotationally moulded polymers. Proc. Inst. Mech. Eng. Part L-J. Mater. -Des. Appl. 2015, 229, 403–418. [Google Scholar] [CrossRef] [Green Version]
- Shen, T.F. Void damage model and service life prediction for solid high polymer. Chin. Sci. Bull. 2001, 46, 1842–1845. [Google Scholar] [CrossRef]
- Summerscales, J.; Fry, S.A. Poisson ratio in fiber-reinforced polymer composites with a high void content. J. Mater. Sci. Lett. 1994, 13, 912–914. [Google Scholar] [CrossRef]
- Wu, Y.N.; Shivpuri, R.; Lee, L.J. Effect of macro and micro voids on elastic properties of polymer composites. J. Reinf. Plast. Compos. 1998, 17, 1391–1402. [Google Scholar] [CrossRef]
- Hocine, N.A.; Hamdi, A.; Zairi, F. Experimental and finite element investigation of void nucleation in rubber-like materials. Int. J. Solids Struct. 2011, 48, 1248–1254. [Google Scholar] [CrossRef]
- Soria, M.H.A.; Aramaki, R.T.; Viviani, E. Experimental Determination of Void Size Curves. In Proceedings of the 1st International Conference on Geo-Filters: Filters in Geotechnical and Hydraulic Engineering, Karlsruhe, Germany, 20–22 October 1992; pp. 43–48. [Google Scholar]
- Sivaneshan, P.; Harishankar, S. Experimental Study on Voided Reinforced Concrete Beams with Polythene Balls. In Proceedings of the International Conference on Civil Engineering and Infrastructural Issues in Emerging Economies (ICCIEE), Thanjavur, India, 17–18 March 2017; p. 80. [Google Scholar]
- He, H.Y. Microscopic Mechanism Analysis and Experimental Study of Void beneath Slab. International Conference on Automation, Communication, Architectonics and Materials. Adv. Res. Autom. Commun. Architecton. Mater. 2011, 225, 692–696. [Google Scholar]
- Wu, Y.T.; Liu, T.; Yang, K.J. Experimental study on detection of void in concrete pavement slab by FWD. In Proceedings of the 6th International Conference on Energy Materials and Enviroment Engineering, Tianjin, China, 24–26 April 2020; p. 508. [Google Scholar]
- Bao, J.Q.; Ji, Z.H.; Yang, Q. Approximation of the effective moduli of particulate composite with the fixed grid finite element method. Finite Elem. Anal. Des. 2014, 78, 1–7. [Google Scholar] [CrossRef]
- Park, H.; Lee, S.W. Cure simulation of thick composite structures using the finite element method. J. Compos. Mater. 2001, 35, 188–201. [Google Scholar] [CrossRef]
- Xiong, X.; Shen, S.Z.; Hua, L.; Liu, J.Z.; Li, X.; Wan, X.; Miao, M. Finite element models of natural fibers and their composites: A review. J. Reinf. Plast. Compos. 2018, 37, 617–635. [Google Scholar] [CrossRef]
- Hampson, P.R.; Moatarnedi, M. A review of composite structures subjected to dynamic loading. Int. J. Crashworthiness 2007, 12, 411–428. [Google Scholar] [CrossRef]
- Tan, P.; Tong, L.; Steven, G.P. Modelling for predicting the mechanical properties of textile composites-A review. Compos. PART A-Appl. Sci. Manuf. 1997, 28, 903–922. [Google Scholar] [CrossRef]
- Rong, B.; Rui, X.T.; Tao, L.; Wang, G.P. Theoretical modeling and numerical solution methods for flexible multibody system dynamics. Nonlinear Dyn. 2019, 98, 1519–1553. [Google Scholar] [CrossRef]
- Contreras, U.; Li, G.; Foster, C.D.; Shabana, A.A.; Jayakumar, P.; Letherwood, M.D. Soil Models and Vehicle System Dynamics. Appl. Mech. Rev. 2013, 65, 041001. [Google Scholar] [CrossRef]
- Nguyen, V.T.; Hwu, C. A Review on Modelling Polymer Composites via Anisotropic Viscoelasticity. J. Aeronaut. Asronautics Aviat. 2021, 53, 387–402. [Google Scholar] [CrossRef]
- Raju, K.; Tay, T.E.; Tan, V.B.C. A review of the FE2 method for composites. Multiscale Multidiscip. Modeling Exp. Des. 2021, 4, 1–24. [Google Scholar] [CrossRef]
- Sharma, A.; Bagha, A.K.; Shukla, D.K.; Bahl, S. Finite element model updating of metallic and composite structures-A state of the art review. AIMS Mater. Sci. 2021, 8, 390–415. [Google Scholar] [CrossRef]
- Jagadeesh, G.V.; Setti, S.G. A review on micromechanical methods for evaluation of mechanical behavior of particulate reinforced metal matrix composites. J. Mater. Sci. 2020, 55, 9848–9882. [Google Scholar] [CrossRef]
- Muzel, S.D.; Bonhin, E.P.; Guimaraes, N.M.; Guidi, E.S. Application of the Finite Element Method in the Analysis of Composite Materials: A Review. Polymers 2020, 12, 818. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Teodorescu-Draghicescu, H.; Stanciu, A.; Vlase, S.; Scutaru, L.; Calin, M.R.; Serbina, L. Finite Element Method Analysis of Some Fibre-Reinforced Composite Laminates. Optoelectron. Adv. Mater. Rapid Commun. 2011, 5, 782–785. [Google Scholar]
- Stanciu, A.; Teodorescu-Drǎghicescu, H.; Vlase, S.; Scutaru, M.L.; Cǎlin, M.R. Mechanical behavior of CSM450 and RT800 laminates subjected to four-point bend tests. Optoelectron. Adv. Mater. Rapid Commun. 2012, 6, 495–497. [Google Scholar]
- Niculiţă, C.; Vlase, S.; Bencze, A.; Mihălcică, M.; Calin, M.R.; Serbina, L. Optimum stacking in a multi-ply laminate used for the skin of adaptive wings. Optoelectron. Adv. Mater. Rapid Commun. 2011, 5, 1233–1236. [Google Scholar]
- Xiao, B.; Huang, Q.; Wang, Y.; Chen, H.; Chen, X.; Long, G. A fractal model for capillary flow through a single tortuous capillary with roughened surfaces in fibrous porous media. Fractals 2020, 29, 2150017. [Google Scholar] [CrossRef]
- Xiao, B.; Zhang, Y.; Jiang, G.; Liang, M.; Wang, Y.; Chen, X.; Long, G. A Fractal Model for Kozeny-Carman Constant and Dimensionless Permeability of Fibrous Porous Media with Roughened Surfaces. Fractals 2019, 27, 1950116. [Google Scholar] [CrossRef]
- Zangana, S.; Epaarachchi, J.; Ferdous, W.; Leng, J. A novel hybridised composite sandwich core with Glass, Kevlar and Zylon fibres—Investigation under low-velocity impact. Int. J. Impact Eng. 2020, 137, 103430. [Google Scholar] [CrossRef]
- Ferdous, W.; Manalo, A.; Peaurila, J.; Salih, C.; Reddy, K.R.; Yu, P.; Schubel, P.; Heyer, T. Testing and modelling the fatigue behaviour of GFRP composites—Effect of stress level, stress concentration and frequency. Eng. Sci. Technol. 2020, 23, 1223–1232. [Google Scholar] [CrossRef]
- Ferdous, W.; Manalo, A.; AlAjarmeh, O.; Mohammed, A.A.; Salih, C.; Yu, P.; Khotbehsara, M.M.; Schubel, P. Static behaviour of glass fibre reinforced novel composite sleepers for mainline railway track. Eng. Struct. 2021, 229, 111627. [Google Scholar] [CrossRef]
- Goodman, M.A.; Cowin, S.C. A continuum theory for granular materials. Arch. Ration. Mech. Anal. 1972, 44, 249–266. [Google Scholar] [CrossRef]
- Nunziato, J.W.; Cowin, S.C. Linear elastic materials with voids. J. Elast. 1983, 13, 125–147. [Google Scholar]
- Iesan, D. A theory of thermoelastic materials with voids. Acta. Mech. 1986, 60, 67–89. [Google Scholar] [CrossRef]
- Rusu, G. On existence and uniqueness in thermoelasticity of bodies with voids. Bull. Acad. Polon. Sci. 1987, 35, 339–346. [Google Scholar]
- Green, A.E.; Rivlin, R.S. Multipolar continuum mechanics. Arch. Ration. Mech. Anal. 1964, 17, 113–147. [Google Scholar] [CrossRef]
- Marin, M. On uniqueness in thermoelasticity of micropolar bodies. Rev. Roum. Math. Pures Appl. 1994, 6, 382–393. [Google Scholar]
- Yu, Y.L.; Ye, J.R.; Wang, Y.; Zhang, B.M.; Qi, G.C. A mesoscale ultrasonic attenuation finite element model of composites with random-distributed voids. Compos. Sci. 2013, 89, 44–51. [Google Scholar] [CrossRef]
- Rinivasulu, G.; Velmurugan, R.; Jayasankar, S. Influence of void microstructure on the effective elastic properties of discontinuous fiber-reinforced composites. J. Compos. Mater. 2015, 49, 2745–2755. [Google Scholar] [CrossRef]
- Wang, M.; Zhang, P.W.; Fei, Q.G.; Guo, F. Computational evaluation of the effects of void on the transverse tensile strengths of unidirectional composites considering thermal residual stress. Compos. Struct. 2019, 227, 111287. [Google Scholar] [CrossRef]
- Chang, X.; Ren, M.F.; Li, T.; Guo, X. Evaluation of mechanical behaviour of unidirectional fibre-reinforced composites considering the void morphology. J. Reinf. Platics Compos. 2017, 36, 1817–1828. [Google Scholar] [CrossRef]
- Dong, C.S. Effects of Process-Induced Voids on the Properties of Fibre Reinforced Composites. J. Mater. Sci. Technol. 2016, 32, 597–604. [Google Scholar] [CrossRef] [Green Version]
- Brinson, L.C.; Knauss, W.G. Finite Element Analysis of Multiphase Viscoelastic Solids. J. Appl. Mech. 1992, 59, 730–737. [Google Scholar] [CrossRef]
- Hahn, H.G. Methode der Finiten Elemente in der Festigkeitslehre; Akademische Verlagsgesellschaft: Franfurt am Main, Germany, 1975. [Google Scholar]
- Schaffer, B.G.; Adams, D.F. Nonlinear Viscoelastic Behavior of a Composite Material using a Finite Element Micromechanical Analysis; Dept. Report UWMW-DR-001-101-1; University of Wyoming: Laramie, WY, USA, 1980. [Google Scholar]
- Teodorescu-Draghicescu, H.; Vlase, S.; Stanciu, M.D.; Curtu, I.; Mihalcica, M. Advanced Pultruded Glass Fibers-Reinforced Isophtalic Polyester Resin. Mater. Plast. 2015, 52, 62–64. [Google Scholar]
- Abbas, I.; Marin, M. Analytical Solutions of a Two-Dimensional Generalized Thermoelastic Diffusions Problem Due to Laser Pulse. Iran. J. Sci. Technol. Trans. Mech. Eng. 2018, 42, 57–71. [Google Scholar] [CrossRef]
- Pietraszkiewicz, W.; Eremeyev, V.A. On vectorially parameterized natural strain measures of the non-linear Cosserat continuum. Int. J. Solids Struct. 2009, 46, 2477–2480. [Google Scholar] [CrossRef] [Green Version]
- Zienkiewicz, O.C. The Finite Element Method: Its Basis and Fundamentals, 6th ed.; Elsevier Butterworth-Heinemann: Oxford, UK, 2005. [Google Scholar]
- He, J.H. Lagrange crisis and generalized variational principle for 3D unsteady flow. Int. J. Numer. Methods Heat Fluid Flow 2020, 30, 1189–1196. [Google Scholar] [CrossRef]
- He, J.H.; Qie, N.; He, C.H.; Saeed, T. On a strong minimum condition of a fractal variational principle. Appl. Math. Lett. 2021, 119, 107199. [Google Scholar] [CrossRef]
- He, J.H.; Anjum, N.; Skrzypacz, P.S. A Variational Principle for a Nonlinear Oscillator Arising in the Microelectromechanical System. J. Appl. Comput. Mech. 2021, 7, 78–83. [Google Scholar]
- Nadeem, M.; He, J.H. He-Laplace variational iteration method for solving the nonlinear equations arising in chemical kinetics and population dynamics. J. Math. Chem. 2021, 59, 1234–1245. [Google Scholar] [CrossRef]
- He, J.H.; Hou, W.F.; Mohammad-Sedighi, H. Hamiltonian-Based Frequency-Amplitude Formulation for Nonlinear Oscillators. Facta Univ.-Ser. Mech. Eng. 2021, 19, 199–208. [Google Scholar]
- He, J.H. On the Fractal Variational Principle for the Telegraph Equation. Fractals 2021, 29, 2150022. [Google Scholar] [CrossRef]
- Zang, L.; Bhatti, M.M.; Marin, M.; Mekheimer, K.S. Entropy analysis on the blood flow through anisotropically tapered arteries filled with magnetic zinc-oxide (ZnO) nanoparticles. Entropy 2020, 22, 1070. [Google Scholar] [CrossRef] [PubMed]
- Modrea, A.; Vlase, S.; Calin, M.R.; Peterlicean, A. The influence of dimensional and structural shifts of the elastic constant values in cylinder fiber composites. J. Optoelectron. Adv. Mater. 2013, 15, 278–283. [Google Scholar]
- Vlase, S.; Marin, M.; Scutaru, M.L.; Munteanu, R. Coupled transverse and torsional vibrations in a mechanical system with two identical beams. AIP Adv. 2017, 7, 065301. [Google Scholar] [CrossRef]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Vlase, S.; Marin, M. Finite Element Method-Based Dynamic Response of Micropolar Polymers with Voids. Polymers 2021, 13, 3727. https://doi.org/10.3390/polym13213727
Vlase S, Marin M. Finite Element Method-Based Dynamic Response of Micropolar Polymers with Voids. Polymers. 2021; 13(21):3727. https://doi.org/10.3390/polym13213727
Chicago/Turabian StyleVlase, Sorin, and Marin Marin. 2021. "Finite Element Method-Based Dynamic Response of Micropolar Polymers with Voids" Polymers 13, no. 21: 3727. https://doi.org/10.3390/polym13213727
APA StyleVlase, S., & Marin, M. (2021). Finite Element Method-Based Dynamic Response of Micropolar Polymers with Voids. Polymers, 13(21), 3727. https://doi.org/10.3390/polym13213727






