Theoretical Investigation of the Influence of Wavelength on the Bandwidth in Multimode W-Type Plastic Optical Fibers with Graded-Index Core Distribution
Abstract
:1. Introduction
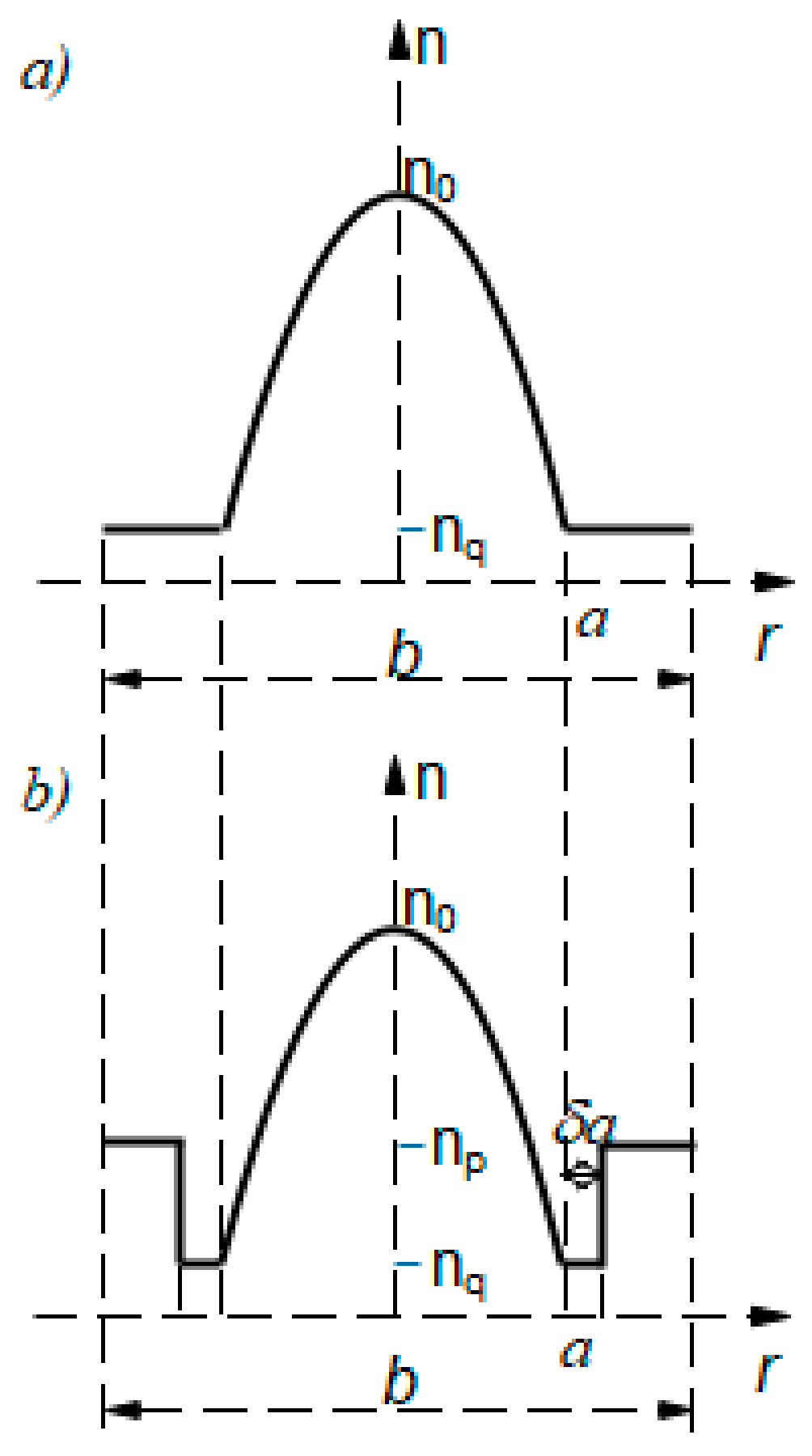
2. Time-Dependent Power Flow Equation
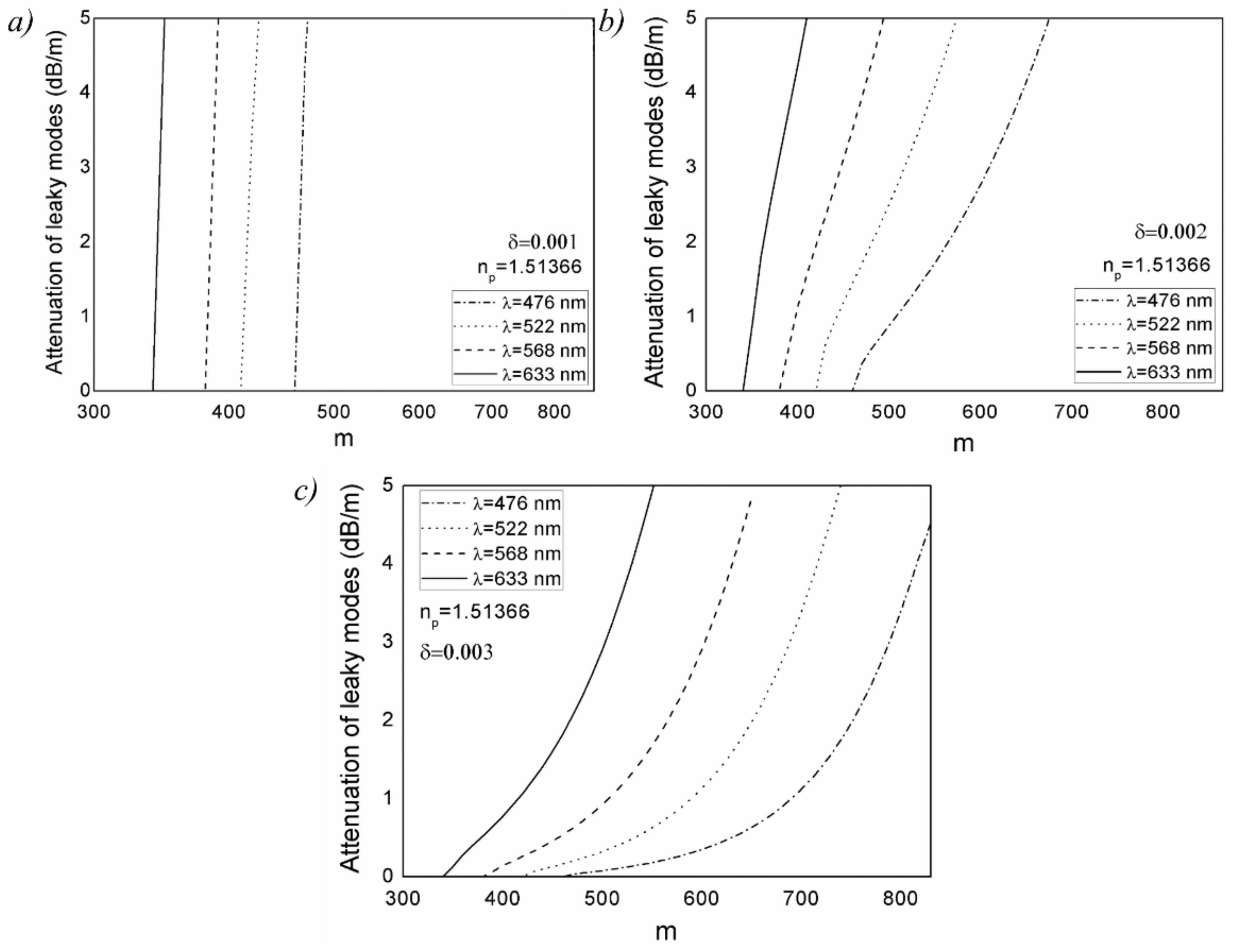
3. Numerical Results and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Ziemann, O.; Krauser, J.; Zamzow, P.E.; Daum, W. Optical Short Range Transmission Systems. In POF Handbook; Springer: Berlin/Heidelberg, Germany, 2008; ISBN 978-3-540-76629-2. [Google Scholar]
- Gloge, D. Optical power flow in multimode fibers. Bell Syst. Tech. J. 1972, 51, 1767–1783. [Google Scholar] [CrossRef]
- Gloge, D. Impulse response of clad optical multomode fibers. Bell Syst. Tech. J. 1973, 52, 801–816. [Google Scholar] [CrossRef]
- Savović, S.; Djordjevich, A. Mode coupling in strained and unstrained step-index plastic optical fibers. Appl. Opt. 2006, 45, 6775–6780. [Google Scholar] [CrossRef]
- Savović, S.; Djordjevich, A. Mode Coupling in Plastic-Clad Silica Fibers and Organic Glass-Clad PMMA Fibers. J. Lightwave Technol. 2014, 32, 1290–1294. [Google Scholar] [CrossRef]
- Eve, M.; Hannay, J.H. Ray theory and random mode coupling in an optical fibre waveguide, I. Opt. Quantum Electron. 1976, 8, 503–508. [Google Scholar] [CrossRef]
- Borecki, M. Light behaviour in polymer optical fibre bend-a new analysis method. Opt. Appl. 2003, 33, 191–204. [Google Scholar]
- Savović, S.; Simović, A.; Drljača, B.; Djordjevich, A.; Stepniak, G.; Bunge, C.A.; Bajić, J. Power flow in graded index plastic optical fibers. J. Lightwave Technol. 2019, 37, 4985–4990. [Google Scholar] [CrossRef]
- Savović, S.; Djordjevich, A. Solution of mode coupling in step-index optical fibers by the Fokker-Planck equation and the Langevin equation. Appl. Opt. 2002, 41, 2826–2830. [Google Scholar] [CrossRef]
- Simović, A.; Djordjevich, A.; Drljača, B.; Savović, S.; Min, R. Investigation of bandwidth in multimode graded index plastic optical fibers. Opt. Express 2021, 29, 29587–29594. [Google Scholar] [CrossRef] [PubMed]
- Patel, K.M.; Ralph, S.E. Enhanced multimode fiber link performance using a spatially resolved receiver. IEEE Photon. Technol. Lett. 2002, 14, 393–395. [Google Scholar] [CrossRef]
- Tyler, E.J.; Webster, M.; Penty, R.V.; White, I.H.; Yu, S.; Rorison, J. Subcarrier modulated transmission of 2.5 Gb/s over 300 m of 62.5-μm-core diameter multimode fiber. IEEE Photon. Technol. Lett. 2002, 14, 1743–1745. [Google Scholar] [CrossRef]
- Zhao, X.; Choa, F.S. Demonstration of 10 Gb/s transmission over 1.5-km-long multimode fiber using equalization techniques. IEEE Photon. Technol. Lett. 2002, 14, 1187–1189. [Google Scholar] [CrossRef]
- Abbott, J.S.; Smith, G.E.; Truesdale, C.M. Multimode Fiber Link Dispersion Compensator. U.S. Patent 6,363,195, 26 March 2002. [Google Scholar]
- Yamashita, T.; Kagami, M. Fabrication of light-induced self-written waveguides with a W-shaped refractive index profile. J. Lightwave Technol. 2005, 23, 2542–2548. [Google Scholar] [CrossRef]
- Asai, M.; Inuzuka, Y.; Koike, K.; Takahashi, S.; Koike, Y. High-bandwidth graded-index plastic optical fiber with low-attenuation, high-bending ability, and high-thermal stability for home-networks. J. Lightvawe Technol. 2011, 29, 1620–1626. [Google Scholar] [CrossRef]
- Mikoshiba, K.; Kajioka, H. Transmission characteristics of multimode W-type optical fiber: Experimental study of the effect of the intermediate layer. Appl. Opt. 1978, 17, 2836–2841. [Google Scholar] [CrossRef] [PubMed]
- Koike, Y.; Koike, K. Progress in low-loss and high-bandwidth plastic optical fibers. J. Polym. Sci. B 2011, 49, 2–17. [Google Scholar] [CrossRef]
- Takahashi, K.; Ishigure, T.; Koike, Y. Index profile design for high-bandwidth W-shaped plastic optical fiber. J. Lightwave Technol. 2006, 24, 2867–2876. [Google Scholar] [CrossRef]
- Tanaka, T.; Yamada, S.; Sumi, M.; Mikoshiba, K. Microbending losses of doubly clad (W-type) optical fibers. Appl. Opt. 1977, 18, 2391–2394. [Google Scholar] [CrossRef]
- Simović, A.; Savović, S.; Drljača, B.; Djordjevich, A. Influence of the fiber design and launch beam on transmission characteristics of W-type optical fibers. Opt. Laser Technol. 2015, 68, 151–159. [Google Scholar] [CrossRef]
- Savović, S.; Simović, A.; Djordjevich, A. Explicit finite difference solution of the power flow equation in W-type optical fibers. Opt. Laser Techn. 2012, 44, 1786–1790. [Google Scholar] [CrossRef]
- Kitayama, K.; Seikai, S.; Uchida, N. Impulse response prediction based on experimental mode coupling coefficient in a 10-km long graded-index fiber. IEEE J. Quant. Electron. 1980, 16, 356–362. [Google Scholar] [CrossRef]
- Olshansky, R. Mode coupling effects in graded-index optical fibers. Appl. Opt. 1975, 14, 935–945. [Google Scholar] [CrossRef] [PubMed]
- Nagano, K.; Kawakami, S. Measurements of mode conversion coefficients in graded-index fibers. Appl. Opt. 1980, 19, 2426–2434. [Google Scholar] [CrossRef]
- Yabre, G. Comprehensive theory of dispersion in graded-index optical fibers. J. Lightwave Technol. 2000, 18, 166–177. [Google Scholar] [CrossRef] [Green Version]
- Ishigure, T.; Endo, H.; Ohdoko, K.; Takahashi, K.; Koike, Y. Modal bandwidth enhancement in a plastic optical fiber by W-refractive index profile. J. Lightwave Technol. 2005, 23, 1754–1762. [Google Scholar] [CrossRef]
- Simović, A.; Djordjevich, A.; Drljača, B.; Savović, S. Power flow in W-type plastic optical fibers with graded index core distribution. Opt. Laser Technol. 2021, 143, 107295. [Google Scholar] [CrossRef]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Simović, A.; Savović, S.; Drljača, B.; Djordjevich, A.; Min, R. Theoretical Investigation of the Influence of Wavelength on the Bandwidth in Multimode W-Type Plastic Optical Fibers with Graded-Index Core Distribution. Polymers 2021, 13, 3973. https://doi.org/10.3390/polym13223973
Simović A, Savović S, Drljača B, Djordjevich A, Min R. Theoretical Investigation of the Influence of Wavelength on the Bandwidth in Multimode W-Type Plastic Optical Fibers with Graded-Index Core Distribution. Polymers. 2021; 13(22):3973. https://doi.org/10.3390/polym13223973
Chicago/Turabian StyleSimović, Ana, Svetislav Savović, Branko Drljača, Alexandar Djordjevich, and Rui Min. 2021. "Theoretical Investigation of the Influence of Wavelength on the Bandwidth in Multimode W-Type Plastic Optical Fibers with Graded-Index Core Distribution" Polymers 13, no. 22: 3973. https://doi.org/10.3390/polym13223973
APA StyleSimović, A., Savović, S., Drljača, B., Djordjevich, A., & Min, R. (2021). Theoretical Investigation of the Influence of Wavelength on the Bandwidth in Multimode W-Type Plastic Optical Fibers with Graded-Index Core Distribution. Polymers, 13(22), 3973. https://doi.org/10.3390/polym13223973







