Assessment of Extremely Cold Subarctic Climate Environment Destruction of the Basalt Fiber Reinforced Epoxy (BFRE) Rebar Using Its Moisture Uptake Kinetics
Abstract
:1. Introduction
2. Materials and Methods
2.1. Raw Materials
2.2. Manufacture of Composite and Specimen Preparation
2.3. Climatic Aging of BFRP Rebar
2.4. Moister Absorption Tests and Kinetic Moisture Uptake Profile
2.5. Fickian Diffusion Model for a Long Cylinder
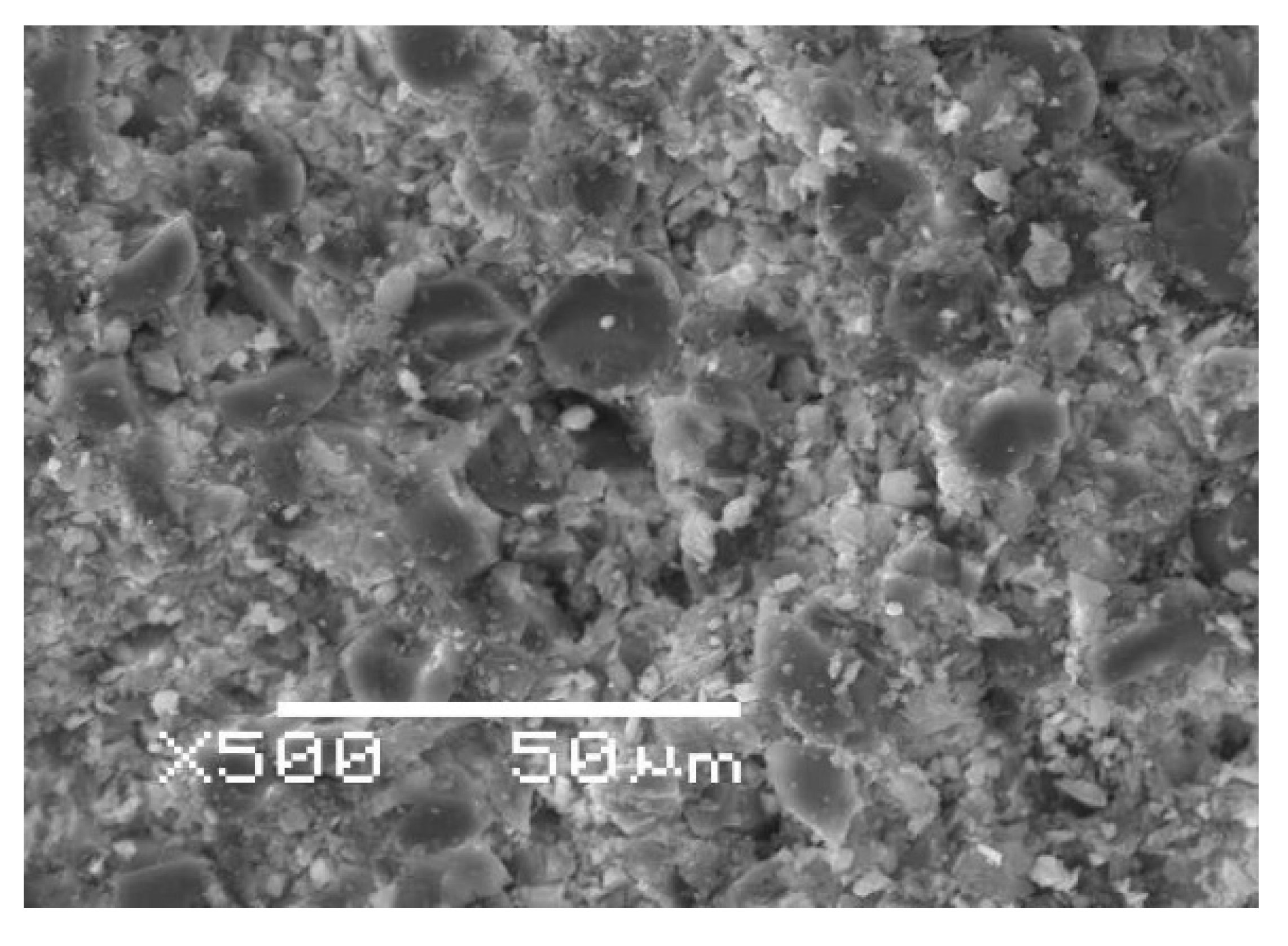
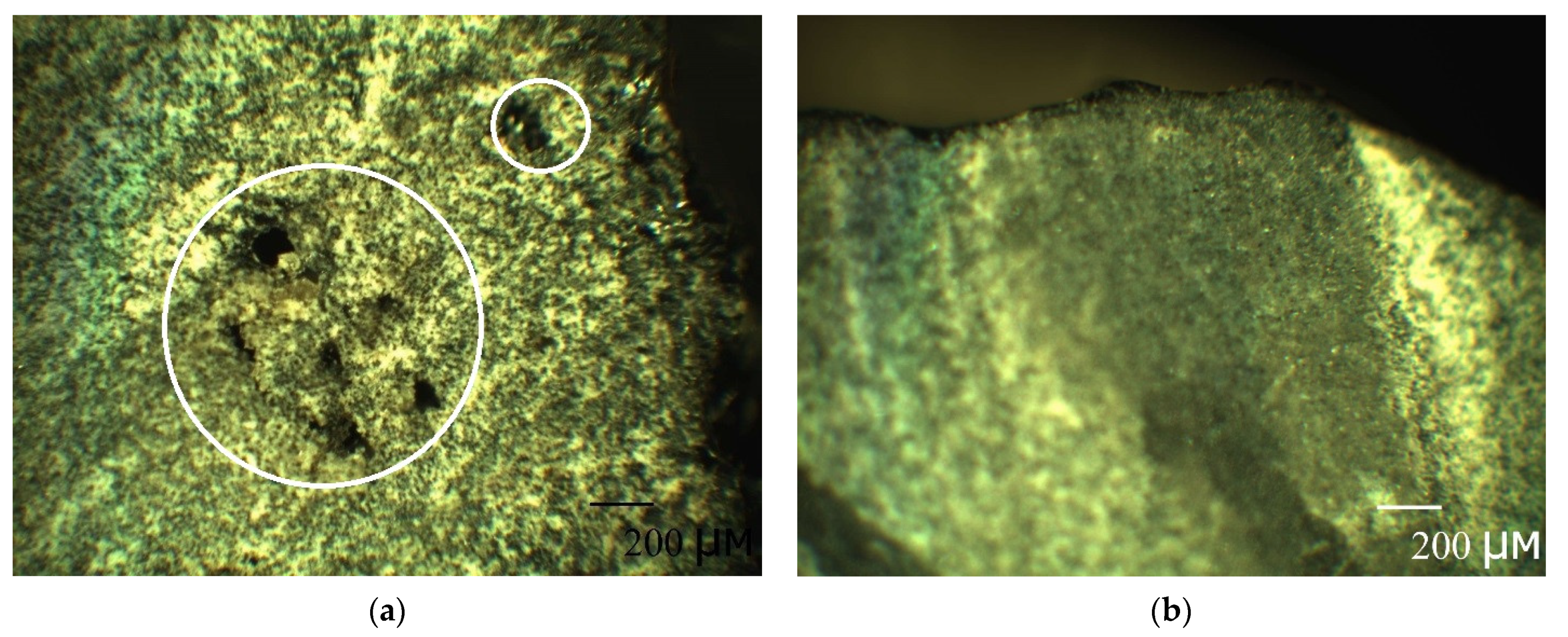
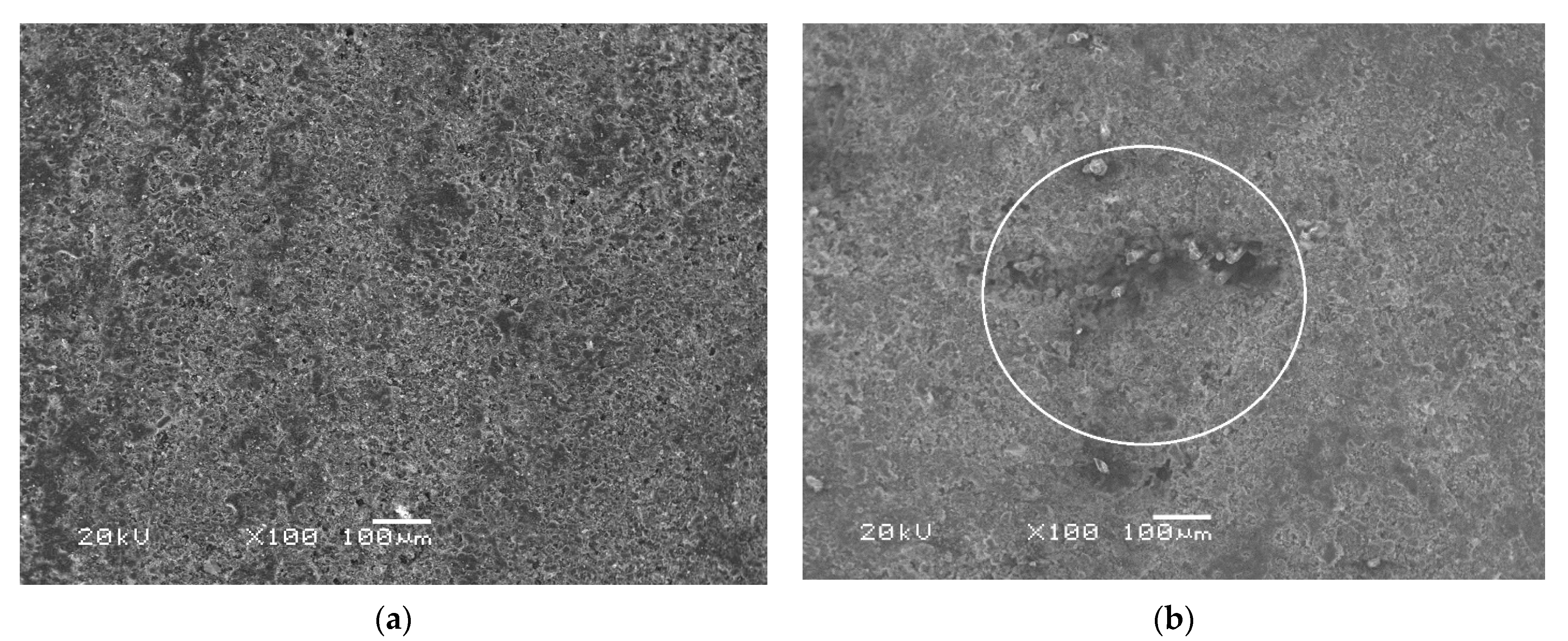
2.6. Macroanalysis of Material Structure
3. Results and Discussions
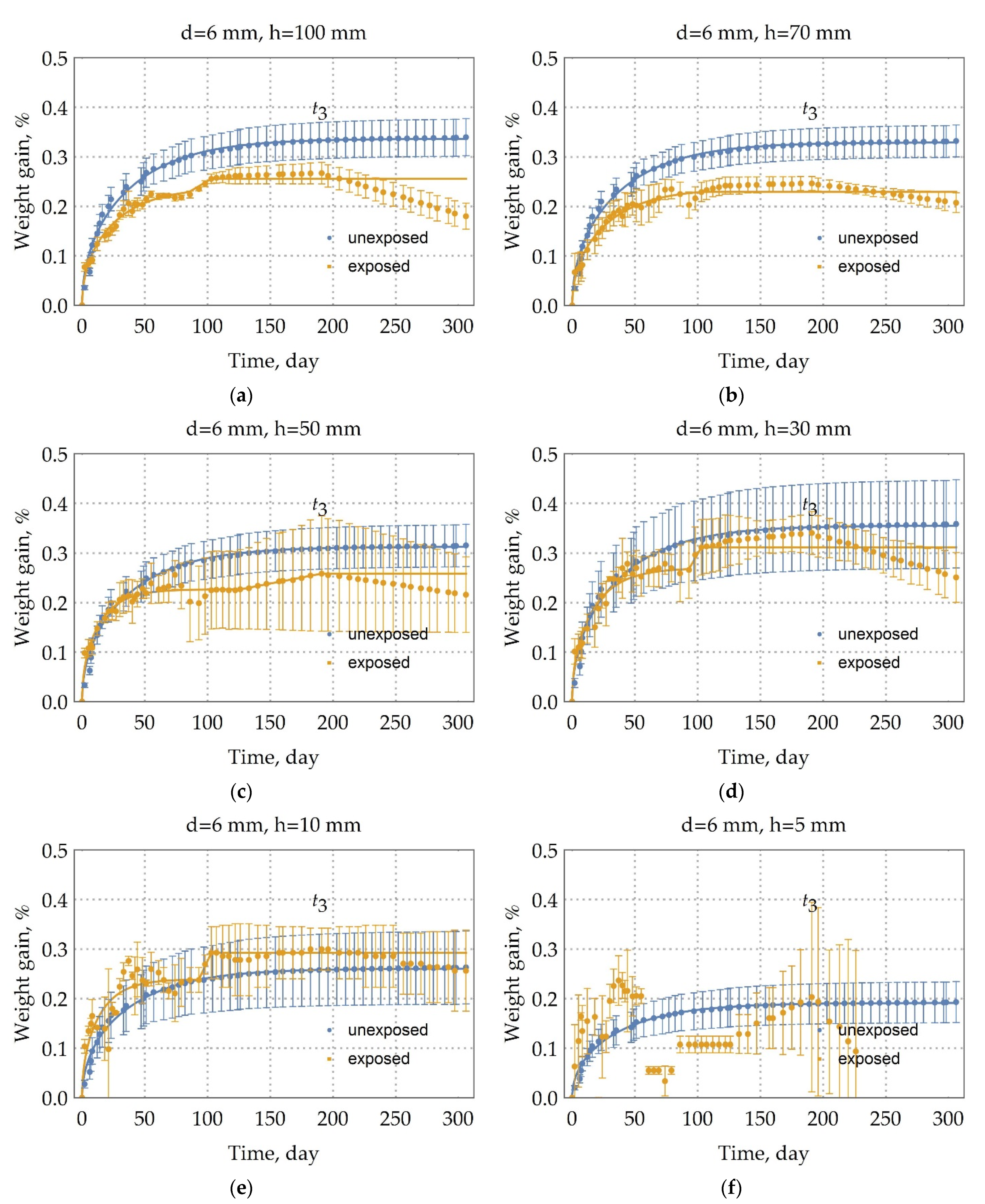
3.1. Experimental Moisture Uptake Kinetics (a Three-Stage Diffusion)
3.2. Approximation of Moisture Uptake Kinetics
3.3. Evaluation of the BFRP Rebar Destruction
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Dexter, H.B. Long-Term Environmental Effects and Flight Service Evaluation of Composite Materials. 1987. Available online: https://ntrs.nasa.gov/citations/19870008425 (accessed on 5 December 2021).
- Baker, D.J. Ten-Year Ground Exposure of Composite Materials Used on the Bell Model 206L Helicopter Flight Service Program. 1994. Available online: https://ntrs.nasa.gov/citations/19950005944 (accessed on 5 December 2021).
- Hoffman, D.J.; Bielawski, W.J. Environmental Exposure Effects on Composite Materials for Commercial Aircraft. 1990. Available online: https://archive.org/details/NASA_NTRS_Archive_19910015045 (accessed on 5 December 2021).
- Vodicka, R. Environmental Exposure of Boron-Epoxy Composite Material; Defence Science and Technology Organisation Melbourne: Melbourn, Australia, 2000. [Google Scholar]
- Martin, R. Ageing of Composites; Martin, R., Ed.; Woodhead Publishing: Cambridge, UK, 2008. [Google Scholar]
- Nishizaki, I.; Sakurada, H.; Tomiyama, T. Durability of pultruded GFRP through ten-year outdoor exposure test. Polymers 2015, 7, 2494–2503. [Google Scholar] [CrossRef] [Green Version]
- Bhide, S.J.; Zurale, M.M. Durability aspects of fibre reinforced composites. In Durability of Building Materials and Components 8; Lacasse, M.A., Vanier, D.J., Eds.; Institute for Research in Construction: Ottawa, ON, Canada, 1999; pp. 1382–1391. [Google Scholar]
- Kablov, E.N.; Startsev, V.O. Klimaticheskoe starenie polimernyh kompozicionnyh materialov aviacionnogo naznacheniya. 1. Ocenka vliyaniya znachimyh faktorov vozdejstviya. Deform. Razrushenie Mater. 2019, 12, 7–16. [Google Scholar] [CrossRef]
- Kablov, E.N.; Startsev, V.O. Klimaticheskoe starenie polimernyh kompozicionnyh materialov aviacionnogo naznacheniya. 2. Razvitie metodov issledovaniya rannih stadij stareniya. Deform. Razrushenie Mater. 2020, 1, 15–21. [Google Scholar] [CrossRef]
- Kablov, E.N.; Startsev, V.O. Sistemnyj analiz vliyaniya klimata na mekhanicheskie svojstva polimernyh kompo-zicionnyh materialov po dannym otechestvennyh i zarubezhnyh istochnikov. Aviac. Mater. Tekhnologii 2018, 2, 47–58. Available online: https://cyberleninka.ru/article/n/sistemnyy-analiz-vliyaniya-klimata-na-mehanicheskie-svoystva-polimernyh-kompozitsionnyh-materialov-po-dannym-otechestvennyh-i/viewer (accessed on 5 December 2021).
- Starcev, V.O. Klimaticheskaya Stojkost’ Polimernyh Kompozicionnyh Materialov i Zashchitnyh Pokrytij v Umerenno-Teplom Climate. Ph.D. Thesis, Degree-Granting University, Moscow, Russia, 2018. [Google Scholar]
- Startsev, V.O.; Lebedev, M.P.; Kychkin, A.K. Influence of moderately warm and extremely cold climate on properties of basalt plastic rebars. Heliyon 2018, 4, e01060. [Google Scholar] [CrossRef] [Green Version]
- Aviacionnye Materialy. Spravochnik v 13 tomah. Tom 13. Klimaticheskaya i Mikrobiologicheskaya Stojkost’ Nemetallicheskih Materialov. 2015. Available online: https://viam.ru/sites/default/files/uploads/news/2019/6435/6435_tom_13_soderzhanie.pdf (accessed on 5 December 2021).
- Nikolaev, E.V.; Barbot’ko, S.L.; Andreeva, N.P.; Pavlov, M.R.; Grashchenkov, D.V. Kompleksnoe issledovanie vozdejstviya klimaticheskih i ekspluatacionnyh faktorov na novoe pokolenie epoksidnogo svyazuyushchego i polimernyh kompozicionnyh materialov na ego osnove. Chast’ 4. Naturnye klimaticheskie ispytaniya polimernyh kompozicionnyh materialov na osnove epoksidnoj matricy. Trudy VIAM 2016, 6, 93–108. Available online: http://viam-works.ru/ru/articles?art_id=975 (accessed on 8 December 2021).
- Panin, S.V.; Starcev, O.V.; Krotov, A.S. Diagnostika nachal’noj stadia klimaticheskogo stareniya PKM po izmeneniyu koefficienta diffuzii vlagi. Trudy VIAM 2014, 7, e9. Available online: http://viam-works.ru/ru/articles?art_id=688 (accessed on 5 December 2021).
- Starcev, O.V.; Kuznecov, A.A.; Krotov, A.S.; Anihovskaja, L.I.; Senatorova, O.G. Modelirovanie vlagoperenosa v sloistyh plastikah i stekloplastikah. Fiz. Mezomeh. 2002, 5, 109–114. [Google Scholar]
- Starcev, O.V.; Krotov, A.S. Sorbciya i diffuziya vlagi v stekloplastikovyh sterzhnyah kruglogo secheniya. Materialovedenie 2012, 6, 24–28. [Google Scholar]
- Alessi, S.; Pitarresi, G.; Spadaro, G. Effect of hydrothermal ageing on the thermal and delamination fracture behaviour of CFRP composites. Compos. Part B Eng. 2014, 67, 145–153. [Google Scholar] [CrossRef]
- Zhang, A.Y.; Li, D.H.; Zhang, D.X.; Lu, H.B.; Xiao, H.Y.; Jia, J. Qualitative separation of the effect of voids on the static mechanical properties of hygrothermally conditioned carbon/epoxy composites. Express Polym. Lett. 2011, 5, 708–716. [Google Scholar] [CrossRef]
- Janas, V.F.; McCullough, R.L. Moisture absorption in unfilled and glass-filled, cross-linked polyester. Compos. Sci. Technol. 1987, 29, 293–315. [Google Scholar] [CrossRef]
- Hodzica, A.; Kim, J.K.; Lowe, A.E.; Stachurski, Z.H. The effects of water aging on the interphase region and interlaminar fracture toughness in polymer–glass composites. Compos. Sci. Technol. 2004, 64, 2185–2195. [Google Scholar] [CrossRef]
- Costa, M.L. Strength of hygrothermally conditioned polymer composites with voids. J. Compos. Mater. 2005, 39, 1943–1961. [Google Scholar] [CrossRef]
- ASTM. Standard Test. Method for Moisture Absorption Properties and Equilibrium Conditioning of Polymer Matrix Composite Materials; ASTM D5229; ASTM International: West Conshohocken, PA, USA, 2014. [Google Scholar]
- Fick, A. Ueber diffusion. Ann. Phys. Chem. 1855, 170, 59–86. [Google Scholar] [CrossRef]
- Crank, J. The Mathematics of Diffusion, 2nd ed.; Glarendon Press: Oxford, UK, 1975. [Google Scholar]
- Glaskova, T.I.; Guedes, R.M.; Morais, J.J.; Aniskevich, A.N. A comparative analysis of moisture transport models as applied to an epoxy binder. Mech. Compos. Mater. 2007, 43, 377–388. [Google Scholar] [CrossRef]
- Flory, P.J. Principles of Polymer Chemistry; Cornell University Press: Ithaca, NY, USA, 1953. [Google Scholar]
- Bagley, E.; Long, F.A. Two-stage sorption and desorption of organic vapors in cellulose acetate. J. Am. Chem. Soc. 1955, 77, 2172–2178. [Google Scholar] [CrossRef]
- Newns, A.C. The sorption and desorption kinetics of water in a re-generated cellulose. Trans. Faraday Soc. 1956, 52, 1533–1545. [Google Scholar] [CrossRef]
- Aniskevich, A.; Glaskova-Kuzmina, T. Effect of moisture on elastic and viscoelastic properties of fiber reinforced plastics: Retrospective and current trends. In Creep and Fatigue in Polymer Matrix Composites; Woodhead Publishing: Sawston, UK, 2019; pp. 83–120. [Google Scholar] [CrossRef]
- Varelidis, P.C.; Kominos, N.P.; Papaspyrides, C.D. Polyamide coated glass fabric in polyester resin: Interlaminar shear strength versus moisture absorption studies. Compos. Part A 1998, 29, 1489–1499. [Google Scholar] [CrossRef]
- Pothan, L.A.; Thomas, S. Effect of hybridization and chemical modification on the water-absorption behavior of banana fiber–reinforced polyester composites. J. Appl. Polym. Sci. 2004, 91, 3856–3865. [Google Scholar] [CrossRef]
- Lukachevskaya, I.G.; Gavrilieva, A.A.; Kychkin, A.K.; Kychkin, A.A.; Struchkov, N.F.; Diakonov, A.A. Estimate of the early stage of climatic aging of basalt- and glass-fiber reinforced plastics. Arct. Subarct. Nat. Resour. 2021, 26, 159–169. [Google Scholar] [CrossRef]
- Kablov, E.N.; Startsev, O.V.; Krotov, A.S.; Kirillov, V.N. Klimaticheskoe starenie kompozicionny‘x materialov aviacionnogo naznacheniya. 2. Relaksaciya isxodnoj strukturnoj neravnoesnosti i gradient svojstv po tolshhine. Deform. Razrushenie Mater. 2010, 12. Available online: https://viam.ru/sites/default/files/scipub/2010/2010-205598.pdf (accessed on 5 December 2021).
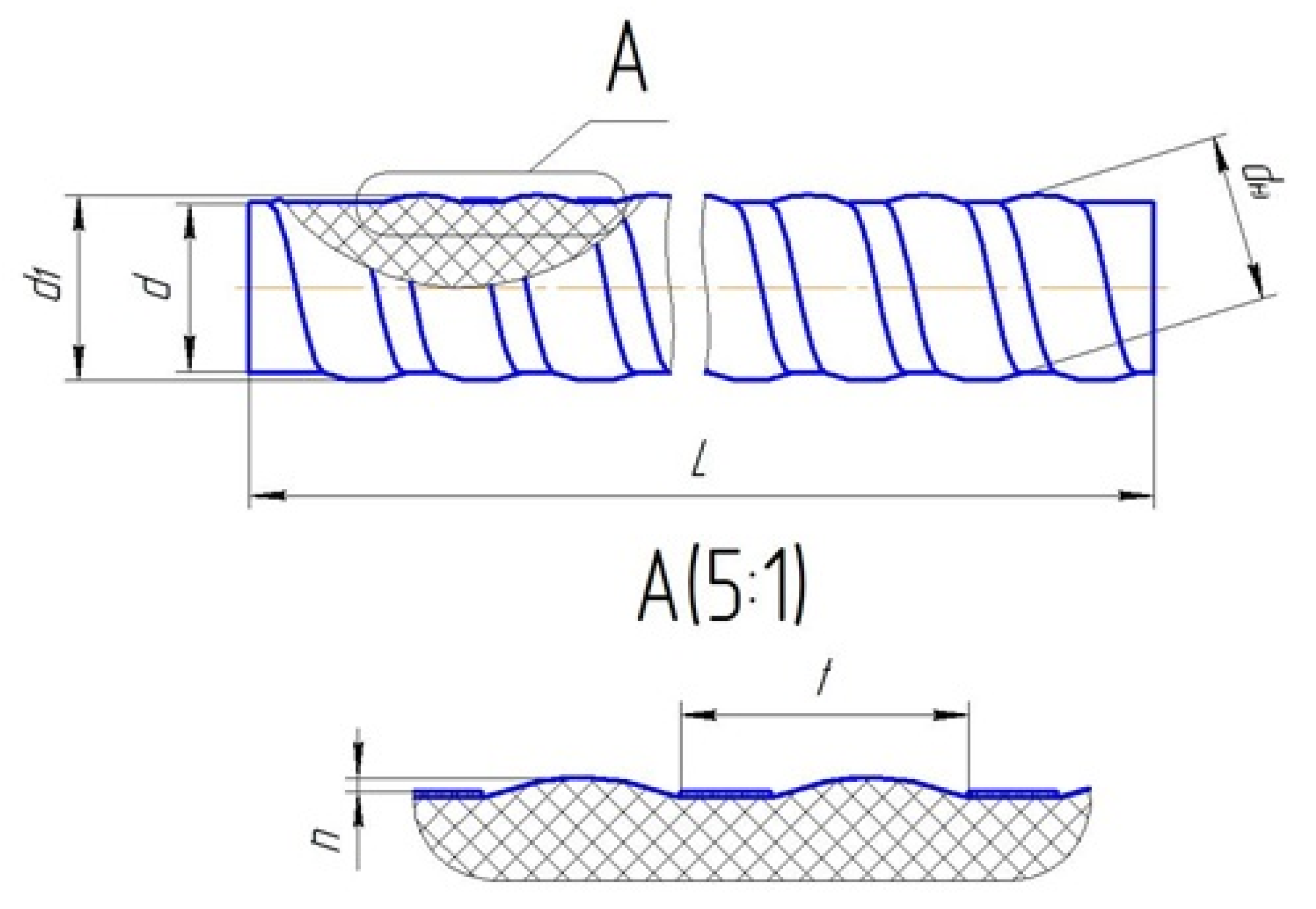


| d (mm) | d1 (mm) | dн (mm) | n (mm) | l (mm) | Fibres Mass Share (%) |
|---|---|---|---|---|---|
| 6 | 7 | 6.5 | 0.5 | 5–7 | 77 |
| 10 | 12 | 10.5 | 1 | 7–9 | 78 |
| 18 | 23 | 18.4 | 1.5 | 8–12 | 70 |
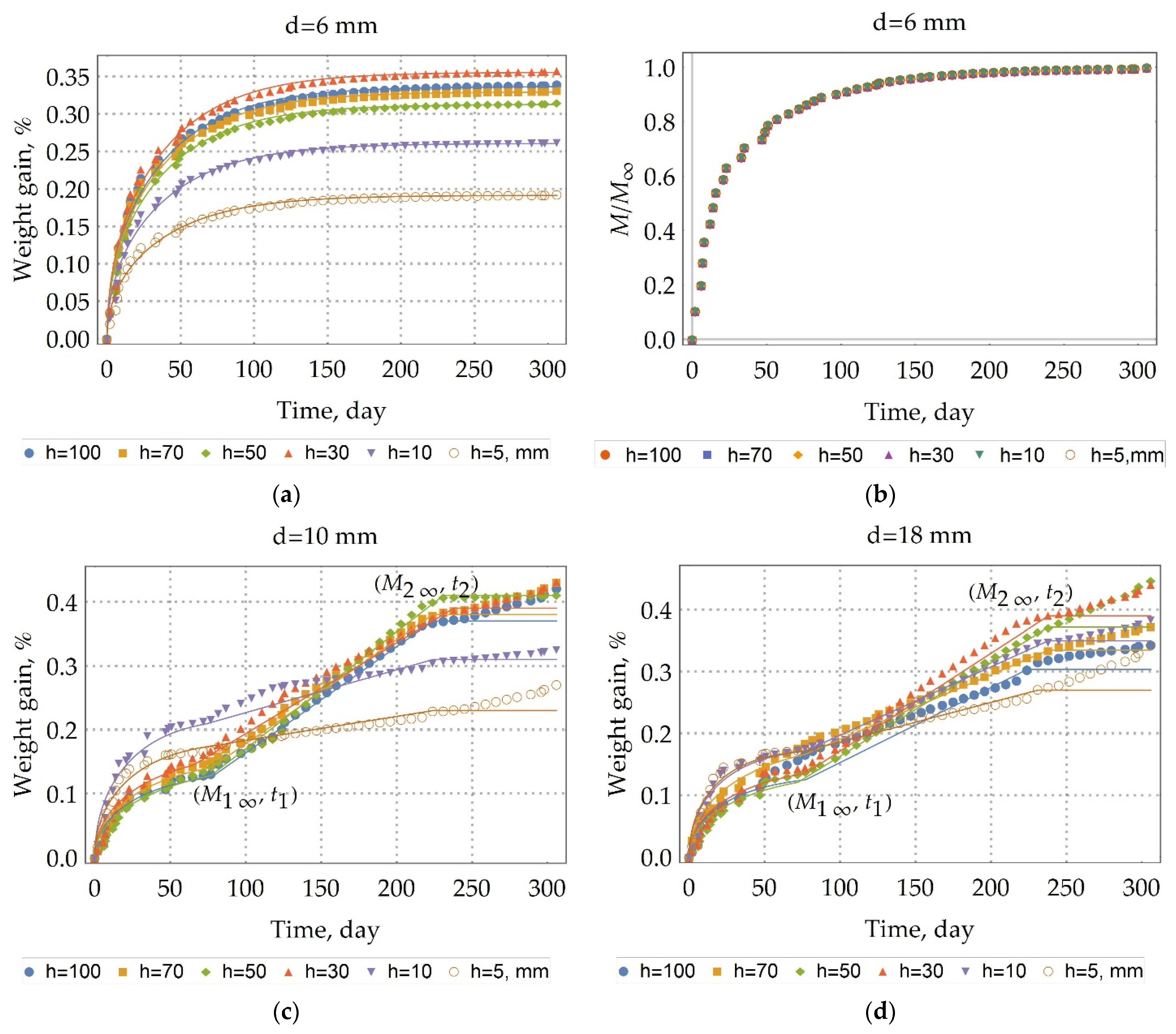
| A Diameter | A Height, mm | t1, Day | t2, day | t3, day | R2 from Equation (3) | ||||
|---|---|---|---|---|---|---|---|---|---|
| 6 mm | 100 | 0.34 | 3.49 | 3.88 | - | - | - | - | 0.94 |
| 70 | 0.33 | 3.49 | 3.88 | - | - | - | - | 0.94 | |
| 50 | 0.31 | 3.49 | 3.88 | - | - | - | - | 0.94 | |
| 30 | 0.36 | 3.49 | 3.88 | - | - | - | - | 0.93 | |
| 10 | 0.26 | 3.49 | 3.88 | - | - | - | - | 0.95 | |
| 5 | 0.19 | 3.49 | 3.88 | - | - | - | - | 0.96 | |
| 10 mm | 100 | 0.14 | 11.87 | 4.75 | 77 | 224 | 0.37 | 224 | 0.95 |
| 70 | 0.16 | 9.84 | 3.94 | 77 | 224 | 0.38 | 224 | 0.94 | |
| 50 | 0.16 | 7.20 | 2.88 | 77 | 231 | 0.41 | 231 | 0.95 | |
| 30 | 0.17 | 10.35 | 4.14 | 65 | 238 | 0.39 | 238 | 0.94 | |
| 10 | 0.22 | 14.47 | 5.79 | 72 | 224 | 0.31 | 224 | 0.93 | |
| 5 | 0.19 | 13.79 | 5.52 | 77 | 224 | 0.23 | 224 | 0.94 | |
| 18 mm | 100 | 0.13 | 40.66 | 5.02 | 65 | 224 | 0.30 | 224 | 0.95 |
| 70 | 0.19 | 29.73 | 3.67 | 77 | 238 | 0.33 | 238 | 0.95 | |
| 50 | 0.14 | 30.12 | 3.72 | 77 | 238 | 0.37 | 238 | 0.94 | |
| 30 | 0.15 | 34.39 | 4.25 | 77 | 238 | 0.39 | 238 | 0.94 | |
| 10 | 0.18 | 50.08 | 6.18 | 77 | 238 | 0.35 | 238 | 0.94 | |
| 5 | 0.18 | 57.05 | 7.04 | 77 | 231 | 0.27 | 231 | 0.94 |
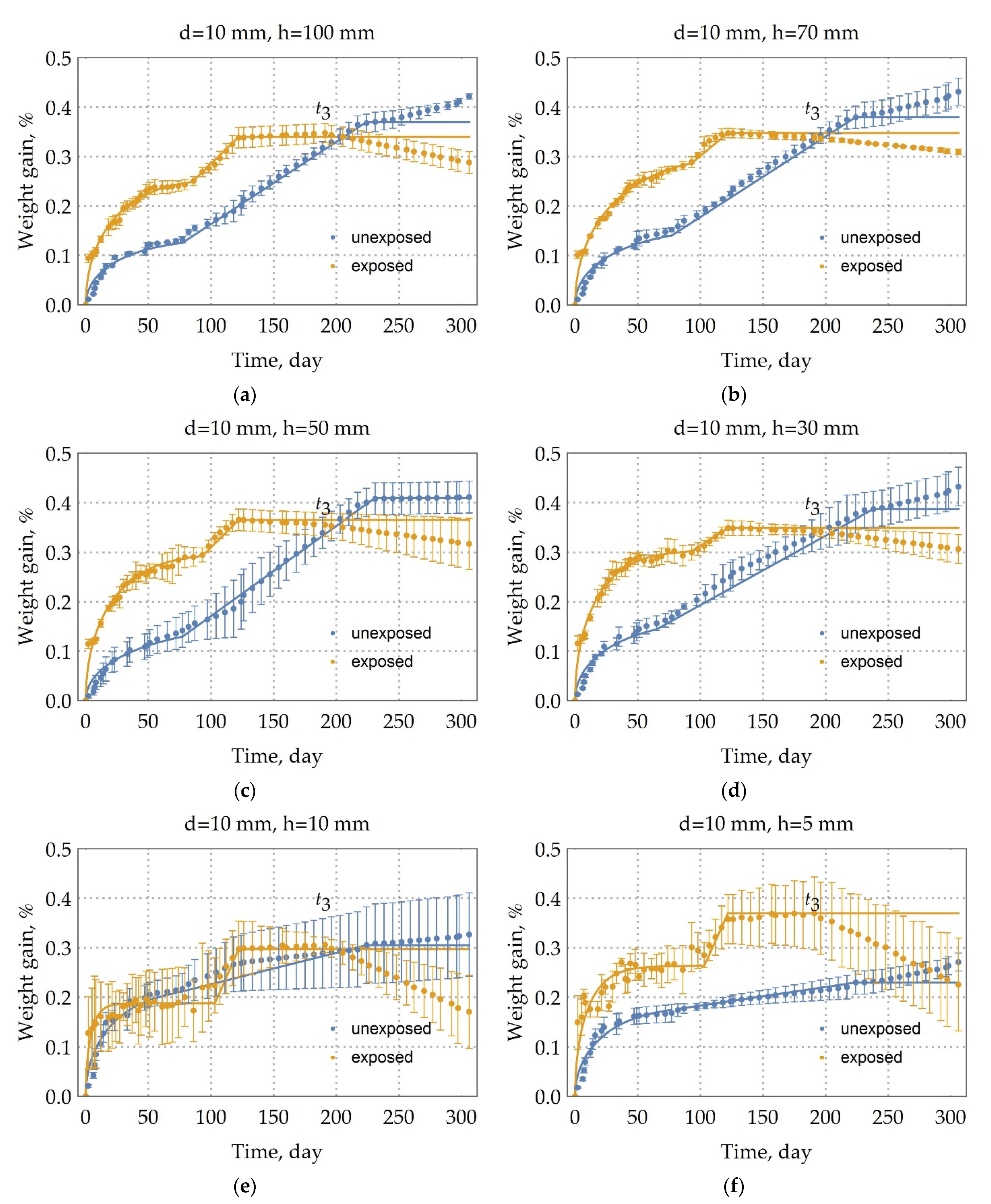
| A Diameter | A Height, mm | from Equation (2) | t1, Day | t2, Day | t3, Day | R2 from Equation (3) | |||
|---|---|---|---|---|---|---|---|---|---|
| 6 mm | 100 | 0.24 | 5.72 | 5.15 | 85 | 103 | 0.26 | 191 | 0.95 |
| 70 | 0.24 | 5.27 | 4.74 | 93 | 103 | 0.23 | 191 | 0.93 | |
| 50 | 0.23 | 10.27 | 9.24 | 126 | 191 | 0.26 | 191 | 0.94 | |
| 30 | 0.27 | 8.38 | 7.54 | 93 | 103 | 0.31 | 191 | 0.91 | |
| 10 | 0.24 | 10.31 | 9.28 | 93 | 103 | 0.29 | 182 | 0.85 | |
| 5 | - | - | - | - | - | - | 0 | - | |
| 10 mm | 100 | 0.26 | 5.74 | 14.35 | 85 | 122 | 0.34 | 191 | 0.96 |
| 70 | 0.31 | 4.15 | 10.38 | 93 | 122 | 0.34 | 191 | 0.95 | |
| 50 | 0.30 | 6.21 | 15.53 | 93 | 122 | 0.37 | 191 | 0.95 | |
| 30 | 0.31 | 7.65 | 19.12 | 93 | 122 | 0.35 | 191 | 0.95 | |
| 10 | 0.19 | 29.87 | 74.68 | 103 | 122 | 0.30 | 191 | 0.91 | |
| 5 | 0.26 | 12.72 | 31.80 | 103 | 122 | 0.37 | 191 | 0.86 | |
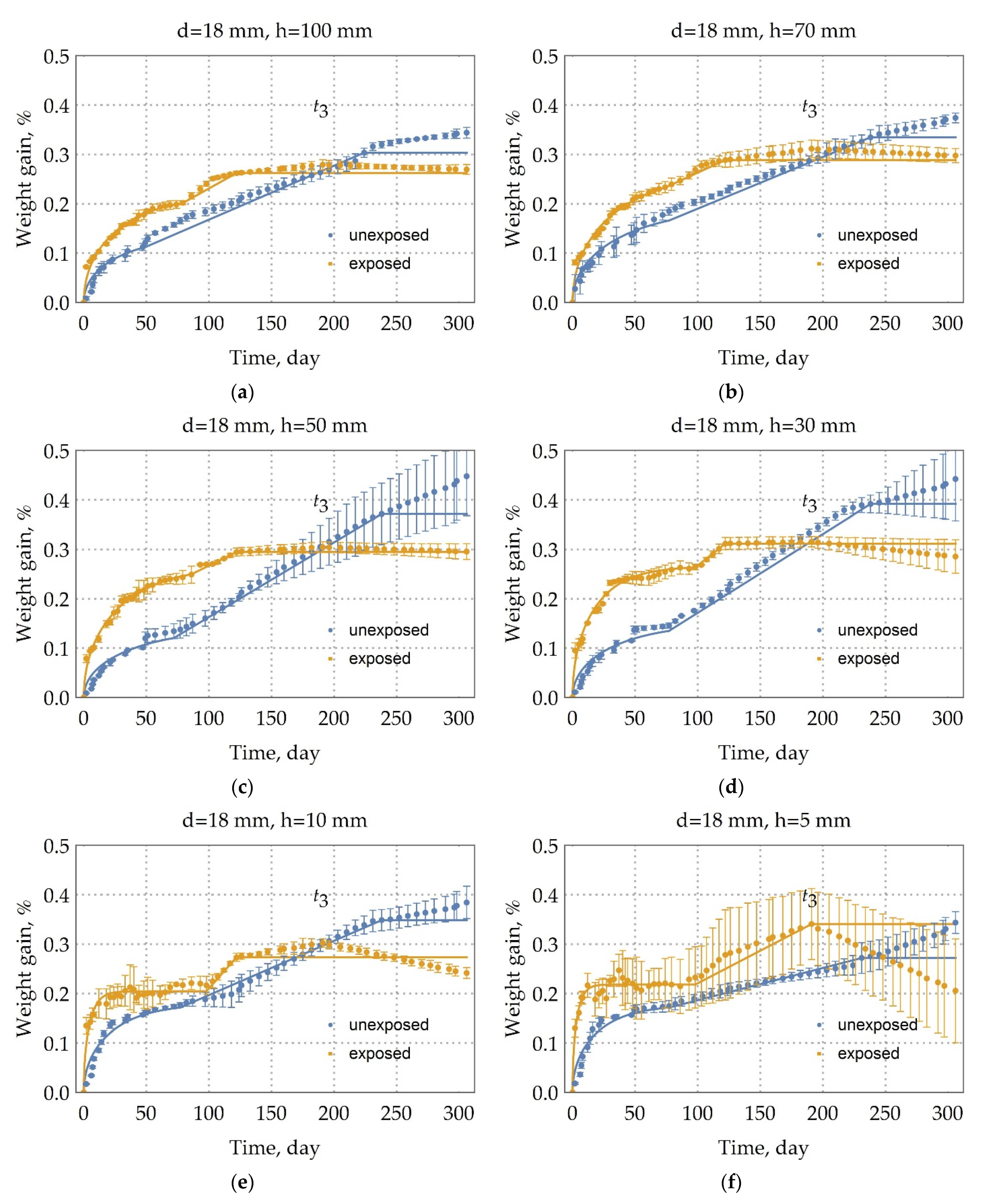
| 18 mm | 100 | 0.22 | 4.98 | 40.35 | 49 | 122 | 0.26 | 191 | 0.97 |
| 70 | 0.27 | 4.07 | 32.97 | 75 | 122 | 0.29 | 191 | 0.96 | |
| 50 | 0.27 | 4.90 | 39.72 | 86 | 122 | 0.29 | - | 0.97 | |
| 30 | 0.26 | 7.63 | 61.82 | 98 | 122 | 0.31 | 191 | 0.95 | |
| 10 | 0.20 | 28.69 | 232.35 | 98 | 122 | 0.27 | 191 | 0.94 | |
| 5 | 0.22 | 38.91 | 315.21 | 98 | 122 | 0.28 | 191 | 0.94 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kychkin, A.K.; Gavrilieva, A.A.; Vasilieva, A.A.; Kychkin, A.A.; Lebedev, M.P.; Sivtseva, A.V. Assessment of Extremely Cold Subarctic Climate Environment Destruction of the Basalt Fiber Reinforced Epoxy (BFRE) Rebar Using Its Moisture Uptake Kinetics. Polymers 2021, 13, 4325. https://doi.org/10.3390/polym13244325
Kychkin AK, Gavrilieva AA, Vasilieva AA, Kychkin AA, Lebedev MP, Sivtseva AV. Assessment of Extremely Cold Subarctic Climate Environment Destruction of the Basalt Fiber Reinforced Epoxy (BFRE) Rebar Using Its Moisture Uptake Kinetics. Polymers. 2021; 13(24):4325. https://doi.org/10.3390/polym13244325
Chicago/Turabian StyleKychkin, Anatoly K., Anna A. Gavrilieva, Alina A. Vasilieva, Aisen A. Kychkin, Mikhail P. Lebedev, and Anastasia V. Sivtseva. 2021. "Assessment of Extremely Cold Subarctic Climate Environment Destruction of the Basalt Fiber Reinforced Epoxy (BFRE) Rebar Using Its Moisture Uptake Kinetics" Polymers 13, no. 24: 4325. https://doi.org/10.3390/polym13244325
APA StyleKychkin, A. K., Gavrilieva, A. A., Vasilieva, A. A., Kychkin, A. A., Lebedev, M. P., & Sivtseva, A. V. (2021). Assessment of Extremely Cold Subarctic Climate Environment Destruction of the Basalt Fiber Reinforced Epoxy (BFRE) Rebar Using Its Moisture Uptake Kinetics. Polymers, 13(24), 4325. https://doi.org/10.3390/polym13244325






