Determination of the Operational Parameters for the Manufacturing of Spherical PVP Particles via Electrospray
Abstract
:1. Introduction
1.1. Motivation
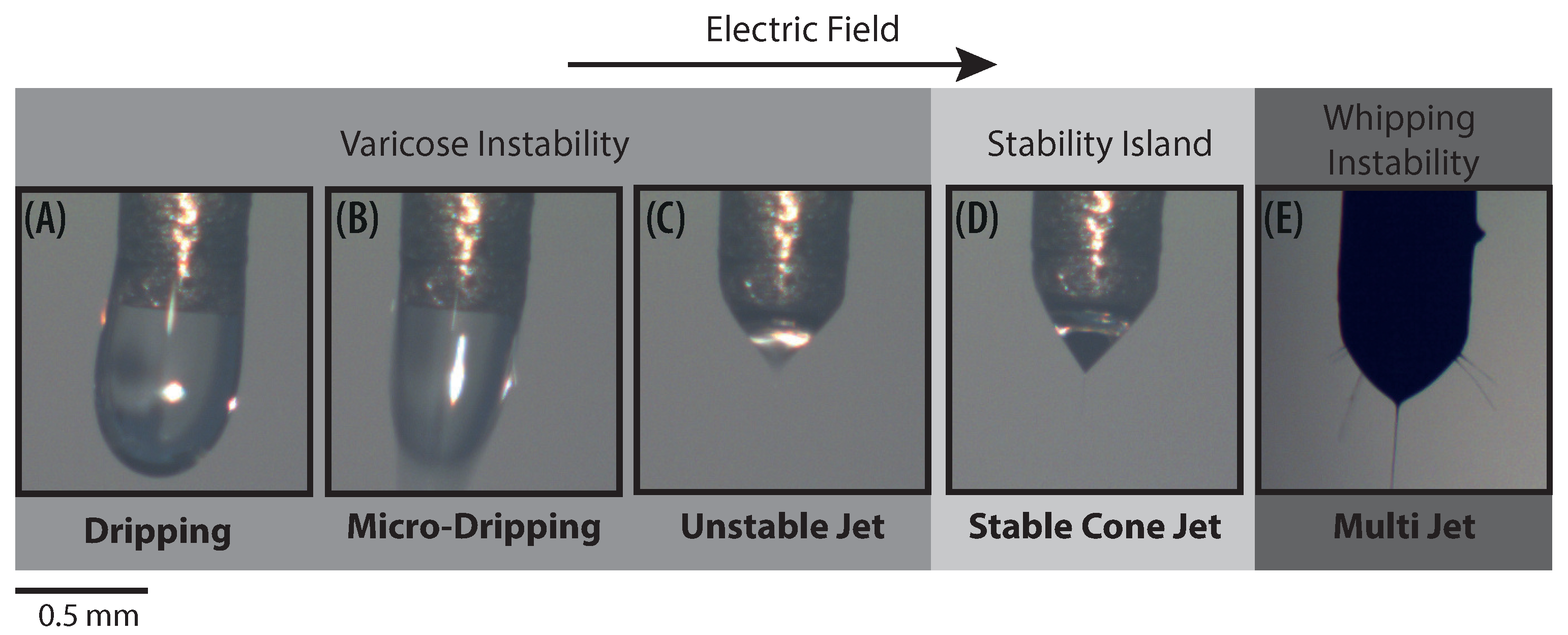
1.2. EHDA and Modes
1.3. CFD Studies
2. Numerical Model: Two-Phase Solver with an Electric Coupling
2.1. Governing Equations for the Fluid Flow
2.2. Maxwell Stress Tensor
2.3. Governing Equations for the Electric Field
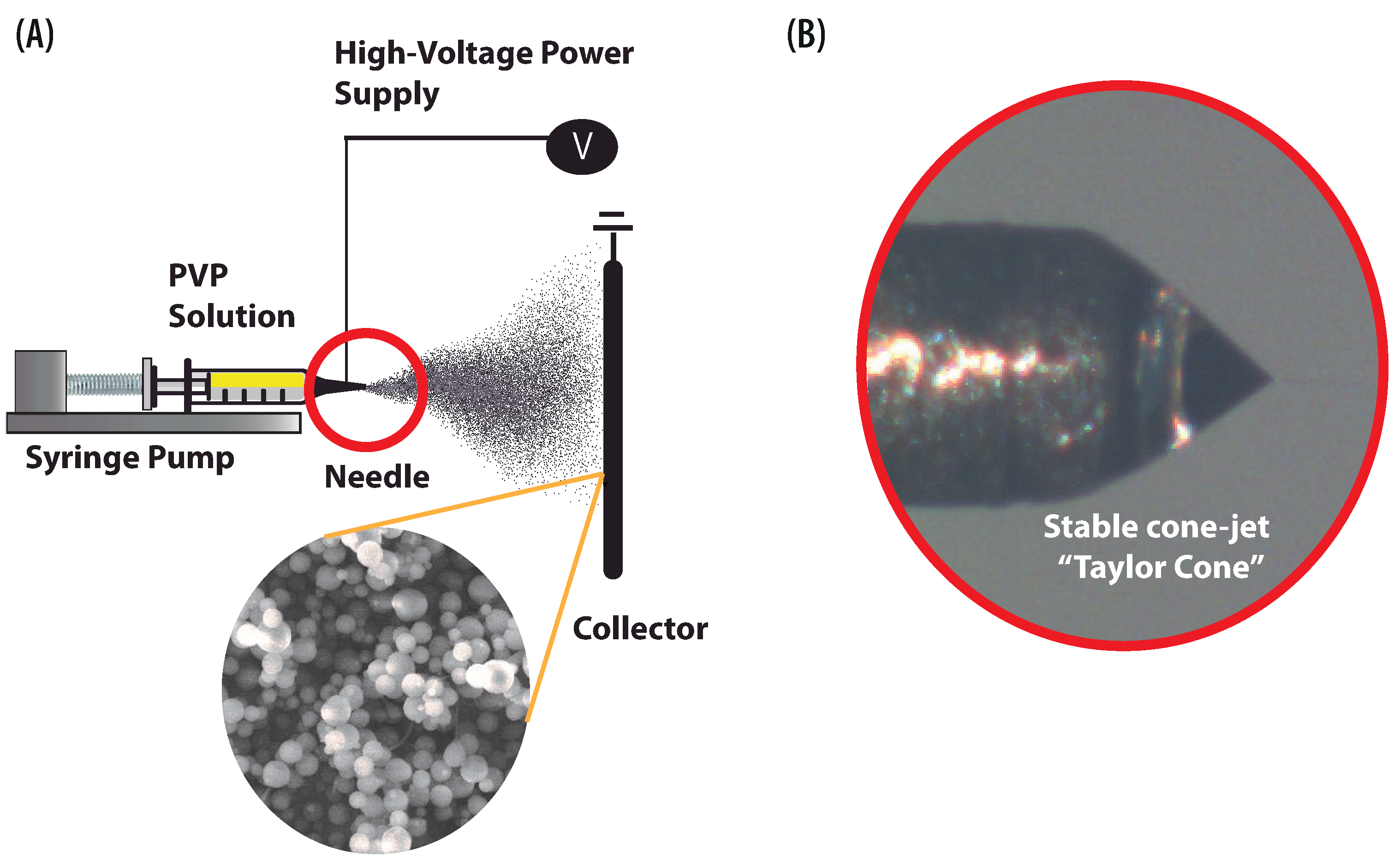
3. Experimental Method
3.1. Material Characterization: Viscosity and Surface Tension Measurement
3.2. Experimental EHDA Setup
3.3. Characterization the Morphology of the PVP Structures
4. Numerical Simulations
4.1. Geometry Domain and Boundary Conditions
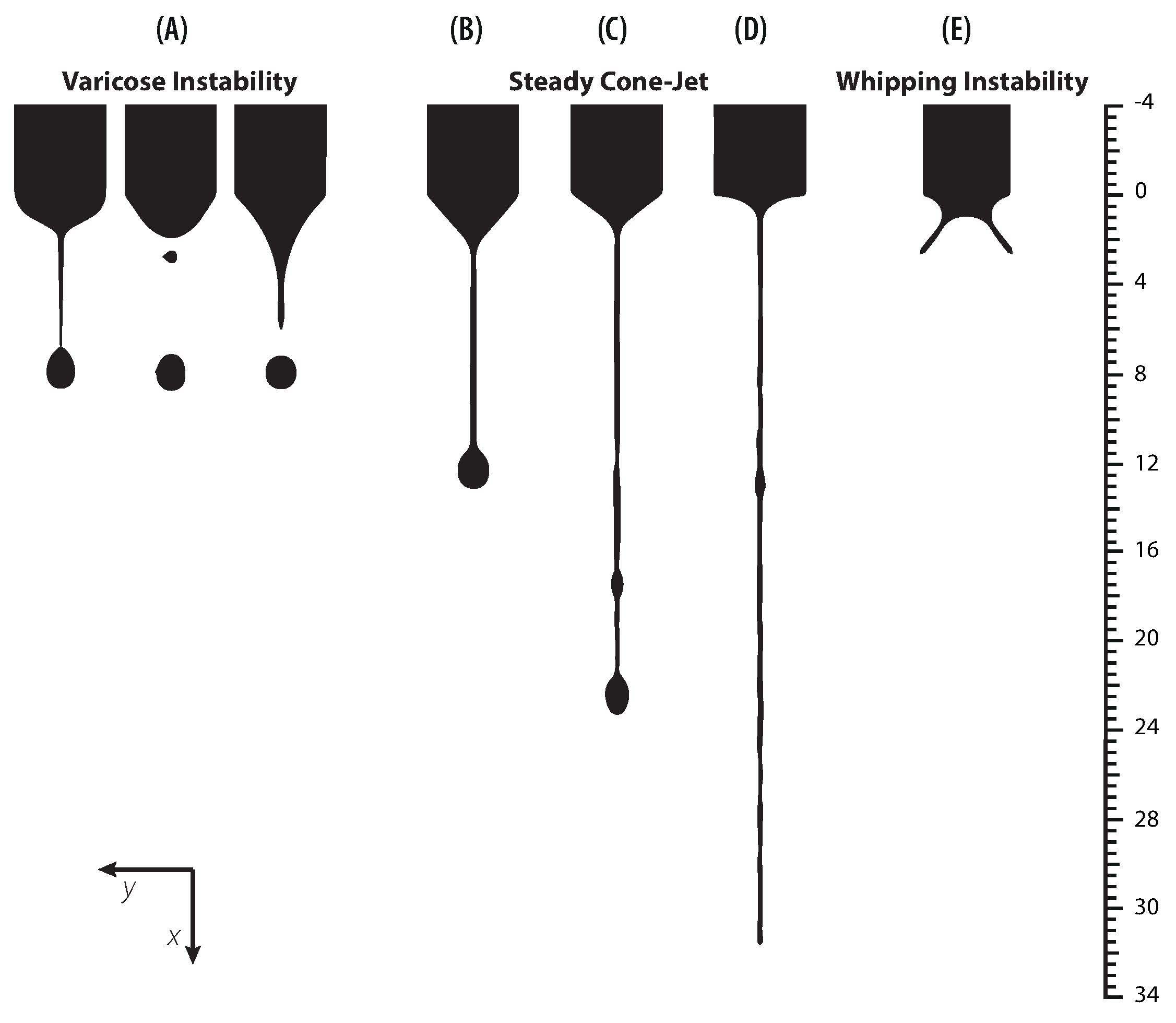
4.2. Validation Case: Taylor Cone Formation
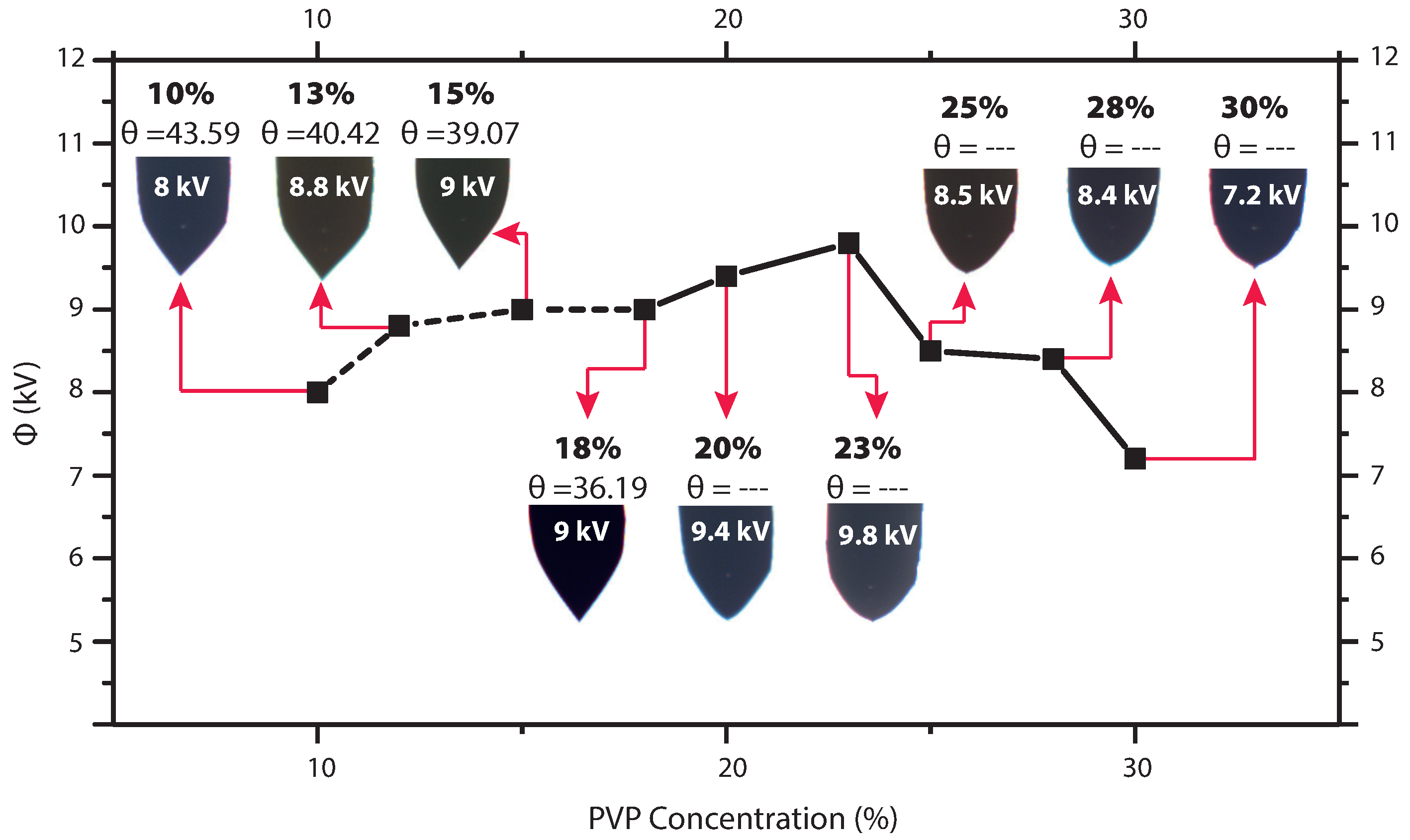
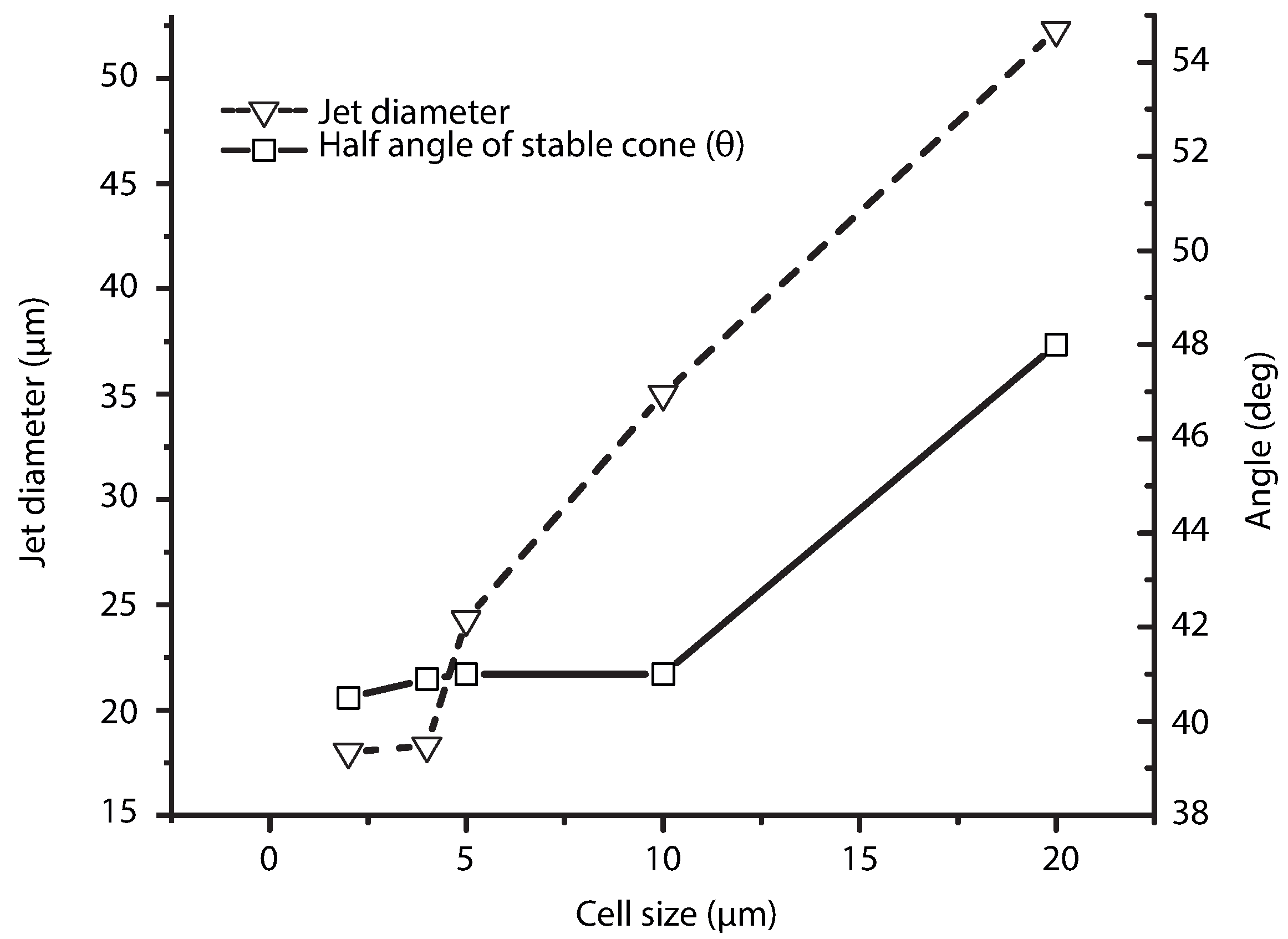
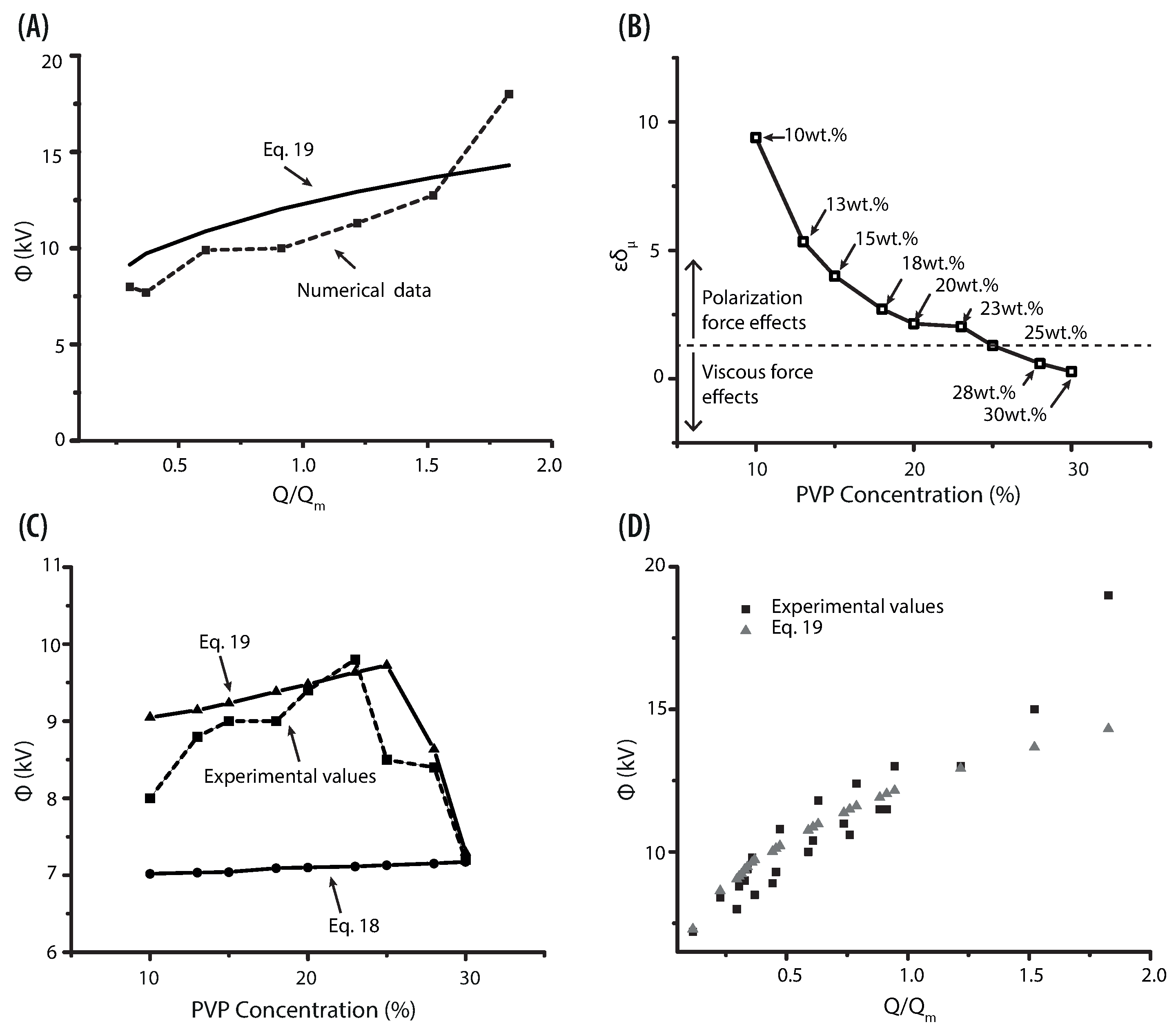
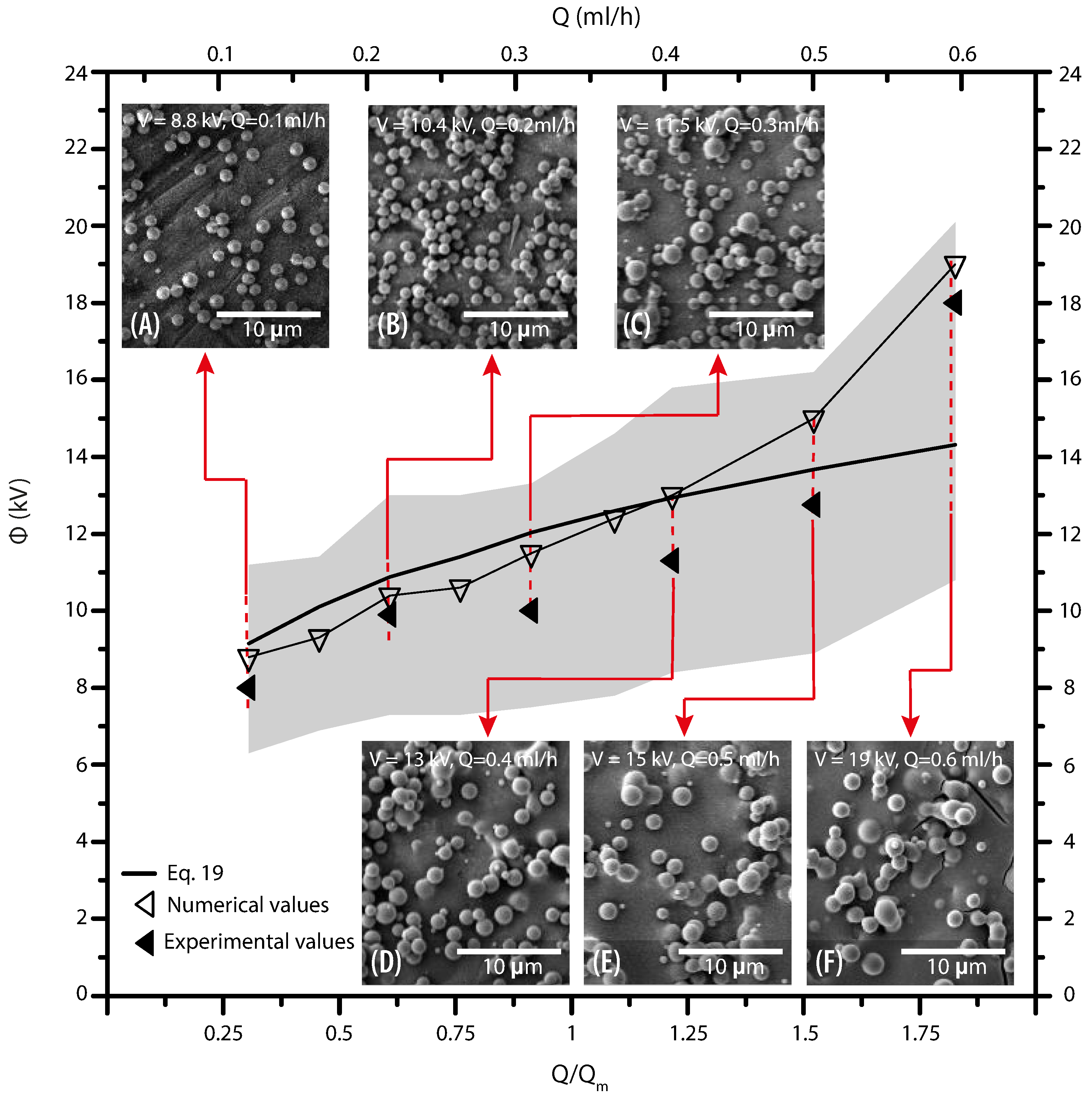
4.3. Prediction of the Operational Parameters
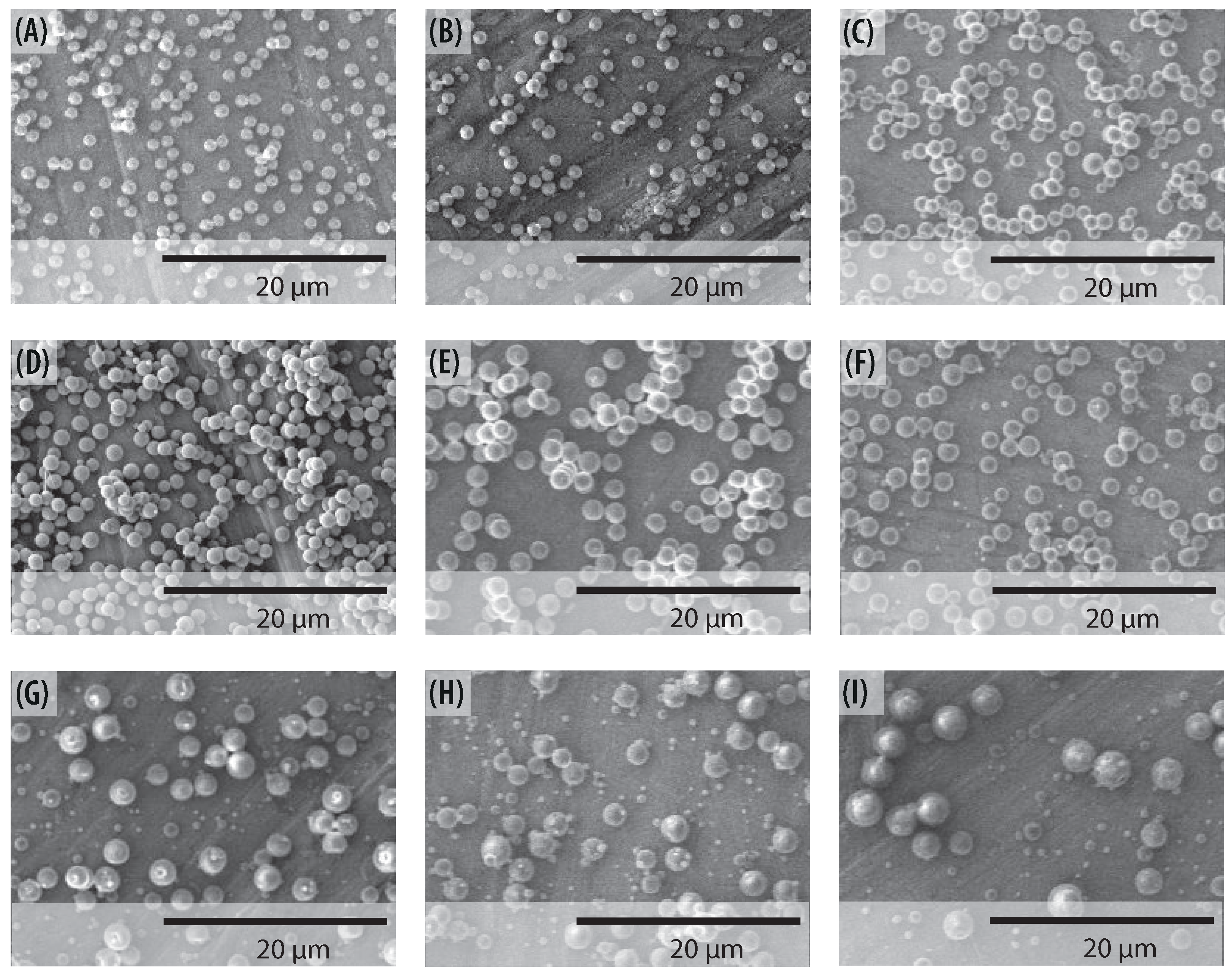
5. Fabrication of PVP Structures
6. Summary and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Wang, Q.; Wang, Z.; Yang, S.; Li, B.; Xu, H.; Yu, K.; Wang, J. Experimental study on electrohydrodynamic atomization (EHDA) in stable cone-jet with middle viscous and low conductive liquid. Exp. Therm. Fluid Sci. 2021, 121, 110260. [Google Scholar] [CrossRef]
- Xie, J.; Jiang, J.; Davoodi, P.; Srinivasan, M.P.; Wang, C.H. Electrohydrodynamic atomization: A two-decade effort to produce and process micro-/nanoparticulate materials. Chem. Eng. Sci. 2015, 125, 32–57. [Google Scholar] [CrossRef] [Green Version]
- Almería, B.; Deng, W.; Fahmy, T.M.; Gomez, A. Controlling the morphology of electrospray-generated PLGA microparticles for drug delivery. J. Colloid Interface Sci. 2010, 343, 125–133. [Google Scholar] [CrossRef] [PubMed]
- Toy, R.; Peiris, P.M.; Ghaghada, K.B.; Karathanasis, E. Shaping cancer nanomedicine: The effect of particle shape on the in vivo journey of nanoparticles. Nanomedicine 2014, 9, 121–134. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kaga, S.; Truong, N.P.; Esser, L.; Senyschyn, D.; Sanyal, A.; Sanyal, R.; Quinn, J.F.; Davis, T.P.; Kaminskas, L.M.; Whittaker, M.R. Influence of size and shape on the biodistribution of nanoparticles prepared by polymerization-induced self-assembly. Biomacromolecules 2017, 18, 3963–3970. [Google Scholar] [CrossRef] [PubMed]
- Yang, Y.; Nie, D.; Liu, Y.; Yu, M.; Gan, Y. Advances in particle shape engineering for improved drug delivery. Drug Discov. Today 2019, 24, 575–583. [Google Scholar] [CrossRef] [PubMed]
- Taylor, G.I. Disintegration of water drops in an electric field. Proc. R. Soc. London. Ser. Math. Phys. Sci. 1964, 280, 383–397. [Google Scholar]
- Ganan-Calvo, A.; Davila, J.; Barrero, A. Current and droplet size in the electrospraying of liquids. Scaling laws. J. Aerosol Sci. 1997, 28, 249–275. [Google Scholar] [CrossRef]
- Gañán-Calvo, A.M.; Rebollo-Muñoz, N.; Montanero, J. The minimum or natural rate of flow and droplet size ejected by Taylor cone–jets: Physical symmetries and scaling laws. New J. Phys. 2013, 15, 033035. [Google Scholar] [CrossRef]
- De La Mora, J.F.; Loscertales, I.G. The current emitted by highly conducting Taylor cones. J. Fluid Mech. 1994, 260, 155–184. [Google Scholar] [CrossRef]
- Ku, B.K.; Kim, S.S. Electrospray characteristics of highly viscous liquids. J. Aerosol Sci. 2002, 33, 1361–1378. [Google Scholar] [CrossRef]
- Gañán-Calvo, A.M.; López-Herrera, J.M.; Herrada, M.A.; Ramos, A.; Montanero, J.M. Review on the physics of electrospray: From electrokinetics to the operating conditions of single and coaxial Taylor cone-jets, and AC electrospray. J. Aerosol Sci. 2018, 125, 32–56. [Google Scholar] [CrossRef]
- Cloupeau, M.; Prunet-Foch, B. Electrohydrodynamic spraying functioning modes: A critical review. J. Aerosol Sci. 1994, 25, 1021–1036. [Google Scholar] [CrossRef]
- Segura, L.J.; Narváez-Muñoz, C.; Zhou, C.; Sun, H. Sketch-Based Tensor Decomposition for Non-Parametric Monitoring of Electrospinning Processes. In Proceedings of the ASME 2020 15th International Manufacturing Science and Engineering Conference, Volume 2: Manufacturing Processes; Manufacturing Systems; Nano/Micro/Meso Manufacturing; Quality and Reliability, Virtual, Online, 3 September 2020. V002T09A002. ASME. [Google Scholar]
- Nampoothiri, K.N.; Bobji, M.S.; Sen, P. Generation of micron-sized droplet streams by high frequency electric fields. Int. J. Heat Mass Transf. 2019, 145, 118709. [Google Scholar] [CrossRef]
- Gamero-Castaño, M.; Magnani, M. Numerical simulation of electrospraying in the cone-jet mode. J. Fluid Mech. 2019, 859, 247–267. [Google Scholar] [CrossRef]
- Narvaez-Muñoz, C.P.; Carrion-Matamoros, L.M.; Vizuete, K.; Debut, A.; Arroyo, C.R.; Guerrero, V.; Almeida-Naranjo, C.E.; Morales-Florez, V.; Mowbray, D.J.; Zamora-Ledezma, C. Tailoring Organic–Organic Poly (vinylpyrrolidone) Microparticles and Fibers with Multiwalled Carbon Nanotubes for Reinforced Composites. ACS Appl. Nano Mater. 2019, 2, 4302–4312. [Google Scholar] [CrossRef]
- Wang, Z.; Wang, Q.; Li, B.; Zhang, Y.; Wang, J.; Tu, J. An experimental investigation on cone-jet mode in electrohydrodynamic (EHD) atomization. Exp. Therm. Fluid Sci. 2020, 114, 110054. [Google Scholar] [CrossRef]
- Scheideler, W.J.; Chen, C.H. The minimum flow rate scaling of Taylor cone-jets issued from a nozzle. Appl. Phys. Lett. 2014, 104, 024103. [Google Scholar] [CrossRef] [Green Version]
- Gamero-Castaño, M.; Magnani, M. The minimum flow rate of electrosprays in the cone-jet mode. J. Fluid Mech. 2019, 876, 553–572. [Google Scholar] [CrossRef]
- Munir, M.M.; Suryamas, A.B.; Iskandar, F.; Okuyama, K. Scaling law on particle-to-fiber formation during electrospinning. Polymer 2009, 50, 4935–4943. [Google Scholar] [CrossRef]
- Levit, N.; Tepper, G. Supercritical CO2-assisted electrospinning. J. Supercrit. Fluids 2004, 31, 329–333. [Google Scholar] [CrossRef]
- Baldino, L.; Cardea, S.; Reverchon, E. A supercritical CO2 assisted electrohydrodynamic process used to produce microparticles and microfibers of a model polymer. J. CO2 Util. 2019, 33, 532–540. [Google Scholar] [CrossRef]
- Feng, J.; Scott, T. A computational analysis of electrohydrodynamics of a leaky dielectric drop in an electric field. J. Fluid Mech. 1996, 331, 286–326. [Google Scholar] [CrossRef]
- Hartman, R.; Brunner, D.; Camelot, D.; Marijnissen, J.; Scarlett, B. Jet Break-up in Electrohydrodynamic Atomization in the Cone-Jet Mode. J. Aerosol Sci. 2000, 31, 65–95. [Google Scholar] [CrossRef]
- Ghasemi, E.; Bararnia, H.; Soleimanikutanaei, S.; Lin, C. Direct numerical simulation and analytical modeling of electrically induced multiphase flow. Int. J. Mech. Sci. 2018, 142, 397–406. [Google Scholar] [CrossRef]
- Lim, L.; Hua, J.; Wang, C.; Smith, K. Numerical Simulation of Cone-Jet Formation in Electrohydrodynamic Atomization. Am. Inst. Chem. Eng. 2011, 57, 217–220. [Google Scholar] [CrossRef]
- Wei, W.; Gu, Z.; Wang, S.; Zhang, Y.; Lei, K.; Kase, K. Numerical Simulation of the Cone–Jet Formation and Current Generation in Electrostatic Spray—Modeling as Regards Space Charged Droplet Effect. J. Micromech. Microeng. 2013, 23, 1–11. [Google Scholar] [CrossRef]
- Xu, Q.; Qin, H.; Yin, Z.; Hua, J.; Pack, D.W.; Wang, C.H. Coaxial electrohydrodynamic atomization process for production of polymeric composite microspheres. Chem. Eng. Sci. 2013, 104, 330–346. [Google Scholar] [CrossRef] [Green Version]
- Jiang, Z.; Gan, Y.; Shi, Y. An improved model for prediction of the cone-jet formation in electrospray with the effect of space charge. J. Aerosol Sci. 2020, 139, 105463. [Google Scholar] [CrossRef]
- López-Herrera, J.M.; Herrada, M.A.; Gamero-Castaño, M.; Gañán-Calvo, A.M. A numerical simulation of coaxial electrosprays. J. Fluid Mech. 2020, 885. [Google Scholar] [CrossRef]
- Tomar, G.; Gerlach, D.; Biswas, G.; Alleborn, N.; Sharma, A.; Durst, F.; Welch, S.; Delgado, A. Two-phase electrohydrodynamic simulations using a volume-of-fluid approach. J. Comput. Phys. 2007, 227, 1267–1285. [Google Scholar] [CrossRef]
- Herrada, M.; Lopez-Herrera, J.; Ganan-Calvo, A.; Vega, E.; Montanero, J.; Popinet, S. Numerical simulation of electrospray in the cone-jet mode. Phys. Rev. E 2012, 86, 026305. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Dastourani, H.; Jahannama, M.; Eslami-Majd, A. A physical insight into electrospray process in cone-jet mode: Role of operating parameters. Int. J. Heat Fluid Flow 2018, 70, 315–335. [Google Scholar] [CrossRef]
- Hirt, C.W.; Nichols, B.D. Volume of fluid (VOF) method for the dynamics of free boundaries. J. Comput. Phys. 1981, 39, 201–225. [Google Scholar] [CrossRef]
- Osher, S.; Fedkiw, R.; Piechor, K. Level set methods and dynamic implicit surfaces. Appl. Mech. Rev. 2004, 57, B15. [Google Scholar] [CrossRef] [Green Version]
- Bjørklund, E. The level-set method applied to droplet dynamics in the presence of an electric field. Comput. Fluids 2009, 38, 358–369. [Google Scholar] [CrossRef]
- Hashemi, M.R.; Ryzhakov, P.; Rossi, R. An enriched finite element/level-set method for simulating two-phase incompressible fluid flows with surface tension. Comput. Methods Appl. Mech. Eng. 2020, 370, 113277. [Google Scholar] [CrossRef]
- Ryzhakov, P.B.; Jarauta, A. An embedded approach for immiscible multi-fluid problems. Int. J. Numer. Methods Fluids 2016, 81, 357–376. [Google Scholar] [CrossRef] [Green Version]
- Jarauta, A.; Ryzhakov, P.; Secanell, M.; Waghmare, P.R.; Pons-Prats, J. Numerical study of droplet dynamics in a polymer electrolyte fuel cell gas channel using an embedded Eulerian-Lagrangian approach. J. Power Sources 2016, 323, 201–212. [Google Scholar] [CrossRef] [Green Version]
- Ryzhakov, P.B.; Jarauta, A.; Secanell, M.; Pons-Prats, J. On the application of the PFEM to droplet dynamics modeling in fuel cells. Comput. Part. Mech. 2017, 4, 285–295. [Google Scholar] [CrossRef] [Green Version]
- Chen, C. Electrohydrodynamic Stability. In Electrokinetics and Electrohydrodynamics in Microsystems; International Centre for Mechanical Sciences: Udine, Italy, 2011; Volume 530, pp. 144–220. [Google Scholar]
- Melcher, J. Continuum Electromechanics; MIT Press: Cambridge, MA, USA, 1981. [Google Scholar]
- Melcher, J. Electric fields and moving media. IEEE Trans. Educ. 1974, 17, 100–110. [Google Scholar] [CrossRef]
- Hua, L.L.J.; Wang, C. Numerical simualtion of deforemation/motion of a drop suspended in viscous liquids under of steady electric fields. Phys. Fluids 2008, 20, 1–16. [Google Scholar] [CrossRef] [Green Version]
- Saville, D. Electrohydrodynamics: The Taylor-Melcher Leaky Dielectric Model. Annu. Rev. Fluid Mech. 1997, 29, 27–64. [Google Scholar] [CrossRef]
- Wilhelmy, L. Ueber die Abhängigkeit der Capillaritäts-Constanten des Alkohols von Substanz und Gestalt des benetzten festen Körpers. Ann. Phys. 1863, 195, 177–217. [Google Scholar] [CrossRef] [Green Version]
- la Mora, J.F.D. The Fluid Dynamics of Taylor Cones. Annu. Rev. Fluid Mech. 2007, 39, 217–243. [Google Scholar] [CrossRef]
- Rosell-Llompart, J.; Grifoll, J.; Loscertales, I.G. Electrosprays in the cone-jet mode: From Taylor cone formation to spray development. J. Aerosol Sci. 2018, 125, 2–31. [Google Scholar] [CrossRef]
- Yarin, A.L.; Koombhongse, S.; Reneker, D.H. Taylor cone and jetting from liquid droplets in electrospinning of nanofibers. J. Appl. Phys. 2001, 90, 4836–4846. [Google Scholar] [CrossRef] [Green Version]
- Michelson, D. Electrostatic Atomization; Hilger, A., Ed.; CRC Press: Boca Raton, FL, USA, 1990. [Google Scholar]
- Park, C.H.; Lee, J. Electrosprayed polymer particles: Effect of the solvent properties. J. Appl. Polym. Sci. 2009, 114, 430–437. [Google Scholar] [CrossRef]
- Roghair, I.; Musterd, M.; van den Ende, D.; Kleijn, C.; Kreutzer, M.; Mugele, F. A numerical technique to simulate display pixels based on electrowetting. Microfluid. Nanofluid. 2015, 19, 465–482. [Google Scholar] [CrossRef] [Green Version]
- Deshpande, S.S.; Anumolu, L.; Trujillo, M.F. Evaluating the performance of the two-phase flow solver interFoam. Comput. Sci. Discov. 2012, 5, 014016. [Google Scholar] [CrossRef]
- Narvaez-Munoz, C. Computational Modelling of Electrohydrodynamic Atomization. Master’s Thesis, The University of Manchester, Manchester, UK, 2015. [Google Scholar]
- Gomez, A.; Tang, K. Charge and fission of droplets in electrostatic sprays. Phys. Fluids 1994, 6, 404–414. [Google Scholar] [CrossRef]
- Rosell-Llompart, J.; De La Mora, J.F. Generation of monodisperse droplets 0.3 to 4 m in diameter from electrified cone-jets of highly conducting and viscous liquids. J. Aerosol Sci. 1994, 25, 1093–1119. [Google Scholar] [CrossRef]
- Smith, D. The Electrohydrodynamic Atomization of Liquids. IEEE Trans. Ind. Appl. 1986, IA-22, 527–535. [Google Scholar] [CrossRef]
- Morais, A.Í.; Vieira, E.G.; Afewerki, S.; Sousa, R.B.; Honorio, L.; Cambrussi, A.N.; Santos, J.A.; Bezerra, R.D.; Furtini, J.A.; Silva-Filho, E.C.; et al. Fabrication of Polymeric Microparticles by Electrospray: The Impact of Experimental Parameters. J. Funct. Biomater. 2020, 11, 4. [Google Scholar] [CrossRef] [Green Version]
- Higuera, F.J. Electric Field Effects. Fluids Colloids Soft Mater. Introd. Soft Matter Phys. 2016, 19–28. [Google Scholar] [CrossRef]
- Barrero, A.; Ganan-Calvo, A.; Davila, J.; Palacio, A.; Gómez-González, E. Low and high Reynolds number flows inside Taylor cones. Phys. Rev. E 1998, 58, 7309. [Google Scholar] [CrossRef]
- Cloupeau, M.; Prunet-Foch, B. Electrostatic spraying of liquids in cone-jet mode. J. Electrost. 1989, 22, 135–159. [Google Scholar] [CrossRef]
- la Mora, J.F.D. On the Outcome of the Coulombic Fission of a Charged Isolated Drop. J. Colloid Interface Sci. 1996, 178, 209–218. [Google Scholar] [CrossRef]
- Morris, T.; Malardier-Jugroot, C.; Jugroot, M. Characterization of electrospray beams for micro-spacecraft electric propulsion applications. J. Electrost. 2013, 71, 931–938. [Google Scholar] [CrossRef]
- Jones, A.; Thong, K. The production of charged monodisperse fuel droplets by electrical dispersion. J. Phys. Appl. Phys. 1971, 4, 1159. [Google Scholar] [CrossRef]
- Krpoun, R. Micromachined Electrospray Thrusters for Spacecraft Propulsion; Technical Report; EPFL: Lausanne, Switzerland, 2009. [Google Scholar]
- Naderi, P.; Shams, M.; Ghassemi, H. Investigation on the onset voltage and stability island of electrospray in the cone-jet mode using curved counter electrode. J. Electrost. 2019, 98, 1–10. [Google Scholar] [CrossRef]
- Hohman, M.; Shin, M.; Rutledge, G.; Brenner, M. Electrospinning and electrically forced jets. I. Stability theory. Phys. Fluids 2001, 13, 2201–2220. [Google Scholar] [CrossRef] [Green Version]
- Tripatanasuwan, S.; Zhong, Z.; Reneker, D.H. Effect of evaporation and solidification of the charged jet in electrospinning of poly (ethylene oxide) aqueous solution. Polymer 2007, 48, 5742–5746. [Google Scholar] [CrossRef]







| PVP wt% | (μS/cm) | (mN/m) | (mPa.s) | (kg/m) |
|---|---|---|---|---|
| 10 | 52.4 | 36.99 | 5.3 | 965 |
| 13 | 52.6 | 37.17 | 9.4 | 990 |
| 15 | 54 | 37.24 | 12.51 | 1001 |
| 18 | 56 | 37.77 | 18.48 | 1015 |
| 20 | 57.4 | 37.88 | 23.3 | 1028 |
| 23 | 59.4 | 38.02 | 24.6 | 1056 |
| 25 | 60.8 | 38.18 | 38.5 | 1067 |
| 28 | 62.1 | 38.41 | 84.8 | 1092 |
| 30 | 63 | 38.65 | 183.6 | 1158 |
| Boundary | U | p | ||
|---|---|---|---|---|
| a–b | U = fixed value | Fixedfluxpressure | N/a | ∇ = 0 |
| b–c | U = 0 | Fixedfluxpressure | ∇ = 0 | |
| b–d | zeroGradient | p = 0 | N/a | ∇ = 0 |
| d–e | zeroGradient | p = 0 | N/a | ∇ = 0 |
| e–f | zeroGradient | Fixedfluxpressure | 0 | ∇ = 0 |
| f–a | zeroGradient | Fixedfluxpressure | N/a | ∇ = 0 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Narváez-Muñoz, C.; Ryzhakov, P.; Pons-Prats, J. Determination of the Operational Parameters for the Manufacturing of Spherical PVP Particles via Electrospray. Polymers 2021, 13, 529. https://doi.org/10.3390/polym13040529
Narváez-Muñoz C, Ryzhakov P, Pons-Prats J. Determination of the Operational Parameters for the Manufacturing of Spherical PVP Particles via Electrospray. Polymers. 2021; 13(4):529. https://doi.org/10.3390/polym13040529
Chicago/Turabian StyleNarváez-Muñoz, Christian, Pavel Ryzhakov, and Jordi Pons-Prats. 2021. "Determination of the Operational Parameters for the Manufacturing of Spherical PVP Particles via Electrospray" Polymers 13, no. 4: 529. https://doi.org/10.3390/polym13040529
APA StyleNarváez-Muñoz, C., Ryzhakov, P., & Pons-Prats, J. (2021). Determination of the Operational Parameters for the Manufacturing of Spherical PVP Particles via Electrospray. Polymers, 13(4), 529. https://doi.org/10.3390/polym13040529








