Finite Element Modelling and Experimental Validation of Scratches on Textured Polymer Surfaces
Abstract
:1. Introduction
2. Experimental
3. Textured Surface Model and Scratch Modelling
3.1. Textured Surfaces
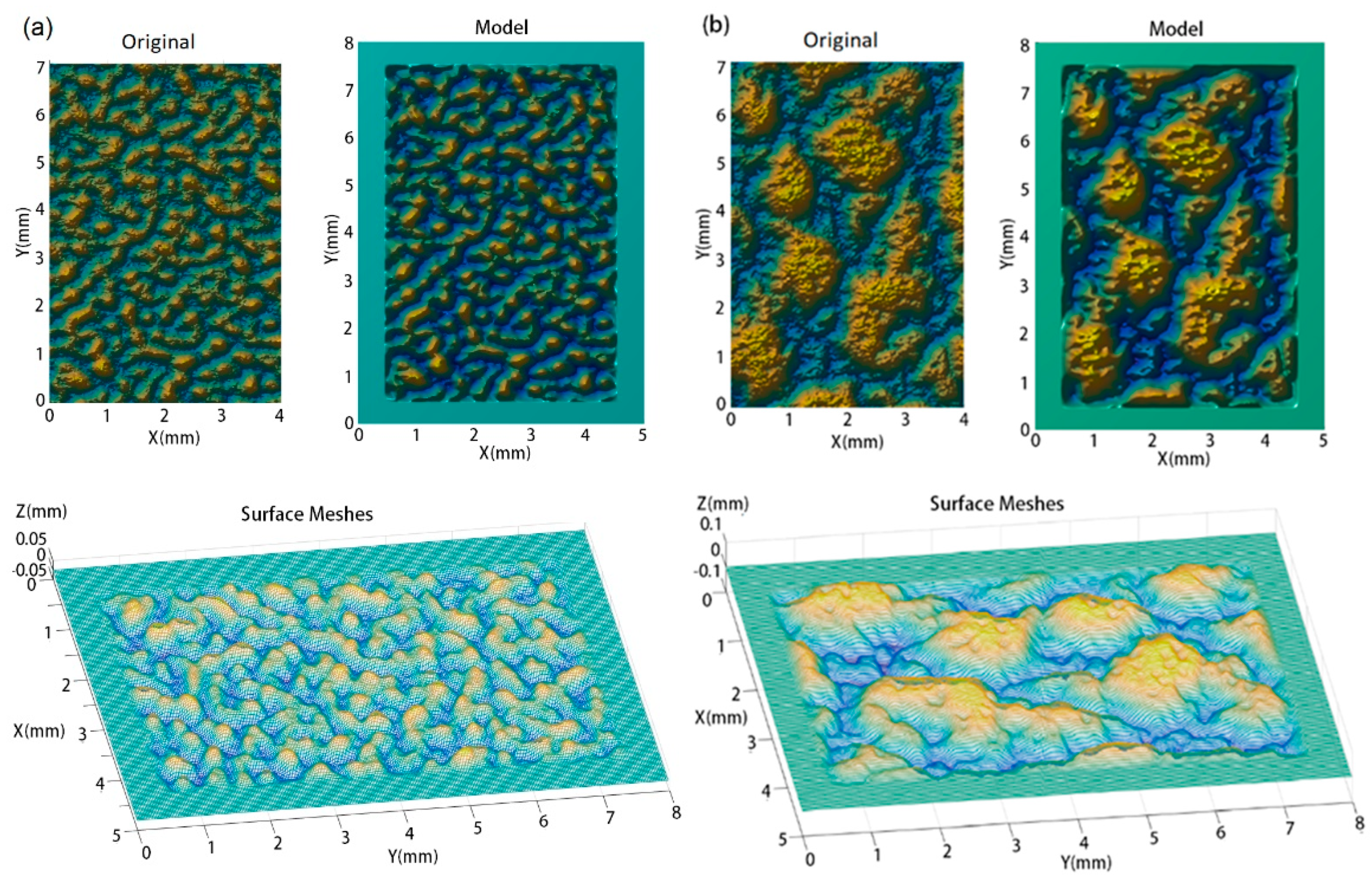
3.2. Surface Models
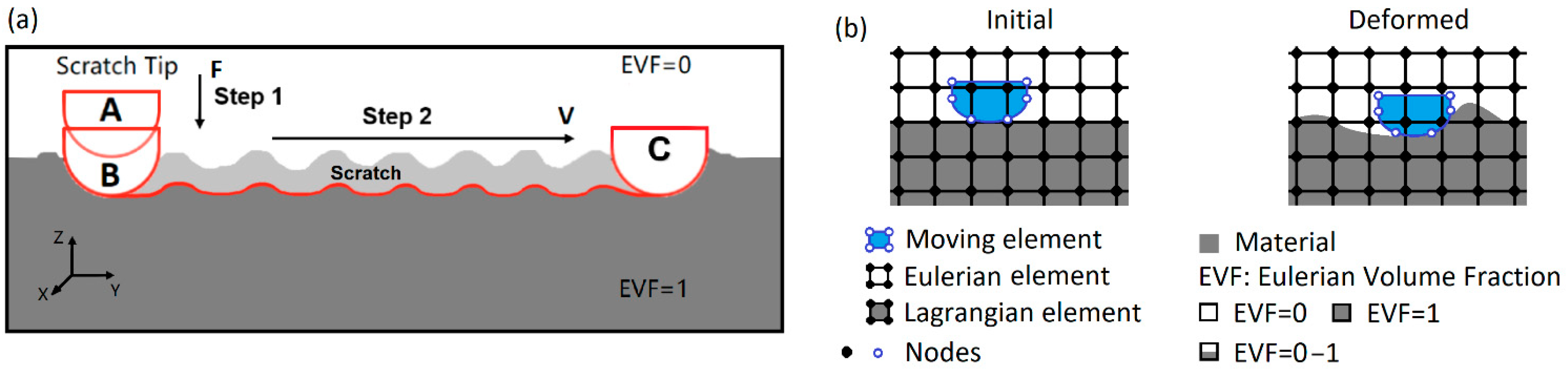
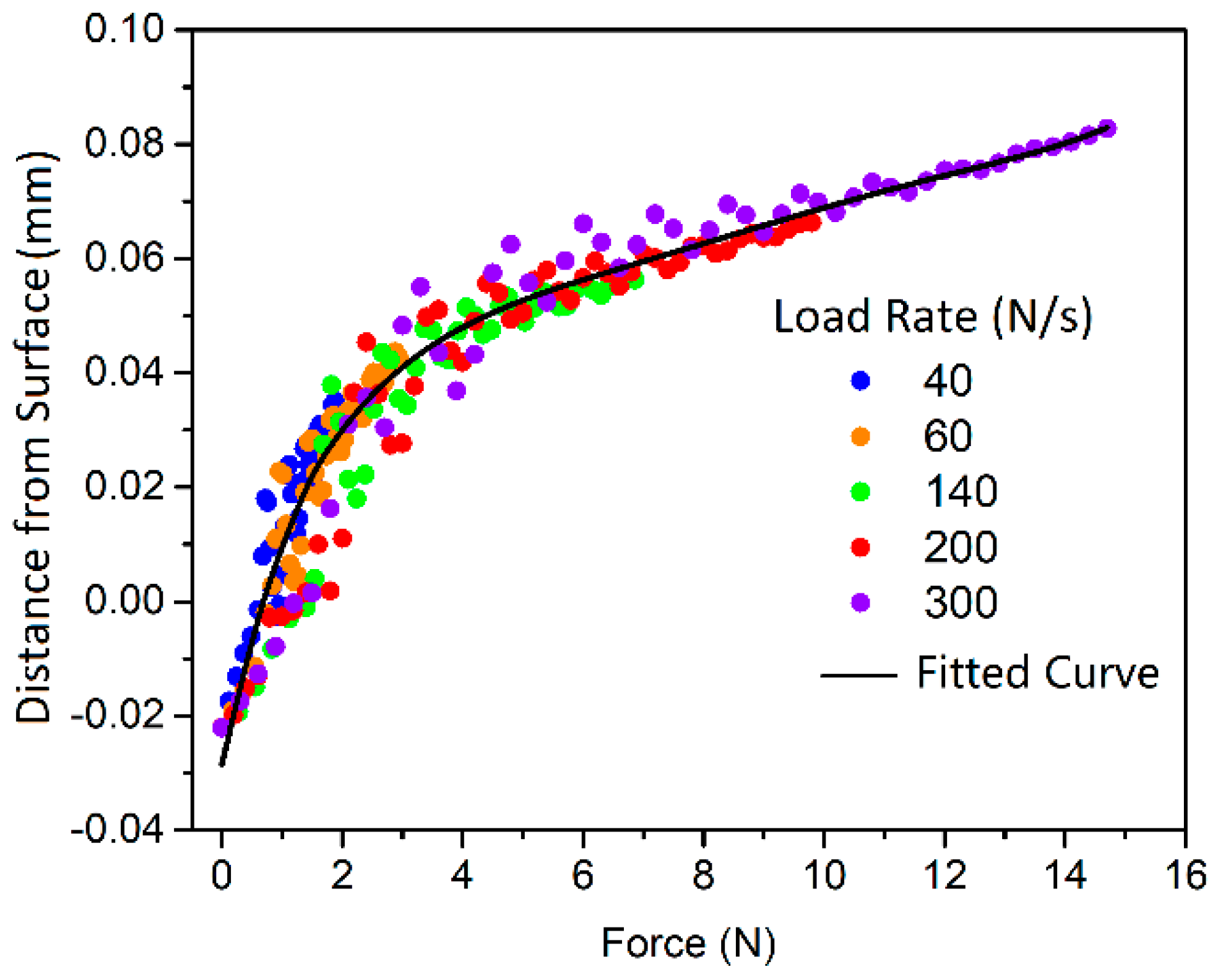
3.3. Scratch Modelling
4. Results and Discussion
4.1. Features of Modelled Texture Surfaces
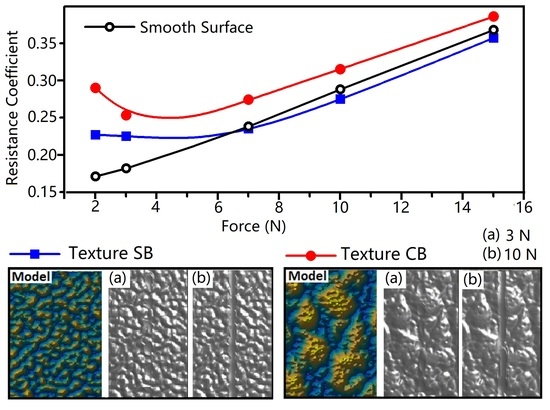
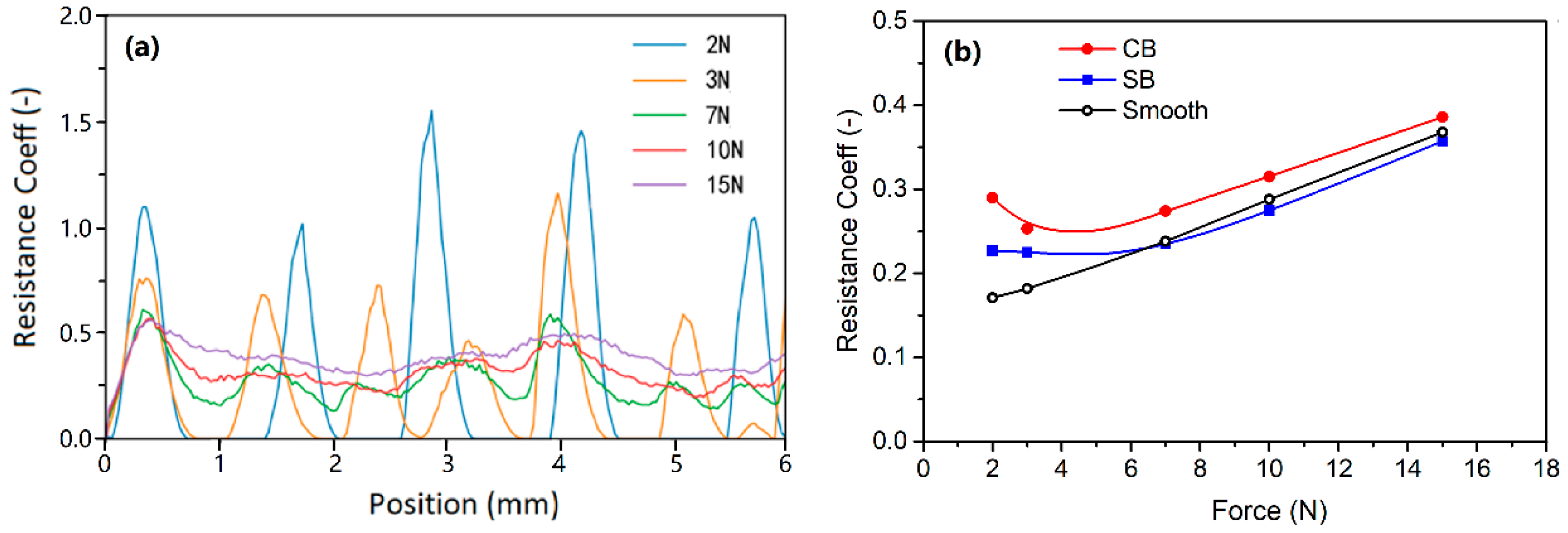
4.2. Indentation and Scratch Resistance
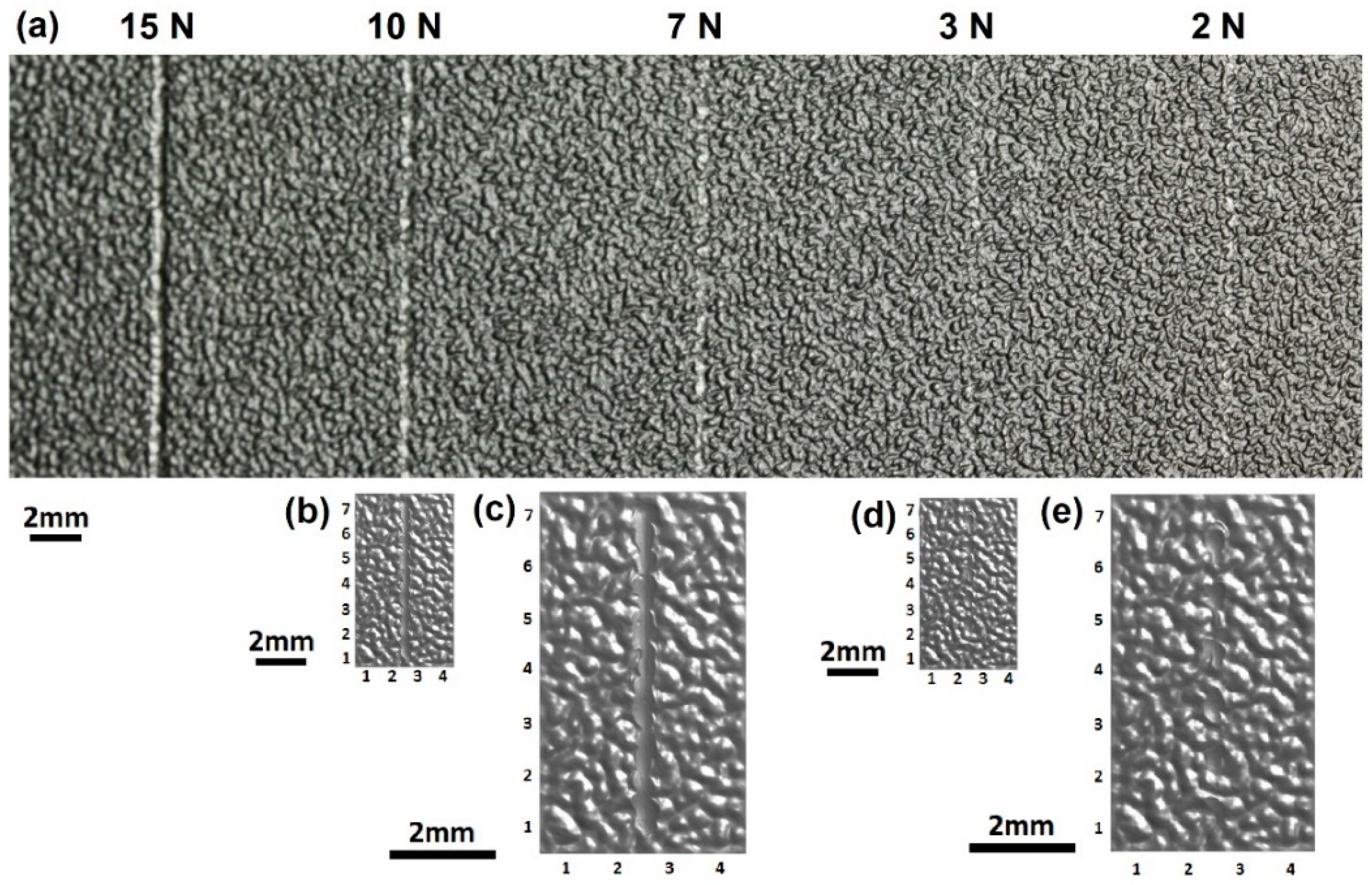
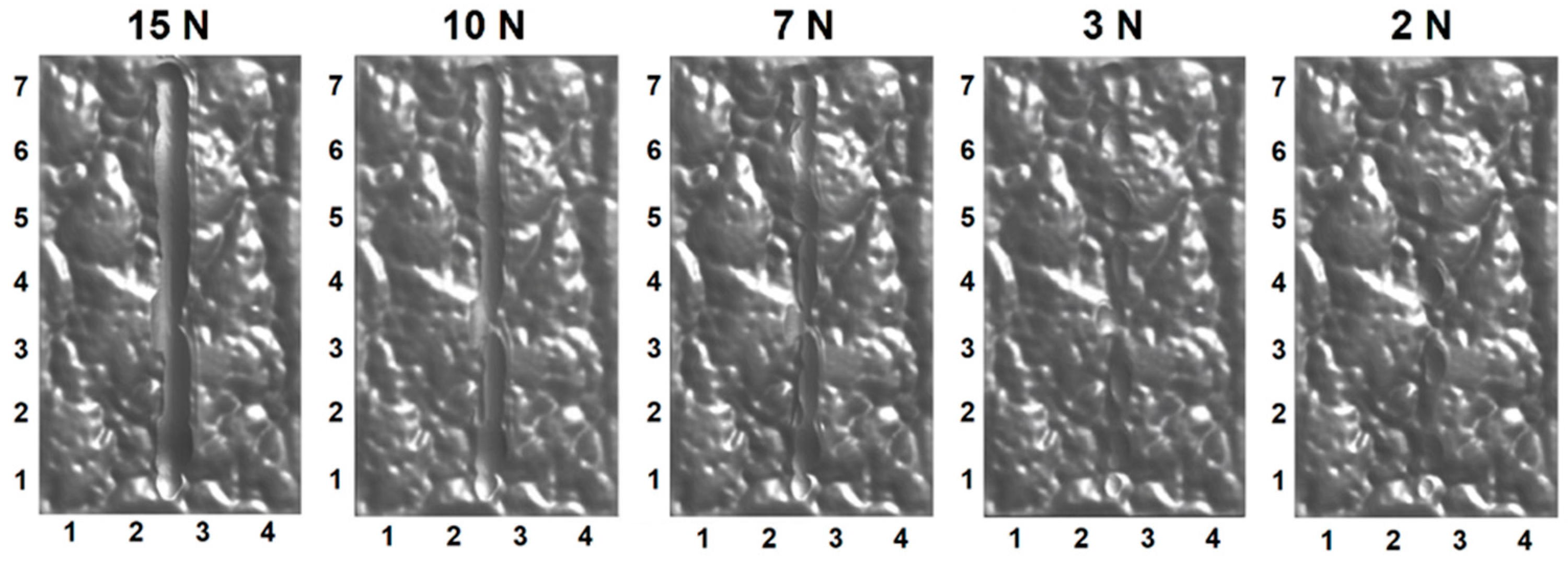
4.3. Surface Appearance
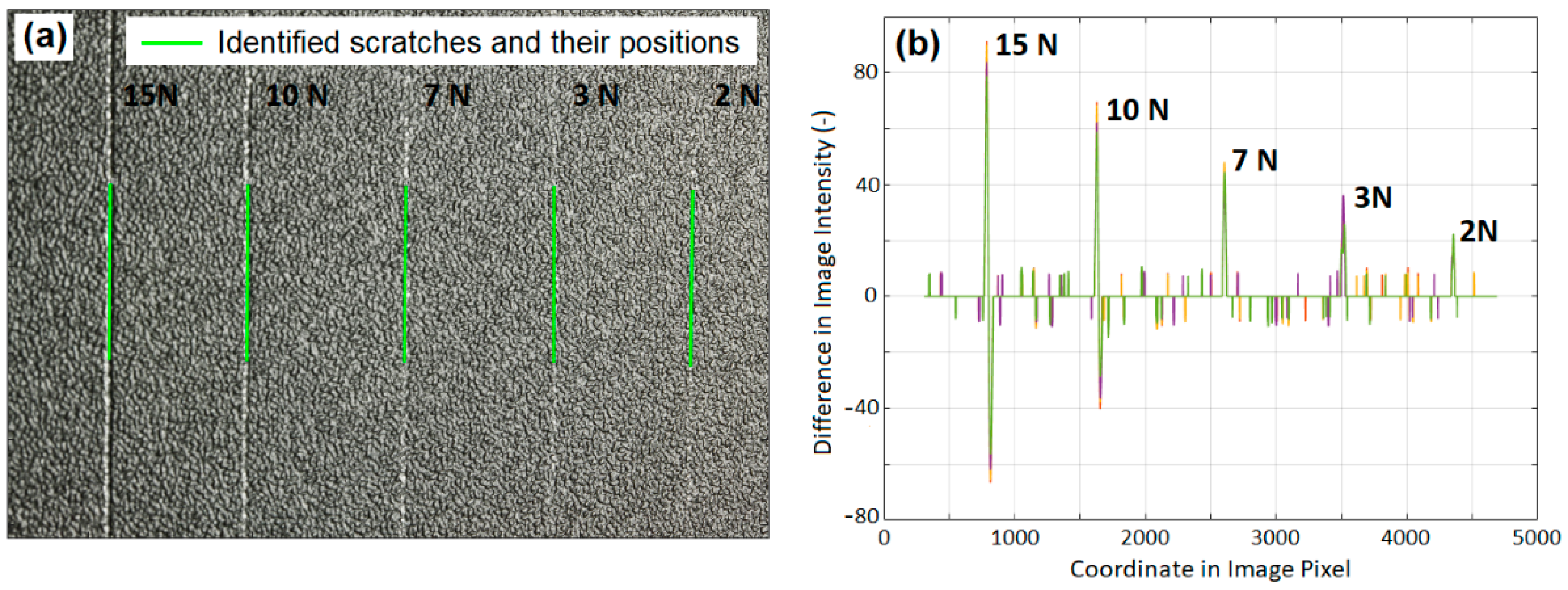
4.4. Surface Damage and Scratch Size
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Grützmacher, P.G.; Profito, F.J.; Rosenkranz, A. Multi-Scale Surface Texturing in Tribology—Current Knowledge and Future Perspectives. Lubricants 2019, 7, 95. [Google Scholar] [CrossRef] [Green Version]
- Zheng, X.F.; Wang, Z.W.; Chen, M.W.; Zheng, H.H.; Wu, J.W. A Review of Surface Texture of Tribological Interfaces. Appl. Mech. Mater. 2010, 37–38, 41–45. [Google Scholar] [CrossRef]
- Rosenkranz, A.; Grützmacher, P.G.; Murzyn, K.; Mathieu, C.; Mücklich, F. Multi-scale surface patterning to tune friction under mixed lubricated conditions. Appl. Nanosci. 2021, 11, 751–762. [Google Scholar] [CrossRef]
- Arslan, A.; Masjuki, H.H.; Kalam, M.A.; Varman, M.; Mufti, R.A.; Mosarof, M.H.; Khuong, L.S.; Quazi, M.M. Surface Texture Manufacturing Techniques and Tribological Effect of Surface Texturing on Cutting Tool Performance: A Review. Crit. Rev. Solid State Mater. Sci. 2016, 41, 447–481. [Google Scholar] [CrossRef]
- Rosenkranz, A.; Grützmacher, P.G.; Gachot, C.; Costa, H.L. Surface Texturing in Machine Elements—A Critical Discussion for Rolling and Sliding Contacts. Adv. Eng. Mater. 2019, 21, 1900194. [Google Scholar] [CrossRef]
- Coblas, D.; Fatu, A.; Maoui, A.; Hajjam, M. Manufacturing textured surfaces: State of art and recent developments. J. Eng. Tribol. 2014, 229. [Google Scholar] [CrossRef]
- Kim, M.S.; Lee, J.H.; Kwak, M.K. Review: Surface Texturing Methods for Solar Cell Efficiency Enhancement. Int. J. Precis. Eng. Manuf. 2020, 21, 1389–1398. [Google Scholar] [CrossRef]
- Zhang, J.-J.; Zhao, L.; Rosenkranz, A.; Song, C.-W.; Yan, Y.-D.; Sun, T. Nanosecond Pulsed Laser Ablation on Stainless Steel—Combining Finite Element Modeling and Experimental Work. Adv. Eng. Mater. 2019, 21, 1900193. [Google Scholar] [CrossRef]
- Zhang, J.; Zhao, L.; Rosenkranz, A.; Song, C.; Yan, Y.; Sun, T. Nanosecond pulsed laser ablation of silicon-finite element simulation and experimental validation. J. Micromech. Microeng. 2019, 29, 75009. [Google Scholar] [CrossRef]
- Schneider, J.; Djamiykov, V.; Greiner, C. Friction reduction through biologically inspired scale-like laser surface textures. Beilstein J. Nanotechnol. 2018, 9, 2561. [Google Scholar] [CrossRef]
- Ignell, S.; Kleist, U.; Rigdahl, M. Visual perception and measurements of texture and gloss of injection-molded plastics. Polym. Eng. Sci. 2009, 49, 344–353. [Google Scholar] [CrossRef]
- Piccolo, L.; Sorgato, M.; Batal, A.; Dimov, S.; Lucchetta, G.; Masato, D. Functionalization of Plastic Parts by Replication of Variable Pitch Laser-Induced Periodic Surface Structures. Micromachines 2020, 11, 429. [Google Scholar] [CrossRef] [Green Version]
- Barr, C.J.; Wang, L.; Coffey, J.K.; Daver, F. Influence of surface texturing on scratch/mar visibility for polymeric materials: A review. J. Mater. Sci. 2017, 52, 1221–1234. [Google Scholar] [CrossRef]
- De Vietro, N.; Belforte, L.; Lambertini, V.G.; Fracassi, F. Low pressure plasma modified polycarbonate: A transparent, low reflective and scratch resistant material for automotive applications. Appl. Surf. Sci. 2014, 307, 698–703. [Google Scholar] [CrossRef]
- Barr, C.J.; Wang, L.; Coffey, J.K.; Gidley, A.; Daver, F. New technique for the quantification of scratch visibility on polymeric textured surfaces. Wear 2017, 384–385, 84–94. [Google Scholar] [CrossRef]
- Hamdi, M.; Sue, H.-J. Effect of color, gloss, and surface texture perception on scratch and mar visibility in polymers. Mater. Des. 2015, 83, 528–535. [Google Scholar] [CrossRef]
- Hossain, M.M.; Jiang, H.; Sue, H.-J. Effect of constitutive behavior on scratch visibility resistance of polymers—A finite element method parametric study. Wear 2011, 270, 751–759. [Google Scholar] [CrossRef]
- Jiang, H.; Browning, R.L.; Hossain, M.M.; Sue, H.-J.; Fujiwara, M. Quantitative evaluation of scratch visibility resistance of polymers. Appl. Surf. Sci. 2010, 256, 6324–6329. [Google Scholar] [CrossRef]
- Wong, M.; Moyse, A.; Lee, F.; Sue, H.-J. Study of surface damage of polypropylene under progressive loading. J. Mater. Sci. 2004, 39, 3293–3308. [Google Scholar] [CrossRef]
- Ni, B.Y.; Faou, A.L. Scratching behaviour of polymer films using blunt spherical styli. J. Mater. Sci. 1996, 31, 3955–3963. [Google Scholar] [CrossRef]
- Ali, S.H.R. Advanced Nanomeasuring Techniques for Surface Characterization. ISRN Opt. 2012, 2012, 23. [Google Scholar] [CrossRef] [Green Version]
- Chivatanasoontorn, V.; Tsukise, S.; Kotaki, M. Surface texture effect on scratch behavior of injection molded plastics. Polym. Eng. Sci. 2012, 52, 1862–1867. [Google Scholar] [CrossRef]
- Le Houérou, V.; Gauthier, C.; Schirrer, R. Energy based model to assess interfacial adhesion using a scratch test. J. Mater. Sci. 2008, 43, 5747–5754. [Google Scholar] [CrossRef]
- Cheng, Q.; Jiang, C.; Zhang, J.; Yang, Z.; Zhu, Z.; Jiang, H. Effect of thermal aging on the scratch behavior of poly (methyl methacrylate). Tribol. Int. 2016, 101, 110–114. [Google Scholar] [CrossRef]
- Zhu, Z.; Cheng, Q.; Jiang, C.; Zhang, J.; Jiang, H. Scratch behavior of the aged hydrogenated nitrile butadiene rubber. Wear 2016, 352–353, 155–159. [Google Scholar] [CrossRef]
- Bouvard, J.L.; Denton, B.; Freire, L.; Horstemeyer, M.F. Modeling the mechanical behavior and impact properties of polypropylene and copolymer polypropylene. J. Polym. Res. 2016, 23, 1–19. [Google Scholar] [CrossRef]
- Şerban, D.-A.; Marşavina, L.; Modler, N. Finite element modelling of the progressive damage and failure of thermoplastic polymers in puncture impact. Procedia Eng. 2015, 109, 97–104. [Google Scholar] [CrossRef] [Green Version]
- Alemi-Ardakani, M.; Milani, A.S.; Yannacopoulos, S. On Complexities of Impact Simulation of Fiber Reinforced Polymer Composites: A Simplified Modeling Framework. Sci. World J. 2014, 2014, 10. [Google Scholar] [CrossRef] [Green Version]
- Jiang, H.; Wei, Y.; Cheng, Q.; Zhu, Z. Scratch behavior of low density polyethylene film: Effects of pre-stretch and aging. Mater. Des. 2018, 157, 235–243. [Google Scholar] [CrossRef]
- Gao, W.M.; Wang, L.; Coffey, J.K.; Daver, F. Finite element simulation of scratch on polypropylene panels. Mater. Des. 2018, 140, 400–408. [Google Scholar] [CrossRef]
- Jiang, H.; Browning, R.; Sue, H.-J. Understanding of scratch-induced damage mechanisms in polymers. Polymer 2009, 50, 4056–4065. [Google Scholar] [CrossRef]
- Bucaille, J.L.; Gauthier, C.; Felder, E.; Schirrer, R. The influence of strain hardening of polymers on the piling-up phenomenon in scratch tests: Experiments and numerical modelling. Wear 2006, 260, 803–814. [Google Scholar] [CrossRef]
- Jiang, H.; Lim, G.T.; Reddy, J.N.; Whitcomb, J.D.; Sue, H.J. Finite element method parametric study on scratch behavior of polymers. J. Polym. Sci. Part B Pol. Phys. 2007, 45, 1435–1447. [Google Scholar] [CrossRef]
- Hossain, M.M.; Browning, R.; Minkwitz, R.; Sue, H.-J. Effect of asymmetric constitutive behavior on scratch-induced deformation of polymers. Tribol. Lett. 2012, 47, 113–122. [Google Scholar] [CrossRef]
- Hossain, M.M.; Minkwitz, R.; Charoensirisomboon, P.; Sue, H.-J. Quantitative modeling of scratch-induced deformation in amorphous polymers. Polymer 2014, 55, 6152–6166. [Google Scholar] [CrossRef]
- Zhang, J.; Jiang, H.; Jiang, C.; Kang, G.; Kan, Q.; Li, Y. Experimental and numerical investigations of evaluation criteria and material parameters’ coupling effect on polypropylene scratch. Polym. Eng. Sci. 2017, 118–122. [Google Scholar] [CrossRef]
- Yeo, N.E.; Cho, W.K.; Kim, D.-I.; Jeong, M.Y. Enhanced anti-scratch performance of nanopatterned anti-reflective polymer films. Appl. Surf. Sci. 2018, 458, 503–511. [Google Scholar] [CrossRef]
- Friedrich, K.; Sue, H.J.; Liu, P.; Almajid, A.A. Scratch resistance of high performance polymers. Tribol. Int. 2011, 44, 1032–1046. [Google Scholar] [CrossRef]
- Gao, W.M.; Wang, L.; Coffey, J.K.; Daver, F. Understanding the scratch behaviour of polymeric materials with surface texture. Mater. Des. 2018, 146, 38–48. [Google Scholar] [CrossRef]
- Browning, R.; Hossain, M.M.; Li, J.; Jones, S.; Sue, H.-J. Contrast-based evaluation of mar resistance of thermoplastic olefins. Tribol. Int. 2011, 44, 1024–1031. [Google Scholar] [CrossRef]
- Yilbas, B.S.; Khaled, M.; Abu-Dheir, N.; Al-Aqeeli, N.; Said, S.A.M.; Ahmed, A.O.M.; Varanasi, K.K.; Toumi, Y.K. Wetting and other physical characteristics of polycarbonate surface textured using laser ablation. Appl. Surf. Sci. 2014, 320, 21–29. [Google Scholar] [CrossRef]
- Feng, B. Effects of surface roughness on scratch resistance and stress-strain fields during scratch tests. AIP Adv. 2017, 7, 035217. [Google Scholar] [CrossRef] [Green Version]
- Jiang, H.; Browning, R.; Fincher, J.; Gasbarro, A.; Jones, S.; Sue, H.-J. Influence of surface roughness and contact load on friction coefficient and scratch behavior of thermoplastic olefins. Appl. Surf. Sci. 2008, 254, 4494–4499. [Google Scholar] [CrossRef]
- Jiang, C.; Jiang, H.; Zhang, J.; Kang, G. Analytical model of friction behavior during polymer scratching with conical tip. Friction 2019, 7, 466–478. [Google Scholar] [CrossRef] [Green Version]
- Yastrebov, V.A.; Durand, J.; Proudhon, H.; Cailletaud, G. Rough surface contact analysis by means of the Finite Element Method and of a new reduced model. C. R. Méc. 2011, 339, 473–490. [Google Scholar] [CrossRef]
- Yu, C.; Yu, H.; Liu, G.; Chen, W.; He, B.; Wang, Q.J. Understanding Topographic Dependence of Friction with Micro- and Nano-Grooved Surfaces. Tribol. Lett. 2014, 53, 145–156. [Google Scholar] [CrossRef]
- Pelletier, H.; Gauthier, C.; Schirrer, R. Strain and stress fields during scratch tests on amorphous polymers: Influence of the local friction. Tribol. Lett. 2008, 32, 109–116. [Google Scholar] [CrossRef]
- Jiang, H.; Cheng, Q.; Jiang, C.; Zhang, J.; Yonghua, L. Effect of stick-slip on the scratch performance of polypropylene. Tribol. Int. 2015, 91, 1–5. [Google Scholar] [CrossRef]
- Chu, J.; Rumao, L.; Coleman, B. Scratch and mar resistance of filled polypropylene materials. Polym. Eng. Sci. 1998, 38, 1906–1914. [Google Scholar] [CrossRef]
- Weon, J.-I.; Song, S.-Y.; Choi, K.-Y.; Lee, S.-G.; Lee, J.H. Quantitative determination of scratch-induced damage visibility on polymer surfaces. J. Mater. Sci. 2010, 45, 2649–2654. [Google Scholar] [CrossRef]
- Gao, W.; Wang, L.; Coffey, J.K.; Wu, H.; Daver, F. An attempt to simulate structure and realistic images of scratches on rough polymeric surfaces. J. Polym. Sci. 2020, 58, 3209–3218. [Google Scholar] [CrossRef]
- Yastrebov, V.A.; Anciaux, G.; Molinari, J.-F. On the accurate computation of the true contact-area in mechanical contact of random rough surfaces. Tribol. Int. 2017, 114, 161–171. [Google Scholar] [CrossRef] [Green Version]
- Roberts, M. Biology: A Functional Approach, 4th ed.; Oxford University Press: Oxford, UK, 1986. [Google Scholar]
- Buchsbaum, W.H. Color. TV Servicing, 3rd ed.; Prentice Hall: Bergen County, NJ, USA, 1975. [Google Scholar]
- Pappas, T.N.; Neuhoff, D.L.; Ridder, H.d.; Zujovic, J. Image Analysis: Focus on Texture Similarity. Proc. IEEE 2013, 101, 2044–2057. [Google Scholar] [CrossRef]
- Zhou, W.; Bovik, A.C.; Sheikh, H.R.; Simoncelli, E.P. Image quality assessment: From error visibility to structural similarity. IEEE Trans. Image Process. 2004, 13, 600–612. [Google Scholar] [CrossRef] [Green Version]
- Mwema, F.M.; Akinlabi, E.T.; Oladijo, O.P.; Oladijo, O.P. The Use of Power Spectrum Density for Surface Characterization of Thin Films. In Photoenergy and Thin Film Materials; Scrivener Publishing LLC: Beverly, MA, USA, 2019; pp. 379–411. [Google Scholar] [CrossRef]
- Ariño, I.; Kleist, U.; Barros, G.G.; Johansson, P.-Å.; Rigdahl, M. Surface texture characterization of injection-molded pigmented plastics. Polym. Eng. Sci. 2004, 44, 1615–1626. [Google Scholar] [CrossRef]
- Tevis, D.B.J.; Till, J.; Lars, P. Quantitative characterization of surface topography using spectral analysis. Surf. Topogr. 2017, 5, 013001. [Google Scholar]
- Zhao, Y.; Wang, G.C.; Lu, T.-M. Characterization of Amorphous and Crystalline Rough Surface: Principles and Applications; Elsevier Science Publishing Co Inc.: Amsterdam, The Netherlands, 2001; Volume 37, p. 15. [Google Scholar]
- Mandelbrot, B.B. The Fractal Geometry of Nature; W.H. Freeman: New York, NY, USA, 1983; Volume 173. [Google Scholar]
- Thompson, M.K.; Thompson, J.M. Considerations for the incorporation of measured surfaces in finite element models. Scanning 2010, 32, 183–198. [Google Scholar] [CrossRef]
- ABAQUS Abaqus 6.13. User’s Guide; Dassault Systèmes Simulia: Providence, RI, USA, 2013. [Google Scholar]
- Hurtado, J.; Lapczyk, I.; Govindarajan, S. Parallel rheological framework to model non-linear viscoelasticity, permanent set and Mullins effect in elastomers. Const. Models Rubber 2013, 8, 95–100. [Google Scholar]
- Bergström, J. 11—Material Modeling Case Studies. In Mechanics of Solid Polymers; Bergström, J., Ed.; William Andrew Publishing: Amsterdam, The Netherlands, 2015; pp. 455–497. [Google Scholar] [CrossRef]
- Qiu, G.; Henke, S.; Grabe, J. Application of a Coupled Eulerian–Lagrangian approach on geomechanical problems involving large deformations. Comput. Geotech. 2011, 38, 30–39. [Google Scholar] [CrossRef]
- Ducobu, F.; Rivière-Lorphèvre, E.; Filippi, E. Application of the Coupled Eulerian-Lagrangian (CEL) method to the modeling of orthogonal cutting. Eur. J. Mech. A-Solid 2016, 59, 58–66. [Google Scholar] [CrossRef]
- Benson, D.J. Computational methods in Lagrangian and Eulerian hydrocodes. Comput. Methods Appl. Mech. Eng. 1992, 99, 235–394. [Google Scholar] [CrossRef]
- Gullerud, A.; Hollenshead, J. Coupled Euler-Lagrange simulations of metal fragmentation in pipe bomb configurations. Procedia Eng. 2017, 204, 202–207. [Google Scholar] [CrossRef]
- Ansari, M.A.; Samanta, A.; Behnagh, R.A.; Ding, H. An efficient coupled Eulerian-Lagrangian finite element model for friction stir processing. Int. J. Adv. Manuf. Tech. 2018. [Google Scholar] [CrossRef]
- Chmelnizkij, A.; Nagula, S.; Grabe, J. Numerical Simulation of Deep Vibration Compaction in Abaqus/CEL and MPM. Procedia Eng. 2017, 175, 302–309. [Google Scholar] [CrossRef]
- Li, K.; Ni, B.Y.; Li, J.C.M. Stick-slip in the scratching of styrene-acrylonitrile copolymer. J. Mater. Res. 2011, 11, 1574–1580. [Google Scholar] [CrossRef]





| Texture | Shape of Grain | Max Height | Grain Size * | Grain Spacing * | RA |
|---|---|---|---|---|---|
| Shallow Bump | Sharp | 0.070 | 213 (124) | 460 (217) | 3.56 |
| Coarse Bump | Round | 0.110 | 700 (374) | 1856 (466) | 2.52 |
| Yeoh Constants | PRF Constants | ||||||
|---|---|---|---|---|---|---|---|
| C10 | 1.71396 | S1 | 122.537 | S2 | 62.0356 | S3 | 12.579 |
| C20 | −0.20298 | τBase1 | 18.9996 | τBase2 | 15.8083 | τBase3 | 12.5455 |
| C30 | 0.0174978 | n1 | 14.2556 | n2 | 14.5184 | n3 | 11.6288 |
| κ | 2000 | m1 | −0.499632 | m2 | −3.01084 × 10−4 | m3 | −3.24107 × 10−6 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gao, W.; Wang, L.; Coffey, J.K.; Wu, H.; Daver, F. Finite Element Modelling and Experimental Validation of Scratches on Textured Polymer Surfaces. Polymers 2021, 13, 1022. https://doi.org/10.3390/polym13071022
Gao W, Wang L, Coffey JK, Wu H, Daver F. Finite Element Modelling and Experimental Validation of Scratches on Textured Polymer Surfaces. Polymers. 2021; 13(7):1022. https://doi.org/10.3390/polym13071022
Chicago/Turabian StyleGao, Weimin, Lijing Wang, Jolanta K. Coffey, Hongren Wu, and Fugen Daver. 2021. "Finite Element Modelling and Experimental Validation of Scratches on Textured Polymer Surfaces" Polymers 13, no. 7: 1022. https://doi.org/10.3390/polym13071022
APA StyleGao, W., Wang, L., Coffey, J. K., Wu, H., & Daver, F. (2021). Finite Element Modelling and Experimental Validation of Scratches on Textured Polymer Surfaces. Polymers, 13(7), 1022. https://doi.org/10.3390/polym13071022