The Relationship between the Morphology and Elasticity of Natural Rubber Foam Based on the Concentration of the Chemical Blowing Agent
Abstract
:1. Introduction
2. Materials and Methods
2.1. Materials
2.2. Preparation of Compounded Latex
2.3. Preparation of Rubber Foam
2.4. Characterisation
3. Results and Discussion
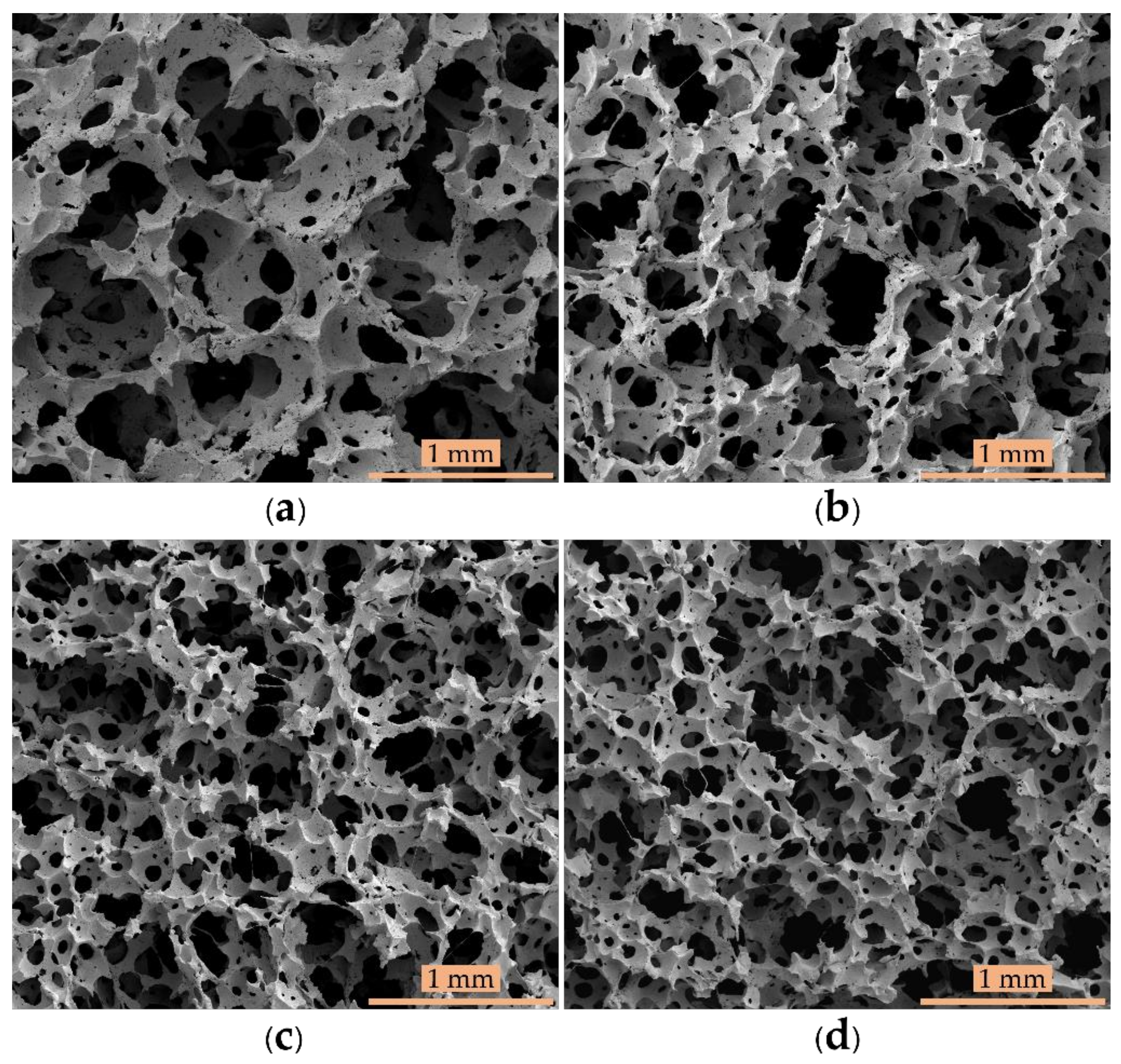
3.1. Morphological and Physical Properties
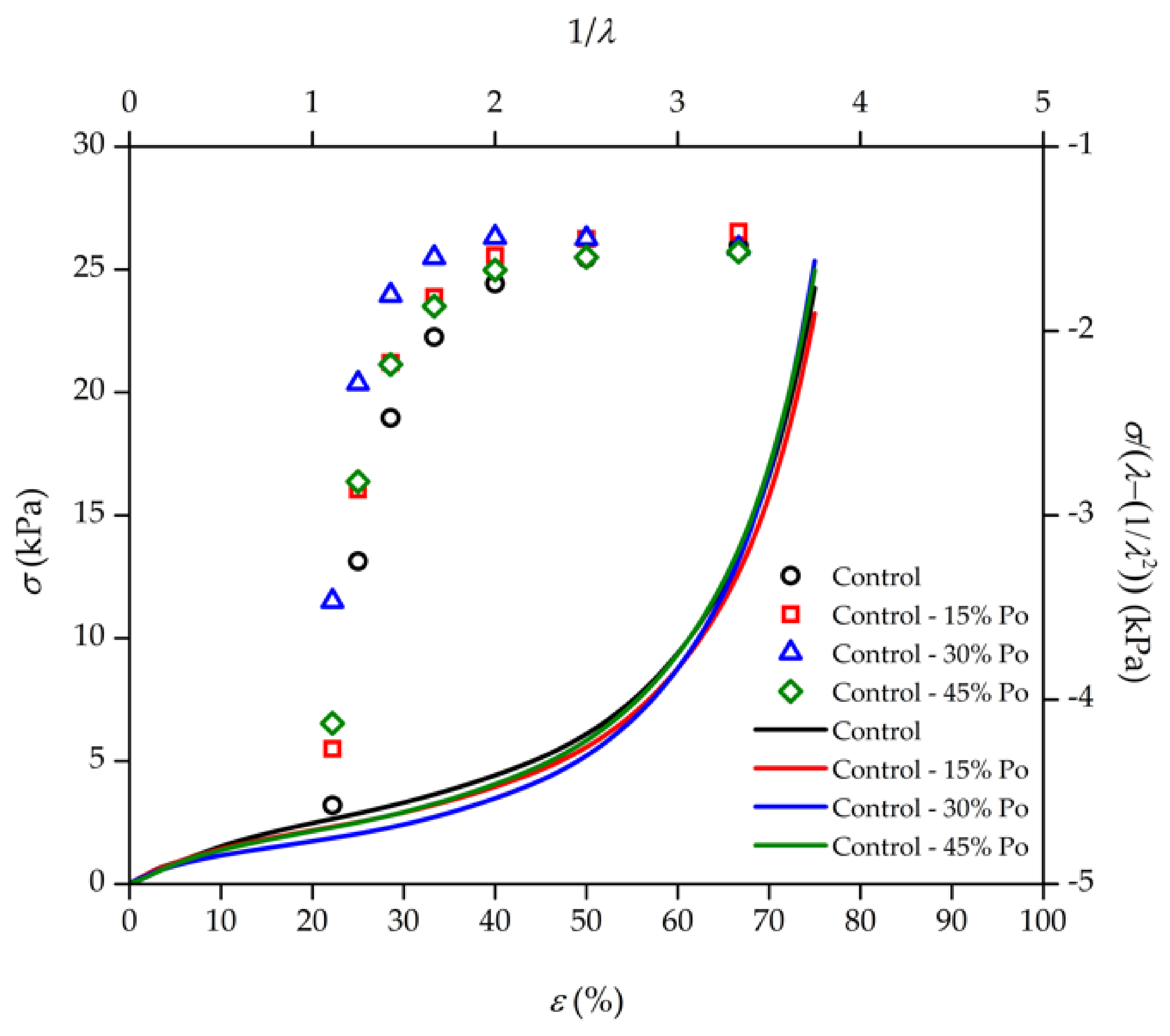
3.2. Physical and Chemical Elasticity
- i.
- at very low deformations, below about 10%, where a so–called thermoplastic inversion is observed due to thermal expansion obscuring the entropy effect [44];
- ii.
- at large deformations, where high degrees of orientation and crystallisation may occur [33].
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Madge, E.W. Latex foam Rubber; Maclaren and Sons: London, UK, 1962. [Google Scholar]
- Blackley, D.C. Polymer Latices: Science and Technology Volume 3: Applications of Latices; Springer: Heidelberg/Berlin, Germany, 1997. [Google Scholar]
- Roslim, R.; Hashim, M.Y.A.; Augurio, P.T. Natural latex foam. J. Eng. Sci. 2012, 8, 15–27. [Google Scholar]
- Kim, J.H.; Choi, K.C.; Yoon, J.M. The foaming characteristics and physical properties of natural rubber foams: Effects of carbon black content and foaming pressure. J. Ind. Eng. Chem. 2006, 12, 795–801. [Google Scholar]
- Murphy, E.A. Recent progress in latex technology. Ind. Eng. Chem. 1952, 44, 756–762. [Google Scholar] [CrossRef]
- British Rubber Development Board. Natural Rubber Latex and Its Applications: The Preparation of Latex Foam Products; British Rubber Development Board: London, UK, 1954. [Google Scholar]
- Ramasamy, S.; Ismail, H.; Munusamy, Y. Effect of rice husk powder on compression behavior and thermal stability of natural rubber latex foam. Bioresources 2013, 8, 4258–4269. [Google Scholar] [CrossRef]
- Wilbanks, A.; Oxford, N.; Mil, D. Textiles for Residential and Commercial Interiors, 4th ed.; Bloomsbury Publishing Inc.: New York, NY, USA, 2015. [Google Scholar]
- Eaves, D. Handbook of Polymer Foams; Smithers Rapra Publishing: Shrewsbury, UK, 2004. [Google Scholar]
- Syahrin, S.M.; Zunaida, Z.; Hakimah, O.; Nuraqmar, S.M.S. Effect of blowing agent on compression and morphological properties of natural rubber latex foam. AIP Conf. Proc. 2020, 2267, 020037. [Google Scholar]
- Najib, N.N.; Ariff, Z.M.; Manan, N.A.; Bakar, A.A.; Sipuat, C.S. Effect of blowing agent concentration on cell morphology and impact properties of natural rubber foam. J. Phys. Sci. 2009, 20, 13–25. [Google Scholar]
- Chen, Y.; Das, R.; Battley, M. Effects of cell size and cell wall thickness variations on the stiffness of closed-cell foams. Int. J. Solids Struct. 2015, 52, 150–164. [Google Scholar] [CrossRef]
- Wang, G.; Zhao, J.; Wang, G.; Mark, L.H.; Park, C.B.; Zhao, G. Low-density and structure-tunable microcellular PMMA foams with improved thermal-insulation and compressive mechanical properties. Eur. Polym. J. 2017, 95, 382–393. [Google Scholar] [CrossRef]
- Lin, G.; Zhang, X.-J.; Liu, L.; Zhang, J.-C.; Chen, Q.-M.; Zhang, L.-Q. Study on microstructure and mechanical properties relationship of short fibers/rubber foam composites. Eur. Polym. J. 2004, 40, 1733–1742. [Google Scholar] [CrossRef]
- Schneider, C.A.; Rasband, W.S.; Eliceiri, K.W. NIH image to ImageJ: 25 years of image analysis. Nat. Methods 2012, 9, 671–675. [Google Scholar] [CrossRef]
- Forest, C.; Chaumont, P.; Cassagnau, P.; Swoboda, B.; Sonntag, P. Polymer nano-foams for insulating applications prepared from CO2 foaming. Prog. Polym. Sci. 2015, 41, 122–145. [Google Scholar] [CrossRef]
- Fukahori, Y.; Seki, W. Molecular behaviour of elastomeric materials under large deformation: 1. Re-evaluation of the Mooney-Rivlin plot. Polymer 1992, 33, 502–508. [Google Scholar] [CrossRef]
- Flory, P.J.; Rehner, J. Statistical mechanics of cross-linked polymer networks II. Swelling. J. Chem. Phys. 1943, 11, 521–526. [Google Scholar] [CrossRef]
- Croll, S.G. Application of the Flory–Rehner equation and the Griffith fracture criterion to paint stripping. J. Coat. Technol. Res. 2009, 7, 49–55. [Google Scholar] [CrossRef]
- Tangboriboon, N.; Rortchanakarn, S.; Petcharoen, K.; Sirivat, A. Effects of foaming agents and eggshell calcium carbonate (CaCO3) filler on natural rubber foam physical-thermal—Mechanical properties. J. Rubber Res. 2016, 19, 71–96. [Google Scholar]
- Pojanavaraphan, T.; Magaraphan, R. Prevulcanized natural rubber latex/clay aerogel nanocomposites. Eur. Polym. J. 2008, 44, 1968–1977. [Google Scholar] [CrossRef]
- Ghari, H.S.; Jalali-Arani, A. Nanocomposites based on natural rubber, organoclay and nano-calcium carbonate: Study on the structure, cure behavior, static and dynamic-mechanical properties. Appl. Clay Sci. 2016, 119, 348–357. [Google Scholar] [CrossRef]
- Smitthipong, W.; Nardin, M.; Schultz, J.; Suchiva, K. Adhesion and self-adhesion of rubbers, crosslinked by electron beam irradiation. Int. J. Adhes. Adhes. 2007, 27, 352–357. [Google Scholar] [CrossRef]
- Suksup, R.; Sun, Y.; Sukatta, U.; Smitthipong, W. Foam rubber from centrifuged and creamed latex. J. Polym. Eng. 2019, 39, 336–342. [Google Scholar] [CrossRef]
- Phomrak, S.; Nimpaiboon, A.; Newby, B.-M.Z.; Phisalaphong, M. Natural rubber latex foam reinforced with micro- and nanofibrillated cellulose via Dunlop method. Polymers 2020, 12, 1959. [Google Scholar] [CrossRef]
- Wang, S.; Ameli, A.; Shaayegan, V.; Kazemi, Y.; Huang, Y.; Naguib, H.E.; Park, C.B. Modelling of rod-like fillers’ rotation and translation near two growing cells in conductive polymer composite foam processing. Polymers 2018, 10, 216. [Google Scholar] [CrossRef] [Green Version]
- Timpano, C.; Abdoli, H.; Leung, S.N.; Melenka, G.W. Characterization of open-cellular polymeric foams using micro-computed tomography. Polymer 2020, 202, 122628. [Google Scholar] [CrossRef]
- Quell, A.; de Bergolis, B.; Drenckhan, W.; Stubenrauch, C. How the locus of initiation influences the morphology and the pore connectivity of a monodisperse polymer foam. Macromolecules 2016, 49, 5059–5067. [Google Scholar] [CrossRef]
- Williams, J.M.; Wrobleski, D.A. Spatial distribution of the phases in water-in-oil emulsions. Open and closed microcellular foams from crosslinked polystyrene. Langmuir 1988, 4, 656–662. [Google Scholar] [CrossRef]
- Mantaranon, N.; Chirachanchai, S. Polyoxymethylene foam: From an investigation of key factors related to porous morphologies and microstructure to the optimization of foam properties. Polymer 2016, 96, 54–62. [Google Scholar] [CrossRef]
- Krause, B.; Mettinkhof, R.; van der Vegt, N.F.A.; Wessling, M. Microcellular foaming of amorphous high-Tg polymers using carbon dioxide. Macromolecules 2001, 34, 874–884. [Google Scholar] [CrossRef]
- Kim, Y.; Park, C.B.; Chen, P.; Thompson, R.B. Towards maximal cell density predictions for polymeric foams. Polymer 2011, 52, 5622–5629. [Google Scholar] [CrossRef]
- Prasopdee, T.; Smitthipong, W. Effect of fillers on the recovery of rubber foam: From theory to applications. Polymers 2020, 12, 2745. [Google Scholar] [CrossRef] [PubMed]
- Tanrattanakul, V.; Chumeka, W. Effect of potassium persulfate on graft copolymerization and mechanical properties of cassava starch/natural rubber foams. J. Appl. Polym. Sci. 2010, 116, 93–105. [Google Scholar] [CrossRef]
- Agrebi, F.; Ghorbel, N.; Bresson, S.; Abbas, O.; Kallel, A. Study of nanocomposites based on cellulose nanoparticles and natural rubber latex by ATR/FTIR spectroscopy: The impact of reinforcement. Polym. Compos. 2019, 40, 2076–2087. [Google Scholar] [CrossRef]
- Jin, F.-L.; Zhao, M.; Park, M.; Park, S.-J. Recent trends of foaming in polymer processing: A review. Polymers 2019, 11, 953. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Messinger, R.J.; Marks, T.G.; Gleiman, S.S.; Milstein, F.; Chmelka, B.F. Molecular origins of macroscopic mechanical properties of elastomeric organosiloxane foams. Macromolecules 2015, 48, 4835–4849. [Google Scholar] [CrossRef]
- Rizvi, A.; Tabatabaei, A.; Vahedi, P.; Mahmood, S.H.; Park, C.B. Non-crosslinked thermoplastic reticulated polymer foams from crystallization-induced structural heterogeneities. Polymer 2018, 135, 185–192. [Google Scholar] [CrossRef]
- Hui Mei, E.L.; Singh, M. The Dunlop process in natural rubber latex foam. Malays. Rubber Dev. Technol. 2010, 10, 23–26. [Google Scholar]
- Bashir, A.S.M.; Manusamy, Y.; Chew, T.L.; Ismail, H.; Ramasamy, S. Mechanical, thermal, and morphological properties of (eggshell powder)-filled natural rubber latex foam. J. Vinyl Addit. Technol. 2017, 23, 3–12. [Google Scholar] [CrossRef]
- Notario, B.; Pinto, J.; Rodríguez-Pérez, M.A. Towards a new generation of polymeric foams: PMMA nanocellular foams with enhanced physical properties. Polymer 2015, 63, 116–126. [Google Scholar] [CrossRef]
- Mangan, R.; Destrade, M.; Saccomandi, G. Strain energy function for isotropic non-linear elastic incompressible solids with linear finite strain response in shear and torsion. Extreme Mech. Lett. 2016, 9, 204–206. [Google Scholar] [CrossRef] [Green Version]
- Kawamura, T.; Urayama, K.; Kohjiya, S. Multiaxial deformations of end-linked poly(dimethylsiloxane) networks 5. Revisit to Mooney-Rivlin approach to strain energy density function. Nihon Reoroji Gakkaishi 2003, 31, 213–217. [Google Scholar] [CrossRef] [Green Version]
- Treloar, L.R.G. The Physics of Rubber Elasticity; Oxford University Press: New York, NY, USA, 1975. [Google Scholar]
- Hiemenz, P.C.; Lodge, T.P. Polymer Chemistry, 2nd ed.; CRC Press: Boca Raton, FL, USA, 2007. [Google Scholar]
- Rubinstein, M.; Colby, R.H. Polymer Physics; Oxford University Press: Oxford, UK, 2003. [Google Scholar]
- Wall, F.T.; Flory, P.J. Statistical thermodynamics of rubber elasticity. J. Chem. Phys. 1951, 19, 1435–1439. [Google Scholar] [CrossRef]
- Deam, R.T.; Edwards, S.F. The theory of rubber elasticity. Philos. Trans. R. Soc. A 1976, 280, 317–353. [Google Scholar]
- Flory, P.J. Molecular theory of rubber elasticity. Polymer 1979, 20, 1317–1320. [Google Scholar] [CrossRef]
- Erman, B.; Mark, J.E. The molecular basis of rubberlike elasticity. In Science and Technology of Rubber, 3rd ed.; Mark, J.E., Erman, B., Eirich, F.R., Eds.; Academic Press: London, UK, 2005. [Google Scholar]
- Mark, J.E.; Erman, B. Rubberlike Elasticity: A Molecular Primer, 2nd ed.; Cambridge University Press: Cambridge, UK, 2007. [Google Scholar]






| Chemical Agents | Control (g) | Control - 15% Po (g) | Control - 30% Po (g) | Control - 45% Po (g) |
|---|---|---|---|---|
| 60% concentrated natural latex | 166.67 | 166.67 | 166.67 | 166.67 |
| 10% potassium oleate aqueous solution (Po) | 16.50 | 14.00 | 11.50 | 9.00 |
| 50% sulphur aqueous dispersion | 4.00 | 4.00 | 4.00 | 4.00 |
| 50% ZDEC aqueous dispersion | 2.00 | 2.00 | 2.00 | 2.00 |
| 50% ZMBT aqueous dispersion | 2.00 | 2.00 | 2.00 | 2.00 |
| 50% antioxidant (Wingstay L) aqueous dispersion | 2.00 | 2.00 | 2.00 | 2.00 |
| 50% ZnO aqueous dispersion | 10.00 | 10.00 | 10.00 | 10.00 |
| 33% DPG aqueous dispersion | 2.00 | 2.00 | 2.00 | 2.00 |
| 12.5% SSF aqueous dispersion | 8.00 | 8.00 | 8.00 | 8.00 |
| Sample | Average Cell Size (±150 µm) | Porosity (±1.00%) | Cell Density (±500 cm−3) |
|---|---|---|---|
| Control | 548 | 49.86 | 10,241 |
| Control - 15% Po | 473 | 54.73 | 15,908 |
| Control - 30% Po | 320 | 56.06 | 50,837 |
| Control - 45% Po | 273 | 54.78 | 82,450 |
| Type of Rubber | Test | λ | C1 | C2 | C2/C1 | Reference |
|---|---|---|---|---|---|---|
| Crosslinked NR foam | Compression | <1 | 1.1809 | 0.1330 | 0.113 | - |
| Uncrosslinked solid NR | Extension | 1 ≤ λ ≤ 2 | 1.7725 | 2.7042 | 1.526 | [42] |
| Crosslinked solid PDMS | Extension | < 1 | 2.91 | 1.98 | 0.682 | [43] |
| Sample | Volume Fraction of Rubber (Vr ± 0.001%) | ΔG (J/mol) | ΔS (J/mol.K) |
|---|---|---|---|
| Control | 0.2594 | −36.3233 | 0.1218 |
| Control - 15% Po | 0.2574 | −35.6039 | 0.1194 |
| Control - 30% Po | 0.2540 | −34.3942 | 0.1154 |
| Control - 45% Po | 0.2524 | −33.8061 | 0.1134 |
| Foam Sample | Compression Limit (λ) | Fu (±5% N) | F @ 298.15 K (±5% N) | Fu/F |
|---|---|---|---|---|
| Control | 0.9 | 6.35 | 9.66 | 0.6573 |
| 0.8 | 8.61 | 12.55 | 0.6863 | |
| 0.7 | 10.37 | 14.57 | 0.7115 | |
| 0.6 | 12.52 | 16.91 | 0.7408 | |
| 0.5 | 15.66 | 20.16 | 0.7767 | |
| 0.4 | 21.68 | 26.34 | 0.8234 | |
| 0.3 | 33.20 | 36.15 | 0.9183 | |
| Control - 15% Po | 0.9 | 4.56 | 6.11 | 0.7463 |
| 0.8 | 6.48 | 8.51 | 0.7617 | |
| 0.7 | 8.16 | 10.43 | 0.7827 | |
| 0.6 | 10.36 | 12.81 | 0.8091 | |
| 0.5 | 13.32 | 15.68 | 0.8498 | |
| 0.4 | 18.89 | 20.91 | 0.9031 | |
| 0.3 | 28.30 | 26.81 | 1.0556 | |
| Control - 30% Po | 0.9 | 3.72 | 4.85 | 0.7663 |
| 0.8 | 5.39 | 6.91 | 0.7800 | |
| 0.7 | 6.88 | 8.52 | 0.8075 | |
| 0.6 | 8.99 | 10.69 | 0.8410 | |
| 0.5 | 11.90 | 13.27 | 0.8966 | |
| 0.4 | 17.46 | 17.97 | 0.9718 | |
| 0.3 | 26.81 | 22.31 | 1.2018 | |
| Control - 45% Po | 0.9 | 4.26 | 5.46 | 0.7814 |
| 0.8 | 6.24 | 7.88 | 0.7918 | |
| 0.7 | 8.20 | 10.20 | 0.8041 | |
| 0.6 | 10.91 | 13.39 | 0.8152 | |
| 0.5 | 14.47 | 17.12 | 0.8450 | |
| 0.4 | 20.85 | 23.51 | 0.8871 | |
| 0.3 | 30.91 | 30.14 | 1.0257 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Suethao, S.; Phongphanphanee, S.; Wong-ekkabut, J.; Smitthipong, W. The Relationship between the Morphology and Elasticity of Natural Rubber Foam Based on the Concentration of the Chemical Blowing Agent. Polymers 2021, 13, 1091. https://doi.org/10.3390/polym13071091
Suethao S, Phongphanphanee S, Wong-ekkabut J, Smitthipong W. The Relationship between the Morphology and Elasticity of Natural Rubber Foam Based on the Concentration of the Chemical Blowing Agent. Polymers. 2021; 13(7):1091. https://doi.org/10.3390/polym13071091
Chicago/Turabian StyleSuethao, Supitta, Saree Phongphanphanee, Jirasak Wong-ekkabut, and Wirasak Smitthipong. 2021. "The Relationship between the Morphology and Elasticity of Natural Rubber Foam Based on the Concentration of the Chemical Blowing Agent" Polymers 13, no. 7: 1091. https://doi.org/10.3390/polym13071091
APA StyleSuethao, S., Phongphanphanee, S., Wong-ekkabut, J., & Smitthipong, W. (2021). The Relationship between the Morphology and Elasticity of Natural Rubber Foam Based on the Concentration of the Chemical Blowing Agent. Polymers, 13(7), 1091. https://doi.org/10.3390/polym13071091





