Experimental and Finite Element Simulation of Polyolefin Elastomer Foams Using Real 3D Structures: Effect of Foaming Agent Content
Abstract
:1. Introduction
2. Materials and Methods
2.1. Materials
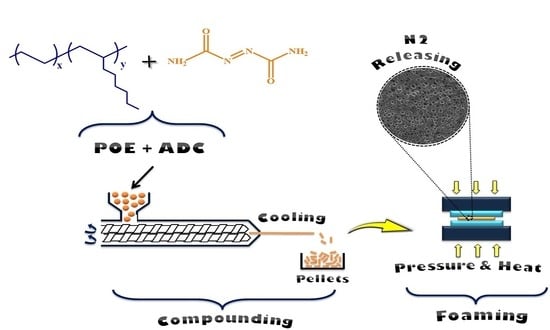
2.2. Preparation of POE-ADC Compounds
2.3. Foams Preparation and Characterization
3. Results and Discussion
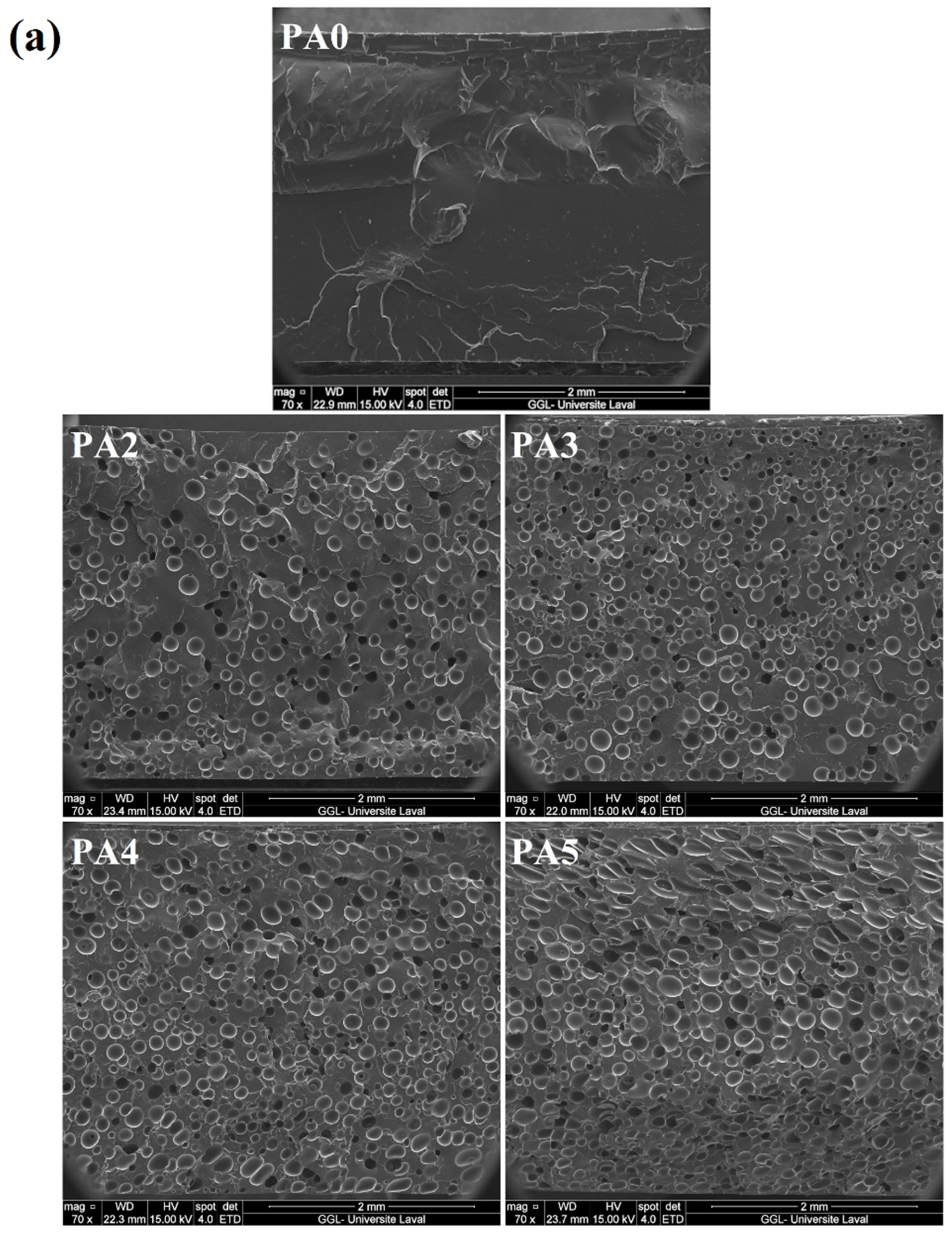
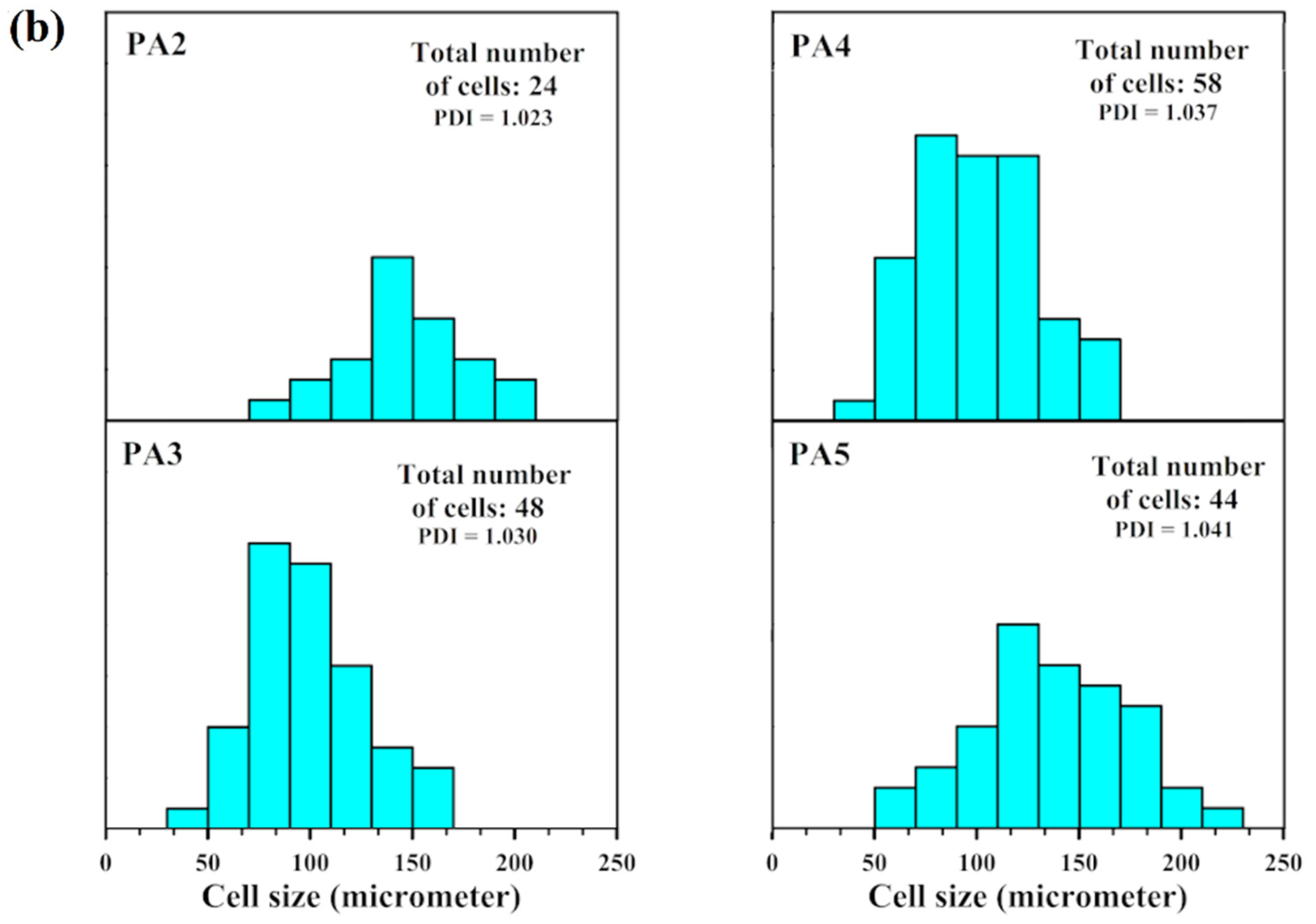
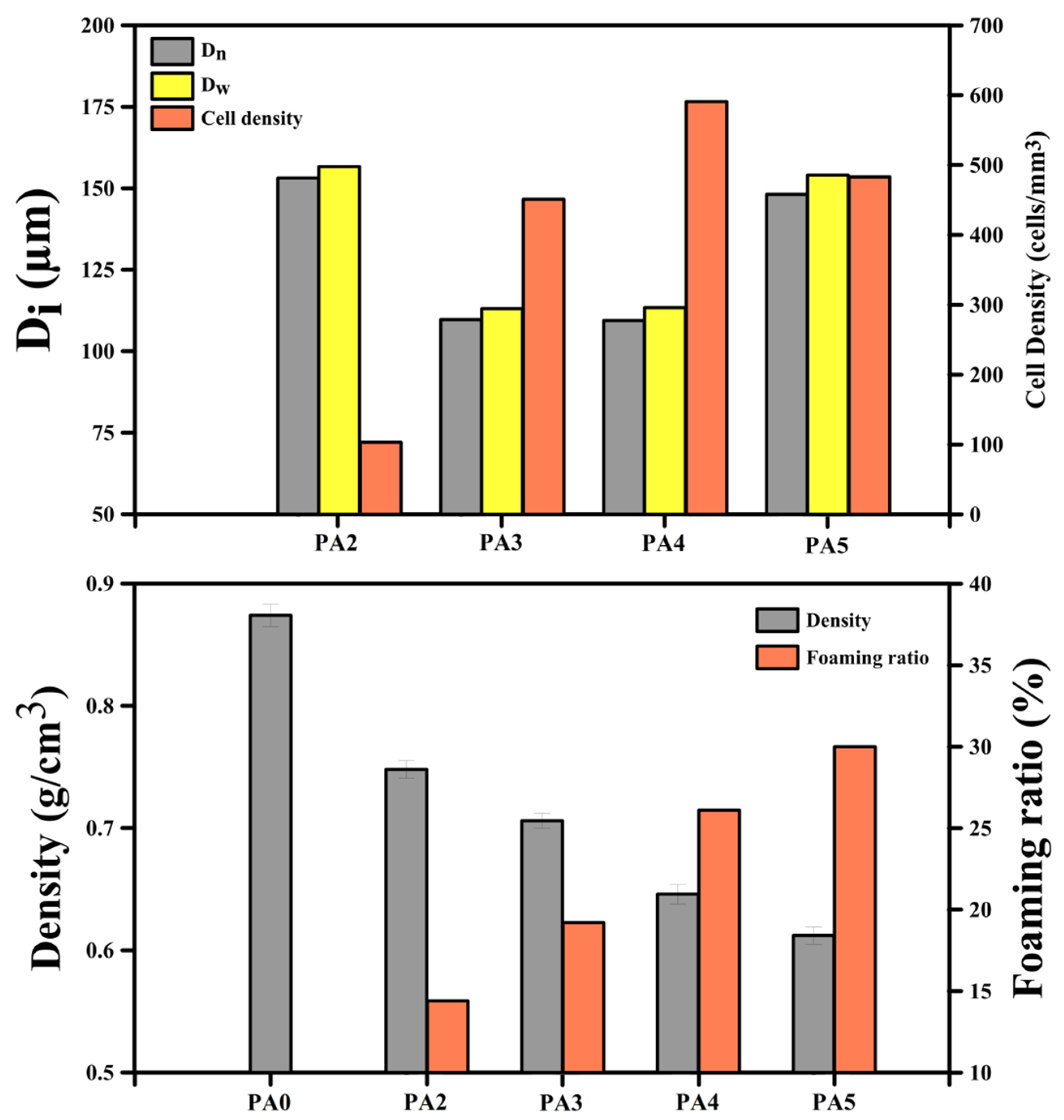
3.1. Morphological and Physical Characterization
3.2. Mechanical Properties
4. Modeling and Simulation
4.1. Dimensional Geometry Modeling and Material Definition
4.2. Boundary and Test Conditions
4.3. Material Definition in FEM Models
4.4. The Limitation of Problem Solving by FEM
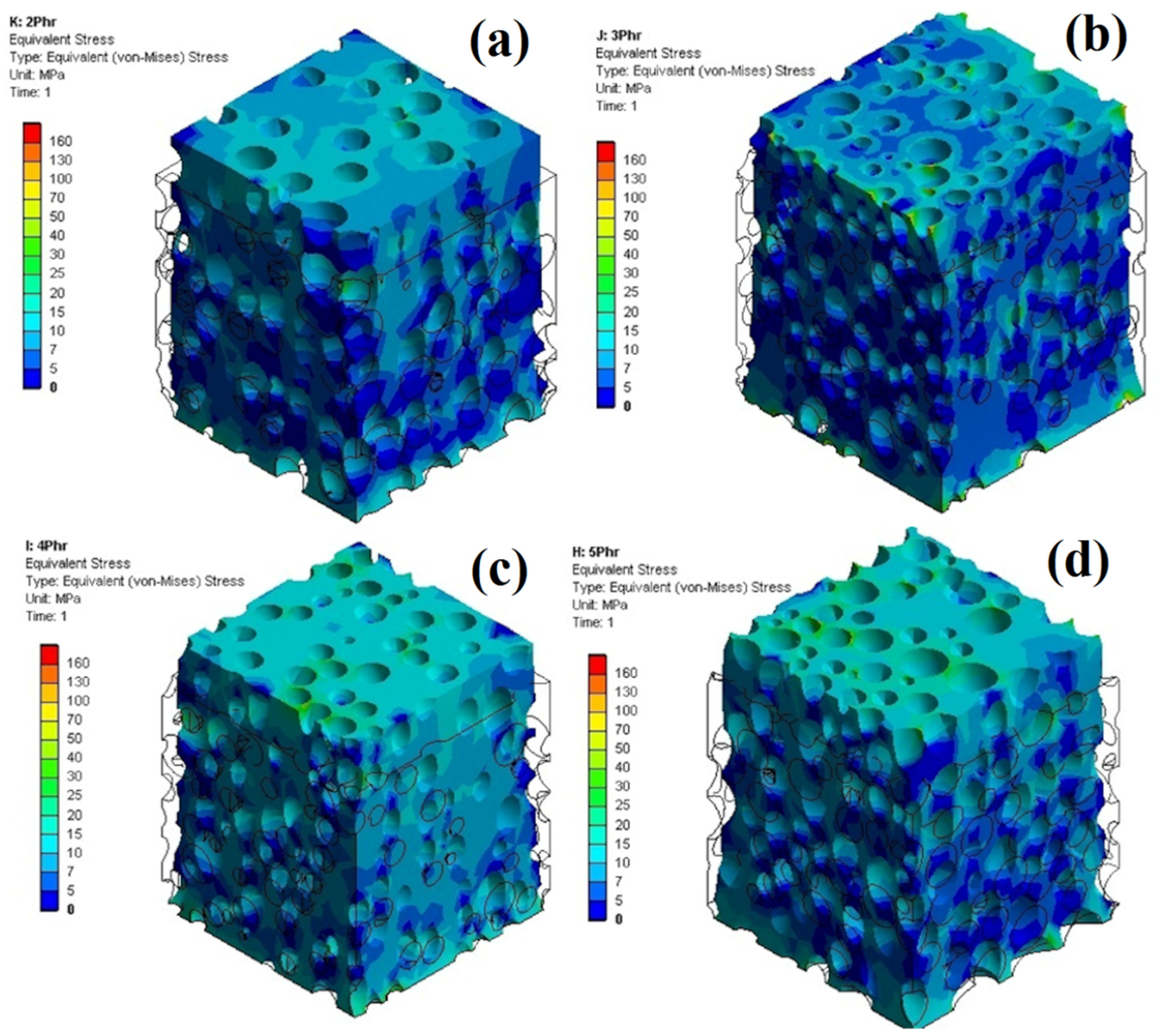
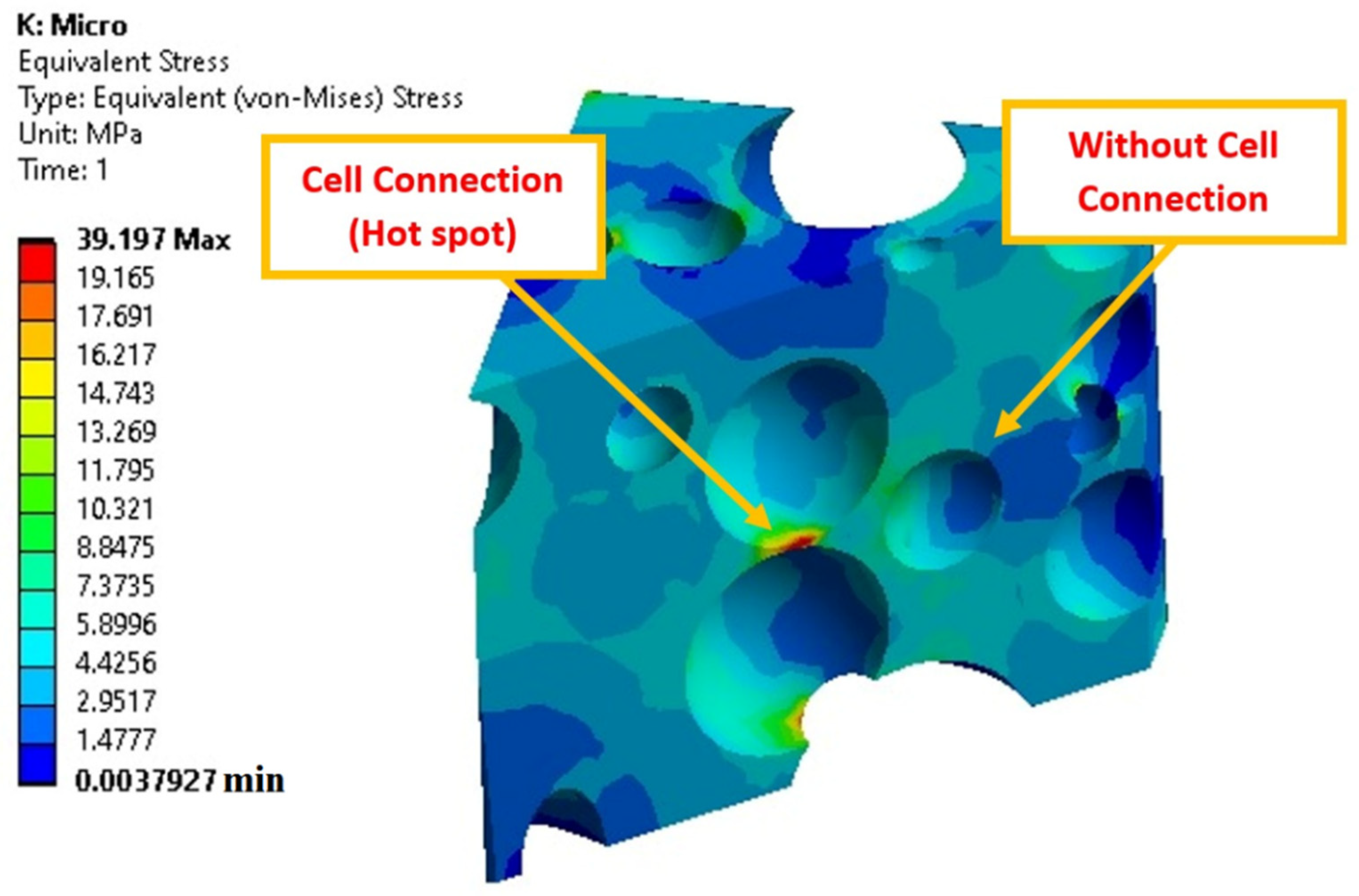
4.5. The Results of FEM
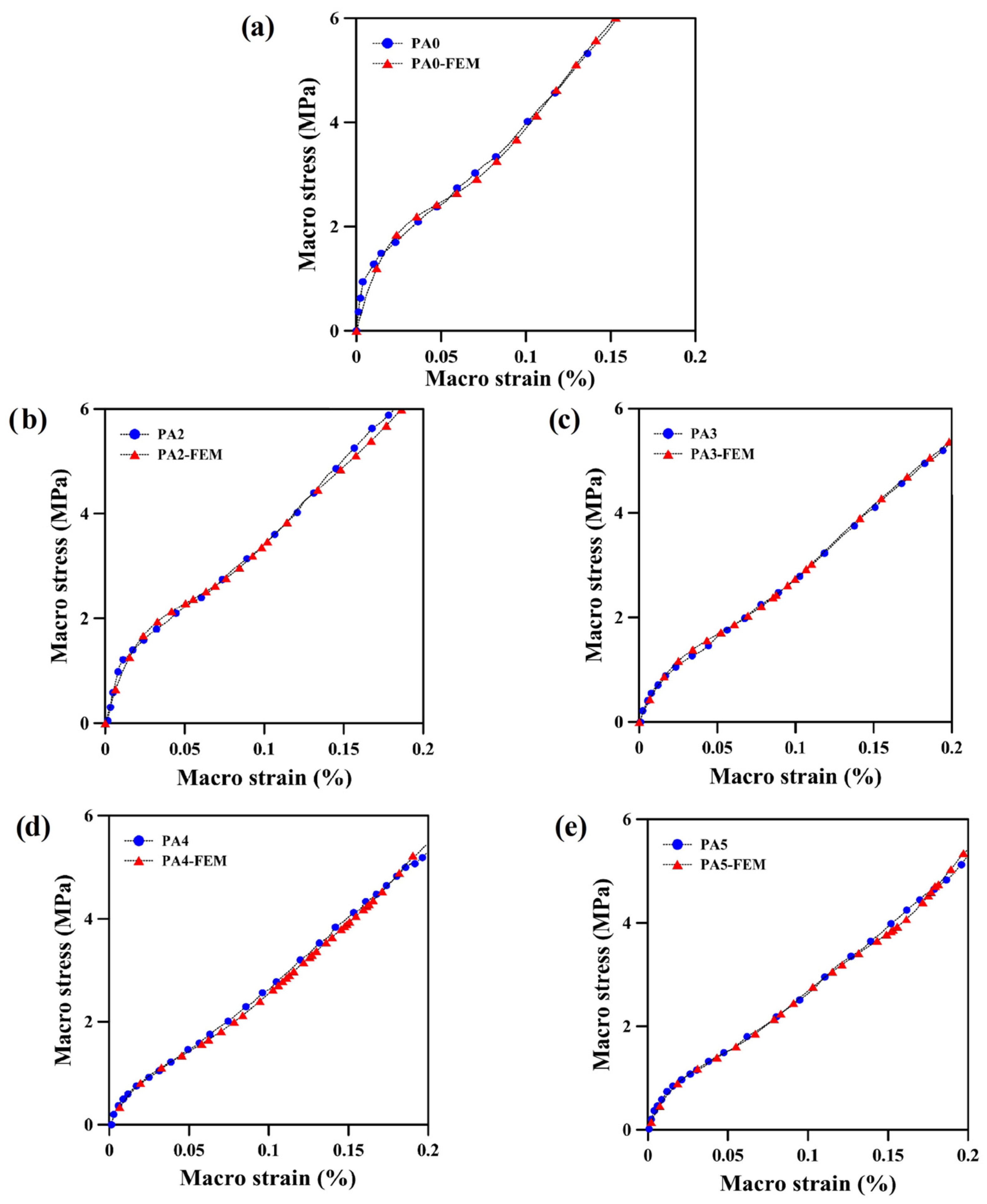
- (a)
- The FEM results coincide well with the experimental results. After analyzing the experimental results, the average value of their RMSE is 1.5, and the percentage of errors between the experimental and numerical results is 9.2%. These results show the validity of the FEM results compared to the experimental results in the field of engineering standards. This can be attributed to a number of factors: fine material definition with hyperelastic models and an accurate 3D-modeled cell structure.
- (b)
- The FEM simulation and experimental results both showed that a relation between stress, strain and ADC content exists. For example, at 4 MPa stress, PA2 has the lowest strain (12%) and PA5 has the highest strain (15%). Similarly, PA2 requires a greater stress (4.9 MPa) than PA5 (3.8 MPa) to reach a strain of 15%. As mentioned before and according to these findings, the tensile properties of the foams are closely related to the foam density and less to the morphological parameters.
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Spontak, R.J.; Patel, N.P. Thermoplastic elastomers: Fundamentals and applications. Curr. Opin. Colloid Interface Sci. 2000, 5, 333–340. [Google Scholar] [CrossRef]
- Fazli, A.; Rodrigue, D. Phase morphology, mechanical, and thermal properties of fiber-reinforced thermoplastic elastomer: Effects of blend composition and compatibilization. J. Reinf. Plast. Comp. 2022, 41, 267–283. [Google Scholar] [CrossRef] [PubMed]
- Wittenberg, E.; Meyer, A.; Eggers, S.; Abetz, V. Hydrogen bonding and thermoplastic elastomers–a nice couple with temperature -adjustable mechanical properties. Soft Matter 2018, 14, 2701–2711. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Zheng, H.; Pan, G.; Huang, P.; Xu, D.; Zhai, W. Fundamental influences of crosslinking structure on the cell morphology, creep property, thermal property, and recycling behavior of microcellular EPDM foams blown with compressed CO2. Ind. Eng. Chem. Res. 2020, 59, 1534–1548. [Google Scholar] [CrossRef]
- Ballini, A.; Di Cosola, M.; Saini, R.; Benincasa, C.; Aiello, E.; Marrelli, B.; Rajiv Saini, S.; Ceruso, F.M.; Nocini, R.; Topi, S.; et al. A Comparison of manual nylon Bristle Toothbrushes versus thermoplastic elastomer toothbrushes in terms of cleaning efficacy and the biological potential role on gingival health. Appl. Sci. 2021, 11, 7180. [Google Scholar] [CrossRef]
- Padmanabhan, R.; Naskar, K.; Nando, G.B. Investigation into the structure–property relationship and technical properties of TPEs and TPVs derived from ethylene octene copolymer (EOC) and polydimethyl siloxane (PDMS) rubber blends. Mater. Res. Express. 2015, 2, 105301. [Google Scholar] [CrossRef]
- Fazli, A.; Rodrigue, D. Waste rubber recycling: A review on the evolution and properties of thermoplastic elastomers. Materials 2020, 13, 782. [Google Scholar] [CrossRef] [Green Version]
- Wang, J.; Li, J.; Li, H.; Zhou, H. Thermoplastic polyurethane (TPU) modifier to develop bimodal cell structure in polypropylene/TPU microcellular foam in presence of supercritical CO2. J. Vinyl Addit. Technol. 2021, 27, 127–136. [Google Scholar] [CrossRef]
- Yang, F.; Pan, L.; Ma, Z.; Lou, Y.; Li, Y.; Li, Y. Highly elastic, strong, and reprocessable cross-linked polyolefin elastomers enabled by boronic ester bonds. Polym. Chem. 2020, 11, 3285–3295. [Google Scholar] [CrossRef]
- Bensason, S.; Minick, J.; Moet, A.; Chum, S.; Hiltner, A.; Baer, E. Classification of homogeneous ethylene-octene copolymers based on comonomer content. J. Polym. Sci. B Polym. Phys. 1996, 34, 1301–1315. [Google Scholar] [CrossRef]
- Nayak, N.C.; Achary, P.G.R.; Das, S.; Begum, S. Effect of carbon black on microcellular behavior of ethylene-octene copolymer vulcanizates. Cell. Polym. 2014, 33, 71–86. [Google Scholar] [CrossRef]
- Maynard, L.A.; DeButts, B.L.; Barone, J.R. Mechanical and thermal properties of polyolefin thermoplastic elastomer blends. Plast. Rubber Compos. 2019, 48, 338–346. [Google Scholar] [CrossRef]
- Vahidifar, A.; Esmizadeh, E.; Rostami, E.; Nouri Khorasani, S.; Rodrigue, D. Morphological, rheological, and mechanical properties of hybrid elastomeric foams based on natural rubber, nanoclay, and nanocarbon black. Polym. Compos. 2019, 40, 4289–4299. [Google Scholar] [CrossRef]
- Zauzi, N.S.A.; Ariff, Z.M.; Shuib, R.K. Development of natural rubber foam with water as a blowing agent via microwave and convection heating methods. Express. Polym. Lett. 2022, 16, 296–317. [Google Scholar] [CrossRef]
- Graul, L.M.; Horn, S.J.; Nash, L.D.; Cheung, T.B.; Clubb, F.J.; Maitland, D.J. Image-Based Evaluation of In Vivo Degradation for Shape-Memory Polymer Polyurethane Foam. Polymers 2022, 14, 4122. [Google Scholar] [CrossRef]
- Sun, X.; Liang, W. Cellular structure control and sound absorption of polyolefin microlayer sheets. Compos. B Eng. 2016, 87, 21–26. [Google Scholar] [CrossRef]
- Vahidifar, A.; Esmizadeh, E.; Rodrigue, D.; Khonakdar, H.A.; Wagenknecht, U. Towards novel super-elastic foams based on isoperene rubber: Preparation and characterization. Polym. Adv. Technol. 2020, 31, 1508–1518. [Google Scholar] [CrossRef]
- Bayat, H.; Fasihi, M.; Zare, Y.; Rhee, K.Y. An experimental study on one-step and two-step foaming of natural rubber/silica nanocomposites. Nanotechnol. Rev. 2020, 9, 427–435. [Google Scholar] [CrossRef]
- Esmizadeh, E.; Vahidifar, A.; Rostami, E.; Nouri Khorasani, S.; Ghayoumi, M.; Khonakdar, H.A. Effect of carbon black on morphological and mechanical properties of rubber foams produced by a single-step method. J. Appl. Res. Chem. Polym. Eng. 2017, 1, 49–60. [Google Scholar]
- Vahidifar, A.; Khorasani, S.N.; Park, C.B.; Khonakdar, H.A.; Reuter, U.; Naguib, H.E.; Esmizadeh, E. Towards the development of uniform closed cell nanocomposite foams using natural rubber containing pristine and organo-modified nanoclays. RSC Adv. 2016, 6, 53981–53990. [Google Scholar] [CrossRef]
- Rostami-Tapeh-Esmaeil, E.; Vahidifar, A.; Esmizadeh, E.; Rodrigue, D. Chemistry, Processing, Properties, and Applications of Rubber Foams. Polymers 2021, 13, 1565. [Google Scholar] [CrossRef] [PubMed]
- Rostami-Tapeh-Esmaeil, E.; Shojaei, S.; Rodrigue, D. Mechanical and Thermal Properties of Functionally Graded Polyolefin Elastomer Foams. Polymers 2022, 14, 4124. [Google Scholar] [CrossRef] [PubMed]
- Sombatsompop, N.; Lertkamolsin, P. Effects of chemical blowing agents on swelling properties of expanded elastomers. J. Elastomers Plast. 2000, 32, 311–328. [Google Scholar] [CrossRef]
- Lobos, J.; Thirumuruganandham, S.P.; Rodríguez-Pérez, M.A. Density Gradients, Cellular Structure and Thermal Conductivity of High-Density Polyethylene Foams by Different Amounts of Chemical Blowing Agent. Polymers 2022, 14, 4082. [Google Scholar] [CrossRef] [PubMed]
- Whitehead, L.W.; Robins, T.G.; Fine, L.J.; Hansen, D.J. Respiratory symptoms associated with the use of azodicarbonamide foaming agent in a plastics injection molding facility. Am. J. Ind. Med. 1987, 11, 83–92. [Google Scholar] [CrossRef]
- Afrinaldi, B.; Vicarneltor, D.N.; Rudianto, R.P.; Hakim, A.R.; Muslim, O.F. Influence of Zinc Oxide Addition on Azodicarbonamide Thermal Decomposition in the Polyethylene/Ethylene Vinyl Acetate Foaming Release. Mater. Sci. Forum. 2021, 1028, 234–239. [Google Scholar]
- Peng, L.; Lei, L.; Liu, Y.; Du, L. Improved mechanical and sound absorption properties of open cell silicone rubber foam with NaCl as the pore-forming agent. Materials 2021, 14, 195. [Google Scholar] [CrossRef]
- Shojaie, S.; Vahidifar, A.; Naderi, G.; Shokri, E.; Mekonnen, T.H.; Esmizadeh, E. Physical Hybrid of Nanographene/Carbon Nanotubes as Reinforcing Agents of NR-Based Rubber Foam. Polymers 2021, 13, 2346. [Google Scholar] [CrossRef]
- Hassan, H.; Al-Dardir, A.; Khaliel, J.; Ayoub, H.; Khairy, S.; Abdel Barya, E.; Elbashar, Y. Effect of foaming agent on the mechanical properties of sulfur and peroxide cured ethylene propylene diene monomer rubbers. Rev. Mex. Fis. 2019, 65, 351–354. [Google Scholar] [CrossRef] [Green Version]
- Charoeythornkhajhornchai, P.; Samthong, C.; Boonkerd, K.; Somwangthanaroj, A. Effect of azodicarbonamide on microstructure, cure kinetics and physical properties of natural rubber foam. J. Cell. Plast. 2017, 53, 287–303. [Google Scholar] [CrossRef]
- Mao, Y.; Qi, R. Preparation of polyethylene–octene elastomer foams by compression molding. J. Appl. Polym. Sci. 2008, 109, 3249–3255. [Google Scholar] [CrossRef]
- Wang, B.; Peng, Z.; Zhang, Y.; Zhang, Y. Study on foaming kinetics and preparation of EPDM foams. Plast. Rubber Compos. 2006, 35, 360–367. [Google Scholar] [CrossRef]
- Wang, B.; Peng, Z.; Zhang, Y.; Zhang, Y. Rheological properties and foam processibility of precured EPDM. J. Appl. Polym. Sci. 2006, 101, 3387–3394. [Google Scholar] [CrossRef]
- Lawindy, A.M.Y.E.; El-Kade, K.M.A.; Mahmoud, W.E.; Hassan, H.H. Physical studies of foamed reinforced rubber composites Part I. Mechanical properties of foamed ethylene–propylene–diene terpolymer and nitrile–butadiene rubber composites. Polym. Int. 2002, 51, 601–606. [Google Scholar] [CrossRef]
- Mahmoud, W.; El-Eraki, M.; El-Lawindy, A.; Hassan, H. A novel application of ADC/K-foaming agent-loaded NBR rubber composites as pressure sensor. J. Phys. D Appl. Phys. 2006, 39, 541. [Google Scholar] [CrossRef]
- Huber, A.; Gibson, L. Anisotropy of foams. J. Mater. Sci. 1988, 23, 3031–3040. [Google Scholar] [CrossRef]
- Ding, Y.; Vyas, C.; Bakker, O.; Hinduja, S.; Bartolo, P. Modelling and Simulation of MuCell®: The Effect of Key Processing Parameters on Cell Size and Weight Reduction. Polymers 2022, 14, 4215. [Google Scholar] [CrossRef]
- Romero, P.A.; Zheng, S.F.; Cuitiño, A.M. Modeling the dynamic response of visco-elastic open-cell foams. J. Mech. Phys. Solids 2008, 56, 1916–1943. [Google Scholar] [CrossRef]
- Luo, G.; Xue, P. Investigations on the mechanism and behavior of dynamic energy absorption of metal foam. Lat. Am. J. Solids Struct. 2018, 15, e47. [Google Scholar] [CrossRef]
- Beckmann, C.; Hohe, J. A probabilistic constitutive model for closed-cell foams. Mech. Mater. 2016, 96, 96–105. [Google Scholar] [CrossRef]
- Mills, N. Deformation mechanisms and the yield surface of low-density, closed-cell polymer foams. J. Mater. Sci. 2010, 45, 5831–5843. [Google Scholar] [CrossRef]
- Lim, T.-J.; Smith, B.; McDowell, D. Behavior of a random hollow sphere metal foam. Acta Mater. 2002, 50, 2867–2879. [Google Scholar] [CrossRef]
- Marvi-Mashhadi, M.; Lopes, C.; LLorca, J. Effect of anisotropy on the mechanical properties of polyurethane foams: An experimental and numerical study. Mech. Mater. 2018, 124, 143–154. [Google Scholar] [CrossRef]
- Raghunath, R.; Juhre, D. Finite element simulation of deformation behaviour of cellular rubber components. Mech. Res. Commun. 2013, 47, 32–38. [Google Scholar] [CrossRef]
- Marvi-Mashhadi, M.; Lopes, C.; LLorca, J. Modelling of the mechanical behavior of polyurethane foams by means of micromechanical characterization and computational homogenization. Int. J. Solids Struct. 2018, 146, 154–166. [Google Scholar] [CrossRef]
- Kırca, M.; Gül, A.; Ekinci, E.; Yardım, F.; Mugan, A. Computational modeling of micro-cellular carbon foams. Finite Elem. Anal. Des. 2007, 44, 45–52. [Google Scholar] [CrossRef]
- Majumder, S.; Roychowdhury, A.; Pal, S. Effectiveness of a foam type hip pad in reduction of hip fracture: A 3D finite element study. J. Biomech. 2008, 41, S453. [Google Scholar] [CrossRef]
- Wicklein, M.; Thoma, K. Numerical investigations of the elastic and plastic behaviour of an open-cell aluminium foam. Mater. Sci. Eng. A 2005, 397, 391–399. [Google Scholar] [CrossRef]
- Brydon, A.; Bardenhagen, S.; Miller, E.; Seidler, G. Simulation of the densification of real open-celled foam microstructures. J. Mech. Phys. Solids 2005, 53, 2638–2660. [Google Scholar] [CrossRef]
- Maruyama, B.; Spowart, J.E.; Hooper, D.J.; Mullens, H.M.; Druma, A.M.; Druma, C.; Alam, M.K. A new technique for obtaining three-dimensional structures in pitch-based carbon foams. Scr. Mater. 2006, 54, 1709–1713. [Google Scholar] [CrossRef]
- Cunsolo, S.; Oliviero, M.; Harris, W.M.; Andreozzi, A.; Bianco, N.; Chiu, W.K.; Naso, V. Monte Carlo determination of radiative properties of metal foams: Comparison between idealized and real cell structures. Inter. J. Therm. Sci. 2015, 87, 94–102. [Google Scholar] [CrossRef] [Green Version]
- Pascon, J.P.; Coda, H.B. Finite deformation analysis of visco-hyperelastic materials via solid tetrahedral finite elements. Finite Elem. Anal. Des. 2017, 133, 25–41. [Google Scholar] [CrossRef]
- Yan, Y.; Liu, B.; Xing, Y.; Carrera, E.; Pagani, A. Free vibration analysis of variable stiffness composite laminated beams and plates by novel hierarchical differential quadrature finite elements. Compos. Struct. 2021, 274, 114364. [Google Scholar] [CrossRef]
- Kabir, H.; Aghdam, M.M. A generalized 2D Bézier-based solution for stress analysis of notched epoxy resin plates reinforced with graphene nanoplatelets. Thin-Walled Struct. 2021, 169, 108484. [Google Scholar] [CrossRef]
- Zauzi, N.A.; Ariff, Z.; Khimi, S. Foamability of natural rubber via microwave assisted foaming with azodicarbonamide (ADC) as blowing agent. Mater Today Proc. 2019, 17, 1001–1007. [Google Scholar] [CrossRef]
- Rostami-Tapeh-Esmail, E.; Golshan, M.; Salami-Kalajahi, M.; Roghani-Mamaqani, H. UV-stabilized self-assembled amphiphilic triblock terpolymers supramolecular structures with low cytotoxicity as doxorubicin carriers. Mater. Sci. Eng. C 2020, 110, 110745. [Google Scholar] [CrossRef]
- Rostami-Tapeh-Esmail, E.; Golshan, M.; Salami-Kalajahi, M.; Roghani-Mamaqani, H.; Kahaie-Khosrowshahi, A. Temperature-induced self-assembly of amphiphilic triblock terpolymers to low cytotoxic spherical and cubic structures as curcumin carriers. J. Mol. Liq. 2020, 313, 113504. [Google Scholar] [CrossRef]
- Rostami-Tapeh-Esmaeil, E.; Golshan, M.; Salami-Kalajahi, M.; Roghani-Mamaqani, H. Synthesis of copper and copper oxide nanoparticles with different morphologies using aniline as reducing agent. Solid State Commun. 2021, 334, 114364. [Google Scholar] [CrossRef]
- Chen, X.; Feng, J.J.; Bertelo, C.A. Plasticization effects on bubble growth during polymer foaming. Polym. Eng. Sci. 2006, 46, 97–107. [Google Scholar] [CrossRef]
- Paulin, I. Stability of close-cell al foams depending on the usage of different foaming agents. Mater. Tehnol. 2015, 49, 983–988. [Google Scholar] [CrossRef]
- Heydari, A.; Esmizadeh, E.; Vahidifar, A.; Naderi, G.; Rodrigue, D. Real 3D Structure-Based Finite Element Simulation of Elastomer Foams: Effect of the Foaming Agent Content. Ind. Eng. Chem. Res. 2022, 61, 7881–7890. [Google Scholar] [CrossRef]
- Yao, J.; Rodrigue, D. Density graded polyethylene foams produced by compression moulding using a chemical blowing agent. Cell. Polym. 2012, 31, 189–206. [Google Scholar] [CrossRef]
- Zhao, C.; Mark, L.H.; Kim, S.; Chang, E.; Park, C.B.; Lee, P.C. Recent progress in micro-/nano-fibrillar reinforced polymeric composite foams. Polym. Eng. Sci. 2021, 61, 926–941. [Google Scholar] [CrossRef]
- Xiang, B.; Jia, Y.; Lei, Y.; Zhang, F.; He, J.; Liu, T.; Luo, S. Mechanical properties of microcellular and nanocellular silicone rubber foams obtained by supercritical carbon dioxide. Polym. J. 2019, 51, 559–568. [Google Scholar] [CrossRef]
- Bargmann, S.; Klusemann, B.; Markmann, J.; Schnabel, J.E.; Schneider, K.; Soyarslan, C.; Wilmers, J. Generation of 3D representative volume elements for heterogeneous materials: A review. Prog. Mater. Sci. 2018, 96, 322–384. [Google Scholar] [CrossRef]
- Kanit, T.; Forest, S.; Galliet, I.; Mounoury, V.; Jeulin, D. Determination of the size of the representative volume element for random composites: Statistical and numerical approach. Inter. J. Solids Struct. 2003, 40, 3647–3679. [Google Scholar] [CrossRef]
- Heydari, A.; Vahidifar, A.; Esmizadeh, E.; Rodrigue, D. Experimental and finite element simulation of natural rubber foams using real 3D structures. Polymer 2020, 197, 122505. [Google Scholar] [CrossRef]
- Hamza, M.N.; Alwan, H.M. Hyperelastic constitutive modeling of rubber and rubber-like materials under finite strain. Eng. Tech. J. 2010, 28, 2560–2575. [Google Scholar]
- Muhr, A. Modeling the stress-strain behavior of rubber. Rubber Chem. Technol. 2005, 78, 391–425. [Google Scholar] [CrossRef]
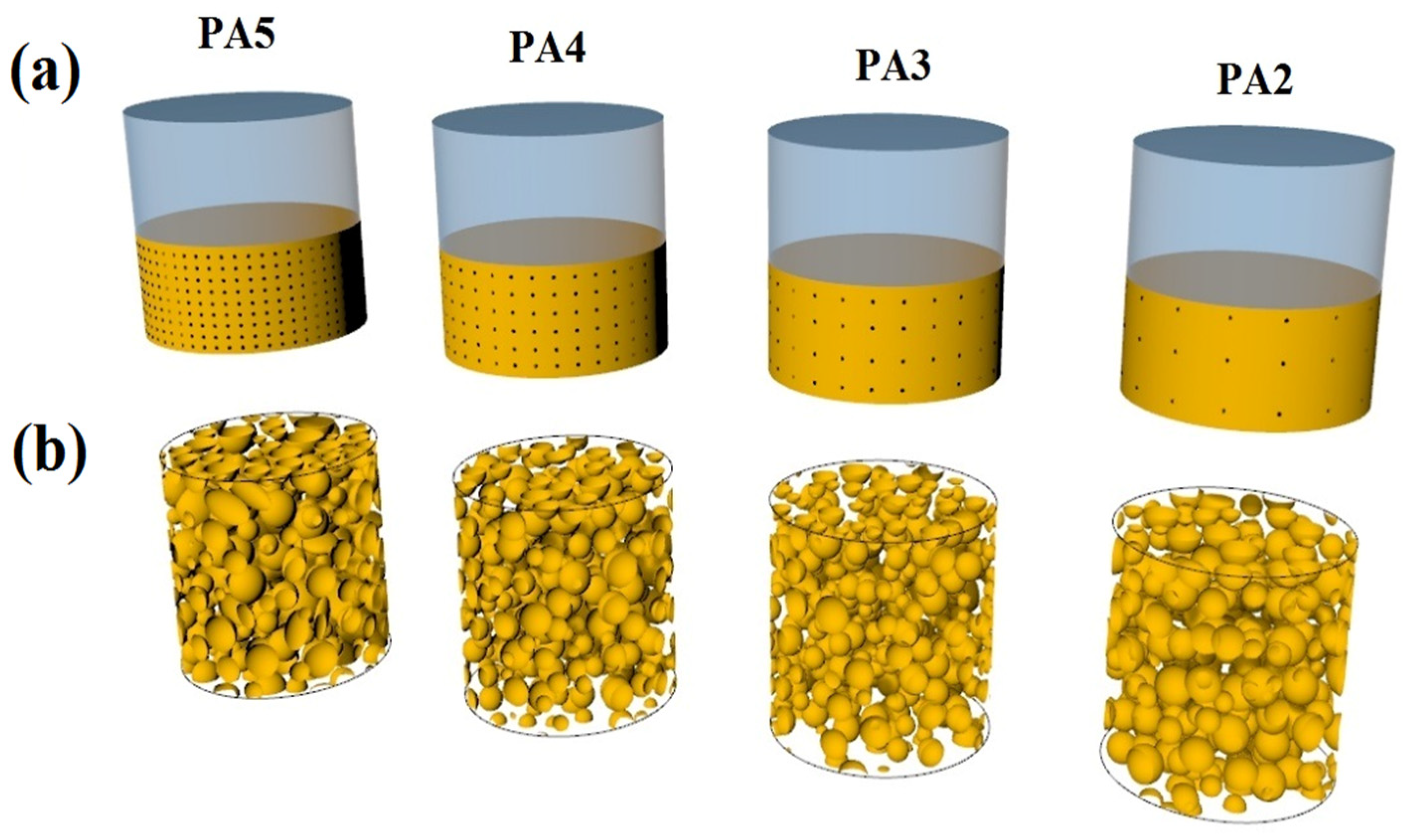
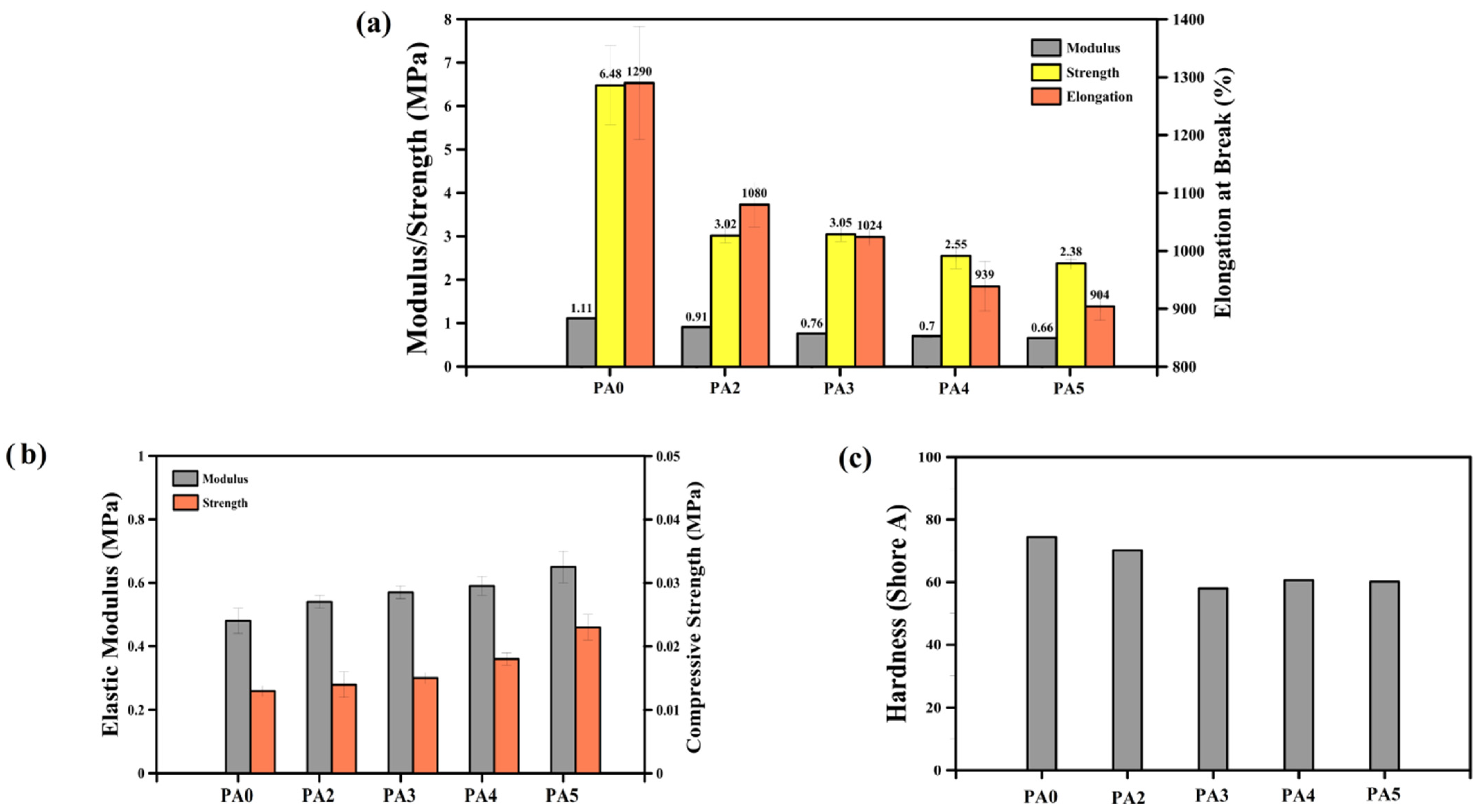


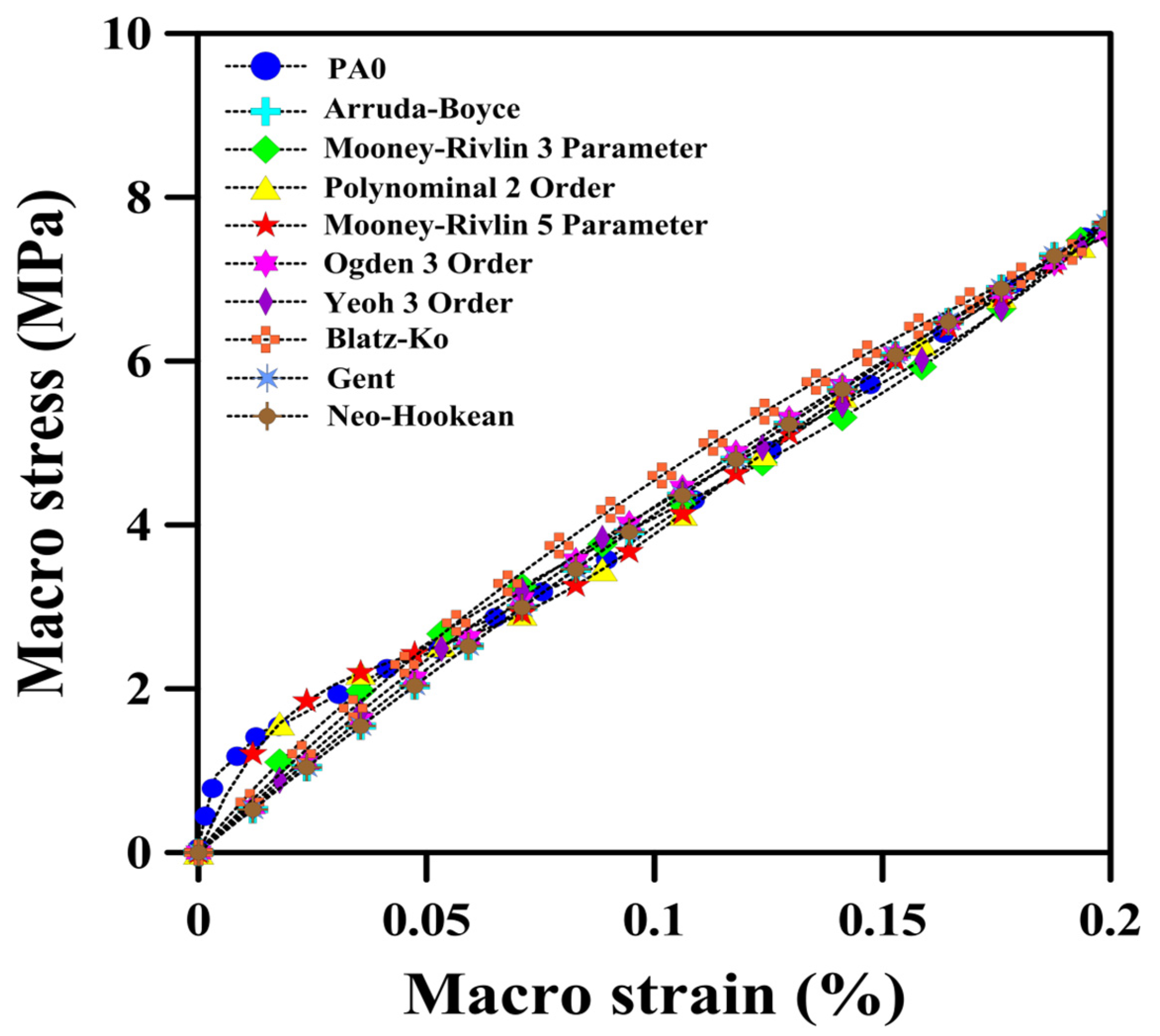

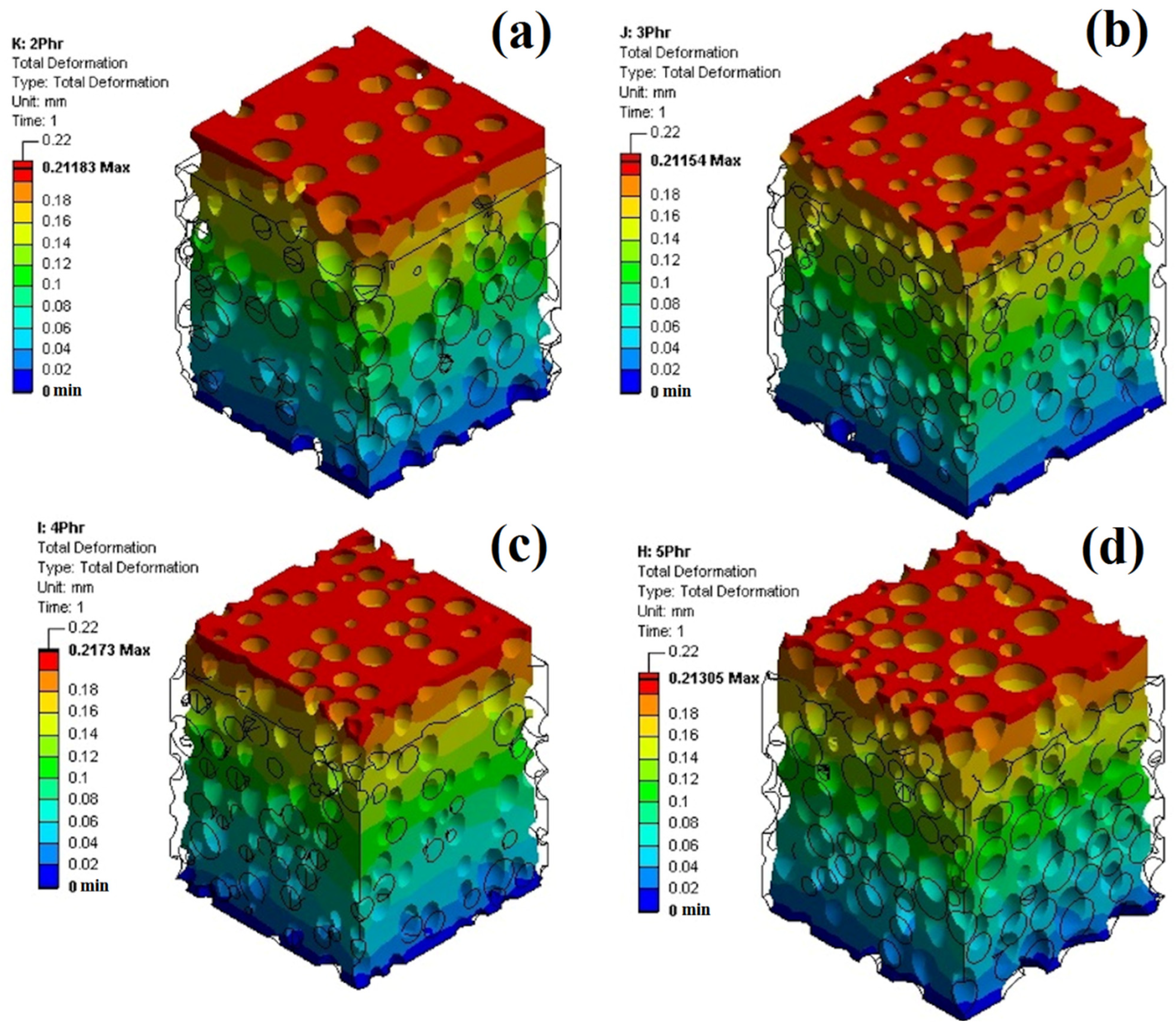

| Sample Code | PEO (phr) | ADC (phr) |
|---|---|---|
| PA0 | 100 | 0 |
| PA2 | 100 | 2 |
| PA3 | 100 | 3 |
| PA4 | 100 | 4 |
| PA5 | 100 | 5 |
| Main Equation | Feature | Equation | |
|---|---|---|---|
| Number average cell size | Number average diameter of cells | (2) | |
| Weight average cell size | Weight average diameter of cells | (3) | |
| Polydispersity index | Cell size distribution | (4) | |
| Cell density | Population and number of cells inside the matrix | (5) |
| Material Constant | Value |
|---|---|
| C01 | 489.3824 |
| C02 | 13,268.73 |
| C10 | −467.077 |
| C11 | −20,653.2 |
| C20 | 8267.356 |
| Hyperelastic Model | Mean Square Error (MSE) | Root Mean Square Error (RMSE) |
|---|---|---|
| Arruda–Boyce | 0.1029 | 0.3208 |
| Mooney–Rivlin 3 Parameter | 0.0542 | 0.2329 |
| Polynomial 2nd Order | 0.0104 | 0.1024 |
| Mooney–Rivlin 5 Parameter | 0.0104 | 0.1023 |
| Ogden 3rd Order | 0.1011 | 0.3179 |
| Yeoh 3rd Order | 0.0836 | 0.2892 |
| Blatz–Ko | 0.1257 | 0.3545 |
| Gent | 0.1031 | 0.3211 |
| Neo-Hookean | 0.10294 | 0.32083 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rostami-Tapeh-Esmaeil, E.; Heydari, A.; Vahidifar, A.; Esmizadeh, E.; Rodrigue, D. Experimental and Finite Element Simulation of Polyolefin Elastomer Foams Using Real 3D Structures: Effect of Foaming Agent Content. Polymers 2022, 14, 4692. https://doi.org/10.3390/polym14214692
Rostami-Tapeh-Esmaeil E, Heydari A, Vahidifar A, Esmizadeh E, Rodrigue D. Experimental and Finite Element Simulation of Polyolefin Elastomer Foams Using Real 3D Structures: Effect of Foaming Agent Content. Polymers. 2022; 14(21):4692. https://doi.org/10.3390/polym14214692
Chicago/Turabian StyleRostami-Tapeh-Esmaeil, Ehsan, Amirhosein Heydari, Ali Vahidifar, Elnaz Esmizadeh, and Denis Rodrigue. 2022. "Experimental and Finite Element Simulation of Polyolefin Elastomer Foams Using Real 3D Structures: Effect of Foaming Agent Content" Polymers 14, no. 21: 4692. https://doi.org/10.3390/polym14214692
APA StyleRostami-Tapeh-Esmaeil, E., Heydari, A., Vahidifar, A., Esmizadeh, E., & Rodrigue, D. (2022). Experimental and Finite Element Simulation of Polyolefin Elastomer Foams Using Real 3D Structures: Effect of Foaming Agent Content. Polymers, 14(21), 4692. https://doi.org/10.3390/polym14214692