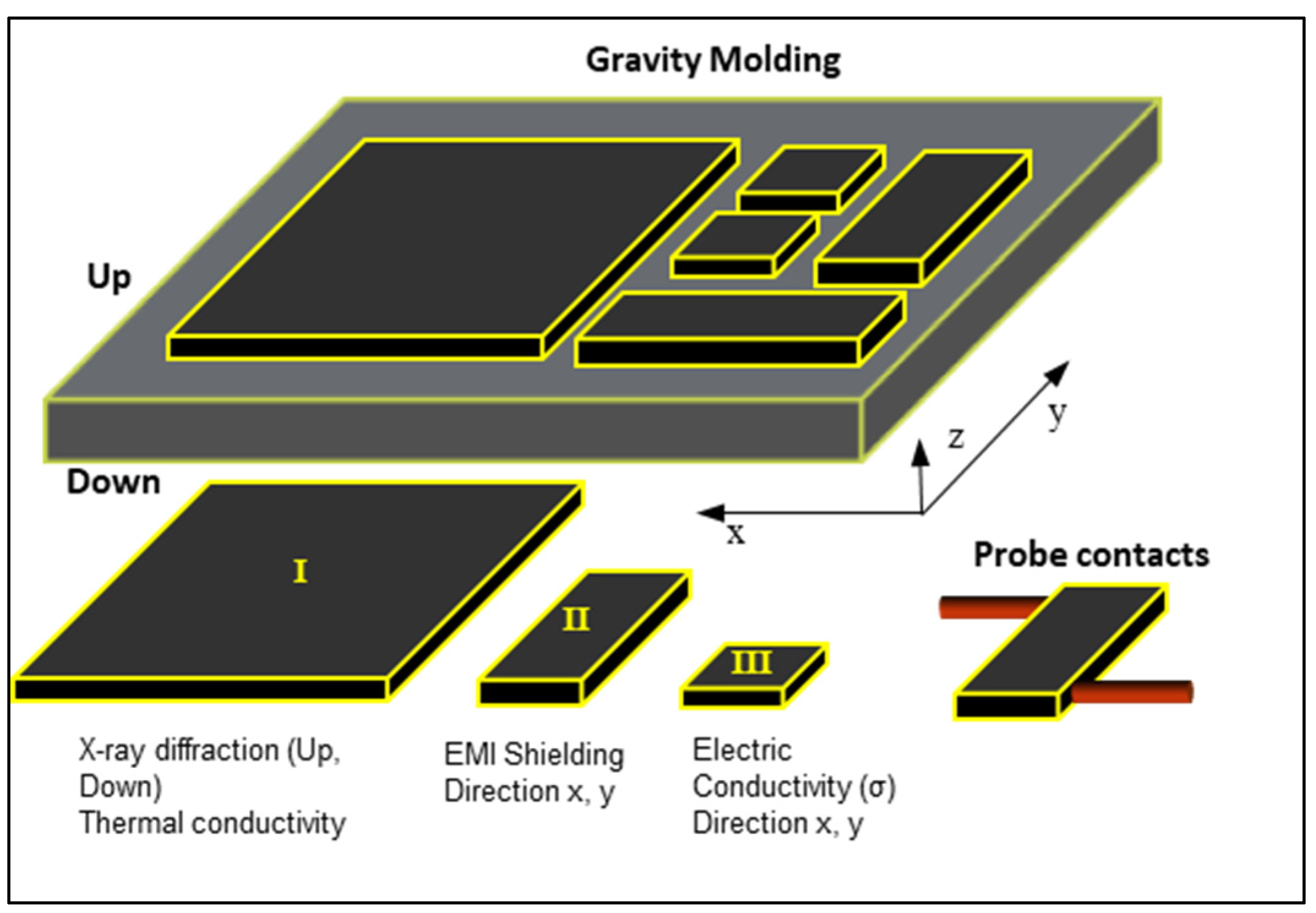
3.1. Analysis of Self-Stratification in Nanocomposites
The effect of GNP layering or sedimentation as a function of the average graphene area was measured by XRD and is presented in
Figure 3, as a function of the sonication time applied. As it can be seen, the first peak belonging to the amorphous halo of the epoxy resin keeps its intensity approximately constant from left to right (upper part), so the type of GNP does not affect the formation of the GNP-poor upper layer. However, the analysis at the top also shows that the GNP content decreases when moving from left to right, indicating that reducing the average lateral size, and increasing the surface area of the graphene produces greater sedimentation, reducing the graphene content on the upper surface. Self-stratification is also verified by the difference in intensity of the main peak between the upper and lower surface belonging to graphite at 26.5°, which corresponds to the (0 0 2) plane COD 96-120-0019 [
51,
52]. The intensity of this peak on the lower surface is twice as high with respect to that recorded on the upper surface. Moreover, the presence and coincidence in intensity of peaks 2θ = 54.6°, 77.4°, and 83.5° belonging respectively to the (0 0 4), (1 1 0), and (1 1 2) planes in all spectra, demonstrate that exfoliation is residual, unlikely, and exists to an insignificant extend.
The non-uniform distribution of the graphene in the thickness of the sample negatively affects the mechanical properties, since in the bottom zone a large number of agglomerates are generated, inducing a situation similar to the one that would be caused by a bad dispersion [
53]. However, it can generate interesting applications or advantages by also modifying the electrical and thermoelectric properties; for example, generating preferential paths in the conductivity for certain applications in multifunctional systems, such as localised heating, is useful. In addition, the selective exploitation of thermoelectric properties near the bottom surface improves the thermal transfer to the substrate, while slightly heating the top surface reduces dilatometric changes and improves compatibility with other coatings of lower thermal resistance.
The dispersion state of GNPs was evaluated by SEM, using previously prepared specimens in their cross section.
Figure 4 shows the panoramic images (stitching) belonging to two systems based on C300 and C750 (only the extremes are analysed), dispersed using sonication times of 30, 60, and 120 min. Panoramic imaging of the areas near the bottom surface of all specimens was performed using the backscattered electron detector (BSED). The contrast by atomic weight difference produced by the detector allows differentiation of the layering and sedimentation of the GNP agglomerates near the bottom surface. Segmentation of the images has allowed us to quantify the dispersion in a region of 1.5 mm from the bottom edge.
As expected, the self-stratification and agglomerate formation are more intense in the C750, as indicated by Stokes’ law. The agglomerates formed in the bottom of this sample are twice the size of those observed in the C300 sample due to their higher specific area. Agglomerate formation and self-stratification are reduced as the sonication time increases, because of fragmentation/breakage of the graphene nanoplatelet agglomerates. On the other hand, this method seems not to produce exfoliation, if not fragmentation of the GNP, so it does not improve the connectivity between graphene platelets. Consequently, it deteriorates the electrical and thermal conductivity of the composite material, as will be shown in the next section.
The segmentation and analysis of the dispersion of nanocomposites have allowed us to quantitatively evaluate the state of agglomerates and GNP fragmentation during processing (
Figure 5). The data obtained were filtered considering that particles greater than 1 μm were designated as agglomerates, while smaller than 1 μm would be considered fragments of the nanoplatelets of the initial graphene. This separation allowed excluding the agglomerates formed during processing, verifying that the longer the sonication time, the more intense the fragmentation and the lower the connectivity.
The effect of sonication is different for each type of graphene, observing that for C300 the fragmentation of the agglomerates mainly occurs in the first 30 min (
Figure 5a), while in C750 the fragmentation of agglomerates occurs during the 120 min test (
Figure 5b). It is also important to note that between 60 and 120 min the C300 system undergoes an increase in the size and number of agglomerates, which allows us to affirm that sonication is ineffective, probably due to an increase in density. The energy provided during sonication is used by the system to facilitate Brownian motions that increase the probability of intricacy between the GNPs, agglomerating them.
On the other hand, it is confirmed that the lateral size after sonication of C300 GNPs is larger than C750 (
Figure 5c,d). Moreover, it is observed how for C300 (
Figure 5c), an increase of the sonication time from 60 to 120 min leads to a higher fragmentation of the GNPs, so that the sonication energy is able to break the GNPs, which does not occur for 30 and 60 min because they almost all form agglomerates in the sedimentation layer. Finally, from
Figure 5d it is concluded that an increase in sonication time generates GNP sizes smaller than 0.3 μm, which are not shown because this is the minimum dimension that the SEM used can study. This implies that an increase in the sonication time generates a breakage of the GNPs, decreasing their lateral size.
The analysis of the cross section by Field Emission Gun—Scanning Electron Microscope (FEG-SEM) applying oriented cryofracture allowed the evaluation of the dispersion of GNPs in the matrix and, at high magnifications, to verify the state of graphene exfoliation.
Figure 6 shows the images obtained after carrying out a progressive sequence from the agglomerate in the self-stratified zone to the detail of the cross section of non-exfoliated graphene packing. It is observed that the non-exfoliated graphene platelets present thicknesses greater than 50 nm in cross section. This could generate how the conductive properties, both thermal and electrical, are lower than expected, being overcome with the use of other types of graphene [
31], as will be confirmed in the next section. In addition to the thickness, the fact that the graphene in this case is not functionalised also plays a role, which results in a weak interface.
3.2. Electrical and Thermal Conductivity Analysis
Figure 7 shows the electrical and thermal conductivity of the composites depending on processing sonication time and the type of graphene. It is important to highlight that the electrical conductivity is studied in the direction of the plane, while the thermal conductivity is studied in the z direction, to know its potential application in heated coatings. The results reveal that, when using C300 and C500, an increase in the sonication time produces a drop in conductivity, and this drop is determinant between 30 and 60 min. This drop in conductivity can be justified by the amorphization transformation undergone in the graphene structure [
47]. However, between 60 and 120 min, a rise in the electrical conductivity is shown, which may be due to the stabilisation of the appearance of defects and amorphization while continuing to generate a better dispersion [
54]. This last hypothesis is justified by the results of XRD, decantation size, and fragmentation observed for C300 in the stratification analysis section (
Figure 3,
Figure 4 and
Figure 5), since it is observed therein that with increasing sonication time from 60 to 120 min, the decantation size and amount of GNPs at the bottom grows; there is not agglomerate breakage, but fragmentation of GNPs is relevant.
It is interesting to note that C300 presents a greater slope of conductivity drop compared to C500, which means that amorphization and GNP fragmentation occurs more easily, which could mean that electrical stabilisation is reached earlier. This greater ease of amorphization would be justified by a lower presence of impurities, while fragmentation is more effective as it has a lower tendency to form agglomerates (due to its smaller specific area).
The results obtained when C750 is used show that the conductivity drop is visible between 30 and 120 min, losing slope as time goes by due to the decrease of the lattice damage produced on the structure. It is interesting to note that these values, together with those observed for C300 and C500, allow us to make a strong hypothesis about the different influence of the surface area and lateral size on the damage stabilisation time, since as the surface area increases and the lateral size of the GNP type decreases, the time until conductivity stabilisation increases. This behaviour observed for C750 is justified by the results observed in XRD (
Figure 3), SEM stitching (
Figure 4), and fragmentation analysis (
Figure 5). In both changes of times, from 30 to 60 min, and 60 to 120 min, the size of sedimentation and the quantity of GNPs are lower; additionally, the fragmentation is high, although it is lower between 60 and 120 min due the increase in viscosity.
A greater specific area, maintaining constant dimensions, seems to lead to a probabilistic increase in the existence of greater rupture events, which from the mechanical point of view are favoured by the possibility of the appearance of greater net stresses linked to larger dimensions, such as bending moments. Increasing the dimensions, for a constant geometry, would lead to the same. However, when observing the induced damage as a linear combination of lateral size and specific surface area, the slope accompanying the lateral size must be greater for the experimentally observed data to have justification, since if both slopes were equal, the one that would take longer to present an increase in conductivity due to exfoliation would be C500. In contrast, if a greater weight is given to the lateral size of the GNPs, the electrical behaviour with the increment of sonication time has justification.
The above is feasible from the physical process point of view, since the collision of a bubble during sonication, which is what gives rise to the external forces acting on the GNPs, has an area of action around few microns (0.1–25 μm) as observed in papers [
55,
56,
57] analysing the evolution of bubbles generated during the sonication process under conditions similar to those present in the current project. Moreover, considering how the dissimilarities between the resin loaded with GNPs and the fluids used in the articles [
55,
56,
57,
58] affect the process, it can be justified that the smaller size is possible because our fluid presents a much higher viscosity, and lower specific heat (and therefore specific heat ratio) and density. The fact that the zone of action of the bubble jet is in the order of microns means that as the lateral size of the GNPs decrease, there is a lower probability of fragmentation as the generation of a bending moment is less probable. This explains the difference in behaviour between the types of GNPs together with the rest of the justifications.
To the above, it must be added that the manufacturer provides the elemental concentration percentages of the different GNP types, indicating that the highest presence of impurities is in C750. This implies that initially the effect of amorphization and damage in its lattice is less because there are particles that are not GNPs present, that means that over a longer period of time you can cause damage. Ultimately, C750 structure is the most suitable to lengthen the effect produced by the damage of sonication.
In summary,
Figure 7a shows that the highest conductivity values occur for specimens with C750 with sonication times of 30 min, followed by C300, and finally C500. The most conductive specimens have in common a minimum sonication time, which allows affirmation that sonication is not adequate beyond 30 min. It can even be doubted, because the conductivity values for times shorter than 30 min are unknown, whether it is really beneficial to perform such a long sonication stage when there is self-stratification. In addition, the longer sonication times end up generating an inversion of the conductivity domain according to the types of GNP, converting the one that at short times was the least conductive into the most conductive, and vice versa. For times of 60 min there seems to be an intermediate transition phase in which C500 already presents a higher conductivity than C300, so that C500 seems to present a great resistance to the drop in conductivity with the passage of time compared to the other types of GNP.
The highest conductivity obtained for C750 is higher for 30 and 60 min, because during the first 30 min, this type of graphene received less damage and amorphization, which has been already justified. Moreover, its effective conductive volume linked to the sedimentation size is higher than in C300 and C500 because the bigger specific area results in high agglomeration (bigger size of agglomerates results in higher sedimentation velocity, according to Stokes’ law). However, sonication times above 60 min generate an increment of sedimentation size in C300 and C500, which produces as a consequence a higher conductivity by its relation with the effective conductive volume. Bonded to this, C750 continues losing conductivity, its value falling under the others due to the reduction of conductive volume (the electrical resistance augment) and increase in fragmentation.
A different behaviour was observed in the thermal conductivity measurements in the Z direction (
Figure 7b). The measurement is made from side to side of the specimen in the Z direction, so that the sedimentation is very influential in the result. Knowing the thermal behaviour in this direction, it is interesting to check the potential use of the material as a heating coating.
In the C300 and C500 systems, the decantation degree decreases with the sonication time, resulting in a greater amount of GNPs in the rest of the sample, which generates a local increase in the thermal conductivities. Added to the previous, it is known that there is an increase in viscosity as the sonication time increases [
33], which generates a better relationship between the exfoliation induced by the cavitation forces and the breakage of the GNP, supporting this increase in thermal conductivity. However, in the C750 system it is the opposite, since increasing the sonication time leads to a decrease in thermal conductivity. For this type of graphene, although it is observed that there is a progressive elimination of the self-stratification zone with the sonication time (
Figure 4), it is observed that the GNP is not properly dispersed throughout the thickness, finding agglomerates of larger sizes than those observed for C300, which are becoming more and more uncommunicated with the pass of sonication time (
Figure 4b). This, together with the increment of GNP fragmentation that decreases the lateral size, are the main causes of the thermal conductivity decrease with increasing sonication time [
59].
It can also be concluded that the thermal conductivity decreases with increasing surface area and decreasing lateral size, that is, when we go from C300, to C500 and C750, since a higher specific surface area can lead to a higher porosity of the GNP, a defect that impairs the thermal conductivity [
47]. Moreover, the smaller lateral size of nanoplatelets negatively influences the thermal conductivity value [
60], not only because it decreases the intrinsic conductivity of GNPs, but also because it induces a lower increase in the glass transition temperature of the compound [
61]. The results obtained from X-ray diffraction (
Figure 3) also explain that the thermal conductivity in C750 should be the lowest, since lower amounts of GNPs are observed in the upper zone. This generates that the zone with the lowest amount of GNPs (the upper one), which act as the limiting part of the system, act as an insulating thermal barrier.
As a conclusion, it can be stated that the composites nanoreinforced with GNPs improve their thermal conductivity for longer dispersion times due to the decrease in the size of the decantation zone, except for the composite type C750, because it has a heterogeneous distribution of graphene in the Z direction (
Figure 1). It is also confirmed that an increase in the specific surface area and a decrease in the lateral size imply a lower thermal conductivity.
The results obtained from the picoammeter are shown in
Figure 8, which shows that all the samples have extremely low values of electrical conductivity in the Z-direction with respect to the conductivities measured on the XY-plane, although in all cases, as it was expected, the conductivity of the neat epoxy resin was improved.
It can be concluded that increasing the sonication time from 30 to 120 min, for all types of GNPs, generally results in a drop in conductivity, which is related to the amorphization, and the decrease in lateral size (fragmentation) generated on the GNPs during sonication. These two parameters are more influential than the dispersion obtained by the agglomeration fragmentation and consequent decrease in thickness of the segregated zones. This is because the XRD spectra indicated that this greater dispersion did not prevent the existence of the upper dielectric layer of low GNP content, whose GNP content is considered as a limiting parameter on the electrical conductivity in the Z direction.
The fact that the conductivity for equal times is higher in C300 than in C500, and superior in C500 than in C750, is justified by stating that the larger lateral size of C300 and C500 causes the GNPs to have a greater difficulty to decant. Because a larger size implies a greater hydrodynamic resistance, this translates into a greater amount of GNPs in the upper zone of the sample. This higher quantity of GNPs in the upper zone is confirmed with the XRD analysis (
Figure 3).
3.3. Joule Heating
The results of the temperatures reached when different voltages are applied in all the specimens and in both directions (x,y) contained in the plane have been obtained; however, only the results of the specimens that have given off noticeable heating will be shown. These samples are those manufactured with 30 min of sonication, because their higher electrical conductivity implies a greater Joule effect due to the increase of the current flow [
62]. It is important to note that the powers used for heating do not exceed 10 W at any time, so the system shows good energy efficiency.
To analyse the thermal behaviour of the fabricated systems, the activation voltages have been studied (
Table 1), which represent the potential difference at which two different zones of the material have to be imposed to mobilise the charges sufficiently to generate the Joule effect. This parameter is related to the ease of each system to be heated, that is to say, a low activation voltage indicates greater ease of heating of the system. In this case, the activation voltage has been defined as the voltage necessary to observe in the thermal chamber an onset of heating of the sample above 10 °C, which is the minimum temperature required in some applications of industrial interest [
63,
64]. It is important to highlight that the activation voltages have been defined as intervals which collect all the values of activation voltages obtained, since several specimens have been analysed in both directions belonging to the plane (x and y).
All the above implies that only systems with a sonication time of 30 min are of interest for their application in industry, since longer sonication times imply too high values of necessary voltage, so only the systems that have been manufactured with that sonication time will be analysed.
Figure 9 shows that the system with C750 has the highest Joule effect heating capacity, followed by C300. This a priori makes physical sense with the results obtained in the characterisation of electrical conductivity in DC, since a higher electrical conductivity leads to a higher current flow (intensity), and it is known that heat generation grows exponentially with increasing current intensity [
62].
The specimens incorporating C750 show a greater Joule effect because the associated impurities cause a lower thermal conductivity [
37,
65], which locally increases the heat, resulting in a greater intervention of phonons in a localised area. This phonon intervention would explain the early onset of the exponential trend since the electrical conductivity is linked to the multiphonon-assisted Joule effect [
66]. The fact that the C750 system shows a higher error in the values obtained is due to the existence of a larger number of specimens that presented preferential percolated conductive paths. Apart from the above, it is known the heat actually accumulates to a greater extent in C750 than in C300 due to lower thermal conductivity (
Figure 7b). That, together with the higher electrical conductivity of C750, make up the basis for the difference between the temperatures reached. The existence of an initial trend with a greater slope is justified by the difficult heat transfer to the outside.
All the analysed specimens have been plotted according to the type of GNP, distinguishing the axis on which the potential difference was established (x or y), and for a constant sonication time of 30 min (
Figure 10), since, as previously mentioned, this is the time that has allowed for reaching the highest temperatures.
Figure 10a,b shows that the Joule effect in the specimens containing C300 and C500 depends weakly on the axis on which the bias is established. This dependency is observable on the difference between the maximum temperatures reached, highlighting that this difference is not high if the deviations of the different specimens analysed are taken into account. The above results show that the processing conditions of C300 expose that there is a slight anisotropy of the thermoelectric properties within the plane. This would not be a problem if you want to do Structural Health Monitoring (SHM) using the Joule effect, but it would have to be taken into consideration when doing SHM. It is important to note that in all the graphs in
Figure 10, the wide errors are associated with the existence of preferential conductive paths, due to how their formation is uncontrolled.
Using C750 (
Figure 10c) allows for reaching temperatures twice those obtained by C300, keeping the bias and activation voltage values similar. Here the difference is that there is one single zone instead of two in which the presence of preferential conductive paths has been detected. Therefore, the homogeneity previously observed for C500 is broken when using C750, since each specimen shows a unique heating, i.e., the system shows unpredictable behaviour from the modelling point of view. The origin of the formation of the preferential conductive paths may be linked to a process of physical self-organisation due to the appearance of preferential zones for the occurrence of electrostatic interactions that generate agglomerates.
In all cases, the existence of four stages has been observed (
Figure 11a). First, there is a voltage range for which the temperature does not vary, and second, where the temperature increases linearly. Then, a potential tendency for an increasing bias (whose exponential value depends on the type of GNPs used) is observable. Finally, a fourth, in which the slope of the potential function increases progressively and is corrected by means of an exponential function depending on the temperature of the material itself (as a product of functions).
Before justifying the existence of these four stages, it is hypothesised that there are only two paths for the transport of charge carriers within these materials (zone “A” is considered to have insufficient conductivity), which could be visualised as two parallel paths (
Figure 11b). The first would be the discontinuous paths (or capacitive path), which are generated in the areas (
Figure 11b zone B) where the material is above and near the percolation threshold. In this type of pathway, we have tunnelling electron transport channels through the epoxy separating the GNPs in the nanocondensers of the system, which dominate for a bias value below a voltage, which we will call critical voltage. Secondly, we have the additional pathways to the previous one formed by the cold emission effect, hopping conduction, and thermionic emission that arise when the critical voltage is exceeded. The next transport path is the so-called continuous path (or resistive path), which appears in areas
(Figure 11b zone C
) of the material above the percolation threshold, where all the above named types of conduction occur with possible ohmic drift conduction in the preferential conductive paths [
67,
68]. All these conduction methods depend on the differently applied bias voltage (
Table 2). Area “A” of
Figure 11b represents the zone that has not attained percolation threshold.
It is important to note that temperature plays an important role (
Table 2), since the increase in temperature leads to an increase in the phonon population. This causes the appearance of hopping conduction (since it is a process that requires thermal activation in which electrons exchange energy with the phonon system), leading to an increase in conductivity (negative temperature coefficient). It also allows the occurrence of thermionic emission and affects the cold field emission [
71]. However, in continuous paths (and in conduction through the GNPs or their agglomerates) the effect is directly proportional to how the temperature modifies the GNP conductivity, and in this case, the negative temperature coefficient of the resistor is depreciable compared to that in discontinuous paths [
73].
In the first stage, the material shows very low conductivity, so no energy dissipation is generated, and the temperature does not vary. The resistance of the continuous (resistive) path is large, and for the given voltage, the current is of the order of microamperes, so the Joule effect is not generated or is negligible. The tunnelling effect condition is hindered by the Coulombic repulsion energy generated in the nanocondensers of the discontinuous (capacitive) paths [
74]. In this first stage, drift (shallow) and tunnel effect conduction (when V < 50 V) are associated with a linear I–V relationship (
Figure 11a, A to B), although for some cases it has been observed that tunnel effect conduction can be associated with a third order polynomial relationship for V > 10 V [
69]. The observed results indicate that conduction below the activation voltage (
), approximately 50 V, does not induce heating.
In the second stage, linear temperature growth is achieved after exceeding the activation voltage. This linear conduction (
Figure 11a, B to C) arises because there is ohmic conduction by drift in the continuous or resistive vias. To this is added the linear behaviour of tunnel transport predicted by the mixed NLRRN + DRRN model at voltages below the critical voltage [
75,
76], which occurs in the conductive or capacitive vias. The tunnel effect can be taken as linear, since in this case up to about 100–125V no potential I–V trend is observed. The measured temperature growth should have second order potential due to the linear I–V relationships (
Table 2); however, with the appropriate coefficients a potential trend can be approximated to a linear one.
In the third stage, the potential growth is available. The critical voltage (
) has been exceeded and, according to the NLRRN (nonlinear random resistor network)–DRRN (dynamic random resistor network) mix model, additional conductive paths formed by the cold emission effect are activated (
Figure 11a, C to D). This effect occurs due to the strong electrostatic fields generated between the GNPs (nanocondensers) in the discontinuous path. In addition, hopping and thermionic emission conduction can be taken into account, although both are exponentially temperature dependent, being depreciable for values below the thermal activation voltage (
). Tunnelling conduction above the critical voltage, i.e., at this stage and the following one, has an associated quadratic–exponential I–V relationship (
Table 2), which explains, together with the quadratic function of the field cold emission, the polynomial trend of the T–V relationship (
Figure 10a). The temperature can generate a variation of the temperature obtained because the field emission has a second order potential I–T relationship.
In the final stage, a potential–exponential growth is observed (
Figure 11a, D to E). The increase in the slope of the potential trend is due to the fact that the increase in temperature allows a hopping conduction (deep conduction) as the phonon population increases, and to a lesser extent a thermionic emission [
68]. Interaction with phonons in the insulating matrix induces current growth [
74], basically phonon-assisted electron hopping is observed, where phonons facilitate electrons having energy higher than the activation energy gap of the nanocomposite [
66]. Other authors say that this behaviour is a consequence of the polymer chains and impurity ions acting as traps for charge carriers to move through the hopping process [
77].
Figure 12b shows the general equivalent electrical circuit proposed for the behaviour observed in the polymer nanocomposite with sedimentation. Each zone is compounded by a basic circuit, where each component represents different effects, and the meaning and phenomena are linked to the components described in the table in
Figure 12c. Components are situated as parallels because the phenomena can occur at the same time, and each phenomenon can create new conductive paths for electrons.
The existence of a possible lower overlapping of the GNPs, which results in less viable area for the generation of the tunnelling effect, explains why there are different critical voltages for specimens of the same system, i.e., the critical voltage could depend on how the GNPs are oriented with respect to each other, as occurred for the electrical resistance in the conductivity model proposed by Sánchez-Romate and co-workers [
31]. The activation and critical voltage would depend only on the resistance of path, which could be found mainly in sedimentation zone. Finally, it is important to note that the tunnel effect is mainly generated in zone C (
Figure 11b), where the tunnel resistivity is lower due to the low tunnel distances between GNPs. This explains why in the thermal chamber a faster heating is observed in the sedimentation zone.
In the videos collected by the thermal camera it is verified that the start of heating is sometimes not generated in the whole area as is common (
Figure 13a), observing this in a marked line (
Figure 13b), so the system achieves a faster heating. This is due to the fact that heat cannot be transmitted at a similar speed as the rest of the specimens due to the lack of graphene in the areas near the preferential path (
Figure 14). This generates a faster increase in temperature, which in turn raises the participation of phonons in the electrical conduction by momentum transmission to the electrons [
78]. Thus, a feedback loop is generated, in which the excess of graphene concentrates the charge, the lack of graphene around it concentrates the heat, the conductivity increases due to the increase in heat, and the heat increases due to the growth of the conductivity.
It is predicted that the origin of this phenomenon is found in the difference in the GNP content between the preferential conductive path and other areas. The low graphene content in the external areas causes thermal isolation due to the lower thermal conductivity coefficient induced. Moreover, the high graphene content generates a lower distance between GNPs, which is translated into high tunnel effect conductivity (high current flow in this path). These two phenomena generate the feedback loop previously mentioned, and as an effect of this appears before the thermal activation condition, in this case hopping conduction, it justifies the existence of lower critical voltage in this path.
For better understanding the origin, this phenomenon was studied using FEG-SEM microscopy (
Figure 14). Observing that the conductivity around the path is limited by the lower GNP content found (
Figure 14a–d), the hypothesis given above was correct. That is, the heating line pattern is caused by a path where the percolation threshold has been exceeded, while in the rest of the sample in the same layer, the GNP content is lower and therefore exerts a greater opposition to the passage of current.
Figure 14e presents the images of the microstructure of the cross section of one of the tested specimens belonging to C750. In this progressive sequence of images taken at different magnifications, it is possible to observe: the carbonized region, the area affected by the heating, the state of the graphene dispersion in the areas surrounding the integrated conductor, the agglomerates present in the layered area, and the inner part of the agglomerate. In summary, although the 30 min sonication cycle leaves a large number of agglomerates, the platelets present a homogeneous distribution in the space between the agglomerates.
Finally, in order to compare the Joule effect generated by each type of GNP,
Figure 15 shows the values of maximum temperature and heating rate reached by the systems analysed, as well as the averages of the two previous values.
The results shown in
Figure 15a allow us to conclude that the maximum temperature reached for all the voltages of interest is linked to the use of C750, reaching up 374.4 °C at the maximum voltage. Additionally, those in
Figure 15b indicate that the average maximum temperature is also obtained using C750 for all the voltages evaluated, at 219.1 °C. The graphene that records the worst temperature values is C500, while C300 exhibits intermediate values between the two previous ones.
Figure 15c shows that the maximum heating rate value is achieved using C750 for all voltages, with a value of 313.3 °C/min for 500 V. On the other hand,
Figure 15d shows that when the heating rates are averaged, C750 is still the optimum system, but with a lower maximum of 104.3 °C/min. Again, in this case it is C500 that presents the worst values, and C300 is in between the other GNP types.
3.4. Electromagnetic Signal Absorption Behaviour in Nanomaterials with Different Specific Surface Areas
The values of conducted electromagnetic shielding at low, medium, and high frequency, that is, between 100 Hz and 20 MHz, have been obtained for all type I specimens, which allows us to have information on how the attenuation of the electromagnetic signal propagated by conduction varies in different material directions, and therefore whether the transmission of the same is independent of the heterogeneities that the material may present, such as the different segregation thicknesses obtained in the different systems.
Figure 16 shows how in C300 there is different behaviour between the signal propagation in the X and Y directions. This difference increases as the sonication time is increased, but for all times it is observed that the shielding difference between the different directions decreases with increasing frequency until reaching a zone where the shielding is independent of direction, sonication time, and frequency. This zone is represented by the mid-frequency band 0.3–3 MHz.
In C500 the same behaviour is also observed of the shielding between the signal propagation according to the direction increasing with the increment of sonication time. Again, the shielding difference decreases until reaching the stability band of 0.3–3 MHz. For C750 it is not observed that the shielding variation between different directions depends on the applied sonication time, but again the existence of the stable shielding zone in the mid-frequency band is observed.
It can therefore be concluded that there is a medium frequency band between 0.3–3 MHz in which the shielding value is independent of the sonication time, the direction of signal propagation, and the frequency, since there are variations of the shielding value of less than 1%. If the variations of the output amplitude versus the signal input amplitude are also analysed, statistically, values lower than 0.5% are obtained. This enables potential SHM applications in this frequency range, since the variations of the deformation state can be related to the variations of the shielding or amplitude ratio in this mid-frequency band. This variation of conductive EM shielding with the deformation has been verified by performing a three-point bending test, concluding that the sensitivity is low due to the high rigidity of the epoxy matrix (it is only possible to observe a significant difference in the signal when the material suffers damage, such as cracks).
The behaviour shown in
Figure 16 reveals three stages, which coincide with the intervals of low, medium, and high frequency. For low frequency, the drop in conductive shielding with increasing frequency is directly related to an increase in the conductivity of the nanocomposite. The conductivity of the nanocomposite increases with increasing frequency because the large amount of GNPs in the matrix produces a strong Maxwell–Wagner–Sillars effect, which disappears as the charges accumulated at the interfaces decrease, resulting in a drop of dielectric constant (Ɛ’), linked to an increase of dielectric loss (proportional to the electrical conductivity) [
79]. This means that the dipoles inside the microcapacitors disappear, giving a high mobility of the carriers through the hopping conduction mechanism by using the defects of the polymeric chains [
80]. This conduction is possible because the losses produced by the impossibility of the dipoles to adapt to the E field provide energy to the medium, increasing the phonons [
81].
In the mid-frequency case, a frequency-independent shielding is observed. This is due to the fact that the MWS effect no longer occurs at these frequencies, thus having frequency-invariant electromagnetic properties. For the high frequency range, there is a minimum in the shielding, where the drop and subsequent rise of the conductive shielding indicate that it is due to a passive disturbance of resonant nature.
In addition, in order to study the influence of the sonication time on the shielding value versus frequency, the type of GNP is kept constant, an average of the values in the different directions is calculated to eliminate the variability of the directionality, and the three frequency intervals (low, medium, and high) are analysed graphically.
Figure 17 shows the shielding values evaluated in the three frequency ranges evaluated for each type of GNP and sonication time. From the exposed low frequency graphs for the three types of GNPs (
Figure 17a,d,g) it can be concluded that the shielding behaviour according to the sonication time is the same that was found when the systems were evaluated for their DC electrical conductivities. This seems to indicate that the factors that determined the DC conductivity, such as lateral size, sonication time, amorphization, fragmentation, impurities, damage, and exfoliation, also determine the shielding of electrical signals for low frequencies. The above can be justified by stating that electrical shielding is related to AC electrical conductivity, since shielding is a measurement of the attenuation of the signal amplitude, with voltage amplitude being proportional to the resistance of the electrical signal flux through the material.
In the figures related to the medium frequency (
Figure 17b,e,h) the shielding value for all types of GNP seems to indicate that the differences, and therefore the dependence of the shielding on the sonication time, is negligible. Therefore, all the factors that were affected by the sonication time, which were those that determined the behaviour of the DC electrical conductivity and the shielding at low frequencies, are no longer influential. This is related to the justification of the relationship between shielding and AC conductivity, since a frequency increment and the electrical conductivities in AC and DC are differentiated, producing a different dependence or even independence of the AC conductivity of the parameters that governed the DC conductivity.
Finally, in the figures related to high frequencies (
Figure 17c,f,i), a recovery of the differences between the shielding values for the different sonication times is observed, but there are fluctuations that show that there is no stable behaviour for any sonication time. To conclude this section, the influence of the type of GNP used is studied (
Figure 18), for which the sonication times and the frequency range are kept constant, while the directionality effect is avoided through averaging.
Figure 18 shows that C300 generates the lowest attenuations and shielding regardless of the sonication time, while C750 appears to generate the highest attenuations for sonication times of 60 and 120 min. For short sonication times of 30 min, the C500 generates the highest shielding. In addition, for longer sonication times of 60 min, above the minimum at 10 MHz, there is a trade-off of influence between the GNPs generating higher and lower shielding. It can be concluded that the type of GNP is determinant in the low and high frequency range, but not in the mid-frequency range, where it is shown to be independent. This may be related to the fact that at low frequencies the dipoles generated within the matrix enclosed between the GNPs (forming the nano or microcondensors) are more numerous the larger the surface area of the GNP used [
82], so the attenuation is higher. This would indicate that the Maxwell–Wagner interfacial polarisation is influenced by the type of GNP used.
In the medium frequency range, the Maxwell–Wagner polarisation is no longer decisive, therefore the conductive shielding becomes independent of the GNP used. Finally, at high frequency it can be observed that a higher GNP surface area implies a higher shielding, probably because at these frequencies the Inductor–Capacitor (LC) behaviour of the system is determinant, as the lateral size and surface area of the GNP are influential in this behaviour [
83].