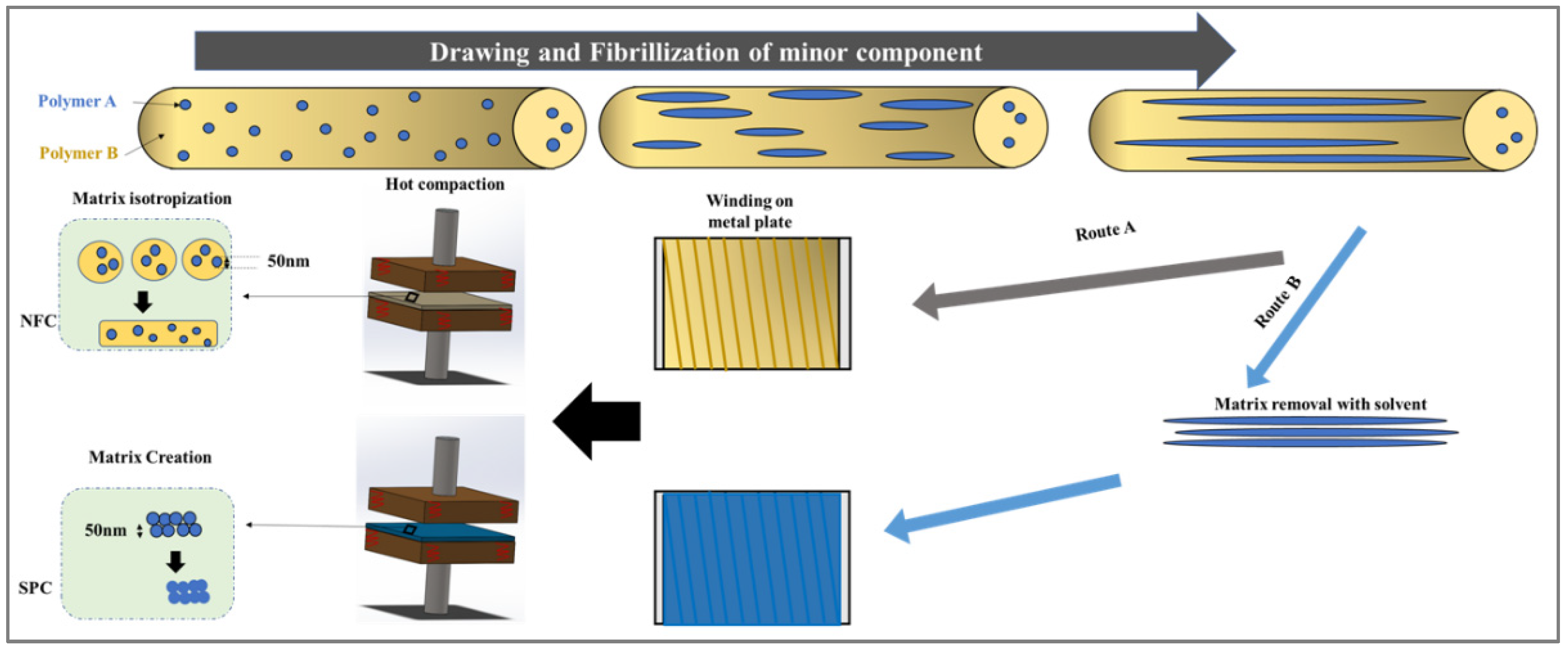
4.1. Newtonian Fluids in Well-Defined Flow Fields
When immiscible fluids are blended in industrial processes, the morphology that is created is the product of droplet deformation, breakup and coalescence. Research on the breakup of Newtonian droplets and threads has been performed for quite some time already and the breakup of a viscous thread in a medium having no or negligible viscosity was described by Rayleigh [
22] as early as in the 19th century. This theory was in the 1930s expanded by Tomotika [
23] to include the breakup of viscous threads in an elongational flow, while during the same decade Taylor [
24] explored how a liquid droplet deformed and broke up due to the motion of an immiscible, viscous suspending fluid. This lead to the publishing of a breakup criterion in both simple shear and hyperbolic flows. Thus, it seems that Taylor [
24] was the first to have systematically investigated aqueous colloids, or in other words, how Newtonian droplets deform and break up at room temperature.
Real mixing devices involve complicated flow fields, and since the deformation and break-up of droplets in such processes are more or less impossible to describe, most authors interpret the results they obtain, at least qualitatively, based on a description of particle break-up in simple flow fields. Taylor [
24] carried out two basic experiments: he started by investigating the deformation of single Newtonian particles suspended in a second immiscible Newtonian continuous matrix under simple shear flow by means of a Couette type-apparatus (
Figure 1) made up of two counter-rotating concentric cylinders. The speed of the two cylinders was adjusted to maintain a droplet in one position while being subjected to deformation at a shear rate of
.
He then built a four-roller mill apparatus to generate a hyperbolic flow so he could explore the mode of deformation and breakup under hyperbolic extensional flow (
Figure 2). The experimental description of all devices used by Taylor has been detailed by Grace [
25] and Levitt et al. [
26].
Taylor found that two dimensionless parameters governed the deformation and breakup of particles in these purely viscous Newtonian systems: the ratio of the viscosity of the dispersed phase to that of its continuous counterpart, denoted k (k = η
d/η
m), and the Weber (or capillary) number, Ca, which is a dimensionless parameter representing the ratio of the viscous stresses exerted on the droplet by the external flow field to the interfacial tension forces restoring the particle to a spherical shape. The capillary number is defined as
where η
m is the viscosity of the continuous phase,
is the shear rate, R is the radius of the droplet prior to deformation, and Γ is the interfacial tension between the particle phase and its continuous counterpart. At a specific shear rate (
), the viscous forces are greater than the interfacial one, causing the droplet to break up. The (Ca) corresponding to the critical shear rate (
) is called the critical capillary number and is denoted by Ca(crit) with
Thus, in the absence of coalescence, the minimum obtainable droplet diameter in Newtonian melt-mixed blend systems (Taylor limit) can be estimated from Ca(crit). Taylor demonstrated that for small deformations in a rotational shear field, an initial spherical particle with a radius ‘a’ becomes deformed to an ellipsoidal shape with length L and width B (
Figure 3). He defined the parameter Def, expressed as a function of the capillary number and the viscosity ratio k, according to:
Rumscheidt and Mason [
28] were able to experimentally demonstrate that the deformation at burst (Def
burst), under steady-state shearing flows (i.e., flows with a gradually increased shear rate and negligible inertia), is equal to the critical capillary number Ca(crit) within a range of viscosity ratios 0.1 ≤ k ≤ 1 with Def
burst ≈ Ca(crit) ≈ 0.5. It should be noted that the term f(k) ranged from 1 to 1.187 as k is increased from 0 to ∞, and that Def = 0 for a sphere. For Def ≥ 0.5 or L ≥ 3B, the droplet broke up [
16,
25].
According to Taylor, the critical shear rate
can be expressed as:
and the critical capillary number as:
Cox [
29] extended Taylor’s relation to time dependent flows (exclusively small deformations) as:
This corresponds to Taylor’s solution in the limits k→∞ or Ca→0 and led to a derivation of an expression for the orientation angle of the deformed droplet α according to:
All the analyses summarized here consider the behavior of isolated particles in a continuous medium. In usual blending operations, the dispersed phase fraction is important and the coalescence is not negligible. The influence of this parameter on the deformation of a droplet was taken into account by Choi and Schowalter [
30]. For moderately concentrated emulsions in simple shear flow, they obtained:
for Ca << 1 and 0 < k ≤ 1, where
is the dispersed phase fraction.
when Ca ≤ 1 and k >> 1.
By using a four roller apparatus to generate a stationary hyperbolic extensional strain rate (
), Taylor theoretically demonstrated that one can predict droplet deformation and break-up from the previously mentioned shear rate equations by replacing
by 2 ×
, thus indicating that an elongational strain rate of magnitude
has the same effect as a shear rate of magnitude 2 ×
. For small deformations (low capillary numbers), the droplet deformation is given by:
where
At high capillary numbers (the interfacial tension is neglected), the deformation of drop and matrix are affine and the deformation
This equation predicts that highly viscous drops (k > l) undergo less deformation than their surrounding medium. As expected, for an iso-viscous drop and matrix (k = 1), the deformation is identical in the two phases. When the viscosity ratio is very small (k << 1), the droplet deforms more than the matrix, thereby giving
The time evolution of the shape of a drop Def(t) that is suddenly subjected to a hyperbolic extensional flow rate
is given by Cox [
29]:
Many other authors have studied the deformation and burst conditions of particles in a shear-field [
31,
32]. Rumscheidt and Mason [
28], for instance, differentiated several categories of deformation and fracture of a drop in a simple shear flow according to the values of the viscosity ratio k and the capillary number Ca (
Figure 4). In the case where no shear is applied, the droplet has a spherical shape (cf. photographs of drop number 1 in
Figure 4), whereas under low deformation (Ca << Ca(crit)), deformation occurs and the particle takes the form of an ellipsoid (cf. photographs of drop number 2). However, for larger shear rates (Ca ≥ Ca(crit)), four types of drop fracture occur (cf. photographs of drops number 3, 4, 5…):
Class (a): for k < 0.2; the particle takes on a sigmoidal shape and tiny drops break away from its ends (tip streaming phenomenon).
Class (b): 0.2 ≤ k < 1; the particle’s central portion suddenly extends into a cylindrical shape, creating a neck in the middle (necking mechanism). The neck becomes progressively thinner until two identical daughter droplets and three satellite droplets are formed.
Class (c): 1 ≤ k < 4; the droplet extends into a long thread that gets progressively longer until breaking up into a large number of fine particles.
Class (d): k > 4; no burst occurs regardless of the applied shear rates. The particle deforms into an ellipsoid and orients along the flow without showing any signs of disintegration even at the upper limit of Ca of the apparatus ( up to 40 s−1). This is predicted by , yielding Def < 0.3 for k > 4, which can be regarded as insufficient for breakup.
At a fixed viscosity ratio k with (1 ≤ k < 4), several modes of deformation and breakup are possible in a shear flow and depend on the capillary number:
Ca < 0.1 Ca(crit): no droplet deformation occurs. The interfacial energy dominates.
Ca(crit) ≤ Ca < Ca(crit): there is slight droplet deformation without break-up, and a stable form is reached.
Ca(crit) ≤ Ca ≤ 2 Ca(crit): the interfacial stress is dominated by the viscous stress causing the droplet to become unstable and breakup to occur as a splitting of the particle into two equal parts before elongation into a filament can be achieved. The radius of the drops can be calculated as:
Ca(crit) < Ca ≤ 4 Ca(crit): particle deformation occurs leading to a long, unstable fiber, followed by fragmentation through ‘end-pinching’ or Rayleigh capillary instabilities giving rise to a large number of smaller drops [
22,
23,
33].
Ca > 4 Ca(crit): the shear stress is much stonger than the interfacial stress, causing the droplets to be deformed into long fibrils that do not break, but rather rotate in the flow field. In this case, the formation of a stable fibrillar structure can be obtained under specific conditions [
17,
34,
35,
36] (see later).
All the aforementioned studies on droplet deformation and breakup in Newtonian systems were realized under quasi-equilibrium conditions, without including the time. In general, the flow field in industrial mixers is not homogeneous. As a result, the flow rate experienced by a moving droplet is time-dependent (transient flow) and the burst does not occur unless there is enough time provided for the breakup. An example of the influence of time-dependent flow on droplet deformation is given in
Figure 5. The Figure displays two single drop experiments in an elongational flow generated by a device with opposed jets [
37]. A polybutadiene droplet (η
d = 12 Pa·s) was used in a polydimethylsiloxane matrix (η
m = 9 Pa·s), and the radius of the droplet was 0.48 mm, whereas the interfacial tension was equal to 4 mN/m. In both experiments, the capillary number was first increased from zero to 1.3 Ca(crit) and then decreased back to zero. In the first experiment, the maximum capillary number reached 42 s after the start of the experiment: the droplet deformed as the capillary number increased and then retracted back to a sphere again. In the second experiment, the capillary number was increased at a slower rate and the maximum capillary number was reached in 46 s. In this case, the drop did not retract back to a sphere but continued to elongate until breakup occurred. It should be noted that the viscosity ratio k = 1.3 with (Ca/Ca(crit) < 2) in both experiments.
For a particle undergoing high deformation in a medium (reduced capillary number Ca/Ca(crit) > 1), breakup is caused by the significant stresses during flow. However, it has been seen that surface instabilities can also give rise to rupture. In particular, when Ca/Ca(crit) > 2, a long thread with L/a > 6 (where L is the length after deformation and ‘a’ is the initial particle diameter (cf.
Figure 6), suspended in a different medium is in non-equilibrium, and its stability therefore depends on the flow conditions, the interfacial tension, its own rheological properties as well as those of the liquid it is suspended in [
38]. Stone et al., [
38] found that particle breakup follows one of two mechanisms depending on the elongation ratio (L/a). When (6 < L/a < 15), the particles broke up because of ‘end-pinching’ (i.e., the pinching off of the ends of a stretched drop from the central thread due to the particle relaxing after a sudden change in flow conditions). When (L/a > 15), capillary instabilities (known as Rayleigh disturbances) are the predominant breakup cause. One should keep in mind that the fact that polymers exhibit high viscosities and low surface tensions result in elevated relaxation times for the drop and consequently, breakup by end-pinching may not occur in such systems. This mechanism would, however, be the dominant mode of rupture for liquids with a low viscosity.
In 1879, Rayleigh was the first to treat capillary instabilities for a jet of a viscous fluid in air and his findings were later extended by Tomotika [
23] to the case of a single cylindrical viscous thread embedded in a quiescent Newtonian medium (after the flow had been stopped).
This theory assumes that under supercritical conditions Ca/Ca(crit) > 2, once the droplet has become highly extended (high L/B with B = 2 R
0, see
Figure 7), very small sinusoidal disturbances appear on the surface of the fibril see
Figure 8. Distortions with a wavelength, λ, larger than the original circumference of the fibril, 2πR
0, give rise to a reduction in interfacial surface area and as a result only these distortions are able to grow (see
Figure 7).
A dimensionless wave number of distortion, X, is given by
where X varies between zero and unity. The distortion amplitude, ε, is assumed to increase exponentially with time, according to
where ε
0 is the amplitude of the distortion at time t = 0. A lower limit of ε
0 can be obtained by thermal fluctuations and was estimated by [
40]:
where κ
B is the Boltzmann constant, T is the absolute temperature and Γ is the interfacial tension. According to [
40], ε
0 ≈ 10
−9 m for Γ = 10 mN.m
−1. Ref. [
41] gaves a higher estimate of 10
−8 to 10
−7 m.
The growth rate of the distortion (q) is expressed as
where η
m is the matrix viscosity, R
0 is the initial radius of the thread (R
0 = B/2; see
Figure 7) and Ω(k, X) is a complex function of the characteristic wave number, X, of the perturbation and the viscosity ratio, k, of the system in question. When the function Ω(k, X) is at its maximum, breakup of the thread occurs. Values of Ω(k, X) can be calculated from Tomotika’s original equation [
39] and
Figure 9 shows the function Ω(k, X) for two immiscible liquids with a viscosity ratio k = 0.91. Tomotika observed that the breakup of the fiber took place at an unique value for the dominant wave number, X
m = 0.568 with a value of Ω(k, X
m) = 0.074.
Figure 10 shows a plot of the values of the dominant growth rate Ω(k, X
m) and the dominant wave number X
m vs. the viscosity ratio k [
17,
20,
42]. For k→0, the dominant growth rate function Ω(k, X
m) approached unity and for k ≈ 100, Ω(k, X
m) it was equal to zero. For k ≈ 0.3, the dominant wavelength λ
m was at its minimum (the wave number X
m was maximal) indicating a maximum amount of capillary instabilities in this region of the viscosity ratio.
For 0.01 ≤ k ≤ 10, [
16] used the following equation to fit the function Ω(k,λ
m):
where b
0 = −2.588, b
1 = −1.154, b
2 = 0.03987, b
3 = 0.0889, and b
4 = 0.01154.
Tomotika estimated that the breakup occurs when the amplitude of the deformation ε reached a critical value corresponding to the average radius of the thread where
and
according to the condition of conservation of volume (see
Figure 6). The droplet size formed upon rupture of the fibril can be calculated from the following equation [
19]:
For example, if the viscosity ratio k = 0.91, Xm = 0.568, so that the diameter of the droplets formed after breakup of the fiber would be approximately twofold that of the original fiber.
The time required for the fiber to rupture can be used to evaluate the stability of a fibrillar structure.
For a thread suspended in a matrix, where both liquids are Newtonian, the breakup time in a shear field, t
b, can be determined using the equation
or in a dimensionless form (reduced breakup time) as
When deformation of the fiber takes place during flow, there occurs a superposition of a supplementary flow-induced deformation on the one due to Rayleigh instabilities, resulting in the breakup time given by Equation (22) no longer being valid [
43,
44]. The fiber extension due to the flow reduces the instability growth rate whereby the fiber stabilizes. This assumes that (i) the fiber deforms affinely with the matrix (Ca/Ca(crit) ≥ 2); (ii) the fiber is ruptured when the instability-induced local diameter reduction rate is greater than that caused by the fluid deformation; and (iii) that the instability wavelength is independent of fluid flow [
43].
The average diameter reduction rate due to the Rayleigh instability (dB/dt)
Rayleigh can be determined as the diameter of the fiber divided by the breakup time:
In a simple shear flow with an affine deformation, if the conservation of volume condition is employed, a spherical droplet with an initial diameter ‘a’ bedomes deformed into a fibril with the smallest dimension B (see
Figure 7b) according to [
17]:
where ‘t’ is time, and
is the shear rate. In the case where the fiber is highly elongated (near breakup),
and the diameter reduction rate is expressed as:
Since rupture is assumed when
is equal to
, breakup should take place when:
which yields the following equation for breakup time:
Consequently, during shear, the breakup time is also a function of the initial particle diameter and of the shear rate. Since there is no theoretical value for the term ln(ε/ε
0), it was evaluated by experimental results [
43,
44]. A value of 20 was found to give the best agreement between theory and experiment.
We can use the same analysis for extensional flow. In this case, the particle deformation is expressed as:
where
is the stretching rate [
19]. The breakup time in elongational flow then becomes:
The deformation of Newtonian droplets into fibers and the time corresponding to their complete breakup under a shear field, (t
b*), have been the subjects of several studies [
17,
38,
42]. Elemans et al. [
17] compared the results concerning the dimensionless time for breakup (t
b*) with data from similar research by Grace [
25] and
Figure 11 summarizes the findings. Grace demonstrated that (t
b*) became lower as Ca/Ca(crit) increased and the viscosity ratio k decreased. The following empirical formula was obtained from
Figure 11:
The importance of this equation is the fact that the time required for particle rupture can be approximated from Ca/Ca(crit) and the viscosity ratio k through comparison with the average residence time of the fiber in the die (this value should be lower than the time of fiber breakup in order to obtain a stable fibrillar morphology). However, according to
Figure 11, the time for breakup did not decrease as Ca/Ca(crit) increased in the case of Elemans experiments. He explained this difference with the fact that Grace possibly observed end-pinching, which indeed would give rise to much lower values for (t
b*).
Experimental data on how the critical capillary number relates to the viscosity ratio in the case of Newtonian blends under shear and elongational flow have been reported by several authors [
15,
32,
45,
46,
47,
48], but the work by Grace [
25] is probably the most cited.
Figure 12 depicts the Grace curve which is one of the most used graphs in the field of dispersive mixing. It relates the critical capillary number Ca(crit) to the viscosity ratio k (k varies between 10
−6 and 950) for the rupture of an initially spherical drop in a quasi-steady homogeneous flow. From Grace’s data one can conclude that the critical capillary number Ca(crit) depends both on the viscosity ratio k and on the flow type. Ca(crit) is, regardless of k, lower for a hyperbolic flow (2D elongation) than for a simple shear flow and it is thus easier to deform and rupture a particle under extensional flow than under shear flow.
Since a hyperbolic flow does not permit the rotation of drops, deformation occurs even for highly viscous droplets (k > 100). However, beyond k ≈ 4, the critical capillary number becomes very large, making it impossible to break a droplet even under simple shear due to the flow’s rotational character. Droplet breakup requires high shear rate values, which are above what can be practically attained. For a viscosity ratio in the range of 0.1 < k < 1, the droplets are readily broken up and in this range of viscosity ratio, Ca(crit) is minimal (~0.5 to 0.6) and independent of the flow type, with the lowest Ca(crit) value obtained around k = 1. As emphasized by Janssen et al. [
17,
18], this does not necessarily imply that the finest morphology is always obtained at k = 1. The reason for this is that a dispersion mechanism via stepwise equilibrium breakup (which is the typical experiment underlying
Figure 12) is improbable in a practical situation. For very low k values << 0.1, a log/log plot of Ca(crit) vs. (k) was fitted to a power function according to
which was in good agreement with the theoretically derived relation by Acrivos and Lo [
47] from a slender body analysis:
An explanation of the highest stability of low-viscosity droplets can be given in terms of Rayleigh waves. Droplet burst can be thought of as an amplification of Rayleigh waves of a dominant wavelength. Since the dominant wavelength increases when the viscosity ratio goes down, the low-viscosity droplets are expected to exhibit highly extended shapes upon burst. As these highly extended shapes undergo high Laplace pressures (i.e., significant pressure differences across the interface) as a result of sharp ends, it can be readily explained that high shear rates are to be applied to make the shear stress exceed the Laplace pressure and to induce breakup.
According to multiple studies, deformation (especially fibrillation) of the dispersed phase in an immiscible polymer blend is facilitated by an elongational flow field and a low viscosity ratio (k ≤ 1) [
36]. This explains why a majority of studies on MFCs and NFCs have been carried out using the slit-die hot stretching-quenching process.
De Bruijn [
48] used experimental data to demonstrate that Ca(crit) can be fitted with the following empirical equation (for k < 4):
Here, the C
i values for shear and extensional flows are given in
Table 1.
Common blending machines involve a mixture of elongational and shear flows and this is also true in most extruder geometries. Bentley et al. [
38,
49] have investigated particle deformation and breakup in various combinations of shear flow and elongational flow and experimentally demonstrated that intermediate flow types yield values of Ca(crit) in between Ca(crit)
elongation and Ca(crit)
shear. Experimental data have been confirmed by numerical studies on the critical capillary number under various types of flow [
46].
The above results were established for diluted Newtonian systems and the values of capillary numbers were obtained at quasi-equilibrium conditions. In commonly used blenders (extruders), the capillary number can be subdivided into different (local) capillary numbers, which vary depending on the position of the blend throughout the screws and the fluctuation of the shear rate according to the screw profile. Indeed, flow fields in an extruder are complex and there is coexistence of elongation and shear flow. Nevertheless, the flow field is much simpler in certain zones of the extruder. In the conveyor zone and the positive mixing zone, shear flow is predominant, whereas elongation flow is prevalent in the converging zone. It is also possible to generate an elongation flow by means of a stretching system at the die exit. Since the critical capillary number Ca(crit) depends on the flow type and Ca is dependent on the shear or elongational rate, the reduced capillary number Ca/Ca(crit) varies as a function of the screw profile and a variety of types of transient deformation can be found in the various extruder zones [
36].
During the blending process, the length scale of the dispersed phase becomes reduced. In the initial stage of mixing, the dispersed domains are in the millimeter size range, wherefore the capillary number is significant (Ca > 1000). Consequently, the drops are deformed affinely with the matrix so as to create long liquid threads. Due to the increasing surface between the phases, the interfacial stress becomes more considerable, the capillary number goes down and the threads break up into droplets.
Depending on their size, the drops that are created may once again go through breakup. Once more, the passage of the melt through a high shear zone (compression zone) induces the stretching of the newly formed droplets into thinner fibers, and so on. Finally, an equilibrium situation is reached in which the droplets are small enough to withstand the disruptive hydrodynamic forces. Coalescence can also take place which favors the formation of new large fibrils as schematized by Luciani et al., [
44]. The authors explained that the evolution of the content of fibrillar phase depends on the concentration (droplet fraction) in the medium. These results are in accordance with the findings of Favis and Chapleau [
12] who discovered that when the fraction of dispersed phase is higher, there is a more pronounced generation of fibrils.
For a low concentration of the dispersed phase, when the blend is well dispersed, the average diameter observed is a result of the dynamic equilibrium in the division and coalescence phenomena governing the size of the droplets in the blend. If the content of dispersed phase increases, so does the number of particles, which enhances the collision-coalescence phenomenon among them. As a consequence, the mean diameter of the droplets increases until the largest particles are no longer stable as drops in the stress field imposed in the blender, at which time they deform into fibers. Depending on the components’ properties (rheological behavior, interfacial tension, etc) and blending conditions, there exists a composition range where both nodular and fibrillar morphologies can be seen simultaneously. A larger amount of dispersed phase increases the fiber content and reduces the droplet concentration in the blend, until phase inversion takes place. The reduction in average diameter of the nodular part as the fiber fraction increases can be related to the deformation of the larger particles into fibers. These can then coalesce with others before breaking up due to the Rayleigh instability. Fiber stability seems to be the main parameter governing whether a blend’s morphology evolves towards a pure fibrillar one (with very stable fibers) or towards a droplet-type morphology (with a high fiber division rate). For these reasons, Luciani et al., [
44] introduced a novel parameter denoted q′ which depends only on the physical characteristics of the two materials involved in the blend. It is defined as:
where ‘B’ is the initial diameter of the thread. Luciani et al. found that the lower the q′, the greater the tendency to readily obtain a stable fibrillar morphology.
Recently, Deyrail et al. [
50,
51] studied the in situ fiber formation in immiscible polymer blends during the crystallization (or solidification) of the dispersed phase under shear flow. They investigated the impact of the crystallization time (or quenching time for an amorphous dispersed phase), the shear rate, and the breakup time on the final morphology. The authors defined a dimensionless parameter denoted λ
DC, which represents the ratio between the crystallization (or quenching) time and the breakup time of the filaments. If λ
DC >> 1, a nodular morphology is expected.
If λDC = 1, thin fibers form nodules and thick fibers adopt a more or less pronounced wavy shape.
If λDC << 1, a fibrillar morphology is expected.
4.2. Newtonian Polymer Blends: Effect of Elasticity
The above-mentioned results were obtained for Newtonian droplets and Newtonian matrix fluids. However, the non-Newtonian viscoelastic behavior of common high-molecular weight polymer blends is expected to influence the deformation and breakup of droplets in a flow field. Indeed, in the majority of immiscible polymer blends, both the drops and the surrounding medium exhibit a viscoelastic behavior so not only is the morphology of the dispersed phase determined by the viscosity force, but it is also influenced by the stress distribution around the droplets caused by elasticity force.
Several studies revealed that the viscoelasticity changes the drop deformation as well as the critical capillary number [
12,
20,
52,
53,
54]. The viscoelastic drop deformation has been shown to decrease as a result of normal stresses in the drop phase (elasticity of the droplet fluid inhibiting the droplet deformation and causing the particle to break at a higher capillary number). However, according to numeorus experiments, the general behavior was that a viscoelastic matrix steadies the droplets, facilitating the deformation of particles into microfibrils (i.e., breakup occurs at a lower capillary number). Gauthier, Goldsmith and Mason [
55], de Bruijn [
48], Varanasi, Ryan and Stroeve [
56], Ghodgaonkar and Sundararaj [
57] found that the critical capillary number Ca(crit) for viscoelastic drops in a Newtonian matrix were higher than that in the corresponding Newtonian mixtures as a result of the hindering effects of drop phase normal stresses.
It is quite a complicated task to determine a quantitative relationship between viscoelasticity and droplet deformation/breakup. This is due to viscoelasticity being manifested in various ways, including first and second normal stress differences for both matrix and droplet fluids, as well as shear thinning in viscous and elastic parts of both liquids. In an attempt to better manage the contributions of fluid viscoelasticity, certain investigations have selected only one viscoelastic liquid (i.e., either the matrix or the droplet), while the other was Newtonian.
The particle behavior in binary blends where only one of the two phases is viscoelastic has been explored. Elmendorp and Maalcke [
20] investigated the impact of elasticity on the breakup of isolated viscoelastic droplets in Newtonian matrices as well as of Newtonian particles in viscoelastic matrices while subjected to a simple shear flow. The authors discovered that the more elastic drops (as measured by the first normal stress difference N
1) remained the most resistant to breakup, while the more elastic matrices gave rise to increasingly unstable drops. Milliken and Leal [
21] conducted an experimental deformation/breakup study of isolated viscoelastic droplets made up of an aqueous polymer solution in a Newtonian fluid matrix subjected to a planar extensional flow generated by a four-roll-mill apparatus. They found that the viscoelastic particles deformed to a lesser extent than their Newtonian counterparts at a given capillary number and that the critical capillary number increased as compared with a Newtonian system at an equivalent viscosity ratio.
The Weissenberg number, Wi, can be used to quantiy elasticity in the droplet or matrix phase. According to Laun [
58], it is the ratio of elastic to viscous forces (defined as the first normal stress difference N
1 divided by the shear stress σ at a given deformation rate (
):
In the limit of low shear rate and frequency [
59]:
In similar fashion to the capillary number, the Weissenberg number increases with the shear rate, as a result of elastic forces generally growing more rapidly with the shear rate as opposed to their viscous counterparts. For a specific droplet size, there is a qualitative proportionality between Wi and Ca; however, since Ca is dependent on the particle diameter and Wi is not, these two dimensionless numbers can be independently varied by changing both the shear rate and the drop size for a specified pair of viscoelastic liquids. Since both phases can be elastic, there are two Weissenberg numbers: one for the droplet denoted Wid and one for the matrix denoted Wim. Since the elastic stresses in the drop are dependent on the strength of its internal flow, which in turn depends on the viscosity ratio (particles with higher viscosity have weaker internal flows), it is clear that there generally exists a connection between the viscosity ratio and the strength of the elastic forces in the particle.
It is possible to have further control if one selects a so-called ‘‘Boger’’ fluid, which is a weakly elastic dilute polymer solution (as the viscoelastic component) in a Newtonian matrix. Boger fluids have the advantage of presenting slight or no shear thinning in the shear viscosity and ideally also in the first normal stress coefficient.
Mighri et al. [
60,
61] investigated a blend of an elastic “Boger” fluid with constant viscosity as the droplet phase in a Newtonian matrix, and determined the impact of the elasticity ratio, as measured by the ratio, k’, of the Maxwell relaxation time (λe
d) of the particle phase (also known as the characteristic elastic time),
to that of matrix phase (λe
m) with
on the droplet deformation as well as on the critical capillary number, Ca(crit), for breakup. The authors found that there was a rise in the degree of droplet deformation and critical capillary number for breakup with an increasing elasticity ratio (Maxwell relaxation-time ratio) between the drops and the matrix, under either elongational or shear flow. This is in accordance with the aforementioned findings of Elmendorp and Berger. Under shear flow, they observed that for an elevated matrix elasticity (k’ < 0.37), the deformation of elastic droplets in an elastic matrix resembled than that of Newtonian particles in a Newtonian medium with the same viscosity ratio and interfacial tension (
Figure 13a). However, for high droplet elasticity (k’ > 0.37), the elastic drops deformed less than a Newtonian droplet in a Newtonian matrix.
Furthermore, they discovered that when the elasticity ratio was low or modest, k’ (= λ
d/λ
m) ≤ 4, the critical capillary number Ca(crit) for droplet breakup under conditions of steady shearing became greater with increasing k’, reaching a plateau of Ca(crit) ≈ 1.75 at the high elasticity ratio (k’ ≈ 4). This can be compared with Ca(crit) ≈ 0.5 for Newtonian drops (
Figure 13b). Thus, the particle’s resistance to deformation and breakup was greater with a higher elasticity ratio between the particle and the matrix phase.
Recently, single viscoelastic drops in Newtonian or viscoelastic media under simple shearing have been explored under a microscope. Lerdwijitjarud et al. [
62] investigated the deformation and breakup of isolated particles of a weakly elastic liquid (Wi
d ≤ 0.02) in a Newtonian medium, and established that the droplet elasticity gave rise to a small increase in Ca(crit) up to 20%. The droplet’s elasticity gave rise to a reduced degree of deformation at any given shear rate and a higher critical deformation at breakup, which resulted in an increased Ca(crit). However, when the Weissenberg number was at its highest (Wi
d ≥ 1), this effect appeared to saturate, leading to only a modest increase in Ca(crit).
In their studies on Boger fluids, Mighri et al. [
60] and Lerdwijitjarud et al. [
62], observed a modest effect of the viscoelasticity on the deformation and breakup of droplets, relative to what is seen in Newtonian fluids. As an example, there was a slight change (of a factor of approximately two) in Ca(crit), whereas for highly elastic melts, very large increases of more than a decade were seen in the capillary number required for droplet breakup, see next paragraph. These studies, and those described below, suggest that large increases in Ca(crit) resulted from a new mode of drop deformation and breakup for highly elastic droplets [
63].
Indeed, Vanoene [
64] considered the impact of elasticity from another viewpoint. In an attempt to describe the influence of normal stresses on particle breakup for a matrix with extensional flow, an expression for the interfacial tension in flow was developed by deriving a term proportional to the difference between a second normal stress function (according to the definition in his original paper) of the particle and the matrix phase,
where Γ
dynamic is the dynamic interfacial tension of a droplet of fluid d in a matrix m, Γ
steady is the interfacial tension of a quiescent polymer blend (in the absence of flow), D is the droplet diameter, N
2,d is the second normal stress difference of the dispersed phase, and N
2,m is the second normal stress difference of the matrix phase, which is dependent on the molecular weight, the molecular weight distribution and the shear stress. Vanoene’s results suggest that the interfacial tension under dynamic flow differed from what it would be under static flow. He demonstrated that, under dynamic flow conditions, the differences in elasticity between a blend’s components may cause the interfacial tension (known as the dynamic interfacial tension) to vary, and the obtained value could be quite different from its counterpart in the absence of flow.
Reignier et al. [
65] rewrote Vanoene’s equation by replacing the second normal stress difference by the first normal stress difference giving
which indicated that for a greater melt elasticity of the matrix as opposed to of the dispersed phase, Γdynamic should decrease as the shear increases, and inversely should become greater when the melt elasticity of the blend matrix is smaller than that of the dispersed phase.
Sundararaj et al. [
59] suggested two ways to incorporate the elastic contribution: (1) by considering only the first normal stress of the drop; and (2) by taking into account the normal stresses of both droplet and matrix. In the former case, the break-up occurs when shear forces ≥ interfacial forces + droplet elasticity. When the shear rates or frequency of rotation are low, the first normal stress difference can be approximated by 2G′, where G′ is the elastic modulus, implying that the break-up condition is:
At high shear rates (>10 s
−1), N
1 > 2G′, but the two quantities are proportional to each other. As a first approximation to obtain the qualitative behavior, the two sides of Equation (37) are equated to obtain an expression for the drop diameter,
In the first case, the normal stress of the matrix was not included. However, in a polymer–polymer blend, since the matrix is also a polymer, its normal stress will also attempt to deform the drop. As a result, in the force proportionality, there exists an additional force that causes the droplet to deform and according to Ghodgaonkar et al. [
57] the deformation and the final shape of the dispersed phase are the outcome of a dynamic equilibrium between the forces deforming the particle (i.e., shear stress and matrix elasticity) and forces resisting the deformation (i.e., droplet elasticity and interfacial tension). Consequently, the breakup takes place according to: shear forces + matrix elasticity ≥ interfacial forces + droplet elasticity or
Again, the first normal stress difference N
1 was approximated by 2G′, and by equating the two sides, the drop diameter equation becomes:
Elastic droplets would thus resist deformation more readily at higher shear rates due to the particle elasticity having a stabilizing effect during deformation, causing the minimum attainable drop diameter to be larger when the dispersed phase is elastic.
Based on the Ghodgaonkar equation, Seo and Kim [
63], introduced a new capillary number, Ca
E, (the elastic capillary number) that they expressed as:
Dispersed droplets become deformed for CaE > 1.
These authors also explored another parameter to elucidate the effect of elasticity: the widening of a drop during deformation in immiscible viscoelastic polymer blends. The effect of elasticity on the droplet widening was first investigated by Levitt and Macosko [
26] and later by Guido and Villone [
66] and, based on the results obtained from these experiments, the droplet widening is inversely proportional to the ratio of the drop-to-matrix elasticities, i.e., G
r = G
d/G
m, where G
d and G
m are respectively the elastic modulus of the drop and the matrix. Based on the simple assumptions that stretching in the hoop direction is greater than that in the thickness direction and that the second normal stress difference is proportional to the first normal stress difference, Levitt and Macosko were able to derive an approximate simplified equation for the drop thickness after deformation according to:
Here, Γ is the interfacial tension and Rnmax is half of the maximum thickness.
Recently, Abbassi-Sourki et al. [
67] discovered that the critical capillary number can be affected by adding a compatibilizer. They expressed a new capillary number, denoted Ca(crit)
compat, corresponding to the critical capillary number in the case where a droplet is modified by an interfacial active agent:
Here, Γ is the interfacial tension. The authors found that the presence of the compatibilizer causes the interfacial tension to decrease and the critical capillary number to increase.
Studies have also been performed on blend systems in which both the dispersed phase and the matrix phase are viscoelastic but without evaluating and taking into account the impact of the elasticity of the polymers in question. Wu [
68] found that the critical capillary number Ca(crit) has a higher importance in the case of viscoelastic blends as compared to Newtonian ones. He also confirmed that the steady-state particle size in blends of extruded viscoelastic polymers at a viscosity ratio of unity is approximately tenfold the corresponding value for Newtonian components at an equivalent viscosity and shear rate. This investigation involved a blend of PA6,6/ethylene-propylene over a wide range of polymer viscosities in which the suspended phase of rubber particles was low (<15%).
Wu [
68] discovered that the variation of Ca(crit) as a function of the viscosity ratio k presented as a V-shape as opposed to the U-shape previously obtained in the case of Newtonian melts (
Figure 14). He introduced an empirical equation as:
where the (+) sign in the exponent applies to viscosity ratio (k = η
d/η
m) values greater than unity and the (−) sign in the exponent applies to values of k below unity. He also put forward an empirical correlation relating the particle size of the suspended phase D
n to the viscosity ratio k for several extruded immiscible polymer blends.
Wu’s correlation is
where Γ is the interfacial tension between the two components, and
, is the shear rate. Here, the minimum of D
n corresponds to the minimum of Ca(crit) and thus to a viscosity ratio k = 1.
As Wu did not take particle coalescence into consideration, Serpe et al. [
69] further developed a modification to Wu’s equation by using the viscosity of the blend
rather than that of the matrix
and by taking into account a term of blend composition to estimate the average drop diameter according to the empirical equations below, in which (φ
d) and (φ
m) are the volume fraction of respectively the suspended phase and the matrix. Serpe was able to confirm Wu’s equation for PE/PA6 blends by using this modified viscosity ratio. He demonstrated that Ca(crit) increased along with the concentration of the suspended phase (
Figure 15).
with
Based on the existing findings when it comes to breakup and coalescence of droplets, Fortelny et al. [
70] investigated the dependence of the drop size of the minor component on its concentration in a polymer blend subjected to a simple steady shear flow. The equation they proposed takes into account both the breakup and coalescence of the particles to predict the droplet size of the minor phase. The equation is expressed
and here r
crit corresponds to the critical droplet radius as calculated from Ca(crit); α represents the probability of coalescence of the drops after collision; f
1 is the slope of a function describing the frequency of droplet breakup at Ca(crit); and Φ is the volume fraction of the suspended phase. This relationship still contains several parameters that are not readily quantifiable for the blending of viscoelastic polymers.
However, the elasticity of the blends was not included in the empirical correlations of Wu and Serpe, even though their results were obtained from viscoelastic materials for which reason Wu’s and Serpe’s equations are not applicable for polymer blends exhibiting elasticity ratios different from those used in the studies of Wu and Serpe.
There are no quantitative relationships linking the critical capillary number to the elasticity of the droplet or matrix phase. For this reason, Lerdwijitjarud et al. [
71] tried to develop quantitative dimensionless plots, similar to those that exist for Newtonian fluids, of Ca(crit) vs. other dimensionless quantities that characterize the melt viscoelasticity. However, when both the matrix and particle phases are viscoelastic, Ca(crit) is dependent on the viscosity ratio, as well as on the dimensionless elasticity of each phase. Since both viscosity and elasticity are shear-rate-dependent quantities, and since elasticity is a function of both the flow type and the flow history, both of which are complex functions of the flow in and around a particle, rigorous correlations are unlikely to be obtained.
Lerdwijitjarud et al. [
71] investigated the impact of elasticity contrast, as measured by the ratio of the first normal stress differences, N
1d/N
1m, between the suspended phase and the medium, on the critical capillary number in uncompatibilized immiscible polymer blends under a simple shear flow. The authors found that in 80/20
w/
w polyethylene/polystyrene blends sheared in a rheometer, the critical capillary numbers ranging from 2 to 30 depended on the relative magnitudes of the normal stress differences in the droplet and matrix phases as well as on the viscosity ratio (0.5, 1 and 2). These capillary numbers were between 4- and 80-fold their counterpart for breakup of a Newtonian drop in a Newtonian matrix. According to the authors, this large increase in critical capillary number (and hence droplet size) was assigned to the role of viscoelasticity. Breakup of viscoelastic particles in a viscoelastic medium is harder than for Newtonian drops in a Newtonian matrix. This is due to the contribution of both the particle elasticity and the shear-thinning of the polymer matrix. For all investigated blends, the critical capillary numbers were seen to increase with N
1d/N
1m, and were correlated by a power law in N
1d/N
1m (
Figure 16), with
Experimental measurements of the normal force N
1 are problematic and most investigations of fibrillar morphology have therefore focused on the effect of the viscosity ratio, neglecting the importance of the elasticity ratio between the dispersed and continuous phase. This has led to contradictions and ambiguity when it comes to drawing a clear parallel between the viscosity ratio and the optimal fiber formation conditions as well as temporal stability in the case of viscoelastic immiscible polymer blends. According to Min and White [
72], fibrils were obtained at a viscosity ratio 0.3 < k = η
d/η
m ≤ 1 for blends of undrawn melt-mixed polyethylene/polystyrene. However, Berger et al. [
52] studied poly (ethylene terephthalate)/polyamide blends and found that pure shearing did not create a droplet-fiber transition when the viscosity ratio k ≤ 1. The undrawn fibrous material appeared as a dispersion of spherical particles in the polymer matrix. Fibril-in-matrix structures were only for k = 3.7 in the undrawn fibrous material. The authors confirmed that it was therefore possible to create fibril-in-matrix structures by pure shearing only when the viscosity of the dispersed phase exceeded that of the continuous one. Drawing of the fibrous material always induces fibril-in-matrix structures.
Later on, Platé et al. [
73] explored different polymer pairs and indicated that good fibrillation could be achieved for a viscosity ratio in the range of 0.1 < k < 10, which was in total contradiction with Berger’s work [
52]. However, the generation of a fibrillar structure by hot stretching of the polymer melt out of the slit-die (elongational flow) was easier and it was generally agreed upon that a low viscosity ratio (k ≤ 1) and stretching flow favored the fibrillation of the dispersed phase in an immiscible polymer blend [
36,
74]. This would explain why most investigations on MFCs have been performed using a slit-die hot-stretching quenching process rather than melt extrusion and solid state cold drawing.
The work by Lerdwijitjarud et al. [
71] is of crucial importance and removes the ambiguity surrounding the conditions for obtaining a fibrillar structure in an immiscible polymer blend. The equations (48 to 50) demonstrate that in pure shear flow, in order to obtain a favorable stable fibrillar structure (low critical capillary number), the elasticity ratio should be less than unity (N
1d/N
1m < 1). At the same time, the viscosity ratio should be larger than two (k ≥ 2). These results are consistent with the experimental findings of Mighri et al. [
60] and Berger et al. [
52].
To summarize the conclusions of the present section we may state that the critical capillary numbers (Cacrit) where all droplets will break strongly depend on the viscoelastic nature of the dispersed phase and matrix which influence the mode of deformation of droplets under a flow field. Hence, a large amount of the present section was devoted to these issues. The theoretical, empirical models and experimental investigation reports describing the drop deformation in both shear and elongational flow were examined. It was highlighted that the extension transition from drops to nanofibrils also depended on the interfacial tension Γ. On the other hand, the stability of the morphology obtained was intimately linked to the relaxation behavior and break-up kinetics of the nanofibrils occurring during NFC preparation.