Evaluation on the Seal Performance of SMP-Based Packers in Oil Wells
Abstract
:1. Introduction
2. Experimental Characterization of the Polymer
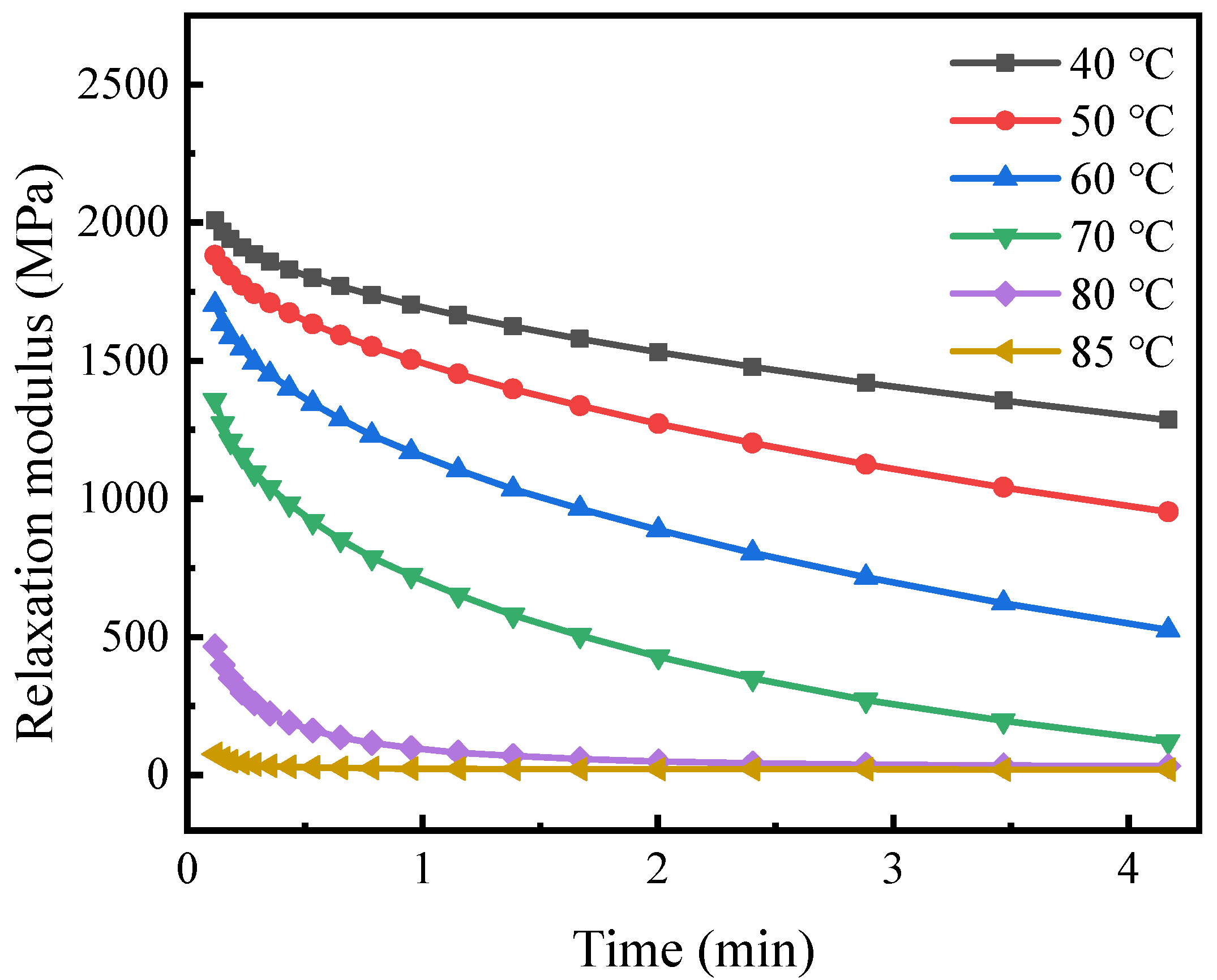
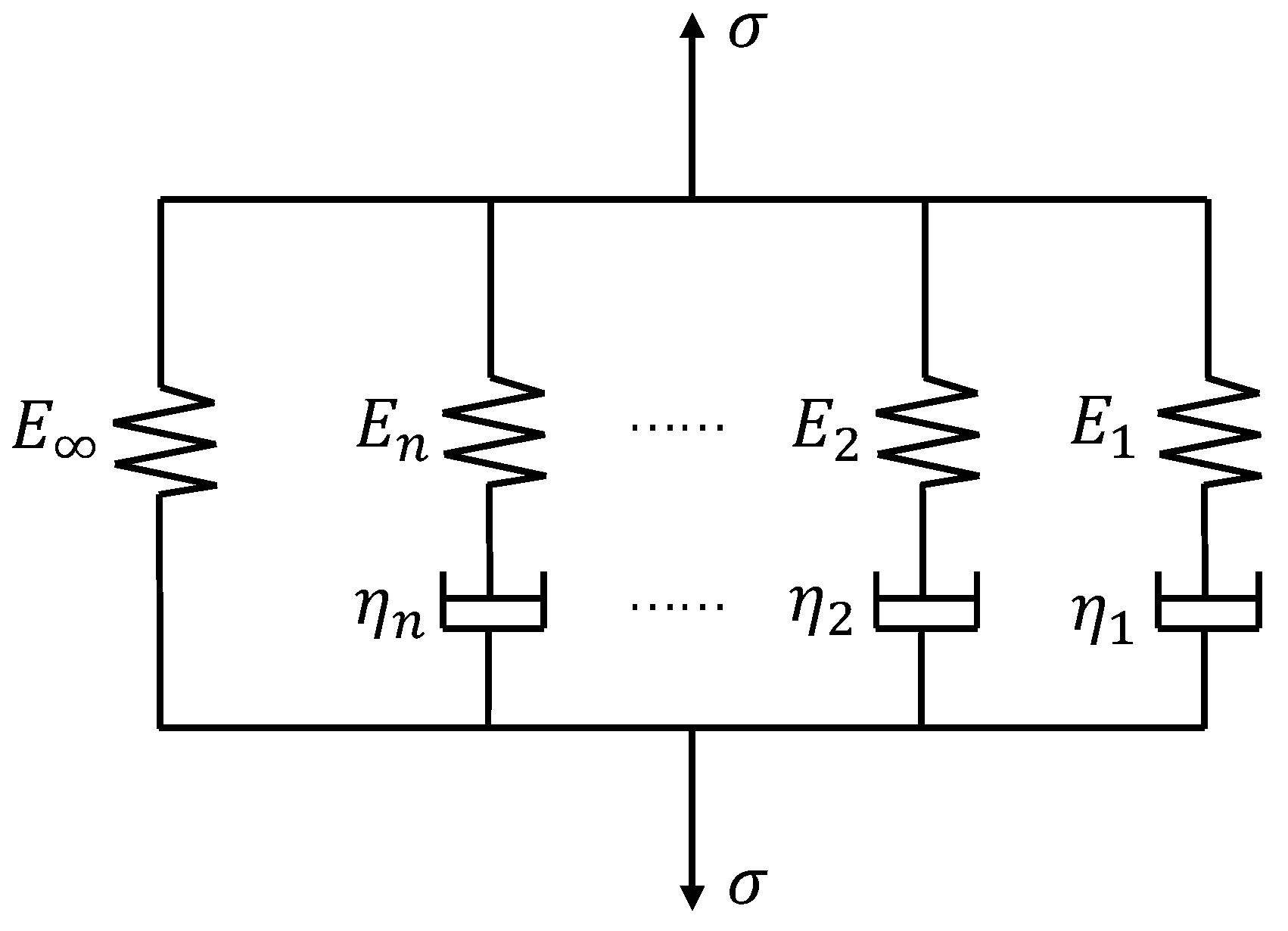
3. Viscoelastic Parameters for SMP
4. Simulation Modeling for the Setting Process
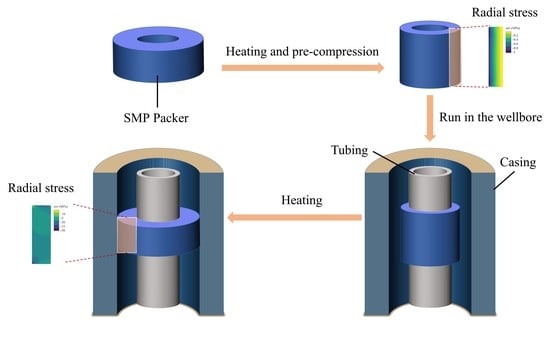
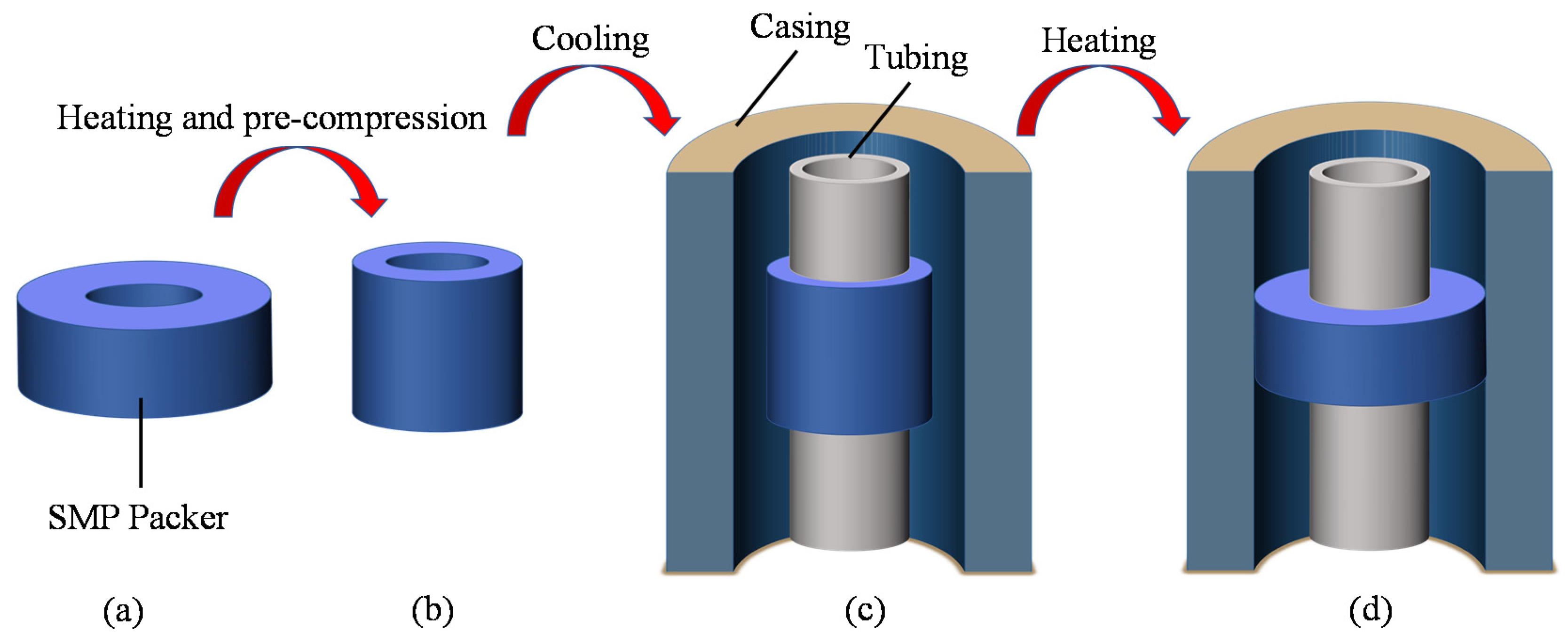
- Increasing the temperature above Tg significantly reduced the modulus of the SMP packer. Then, a radial compressive load is applied to the packer to extend its axial length and reduce its outer diameter. The compressed packer clings firmly to the mandril, making it easier to run in the wellbore.
- Keep the compressive load and decrease the temperature well below Tg.
- Slowly release the load, and the SMP packer store the compressed shape.
- The packer was lowered into the well. When it arrives at the setting position, it is heated again above Tg using a downhole heater. Finally, the packer gradually returns to its original shape. Then, it expands to contact the inner wall of the casing or the formation, which produces contact stress to achieve sealing.
4.1. Material Parameters in FEA
4.2. The Geometry of the Model
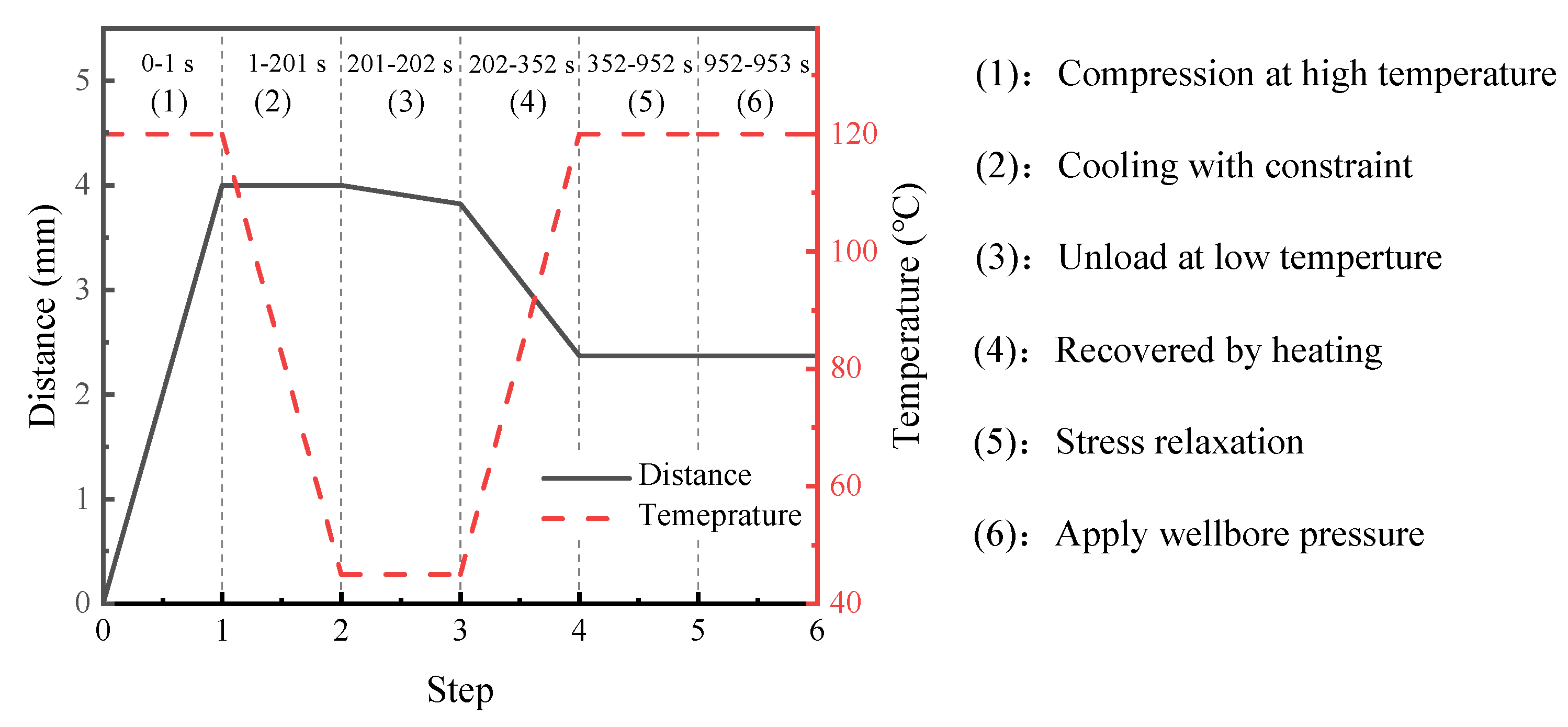
4.3. Simulation Steps and Boundary Conditions
- The initial step defines the temperature field as 120 °C (>Tg) and constrains the radial displacement on the left side of the packer.
- The first viscoelastic step applies a radial displacement (−10 mm in the radial direction) on the right side to compress the packer under the constant temperature defined in the initial step.
- In the second viscoelastic step, the field temperature decreased to 50 °C with compression.
- During the third viscoelastic step, the model keeps the low temperature unchanged and releases the displacement load. Meanwhile, the rigid casing moves radially to form an annular gap between the tubing and casing.
- The fourth viscoelastic step increases the temperature again above Tg to activate shape recovery.
- Considering the stress relaxation of polymers, the fifth step holds the temperature for a while until the contact stress between the packer and the casing reaches a steady value.
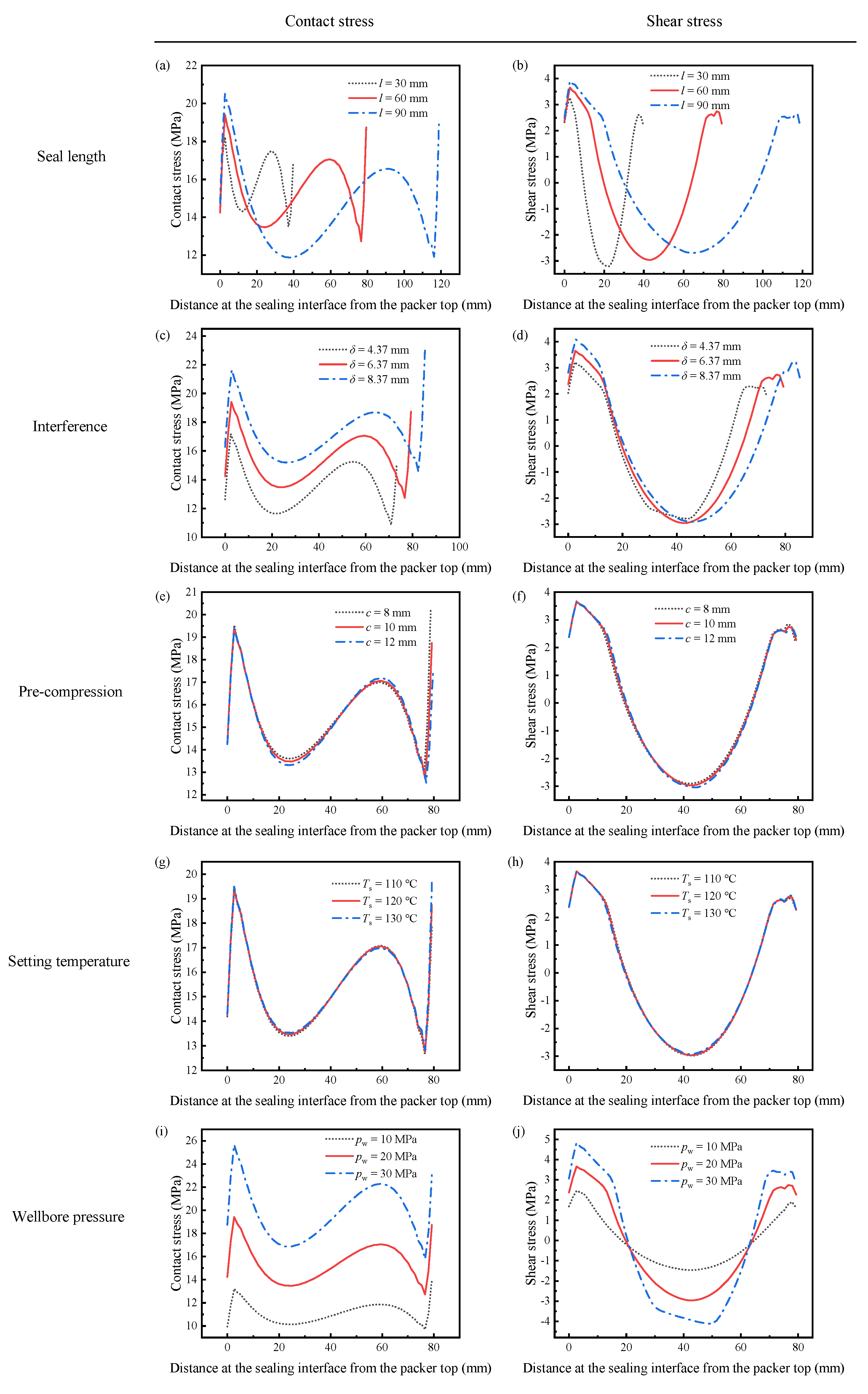
- Finally, the extra general step applies pressure (20 MPa) to the top edge of the packer. Under subsurface conditions, the packer must withstand the pressure difference between the annular and pore fluids. In an extreme case, only one end of the packer is subjected to wellbore pressure.
5. Results and Discussions
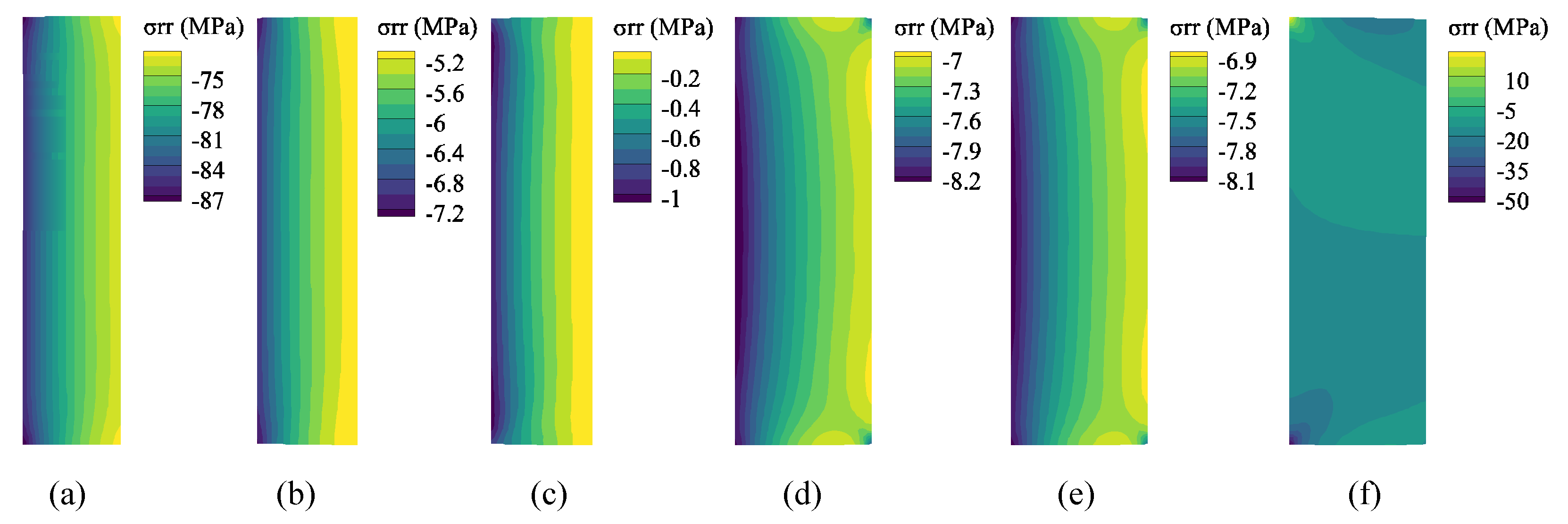
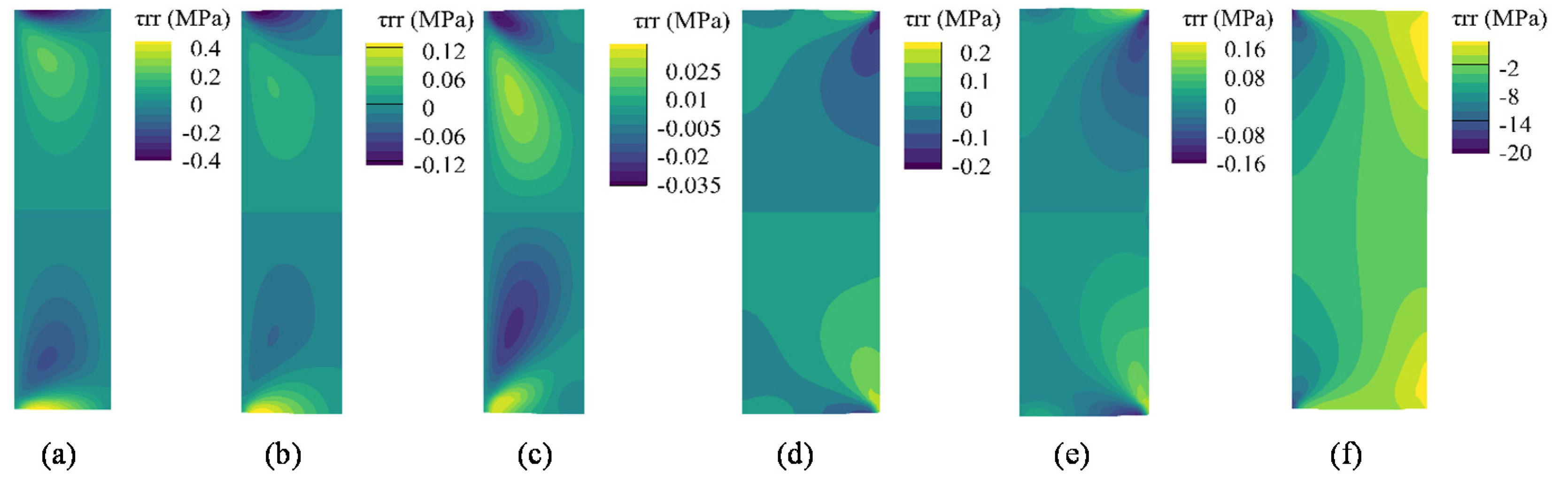
5.1. Base Case Simulation for the Seal Performance
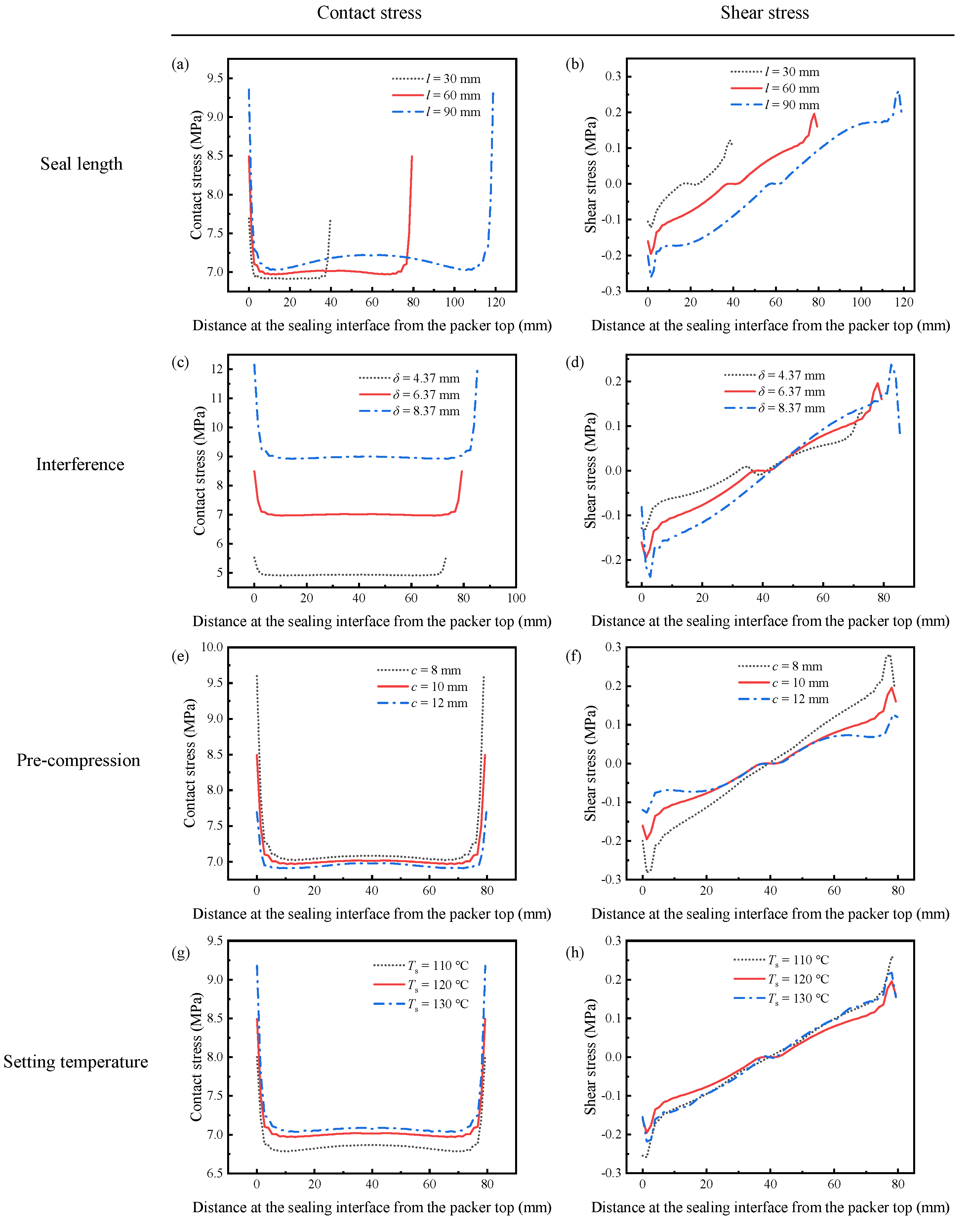
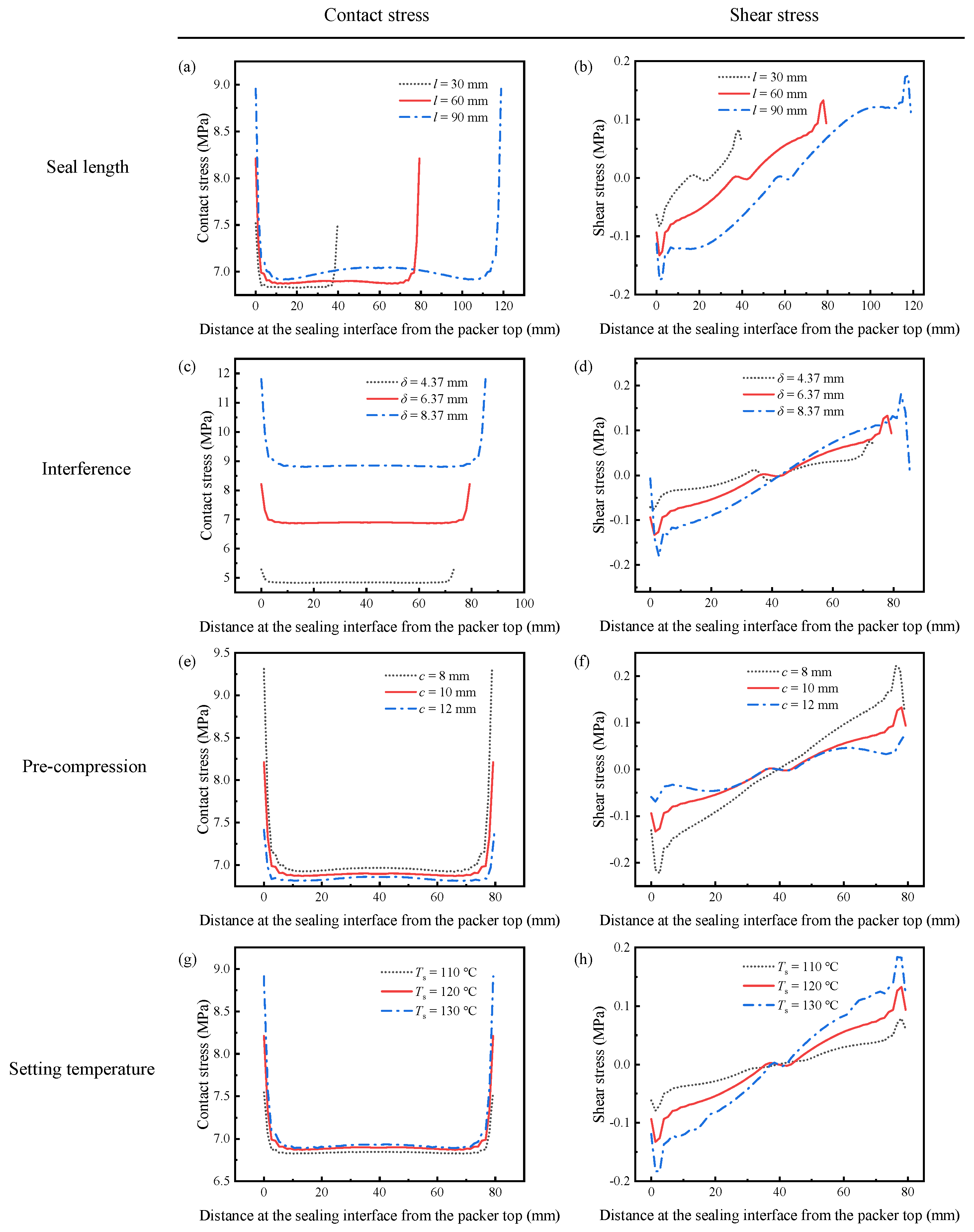
5.2. Effects of Seal Length, Interference, Pre-Compression, Temperature, and Wellbore Pressure
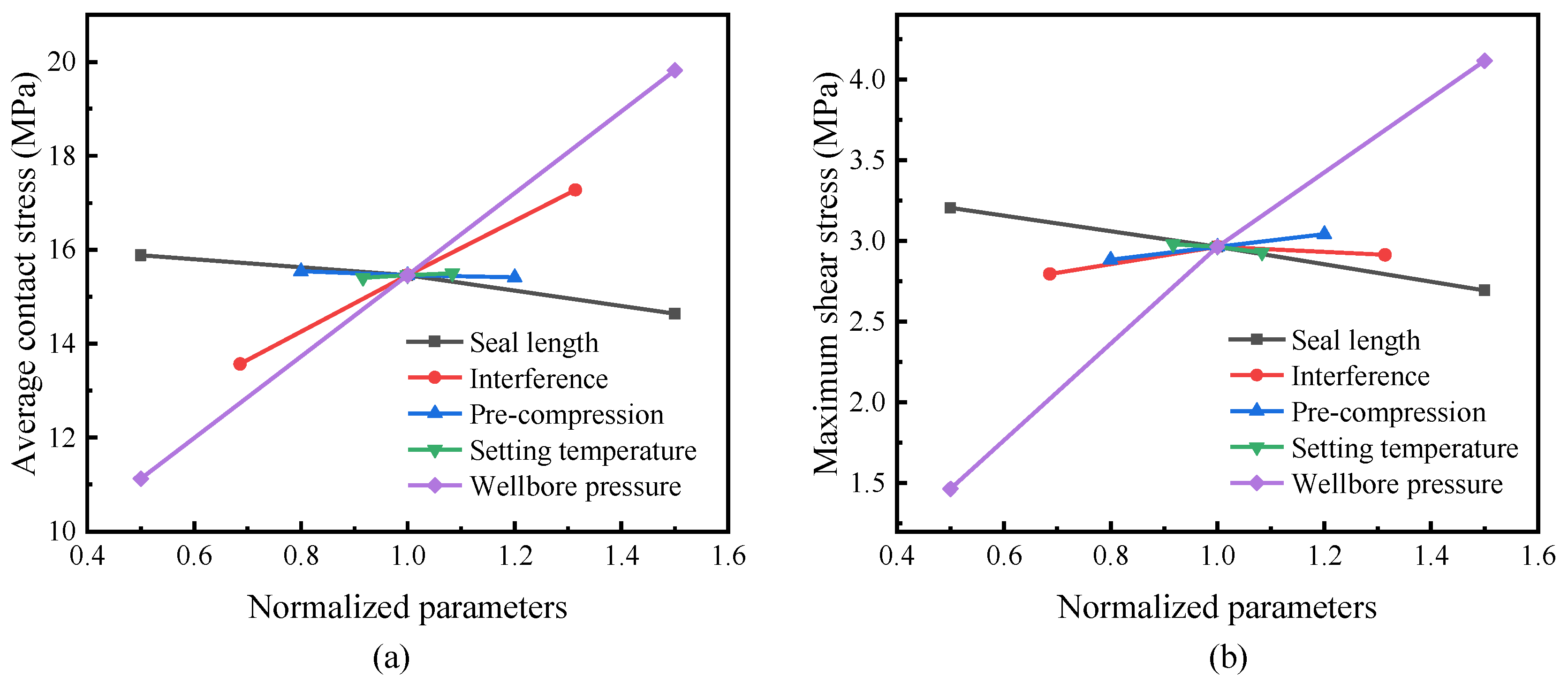
5.3. Sensitivity Analysis
6. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Meng, H.; Li, G. A review of stimuli-responsive shape memory polymer composites. Polymer 2013, 54, 2199–2221. [Google Scholar] [CrossRef] [Green Version]
- Wang, W.; Liu, Y.; Leng, J. Recent developments in shape memory polymer nanocomposites: Actuation methods and mechanisms. Coordin. Chem. Rev. 2016, 320, 38–52. [Google Scholar] [CrossRef]
- Al Azzawi, W.; Herath, M.; Epaarachchi, J. Modeling, analysis, and testing of viscoelastic properties of shape memory polymer composites and a brief review of their space engineering applications. In Creep and Fatigue in Polymer Matrix Composites, 2nd ed.; Guedes, R.M., Ed.; Woodhead Publishing: Sawston, UK, 2019. [Google Scholar]
- Quadrini, F.; Bellisario, D.; Iorio, L.; Santo, L.; Pappas, P.; Koutroumanis, N.; Anagnostopoulos, G.; Galiotis, C. Shape memory composite sandwich structures with self-healing properties. Polymers 2021, 13, 3056. [Google Scholar] [CrossRef] [PubMed]
- Liu, T.; Liu, L.; Yu, M.; Li, Q.; Zeng, C.; Lan, X.; Liu, Y.; Leng, J. Integrative hinge based on shape memory polymer composites: Material, design, properties and application. Compos. Struct. 2018, 206, 164–176. [Google Scholar] [CrossRef]
- Delaey, J.; Dubruel, P.; Van Vlierberghe, S. Shape-memory polymers for biomedical applications. Adv. Funct. Mater. 2020, 30, 1909047. [Google Scholar] [CrossRef]
- Vakil, A.U.; Petryk, N.M.; Shepherd, E.; Monroe, M.B.B. Biostable shape memory polymer foams for smart biomaterial applications. Polymers 2021, 13, 4084. [Google Scholar] [CrossRef]
- Mahdi Rafiee, M.; Baniassadi, M.; Wang, K.; Baniasadi, M.; Baghani, M. Mechanical properties improvement of shape memory polymers by designing the microstructure of multi-phase heterogeneous materials. Comp. Mater. Sci. 2021, 196, 110523. [Google Scholar] [CrossRef]
- Mansour, A.; Dahi Taleghani, A.; Salehi, S.; Li, G.; Ezeakacha, C. Smart lost circulation materials for productive zones. J. Pet. Explor. Prod. Technol. 2019, 9, 281–296. [Google Scholar] [CrossRef] [Green Version]
- Shen, D.; Shi, S.; Xu, T.; Huang, X.; Liao, G.; Chen, J. Development of shape memory polyurethane based sealant for concrete pavement. Constr. Build. Mater. 2018, 174, 474–483. [Google Scholar] [CrossRef]
- Fan, J.; Santos, L.Y.; Dahi Taleghani, A.; Li, G. Stimuli-responsive petroleum cement composite with giant expansion and enhanced mechanical properties. Constr. Build. Mater. 2020, 259, 119783. [Google Scholar] [CrossRef]
- Yuan, Y.; Goodson, J.; Johnson, M.; Gerrard, D. In-situ mechanical- and functional-behavior characterization of a shape-memory polymer for sand-control applications. SPE Drill. Complet. 2012, 27, 253–263. [Google Scholar]
- Wang, X.; Osunjaye, G. Advancement in openhole sand control applications using shape memory polymer. In Proceedings of the SPE Annual Technical Conference and Exhibition, Dubai, United Arab Emirates, 26–28 September 2016. [Google Scholar]
- Ismail, M.S.; Yahia, Z.; Rozlan, M.R.; Bakar, M.F.; Amsidom, A.A.; Chaemchaeng, P.; Mohammad, R.M.Z. Paradigm shift in downhole sand control; The first installation of shape memory polymer as an alternative to gravel packing at bs field, offshore Malaysia. In Proceedings of the Offshore Technology Conference, Houston, TX, USA, 4–7 May 2020. [Google Scholar]
- O'malley, E.J.; Richard, B.M. Packer sealing element with shape memory material. U.S. Patent 7743825, 2010. [Google Scholar]
- Patil, D.; Song, G. A review of shape memory material’s applications in the offshore oil and gas industry. Smart Mater. Struct. 2017, 26, 093002. [Google Scholar] [CrossRef]
- Guo, W.; Wang, Z.; Sun, Y.; Wang, Y.; Deng, S.; Li, Q.; Sciubba, E. Effects of packer locations on downhole electric heater performance: Experimental test and economic analysis. Energies 2020, 13, 377. [Google Scholar] [CrossRef] [Green Version]
- Guizada, P.; Nugraha, I.; Alrashed, A.; Soriano, E.; Robles, F.; Vega, R. Stimulating low permeability carbonate reservoirs by applying acid fracturing with open-hole multi-stage completions combining sliding sleeves and swellable packers for effective fracture placement. In Proceedings of the SPE Asia Pacific Hydraulic Fracturing Conference, Beijing, China, 24–26 August 2016. [Google Scholar]
- Yu, C.; Xu, Z.; Shyu, G.-D.; Prieto, C. Development of advanced seal material and downhole packer for high temperature applications. In Proceedings of the Offshore Technology Conference, Houston, TX, USA, 1–4 May 2017. [Google Scholar]
- Tong, Z.; Pei, X.; Shen, Z.; Wei, S.; Gao, Y.; Huang, P.; Shi, B.; Sun, F.; Fu, T. An enhanced thermo-actuated shape memory polymer composite coupled with elastomer. Petrol. Explor. Dev. 2016, 43, 1097–1106. [Google Scholar] [CrossRef]
- Snyder, D.J.; Seale, R. Optimization of completions in unconventional reservoirs for ultimate recovery—Case studies. In Proceedings of the SPE EUROPEC/EAGE Annual Conference and Exhibition, Vienna, Austria, 23–26 May 2011. [Google Scholar]
- Koløy, T.R.; Andersen, K.; Nijhof, J. Valhall—Pushing the limits for openhole zonal isolation—Qualification and field trial of 10,000-psi oil-swelling packers. In Proceedings of the SPE/DGS Saudi Arabia Section Technical Symposium and Exhibition, Barcelona, Spain, 15–18 May 2011. [Google Scholar]
- Druecke, B.; Dussan, V.E.B.; Wicks, N.; Hosoi, A.E. Large elastic deformation as a mechanism for soft seal leakage. J. Appl. Phys. 2015, 117, 104511. [Google Scholar] [CrossRef]
- Chen, X.; Bartos, J.; Salem, H.; Zonoz, R. Elastomers for high pressure low temperature HPLT sealing. In Proceedings of the Offshore Technology Conference, Houston, TX, USA, 2–5 May 2016. [Google Scholar]
- Lehr, D.; Furlan, W. Seal life prediction and design reliability in downhole tools. In Proceedings of the SPE Annual Technical Conference and Exhibition, San Antonio, TX, USA, 9–11 October 2017. [Google Scholar]
- Hu, G.; Zhang, P.; Wang, G.; Zhang, M.; Li, M. The influence of rubber material on sealing performance of packing element in compression packer. J. Nat. Gas. Sci. Eng. 2017, 38, 120–138. [Google Scholar] [CrossRef]
- Salehi, S.; Ezeakacha, C.P.; Kwatia, G.; Ahmed, R.; Teodoriu, C. Performance verification of elastomer materials in corrosive gas and liquid conditions. Polym. Test. 2019, 75, 48–63. [Google Scholar] [CrossRef]
- Zhang, Y.; Wang, H.; Che, J.; Du, M.; Dou, M.; Liang, G. Theoretical analysis of pressure-bearing performance on compression packer in low permeability reservoirs. Int. J. Pres. Ves. Pip. 2021, 191, 104325. [Google Scholar] [CrossRef]
- Tu, B.; Cheng, H.L. Alternative methodology for elastomeric seal RGD and aging testing validates long-term subsea seal performance and integrity. In Proceedings of the Offshore Technology Conference, Houston, TX, USA, 2–5 May 2016. [Google Scholar]
- Dong, L.; Li, K.; Zhu, X.; Li, Z.; Zhang, D.; Pan, Y.; Chen, X. Study on high temperature sealing behavior of packer rubber tube based on thermal aging experiments. Eng. Fail. Anal. 2020, 108, 104321. [Google Scholar] [CrossRef]
- Zheng, C.; Zheng, X.; Qin, J.; Liu, P.; Aibaibu, A.; Liu, Y. Nonlinear finite element analysis on the sealing performance of rubber packer for hydraulic fracturing. J. Nat. Gas. Sci. Eng. 2021, 85, 103711. [Google Scholar] [CrossRef]
- Zhong, A.; Johnson, M.; Kohn, G.; Koons, B.; Saleh, M. Performance evaluation of a large bore expandable liner hanger for field operations in the Gulf of Mexico. In Proceedings of the Offshore Technology Conference, Houston, TX, USA, 4–7 May 2015. [Google Scholar]
- Patel, H.; Salehi, S. Investigation of elastomer seal energization: Implications for conventional and expandable hanger assembly. Energies 2019, 12, 763. [Google Scholar] [CrossRef] [Green Version]
- Al-Hiddabi, S.A.; Pervez, T.; Qamar, S.Z.; Al-Jahwari, F.K.; Marketz, F.; Al-Houqani, S.; van de Velden, M. Analytical model of elastomer seal performance in oil wells. Appl. Math. Model. 2015, 39, 2836–2848. [Google Scholar] [CrossRef]
- Patel, H.; Salehi, S.; Teodoriu, C.; Ahmed, R. Performance evaluation and parametric study of elastomer seal in conventional hanger assembly. J. Pet. Sci. Eng. 2019, 175, 246–254. [Google Scholar] [CrossRef]
- Duan, P.; Rosenblatt, S. Shape Memory Material Packer for Subterranean Use. U.S. Patent 8739408, 2014. [Google Scholar]
- Fuxa, J.; Di Giampaolo, P.; Ferrara, G.; Di Pietro, M.; Sportelli, M.; Ripa, G.; Di Campli, A. Shaped memory polymer: An innovative approach to sand control open hole completion in thin, multilayered, depleted low permeability gas reservoirs. In Proceedings of the International Petroleum Technology Conference, Beijing, China, 26–28 March 2019. [Google Scholar]
- Williams, M.L.; Landel, R.F.; Ferry, J.D. The temperature dependence of relaxation mechanisms in amorphous polymers and other glass-forming Liquids. J. Am. Chem. Soc. 1955, 77, 3701–3707. [Google Scholar] [CrossRef]
- Azzawi, W.A.; Epaarachchi, J.A.; Islam, M.; Leng, J. Implementation of a finite element analysis procedure for structural analysis of shape memory behaviour of fibre reinforced shape memory polymer composites. Smart Mater. Struct. 2017, 26, 125002. [Google Scholar] [CrossRef]
- Ardebili, H.; Pecht, M. Materials and Processes for Electronic Applications, 1st ed.; Elsevier: Amsterdam, The Netherlands, 2009. [Google Scholar]
- Shardakov, I.N.; Trufanov, A.N. Identification of the temperature dependence of the thermal expansion coefficient of polymers. Polymers 2021, 13, 3035. [Google Scholar] [CrossRef]
- Sadd, M.H. Elasticity: Theory, Applications, and Numerics, 3rd ed.; Academic Press: Cambridge, MA, USA, 2014. [Google Scholar]
- Gutierrez-Lemini, D. Engineering Viscoelasticity; Springer: New York, NY, USA, 2014. [Google Scholar]
- Liu, R.; Xu, S.; Luo, X.; Liu, Z. Theoretical and numerical analysis of mechanical behaviors of a metamaterial-based shape memory polymer stent. Polymers 2020, 12, 1784. [Google Scholar] [CrossRef]
- Chae, S.-H.; Zhao, J.-H.; Edwards, D.R.; Ho, P.S. Characterization of the viscoelasticity of molding compounds in the time domain. J. Electron. Mater. 2010, 39, 419–425. [Google Scholar] [CrossRef]
- Gergesova, M.; Zupančič, B.; Emri, I. A Note on automated time-temperature and time-pressure shifting. In Time Dependent Constitutive Behavior and Fracture/Failure Processes; Proulx, T., Ed.; Springer: New York, NY, USA, 2011; Volume 3, pp. 199–211. [Google Scholar]
- ABAQUS. Analysis User’s Manual; Dassault Systèmes: Vélizy-Villacoublay, France, 2014. [Google Scholar]



| i | τi (s) | Ei (MPa) |
|---|---|---|
| 1 | 0.01 | 490.60 |
| 2 | 0.39 | 769.50 |
| 3 | 3.27 | 313.27 |
| 4 | 26.67 | 323.98 |
| 5 | 247.24 | 2.18 |
| 6 | 6.65 × 105 | 35.31 |
| Parameters | Parameter Value (Normalized Value) | ||
|---|---|---|---|
| Seal length (mm) | 30 (0.5) | 60 (1.0) | 90 (1.5) |
| Interference (mm) | 4.37 (0.69) | 6.37 (1.0) | 8.37 (1.31) |
| Pre-compression (mm) | 8 (0.8) | 10 (1.0) | 12 (1.2) |
| Setting temperature (°C) | 110 (0.92) | 120 (1.0) | 130 (1.08) |
| Wellbore pressure (MPa) | 10 (0.5) | 20 (1.0) | 30 (1.5) |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, N.; Dong, X.; Ma, Y. Evaluation on the Seal Performance of SMP-Based Packers in Oil Wells. Polymers 2022, 14, 836. https://doi.org/10.3390/polym14040836
Chen N, Dong X, Ma Y. Evaluation on the Seal Performance of SMP-Based Packers in Oil Wells. Polymers. 2022; 14(4):836. https://doi.org/10.3390/polym14040836
Chicago/Turabian StyleChen, Naihan, Xuelin Dong, and Yinji Ma. 2022. "Evaluation on the Seal Performance of SMP-Based Packers in Oil Wells" Polymers 14, no. 4: 836. https://doi.org/10.3390/polym14040836
APA StyleChen, N., Dong, X., & Ma, Y. (2022). Evaluation on the Seal Performance of SMP-Based Packers in Oil Wells. Polymers, 14(4), 836. https://doi.org/10.3390/polym14040836