Synchronized 3D Printing and Corona Charging for One-Step Prototyping of Polarized Polylactic Acid Electrets
Abstract
:1. Introduction
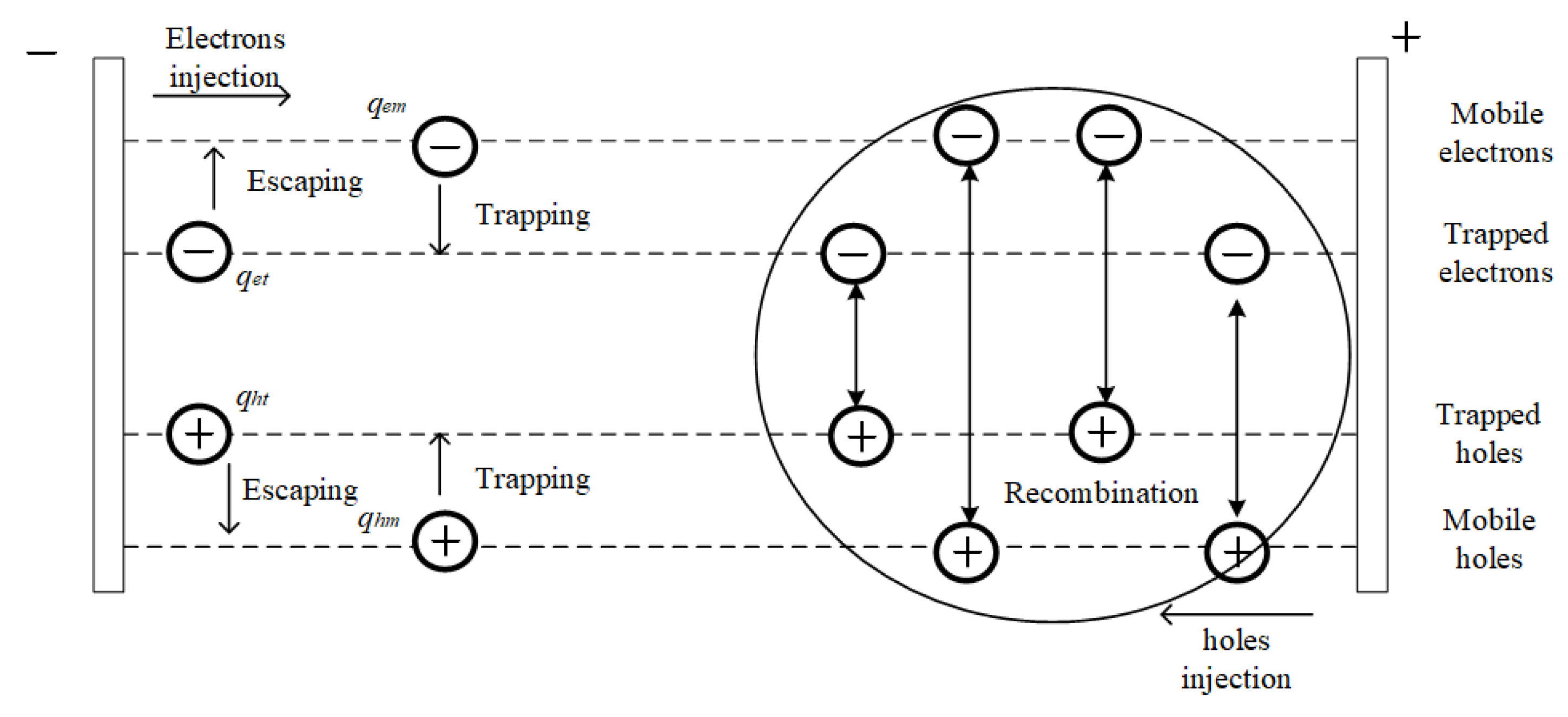
2. Model
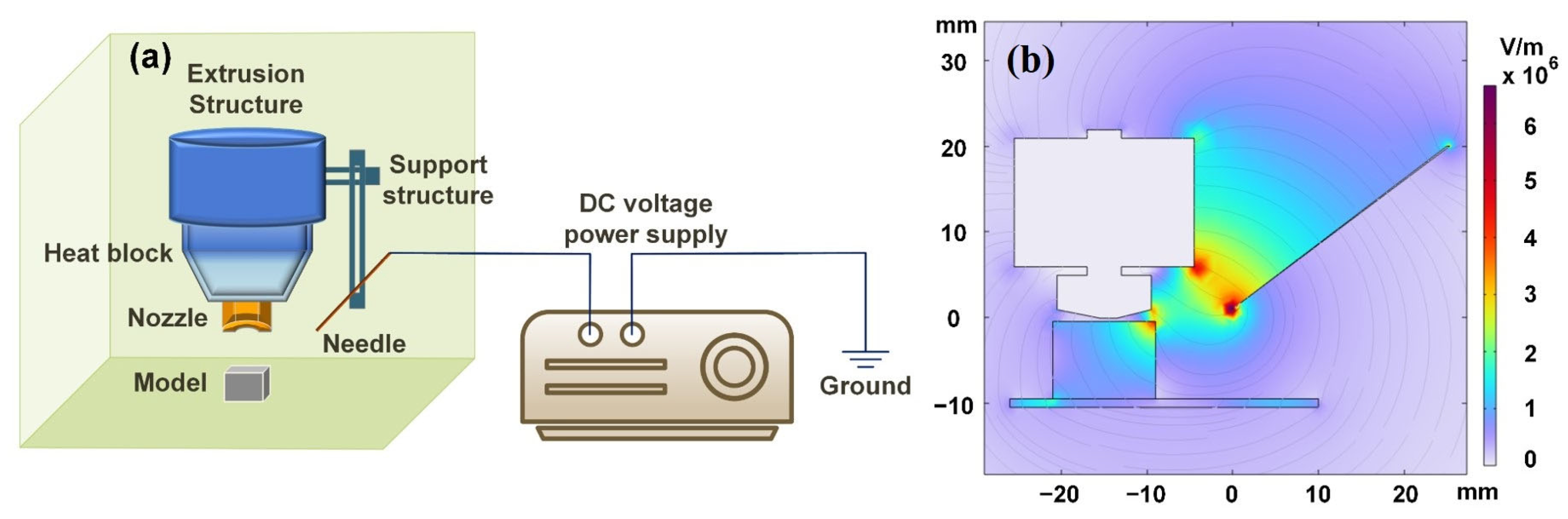
3. Methods
4. Results and Discussion
4.1. Printer Nozzle Refitting
4.2. SEM Photos of Printed Samples
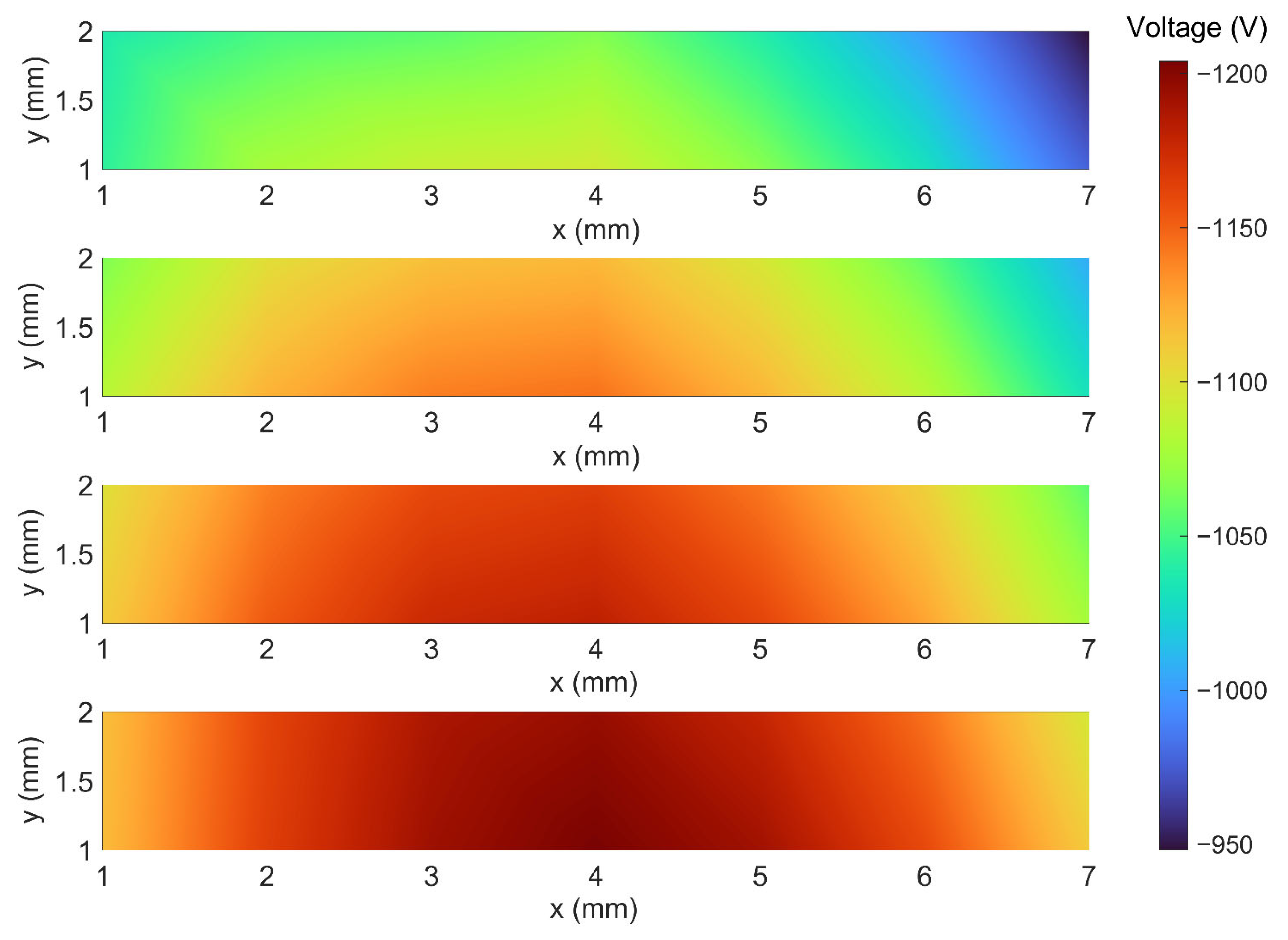
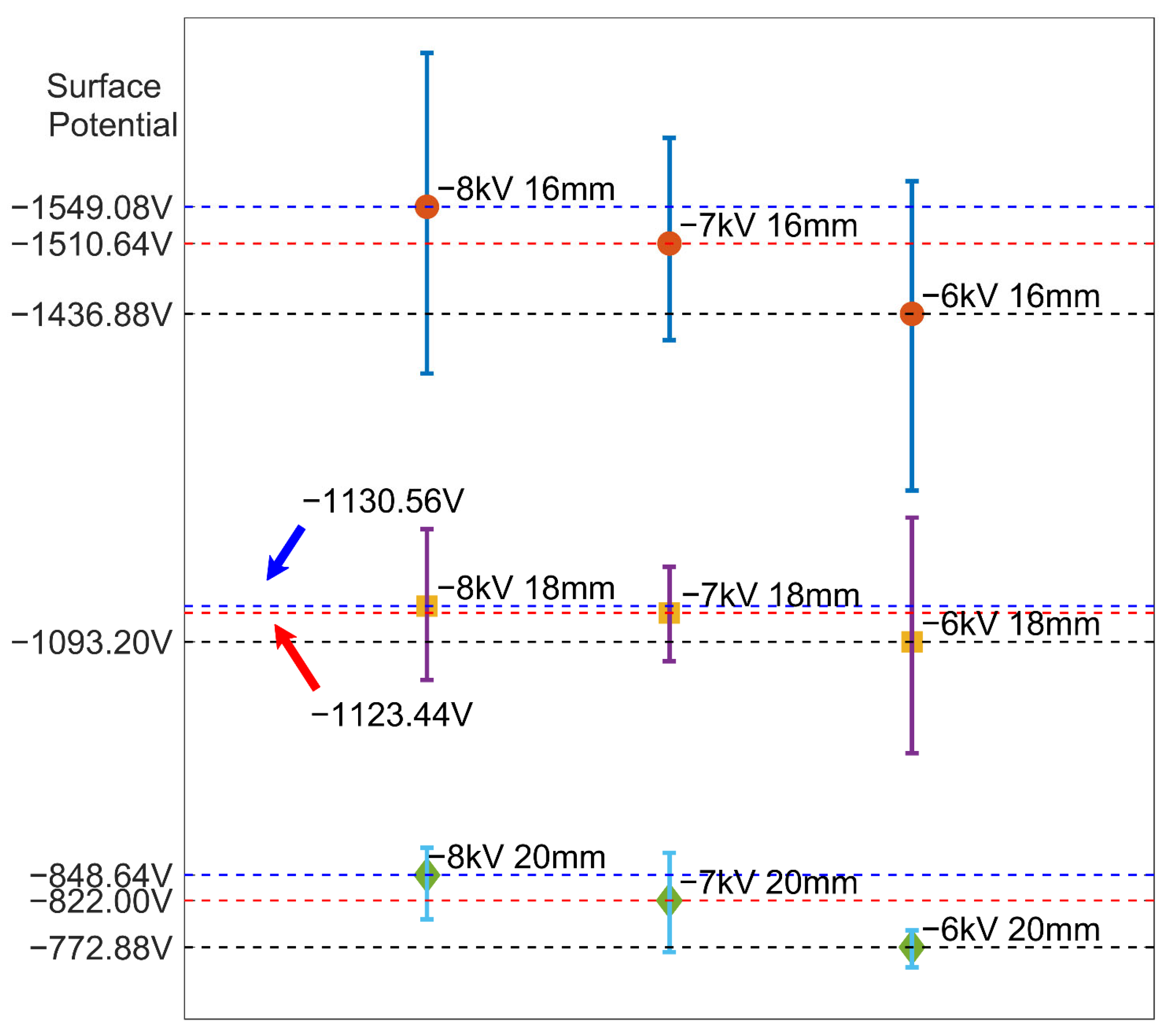
4.3. Surface Potential Measurement
4.4. Surface Potential Decay Analysis
5. Conclusions
- Relatively uniform charge distribution can be achieved on a sufficiently large sample surface, with the standard deviation of surface potential not exceeding 36.98%;
- The surface potential retention rate is improved by 5.40~32.94-fold compared to ordinary corona-charged samples;
- The proposed method has at least a 32.79% improvement in surface potential decay;
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Ngo, T.D.; Kashani, A.; Imbalzano, G.; Nguyen, K.T.Q.; Hui, D. Additive Manufacturing (3D Printing): A Review of Materials, Methods, Applications and Challenges. Compos. Pt. B Eng. 2018, 143, 172–196. [Google Scholar] [CrossRef]
- Murr, L.E. Metallurgy Principles Applied to Powder Bed Fusion 3D Printing/Additive Manufacturing of Personalized and Optimized Metal and Alloy Biomedical Implants: An Overview. J. Mater. Res. Technol. 2020, 9, 1087–1103. [Google Scholar] [CrossRef]
- Rodríguez-Reyna, S.L.; Mata, C.; Díaz-Aguilera, J.H.; Acevedo-Parra, H.R.; Tapia, F. Mechanical Properties Optimization for PLA, ABS and Nylon + CF Manufactured by 3D FDM Printing. Mater. Today Commun. 2022, 33, 104774. [Google Scholar] [CrossRef]
- Sandhu, K.; Singh, S.; Mustansar Hussain, C. 3D Printing of Nanomaterials Using Inkjet Printing. In Additive Manufacturing with Functionalized Nanomaterials; Micro and Nano Technologies; Singh, S., Hussain, C.M., Eds.; Elsevier: Amsterdam, The Netherlands, 2021; pp. 155–192. ISBN 978-0-12-823152-4. [Google Scholar]
- Khoshnevis, B. Automated Construction by Contour Crafting—Related Robotics and Information Technologies. Autom. Constr. 2004, 13, 5–19. [Google Scholar] [CrossRef]
- Yuan, C.; Wang, T.; Dunn, M.L.; Qi, H.J. 3D Printed Active Origami with Complicated Folding Patterns. Int. J. Precis Eng. Manuf.-Green Technol. 2017, 4, 281–289. [Google Scholar] [CrossRef]
- Hao, W.; Liu, Y.; Zhou, H.; Chen, H.; Fang, D. Preparation and Characterization of 3D Printed Continuous Carbon Fiber Reinforced Thermosetting Composites. Polym. Test. 2018, 65, 29–34. [Google Scholar] [CrossRef]
- Du, J.; Fu, G.; Xu, X.; Elshahawy, A.M.; Guan, C. 3D Printed Graphene-Based Metamaterials: Guesting Multi-Functionality in One Gain. Small 2023, 2207833. [Google Scholar] [CrossRef]
- Bauer, S. Poled Polymers for Sensors and Photonic Applications. J. Appl. Phys. 1996, 80, 5531–5558. [Google Scholar] [CrossRef]
- Singh, R. A Review of Developments in Thermal Techniques for Charge Profile Measurements in Polymer Electrets. J. Electrost. 2014, 72, 322–329. [Google Scholar] [CrossRef]
- Zhang, B.; Zhou, Y.; Wang, N.; Liang, X.; Guan, Z.; Takada, T. Polarity Reversal Charging of Polypropylene Films under DC Corona Discharge. J. Electrost. 2005, 63, 657–663. [Google Scholar] [CrossRef]
- Das, D.; Waychal, A. On the Triboelectrically Charged Nonwoven Electrets for Air Filtration. J. Electrost. 2016, 83, 73–77. [Google Scholar] [CrossRef]
- Montanari, G.C. Electrical Measurements for the Characterization of Electret Properties of Cellular Polymers. IEEE Trans. Dielect. Electr. Insul. 2003, 10, 615–622. [Google Scholar] [CrossRef]
- Najihi, I.; Ennawaoui, C.; Hajjaji, A.; Boughaleb, Y. 3D Printed Cellular Piezoelectric Polymers for Smart Sensors/Autonomous Energy Harvesters. Mater. Today Proc. 2022, 66, 437–440. [Google Scholar] [CrossRef]
- Assagra, Y.A.O.; Altafim, R.A.P.; do Carmo, J.P.; Altafim, R.A.C.; Rychkov, D.; Wirges, W.; Gerhard, R. A New Route to Piezo-Polymer Transducers: 3D Printing of Polypropylene Ferroelectrets. IEEE Trans. Dielectr. Electr. Insul. 2020, 27, 1668–1674. [Google Scholar] [CrossRef]
- Mirkowska, A.; Kacprzyk, R.; Rozmaryniewicz, K. Piezoelectric Structure With a 3-D Printed Mesh Layer. IEEE Trans. Dielectr. Electr. Insul. 2022, 29, 823–828. [Google Scholar] [CrossRef]
- Palitó, T.T.C.; Assagra, Y.A.O.; Altafim, R.A.P.; Carmo, J.P.; Altafim, R.A.C. Hydrophone Based on 3D Printed Polypropylene (PP) Piezoelectret. Electron. Lett. 2019, 55, 203–204. [Google Scholar] [CrossRef]
- Kierzewski, I.; Bedair, S.S.; Hanrahan, B.; Tsang, H.; Hu, L.; Lazarus, N. Adding an Electroactive Response to 3D Printed Materials: Printing a Piezoelectret. Addit. Manuf. 2020, 31, 100963. [Google Scholar] [CrossRef]
- Alison, J.M.; Hill, R.M. A Model for Bipolar Charge Transport, Trapping and Recombination in Degassed Crosslinked Polyethene. J. Phys. D Appl. Phys. 1994, 27, 1291–1299. [Google Scholar] [CrossRef]
- Cai, X.; Wang, X.; Pang, D.; Zou, X.; Yu, J.; Lu, Z. Investigation of Current Density, Recombination Rate and Space Charge Density in Polyethylene Thin Films Based on Bipolar Charge Transport Model. Mater. Res. Express 2019, 6, 096451. [Google Scholar] [CrossRef]
- Galikhanov, E.; Lounev, I.; Guzhova, A.; Gusev, Y.; Galikhanov, M.; Vasilyeva, M.; Akkus, B.; Oktem, Y.; Yalcin, L.S.; Mutlu, R.B.C.; et al. (Eds.) Study of Polylactic Acid Electret by Dielectric Spectroscopy. In Proceedings of the 9th International Physics Conference of the Balkan Physical Union (bpu-9), Istanbul, Turkey, 24–27 August 2023; Amer Inst Physics: Melville, NY, USA, 2016; Volume 1722, p. 290002. [Google Scholar]
- Roudný, P.; Syrový, T. Thermal Conductive Composites for FDM 3D Printing: A Review, Opportunities and Obstacles, Future Directions. J. Manuf. Process. 2022, 83, 667–677. [Google Scholar] [CrossRef]
- Song, Y.; Li, Y.; Song, W.; Yee, K.; Lee, K.-Y.; Tagarielli, V.L. Measurements of the Mechanical Response of Unidirectional 3D-Printed PLA. Mater. Des. 2017, 123, 154–164. [Google Scholar] [CrossRef]
- Guzhova, A.A.; Galikhanov, M.F.; Gorokhovatsky, Y.A.; Temnov, D.E.; Fomicheva, E.E.; Karulina, E.A.; Yovcheva, T.A. Improvement of Polylactic Acid Electret Properties by Addition of Fine Barium Titanate. J. Electrost. 2016, 79, 1–6. [Google Scholar] [CrossRef]





| Physical Properties of PLA | Value |
|---|---|
| Density | 1.25 g/cm3 |
| Melting point | 449.15 K |
| Intrinsic viscosity | 1.20 dL/g |
| Glass transition temperature | 333.15 K |
| Tensile strength | 40.00 MPa |
| Elastic modulus | 3000.00 Mpa |
| Flexural modulus | 100.00 Mpa |
| Dielectric dissipation factor | 0.01 |
| Relative permittivity | 3.10 |
| Volume resistivity | 4.30 × 1017 Ω·cm |
| Process Variables | Value |
|---|---|
| Length of cuboid sample | 12 mm |
| Width of cuboid sample | 12 mm |
| Height of cuboid sample | 30 mm |
| Filling percentage | 10% |
| Printing speed | 75 mm/s |
| Printing temperature | 483.15 K |
| Charging and Printing | Time Constant τ1 | Ratio | Time Constant τ2 | Ratio |
|---|---|---|---|---|
| −8 kV, 16 mm when printing | 0.62344 | 97.8% | 58.65102 | 749.9% |
| −7 kV, 16 mm when printing | 0.90991 | 142.6% | 265.46323 | 3394.2% |
| −6 kV, 16 mm when printing | 0.03631 | 5.7% | 55.18763 | 705.6% |
| −8 kV, 18 mm when printing | 0.30525 | 47.9% | 124.25447 | 1588.7% |
| −7 kV, 18 mm when printing | 0.03888 | 6.1% | 150.76134 | 1927.6% |
| −6 kV, 18 mm when printing | 0.03671 | 5.7% | 53.67686 | 686.3% |
| −8 kV, 20 mm when printing | 0.64184 | 100.6% | 89.84725 | 1148.8% |
| −7 kV, 20 mm when printing | 0.03602 | 5.6% | 50.07511 | 640.2% |
| −6 kV, 20 mm when printing | 0.36805 | 57.7% | 68.63417 | 877.6% |
| −7.7 kV, 20 mm after printing | 0.63775 | 100.0% | 7.82101 | 100.0% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, Z.; Song, Q.; Wu, H.; Feng, B.; Li, Y.; Bu, L. Synchronized 3D Printing and Corona Charging for One-Step Prototyping of Polarized Polylactic Acid Electrets. Polymers 2023, 15, 2520. https://doi.org/10.3390/polym15112520
Wang Z, Song Q, Wu H, Feng B, Li Y, Bu L. Synchronized 3D Printing and Corona Charging for One-Step Prototyping of Polarized Polylactic Acid Electrets. Polymers. 2023; 15(11):2520. https://doi.org/10.3390/polym15112520
Chicago/Turabian StyleWang, Zhiwei, Qinghua Song, Huarui Wu, Baolong Feng, Yeyuan Li, and Ling Bu. 2023. "Synchronized 3D Printing and Corona Charging for One-Step Prototyping of Polarized Polylactic Acid Electrets" Polymers 15, no. 11: 2520. https://doi.org/10.3390/polym15112520
APA StyleWang, Z., Song, Q., Wu, H., Feng, B., Li, Y., & Bu, L. (2023). Synchronized 3D Printing and Corona Charging for One-Step Prototyping of Polarized Polylactic Acid Electrets. Polymers, 15(11), 2520. https://doi.org/10.3390/polym15112520






