3.1. Structural Characteristics of Neat PEF and PEF Nanocomposites
Incorporating carbon fillers in a polymer can induce changes in the crystalline and molecular structure of the matrix, and therefore, a structural analysis of the prepared PEF materials has been conducted to obtain further information. For this reason, XRD, FTIR-ATR, and XPS measurements were employed to investigate the effect of GNPs, CNTs, and their synergistic effect on the crystalline phase and molecular conformation of the PEF matrix.
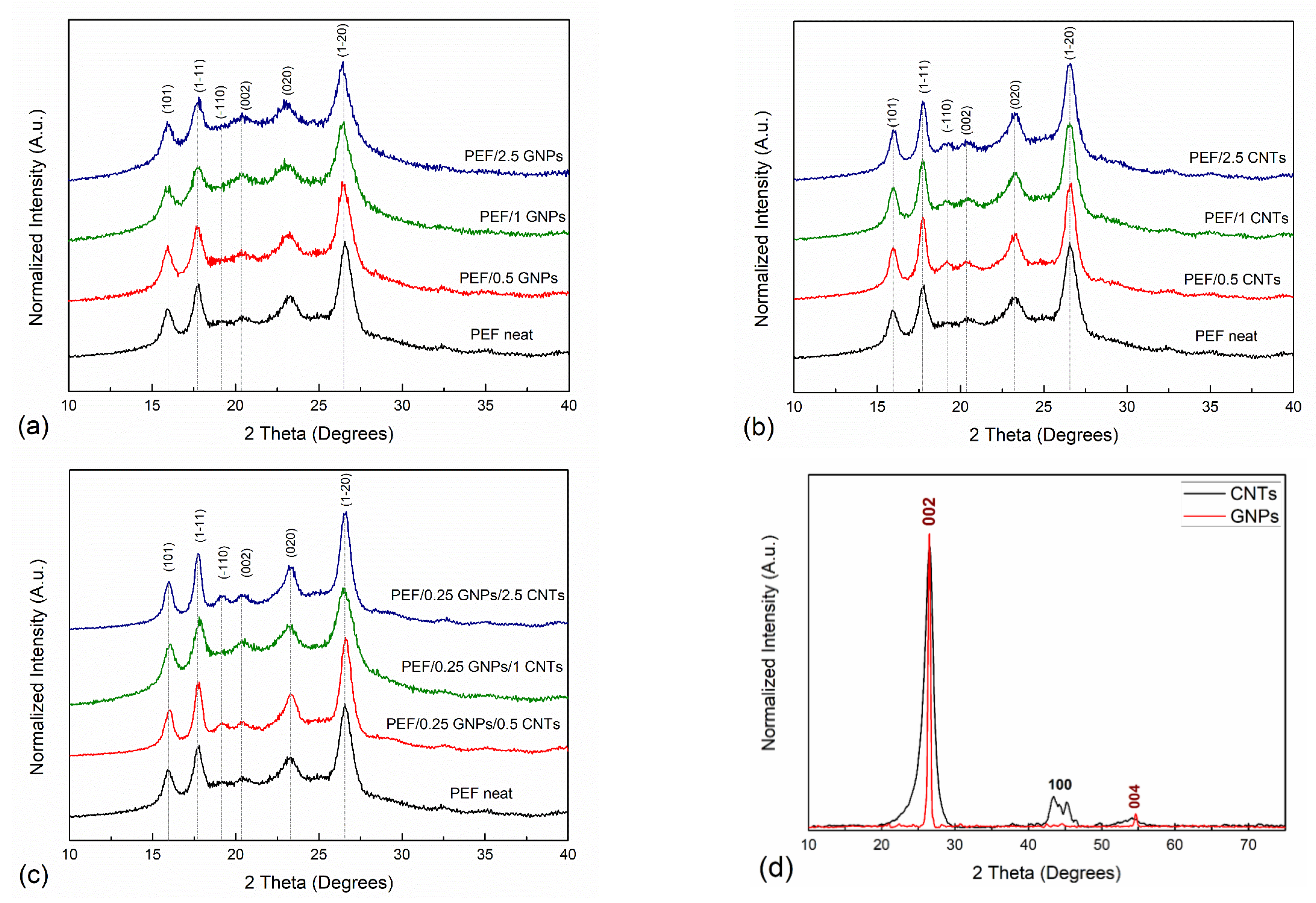
The XRD patterns of the pristine GNPs and CNTs that were incorporated in the PEF matrix are presented in
Figure 1. The main diffraction peaks of GNPs appear at 26.55° and 54.65° correspond to (002) [(0002)] and (004) [(0004)] planes of the graphitic structure with a d-spacing value of 3.36 Å and 1.68 Å, respectively, which agrees with the reported literature values [
36,
37]. The corresponding CNTs diffractogram presents diffraction peaks at 26.45°, 43.45°, and 54°, corresponding to the (002) [(0002)], (100) [(10-10)], and (004) [(0004)] crystallographic planes, respectively. The (002) and (004) planes correspond to a d-spacing of 3.35 Å and 1.675 Å, respectively, while the (100), which originated from the two-dimensional lattice of the CNTs, corresponds to a d-spacing of 2.08 Å, which is in agreement with the reported literature values [
38]. It can be observed that the diffraction peaks of CNTs are wider compared to GNPs, suggesting the formation of smaller crystals [
38], as will be shown from the TEM images later in the manuscript.
Figure 1a–c present the XRD patterns of cold crystallized at 418 K neat PEF with PEF/GNPs, PEF/GNPs, PEF/CNTs, and PEF/0.25 GNPs/CNTs nanocomposites, respectively. All the PEF samples present six diffraction peaks at 2θ: 15.9°, 17.7°, 19.2°, 20.4°, 23.2°, and 26.6°, corresponding to (101), (1-11), (-110), (002), (020), and (1-20) of the triclinic α-phase of PEF, correspondingly [
39]. At first glance, it can be observed that the crystallographic peaks of PEF/CNTs and PEF/0.25 GNPs/CNTs nanocomposites appear to be slightly sharper compared to neat PEF and PEF/GNPs nanocomposites, suggesting finer crystal formation in these cases. To further evaluate the crystal structure of all the prepared PEF materials, the unit cell parameters, the lamellar thickness oriented along the reported crystallographic planes, and the crystallinity degree were calculated.
The interplanar spacings of a material’s diffraction peaks were calculated from the Bragg diffraction formula:
where
n indicates the reflection order,
λ is the wavelength of CuK
α radiation (
λ = 1.5406 Å) [
40], and
θ is the diffraction peak’s angle. When the crystal system of a material is known, the unit cell constants, namely the a, b, and c unit cell vectors, then α, β, and γ angles can be obtained. The obtained values were calculated in this work using the Jade software (Materials Data Inc., Livermore, CA, USA).
Peak profile analysis was applied in this work to calculate the crystal size (
Lhkl) using Scherrer’s equation [
41] (Equation (2)):
where
B is the full-width half maximum of the corresponding peak at angle
θ and
K is the geometrical factor, here equal to 0.9 [
42]. The crystal size calculations were applied for the main peaks of all the prepared materials (neat PEF and its nanocomposites).
The crystallinity degree of a semicrystalline material can be calculated from the XRD patterns. Amorphous content in a sample is identified by the presence of a broad halo in the XRD pattern. The weight crystalline fractions of all the prepared annealed materials were calculated in this work using the equation proposed by Matthews et al. [
43]:
where
Xc is the crystallinity degree and
Acr and
Aam correspond to the calculated areas of crystalline and amorphous peaks, respectively.
Table 3 presents the lattice parameters of neat PEF and all the prepared PEF nanocomposites. Several studies focus on the structural characteristics of PEF. In 1968, Kazaryan et al. firstly proposed the triclinic cell with dimensions of a = 5.75 Å, b = 5.35 Å, c = 20.1 Å and α = 113.5°, β = 90°, and γ = 112° [
44]. However, later, these proposed unit cell parameters have been dismissed due to causing a false positioning of the main peaks in the XRD pattern [
39]. A more recent study by Mao et al. proposed, for the case of PEF fibers, a monoclinic cell with unit cell parameters of a = 5.784 Å, b = 6.780 Å, c = 20.296 Å, and γ = 103.3° [
45]. Recently, however, Maini et al. investigated the polymorphs of PEF obtained by usual methods, such as the one used in this work [
39]. They have concluded that in the case of PEF’s α-phase the triclinic cell is proposed with the unit cell parameters of a = 5.729 Å, b = 7.89 Å, c = 9.62 Å, α = 98.1°, β = 65.1°, γ = 101.3° [
39]. Considering the above, the unit cell parameters a, b, and c, and their mutual angles α, β, and γ were calculated, and are close in value to the ones reported by Maini et al. The a, b, and c unit cell lengths values do not present significant deviations between neat PEF and PEF nanocomposites in any case of incorporated carbon filler; the same applies to the calculated β and γ angles. On the other hand, the α angle, which is the angle between the b and c axis, presents higher values in the case of the PEF nanocomposites (1–2° increase) when compared to neat PEF. This augmentation does not seem to be related to each filler’s content. The altered values of α angle suggest that incorporating any of the selected carbon fillers imposes a minor slide between the molecular segments that form the unit cell along the c-axis. The unit cell volume of all the prepared PEF materials was also calculated. No significant variations of V were observed in the case of PEF/GNPs and PEF/CNTs nanocomposites compared to the neat PEF. However, hybrid PEF/0.25 GNPs/CNTs unit cell volume slightly increases with increasing CNTs content compared to the rest of the PEF samples.
It has been reported that the lamellar thickness of a polymer increases with molecular weight [
46,
47]. To investigate the effect of both molecular weight and each filler’s content on the crystal size of the PEF matrix, the lamellar thickness of the polymer crystals oriented across (101), (1-11), (-110), (002) (020), and (1-20) planes were calculated by the Scherrer–Debye equation and are presented in
Table 3. For the sake of accuracy and minimization of experimental errors, the lamellar thickness corresponding to the most distinct diffraction peaks, i.e., (101), (1-11), and (1-20), will be taken into account to analyze the crystal behavior of the PEF nanocomposites. The calculated crystal thicknesses of the prepared PEF samples do not seem to follow a specific trend related to their average molecular weight. Therefore, it is assumed that the fillers’ effect is the predominant factor that controls the lamellar thicknesses’ behavior in the PEF nanocomposites.
The crystallization process is controlled by two factors: nucleation, where heterogeneous nucleation is favored in the case of an incorporating filler, and diffusion, where the macromolecular chains migrate to the surface of a formed nucleus. Introducing a filler particle can affect both factors, either by facilitating the crystal growth or constraining it. Regarding the PEF nanocomposites, the values of the calculated lamellar thicknesses of PEF/GNPs nanocomposites decrease with increasing filler content. Similar findings have been reported in the case of HDPE/GNPs nanocomposites [
48]. This behavior is attributed to the hindrance of the molecular chains’ diffusion to the surface of the existing nuclei caused by the GNPs, despite the heterogeneous nucleation effect of the platelets on the polymer chains.
On the other hand, the PEF/CNTs nanocomposites present a rather different behavior; the lamellar thicknesses are higher than neat PEF and increase with increasing the CNTs content. Similar behavior has been observed in the case of iPP/CNTs nanocomposites [
49,
50]. The two-dimensional character of the CNTs facilitates the initial heterogeneous nucleation of the PEF molecular chains and does not obscure the migration of the polymer chains to the surface of the formed nuclei, thus allowing further crystal growth. In the case of the hybrid PEF nanocomposites, the GNPs and CNTs appear to have a competitive effect on the lamellar thicknesses of PEF. Compared to neat PEF, all hybrid nanocomposites present thicker lamellae. However, these values are lower than their PEF/CNTs nanocomposites counterparts for 0.5 and 1 wt.% of CNTs. The small number of GNPs (0.25 wt.%) is enough to constrain the crystal growth of the PEF matrix; nonetheless, this effect is diminished when the CNTs content of the hybrid nanocomposites reaches 2.5 wt.%.
The crystallinity degree of the annealed PEF materials has been calculated using Equation (3), and the results are reported in
Table 4 and illustrated in
Figure 2. In any case of the PEF nanocomposites, the X
c values increase with the augmentation of the filler. PEF/CNTs present slightly higher crystalline fraction values than the PEF/GNPs counterparts, with the exception of 2.5 wt.% filler content, which presents the same value. Increased crystallinity in polymers results in improved mechanical properties; in our previous works, the crystalline PEF/GNPs and PEF/CNTs nanocomposites presented significantly higher hardness and elastic modulus values when compared to their amorphous counterpart and neat PEF [
28,
29]. This behavior is attributed to the highly ordered lamellae which are characterized by a more significant intermolecular bonding leading to an enhanced hardness, strength, and elastic modulus. The hybrid nanocomposites show significantly higher crystallinity degree values at any CNTs content compared to the PEF/CNTs and PEF/GNPs nanocomposites counterparts, suggesting that the heterogeneous nucleation was promoted due to the larger number of nucleating sites, i.e., each filler’s particles, and/or due to the contribution of the crystalline peaks of the fillers which are not clearly visible in the respective diffractograms; this will be mentioned later in the manuscript.
ATR measurements were also conducted on the annealed (at 423 K) prepared PEF materials. It has been reported that the crystalline and amorphous regions of PEF are sensitive to specific conformational preferences observed through their absorbance spectrum [
51]. A possible bond formation between the incorporated fillers and the PEF matrix can also be confirmed or disproved by the ATR spectra of the PEF nanocomposites. The ATR absorbance spectra of neat PEF with PEF/GNPs and PEF/CNTs have been presented in our previous work [
27], and the corresponding ones of PEF/0.25 GNPs/CNTs nanocomposites are presented in
Figure 3a. No noticeable differences have been observed between the neat PEF and PEF/Carbon nanofillers nanocomposites. The main absorbance peaks around 3245–3045 cm
−1 and 3045–2865 cm
−1 (noted with yellow and Turquoise squares, respectively) are attributed to the C-H asymmetric and symmetric stretching of the furan ring’s carbon–hydrogen bonds, and the methylene group’s asymmetric stretching of the EG fragment of the PEF’s molecule. The large peak between 1840–1615 cm
−1 corresponds to the stretching of C=O ester bonds, while the peak at 1575 cm
−1 is assigned to the asymmetric stretching of the C=C double bond on the furan ring. The latter bond is reported to be sensitive to the polymer–filler interactions [
52], i.e., when bonds between the incorporated filler and the polymer matrix are formed, the peak migrates to lower wavenumbers. As seen in
Figure 3b, no migration of the aforementioned peak has been observed, and therefore, it can be suggested that no chemical interactions between the incorporated filler and the PEF matrix are present, as has been already mentioned in our previous work for the PEF/GNPs and PEF/CNTs nanocomposites [
27].
Additional information regarding the crystalline and amorphous state of the PEF materials can be derived through the preferred polymer chain’s conformation, which can be observed at the ATR spectra. More particularly, it has been reported by Araujo et al. that the preferred conformations of the FDCA and EG fragments for the semicrystalline and amorphous PEF are the syn
FDCA-trans
EG and anti
FDCA-gauche
EG (helix) conformations, respectively [
51]. The trans conformation of the EG fragment (O−CH
2−CH
2−O) is characterized by a dihedral angle of 180° and the gauche conformation by a dihedral angle of 60° [
51]. Accordingly, the syn and anti-isomerisms of PEF’s molecule FDCA fragment (O
ring−C
ring−C=O) have a dihedral angle of 0° and 180° [
51].
Figure S1a,b present the ATR spectra of the prepared PEF materials at 1500–1320 cm
−1 and 680–540 cm
−1 wavenumber range. The absorbance peak of 1340 cm
−1 results from the wagging vibrations of CH
2 groups in trans-EG units [
51]. The gauche EG segments, which are more prominent in the amorphous PEF, cause the shift of the later vibration to ≈1375 cm
−1. Accordingly, the deformation of trans-CH
2 groups presents an absorbance band at 1477 cm
−1, while the corresponding one of the gauche conformation shifts to ≈1455 cm
−1 [
51]. As seen in
Figure S1a, both conformations are visible on both neat and nanocomposites PEF materials, confirming the semicrystalline character of the annealed samples. The FDCA fragment’s anti and syn isomerisms of PEF yield a broad absorbance band between 630 and 580 cm
−1 as a result of the ring deformation mode, presented in
Figure S1b [
51]. The syn isomerism of the crystalline region is represented by the major peak at 609 cm
−1, while the shoulder peak around 621 cm
−1 is assigned to the FDCA fragment’s anti-isomerism of amorphous regions [
51]. An enlarged image of the later peak of all the prepared PEF materials is presented in
Figure S1b(ii); the bold dotted line represents the neat PEF spectrum region. It is observed that the shoulder peak of 621 cm
−1 is more distinct compared to the ones of PEF/Carbon nanofillers, suggesting that the anti-isomerism found in the amorphous region of PEF is in greater amount, i.e., the nanocomposites are characterized by a higher degree of the crystalline fraction compared to neat PEF, as previously concluded by the XRD analysis.
To further study the intermolecular interactions of neat PEF and PEF/Carbon nanofillers, XPS measurements were employed on selected PEF samples.
Figure S2a–d present the wide scan of neat PEF, PEF/1 GNPs, PEF/1 CNTs, and PEF/0.25 GNPs/1 CNTs, respectively, while the corresponding atomic concentration of carbon and oxygen are reported in
Table S1. Small amounts of Si were found on the surface of the understudied samples, possibly due to glass contamination from the glass flask. Oxygen’s atomic concentration of neat PEF is lower than those of the PEF nanocomposites. It is known that hydroxyl, epoxy, and carbonyl groups are attached to the graphitic sheets of GNPs and CNTs to a small degree. However, the ratio of carbon to oxygen for the PEF nanocomposite is considerably lower compared to the one of neat PEF. The C1s and O1s peaks deconvolution of all the selected materials was conducted to examine the later behavior of the nanocomposites further.
Figure 4a–d present the C1s and
Figure S3a–d present the O1s peak deconvolution of neat PEF, PEF/1 GNPs, PEF/1 CNTs, and PEF/0.25 GNPs/1 CNTs, respectively, while the corresponding binding energies and areas of each deconvoluted peak are reported in
Table 5 and
Table 6, for the C1s and O1s core levels. The deconvoluted carbon peaks found in all the PEF samples at binding energies of 284.6, 285.2, 286, 286.8, and 289 eV (±0.2 eV error values) are attributed to the C=C sp
2 and C-C sp
3 carbon hybridization, and the C-OH, C-O, and C=O/O=C-O bonds [
53,
54], respectively. In the case of the PEF nanocomposites, an extra peak is found at 292 eV attributed to the π-π* transition of the GNPs’ and CNTs’ graphitic layers [
55]. Accordingly, the deconvoluted oxygen peaks were found near 531.4, 532, and 533.6 eV for all the PEF materials and correspond to the O-
C=O, C-OH, and
O-C=O bonds, respectively [
53,
54]. The sp
2 to sp
3 ratio for neat PEF is close to 3, confirming the 6 C-C bonds with sp
2 and 2 C-C bonds with sp
3 hybridization on the PEF monomer; incorporating any of the carbon nanofillers, the corresponding ratio increases due to the sp
2 carbon hybridization of the graphitic layers of the GNPs and CNTs.
To a minor degree, the increase in oxygen atomic concentration and the carbon–oxygen bonds on the XPS spectra of the nanocomposites is attributed to the addition of the carbon nanofillers, as earlier suggested. Since the ATR spectra of the PEF nanocomposites did not suggest the formation of a chemical bond between the filler and the polymer matrix, this possibility is dismissed.
Prior to the polymerization process of PEF nanocomposites, the selected fillers’ dispersion was achieved by their ultrasonication in ethylene glycol. Dispersion of a nanofiller succeeded due to the propagation of the sound waves in altering high and low-pressure cycles through the particles’ aggregates. More specifically, in the case of GNPs and CNTs, during the low-pressure cycles, vapor bubbles are generated in the EG, increasing in size (cavitations). These generated cavitations consecutively collapse throughout the high-pressure cycles releasing high mechanical and thermal energy which causes a temperature increase in the EG and causes the splitting of the larger particles/aggregates. Insertion of the EG molecules between the graphene layers also takes place, causing the GNPs exfoliation [
56]. EG molecules can also be embedded in the CNTs cavities during the ultrasonication process.
As earlier stated, pause-time was applied during ultrasonication of the EG/GNPs or/and CNTs dispersion to avoid excessive heating and the increase of the fluid’s viscosity. During the process, the collapse of the microcavities causes a local increase in pressure and temperature. Given that both of the nanofillers used are characterized by high thermal conductivity, the temperature throughout the fluid’s volume increases rapidly. Pause time was also introduced to avoid viscosity augmentation, especially in the case of hybrid nanocomposites. It has been shown that by increasing the filler’s loading in the liquid medium and applying ultrasonication, the viscosity increases depending on the sonication time [
57]; above a time threshold, the viscosity decreases, due to the finer particles formed during the process. Long-time exposure to ultrasound was avoided in this work, to avoid the excessive formation of structural and topological defects on the GNPs and CNTs (amorphous sp
3-carbon formation, excessive exfoliation, unzipping of the nanotubes to graphenoids, length reduction) [
58]. Therefore, the introduced time breaks in the case of the PEF/GNPs and PEF/CNTs were 30 sec per 5 min, while in the case of the PEF/0.25 GNPs/CNTs they were raised to 1 min per 3 min.
Ultrasonication can also induce oxygenated species, aside from defects, onto the graphene sheets, as suggested by Skaltsas et al. [
59]. The presence of carboxylic acids and ether oxides introduced on the surface of the exfoliated graphene sheets or CNTs was found to be independent of the liquid medium. Thus, the increase in oxygen atomic concentration and the carbon–oxygen bonds on the XPS spectra could be attributed to the introduction of the functionalities on each filler’s surface. The longer pause period that was applied during the ultrasonication process (1 min instead of 30 sec) in the case of the hybrid nanocomposites, as well as the shorter application time (3 instead of 5 min), is possibly the cause of the smaller oxygen atomic concentration compared to the PEF/1 GNPs and PEF/1 CNTs nanocomposites. During ultrasonication, the shorter cycle time of application does not allow the same degree of the nanoparticles’ dispersion or functionalization, as in the rest of the nanocomposites. Additionally, the longer pause period might cause several neighboring dispersed nanoparticles (GNPs or CNTs) to re-agglomerate due to van der Waals’s attractions. Consequently, the following cycle of sonication causes the breaking of these new aggregates, possibly lowering the degree of further functionalization of each filler’s particles.
TEM imaging of the hybrid nanocomposite PEF/0.25 GNPs/1 CNTs was implemented to investigate the CNTs’ morphological and structural characteristics when GNPs are also present in the PEF matrix. In
Figure 5, TEM micrographs of the hybrid PEF nanocomposite are shown, where both carbon fillers seem to be effectively embedded in the PEF matrix (
Figure 5a). The majority of CNTs are organized in the form of large aggregates, with diameters in the range of 200 nm to several μm, comprising large numbers of CNTs that appear bowed and twisted, forming a bundle-like structure. The corresponding ring-type selected area electron diffraction (SAED) patterns of the observed bundles (insets in
Figure 5b,c) confirm that they consist of randomly oriented crystalline CNTs. Here, the (0002) graphitic planes of the CNTs are indicated by arrows. Moreover, the continuous and rather broad form of the rings in the SAED patterns suggests that the dimensions of crystalline CNTs are in the nanoscale range (<100 nm) [
60]. Indeed, as seen in
Figure 5d, measurements of the nanotubes’ average outer (red lines) and inner (yellow lines) diameters resulted in values of 57.7 ± 5.2 nm and 9.16 ± 1.4 nm, respectively. Despite the formation of large CNT aggregates, it was observed that these bundles were homogeneously distributed throughout the PEF matrix. Furthermore, GNPs, consisting of a few single crystalline graphene layers, were also observed in the vicinity of the CNT bundles (
Figure 5a), regardless of their very low density. These observations comply with our initial assumption, i.e., incorporating both fillers in the matrix may result in large aggregates, due to the different ultrasonic procedures followed to avoid excessive heating and increased viscosity (see above).
3.2. Thero-Oxidative Behavior of Neat PEF and PEF Nanocomposites
The thermo-oxidative degradation behavior of neat PEF, PEF/1 GNPs, PEF/1 CNTs, and PEF/0.25 GNPs/1 CNTs was also evaluated by conducting TGA measurements under a controlled dry air atmosphere.
Figure 6a,b present the mass loss and dTG curves of the understudy PEF materials versus temperature, respectively. The temperatures at 2% and 50% mass loss and the peak temperatures of the dTG curve of neat PEF and its nanocomposites are presented in
Table 7. As seen from the dTG curves, the thermo-oxidative degradation takes place in two steps, where the first occurs between 500–730/750 K and is related to the random scission of the ester linkage, and the second one (730/750–870 K) occurs with a subsequent reaction of carbonization [
61]. As seen in
Table 7, the temperature at which the thermo-oxidative degradation begins (T
2%) does not present large deviations between the understudy PEF materials. PEF/1 CNTs nanocomposite appears to be the most thermally stable, while PEF/1 GNPs degrade earlier in temperature compared to the rest of the PEF samples. In terms of thermal stability, the first step’s temperatures that the maximum degradation rate for PEF/1 CNTs and PEF/0.25 GNPs/1 CNTs rate occur are at slightly higher temperatures compared to neat PEF and PEG/1 GNPs; at the same time, the dTG peak of the second degradation step seems to be affected to a greater degree by the presence of the CNTs compared to the first peak. PEF/1 CNTs and PEF/0.25 GNPs/1 CNTs nanocomposites present the second dTG peak at considerably higher temperatures than neat PEF and PEF/GNPs.
The exact reactions and mechanism of PEF’s thermo-oxidative degradation have not been studied in detail yet and are expected to be complex. However, the most probable scenario is that the thermo-oxidative degradation of PEF might be similar to the one of PET, i.e., the initial reaction with oxygen at elevated temperatures involves oxidation at the α-methylene carbon resulting in the formation of hydroperoxide, and homolytic cleavage of the oxygen–carbon bond adjacent to the ester, which results in the formation of aliphatic and benzoic (in the case of PET) acids [
62].
The thermo-oxidative degradation’s effective activation energy of neat PEF, PEF/1 GNPs, PEF/1 CNTs, and PEF/0.25 GNPs/1 CNTs was calculated by the isoconversional differential method of Friedman. Generally, isoconversional analysis can be applied without any assumption on the reaction mechanism and, for this reason, is referred to as a model-free method.
Isoconversional methods follow the isoconversional principle, which declares that the reaction rate is a function of temperature at a constant conversion α, as follows [
31]:
where
α stands for the values related at a given extent of conversion of the process and is calculated by the following equation [
63]:
where
m0 and
mf are the sample’s initial and final weight, and
mt is the sample’s weight at time (
t). According to the isoconversional methods, the activation energy remains constant with temperature and varies with varying degree of conversion [
64]. The isoconversional methods are categorized into differential and integral methods. The most commonly used differential method is the one proposed by Friedman and is given by the following equation [
31]:
where
α stands for the values related at a given extent of conversion. By plotting
ln(
dα/dt) versus 1/
Tα, the value of −Δ
Eα/R for a given degree of crystallinity can be obtained by the slope of the fitted line. The activation energy values of the selected PEF samples versus the degree of conversion are presented in
Figure 7.
For neat PEF, the values of Eα increase with the degree of conversion almost linearly until α = 0.8 and then abruptly shift to lower values (≈100 kJ/mol). Similar behavior is observed in the case of PEF/1 CNTs, while for PEF/1 GNPs, the activation energy is almost constant until α < 0.7 and then drops to 60 kJ/mol. On the contrary, PEF/0.25 GNPs/1 CNTs present rather different behavior. In the initial and middle stages of the degradation, increasing the degree of conversion, the activation energy increases as in the case of neat PEF and PEF/1 CNTs until α = 0.7. When the extent of conversion is between 0.7 and 0.8, during the second step of the thermo-oxidative degradation, the Εα values increase rapidly, and for α > 0.8 they drop to 120 kJ/mol. These changes in the Εα values at the later stages of degradation (α > 0.6) in any case of the PEF materials correspond to the second step of the degradation, the carbonization reaction. Therefore, in the case of the hybrid PEF nanocomposite, the presence of small amounts of GNPs together with CNTs creates a synergistic effect and increases the energy barrier for the carbonization step. The lowest activation energy for the later stages of the degradation is observed for the PEF/1 GNPs nanocomposite, while the highest is for PEF/0.25 GNPs/1 CNTs, as earlier stated. The initial and middle stages of the degradation do not deviate significantly regarding the activation energy values between the neat PEF and nanocomposites.
3.3. Lifetime Prediction
Kinetic Analysis can be used to estimate the lifetime of a material. The lifetime of a material is defined as the time after which a material’s property decays to such an extent that it cannot fulfill the desired application’s function. Using TGA, one can predict the lifetime of a sample when it is exposed to heat and deteriorates due to thermal aging. However, the aging of a material can be caused or accelerated by other factors such as humidity, pressure, mechanical stress, etc. Therefore, the mass loss measurements of TGA can provide limited information on the decay of other properties, such as the mechanical behavior of a polymer. Nevertheless, it is known that a polymer’s mechanical properties deteriorate when the macromolecular chains start to break before the formation of low molecular mass volatile products causes a detectable mass loss in TGA. Thus, one can express a limiting decay of a property in terms of a specific extent of conversion value as an assumption, i.e., when α = 0.05, the deterioration of physical properties is considered significant, thus the lifetime of a material can be approximated by calculating the time needed by a material under a temperature T to reach this value of the conversion [
31].
Lifetime predictions can take place using experiments under any temperature program. Depending on the temperature program, one can select a different approach. Model-based and model-free methods have been proposed to estimate the lifetime of a material. In this work, the resulting E
α derived by the isoconversional method of Friedman was used to estimate the lifetime of selected PEF materials under continuous air flow. The predictive equation was proposed as follows [
65]:
where
tα is the predicted lifetime,
Tα is the temperature at which the selected
α is reached, and
T0 is the selected isothermal temperature at which the prediction is calculated. Due to the lack of a model’s assumption, the method is called model-free prediction. Its main advantage is that it applies to processes for which the effective activation energy varies with the degree of conversion
α, thus providing more reliable results [
31].
Thus, using the results from the isoconversional analysis for the thermo-oxidative degradation of neat PEF, PEF/1 GNPs, PEF/1 CNTs, and PEF/0.25 GNPs/1 CNTs, lifetime predictions were conducted using Equation (7). Through this analysis, the time needed for the understudy material to reach a selected thermo-oxidative degradation’s extent of conversion under isothermal conditions can be calculated. The selected degree of conversions was set to α = 0.05 (approximately 5% mass loss), which corresponds to the early stages of thermo-oxidative decomposition [
66,
67], and the materials’ properties (mechanical, thermal) begin to deteriorate. Therefore, one can calculate a product’s lifetime at the operational temperature, depending on the application, and evaluate the overall endurance of the material through time.
Figure 8 presents the lifetime in years versus selected temperatures for neat PEF, PEF/1 GNPs, PEF/1 CNTs, and PEF/0.25 GNPs/1 CNTs. As seen, the lifetime decreases with increasing temperature for all the understudy materials, as expected. When the temperature is 298 K (25 °C), neat PEF’s thermo-oxidative degradation begins after 5.8 × 10
12 years, while the corresponding lifetimes for PEF/1 GNPs, PEF/1 CNTs, and PEF/0.25 GNPs/1 CNTs are 1.3 × 10
11, 3.5 × 10
11, and 7.3 × 10
9 years, respectively. Therefore, considering that the corresponding effective activation energy values for neat PEF, PEF/1 GNPs, PEF/1 CNTs, and PEF/0.25 GNPs/1 CNTs, when α = 0.05 are 175, 151, 158, and 145 kJ/mol, it can be seen that the lifetime for the given conversion follows the same trend. The above results indicate that the nanocomposite PEF materials deteriorate faster when exposed to ambient temperatures, i.e., when the materials are disposed of to the environment as plastic waste which is a desired trait. As the temperature increases, the lifetime of the understudy PEF materials presents smaller deviations from one another, while there is a turning point temperature at 573 K, where PEF/1 GNPs and PEF/1 CNTs present a higher lifetime than neat PEF. More specifically, neat PEF presents a lifetime equal to ≈4.5 days, while the corresponding lifetimes for PEF/1 GNPs, PEF/1 CNTs, and PEF/0.25 GNPs/1 CNTs nanocomposites are ≈8.8, 6.3, and 1.9 days, respectively. Finally, when the temperature reaches 673 K, the corresponding lifetimes for neat PEF, PEF/1 GNPs, PEF/1 CNTs, and PEF/0.25 GNPs/1 CNTs are 27.9, 113.6, 65.7, and 30.4 min, meaning that with increasing temperature, the lifetime of the nanocomposites exceeds the one of neat PEF, while at lower temperatures, neat PEF is more stable. However, it must be noted that these estimations are made based on the assumption that the temperature is always stable, and the environment consists only of dry air under a constant flow rate without taking into consideration other parameters such as the light (UV radiation), humidity, possible microorganisms’ presence, mechanical loadings, etc. [
67]. When the other parameters are present, the lifetime of each material reduces significantly, but the above analysis is a satisfactory first approach to the service life predictions of the prepared PEF materials.