Advanced FFF of PEEK: Infill Strategies and Material Characteristics for Rapid Tooling
Abstract
:1. Introduction
- How does thermal post-treatment of PEEK affect its compression properties?
- What impact does the choice of material have on the characteristics of the infill patterns?
- How do isotropic and anisotropic infill patterns perform, and what significance does the orientation of the components within the build space hold?
- How do physical parameters, such as infill density and layer thickness, influence the compression properties?
- What infill pattern and density are required to achieve efficient and economical utilization in Rapid Prototyping Tooling (RPT) for PEEK molds?
2. Materials and Methods
2.1. Specimen Design
2.2. FFF Laboratory Plant
2.3. Uniaxial Compression Test
2.4. Thermal Post Treatment
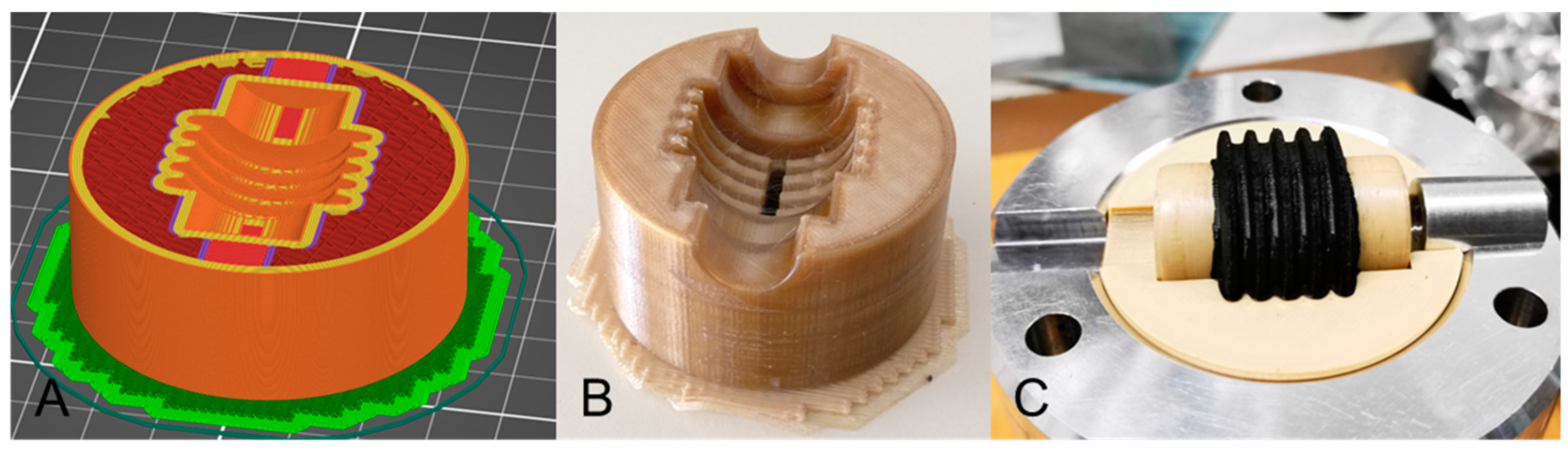
2.5. Proof of Concept
3. Results
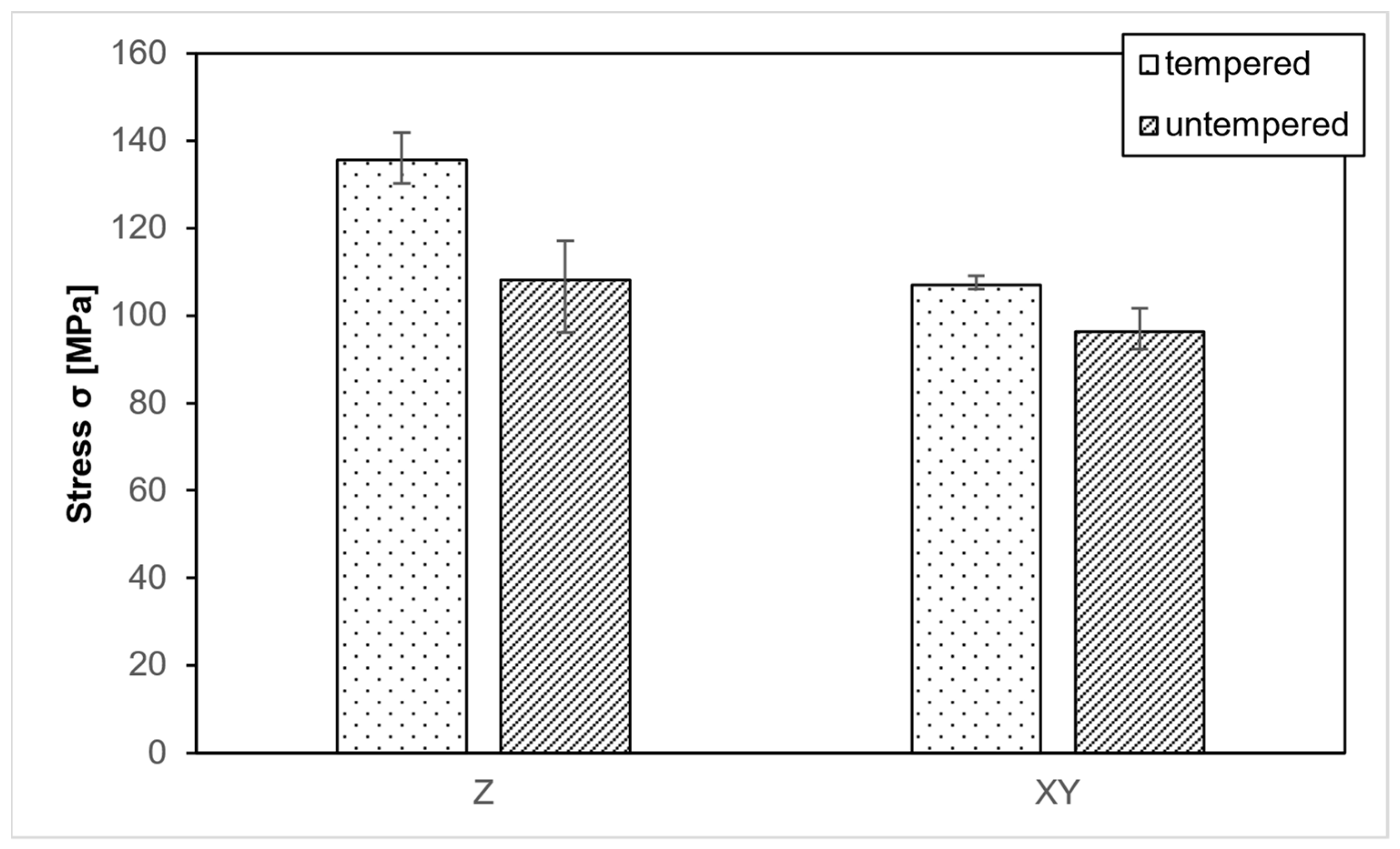
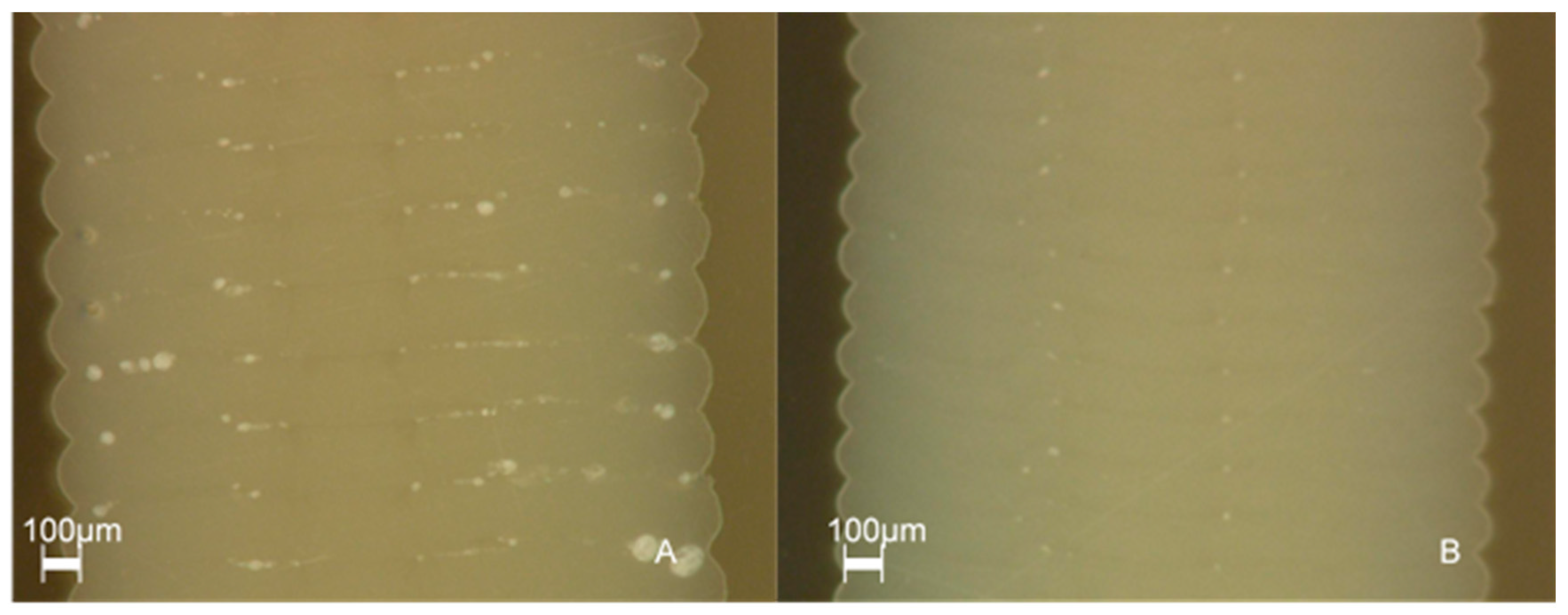
3.1. Effect of the Post-Heat Treatment
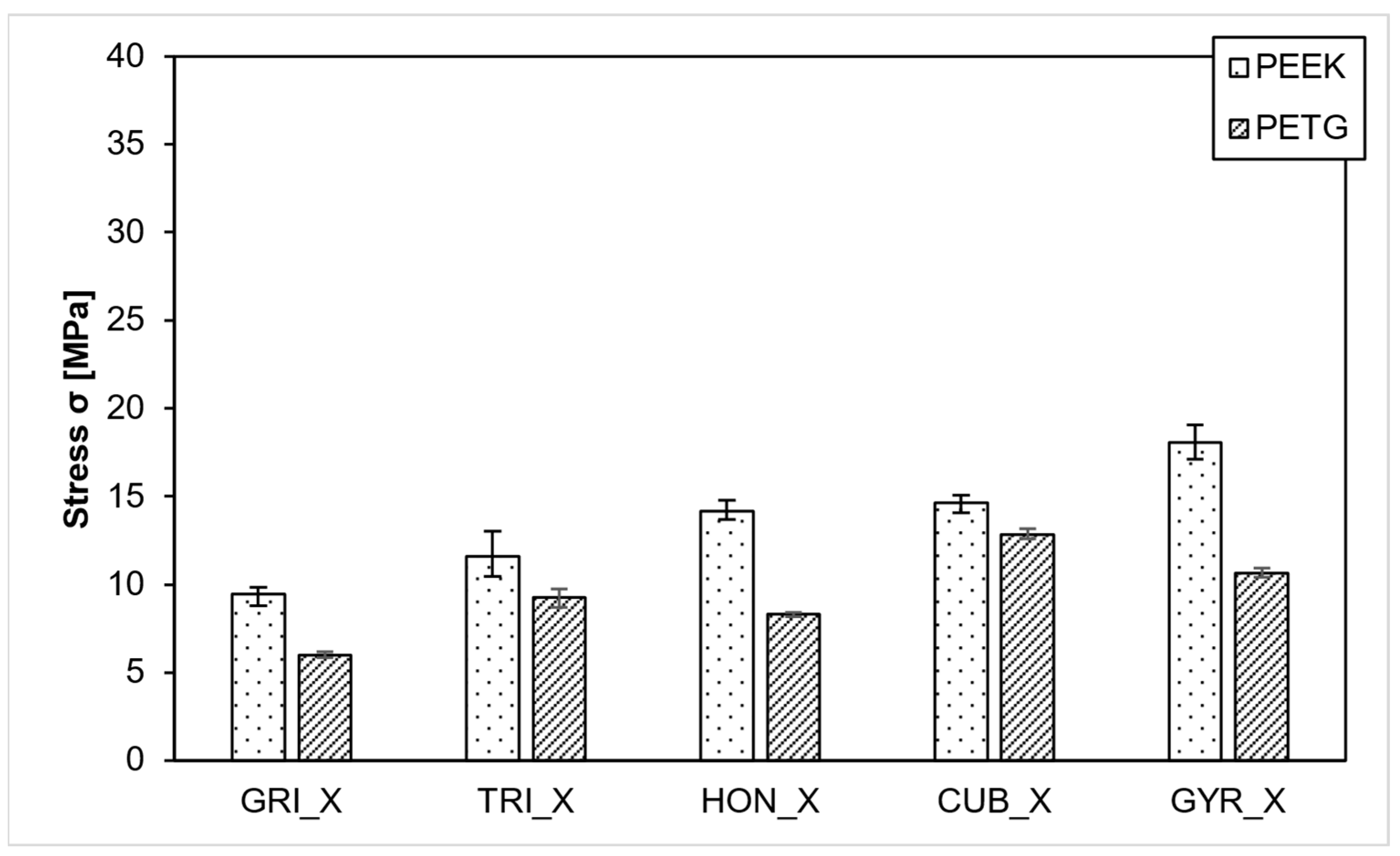
3.2. Material Comparison
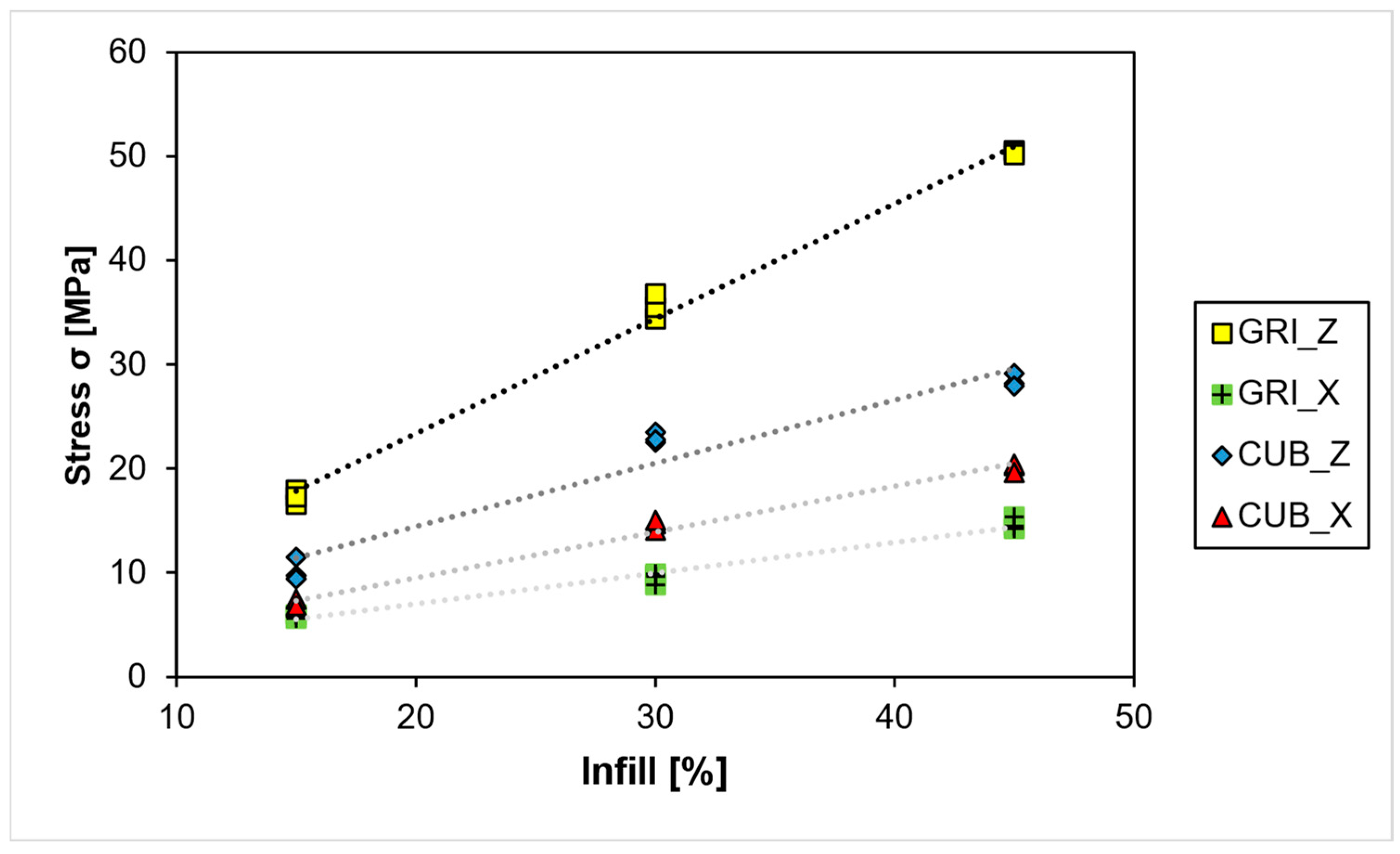
3.3. Effect of the Infill Density
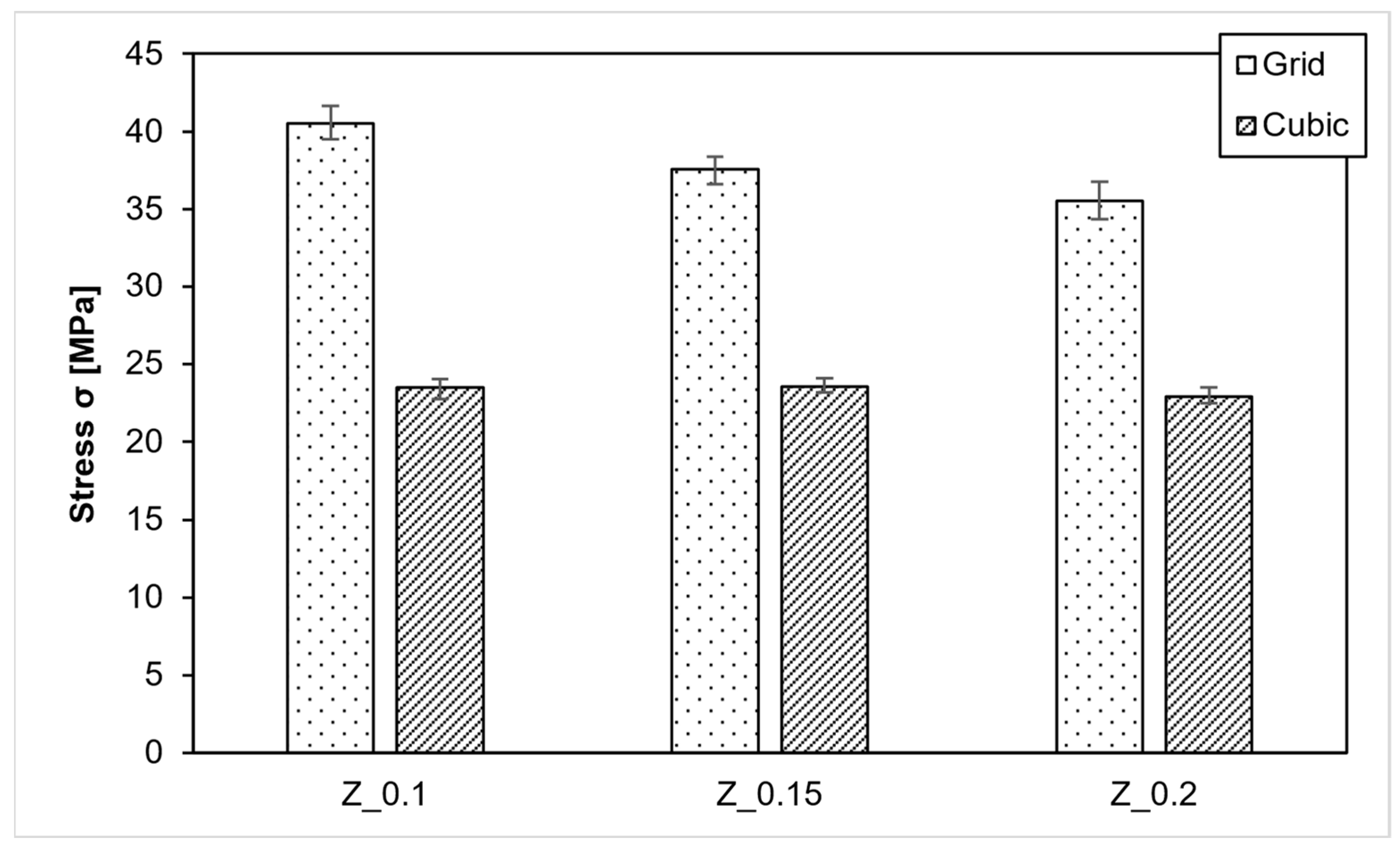
3.4. Effect of the Layer Height
3.5. Proof of Concept
4. Discussion and Conclusions
- Processing of PEEK at room temperature is possible, but it should be noted that the components must be thermally post-treated to exploit the full potential of the mechanical properties.
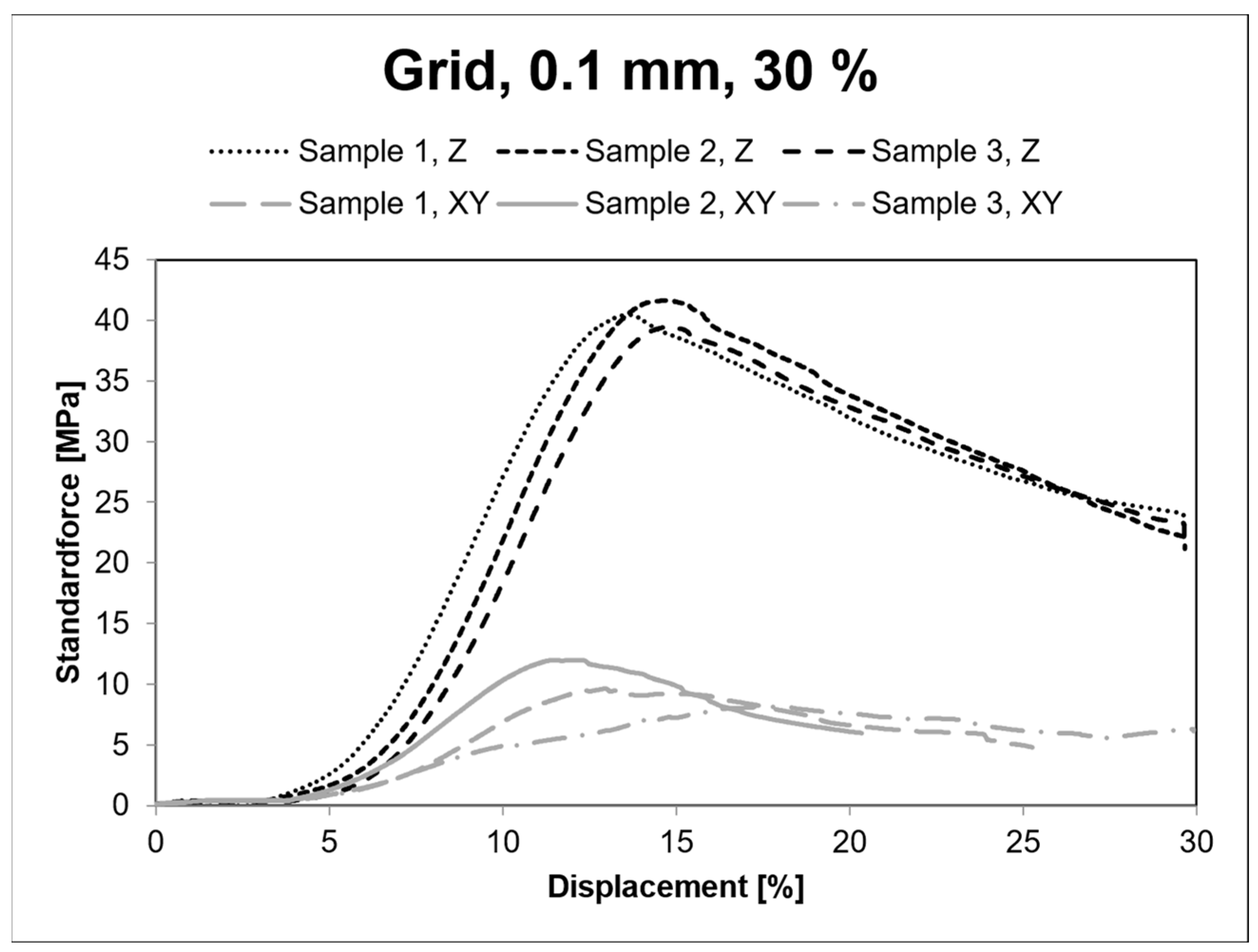
- For components subject to significant stress in the Z direction, the most effective infill pattern is the grid, offering a strength of 35.6 MPa when using a 30% density. Conversely, when dealing with high-stress scenarios in all directions, it is recommended to employ cubic infill, which achieves a strength of 14.6 MPa at a 30% infill density.
- A layer height of 0.1 mm results in greater strength compared to a 0.15 mm layer height, which, in turn, exhibits greater strength than a 0.2 mm layer height. This effect is more pronounced with cubic infill, where transitioning from a 0.2 mm to a 0.1 mm layer height increases strength by 15%, whereas the same layer height change with a grid pattern only provides a 5% strength gain. Interlayer bonding plays an essential role in influencing compressive strength.
- Using the adapted infill rate, resources and costs can be saved in the rapid tooling of vulcanization molds. Production costs can be reduced by more than 70%. PEEK is suitable for the vulcanization injection molding of rubber components thanks to its excellent material properties.
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Frohn-Sörensen, P.; Geueke, M.; Engel, B.; Löffler, B.; Bickendorf, P.; Asimi, A.; Bergweiler, G.; Schuh, G. Design for 3D Printed Tools: Mechanical Material Properties for Direct Polymer Additive Tooling. Polymers 2022, 14, 1694. [Google Scholar] [CrossRef] [PubMed]
- Gebhardt, A. Rapid Prototyping, 1st ed.; Hanser Gardner: Munich, Germany, 2003. [Google Scholar]
- Gebhardt, A.; Hötter, J.-S. Additive Manufacturing: 3D Printing for Prototyping and Manufacturing; Hanser Publications: Munich, Germany, 2016. [Google Scholar]
- Kuo, C.-C.; Pan, X.-Y. Development of a Rapid Tool for Metal Injection Molding Using Aluminum-Filled Epoxy Resins. Polymers 2023, 15, 3513. [Google Scholar] [CrossRef] [PubMed]
- Kampker, A.; Ayvaz, P.; Lukas, G. Direct Polymer Additive Tooling—Economic Analysis of Additive Manufacturing Technologies for Fabrication of Polymer Tools for Injection Molding. Key Eng. Mater. 2020, 843, 9–18. [Google Scholar] [CrossRef]
- Apium, Case Study: Mould Making with FFF 3D Printed CFR PEEK Parts—Time and Cost Saving Potential [Internet]. 2019. Available online: https://apiumtec.com/en/case-study-mould-making-with-fff-3d-printed-cfr-peek-parts-time-and-cost-saving-potential (accessed on 24 April 2022).
- Hanemann, T.; Klein, A.; Baumgärtner, S.; Jung, J.; Wilhelm, D.; Antusch, S. Material Extrusion 3D Printing of PEEK-Based Composites. Polymers 2023, 15, 3412. [Google Scholar] [CrossRef]
- Gohn, A.M.; Brown, D.; Mendis, G.; Forster, S.; Rudd, N.; Giles, M. Mold inserts for injection molding prototype applications fabricated via material extrusion additive manufacturing. Addit. Manuf. 2022, 51, 102595. [Google Scholar] [CrossRef]
- Farioli, D.; Strano, M.; Vangosa, F.B.; Zaragoza, V.G.; Aicardi, A. Rapid tooling for injection molding inserts. ESAFORM 2021, 2021. [Google Scholar] [CrossRef]
- Dizon, J.R.C.; Valino, A.D.; Souza, L.R.; Espera, A.H.; Chen, Q.; Advincula, R.C. 3D Printed Injection Molds Using Various 3D Printing Technologies. Mater. Sci. Forum 2020, 1005, 150–156. [Google Scholar] [CrossRef]
- Park, S.J.; Lee, J.E.; Park, J.; Lee, N.-K.; Son, Y.; Park, S.-H. High-temperature 3D printing of polyetheretherketone products: Perspective on industrial manufacturing applications of super engineering plastics. Mater. Des. 2021, 211, 110163. [Google Scholar] [CrossRef]
- Huzaim, N.H.M.; Rahim, S.Z.A.; Musa, L.; Abdellah, A.E.-H.; Abdullah, M.M.A.B.; Rennie, A.; Rahman, R.; Garus, S.; Błoch, K.; Sandu, A.V.; et al. Potential of Rapid Tooling in Rapid Heat Cycle Molding: A Review. Materials 2022, 15, 3725. [Google Scholar] [CrossRef]
- Vassallo, M.; Rochman, A. Rapid prototyping solution for the production of vulcanized rubber components. In Proceedings of the 22nd International Esaform Conference on Material Forming: Esaform 2019, Vitoria-Gasteiz, Spain, 8–10 May 2019; p. 150014. [Google Scholar]
- Abbas, K.; Balc, N.; Bremen, S.; Skupin, M. Crystallization and Aging Behavior of Polyetheretherketone PEEK within Rapid Tooling and Rubber Molding. J. Manuf. Mater. Process. 2022, 6, 93. [Google Scholar] [CrossRef]
- Panda, B.; Leite, M.; Biswal, B.B.; Niu, X.; Garg, A. Experimental and numerical modelling of mechanical properties of 3D printed honeycomb structures. Measurement 2018, 116, 495–506. [Google Scholar] [CrossRef]
- Qi, C.; Jiang, F.; Yang, S. Advanced honeycomb designs for improving mechanical properties: A review. Compos. Part B Eng. 2021, 227, 109393. [Google Scholar] [CrossRef]
- Schoen, A.H. Infinite Periodic Minimal Surfaces without Self-Intersections: Three Dimensional Euclidean Space Partitioned into Interpenetrating Labyrinths by Infinite Periodic Minimal Surfaces without Self Intersections; NASA Technical Note: Cambridge, MA, USA, 1970. [Google Scholar]
- Karcher, H. The triply periodic minimal surfaces of Alan Schoen and their constant mean curvature companions. Manuscripta Math. 1989, 64, 291–357. [Google Scholar] [CrossRef]
- Abueidda, D.W.; Bakir, M.; Al-Rub, R.K.A.; Bergström, J.S.; Sobh, N.A.; Jasiuk, I. Mechanical properties of 3D printed polymeric cellular materials with triply periodic minimal surface architectures. Mater. Des. 2017, 122, 255–267. [Google Scholar] [CrossRef]
- Al-Ketan, O.; Abu Al-Rub, R.K. Multifunctional Mechanical Metamaterials Based on Triply Periodic Minimal Surface Lattices. Adv. Eng. Mater. 2019, 21, 1900524. [Google Scholar] [CrossRef]
- Pandzic, A.; Hodzic, D.; Milovanovic, A. Effect of Infill Type and Density on Tensile Properties of PLA Material for FDM Process. In Proceedings of the 30th International DAAAM Symposium 2019. DAAAM Proceedings, Zadar, Croatia, 23–26 October 2019; Katalinic, B., Ed.; DAAAM International Vienna: Maria Lankowitz, Austria, 2019. [Google Scholar]
- Cerda-Avila, S.N.; Medellín-Castillo, H.I.; Lim, T. An experimental methodology to analyse the structural behaviour of FDM parts with variable process parameters. Rapid Prototyp. J. 2020, 26, 1615–1625. [Google Scholar] [CrossRef]
- Mishra, S.B.; Malik, R.; Mahapatra, S.S. Effect of External Perimeter on Flexural Strength of FDM Build Parts. Arab. J. Sci. Eng. 2017, 42, 4587–4595. [Google Scholar] [CrossRef]
- Lužanin, O.; Movrin, D.; Plančak, M. Effect Of Layer Thickness, Deposition Angle, and Infill on Maximum Flexural Force in Fdm-Built Specimens. J. Technol. Plast. 2014, 39, 49–58. [Google Scholar]
- Sood, A.K.; Ohdar, R.K.; Mahapatra, S.S. Experimental investigation and empirical modelling of FDM process for compressive strength improvement. J. Adv. Res. 2012, 3, 81–90. [Google Scholar] [CrossRef]
- Abbas, T.; Othman, F.M.; Ali, H.B. Effect of Infill Parameter on Compression Property in FDM Process; International Journal of Engineering Research and Applications; International Organization of Scientific Research: Ghaziabad, India, 2017. [Google Scholar]
- Dey, A.; Yodo, N. A Systematic Survey of FDM Process Parameter Optimization and Their Influence on Part Characteristics. J. Manuf. Mater. Process. 2019, 3, 64. [Google Scholar] [CrossRef]
- Bakhtiari, H.; Nikzad, M.; Tolouei-Rad, M. Influence of Three-Dimensional Printing Parameters on Compressive Properties and Surface Smoothness of Polylactic Acid Specimens. Polymers 2023, 15, 3827. [Google Scholar] [CrossRef] [PubMed]
- Borah, J.; Chandrasekaran, M.; Selvarajan, L. Investigation on Fdm-3d Printing of Peek Parts as Biomedical Implants for Improving Mechanical Strength and Surface Quality. Available online: https://papers.ssrn.com/sol3/papers.cfm?abstract_id=4484802 (accessed on 26 July 2023).
- Arikan, V. Optimizing Infill Parameters for Improved Mechanical Performance and Cost Savings in Additive Manufacturing. Int. J. Comput. Exp. Sci. Eng. 2023, 9, 225–232. [Google Scholar] [CrossRef]
- Kaiser, W. Kunststoffchemie für Ingenieure: Von der Synthese bis zur Anwendung, 5th ed.; Hanser: München, Germany, 2021. [Google Scholar]
- Rahman, K.M.; Letcher, T.; Reese, R. Mechanical Properties of Additively Manufactured PEEK Components Using Fused Filament Fabrication, Volume 2A: Advanced Manufacturing. In Proceedings of the ASME 2015 International Mechanical Engineering Congress and Exposition, Houston, TX, USA, 13–19 November 2015; American Society of Mechanical Engineers: New York, NY, USA, 2015. [Google Scholar]
- Xu, Y.; Zhang, F.; Zhai, W.; Cheng, S.; Li, J.; Wang, Y. Unraveling of Advances in 3D-Printed Polymer-Based Bone Scaffolds. Polymers 2022, 14, 566. [Google Scholar] [CrossRef] [PubMed]
- Moncayo-Matute, F.P.; Vázquez-Silva, E.; Peña-Tapia, P.G.; Torres-Jara, P.B.; Moya-Loaiza, D.P.; Viloria-Ávila, T.J. Finite Element Analysis of Patient-Specific 3D-Printed Cranial Implant Manufactured with PMMA and PEEK: A Mechanical Comparative Study. Polymers 2023, 15, 3620. [Google Scholar] [CrossRef] [PubMed]
- Chung, H.-J.; Lee, H.-B.; Park, K.-M.; Jung, T.-G.; Kim, S.-B.; Lee, B.-G.; Kim, W.-C.; Lee, J.-K. Feasibility of 3D-Printed Locking Compression Plates with Polyether Ether Ketone (PEEK) in Tibial Comminuted Diaphyseal Fractures. Polymers 2023, 15, 3057. [Google Scholar] [CrossRef] [PubMed]
- Kang, J.; Zheng, J.; Hui, Y.; Li, D. Mechanical Properties of 3D-Printed PEEK/HA Composite Filaments. Polymers 2022, 14, 4293. [Google Scholar] [CrossRef]
- Zanjanijam, A.R.; Major, I.; Lyons, J.G.; Lafont, U.; Devine, D.M. Fused Filament Fabrication of PEEK: A Review of Process-Structure-Property Relationships. Polymers 2020, 12, 1665. [Google Scholar] [CrossRef]
- Wu, W.; Geng, P.; Li, G.; Zhao, D.; Zhang, H.; Zhao, J. Influence of Layer Thickness and Raster Angle on the Mechanical Properties of 3D-Printed PEEK and a Comparative Mechanical Study between PEEK and ABS. Materials 2015, 8, 5834–5846. [Google Scholar] [CrossRef]
- Wächter, J.; Elsner, M.; Moritzer, E. Investigation of the Processability of Different PEEK Materials in the FDM Process with Regard to the Weld Seam Strength; University of Texas at Austin: Austin, TX, USA, 2019. [Google Scholar]
- Timoumi, M.; Barhoumi, N.; Znaidi, A.; Maazouz, A.; Lamnawar, K. Mechanical behavior of 3D-printed PEEK and its application for personalized orbital implants with various infill patterns and densities. J. Mech. Behav. Biomed. Mater. 2022, 136, 105534. [Google Scholar] [CrossRef]
- Paszkiewicz, S.; Lesiak, P.; Walkowiak, K.; Irska, I.; Miądlicki, K.; Królikowski, M.; Piesowicz, E.; Figiel, P. The Mechanical, Thermal, and Biological Properties of Materials Intended for Dental Implants: A Comparison of Three Types of Poly(aryl-ether-ketones) (PEEK and PEKK). Polymers 2023, 15, 3706. [Google Scholar] [CrossRef]
- Mrówka, M.; Machoczek, T.; Jureczko, P.; Joszko, K.; Gzik, M.; Wolański, W.; Wilk, K. Mechanical, Chemical, and Processing Properties of Specimens Manufactured from Poly-Ether-Ether-Ketone (PEEK) Using 3D Printing. Materials 2021, 14, 2717. [Google Scholar] [CrossRef] [PubMed]
- DIN EN ISO 604:2003-12; Kunststoffe_-Bestimmung von Druckeigenschaften (ISO_604:2002). Deutsche Fassung EN_ISO_604:2003. Beuth Verlag GmbH: Berlin, Germany, 2003. [CrossRef]
- Bean, P.; Lopez-Anido, R.A.; Vel, S. Numerical Modeling and Experimental Investigation of Effective Elastic Properties of the 3D Printed Gyroid Infill. Appl. Sci. 2022, 12, 2180. [Google Scholar] [CrossRef]
- Victrex, Victrex_PROCESSING-Finishing-Operations_DE. Available online: https://www.victrex.com/de/downloads/technical-guides/victrex-peek-finishing-operations-brochure; (accessed on 8 January 2023).
- Dua, R.; Rashad, Z.; Spears, J.; Dunn, G.; Maxwell, M. Applications of 3D-Printed PEEK via Fused Filament Fabrication: A Systematic Review. Polymers 2021, 13, 4046. [Google Scholar] [CrossRef]
- Wang, P.; Zou, B.; Xiao, H.; Ding, S.; Huang, C. Effects of printing parameters of fused deposition modeling on mechanical properties, surface quality, and microstructure of PEEK. J. Mater. Process. Technol. 2019, 271, 62–74. [Google Scholar] [CrossRef]
- Sola, A. Materials Requirements in Fused Filament Fabrication: A Framework for the Design of Next-Generation 3D Printable Thermoplastics and Composites. Macro. Mater. Eng. 2022, 307, 2200197. [Google Scholar] [CrossRef]
- Yang, D.; Cao, Y.; Zhang, Z.; Yin, Y.; Li, D. Effects of crystallinity control on mechanical properties of 3D-printed short-carbon-fiber-reinforced polyether ether ketone composites. Polym. Test. 2021, 97, 107149. [Google Scholar] [CrossRef]
- Ritter, T.; McNiffe, E.; Higgins, T.; Sam-Daliri, O.; Flanagan, T.; Walls, M.; Ghabezi, P.; Finnegan, W.; Mitchell, S.; Harrison, N.M. Design and Modification of a Material Extrusion 3D Printer to Manufacture Functional Gradient PEEK Components. Polymers 2023, 15, 3825. [Google Scholar] [CrossRef]
- Li, Q.; Zhao, W.; Yang, W.; Wang, G. Flexural Properties and Fracture Behavior of CF/PEEK in Orthogonal Building Orientation by FDM: Microstructure and Mechanism. Polymers 2019, 11, 656. [Google Scholar] [CrossRef]

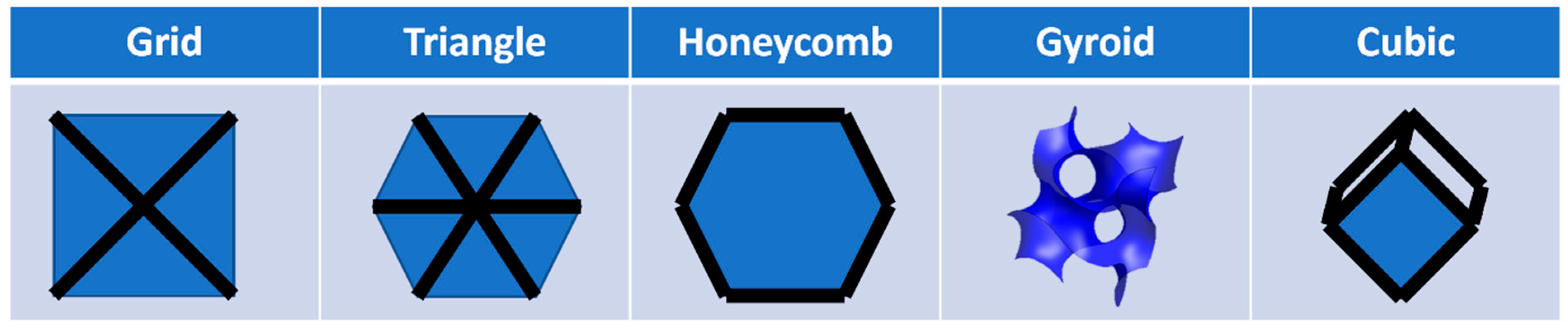



| Study | Analysis | Material | Parameter | Results |
|---|---|---|---|---|
| Pandzic [21] | Tensile | PLA | Pattern (Grid, Lines, Triangles, Tri-Hexagonal, Cubic, Cubic-Subdivision, Octet, Quarter Cubic, Concentric, Zig Zag, Cross, Cross 3D, Gyroid) | Concentric Infill has the highest tensile strength |
| Cerda [22] | Flexural | PLA | Density (20%, 40%, 80%, 100%), Orientation (X, Y, Z) | Higher infill higher strength Orientation is a key parameter |
| Mishra [23] | Flexural | ABS | Contours (1, 3, 5), layer thickness (0.18 mm, 0.25 mm, 0.33 mm), raster width, raster angle, part orientation, air gap | Higher strength with thicker walls |
| Luzanin [24] | Flexural | PLA | Layer height (0.1 mm, 0.2 mm, 0.3 mm), orientation (0°, 30°, 60°), density (10%, 20%, 30%) | Layer thickness has the greatest effect on strength The combination of orientation and density also has a significant influence |
| Sood [25] | Compression | ABS | Layer thickness, orientation, raster angle, raster width, air gap | Good connection between material webs is important for strength |
| Abbas [26] | Compression | PLA | Straight-line infill, 20%, 35%, 50%, 65%, 80% | Higher density leads to higher strength |
| Bakthiari [28] | Compression, Surface roughness, Density | PLA | layer height (0.05, 0.15, 0.25), extrusion width (0.45 mm, 0.55 mm, 0.65 mm), nozzle temperature (190 °C, 205 °C, 220 °C), printing speed (30 mm/s, 50 mm/s, 70 mm/s) | 0.05 mm layers and 0.65 mm extrusion width |
| Borah [29] | Surface roughness, Hardness, Tensile, Elongation | PEEK | infill density (60%, 70%, 80%), print speed (25 mm/s, 30 mm/s, 35 mm/s), and infill pattern (octet, gyroid, triangular) | High density, low print speed |
| Arikan [30] | Compression | PLA | Pattern (Line, Cubic, Octet, Triangles, Tri-Hexagon), density (10%, 15%, 20%) | Triangular and tri-hexagonal, high density |
| Rahman [32] | Tensile, Compression, Flexural, Impact | PEEK | Solid infill angle (0°, 90°, 0°/90°) | Compression: 50–75 MPa |
| Wu [38] | Compression | PEEK | FFF compared to Injection molding | 76.7% of injection-molded force |
| Wächter [39] | Tensile | PEEK | Nozzle (430 °C, 440 °C, 450 °C) and build chamber temperature (150 °C, 200 °C, 250 °C) | Best results: Nozzle 450 °C, Chamber 250 °C |
| Paszkiewicz [41] | DSC TGA Tensile Impact XRD | PEEK/PEKK | Nozzle temperature (360–380 °C), print speed (15–25mm/s), infill (20–100%), layer height (0.2 mm), BCT (90 °C) | PEKK HT with the most promising mech. properties and less bacterial adhesion for medical use |
| Timoumi [40] | Tensile | PEEK | Nozzle temperature (420 °C, 450 °C), print speed (20 mm/s, 30 mm/s), layer thickness (0.1 mm, 0.2 mm), density (40%, 55%, 70%) | Nozzle 450 °C, 0.1 mm layers, 20 mm/s speed Higher density leads to higher strength |
| Mrowka [42] | Tensile Tree-point-bending Impact | PEEK | Nozzle temperature (425 °C), print speed (30 mm/s), infill (100%), layer height (0.15 mm), orientation (0°, 90° X, 90° Y) Parts were tested in amorphous and crystalline form | Lowest mech. properties of vertical specimen, 4.82–43.67 MPa tensile strength, impact strength 6.57–112.09 kj/m² |
| Section | Samples | Parameter |
|---|---|---|
| 1 | 12—3 per point | Density: 100% Heat treatment: Y/N Orientation: XY/Z Material: PEEK |
| 2 | 60—3 per point | Density: 30% Pattern: Grid, triangle, honeycomb, cubic, gyroid Orientation: XY/Z Material: PEEK/PETG |
| 3 | 36—3 per point | Density: 15%, 30%, 45% Pattern: Grid, cubic Orientation: XY/Z Material: PEEK |
| 4 | 36—3 per point | Density: 30% Pattern: Grid, cubic Layer thickness: 0.1 mm, 0.15 mm, 0.2 mm Orientation: XY/Z Material: PEEK |
| Parameter | Value |
|---|---|
| Nozzle temperature | 410 °C |
| Build plate temperature | 150 °C |
| Chamber temperature | Room temperature |
| Print speed | 40 mm/s |
| Pattern/Density | Grid | Triangle | Honeycomb | Cubic | Gyroid |
|---|---|---|---|---|---|
| 15% | 2 h 2 min | 1 h 50 min | 2 h 16 min | 1 h 58 min | 2 h 20 min |
| 30% | 2 h 18 min | 2 h 23 min | 3 h 22 min | 2 h 25 min | 3 h 30 min |
| 45% | 2 h 56 min | 2 h 55 min | 4 h 43 min | 2 h 53 min | 4 h 50 min |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Abbas, K.; Hedwig, L.; Balc, N.; Bremen, S. Advanced FFF of PEEK: Infill Strategies and Material Characteristics for Rapid Tooling. Polymers 2023, 15, 4293. https://doi.org/10.3390/polym15214293
Abbas K, Hedwig L, Balc N, Bremen S. Advanced FFF of PEEK: Infill Strategies and Material Characteristics for Rapid Tooling. Polymers. 2023; 15(21):4293. https://doi.org/10.3390/polym15214293
Chicago/Turabian StyleAbbas, Karim, Lukas Hedwig, Nicolae Balc, and Sebastian Bremen. 2023. "Advanced FFF of PEEK: Infill Strategies and Material Characteristics for Rapid Tooling" Polymers 15, no. 21: 4293. https://doi.org/10.3390/polym15214293
APA StyleAbbas, K., Hedwig, L., Balc, N., & Bremen, S. (2023). Advanced FFF of PEEK: Infill Strategies and Material Characteristics for Rapid Tooling. Polymers, 15(21), 4293. https://doi.org/10.3390/polym15214293








