Limited Optimal Plastic Behavior of RC Beams Strengthened by Carbon Fiber Polymers Using Reliability-Based Design
Abstract
:1. Introduction
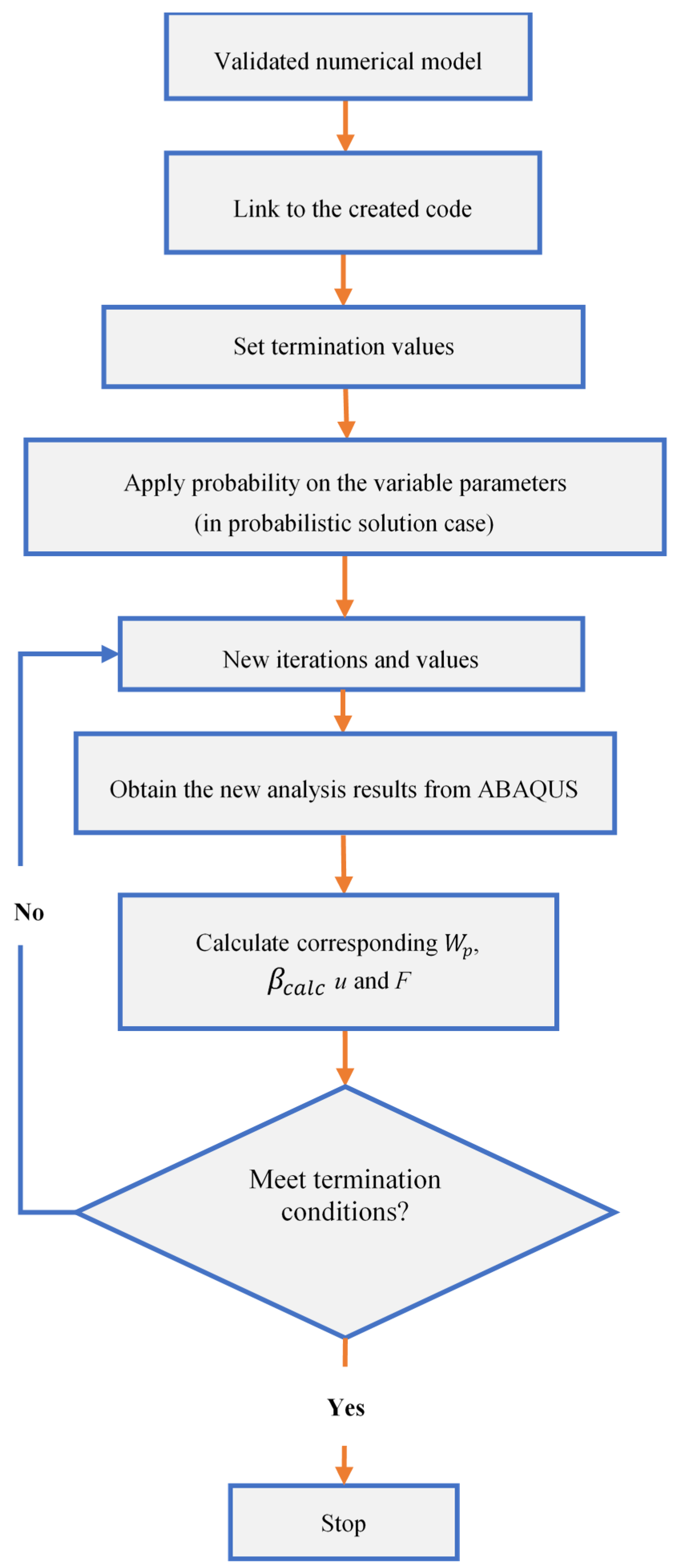
2. Methodology
2.1. Plastic Behavior Limitation Principal
2.2. Probability Theory
2.3. Optimum Solution
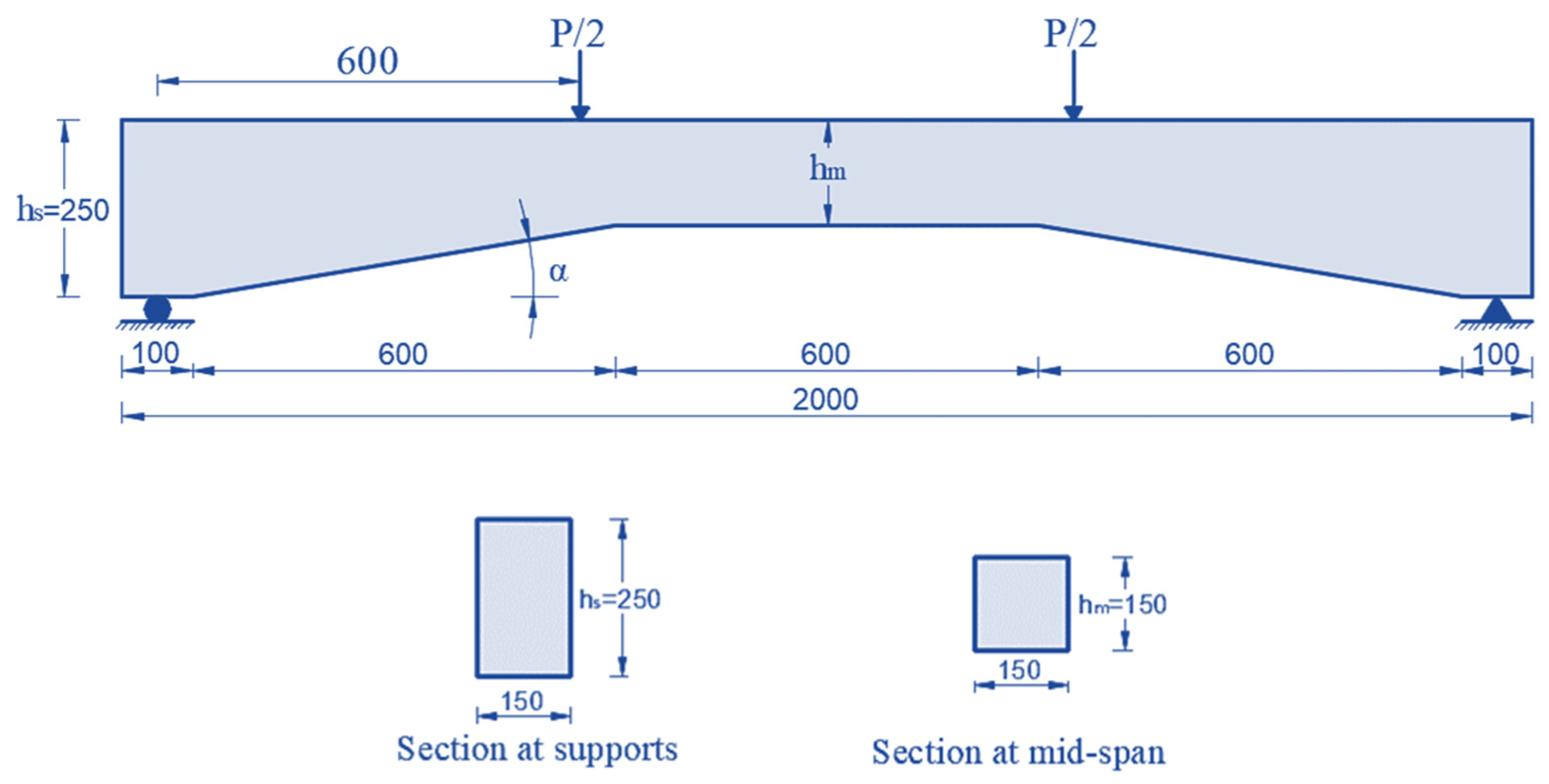
3. Model Validation
4. Results and Discussion
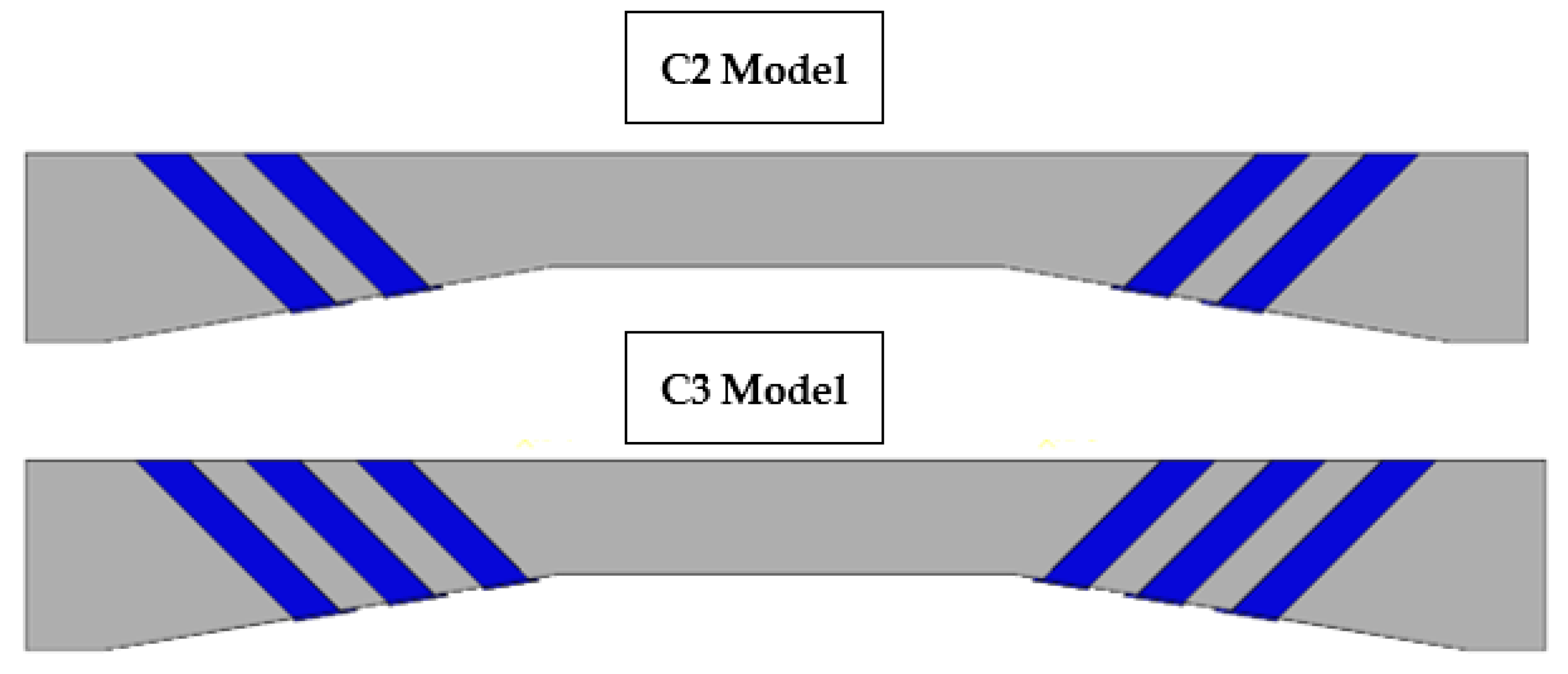
4.1. The Case of C2 Model with Two U-Wrap CFRP Strips
4.2. The Case of C3 Model with Three U-Wrap CFRP Strips
5. Conclusions
- It can be seen from the results that the randomness of the presented variables affected the load and deflection values clearly.
- The reliability index value increases as the corresponding load value decreases, and that reflects the role of increased probability of failure in the models subjected to higher loading conditions.
- Tension concrete damage percentages dt % are reflected in the damage pattern presented in the results, where it can be concluded that as the produced load increase the damage intensity also increases, declaring that extra load applies extra initial stresses inside steel and concrete materials. This is how the complementary strain energy role is highlighted by reflecting the plastic damage, and as it is controlled, the plastic damage and failure will also be controlled.
- The effectiveness of CFRP strips in absorbing the extra stresses caused by increasing applied loads can also be seen by comparing C2 and C3 results, where it is clear that the presence of the additional CFRP strips reduced the damaged areas even though the corresponding models’ strengths are increased.
- This research accounts for the concrete material’s uncertainties, which is its true state, since it is hard to determine its properties without probability. Since CFRP is one of the most effective materials for strengthening structural elements, the uncertainties of its characteristics were considered to explore how these probabilities affect the behaviour of reinforced concrete beams enhanced with varied numbers of CFRP strips with variable properties.
- It can be realised that in the deterministic cases, as the complementary strain, energy is spotted with different values, and the corresponding load and deflection values are changing. In addition, if it is taken into consideration, the complementary strain values increase significantly when approaching the ultimate load where plastic behaviour controls, and thus initiates, higher loading and deflection values.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Haque, M. Non-linear finite element formulations of reinforced concrete haunched beam (RCHB). Asian J. Civ. Eng. 2022, 23, 1029–1064. [Google Scholar] [CrossRef]
- López-Chavarrıa, S.; Luévanos-Rojas, A.; Medina-Elizondo, M.; Garcıa-Galván, M.; Sandoval-Rivas, R.; Garay-Gallardo, J.R.; Rivera-Mendoza, J.B. Optimal cost design of reinforced concrete t-beams with straight haunches. Int. J. Innov. Comput. Inf. Control 2022, 18, 739–754. [Google Scholar] [CrossRef]
- Vasudevan, G.; Kothandaraman, S.; Azhagarsamy, S. Study on non-linear flexural behavior of reinforced concrete beams using ANSYS by discrete reinforcement modeling. Strength Mater. 2013, 45, 231–241. [Google Scholar] [CrossRef]
- Tahenni, T.; Bouziadi, F.; Boulekbache, B.; Amziane, S. Experimental and nonlinear finite element analysis of shear behaviour of reinforced concrete beams. In Structures; Elsevier: Amsterdam, The Netherlands, 2021; Volume 29, pp. 1582–1596. [Google Scholar] [CrossRef]
- Sena-Cruz, J.; Barros, J.A.; Azevedo, Á.F.; Gouveia, A.V. Numerical simulation of the nonlinear behavior of RC beams strengthened with NSM CFRP strips. In Proceedings of the CMNE/CILAMCE 2007, Oporto, Portugal, 13–15 June 2007; Available online: http://hdl.handle.net/1822/9129 (accessed on 20 November 2022).
- Hatzigeorgiou, G.D.; Liolios, A.A. Nonlinear behaviour of RC frames under repeated strong ground motions. Soil Dyn. Earthq. Eng. 2010, 30, 1010–1025. [Google Scholar] [CrossRef]
- Lu, C.L.; Ouyang, K.; Guo, C.; Wang, Q.; Chen, H.Q.; Zhu, W.X. Axial compressive performance of RC columns strengthened with prestressed CFRP fabric combined with UHPC jacket. Eng. Struct. 2023, 275, 115113. [Google Scholar] [CrossRef]
- Alabdulhady, M.Y.; Chkheiwer, A.H.; Ojaimi, M.F. The efficiency of CFRP strengthening and repair system on the flexural behavior of RC beams constructed with different concrete compressive strength. Results Eng. 2022, 16, 100763. [Google Scholar] [CrossRef]
- Zhou, C.; Xie, Y.; Wang, W.; Zheng, Y. Performance-based design for improving impact resistance of RC bridge piers with CFRP grid-reinforced ECC. Eng. Struct. 2023, 275, 115217. [Google Scholar] [CrossRef]
- Fan, L.; Jin, L.; Du, X. Modeling of size effect on compressive behavior of CFRP-confined rectangular RC columns: Influence of corner radius and aspect ratio. Compos. Struct. 2022, 293, 116400. [Google Scholar] [CrossRef]
- Xian, G.; Guo, R.; Li, C.; Wang, Y. Mechanical performance evolution and life prediction of prestressed cfrp plate exposed to hygrothermal and freeze-thaw environments. Compos. Struct. 2022, 293, 115719. [Google Scholar] [CrossRef]
- Yang, Y.; Silva, M.A.; Biscaia, H.; Chastre, C. Bond durability of CFRP laminates-to-steel joints subjected to freeze-thaw. Compos. Struct. 2019, 212, 243–258. [Google Scholar] [CrossRef]
- Li, C.; Xian, G. Mechanical property evolution and life prediction of carbon fiber and pultruded carbon fiber reinforced polymer plate exposed to elevated temperatures. Polym. Compos. 2020, 41, 5143–5155. [Google Scholar] [CrossRef]
- Nassif, H.; Habib, M.; Obeidah, A.; Abed, M. Restrained Shrinkage of High-Performance Ready-Mix Concrete Reinforced with Low Volume Fraction of Hybrid Fibers. Polymers 2022, 14, 4934. [Google Scholar] [CrossRef] [PubMed]
- Tamrazyan, A.; Alekseytsev, A.V. Optimization of reinforced concrete beams under local mechanical and corrosive damage. Eng. Optim. 2022, 54, 1–18. [Google Scholar] [CrossRef]
- Zheng, D.; Kou, J.; Wei, H.; Zhang, T.; Guo, H. Experimental study on flexural behavior of damaged concrete beams strengthened with high ductility concrete under repeated load. Eng. Struct. 2023, 274, 115203. [Google Scholar] [CrossRef]
- Hassanzadeh, A.M.; Nazarpour, H.; Dehestani, M. A probabilistic framework to model bond strength between concrete and GFRP rebar using Bayesian linear regression. In Structures; Elsevier: Amsterdam, The Netherlands, 2022; Volume 45, pp. 2173–2184. [Google Scholar] [CrossRef]
- Suhartono, H.A.; Rustianto, B. Estimating of measurement uncertainty of tensile strength test for deformed steel bars for concrete reinforcement. In AIP Conference Proceedings; AIP Publishing LLC: Melville, NY, USA, 2022; Volume 2664, p. 020006. [Google Scholar] [CrossRef]
- Sykora, M.; Krejsa, J.; Mlcoch, J.; Prieto, M.; Tanner, P. Uncertainty in shear resistance models of reinforced concrete beams according to fib MC2010. Struct. Concr. 2018, 19, 284–295. [Google Scholar] [CrossRef] [Green Version]
- Ibrahim, S.K.; Rad, M.M. Numerical Plastic Analysis of Non-Prismatic Reinforced Concrete Beams Strengthened by Carbon Fiber Reinforced Polymers. In Proceedings of the 13th fib International PhD Symposium in Civil Engineering, Marne-la-Vallée, Paris, France, 26–28 August 2020; pp. 208–215. [Google Scholar]
- Rad, M.M.; Ibrahim, S.K. Optimal plastic analysis and design of pile foundations under reliable conditions. Period. Polytech. Civ. Eng. 2021, 65, 761–767. [Google Scholar] [CrossRef]
- Kaliszky, S.; Lógó, J. Optimal plastic limit and shake-down design of bar structures with constraints on plastic deformation. Eng. Struct. 1997, 19, 19–27. [Google Scholar] [CrossRef]
- Kaliszky, S.; Logo, J. Optimal strengthening of elasto-plastic trusses with plastic deformation and stability constraints. Struct. Optim. 1999, 18, 296–299. [Google Scholar] [CrossRef]
- Lógó, J.; Rad, M.M.; Knabel, J.; Tauzowski, P. Reliability based design of frames with limited residual strain energy capacity. Period. Polytech. Civ. Eng. 2011, 55, 13–20. [Google Scholar] [CrossRef]
- Murzewski, J. Probability, Reliability and Statistical Methods in Engineering Design: A. Halder and S. Mahadevan, John Wiley & Sons, New York, 2000, xi+304 pp. Struct. Saf. 2001, 23, 93–102. [Google Scholar] [CrossRef]
- Haldar, A.; Mahadevan, S. Probability, Reliability, and Statistical Methods in Engineering Design; John Wiley & Sons: New York, NY, USA, 2000. [Google Scholar]
- BS EN 1990:2002, Eurocode—Basis of Structural Design. 2002. Available online: https://www.phd.eng.br/wp-content/uploads/2015/12/en.1990.2002.pdf (accessed on 20 November 2022).
- Systèmes, D. Abaqus Analysis User’s Guide Volume IV: Elements; Dassault Systèmes Simulia Corp.: Providence, RI, USA, 2018. [Google Scholar]


| E11 (MPa) | E22 (MPa) | Nu12 | G12 (MPa) | G13 (MPa) | G23 (MPa) |
|---|---|---|---|---|---|
| 140,000 | 10,000 | 0.26 | 5200 | 5200 | 3500 |
| Case | (N·mm) | (MPa) | (MPa) | F (kN) | u (mm) | ||
|---|---|---|---|---|---|---|---|
| Deterministic | C2-0 | 120 | - | 35 | 26,420 | 103 | 20 |
| C2-0-1 | 98 | 95 | 17.2 | ||||
| C2-0-2 | 27 | 80 | 14.4 | ||||
| Probabilistic | C2-1 | Randomly changed by 10% | 3.1 | Randomly changed by 5% | 95 | 17 | |
| C2-2 | 3.5 | 82 | 15 | ||||
| C2-3 | 4.8 | 79 | 14 | ||||
| Case | dt % | Tension Damage Pattern |
|---|---|---|
 | ||
| C2-0 | 31 |  |
| C2-1 | 29 |  |
| C2-2 | 28 | 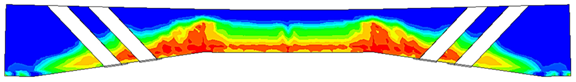 |
| C2-3 | 26 | 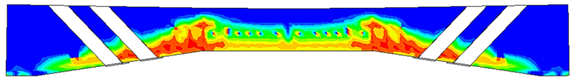 |
| Case | (N·mm) | (MPa) | (MPa) | F (kN) | u (mm) | ||
|---|---|---|---|---|---|---|---|
| Deterministic | C3-0 | 90 | - | 35 | 26,420 | 110 | 23 |
| C3-0-1 | 66 | 91 | 13 | ||||
| C3-0-2 | 21 | 80 | 11.3 | ||||
| Probabilistic | C3-1 | Randomly changed by 10% | 3.1 | Randomly changed by 5% | 104 | 21 | |
| C3-2 | 3.6 | 93 | 19 | ||||
| C3-3 | 4.9 | 82 | 16 | ||||
| Case | dt % | Tension Damage Pattern |
|---|---|---|
 | ||
| C3-0 | 27 | 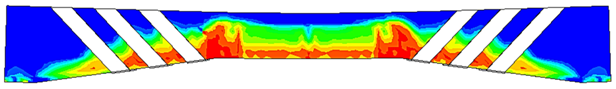 |
| C3-1 | 25 |  |
| C3-2 | 23 | 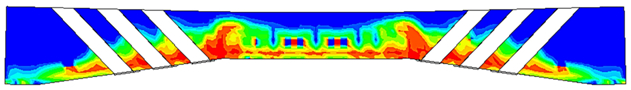 |
| C3-3 | 21 | 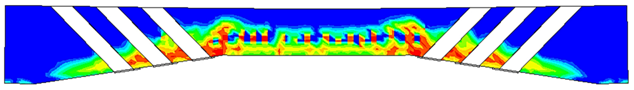 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Khaleel Ibrahim, S.; Movahedi Rad, M. Limited Optimal Plastic Behavior of RC Beams Strengthened by Carbon Fiber Polymers Using Reliability-Based Design. Polymers 2023, 15, 569. https://doi.org/10.3390/polym15030569
Khaleel Ibrahim S, Movahedi Rad M. Limited Optimal Plastic Behavior of RC Beams Strengthened by Carbon Fiber Polymers Using Reliability-Based Design. Polymers. 2023; 15(3):569. https://doi.org/10.3390/polym15030569
Chicago/Turabian StyleKhaleel Ibrahim, Sarah, and Majid Movahedi Rad. 2023. "Limited Optimal Plastic Behavior of RC Beams Strengthened by Carbon Fiber Polymers Using Reliability-Based Design" Polymers 15, no. 3: 569. https://doi.org/10.3390/polym15030569
APA StyleKhaleel Ibrahim, S., & Movahedi Rad, M. (2023). Limited Optimal Plastic Behavior of RC Beams Strengthened by Carbon Fiber Polymers Using Reliability-Based Design. Polymers, 15(3), 569. https://doi.org/10.3390/polym15030569







