Quantitative Evaluation of Thermal Ageing State of Cross-Linked Polyethylene Insulation Based on Polarization and Depolarization Current
Abstract
:1. Introduction
2. Basic Theory
2.1. Principle of PDC Method
2.2. Extended Debye Model
3. Experimental Design
3.1. Sample Preparation and Pre-treatment
3.2. Accelerated Thermal Ageing of XLPE
3.3. Scanning Electron Microscope(SEM) Test
3.4. PDC and Mechanical Measurement
4. Results and Analysis
4.1. SEM Analysis
4.2. Polarization and Depolarization Current
4.3. Trap Parameters of XLPE Samples
4.4. Conductivity
4.5. Elongation at Break Retention Rate (ER%)
4.6. Parameter Identification of Extended Debye Model
5. Quantitative Evaluation of Thermal Aging State from PDC
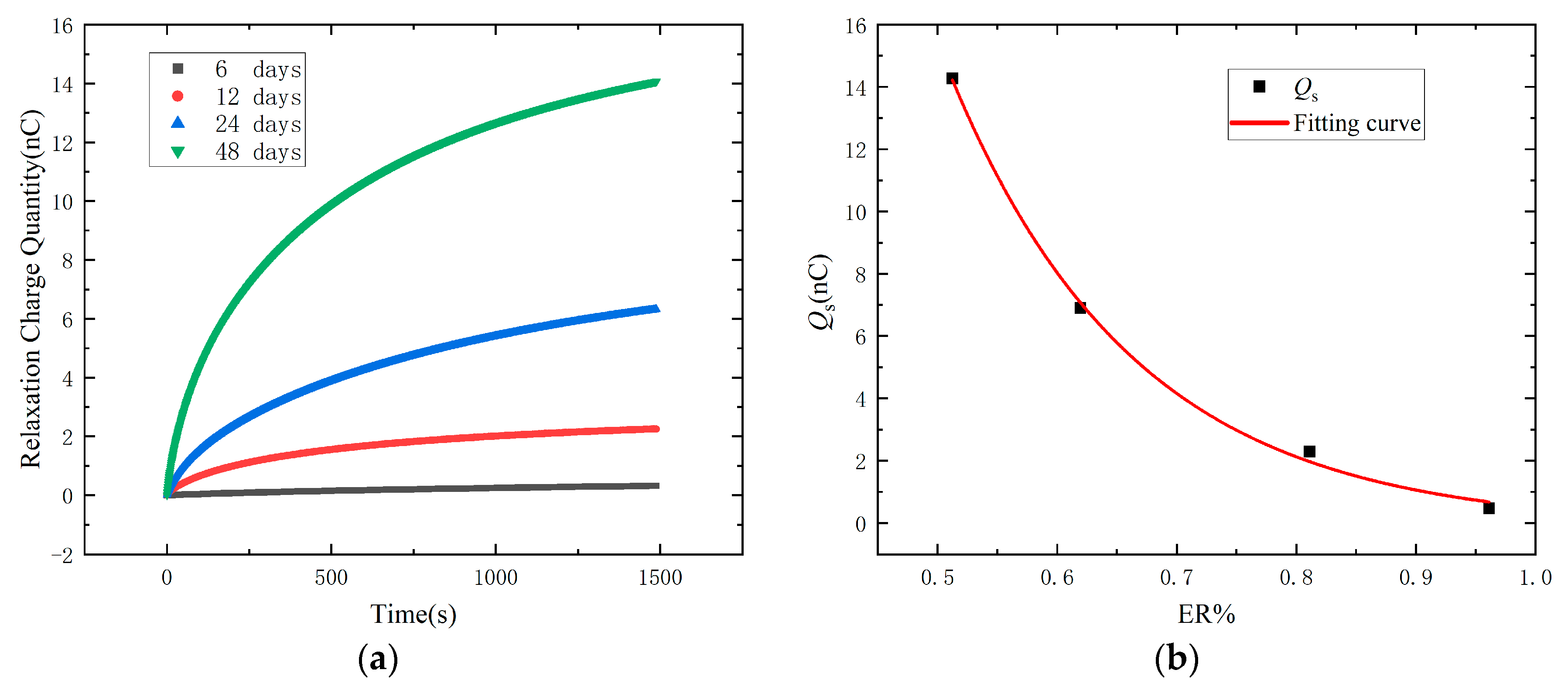
5.1. Time Domain Characteristic Parameter: Stable relaxation Charge Quantity (Qs)
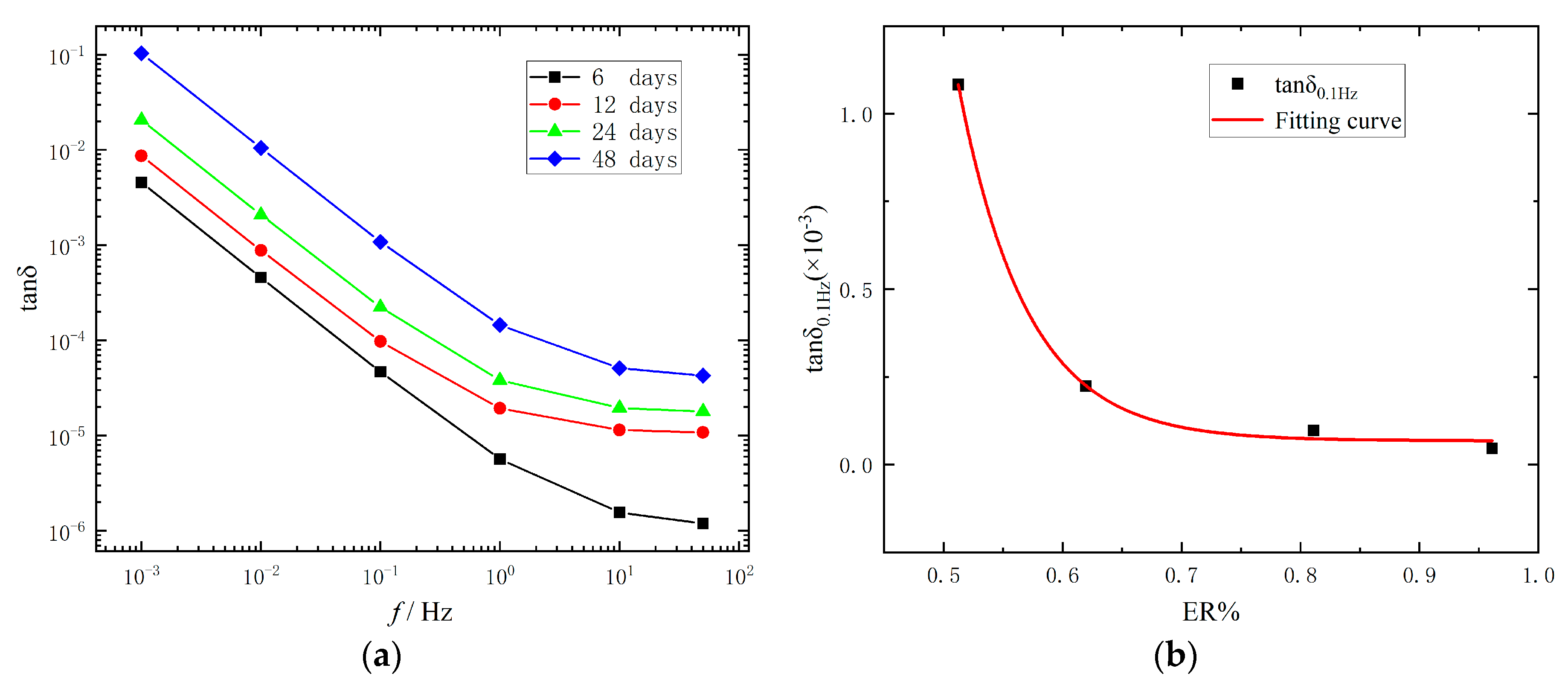
5.2. Frequency Domain Characteristic Parameter: Dissipation Factor at 0.1 Hz (tanδ0.1Hz)
6. Conclusions
- Based on the PDC measurement, both polarization current and depolarization current gradually increase with the thermal aging degree of the XLPE insulation, which has a good consistency in characterizing the deterioration of the XLPE insulation, and can make a preliminary assessment of the XLPE insulation state, along with ageing time and the conductivity increments.
- The depolarization current data at the different ageing duration are applied to plot the idep(t) × t vs. log10t curves which reflect the information about the changes of trap depth and density.
- The number of relaxation branches of the extended Debye model will increase with ageing time, indicating that new relaxation types have emerged. The resistance and capacitance of XLPE will also decrease with thermal ageing.
- ER% decreases with ageing time. It dwindles to 50.12% after 48 days ageing, which is close to the end of XLPE insulation life. The thermal ageing state can be determined by ER%.
- Relaxation charge quantity and tanδ calculated by PDC are increasing with the increase of ageing degree. Qs and tanδ0.1Hz proposed in this paper have a strong correlation with the ER%, which are expected to be a potential tool for quantitatively evaluating the ageing state.
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Ouyang, B.H.; Li, H.; Zhang, X.; Wang, S.H.; Li, J.Y. The role of micro-structure changes on space charge distribution of XLPE during thermo-oxidative ageing. IEEE Trans. Dielectr. Electr. Insul. 2017, 24, 3849–3859. [Google Scholar] [CrossRef]
- Nie, Y.J.; Zhao, X.P.; Li, S.T. Research progress in condition monitoring and insulation diagnosis of XLPE cable. High Volt. Eng. 2020, 46, 1361–1371. [Google Scholar]
- Ye, G.; Li, H.; Lin, F.C.; Tong, J.; Wu, X.G. Condition assessment of XLPE insulated cables based on polarization/depolarization current method. IEEE Trans. Dielectr. Electr. Insul. 2016, 23, 721–729. [Google Scholar] [CrossRef]
- Boukezzi, L.; Boubakeur, A. Characterization tests and analysis of cross-linked polyethylene (XLPE) used in high voltage cables insulation under thermal constraint. In Proceedings of the International Conference on Electrical Sciences and Technologies in Maghreb (CISTEM 2014), Tunis, Tunisia, 3–6 December 2014. [Google Scholar]
- Lv, H.K.; Lu, T.H.; Xiong, L.Q.; Zheng, X.G.; Huang, Y.Y.; Ying, M.L.; Cai, J.C.; Li, Z. Assessment of thermally aged XLPE insulation material under extreme operating temperatures. Polym. Test. 2020, 88, 106569. [Google Scholar] [CrossRef]
- Samee, A.; Li, Z.H.; Zhang, C.H.; Huang, Z.P. Investigation on electrical treeing characteristics of thermally aged XLPE cable. In Proceedings of the 9th International Conference on the Properties and Applications of Dielectric Materials, Harbin University of Science and Technology, Harbin, China, 19–23 July 2009. [Google Scholar]
- Kim, C.N.; Jin, Z.J.; Huang, X.Y. Investigation on water treeing behaviors of thermally aged XLPE cable insulation. Polym. Degrad. Stabil. 2007, 92, 537–544. [Google Scholar] [CrossRef]
- Bertini, G.J.; Chy, H. Rejuvenation addresses partial discharge in power cables. IEEE Trans. Dielectr. Electr. Insul. 2021, 28, 688–696. [Google Scholar] [CrossRef]
- Liu, Y.; Cao, X.L. Insulation performance evaluation of HV AC/DC XLPE cables by 0.1 Hz tan delta test on circumferentially peeled samples. IEEE Trans. Dielectr. Electr. Insul. 2017, 24, 3941–3950. [Google Scholar] [CrossRef]
- Kang, S.D.; Kim, J.H. Investigation on the insulation resistance characteristics of low voltage cable. Energies 2020, 13, 3611. [Google Scholar] [CrossRef]
- Lin, T.D.; Zhang, C.; Liu, H.; Liu, Q.; Yu, B.; Liu, Z.G. Insulation state evaluation of 110kV cable based on isothermal relaxation current. Adv. Technol. Electr. Eng. Energy 2020, 39, 43–52. [Google Scholar]
- Zhao, A.X.; Xu, L.; Zhang, X.; Deng, J.B.; Zhang, G.J.; Zhao, X.F. Research on aging parameters of XLPE cable based on isothermal relaxation current. AIP Adv. 2018, 8, 075323. [Google Scholar] [CrossRef] [Green Version]
- Morsalin, S.; Phung, B.T. Dielectric response study of service-aged XLPE cable based on polarisation and depolarisation current method. IEEE Trans. Dielectr. Electr. Insul. 2020, 27, 58–66. [Google Scholar] [CrossRef]
- Wolny, S. Aging degree evaluation for paper-oil insulation using the recovery voltage method. IEEE Trans. Dielectr. Electr. Insul. 2015, 22, 2455–2462. [Google Scholar] [CrossRef]
- Jahromi, A.N.; Pattabi, P.; Densley, J.; Lamarre, L. Medium voltage XLPE cable condition assessment using frequency domain spectroscopy. IEEE Elect. Insul. Mag. 2020, 36, 9–18. [Google Scholar] [CrossRef]
- Dai, X.Z.; Hao, J.; Jian, Z.; Gao, Z.; Zheng, X.L. Ageing state identification and analysis of AC 500 kV XLPE submarine cable based on high-voltage frequency dielectric response. IET Sci. Meas. Technol. 2020, 14, 977–984. [Google Scholar] [CrossRef]
- Leibfried, T.; Kachler, A.J. Insulation diagnostics on power transformers using the polarisation and depolarisation current (PDC) analysis. In Proceedings of the IEEE International Symposium on Electrical Insulation, Boston, MA, USA, 7–10 April 2002. [Google Scholar]
- Mishra, D.; Haque, N.; Baral, A. Assessment of interfacial charge accumulation in oil-paper interface in transformer insulation from polarization-depolarization current measurements. IEEE Trans. Dielectr. Electr. Insul. 2017, 24, 1665–1673. [Google Scholar] [CrossRef]
- Morsalin, S.; Phung, B.T. Modeling of dielectric dissipation factor measurement for XLPE cable based on Davidson-Cole model. IEEE Trans. Dielectr. Electr. Insul. 2019, 26, 1018–1026. [Google Scholar] [CrossRef]
- Morsalin, S.; Phung, B.T.; Danikas, M. Diagnostic challenges in dielectric loss assessment and interpretation: A review. IET Sci. Meas. Technol. 2019, 13, 767–782. [Google Scholar] [CrossRef]
- Li, H.; Li, J.Y.; Ma, Y.X.; Yan, Q.M. Effects of thermal aging on the crystal structures of the XLPE cable insulating material at different temperatures. Proc. CSEE 2017, 37, 6740–6748. [Google Scholar]
- Beigert, M.; Kranz, H.G. Destruction free ageing diagnosis of power cable insulation using the isothermal relaxation current analysis. In Proceedings of the 1994 IEEE International Symposium on Electrical Insulation, Pittsburgh, PA, USA, 5–8 June 1994. [Google Scholar]
- Morsalin, S.; Phung, B.T. Dielectric response measurement on service-aged XLPE cables: From very low frequency to power frequency. IEEE Elect. Insul. Mag. 2020, 36, 19–31. [Google Scholar] [CrossRef]
- Dai, X.Z.; Hao, J.; Liao, R.J.; Zheng, X.L.; Gao, Z.; Peng, W.L. Multi-dimensional analysis and correlation mechanism of thermal degradation characteristics of XLPE insulation for extra high voltage submarine cable. IEEE Trans. Dielectr. Electr. Insul. 2021, 28, 1488–1496. [Google Scholar] [CrossRef]
- Montanari, G.C.; Motori, A. Short-term thermal endurance characterization of polymeric cable insulating materials use of oxidative stability measurements. IEEE Trans. Dielectr. Electr. Insul. 1996, 3, 561–566. [Google Scholar] [CrossRef]
- Zhang, C.M.; Su, S.C.; Xie, M.; Zhou, K.; Zhao, S.L.; Xie, S.J. Parameter identification for dielectric response of XLPE power cable based on matrix pencil algorithm. High Volt. Eng. 2019, 45, 1647–1653. [Google Scholar]
- Zaengl, W.S. Dielectric spectroscopy in time and frequency domain for HV power equipment, Part I: Theoretical considerations. IEEE Elect. Insul. Mag. 2003, 19, 5–19. [Google Scholar] [CrossRef]
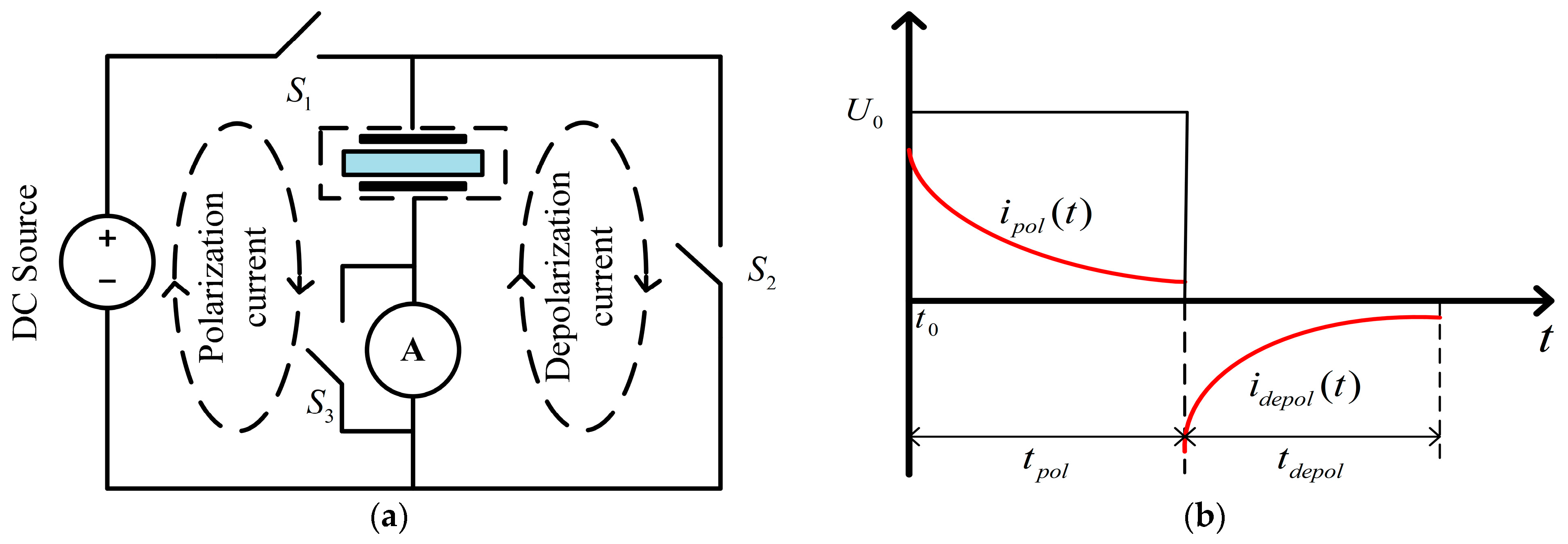
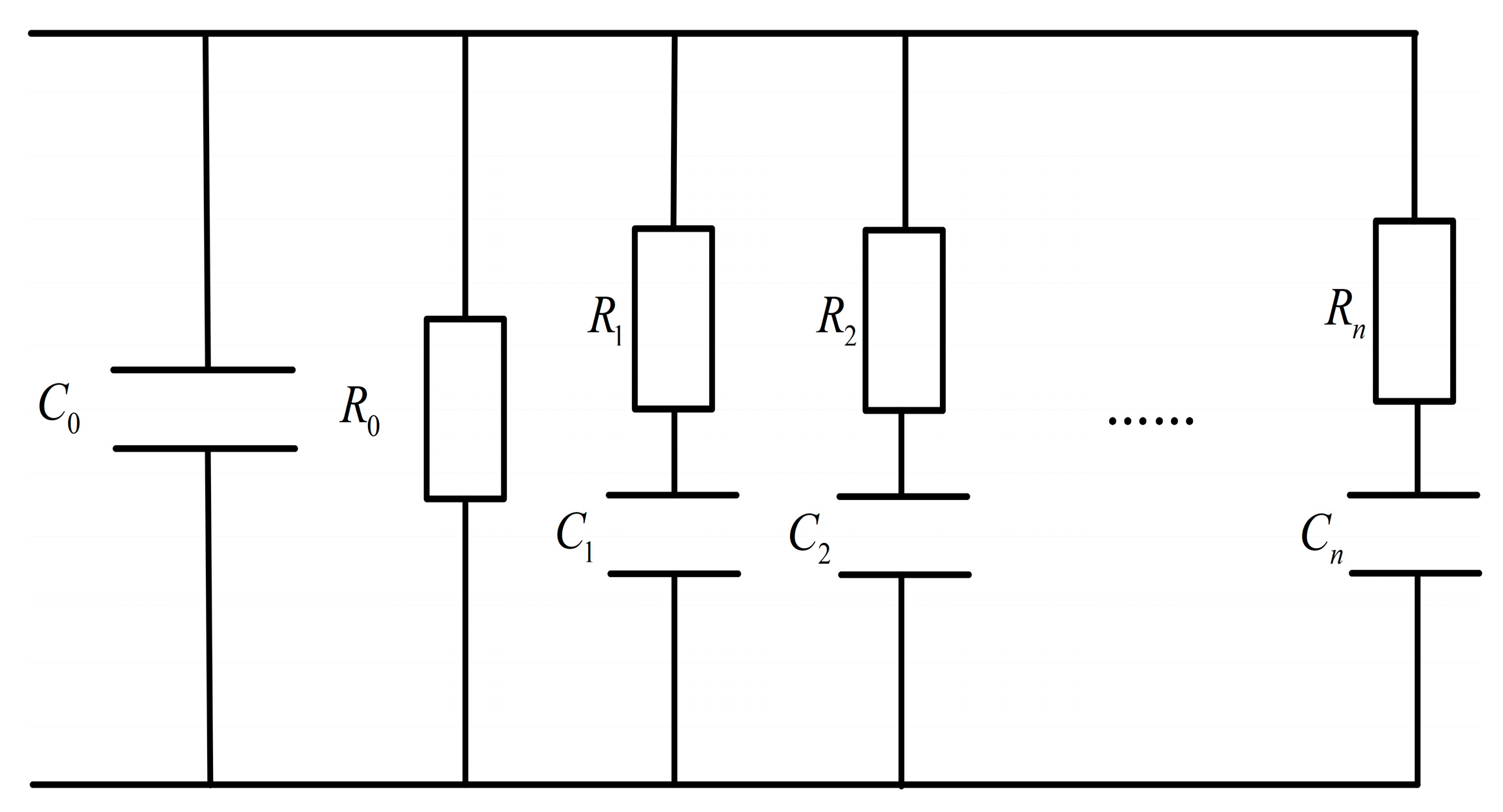




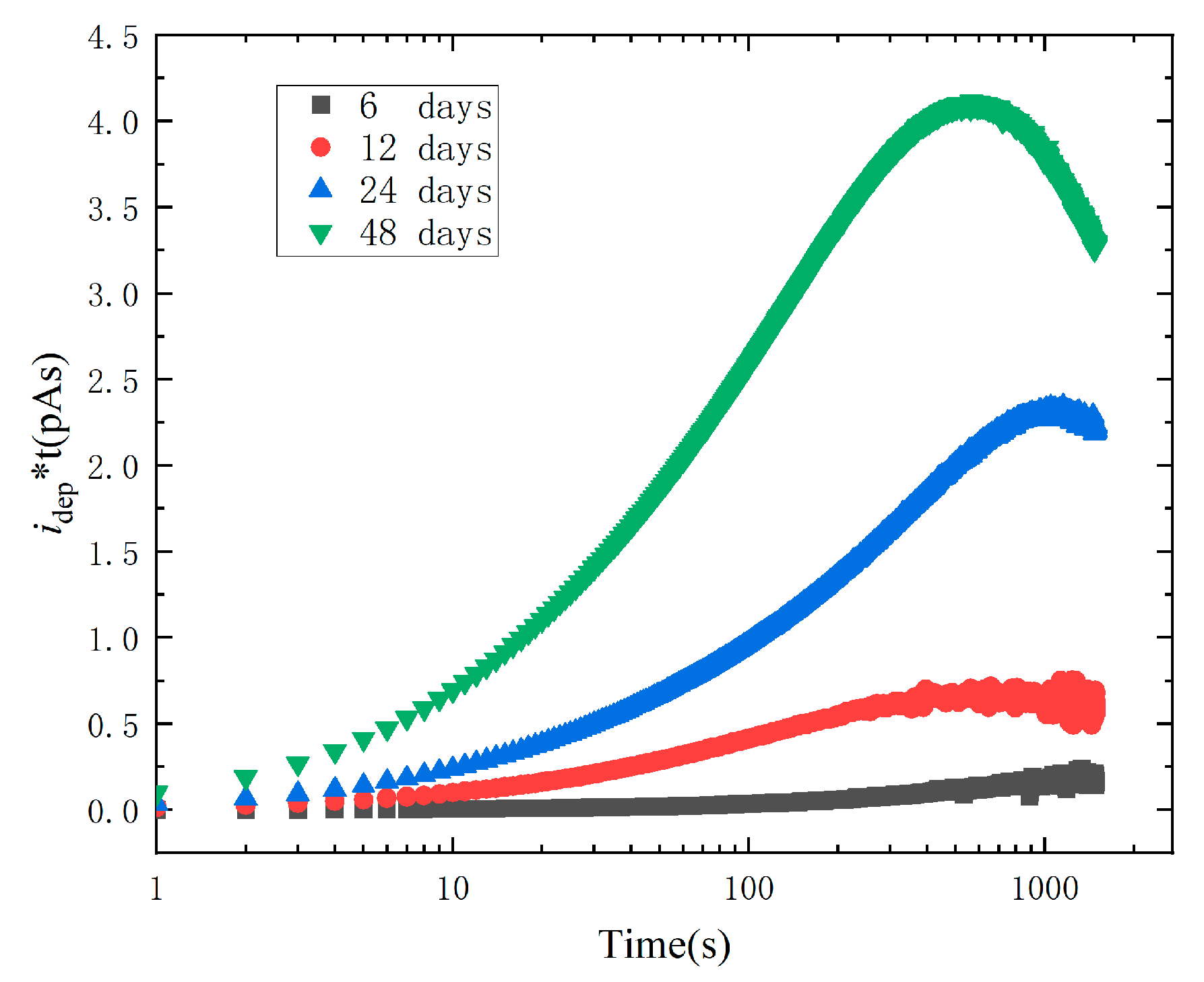
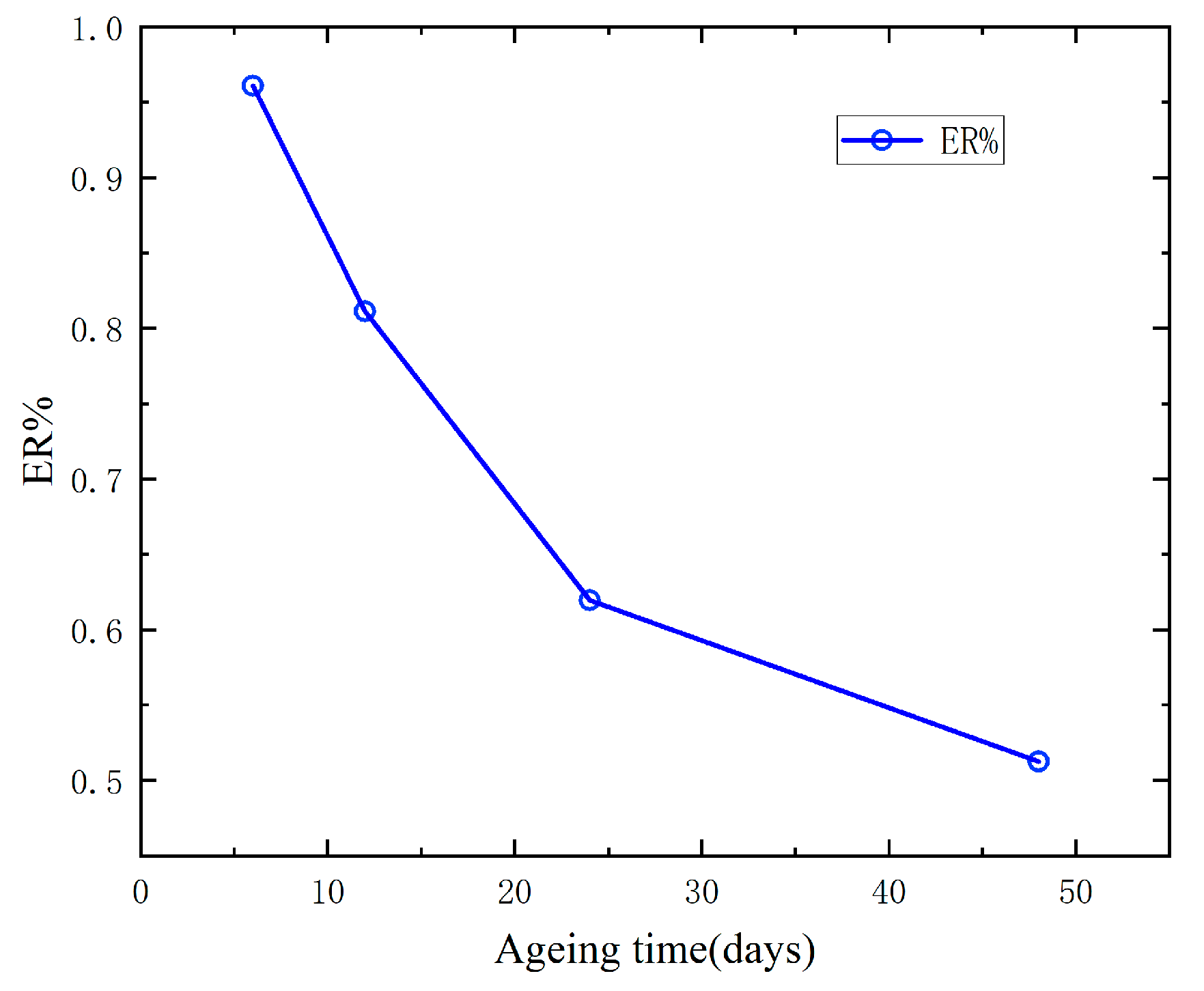
| Branch | Ai (A) | τi (s) | Ri (Ω) | Ci (F) |
|---|---|---|---|---|
| 1 | 2.50 × 10−13 | 45.80 | 8.01 × 1014 | 5.72 × 10−14 |
| 2 | 3.33 × 10−13 | 1418.13 | 3.92 × 1014 | 3.62 × 10−12 |
| Branch | Ai (A) | τi (s) | Ri (Ω) | Ci (F) |
|---|---|---|---|---|
| 1 | 6.59 × 10−12 | 7.99 | 3.03 × 1013 | 2.63 × 10−13 |
| 2 | 4.37 × 10−12 | 53.35 | 4.58 × 1013 | 1.17 × 10−12 |
| 3 | 3.48 × 10−12 | 252.10 | 5.73 × 1013 | 4.40 × 10−12 |
| 4 | 1.22 × 10−12 | 1328.03 | 1.11 × 1014 | 1.20 × 10−11 |
| Branch | Ai (A) | τi (s) | Ri (Ω) | Ci (F) |
|---|---|---|---|---|
| 1 | 7.12 × 10−12 | 3.87 | 2.81 × 1013 | 1.38 × 10−13 |
| 2 | 1.04 × 10−11 | 15.27 | 1.92 × 1013 | 7.94 × 10−13 |
| 3 | 9.37 × 10−12 | 55.99 | 2.13 × 1013 | 2.62 × 10−12 |
| 4 | 5.06 × 10−12 | 216.16 | 3.95 × 1013 | 5.47 × 10−12 |
| 5 | 5.41 × 10−12 | 1147.95 | 2.69 × 1013 | 4.26 × 10−11 |
| Branch | Ai (A) | τi (s) | Ri (Ω) | Ci (F) |
|---|---|---|---|---|
| 1 | 1.83 × 10−11 | 4.03 | 1.09 × 1013 | 3.69 × 10−13 |
| 2 | 3.23 × 10−11 | 15.45 | 6.19 × 1012 | 2.49 × 10−12 |
| 3 | 2.61 × 10−11 | 52.66 | 7.66 × 1012 | 6.87 × 10−12 |
| 4 | 1.95 × 10−11 | 211.73 | 1.02 × 1013 | 2.06 × 10−11 |
| 5 | 1.09 × 10−11 | 918.19 | 1.48 × 1013 | 6.22 × 10−11 |
| Ageing Time (Days) | Fitting Formula | R2 |
|---|---|---|
| 6 | 0.999 | |
| 12 | 0.993 | |
| 24 | 0.996 | |
| 48 | 0.993 |
| Ageing Time (Days) | Fitting Formula | R2 |
|---|---|---|
| Qs | 0.995 | |
| tanδ0.1Hz | 0.998 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Huang, P.; Yu, W.; Lu, C.; He, X.; Zhang, Y.; Liu, Y.; Zhou, J.; Liang, Y. Quantitative Evaluation of Thermal Ageing State of Cross-Linked Polyethylene Insulation Based on Polarization and Depolarization Current. Polymers 2023, 15, 1272. https://doi.org/10.3390/polym15051272
Huang P, Yu W, Lu C, He X, Zhang Y, Liu Y, Zhou J, Liang Y. Quantitative Evaluation of Thermal Ageing State of Cross-Linked Polyethylene Insulation Based on Polarization and Depolarization Current. Polymers. 2023; 15(5):1272. https://doi.org/10.3390/polym15051272
Chicago/Turabian StyleHuang, Ping, Wenyao Yu, Chunhao Lu, Xinghua He, Yiyi Zhang, Yansong Liu, Jiaheng Zhou, and Yuwang Liang. 2023. "Quantitative Evaluation of Thermal Ageing State of Cross-Linked Polyethylene Insulation Based on Polarization and Depolarization Current" Polymers 15, no. 5: 1272. https://doi.org/10.3390/polym15051272
APA StyleHuang, P., Yu, W., Lu, C., He, X., Zhang, Y., Liu, Y., Zhou, J., & Liang, Y. (2023). Quantitative Evaluation of Thermal Ageing State of Cross-Linked Polyethylene Insulation Based on Polarization and Depolarization Current. Polymers, 15(5), 1272. https://doi.org/10.3390/polym15051272







