Thermal Stability and Non-Isothermal Kinetic Analysis of Ethylene–Propylene–Diene Rubber Composite
Abstract
1. Introduction
2. Materials and Methods
2.1. Preparation of EPDM Composites
2.2. Thermogravimetric Analysis
3. Theoretical Approach
3.1. Models for Thermal Kinetic Analysis
3.2. Thermodynamic Analysis
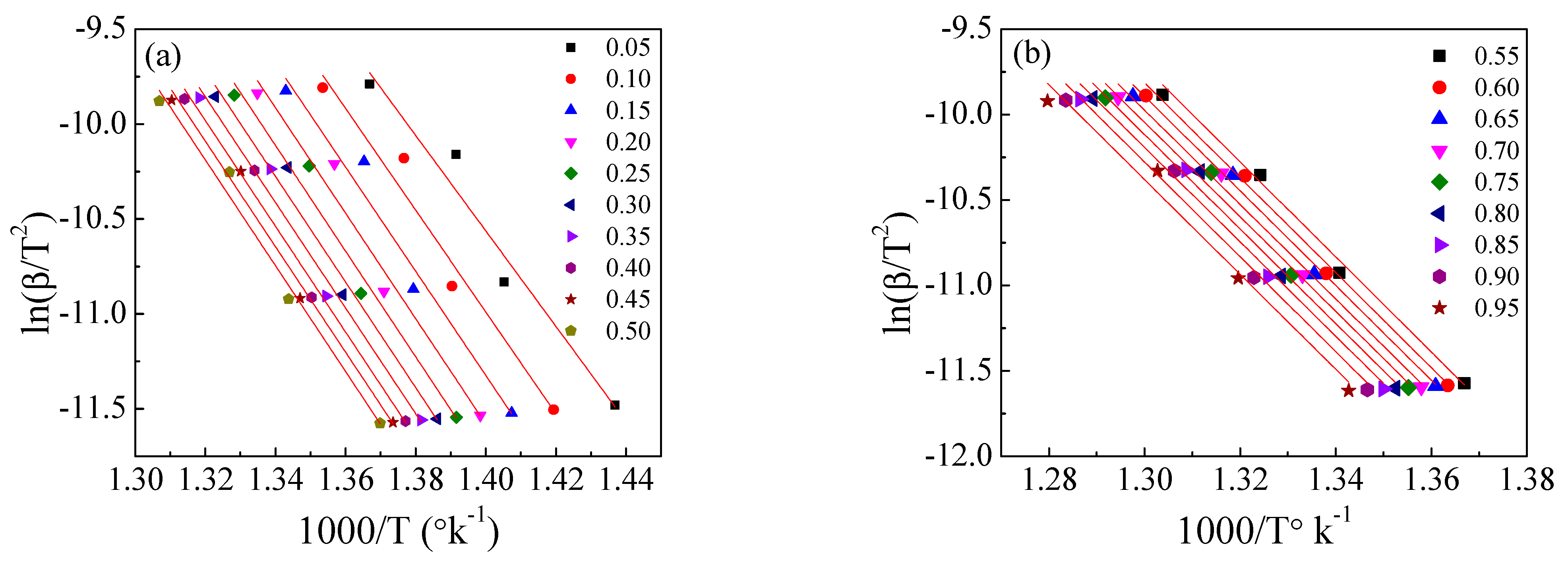
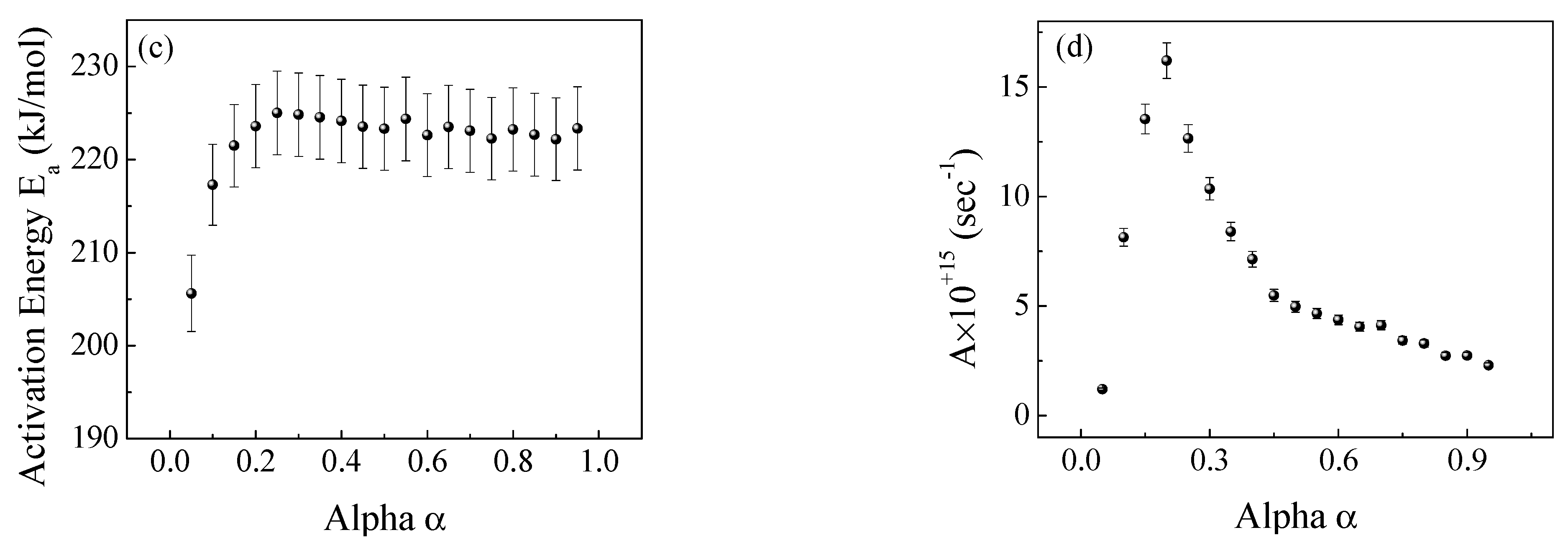
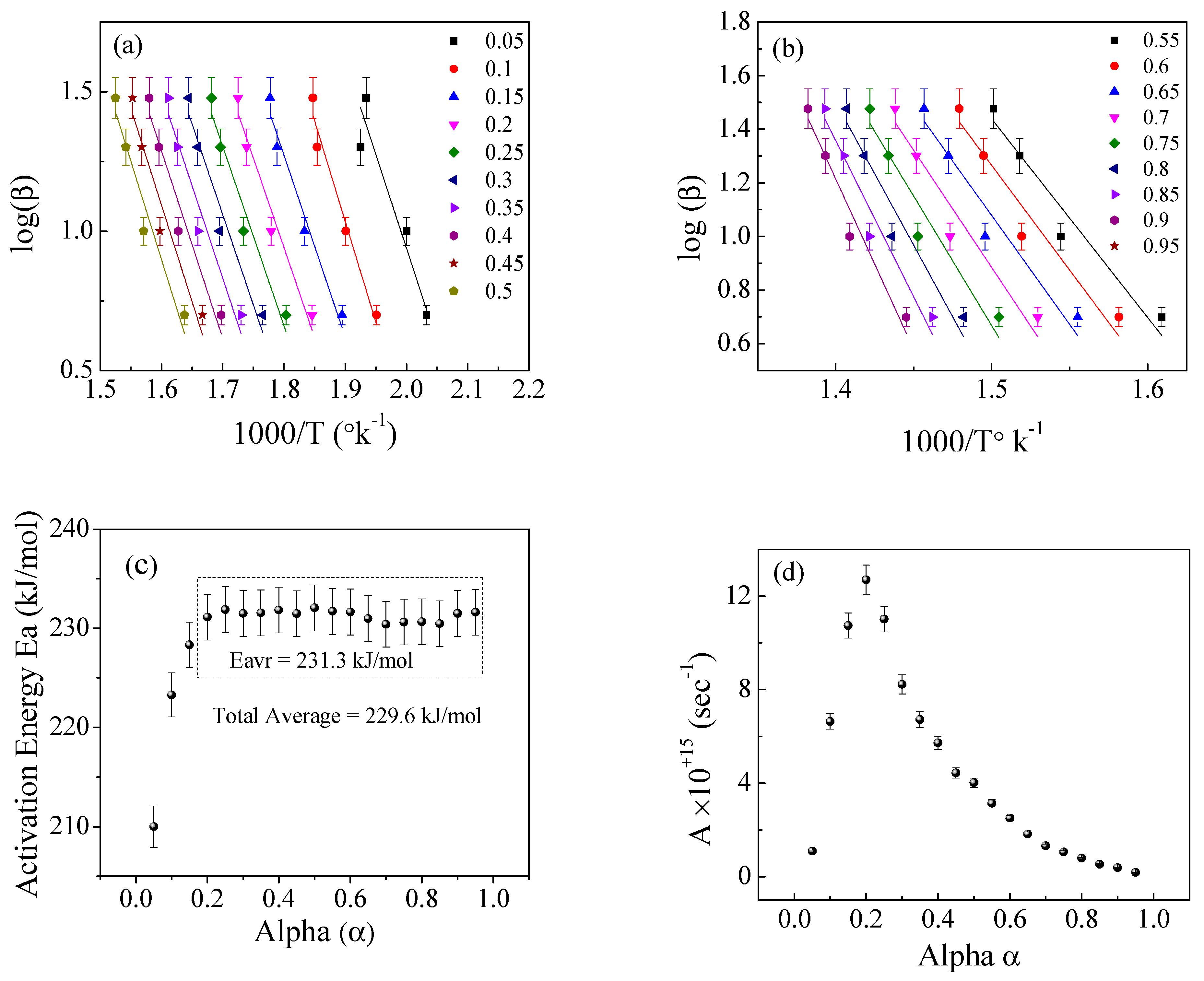
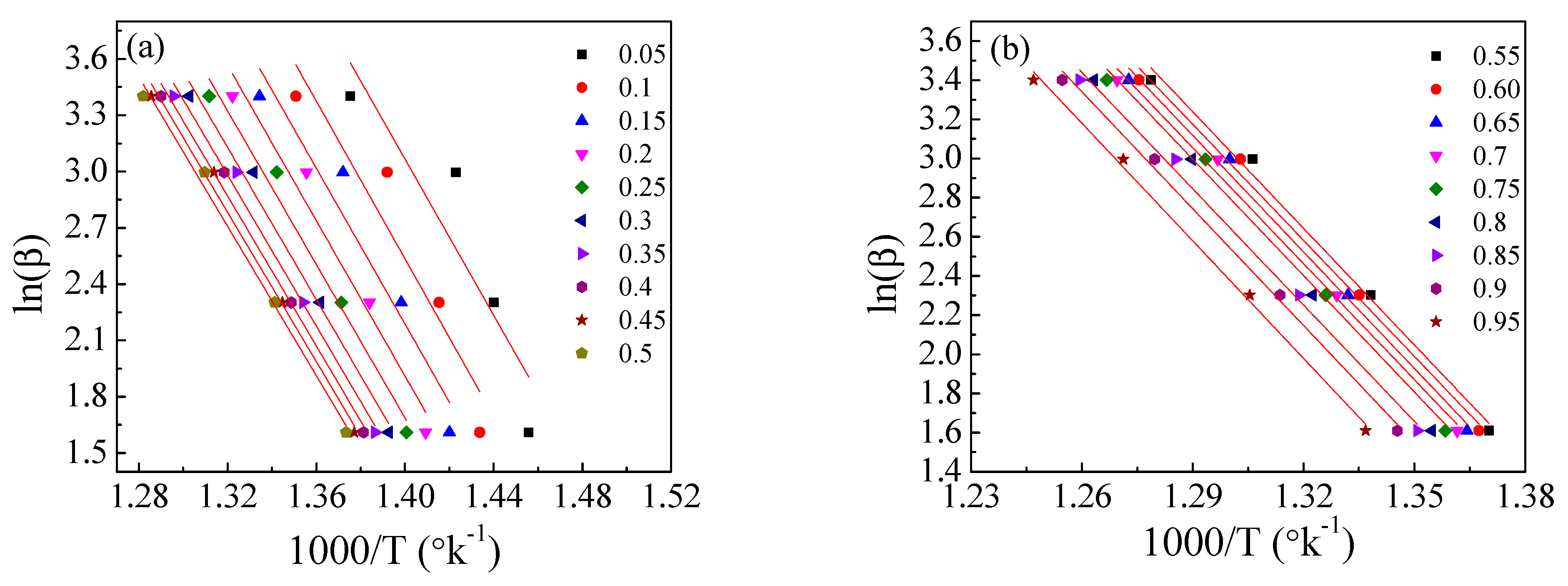
4. Results and Discussion
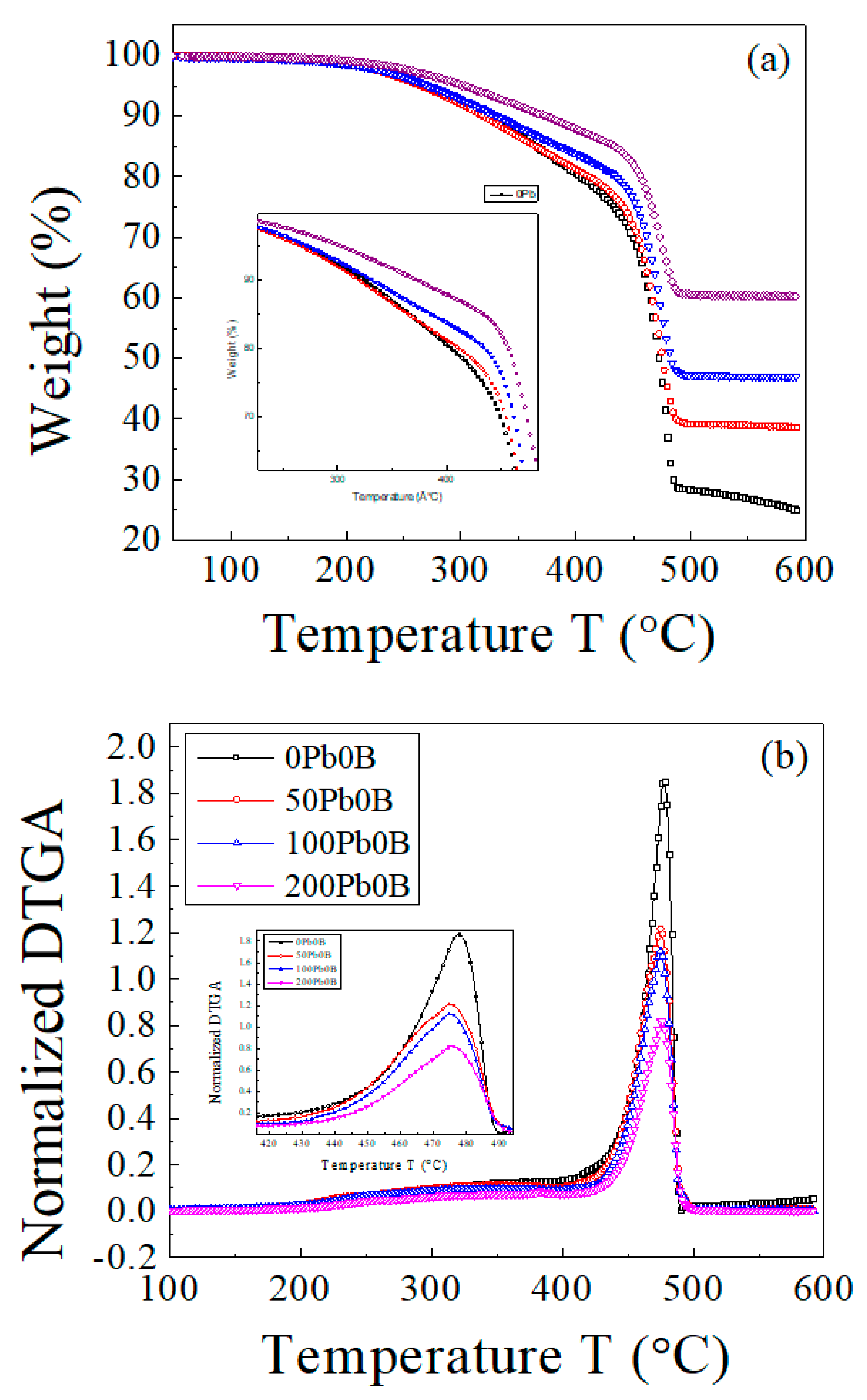
4.1. Thermogravimetric Analysis
- The effect of lead powder on the main decomposition peak (maximum decomposition rate) indicates that there was no chemical interaction between lead and EPDM. The variations in these peak positions are within the experimental error. Through previous research, we note that some additives may not affect position the maximum decomposition rate of EPDM rubber [37,38].
- Lead powder’s effect is evident between 200 and 400 °C, especially at higher concentrations.
- The decomposed mass at T > 250 °C decreased with increasing lead concentration. This can be attributed to the following: Lead particles absorb a large amount of thermal energy and, in turn, delay the decomposition of the host composite. During the preparation and vulcanization process, the lead increases the thermal homogeneity of the sample, leading to the formation of a homogenous network of cross-linked chains. There has been some evidence that conductive fillers impact EPDM’s thermal behavior, especially its thermal conductivity [39,40,41].
- As the lead powder concentration increased, the residual mass increased [42].
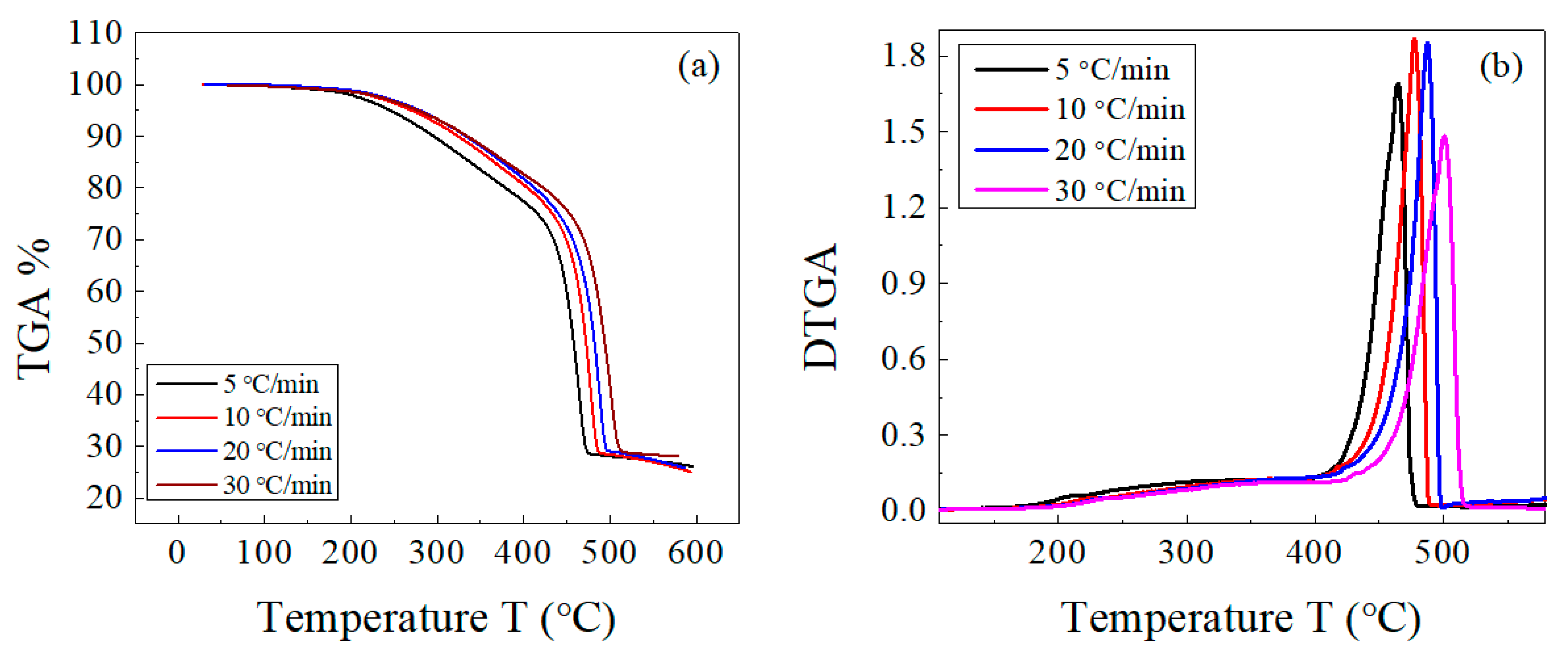
4.2. Kinetic Analysis
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Abdulrahman, S.T.; Patanair, B.; Vasukuttan, V.P.; Thomas, S.; Cadel, E.; Cuvilly, F.; Saiter-Fourcin, A.; Ahmad, Z.; Jaroszewski, M.; Strankowski, M. High-density polyethylene/EPDM rubber blend composites of boron compounds for neutron shielding application. Express Polym. Lett. 2022, 16, 558–572. [Google Scholar] [CrossRef]
- Barala, S.S.; Manda, V.; Jodha, A.S.; Meghwal, L.R.; Gopalani, D. Ethylene-propylene diene monomer-based polymer composite for attenuation of high energy radiations. J. Appl. Polym. Sci. 2021, 138, 50334. [Google Scholar] [CrossRef]
- Chang, B.; Li, S.; Li, M.; Chen, R. Research Progress of Neutron Shielding Materials. In Proceedings of the 29th International Conference on Nuclear Engineering, Shenzhen, China, 8–12 August 2022; p. V002T002A050. [Google Scholar]
- Barala, S.S.; Manda, V.; Jodha, A.S.; Gopalani, D. Thermal stability of gamma irradiated ethylene propylene diene monomer composites for shielding applications. J. Appl. Polym. Sci. 2022, 139, e52975. [Google Scholar] [CrossRef]
- Lu, S.; Li, B.; Ma, K.; Wang, S.; Liu, X.; Ma, Z.; Lin, L.; Zhou, G.; Zhang, D. Flexible MXene/EPDM rubber with excellent thermal conductivity and electromagnetic interference performance. Appl. Phys. A 2020, 126, 513. [Google Scholar] [CrossRef]
- Li, C.; Guo, J.; Xu, P.; Hu, W.; Lv, J.; Shi, B.; Zhang, Z.; Li, R. Facile preparation of superior compressibility and hydrophobic reduced graphene oxide@ cellulose nanocrystals/EPDM composites for highly efficient oil/organic solvent adsorption and enhanced electromagnetic interference shielding. Sep. Purif. Technol. 2023, 307, 122775. [Google Scholar] [CrossRef]
- Stelescu, M.D.; Airinei, A.; Manaila, E.; Fifere, N.; Craciun, G.; Varganici, C.; Doroftei, F. Exploring the effect of electron beam irradiation on the properties of some EPDM-flax fiber composites. Polym. Compos. 2019, 40, 315–327. [Google Scholar] [CrossRef]
- Güngör, A.; Akbay, I.; Özdemir, T. Waste walnut shell as an alternative bio-based filler for the EPDM: Mechanical, thermal, and kinetic studies. J. Mater. Cycles Waste Manag. 2019, 21, 145–155. [Google Scholar] [CrossRef]
- Güngör, A.; Akbay, İ.; Yaşar, D.; Özdemir, T. Flexible X/Gamma ray shielding composite material of EPDM rubber with bismuth trioxide: Mechanical, thermal investigations and attenuation tests. Prog. Nucl. Energy 2018, 106, 262–269. [Google Scholar] [CrossRef]
- Chen, G.; Gupta, A.; Mekonnen, T.H. Silane-modified wood fiber filled EPDM bio-composites with improved thermomechanical properties. Compos. Part A Appl. Sci. Manuf. 2022, 159, 107029. [Google Scholar] [CrossRef]
- Yao, W.; Xu, X.; Zhou, J.; Chen, M.; Meng, Q.; Wang, P.; Zhang, B.; Zhou, Y.; Ding, Y. Mechanically robust and flame-retarded EPDM composites with high loading of Mg(OH)2 based on reversible crosslinking network from Diels-Alder reactions. Polym. Degrad. Stab. 2022, 202, 110029. [Google Scholar] [CrossRef]
- Jung, J.K.; Lee, C.H.; Baek, U.B.; Choi, M.C.; Bae, J.W. Filler Influence on H2 Permeation Properties in Sulfur-CrossLinked Ethylene Propylene Diene Monomer Polymers Blended with Different Concentrations of Carbon Black and Silica Fillers. Polymers 2022, 14, 592. [Google Scholar] [CrossRef] [PubMed]
- Mayasari, H.E.; Wirapraja, A.Y. The Effect of Accelerator on Mechanical and Swelling Properties of NBR/EPDM/BIIR Composite. J. Teknol. Proses Dan Inov. Ind. 2021, 6, 7–11. [Google Scholar]
- Rahman Parathodika, A.; Raju, A.T.; Das, M.; Bhattacharya, A.B.; Neethirajan, J.; Naskar, K. Exploring hybrid vulcanization system in high-molecular weight EPDM rubber composites: A statistical approach. J. Appl. Polym. Sci. 2022, 139, e52721. [Google Scholar] [CrossRef]
- Rizwan, M.; Chandan, M.R. Mechanistic insights into the ageing of EPDM micro/hybrid composites for high voltage insulation application. Polym. Degrad. Stab. 2022, 204, 110114. [Google Scholar] [CrossRef]
- Lee, S.-H.; Park, S.-Y.; Chung, K.-H.; Jang, K.-S. Phlogopite-reinforced natural rubber (NR)/ethylene-propylene-diene monomer rubber (EPDM) composites with aminosilane compatibilizer. Polymers 2021, 13, 2318. [Google Scholar] [CrossRef]
- Zhang, X.; Li, J.; Chen, Z.; Pang, C.; He, S.; Lin, J. Study on thermal-oxidative aging properties of ethylene-propylene-diene monomer composites filled with silica and carbon nanotubes. Polymers 2022, 14, 1205. [Google Scholar] [CrossRef]
- Ashok, N.; Prakash, K.; Selvakumar, D.; Balachandran, M. Synergistic enhancement of mechanical, viscoelastic, transport, thermal, and radiation aging characteristics through chemically bonded interface in nanosilica reinforced EPDM-CIIR blends. J. Appl. Polym. Sci. 2021, 138, 50082. [Google Scholar] [CrossRef]
- Ashok, N.; Balachandran, M.; Lawrence, F.; Sebastian, N. EPDM–chlorobutyl rubber blends in γ-radiation and hydrocarbon environment: Mechanical, transport, and ageing behavior. J. Appl. Polym. Sci. 2017, 134, 45195. [Google Scholar] [CrossRef]
- Ashok, N.; Balachandran, M.; Lawrence, F. Organo-modified layered silicate nanocomposites of EPDM–chlorobutyl rubber blends for enhanced performance in γ radiation and hydrocarbon environment. J. Compos. Mater. 2018, 52, 3219–3231. [Google Scholar] [CrossRef]
- Ashok, N.; Webert, D.; Suneesh, P.; Balachandran, M. Mechanical and sorption behaviour of organo-modified montmorillonite nanocomposites based on EPDM–NBR Blends. Mater. Today Proc. 2018, 5, 16132–16140. [Google Scholar] [CrossRef]
- Güngör, A.; Akbay, I.; Özdemir, T. EPDM rubber with hexagonal boron nitride: A thermal neutron shielding composite. Radiat. Phys. Chem. 2019, 165, 108391. [Google Scholar] [CrossRef]
- Da Silva Sirqueira, A.; Guenther Soares, B. Kinetic analysis of thermal degradation of NR/EPDM blends: Effect of the reactive compatibilization. J. Appl. Polym. Sci. 2007, 103, 2669–2675. [Google Scholar] [CrossRef]
- Perejón, A.; Sánchez-Jiménez, P.E.; Gil-González, E.; Pérez-Maqueda, L.A.; Criado, J.M. Pyrolysis kinetics of ethylene–propylene (EPM) and ethylene–propylene–diene (EPDM). Polym. Degrad. Stab. 2013, 98, 1571–1577. [Google Scholar] [CrossRef]
- Kamath, V.A.; Kumaraswamy, S.; Kiran, K.U.; Somashekarappa, H.M. Optimization of PbO/Fe3O4/EPDM flexible composites for gamma shielding applications. In Proceedings of the International Conference on Physics of Materials and Nanotechnology 2019, Mangalore, India, 19–21 September 2019; p. 040004. [Google Scholar]
- El-Khatib, A.M.; Doma, A.; Badawi, M.S.; Abu-Rayan, A.E.; Aly, N.; Alzahrani, J.S.; Abbas, M.I. Conductive natural and waste rubbers composites-loaded with lead powder as environmental flexible gamma radiation shielding material. Mater. Res. Express 2020, 7, 105309. [Google Scholar] [CrossRef]
- Brown, M.E. Introduction to Thermal Analysis: Techniques and Applications; Springer: Berlin/Heidelberg, Germany, 2001. [Google Scholar]
- Kissinger, H.E. Reaction kinetics in differential thermal analysis. Anal. Chem. 1957, 29, 1702–1706. [Google Scholar] [CrossRef]
- Ozawa, T. A new method of analyzing thermogravimetric data. Bull. Chem. Soc. Jpn. 1965, 38, 1881–1886. [Google Scholar] [CrossRef]
- Ozawa, T. Kinetic analysis of derivative curves in thermal analysis. J. Therm. Anal. 1970, 2, 301–324. [Google Scholar] [CrossRef]
- Friedman, H.L. Kinetics of thermal degradation of char-forming plastics from thermogravimetry. Application to a phenolic plastic. In Journal of Polymer Science Part C: Polymer Symposia; Wiley Subscription Services, Inc., A Wiley Company: New York, NY, USA, 1964; pp. 183–195. [Google Scholar]
- Kim, Y.S.; Kim, Y.S.; Kim, S.H. Investigation of Thermodynamic Parameters in the Thermal Decomposition of Plastic Waste−Waste Lube Oil Compounds. Environ. Sci. Technol. 2010, 44, 5313–5317. [Google Scholar] [CrossRef]
- Singh, B.; Singh, S.; Kumar, P. In-depth analyses of kinetics, thermodynamics and solid reaction mechanism for pyrolysis of hazardous petroleum sludge based on isoconversional models for its energy potential. Process Saf. Environ. Prot. 2021, 146, 85–94. [Google Scholar] [CrossRef]
- Gamlin, C.; Dutta, N.; Roy Choudhury, N.; Kehoe, D.; Matisons, J. Influence of ethylene–propylene ratio on the thermal degradation behaviour of EPDM elastomers. Thermochim. Acta 2001, 367–368, 185–193. [Google Scholar] [CrossRef]
- Praveen, K.M.; Taleb, K.; Pillin, I.; Kervoelen, A.; Grohens, Y.; Thomas, S.; Haponiuk, J.T. Comparative mechanical, morphological, rheological, and thermal properties of polypropylene/ethylene-propylene-diene rubber blends. Polym. Adv. Technol. 2022, 33, 3296–3311. [Google Scholar] [CrossRef]
- Kim, G.; Sahu, P.; Oh, J.S. Physical Properties of Slide-Ring Material Reinforced Ethylene Propylene Diene Rubber Composites. Polymers 2022, 14, 2121. [Google Scholar] [CrossRef] [PubMed]
- Bianchi, M.; Valentini, F.; Fredi, G.; Dorigato, A.; Pegoretti, A. Thermo-Mechanical Behavior of Novel EPDM Foams Containing a Phase Change Material for Thermal Energy Storage Applications. Polymers 2022, 14, 4058. [Google Scholar] [CrossRef] [PubMed]
- Gschwind, L.; Jordan, C.S.; Vennemann, N.; Susoff, M.L. Mechanochemical devulcanization of EPDM rubber waste. Correlation of process parameters with sol–gel analyses and revulcanization properties. J. Appl. Polym. Sci. 2023, 140, e53768. [Google Scholar] [CrossRef]
- Ma, A.; Wang, X.; Chen, Y.; Yu, J.; Zheng, W.; Zhao, Y. Largely enhanced thermal conductivity of ethylene-propylene-diene monomer composites by addition of graphene ball. Compos. Commun. 2019, 13, 119–124. [Google Scholar] [CrossRef]
- Chen, Y.; Gao, S.; Liu, C.; Situ, Y.; Liu, J.; Huang, H. Preparation of PE-EPDM based phase change materials with great mechanical property, thermal conductivity and photo-thermal performance. Sol. Energy Mater. Sol. Cells 2019, 200, 109988. [Google Scholar] [CrossRef]
- Zarrinjooy Alvar, M.; Abdeali, G.; Bahramian, A.R. Influence of graphite nano powder on ethylene propylene diene monomer/paraffin wax phase change material composite: Shape stability and thermal applications. J. Energy Storage 2022, 52, 105065. [Google Scholar] [CrossRef]
- Brito, Z.; Sanchez, G. Influence of metallic fillers on the thermal and mechanical behaviour in composites of epoxy matrix. Compos. Struct. 2000, 48, 79–81. [Google Scholar] [CrossRef]
- Guo, M.; Li, J.; Xi, K.; Liu, Y.; Ji, J. Effect of multi-walled carbon nanotubes on thermal stability and ablation properties of EPDM insulation materials for solid rocket motors. Acta Astronaut. 2019, 159, 508–516. [Google Scholar] [CrossRef]
- Boswell, P. On the calculation of activation energies using a modified Kissinger method. J. Therm. Anal. Calorim. 1980, 18, 353–358. [Google Scholar] [CrossRef]
- Zhang, X. Applications of kinetic methods in thermal analysis: A review. Eng. Sci. 2020, 14, 1–13. [Google Scholar] [CrossRef]
- Arcenegui-Troya, J.; Sánchez-Jiménez, P.E.; Perejón, A.; Pérez-Maqueda, L.A. Determination of the activation energy under isothermal conditions: Revisited. J. Therm. Anal. Calorim. 2022, 148, 1679–1686. [Google Scholar] [CrossRef]
- Zaharescu, T.; Catauro, M.; Blanco, I. Kinetics of the Non-isothermal Degradation of POSS/EPDM Hybrids Composites. Macromol. Symp. 2021, 395, 2000221. [Google Scholar] [CrossRef]
- Açıkalın, K. Determination of kinetic triplet, thermal degradation behaviour and thermodynamic properties for pyrolysis of a lignocellulosic biomass. Bioresour. Technol. 2021, 337, 125438. [Google Scholar] [CrossRef]
- Marinović-Cincović, M.; Janković, B.; Jovanović, V.; Samaržija-Jovanović, S.; Marković, G. The kinetic and thermodynamic analyses of non-isothermal degradation process of acrylonitrile–butadiene and ethylene–propylene–diene rubbers. Compos. Part B Eng. 2013, 45, 321–332. [Google Scholar] [CrossRef]
- Prasopdee, T.; Smitthipong, W. Effect of Fillers on the Recovery of Rubber Foam: From Theory to Applications. Polymers 2020, 12, 2745. [Google Scholar] [CrossRef]







| Material | Role | Amount (phr) |
|---|---|---|
| EPDM | Rubber base | 100 phr |
| Carbon Black (N–220-(Degussa)) | Reinforcement (filler) | 50 phr |
| Paraffin Oil (ZMTH-Egypt) | Softener (plasticizer) | 50 phr |
| Stearic acid (Behn Mayer) | Activator | 2 phr |
| ZnO (Zink Egypt) | Accelerator | 5 phr |
| ZDMC * (Behn Mayer) | Accelerator | 3 phr |
| Sulfur (Polychem-egypt) | Curing agent | 3 phr |
| Lead powder (Pb-Indiamart) | Variable (50, 100, 200) |
| Sample | Tonset1 °C | Tonset2 °C | Tmax2 °C | M65% | m@450 °C (%) | m@550 °C (%) | Mres |
|---|---|---|---|---|---|---|---|
| EPDM0L | 239.4 | 455.8 | 477.5 | 458.0 | 69.9 | 27.0 | 24.6 |
| EPDM50L | 237.4 | 455.2 | 475.3 | 461 | 72.3 | 39.0 | 38.6 |
| EPDM100L | 235.4 | 453.7 | 475.0 | 467.8 | 76.5 | 47.1 | 47.0 |
| EPDM200L | 252.8 | 454.8 | 475.9 | 479.7 | 82.5 | 60.5 | 60.3 |
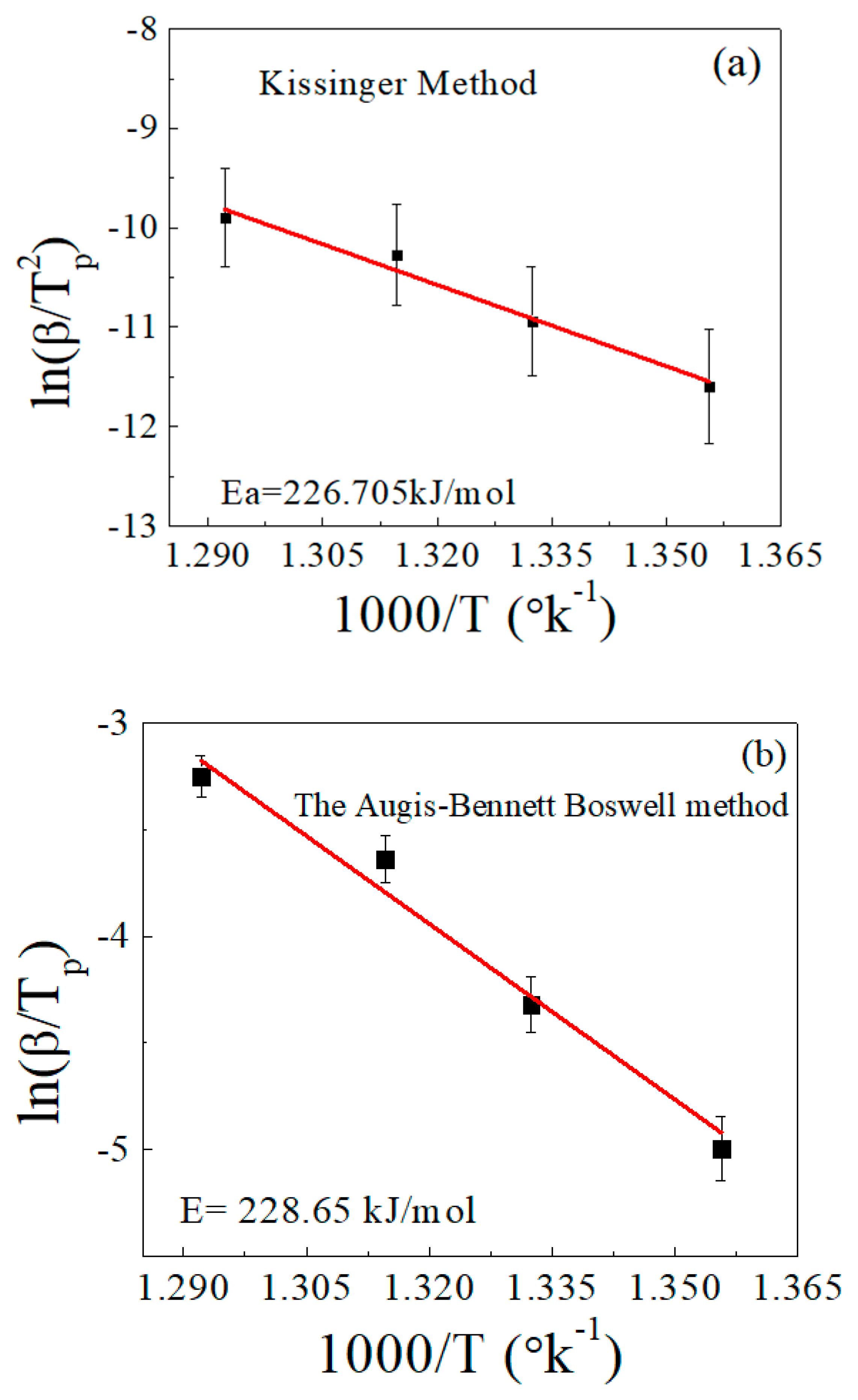
| Eavr (α from 0.05 to 0.95) | Eavr (α from 0.2 to 0.8) | |||
|---|---|---|---|---|
| Eavr (kJ/mol) | STD | Eavr (kJ/mol) | STD | |
| Kissinger | 226.7 | - | 226.7 | - |
| Augis–Bennett/Boswell | 228.7 | - | 228.7 | - |
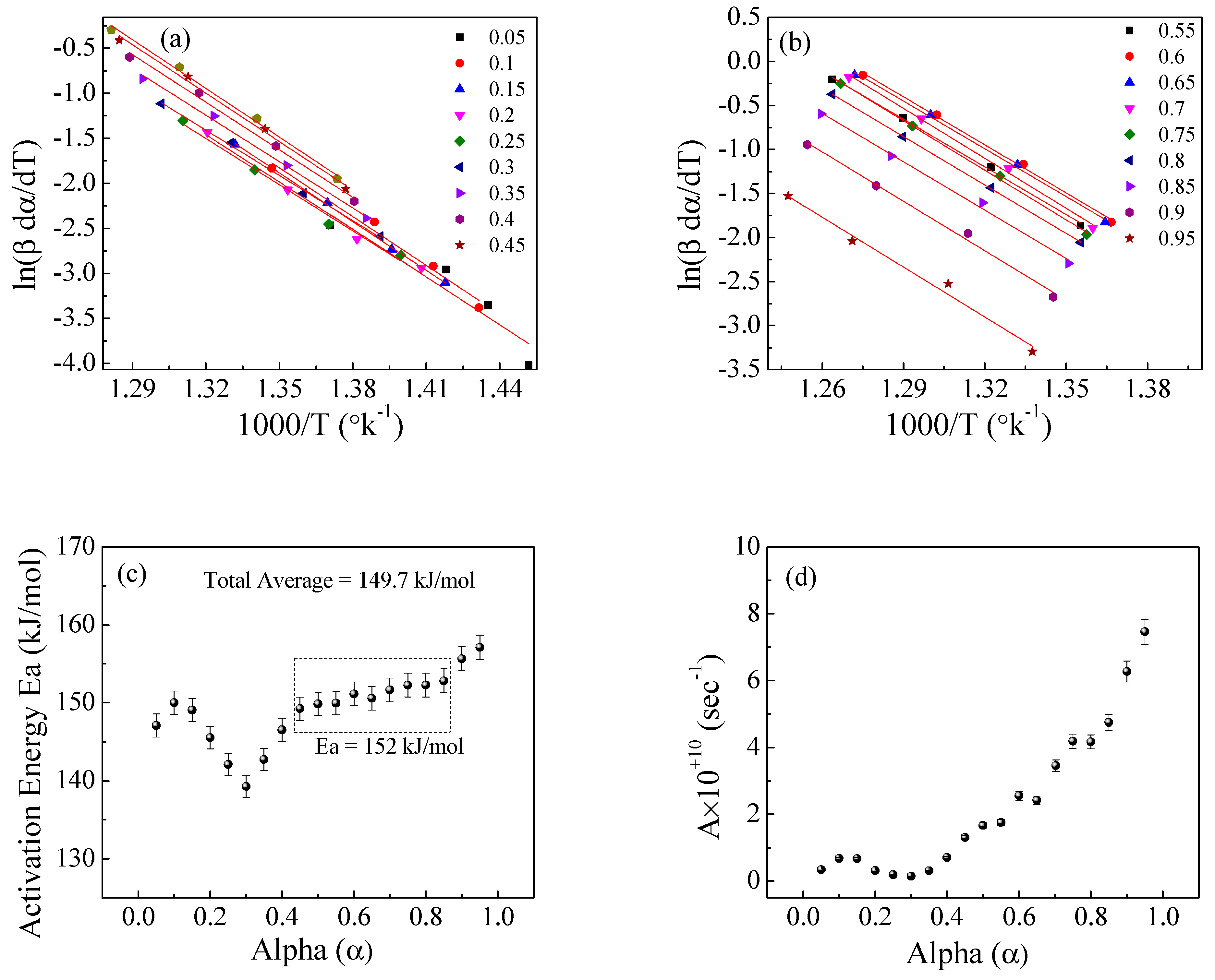
| KAS | 229.2 | 5.26 | 231.0 | 0.64 |
| FWO | 229.6 | 5.15 | 231.4 | 0.55 |
| Friedman | 231.0 | 8.20 | 227.3 | 3.31 |
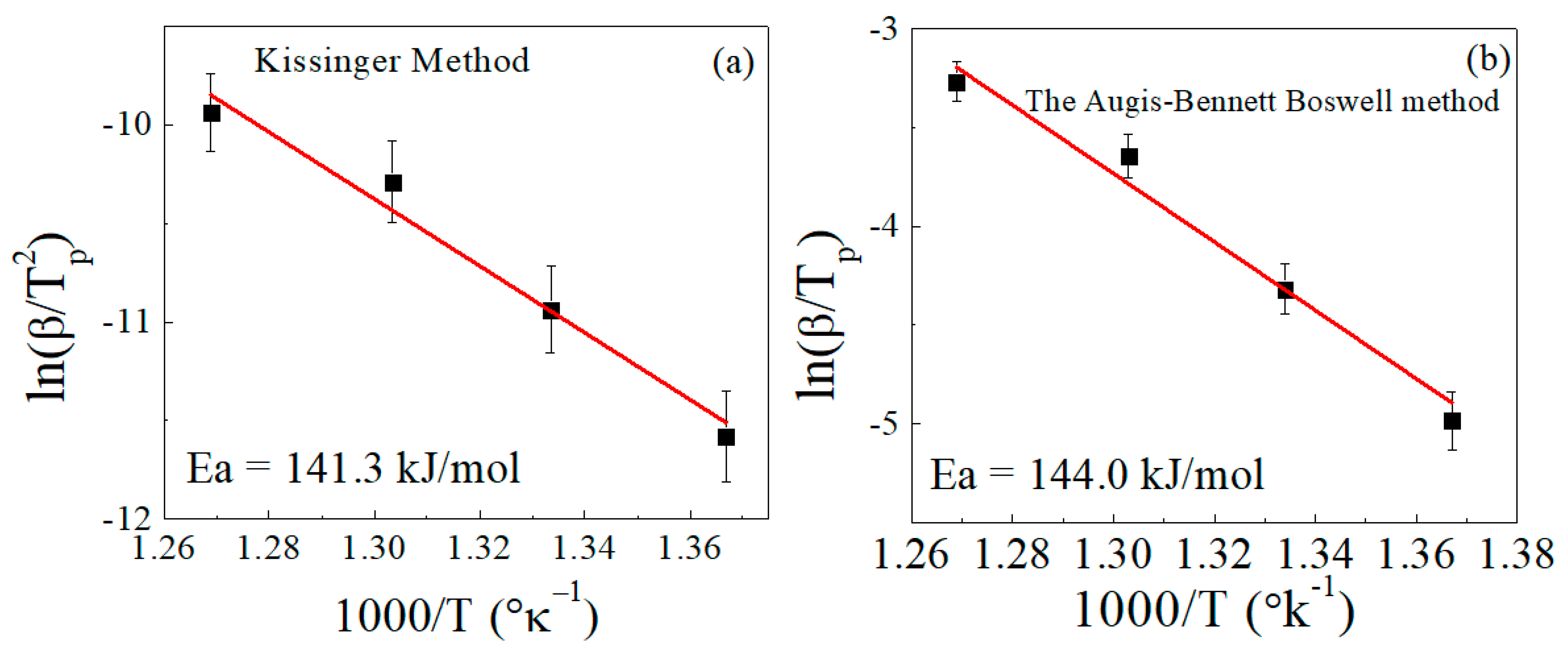
| Eavr (α from 0.05 to 0.95) | Eavr (α from 0.4 to 0.95) | |||
|---|---|---|---|---|
| Eavr (kJ/mol) | STD | Eavr (kJ/mol) | STD | |
| Kissinger | 141.3 | - | 141.3 | - |
| Augis–Bennett/Boswell | 144.0 | - | 144.0 | - |
| KAS | 155.0 | 3.6 | 152.7 | 0.5 |
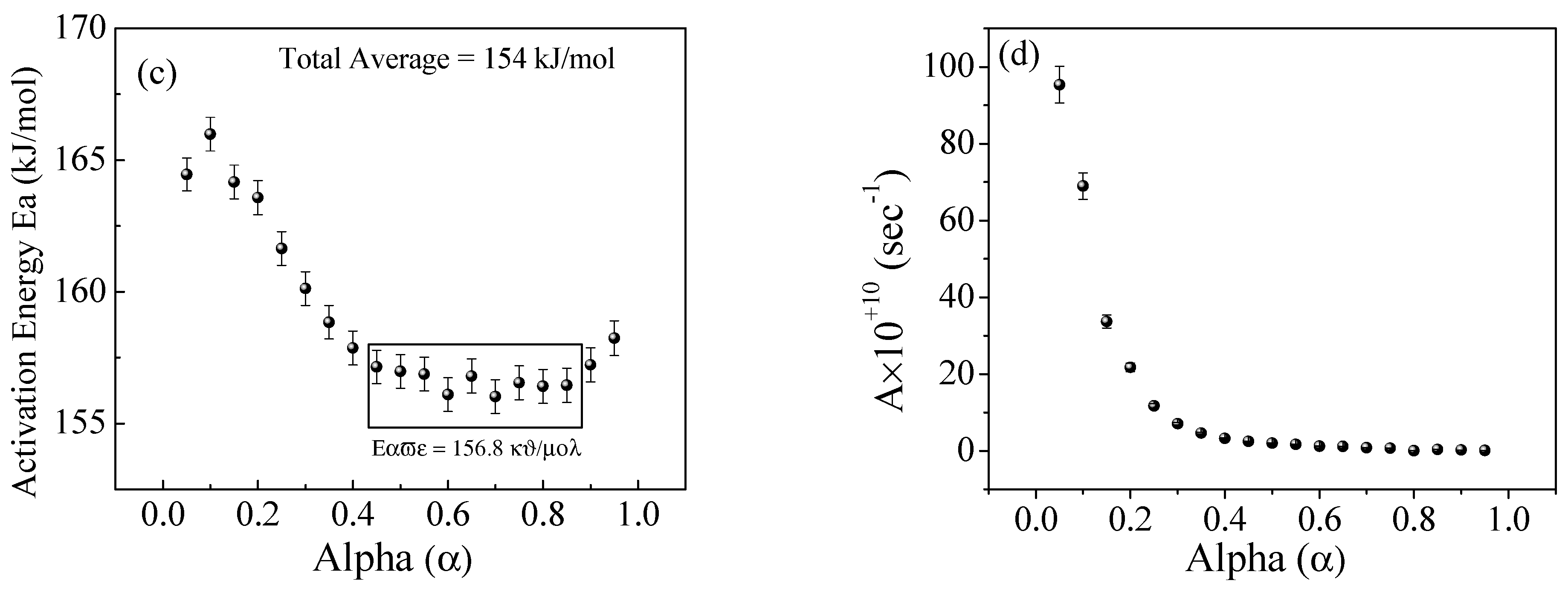
| FWO | 159.0 | 3.3 | 156.8 | 0.7 |
| Friedman | 149.2 | 4.5 | 151.6 | 2.8 |
| α | EPDM Host | EPDM/100 phr Lead | ||||
|---|---|---|---|---|---|---|
| Alpha | KAS | FWO | Freidman | KAS | FWO | Freidman |
| 0.05 | 209.07 | 210.00 | 245.46 | 161.54 | 164.46 | 147.10 |
| 0.10 | 222.91 | 223.29 | 240.06 | 161.46 | 165.98 | 150.00 |
| 0.15 | 228.12 | 228.34 | 237.75 | 161.27 | 164.16 | 149.08 |
| 0.20 | 231.00 | 231.15 | 235.96 | 160.56 | 163.57 | 145.56 |
| 0.25 | 231.71 | 231.88 | 231.38 | 158.89 | 161.64 | 142.11 |
| 0.30 | 231.29 | 231.52 | 229.67 | 156.50 | 160.13 | 139.29 |
| 0.35 | 231.30 | 231.57 | 226.89 | 154.53 | 158.85 | 142.74 |
| 0.40 | 231.56 | 231.86 | 227.00 | 154.18 | 157.87 | 146.55 |
| 0.45 | 231.14 | 231.49 | 226.23 | 152.99 | 157.15 | 149.24 |
| 0.50 | 231.72 | 232.08 | 225.46 | 152.91 | 156.97 | 149.86 |
| 0.55 | 231.33 | 231.73 | 225.24 | 152.51 | 156.87 | 149.97 |
| 0.60 | 231.21 | 231.65 | 225.28 | 152.51 | 156.09 | 151.14 |
| 0.65 | 230.49 | 230.99 | 224.04 | 152.84 | 156.80 | 150.56 |
| 0.70 | 229.87 | 230.43 | 224.87 | 152.36 | 156.02 | 151.65 |
| 0.75 | 230.06 | 230.63 | 225.21 | 152.85 | 156.55 | 152.28 |
| 0.80 | 230.08 | 230.67 | 227.12 | 152.49 | 156.41 | 152.28 |
| 0.85 | 229.86 | 230.48 | 226.39 | 152.31 | 156.45 | 152.81 |
| 0.90 | 230.89 | 231.50 | 230.01 | 152.90 | 157.23 | 155.64 |
| 0.95 | 230.98 | 231.62 | 254.20 | 152.88 | 158.24 | 157.10 |
| α | EPDM Host | EPDM/100 phr Lead | ||||
|---|---|---|---|---|---|---|
| Alpha | KAS | FWO | Freidman | KAS | FWO | Freidman |
| 0.05 | 1.20 × 1015 | 1.09 × 1015 | 6.73 × 1016 | 4.52 × 1011 | 9.53 × 1011 | 3.43 × 109 |
| 0.10 | 8.14 × 1015 | 6.64 × 1015 | 2.54 × 1016 | 2.58 × 1011 | 6.89 × 1011 | 6.74 × 109 |
| 0.15 | 1.35 × 1016 | 1.07 × 1016 | 1.77 × 1016 | 1.70 × 1011 | 3.37 × 1011 | 6.69 × 109 |
| 0.20 | 1.62 × 1016 | 1.27 × 1016 | 1.39 × 1016 | 1.08 × 1011 | 2.17 × 1011 | 3.16 × 109 |
| 0.25 | 1.27 × 1016 | 1.10 × 1016 | 7.05 × 1015 | 6.08 × 1010 | 1.17 × 1011 | 1.87 × 109 |
| 0.30 | 1.04 × 1016 | 8.22 × 1015 | 5.80 × 1015 | 3.16 × 1010 | 7.06 × 1010 | 1.40 × 109 |
| 0.35 | 8.40 × 1015 | 6.72 × 1015 | 4.00 × 1015 | 1.85 × 1010 | 4.60 × 1010 | 3.08 × 109 |
| 0.40 | 7.14 × 1015 | 5.73 × 1015 | 3.77 × 1015 | 1.45 × 1010 | 3.24 × 1010 | 7.06 × 109 |
| 0.45 | 5.48 × 1015 | 4.44 × 1015 | 4.03 × 1015 | 1.00 × 1010 | 2.43 × 1010 | 1.31 × 1010 |
| 0.50 | 4.97 × 1015 | 4.02 × 1015 | 4.77 × 1015 | 8.39 × 109 | 1.99 × 1010 | 1.67 × 1010 |
| 0.55 | 4.66 × 1015 | 3.14 × 1015 | 4.27 × 1015 | 6.62 × 109 | 1.65 × 1010 | 1.76 × 1010 |
| 0.60 | 4.37 × 1015 | 2.51 × 1015 | 4.58 × 1015 | 5.55 × 109 | 1.22 × 1010 | 2.56 × 1010 |
| 0.65 | 4.06 × 1015 | 1.83 × 1015 | 4.05 × 1015 | 4.86 × 109 | 1.13 × 1010 | 2.42 × 1010 |
| 0.70 | 4.12 × 1015 | 1.32 × 1015 | 5.53 × 1015 | 3.63 × 109 | 8.05 × 109 | 3.46 × 1010 |
| 0.75 | 3.43 × 1015 | 1.06 × 1015 | 6.65 × 1015 | 3.08 × 109 | 6.85 × 109 | 4.19 × 1010 |
| 0.80 | 3.29 × 1015 | 7.92 × 1014 | 1.03 × 1016 | 2.18 × 109 | 1.18E × 106 | 4.17 × 1010 |
| 0.85 | 2.73 × 1015 | 5.35 × 1014 | 1.07 × 1016 | 1.46 × 109 | 3.48 × 109 | 4.75 × 1010 |
| 0.90 | 2.74 × 1015 | 3.85 × 1014 | 2.25 × 1016 | 9.71 × 108 | 2.37 × 109 | 6.27 × 1010 |
| 0.95 | 2.30 × 1015 | 1.77 × 1014 | 2.29 × 1016 | 4.12 × 108 | 1.18 × 109 | 7.46 × 1010 |
| EPDM 100 Lead at Rate 10 °C/min | EPDM 0 phr Lead at Rate 10 °C/min | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| α | E | A | ΔH | ΔG | ΔS | E | A | ΔH | ΔG | ΔS |
| (kJ/mol) | (sec−1) | (kJ/mol) | (kJ/mol) | (J/mol) | (kJ/mol) | (sec−1) | (kJ/mol) | (kJ/mol) | (J/mol) | |
| 0.05 | 206 | 1.47 × 1012 | 199.7 | 220.4 | −27.6 | 162 | 3.51 × 109 | 155.8 | 211.6 | −77.5 |
| 0.1 | 217 | 1.01 × 1013 | 211.3 | 220.0 | −11.6 | 161 | 3.13 × 109 | 155.1 | 211.6 | −78.6 |
| 0.15 | 221 | 2.01 × 1013 | 215.5 | 219.9 | −5.9 | 161 | 3.20 × 109 | 155.1 | 211.6 | −78.5 |
| 0.2 | 224 | 2.84 × 1013 | 217.5 | 219.9 | −3.1 | 160 | 2.60 × 109 | 153.9 | 211.6 | −80.4 |
| 0.25 | 225 | 3.59 × 1013 | 218.9 | 219.8 | −1.2 | 159 | 2.10 × 109 | 152.6 | 211.7 | −82.2 |
| 0.3 | 225 | 3.49 × 1013 | 218.7 | 219.8 | −1.5 | 156 | 1.33 × 109 | 149.9 | 211.8 | −86.0 |
| 0.35 | 225 | 3.33 × 1013 | 218.4 | 219.8 | −1.9 | 155 | 1.01 × 109 | 148.3 | 211.8 | −88.4 |
| 0.4 | 224 | 3.12 × 1013 | 218.0 | 219.8 | −2.5 | 154 | 9.41 × 108 | 147.9 | 211.8 | −89.0 |
| 0.45 | 224 | 2.82 × 1013 | 217.4 | 219.9 | −3.3 | 153 | 7.72 × 108 | 146.7 | 211.9 | −90.7 |
| 0.5 | 223 | 2.72 × 1013 | 217.1 | 219.9 | −3.7 | 153 | 7.60 × 108 | 146.6 | 211.9 | −90.8 |
| 0.55 | 224 | 3.23 × 1013 | 218.2 | 219.8 | −2.2 | 153 | 7.62 × 108 | 146.6 | 211.9 | −90.8 |
| 0.6 | 223 | 2.43 × 1013 | 216.4 | 219.9 | −4.6 | 153 | 7.15 × 108 | 146.2 | 211.9 | −91.4 |
| 0.65 | 224 | 2.80 × 1013 | 217.3 | 219.9 | −3.4 | 153 | 7.20 × 108 | 146.3 | 211.9 | −91.3 |
| 0.7 | 223 | 2.61 × 1013 | 216.9 | 219.9 | −4.0 | 152 | 6.85 × 108 | 146.0 | 211.9 | −91.8 |
| 0.75 | 222 | 2.28 × 1013 | 216.0 | 219.9 | −5.2 | 153 | 7.50 × 108 | 146.5 | 211.9 | −91.0 |
| 0.8 | 223 | 2.68 × 1013 | 217.0 | 219.9 | −3.8 | 152 | 7.00 × 108 | 146.1 | 211.9 | −91.6 |
| 0.85 | 223 | 2.45 × 1013 | 216.4 | 219.9 | −4.6 | 152 | 6.77 × 108 | 145.8 | 211.9 | −91.9 |
| 0.9 | 222 | 2.26 × 1013 | 215.9 | 219.9 | −5.3 | 152 | 6.94 × 108 | 146.0 | 211.9 | −91.8 |
| 0.95 | 223 | 2.73 × 1013 | 217.0 | 219.9 | −3.8 | 153 | 7.22 × 108 | 155.8 | 211.6 | −77.5 |
| FWO | EPDM 0 phr Lead at Rate 10 °C/min | EPDM 100 phr Lead at Rate 10 °C/min | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| α | E | A | ΔH | ΔG | ΔS | E | A | ΔH | ΔG | ΔS |
| (kj/mol) | (sec−1) | (kJ/mol) | (kJ/mol) | (J/mol) | (kj/mol) | (sec−1) | (kJ/mol) | (kJ/mol) | (j/mol) | |
| 0.05 | 210.0 | 3.0 × 1012 | 204.1 | 220.3 | −21.5 | 164.5 | 5.74 × 109 | 158.7 | 211.4 | −73.5 |
| 0.1 | 223.3 | 2.7 × 1013 | 217.3 | 219.9 | −3.4 | 166.0 | 7.48 × 109 | 160.1 | 211.4 | −71.4 |
| 0.15 | 228.3 | 6.2 × 1013 | 222.3 | 219.7 | 3.4 | 164.2 | 5.46 × 109 | 158.2 | 211.5 | −74.1 |
| 0.2 | 231.1 | 9.9 × 1013 | 225.1 | 219.7 | 7.2 | 163.6 | 4.92 × 109 | 157.6 | 211.5 | −75.0 |
| 0.25 | 231.9 | 1.1 × 1014 | 225.8 | 219.6 | 8.2 | 161.6 | 3.52 × 109 | 155.6 | 211.6 | −77.9 |
| 0.3 | 231.5 | 1.0 × 1014 | 225.4 | 219.6 | 7.7 | 160.1 | 2.71 × 109 | 154.0 | 211.6 | −80.1 |
| 0.35 | 231.6 | 1.1 × 1014 | 225.4 | 219.6 | 7.7 | 158.8 | 2.17 × 109 | 152.7 | 211.7 | −82.0 |
| 0.4 | 231.9 | 1.1 × 1014 | 225.7 | 219.6 | 8.1 | 157.9 | 1.83 × 109 | 151.7 | 211.7 | −83.5 |
| 0.45 | 231.5 | 1.0 × 1014 | 225.3 | 219.6 | 7.5 | 157.1 | 1.61 × 109 | 151.0 | 211.7 | −84.5 |
| 0.5 | 232.1 | 1.1 × 1014 | 225.9 | 219.6 | 8.3 | 157.0 | 1.57 × 109 | 150.8 | 211.7 | −84.8 |
| 0.55 | 231.7 | 1.1 × 1014 | 225.5 | 219.6 | 7.8 | 156.9 | 1.54 × 109 | 150.7 | 211.7 | −85.0 |
| 0.6 | 231.7 | 1.1 × 1014 | 225.4 | 219.6 | 7.7 | 156.1 | 1.34 × 109 | 149.9 | 211.8 | −86.1 |
| 0.65 | 231.0 | 9.6 × 1013 | 224.8 | 219.7 | 6.8 | 156.8 | 1.52 × 109 | 150.6 | 211.7 | −85.1 |
| 0.7 | 230.4 | 8.8 × 1013 | 224.2 | 219.7 | 6.0 | 156.0 | 1.33 × 109 | 149.8 | 211.8 | −86.3 |
| 0.75 | 230.6 | 9.1 × 1013 | 224.4 | 219.7 | 6.3 | 156.5 | 1.45 × 109 | 150.3 | 211.7 | −85.5 |
| 0.8 | 230.7 | 9.1 × 1013 | 224.4 | 219.7 | 6.3 | 156.6 | 1.46 × 109 | 150.3 | 211.7 | −85.5 |
| 0.85 | 230.5 | 8.8 × 1013 | 224.2 | 219.7 | 6.0 | 156.5 | 1.43 × 109 | 150.2 | 211.7 | −85.7 |
| 0.9 | 231.5 | 1.0 × 1014 | 225.2 | 219.6 | 7.4 | 157.2 | 1.64 × 109 | 150.9 | 211.7 | −84.6 |
| 0.95 | 231.6 | 1.1 × 1014 | 225.3 | 219.6 | 7.6 | 158.2 | 1.95 × 109 | 151.9 | 211.7 | −83.2 |
| EPDM 0 phr Lead at Rate 10 °C/min | EPDM 100 phr Lead at Rate 10 °C/min | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| α | E | A | ΔH | ΔG | ΔS | E | A | ΔH | ΔG | ΔS |
| (kJ/mol) | (sec−1) | (kJ/mol) | (kJ/mol) | (J/mol) | (kJ/mol) | (sec−1) | (kJ/mol) | (kJ/mol) | (J/mol) | |
| 0.05 | 245.5 | 1.0 × 1015 | 239.5 | 219.3 | 27.0 | 147.1 | 2.8 × 108 | 141.3 | 212.1 | −98.5 |
| 0.1 | 240.1 | 4.3 × 1014 | 234.1 | 219.4 | 19.5 | 150.0 | 4.7 × 108 | 144.1 | 212.0 | −94.5 |
| 0.15 | 237.8 | 2.9 × 1014 | 231.7 | 219.5 | 16.3 | 149.1 | 4.0 × 108 | 143.1 | 212.0 | −95.9 |
| 0.2 | 236.0 | 2.2 × 1014 | 229.9 | 219.5 | 13.8 | 145.6 | 2.2 × 108 | 139.6 | 212.2 | −101.1 |
| 0.25 | 231.4 | 1.0 × 1014 | 225.3 | 219.6 | 7.5 | 142.1 | 1.2 × 108 | 136.0 | 212.3 | −106.1 |
| 0.3 | 229.7 | 7.7 × 1013 | 223.6 | 219.7 | 5.1 | 139.3 | 7.2 × 107 | 133.2 | 212.4 | −110.3 |
| 0.35 | 226.9 | 4.9 × 1013 | 220.7 | 219.8 | 1.3 | 142.7 | 1.3 × 108 | 136.6 | 212.3 | −105.3 |
| 0.4 | 228.5 | 6.4 × 1013 | 222.4 | 219.7 | 3.5 | 146.6 | 2.6 × 108 | 140.4 | 212.1 | −99.8 |
| 0.45 | 227.4 | 5.3 × 1013 | 221.2 | 219.8 | 2.0 | 149.2 | 4.1 × 108 | 143.1 | 212.0 | −96.0 |
| 0.5 | 229.8 | 7.9 × 1013 | 223.6 | 219.7 | 5.2 | 149.9 | 4.5 × 108 | 143.7 | 212.0 | −95.1 |
| 0.55 | 228.0 | 5.9 × 1013 | 221.8 | 219.7 | 2.8 | 150.0 | 4.6 × 108 | 143.8 | 212.0 | −95.0 |
| 0.6 | 225.3 | 3.8 × 1013 | 219.1 | 219.8 | −1.0 | 151.1 | 5.7 × 108 | 144.9 | 212.0 | −93.3 |
| 0.65 | 223.8 | 2.9 × 1013 | 217.5 | 219.9 | −3.1 | 150.6 | 5.1 × 108 | 144.3 | 212.0 | −94.1 |
| 0.7 | 224.9 | 3.5 × 1013 | 218.6 | 219.8 | −1.6 | 151.7 | 6.2 × 108 | 145.4 | 211.9 | −92.6 |
| 0.75 | 225.2 | 3.7 × 1013 | 219.0 | 219.8 | −1.1 | 152.3 | 6.9 × 108 | 146.0 | 211.9 | −91.7 |
| 0.8 | 227.1 | 5.1 × 1013 | 220.9 | 219.8 | 1.5 | 152.3 | 6.9 × 108 | 146.0 | 211.9 | −91.7 |
| 0.85 | 226.4 | 4.5 × 1013 | 220.1 | 219.8 | 0.4 | 152.8 | 7.6 × 108 | 146.5 | 211.9 | −91.0 |
| 0.9 | 230.0 | 8.2 × 1013 | 223.7 | 219.7 | 5.4 | 155.6 | 1.2 × 109 | 149.3 | 211.8 | −86.9 |
| 0.95 | 254.2 | 4.3 × 1015 | 247.9 | 219.1 | 38.4 | 157.1 | 1.6 × 109 | 150.7 | 211.7 | −84.9 |
| EPDM 0 phr Lead at Rate 10 °C/min | EPDM 100 phr Lead at Rate 10 °C/min | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| KAS | Max | 225.0 | 3.6 × 101³ | 218.9 | 220.4 | −1.2 | 162.0 | 3.5 × 109 | 155.8 | 211.9 | −77.5 |
| Min | 206.0 | 1.5 × 1012 | 199.7 | 219.8 | −27.6 | 152.0 | 6.8 × 108 | 145.8 | 211.6 | −91.9 | |
| Average | 222.2 | 2.6 × 101³ | 216.0 | 219.9 | −5.2 | 155.1 | 1.4 × 109 | 149.3 | 211.8 | −86.9 | |
| FWO | Max | 232.1 | 1.1 × 1014 | 225.9 | 220.3 | 8.3 | 166.0 | 7.5 × 109 | 160.1 | 211.8 | −71.4 |
| Min | 210.0 | 3.0 × 1012 | 204.1 | 219.6 | −21.5 | 156.0 | 1.3 × 109 | 149.8 | 211.4 | −86.3 | |
| Average | 229.6 | 9.0 × 101³ | 223.5 | 219.7 | 5.0 | 159.0 | 2.7 × 109 | 152.9 | 211.6 | −81.8 | |
| FM | Max | 254.2 | 4.3 × 1015 | 247.9 | 219.9 | 38.4 | 157.1 | 1.6 × 109 | 150.7 | 212.4 | −84.9 |
| Min | 223.8 | 2.9 × 101³ | 217.5 | 219.1 | −3.1 | 139.3 | 7.2 × 109 | 133.2 | 211.7 | −110.3 | |
| Average | 231.5 | 3.7 × 1014 | 225.3 | 219.7 | 7.5 | 149.2 | 5.2 × 109 | 143.1 | 212.0 | −96.0 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Alfannakh, H.; Alnaim, N.; Ibrahim, S.S. Thermal Stability and Non-Isothermal Kinetic Analysis of Ethylene–Propylene–Diene Rubber Composite. Polymers 2023, 15, 1890. https://doi.org/10.3390/polym15081890
Alfannakh H, Alnaim N, Ibrahim SS. Thermal Stability and Non-Isothermal Kinetic Analysis of Ethylene–Propylene–Diene Rubber Composite. Polymers. 2023; 15(8):1890. https://doi.org/10.3390/polym15081890
Chicago/Turabian StyleAlfannakh, Huda, Nisrin Alnaim, and Sobhy S. Ibrahim. 2023. "Thermal Stability and Non-Isothermal Kinetic Analysis of Ethylene–Propylene–Diene Rubber Composite" Polymers 15, no. 8: 1890. https://doi.org/10.3390/polym15081890
APA StyleAlfannakh, H., Alnaim, N., & Ibrahim, S. S. (2023). Thermal Stability and Non-Isothermal Kinetic Analysis of Ethylene–Propylene–Diene Rubber Composite. Polymers, 15(8), 1890. https://doi.org/10.3390/polym15081890






