Three-Dimensional Stress Fields in Thick Orthotropic Plates with Sharply Curved Notches under In-Plane and Out-of-Plane Shear
Abstract
:1. Introduction
- To provide evidence that the 3D solution derived by Zappalorto and Carraro [19] for pointed notches can be extended also to orthotropic plates with holes or lateral radiused notches with any notch opening angle, under the hypothesis of a sufficiently small notch tip radius;
- To show that, on the basis of the plane solution, stress components σxx, σyy and τxy in a thick 3D anisotropic notched plate (i.e., the in-plane stress fields) can be accurately determined, whereas out-of-plane shear stresses, and , can be assessed using the pure antiplane shear solution;
- To also document the presence of coupled modes for orthotropic thick plates weakened by holes or lateral notches with any notch opening angle, as reported in other research articles concerning isotropic components.
2. Simplified Three-Dimensional Elasticity Theory for Orthotropic Thick Plates
2.1. Basic Field Equation
2.2. Solution for the Quasi-Biharmonic Equation (In-Plane Stress Field Components and Out-of-Plane Normal Stress σzz)
2.3. Solution for the Quasi-Harmonic Equation (Out-of-Plane Shear Stresses)
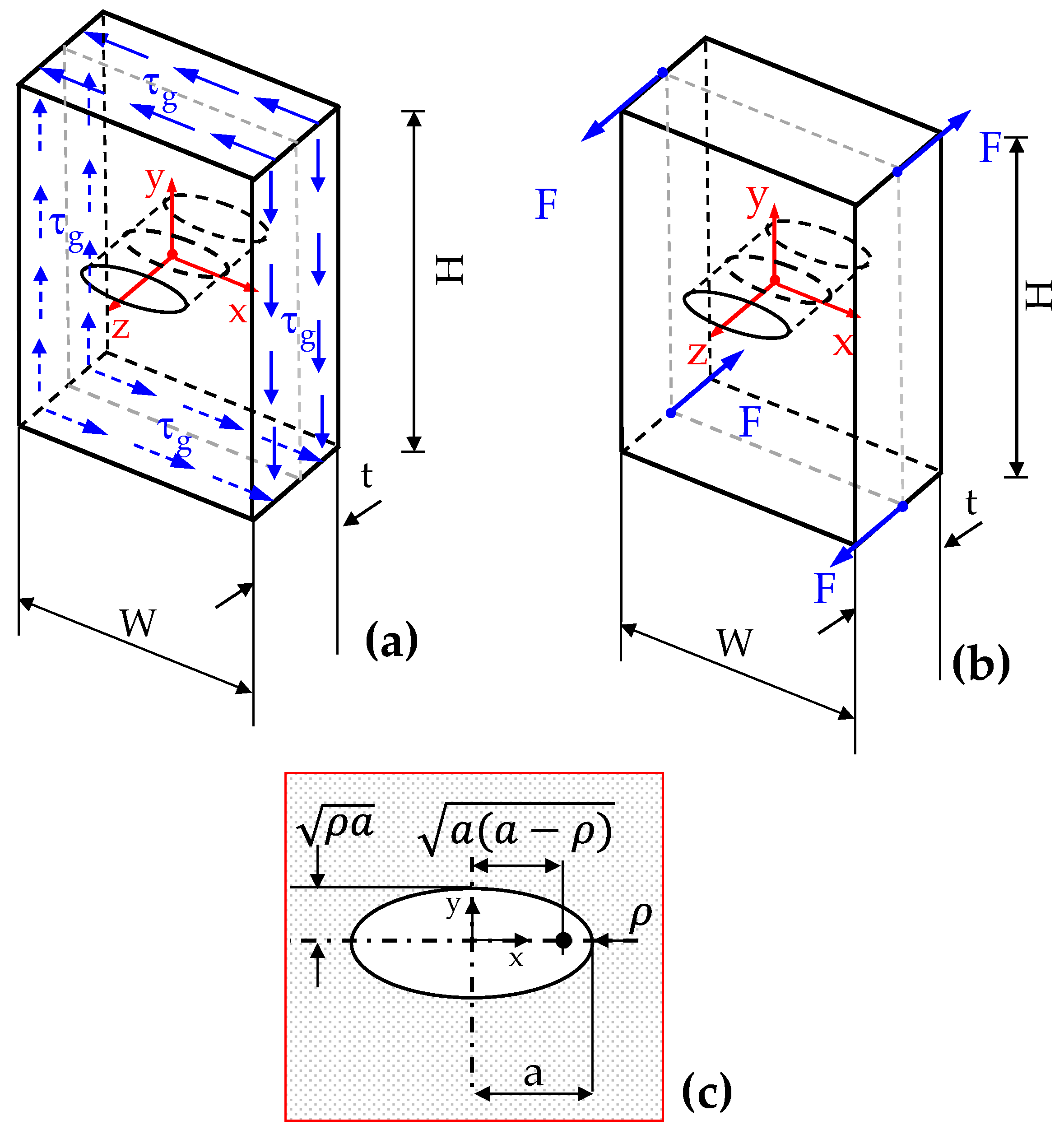
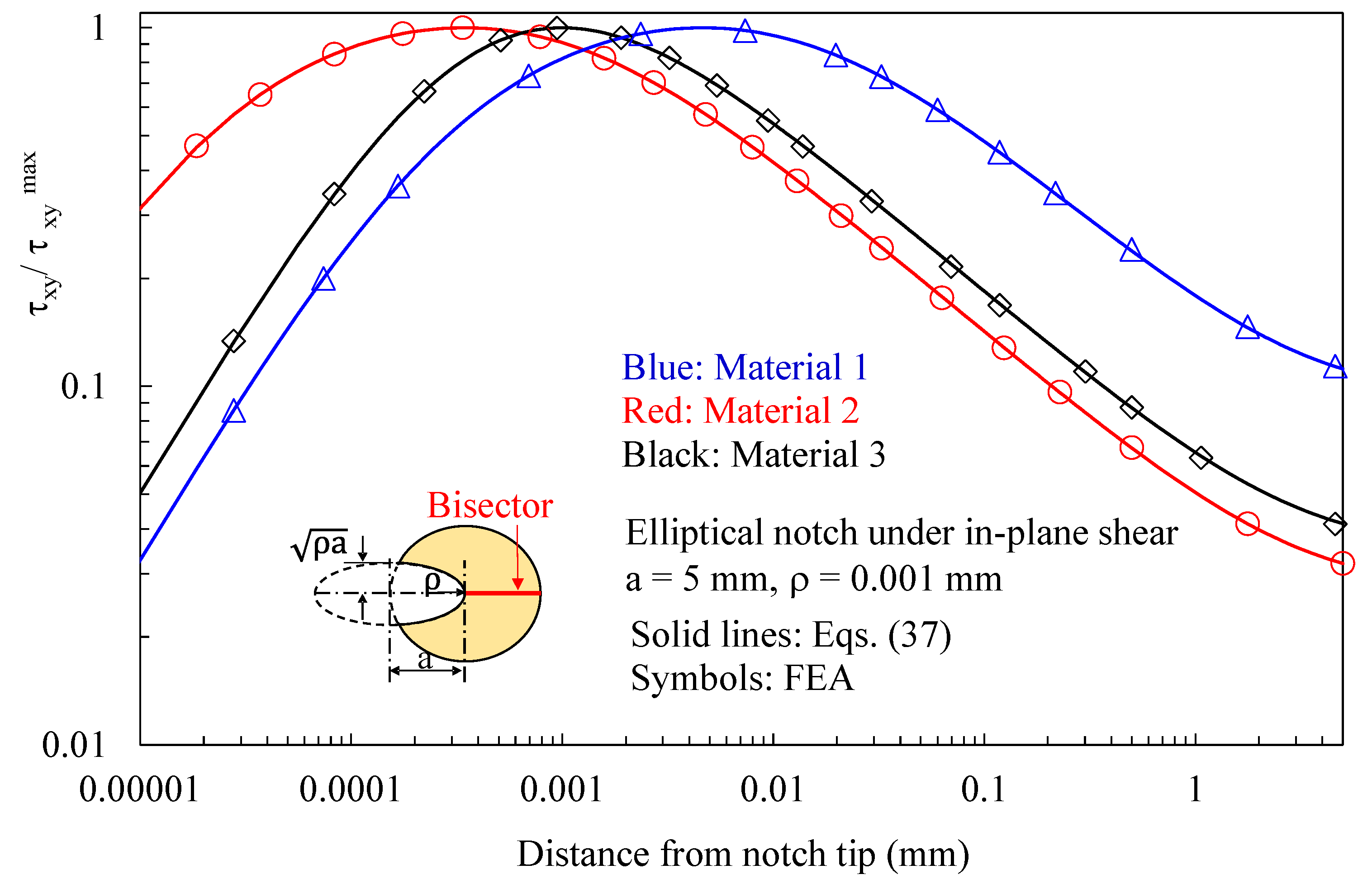
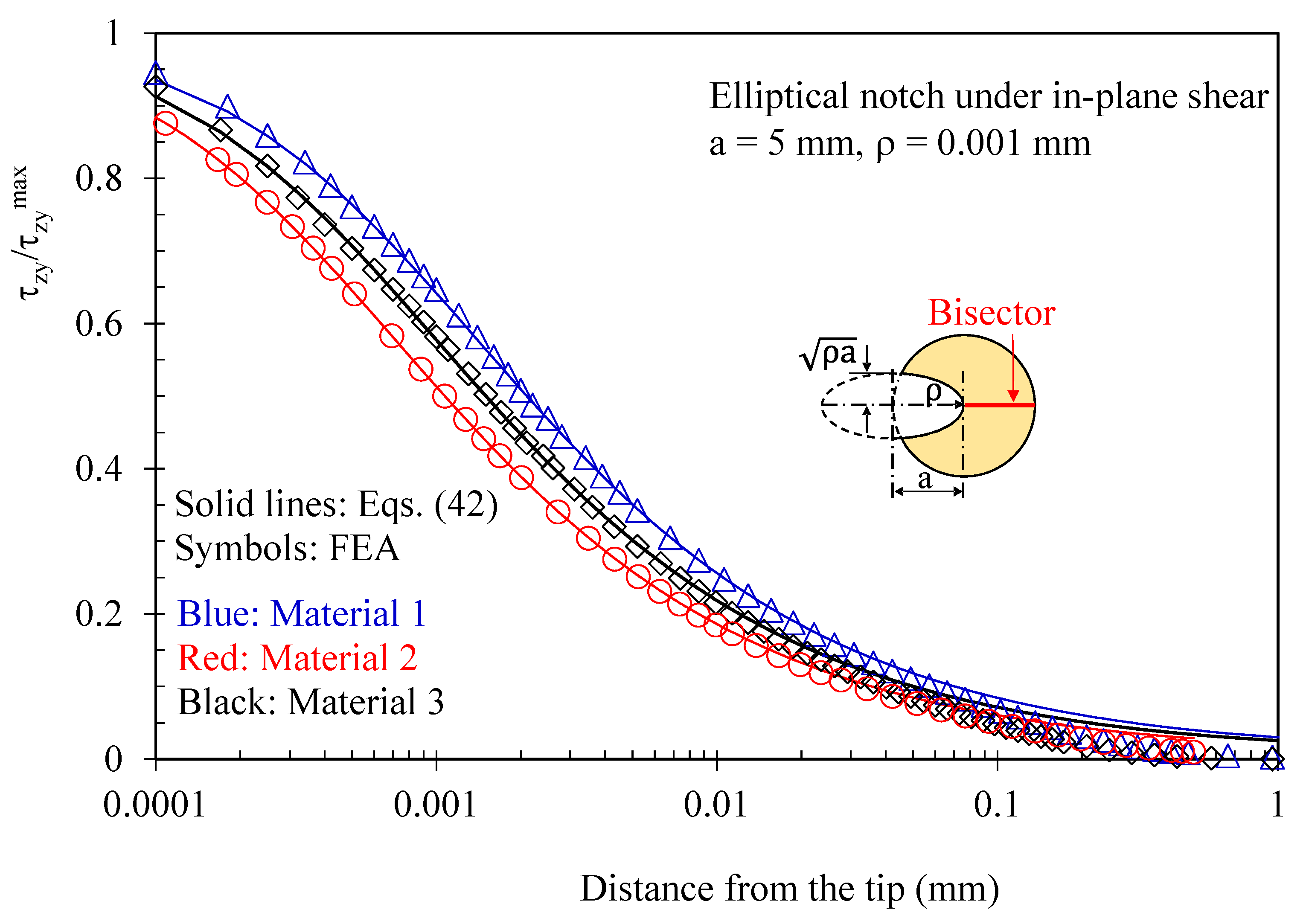
3. Elliptical Hole in a Thick Plate under Shear
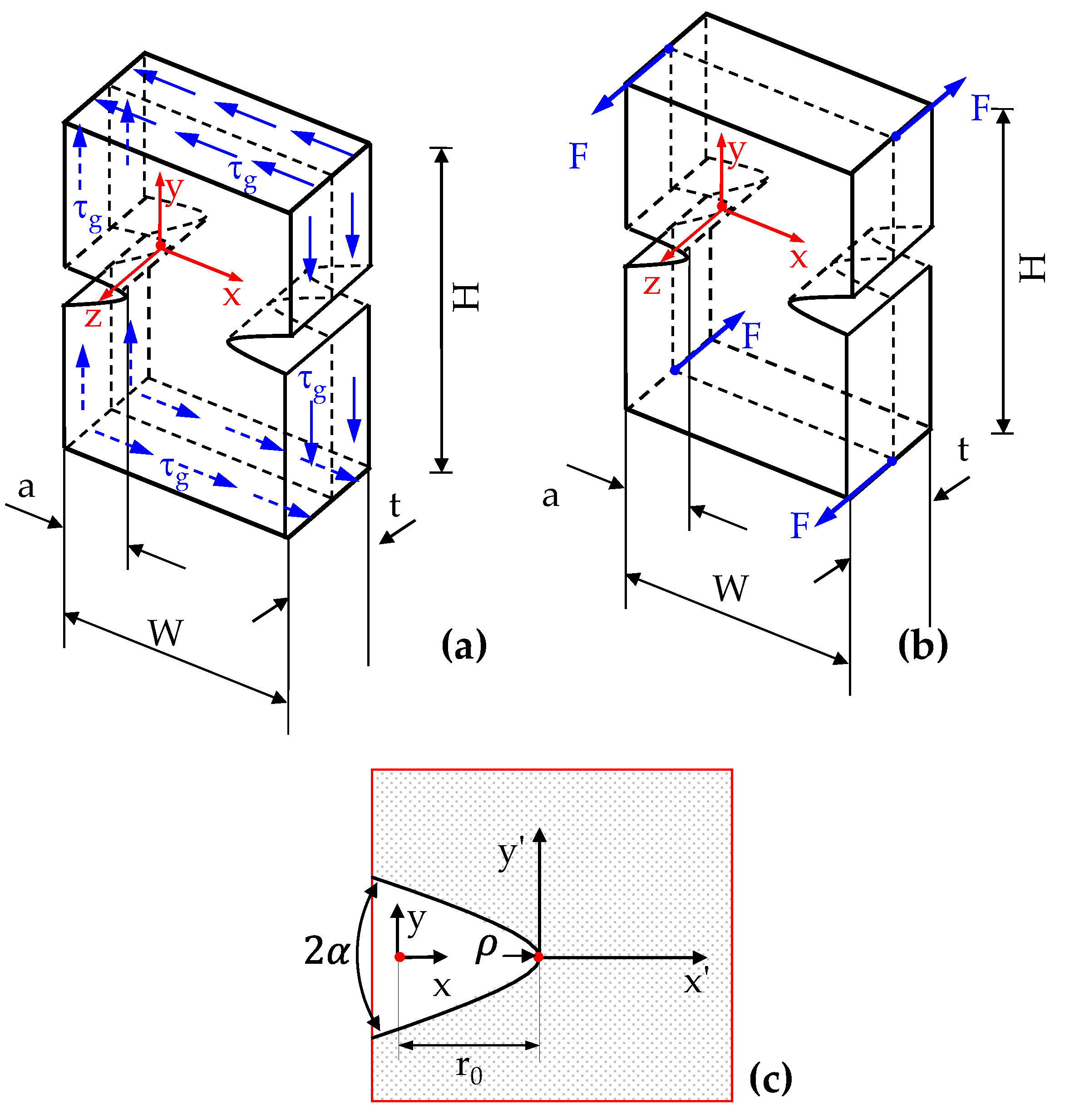
4. Lateral Radiused Notch under Shear
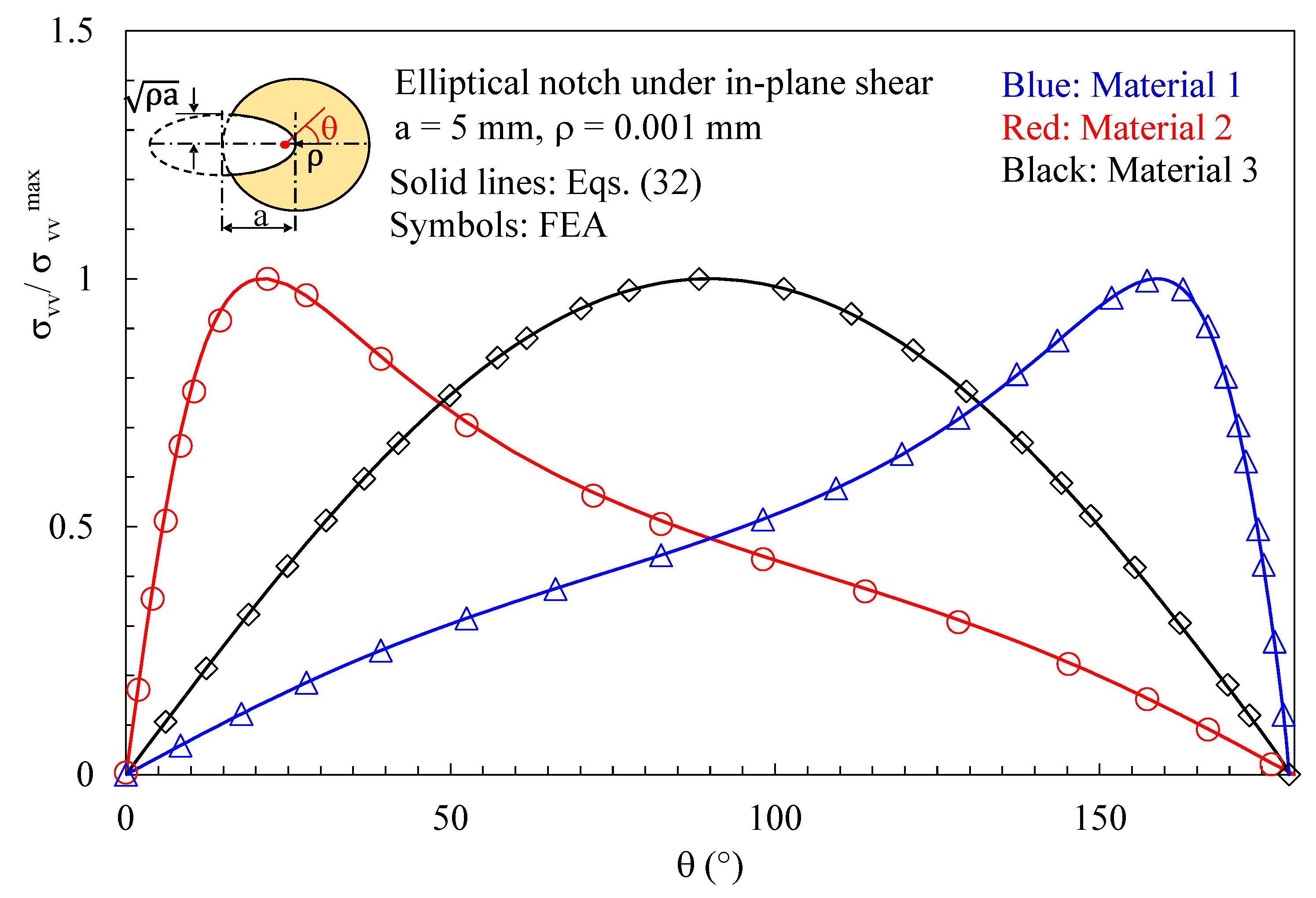
5. Discussion and Results
- Material 1 represents a unidirectional carbon-fibre-reinforced epoxy laminate with the fibres oriented in the direction of the notch bisector;
- Material 2 represents the same material with fibres oriented in the direction normal to the notch bisector;
- Material 3 represents a quasi-isotropic carbon-fibre-reinforced epoxy laminate (e.g., [(0/±45/90)n]S).
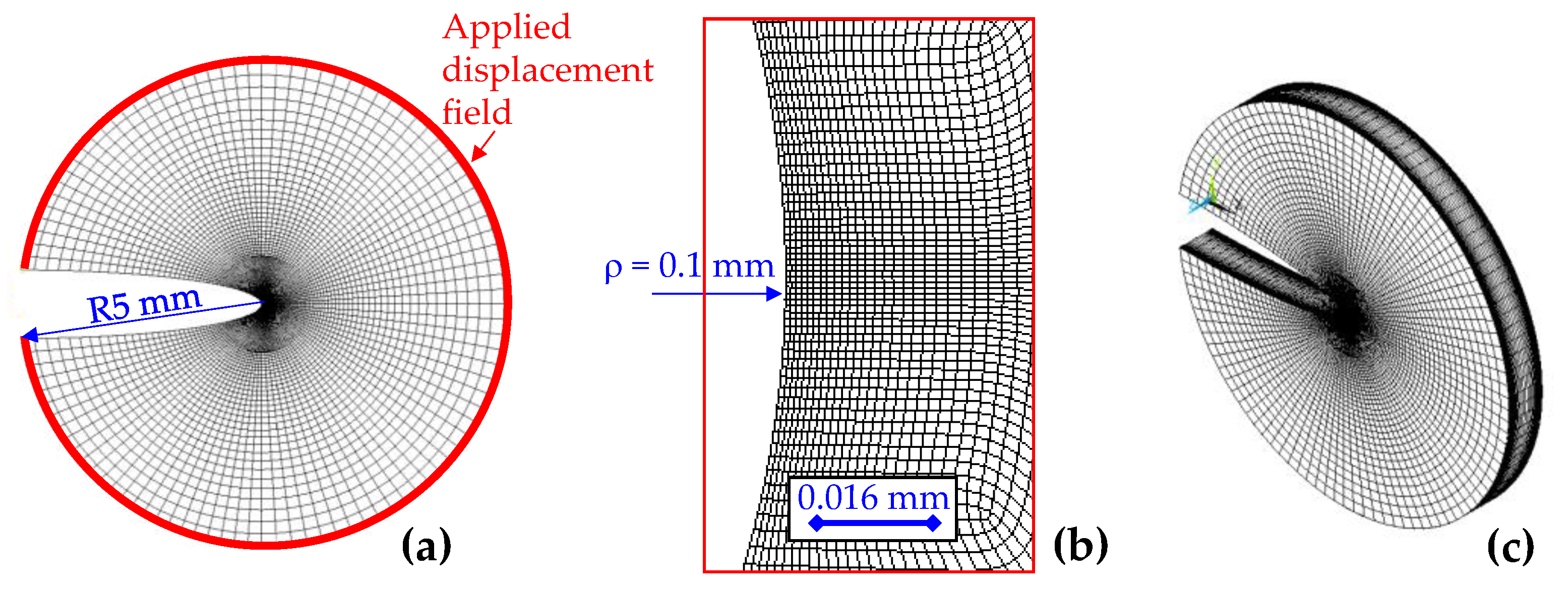
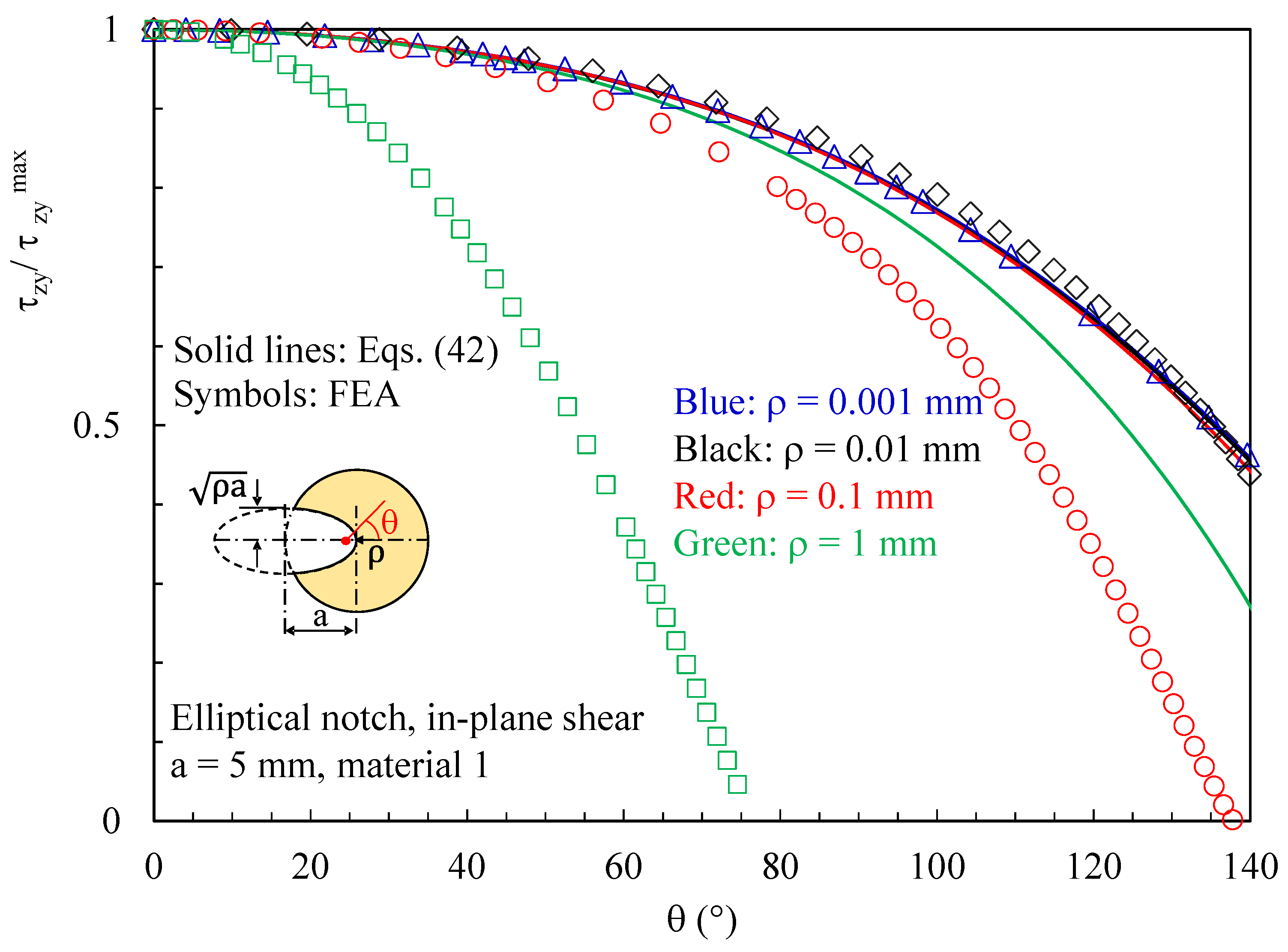
- Semi-elliptical notches with notch depth a = 5 mm and different notch root radii ( = 0.001 mm, 0.01 mm, 0.1 mm and 1 mm); in this case, the radius of the disc was 5 mm and various thicknesses were considered (t = 1 mm, 5 mm and 10 mm);
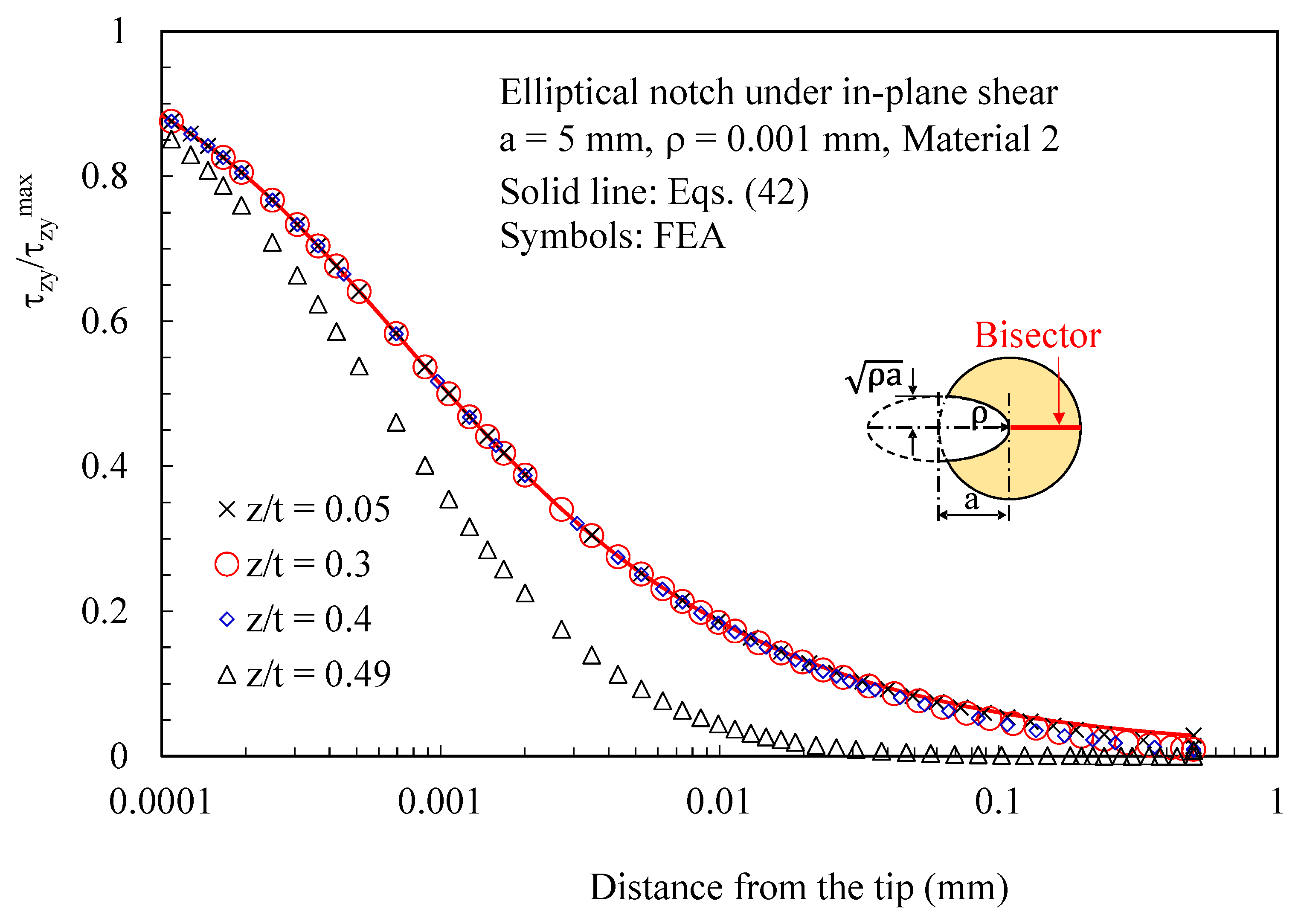
- The maximum in-plane shear stress remains constant for most of the plane thickness, and significantly increases as approaching the free surface of the plate (Figure 9a);
- The maximum out-of-plane shear stress has an almost linear trend up to z/t around 0.3. When approaching the free surface of the disc the trend becomes strongly nonlinear;
- The accuracy of Equation (42) decreases. Recalling the analytical treatise presented in Section 2, this is due to the terms and , which are more and more relevant when decreasing the value of the notch root radius, when compared to and .
- The region ahead of the notch tip where the out-of-plane shear stresses are significant progressively reduces.
6. Conclusions
- Three-dimensional effects in thick plates or discs induce coupling phenomena between loading modes. In particular, out-of-plane shear stresses (mode 3) are induced on in-plane shear-loaded (mode 2) solids and can be accurately predicted using the solutions derived for the antiplane deformation problem. The vice versa is also true, independently of the orthotropic material system considered.
- Increasing the disc or plate thickness results in an increase in the intensity of stresses induced by 3D effects;
- The intensity of induced stresses is also significantly affected by the notch root radius, and the phenomenon tends to become negligible in the presence of large notch root radii.
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
Appendix A
Appendix A.1. Displacement Fields for Elliptical Notches under Mode 2
Appendix A.2. Displacement Fields for Hyperbolic Notches under Mode 2 [34]
Appendix A.3. Displacement Fields for Hyperbolic Notches under Mode 3 [31]
References
- Neuber, H. Theory of Notch Stresses; Springer: Berlin, Germany, 1958. [Google Scholar]
- Creager, M.; Paris, P.C. Elastic field equations for blunt cracks with reference to stress corrosion cracking. Int. J. Fract. Mech. 1967, 3, 247–252. [Google Scholar] [CrossRef]
- Lazzarin, P.; Tovo, R. A unified approach to the evaluation of linear elastic stress fields in the neighborhood of cracks and notches. Int. J. Fract. 1996, 78, 3–19. [Google Scholar] [CrossRef]
- Zappalorto, M.; Lazzarin, P.; Filippi, S. Stress field equations for U and blunt V-shaped notches in axisymmetric shafts under torsion. Int. J. Fract. 2010, 164, 253–269. [Google Scholar] [CrossRef]
- Zappalorto, M.; Lazzarin, P. Stress fields due to inclined notches and shoulder fillets in shafts under torsion. J. Strain Anal. Eng. Des. 2011, 46, 187–199. [Google Scholar] [CrossRef]
- Zappalorto, M.; Lazzarin, P. In-plane and out-of-plane stress field solutions for V-notches with end holes. Int. J. Fract. 2011, 168, 167–180. [Google Scholar] [CrossRef]
- Lekhnitskii, S.G. Theory of Elasticity of an Anisotropic Body; Mir Publishers: Moskow, Russia, 1984. [Google Scholar]
- Bonora, N.; Costanzi, M.; Marchetti, M. On closed form solution for the elastic stress field around holes in orthotropic composite plates under in-plane stress conditions. Compos. Struct. 1993, 25, 139–156. [Google Scholar] [CrossRef]
- Bonora, N.; Costanzi, M.; Marchetti, M. A computational procedure to calculate stress-strain field around simple shape holes in composite laminates. Compos. Struct. 1994, 53, 1167–1179. [Google Scholar] [CrossRef]
- Zappalorto, M.; Carraro, P.A. Stress distributions for blunt cracks and radiused slits in anisotropic plates under in-plane loadings. Int. J. Solids Struct. 2015, 56–57, 136–141. [Google Scholar] [CrossRef]
- Sih, G.C.; Paris, P.C.; Irwin, G.R. On cracks in rectilinearly anisotropic bodies. Int. J. Fract. Mech. 1965, 1, 189–203. [Google Scholar] [CrossRef]
- Kazberuk, A.; Savruk, M.P.; Chornenkyi, A.B. Stress distribution at sharp and rounded V-notches in quasi-orthotropic plane. Int. J. Solids Struct. 2016, 85–86, 134–143. [Google Scholar] [CrossRef]
- Prabhu, S.; Lambros, J. A Numerical Investigation of Three Dimensional Effects in Cracked Orthotropic Plates. J. Compos. Mater. 2000, 34, 116–134. [Google Scholar] [CrossRef]
- Cheng, C.Z.; Zhou, W.; Niu, Z.R.; Recho, N. Stress singularity analysis for orthotropic V-notches in the generalised plane strain state. Fatigue Fract. Eng. Mater. Struct. 2015, 38, 881–896. [Google Scholar] [CrossRef]
- Pageau, S.S.; Biggers, S.B.J. A finite element approach to three-dimensional singular stress states in anisotropic multi-material wedges and junctions. Int. J. Solids Struct. 1996, 33, 33–47. [Google Scholar] [CrossRef]
- Choi, Y.K.; Folias, E.S. The 3D stress field in a laminated composite plate with a hole based on an h-r finite element method. In Failure Mechanics in Advanced Polymeric Composites; ASME AMD: New York, NY, USA, 1994; Volume 196, pp. 31–42. [Google Scholar]
- Kotousov, A.; Wang, C.H. Three-dimensional solutions for transversally isotropic composite plates. Compos. Struct. 2002, 57, 445–452. [Google Scholar] [CrossRef]
- Kotousov, A.; Wang, C.H. A generalized plane-strain theory for transversally isotropic plates. Acta Mech. 2003, 161, 53–64. [Google Scholar] [CrossRef]
- Zappalorto, M.; Carraro, P.A. Stress fields at sharp angular corners in thick anisotropic composite plates. Compos. Struct. 2014, 117, 346–353. [Google Scholar] [CrossRef]
- Zappalorto, M.; Carraro, P.A.; Quaresimin, M. Analytical solution for the three-dimensional stress fields in anisotropic composite bimaterial corners. Comp. Struct. 2015, 122, 127–138. [Google Scholar] [CrossRef]
- Zappalorto, M. On the stress state in rectilinear anisotropic thick plates with blunt cracks. Fatigue Fract. Eng. Mater. Struct. 2017, 40, 103–119. [Google Scholar] [CrossRef]
- Shi, Y.; Wang, B.; Wu, H.; Wang, B.; Liu, C.; Li, R. A Theoretical and Experimental Study on Extreme Stress Concentration-Free Designs of Circumferentially Notched Thin Cylindrical Shells. J. Appl. Mech. 2020, 87, 021004. [Google Scholar] [CrossRef]
- Wang, B.; Shi, Y.; Li, R.; Wang, B. A Simplified Indirect Measuring Method for the Notch Stress in a Thin Cylindrical Shell. J. Appl. Mech. 2018, 85, 071009. [Google Scholar] [CrossRef]
- Kotousov, A.; Lew, Y.T. Stress singularities resulting from various boundary conditions in angular corners of plates of arbitrary thickness in extension. Int. J. Solids Struct. 2006, 43, 5100–5109. [Google Scholar] [CrossRef]
- Berto, F.; Lazzarin, P.; Kotousov, A.; Pook, L.P. Induced out-of-plane mode at the tip of blunt lateral notches and holes under in-plane shear loading. Fatigue Fract. Eng. Mater. Struct. 2012, 35, 538–555. [Google Scholar] [CrossRef]
- Berto, F.; Marangon, C. Three-dimensional effects in finite thickness plates weakened by rounded notches and holes under in-plane shear. Fatigue Fract. Eng. Mater. Struct. 2013, 36, 1139–1152. [Google Scholar] [CrossRef]
- Lazzarin, P.; Zappalorto, M. A three-dimensional stress field solution for pointed and sharply radiused V-notches in plates of finite thickness. Fatigue Fract. Eng. Mater. Struct. 2012, 35, 1105–1119. [Google Scholar] [CrossRef]
- Zappalorto, M.; Lazzarin, P. Three-dimensional elastic stress fields ahead of notches in thick plates under various loading conditions. Eng. Fract. Mech. 2013, 108, 75–88. [Google Scholar] [CrossRef]
- Lazzarin, P.; Zappalorto, M.; Berto, F. Three-dimensional stress fields due to notches in plates under linear elastic and elastic–plastic conditions. Fatigue Fract. Eng. Mater. Struct. 2015, 38, 140–153. [Google Scholar] [CrossRef]
- Zappalorto, M.; Carraro, P.A. An engineering formula for the stress concentration factor of orthotropic composite plates. Composites Part B 2015, 68, 51–58. [Google Scholar] [CrossRef]
- Zappalorto, M.; Salviato, M. Antiplane shear stresses in orthotropic plates with lateral blunt notches. Eur. J. Mech. A-Solids 2019, 77, 103815. [Google Scholar] [CrossRef]
- Savin, G.N. Stress Concentration Around Holes; Pergamon: London, UK, 1961. [Google Scholar]
- Zappalorto, M.; Carraro, P.A. Two-dimensional stress distributions in tensioned orthotropic plates weakened by blunt V-shaped notches. Fatigue Fract. Eng. Mater. Struct. 2017, 40, 804–819. [Google Scholar] [CrossRef]
- Pastrello, M.; Salviato, M.; Zappalorto, M. Stress distributions in orthotropic solids with blunt notches under in-plane shear loadings. Eur. J. Mech. A-Solids 2022, 92, 104436. [Google Scholar] [CrossRef]
- Zappalorto, M.; Lazzarin, P. A unified approach to the analysis of nonlinear stress and strain fields ahead of mode III-loaded notches and cracks. Int J. Solids Struct. 2010, 47, 851–864. [Google Scholar] [CrossRef]



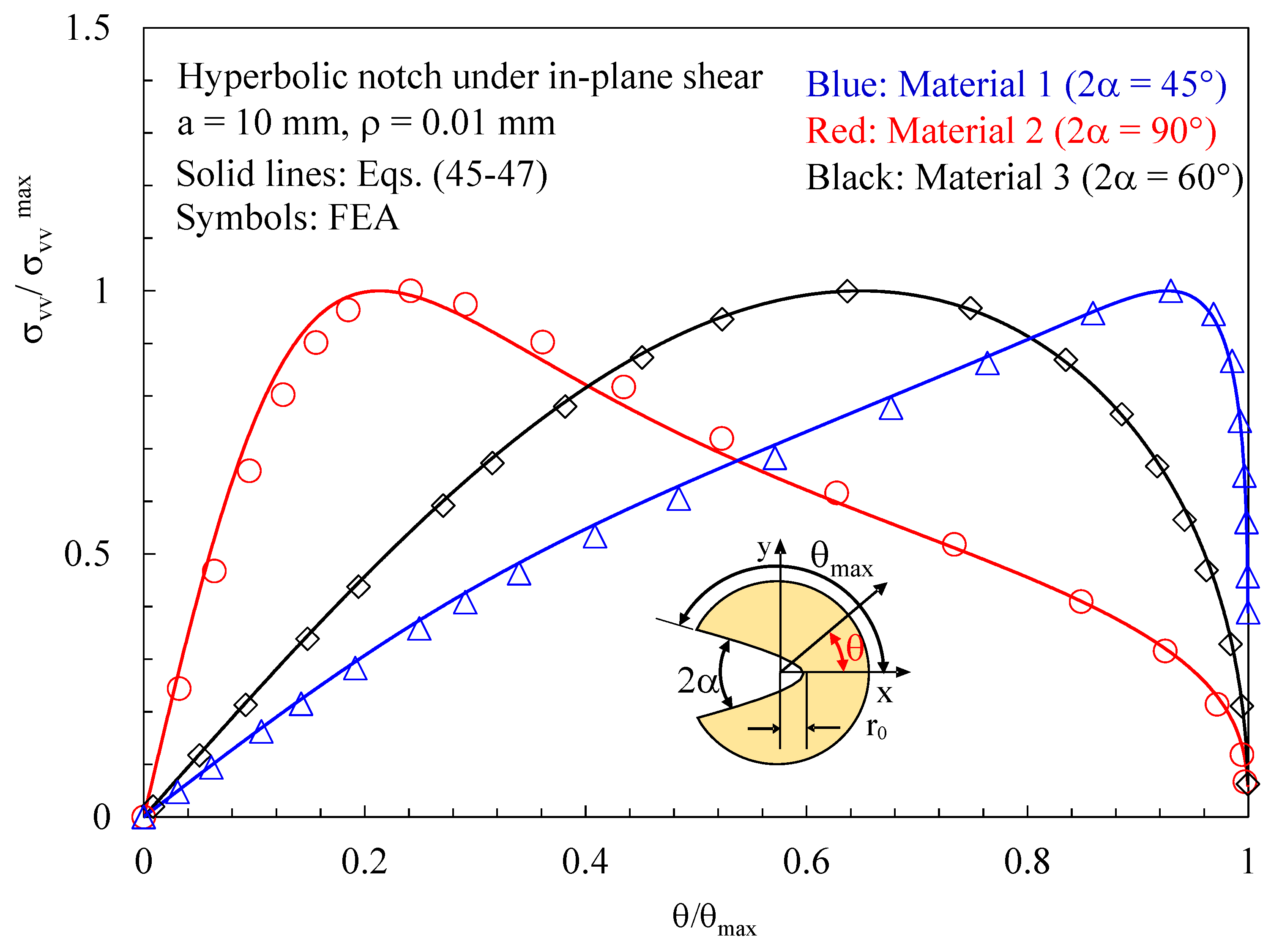
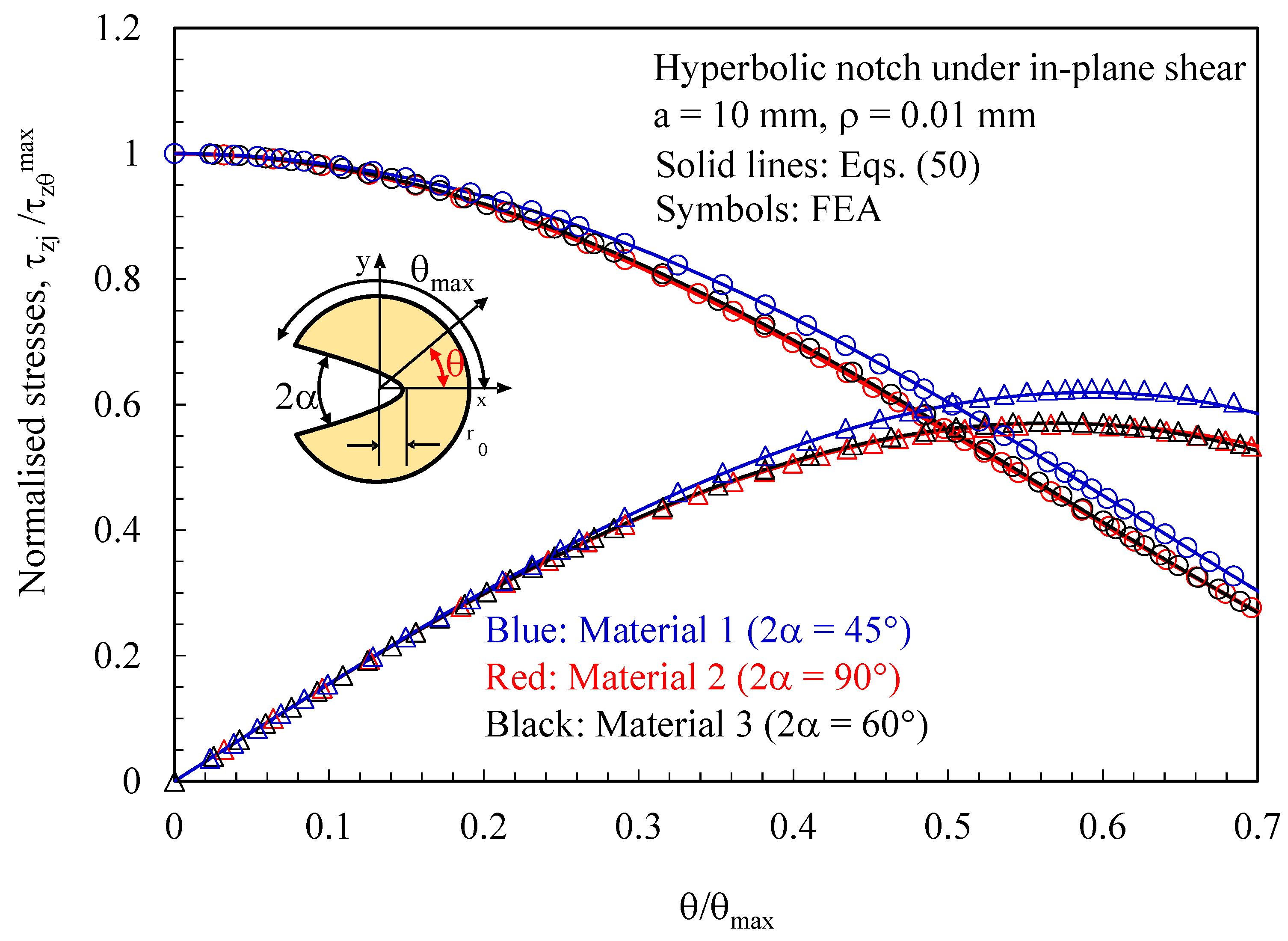


| Ex (GPa) | Ey (GPa) | Ez (GPa) | νxy | νxz | νyz | Gxy (GPa) | Gxz (GPa) | Gyz (GPa) | β1 | β2 | β3 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Material 1 | 160 | 10 | 10 | 0.3 | 0.3 | 0.4 | 5 | 5 | 3.57 | 0.6614 | 5.5587 | 1.1835 |
| Material 2 | 10 | 160 | 10 | 0.01875 | 0.4 | 0.3 | 5 | 3.57 | 5 | 0.1798 | 1.5120 | 0.8450 |
| Material 3 | 70 | 70 | 70 | 0.3 | 0.3 | 0.3 | 26.9 | 26.9 | 26.9 | 0.9993 | 1.0006 | 1.0000 |
| t2 | λ2 | μ2 | ζ2 | χ12 | χ21 | χ22 | χ23 | |
|---|---|---|---|---|---|---|---|---|
| Material 1, 2α = 45° | 1.6849 | 0.8757 | 0.3533 | −0.3806 | 0.2997 | −0.2733 | 0.0888 | −0.4661 |
| Material 2, 2α = 90° | 1.7319 | 0.7788 | 0.2551 | −0.8948 | 0.1629 | −0.4744 | 0.0152 | −0.0417 |
| Material 3, 2α = 60° | 1.5200 | 0.7309 | 0.1924 | −3.7178 | 0.0738 | −0.9996 | −0.0734 | −4.3·10−07 |
| t3 | λ3 | q | |
|---|---|---|---|
| Material 1, 2α = 45° | 1.8111 | 0.5848 | 1.7500 |
| Material 2, 2α = 90° | 1.6055 | 0.6438 | 1.5000 |
| Material 3, 2α = 60° | 1.7519 | 0.6000 | 1.6667 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pontefisso, A.; Pastrello, M.; Zappalorto, M. Three-Dimensional Stress Fields in Thick Orthotropic Plates with Sharply Curved Notches under In-Plane and Out-of-Plane Shear. Polymers 2023, 15, 2013. https://doi.org/10.3390/polym15092013
Pontefisso A, Pastrello M, Zappalorto M. Three-Dimensional Stress Fields in Thick Orthotropic Plates with Sharply Curved Notches under In-Plane and Out-of-Plane Shear. Polymers. 2023; 15(9):2013. https://doi.org/10.3390/polym15092013
Chicago/Turabian StylePontefisso, Alessandro, Matteo Pastrello, and Michele Zappalorto. 2023. "Three-Dimensional Stress Fields in Thick Orthotropic Plates with Sharply Curved Notches under In-Plane and Out-of-Plane Shear" Polymers 15, no. 9: 2013. https://doi.org/10.3390/polym15092013
APA StylePontefisso, A., Pastrello, M., & Zappalorto, M. (2023). Three-Dimensional Stress Fields in Thick Orthotropic Plates with Sharply Curved Notches under In-Plane and Out-of-Plane Shear. Polymers, 15(9), 2013. https://doi.org/10.3390/polym15092013







