Effects of Curing Defects in Adhesive Layers on Carbon Fiber–Quartz Fiber Bonded Joint Performance
Abstract
1. Introduction
2. Experimental Section
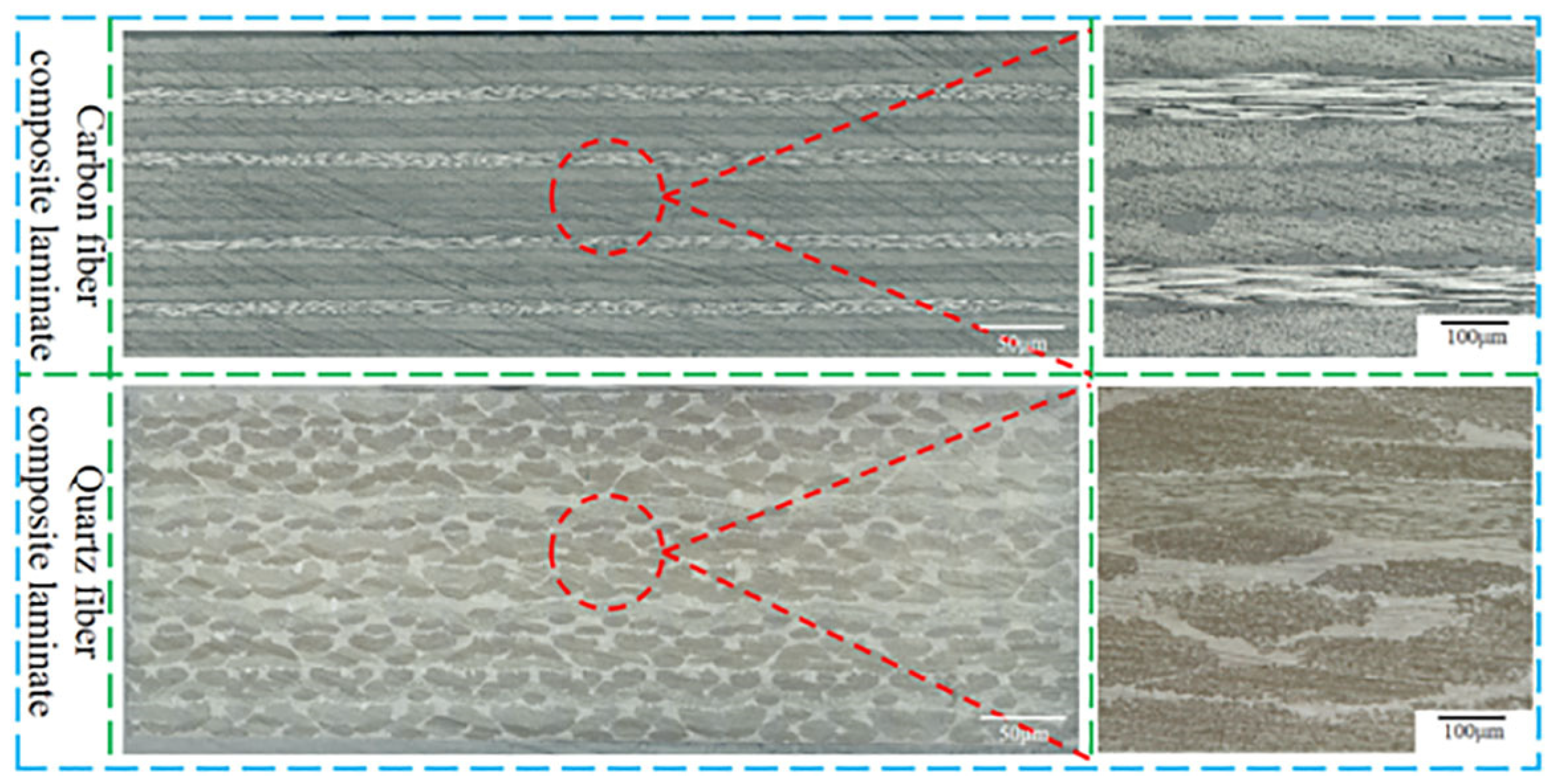
2.1. Materials
2.2. Testing Thermal Physical Parameters of Film
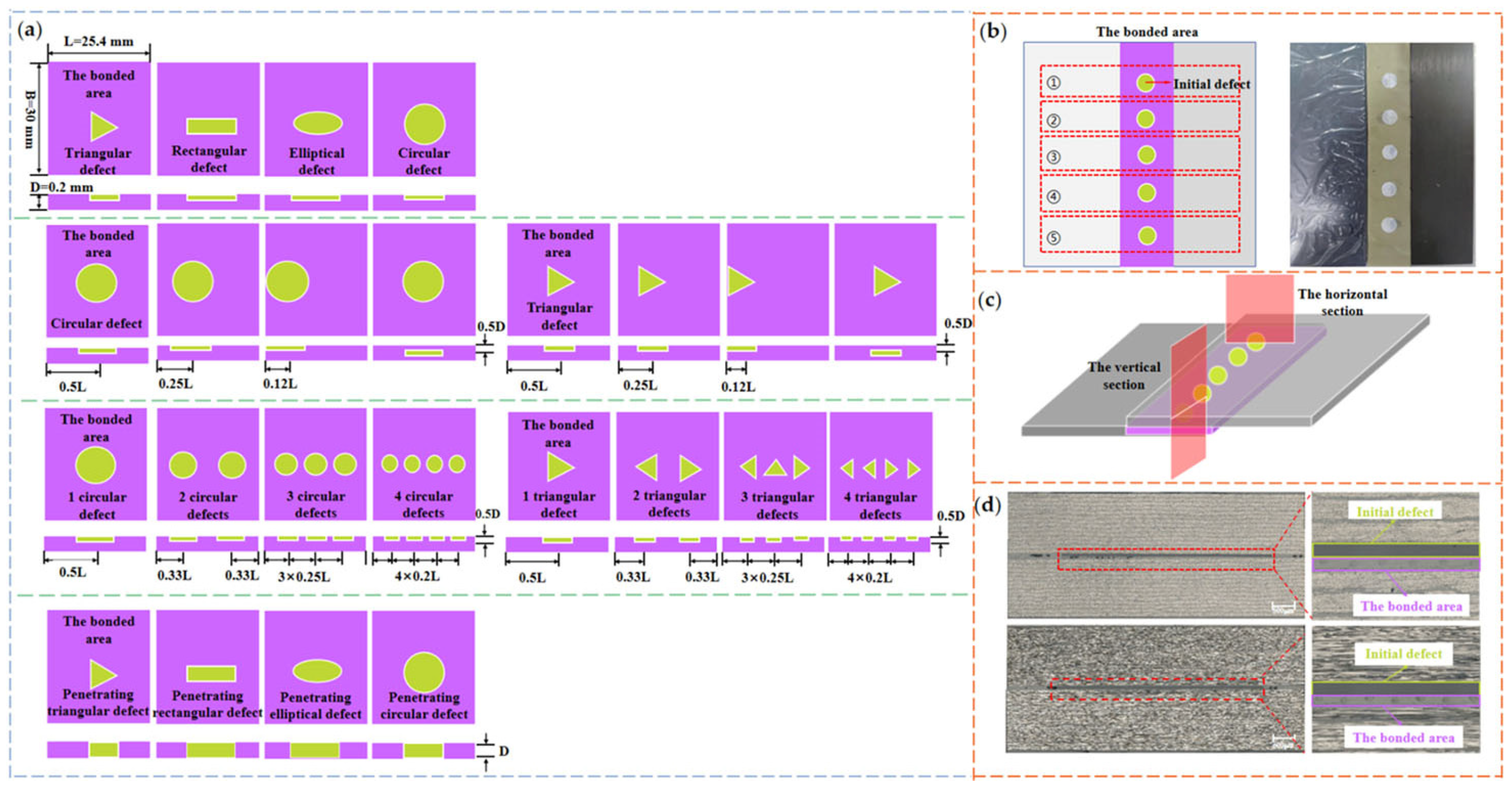
2.3. Adhesive Bonded Specimen and Defect Design
2.4. Orthogonal Experimental Method of Bonding Process
2.4.1. Difference Analysis Method
2.4.2. Variance Analysis Method
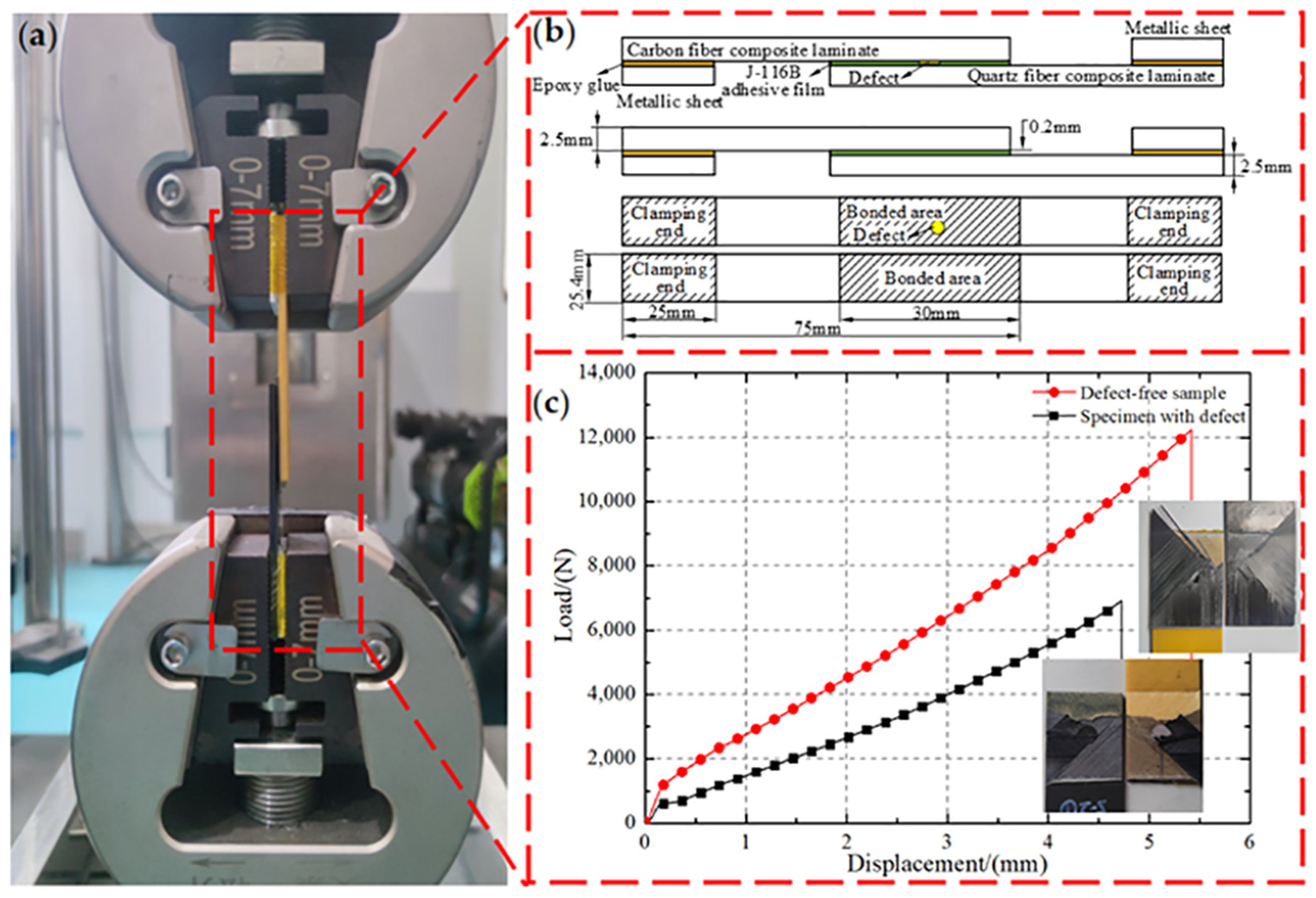
2.5. Mechanical Performance Testing
3. Results and Discussion
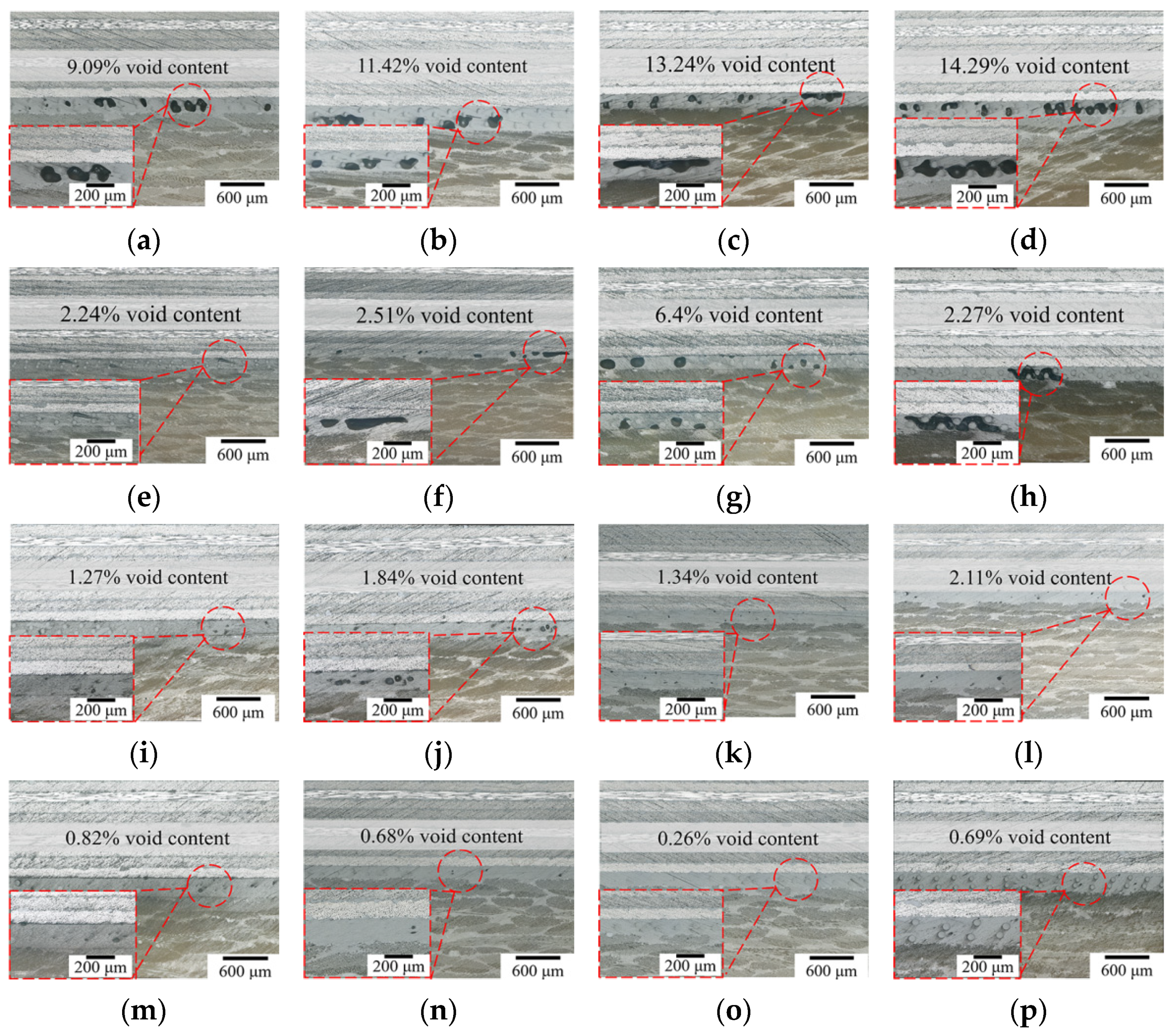
3.1. Thermophysical Parameter Analysis of Film
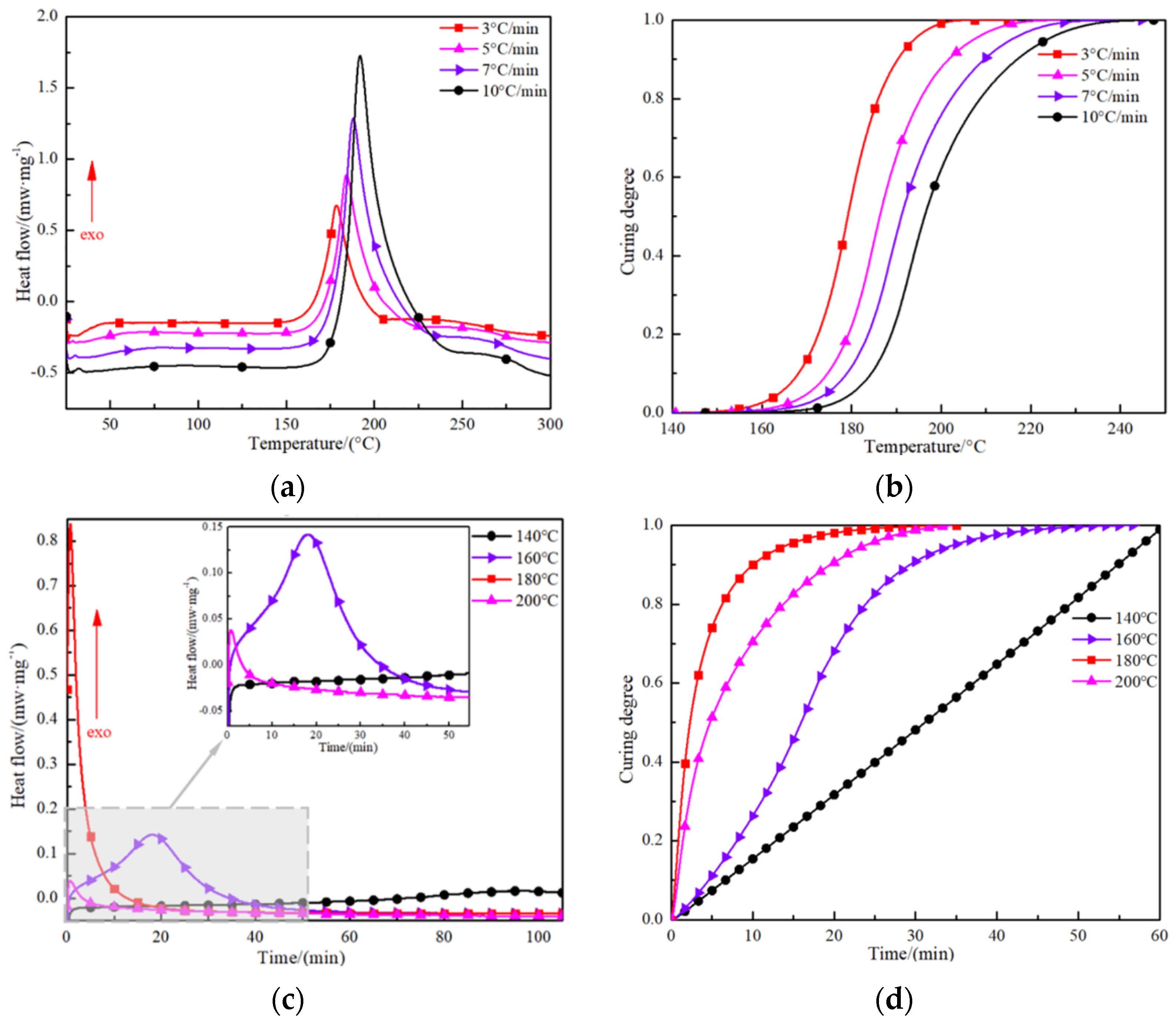
3.1.1. Non-Isothermal and Isothermal Parameter Analysis of Film
3.1.2. TGA Test for Film and Resin
3.2. Effect of Bonding Defects on Mechanical Performance
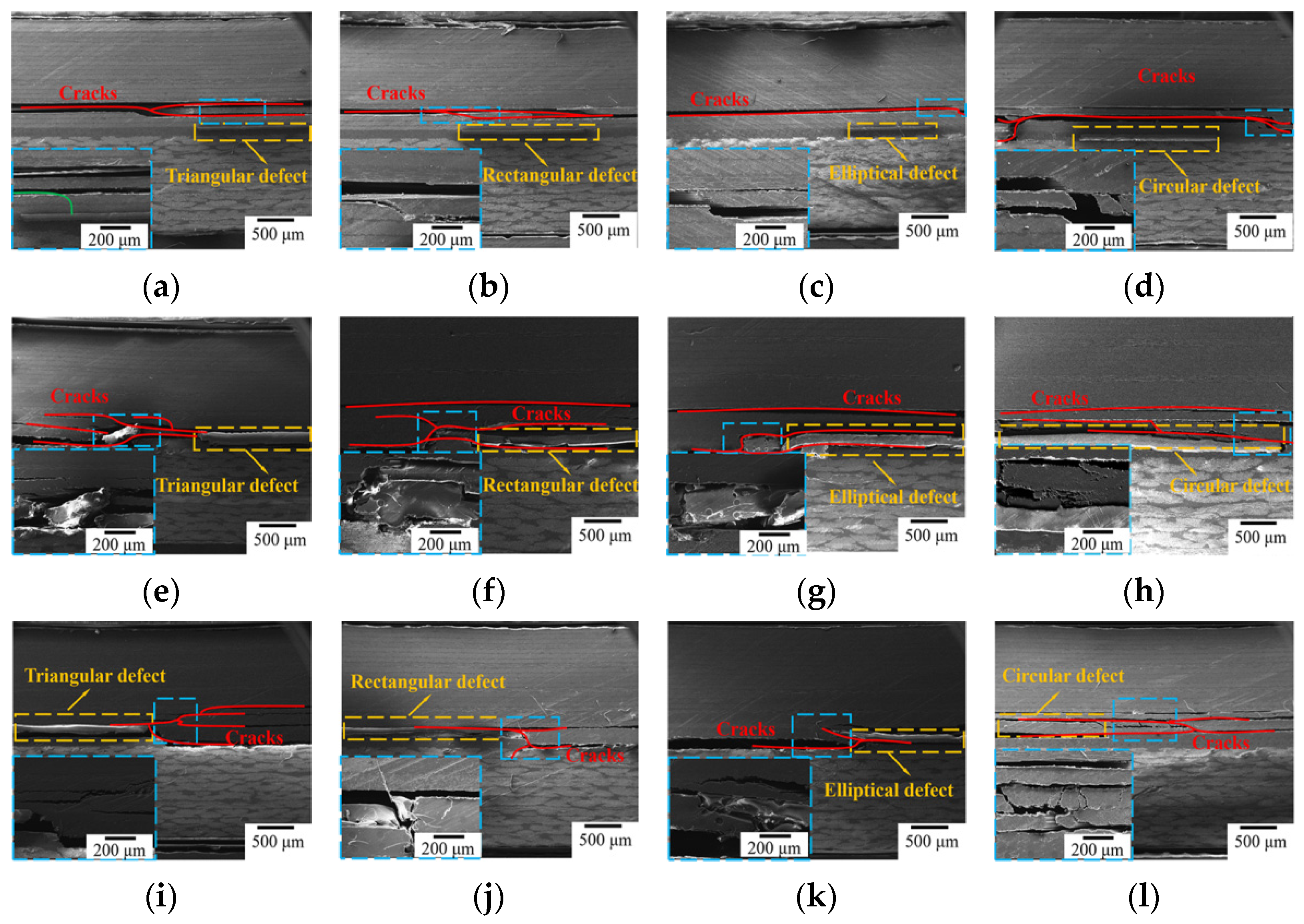
3.2.1. Effect of Defect Shape and Size
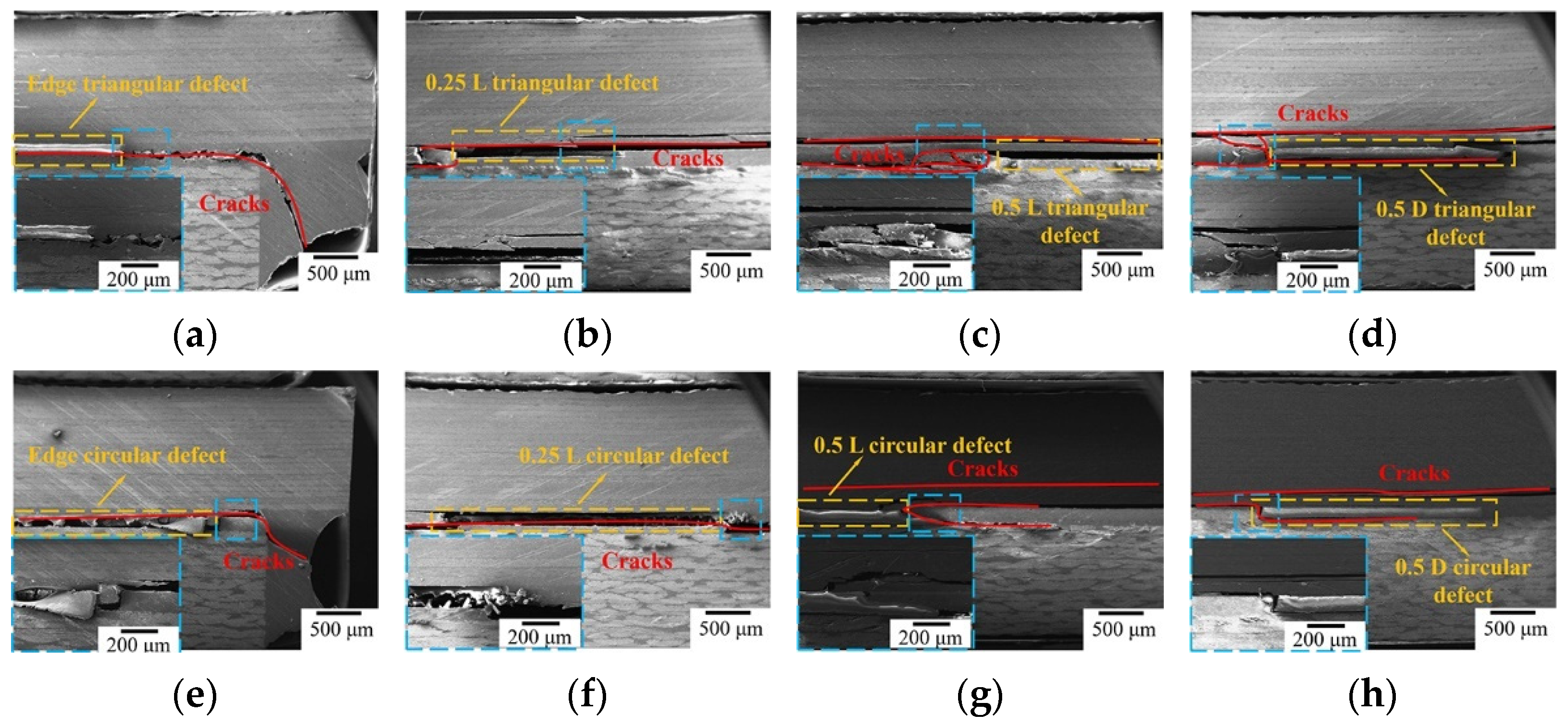
3.2.2. Effect of Defect Location on Performance
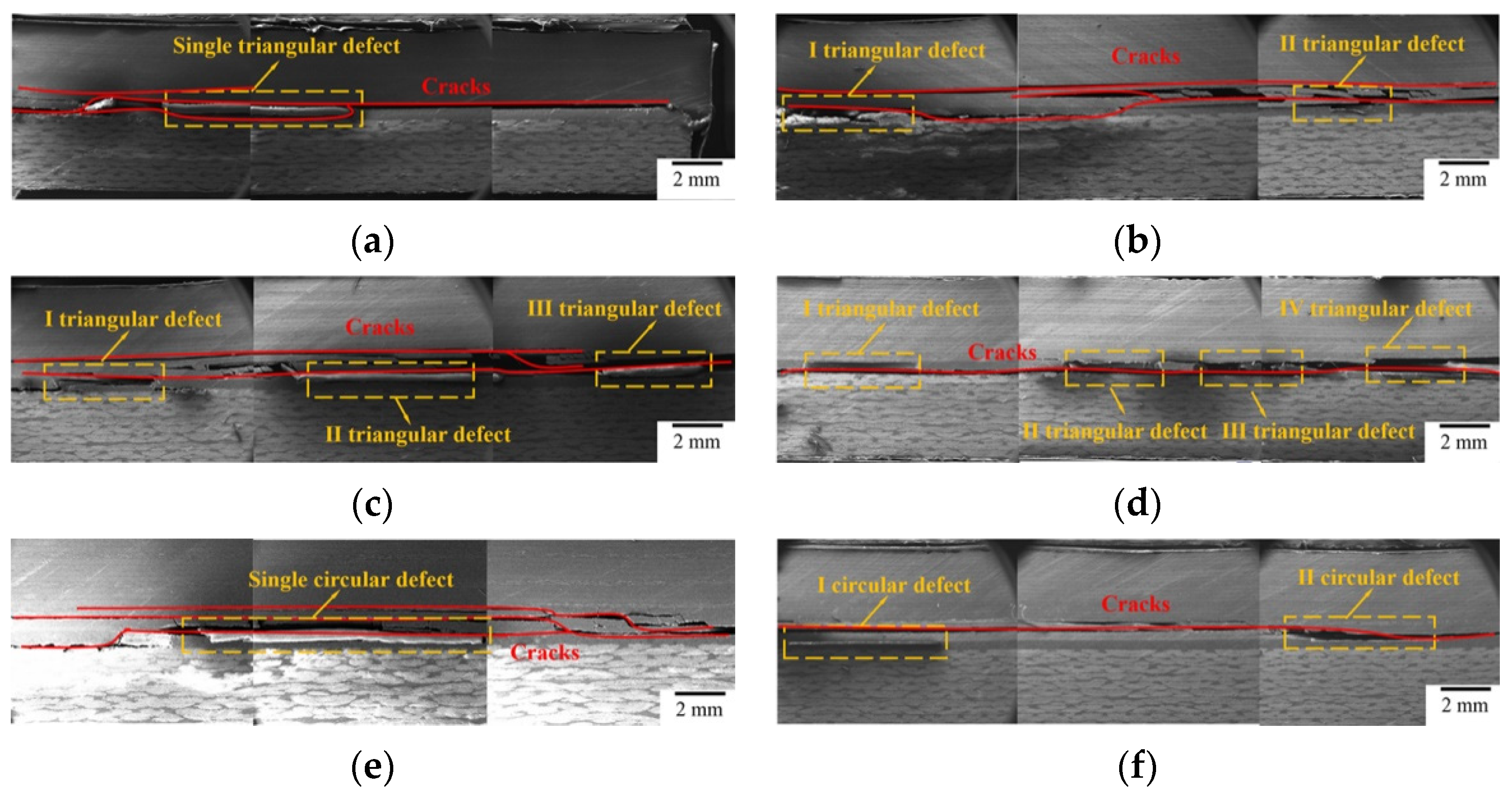
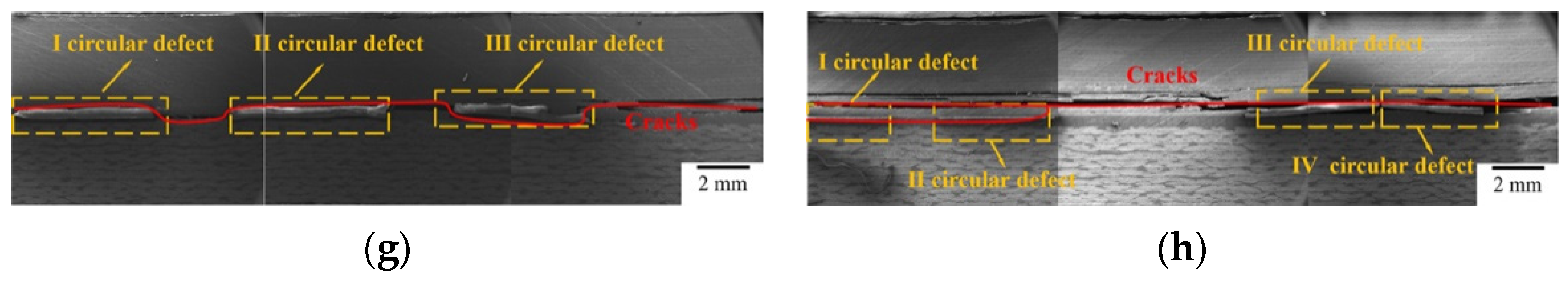
3.2.3. Effect of Defect Number on Performance
3.2.4. Effect of Penetrating Defect on Performance
3.3. Orthogonal Test Analysis of Bonding Process
3.3.1. Difference Analysis
3.3.2. Variance Analysis
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Tiwary, A.; Kumar, R.; Chohan, J.S. A review on characteristics of composite and advanced materials used for aerospace applications. Mater. Today Proc. 2022, 51, 865–870. [Google Scholar] [CrossRef]
- Morgan, R.J.; Shin, E.; Lincoln, J.E.; Zhou, J. Overview of polymer matrix composites performance and materials development for aerospace applications. Sampe J. 2001, 37, 102–107. [Google Scholar]
- Chu, H.; Zhang, Z.; Liu, Y.; Leng, J. Silver particles modified carbon nanotube paper/glassfiber reinforced polymer composite material for high temperature infrared stealth camouflage. Carbon 2016, 98, 557–566. [Google Scholar] [CrossRef]
- He, D.; Sawa, T.; Iwamoto, T.; Hirayama, Y. Stress analysis and strength evaluation of scarf adhesive joints subjected to static tensile loadings. Int. J. Adhes. Adhes. 2010, 30, 387–392. [Google Scholar] [CrossRef]
- Zhang, J.; Liu, Z.; Han, M.; Zhang, J.; Tang, Y.; Gu, J. Block copolymer functionalized quartz fibers/cyanate ester wave-transparent laminated composites. J. Mater. Sci. Technol. 2023, 139, 189–197. [Google Scholar] [CrossRef]
- Wu, C.; Zhao, X.; Duan, W.H.; Al-Mahaidi, R. Bond characteristics between ultra high modulus CFRP laminates and steel. Thin-Walled Struct. 2012, 51, 147–157. [Google Scholar] [CrossRef]
- Homayouni, S.M.; Vasili, M.R.; Hong, T.S. Bonding Technologies in Manufacturing Engineering. Compr. Mater. Process 2014, 6, 237–246. [Google Scholar]
- Kastratovića, G.; Grbovićb, A.; Sedmakb, A.; Božićc, Ž.; Sedmak, S. Composite material selection for aircraft structures based on experimental and numerical evaluation of mechanical properties. Procedia Struct. Integr. 2021, 31, 127–133. [Google Scholar] [CrossRef]
- Wei, Y.; Jin, X.; Luo, Q.; Li, Q.; Sun, G. Adhesively bonded joints—A review on design, manufacturing, experiments, modeling and challenges. Compos. B Eng. 2024, 276, 111225. [Google Scholar] [CrossRef]
- Jeevi, G.; Nayak, S.K.; Kader, M.A. Review on adhesive joints and their application in hybrid composite structures. J. Adhes. Sci. Technol. 2019, 33, 1497–1520. [Google Scholar] [CrossRef]
- Neves, M.d.A.; Coutinho, E.; Cardoso, M.V.; Jaecques, S.; Lambrechts, P.; Sloten, J.V.; Oosterwyck, H.V.; Meerbeek, B.V. Influence of notch geometry and interface on stress concentration and distribution in micro-tensile bond strength specimens. J. Dent. 2008, 36, 808–815. [Google Scholar] [CrossRef] [PubMed]
- Agius, S.L.; Magniez, K.J.C.; Fox, B.L. Fracture behaviour of a rapidly cured polyethersulfone toughened carbon fibre/epoxy composite. Compos. Struct. 2010, 92, 2119–2127. [Google Scholar] [CrossRef]
- Nele, L.; Caggiano, A.; Teti, R. Autoclave Cycle Optimization for High Performance Composite Parts Manufacturing. Procedia CIRP 2016, 57, 241–246. [Google Scholar] [CrossRef]
- Chang, T.; Zhan, L.; Tan, W.; Li, S. Effect of autoclave pressure on interfacial properties at micro- and macro- level in polymer-matrix composite laminates. Fiber. Polym. 2017, 18, 1614–1622. [Google Scholar] [CrossRef]
- Liu, L.; Zhang, B.; Wang, D.; Wu, Z. Effects of cure cycles on void content and mechanical properties of composite laminates. Compos. Struct. 2006, 73, 303–309. [Google Scholar] [CrossRef]
- Grunenfelder, L.K.; Nutt, S.R. Void formation in composite prepregs—Effect of dissolved moisture. Compos. Sci. Technol. 2010, 70, 2304–2309. [Google Scholar] [CrossRef]
- Budhe, S.; Banea, M.D.; Barros, S.D.; Silva, L.F.M.D. An updated review of adhesively bonded joints in composite materials. Int. J. Adhes. Adhes. 2017, 72, 30–42. [Google Scholar] [CrossRef]
- Kumar, R.L.V.; Bhat, M.R.; Murthy, C.R.L. Evaluation of kissing bond in composite adhesive lap joints using digital image correlation: Preliminary studies. Int. J. Adhes. Adhes. 2013, 42, 60–68. [Google Scholar] [CrossRef]
- Fame, C.M.; Correia, J.R.; Ghafoori, E.; Wu, C. Damage tolerance of adhesively bonded pultruded GFRP double-strap joints. Compos. Struct. 2021, 263, 113625. [Google Scholar] [CrossRef]
- Fame, C.M.; Wu, C.; Feng, P.; Tam, L.-H. Numerical investigations on the damage tolerance of adhesively bonded pultruded GFRP joints with adhesion defects. Compos. Struct. 2022, 301, 116223. [Google Scholar] [CrossRef]
- Liu, Y.; Zhang, X.; Lemanski, S.; Nezhad, H.Y.; Ayre, D. Experimental and numerical study of process-induced defects and their effect on fatigue debonding in composite joints. Int. J. Fatigue 2019, 125, 47–57. [Google Scholar] [CrossRef]
- Yang, Y. Effect of Parameters on Strength of Composites Single-Lap Adhesive Joints. Adv. Mat. Res. 2012, 490, 2250–2253. [Google Scholar]
- Elhannani, M.; Madani, K.; Legrand, E.; Touzain, S.; Feaugas, X. Numerical analysis of the effect of the presence, number and shape of bonding defect on the shear stresses distribution in an adhesive layer for the single-lap bonded joint; Part 1. Aerosp. Sci. Technol. 2017, 62, 122–135. [Google Scholar] [CrossRef]
- Wang, D.; Li, Y.; Zou, T.; Fu, J.; Liu, Z. Increasing strength and fracture toughness of carbon fibre-reinforced plastic adhesively bonded joints by combining peel-ply and oxygen plasma treatments. Appl. Surf. Sci. 2023, 612, 155768. [Google Scholar] [CrossRef]
- Barakat, W.S.; Younis, M.K.; Sadoun, A.M.; Fathy, A.; Habba, M.I.A. Optimization of the accumulative roll bonding process parameters and SiC content for optimum enhancement in mechanical properties of Al-Ni-SiC composites. Alex. Eng. J. 2023, 76, 131–151. [Google Scholar] [CrossRef]
- Rafiei, J.; Ghasemi, A.R. Development of thermo-mechanical simulation of WC/Inconel 625 metal matrix composites laser cladding and optimization of process parameters. Int. J. Therm. Sci. 2024, 198, 10883. [Google Scholar] [CrossRef]
- Sahu, P.; Mishra, P.C. Combine experimental and FEM analysis of adhesive bonded single lap joint with Al-alloy flat adherends and pre-embedded artificial defects. SN. Appl. Sci. 2019, 1, 1455. [Google Scholar] [CrossRef]
- Senthil, K.; Arockiarajan, A.; Palaninathan, R.; Santhosh, B.; Usha, K.M. Defects in composite structures: Its effects and prediction methods—A comprehensive review. Compos. Struct. 2013, 106, 139–149. [Google Scholar] [CrossRef]
- Heidarpour, F.; Farahani, M.; Ghabezi, P. Experimental investigation of the effects of adhesive defects on the single lap joint strength. Int. J. Adhes. Adhes. 2018, 80, 128–132. [Google Scholar] [CrossRef]
- Costa, M.L.; Almeida, S.F.M.D.; Rezende, M.C. The influence of porosity on the interlaminar shear strength of carbon/epoxy and carbon/bismaleimide fabric laminates. Compos. Sci. Technol. 2001, 61, 2101–2108. [Google Scholar] [CrossRef]
- Wu, J.; Gao, X.; Huang, Y.; Ye, G.; Zhang, Y.; Gao, P.P. Parameter optimization and quality analysis of pulsed laser joining of 316L stainless steel and polylactic acid. Opt. Laser Technol. 2023, 159, 108965. [Google Scholar] [CrossRef]
- Chang, T.; Zhan, L.; Tan, W.; Li, S. Optimization of curing process for polymer-matrix composites based on orthogonal experimental method. Fiber. Polym. 2017, 18, 148–154. [Google Scholar] [CrossRef]
- ASTM D3165; Standard Test Method for Strength Properties of Adhesives in Shear by Tension Loading of Single-Lap-Joint Laminated Assemblies. ASTM: West Conshohocken, PA, USA, 2004.
- Yang, T.; Zhang, J.; Mouritz, A.P.; Wang, C.H. Healing of carbon fibre–epoxy composite T-joints using mendable polymer fibre stitching. Compos. B Eng. 2013, 45, 1499–1507. [Google Scholar] [CrossRef]
- Hernández, S.; Sket, F.; Molina-Aldareguía, J.M.; González, C.; LLorca, J. Effect of curing cycle on void distribution and interlaminar shear strength in polymer-matrix composites. Compos. Sci. Technol. 2011, 71, 1331–1341. [Google Scholar] [CrossRef]
- An, Z.; Cheng, X.; Zhao, D.; Ma, Y.; Guo, X.; Cheng, Y. Tensile and Compressive Properties of Woven Fabric Carbon Fiber-Reinforced Polymer Laminates Containing Three-Dimensional Microvascular Channels. Polymers 2024, 16, 665. [Google Scholar] [CrossRef] [PubMed]
- Qin, Z.; Yang, K.; Wang, J.; Zhang, L.; Huang, J.; Peng, H.; Xu, J. The effects of geometrical dimensions on the failure of composite-to-composite adhesively bonded joints. J. Adhes. 2021, 97, 1024–1051. [Google Scholar] [CrossRef]
- Zhao, Y.; Kikugawa, G.; Kawagoe, Y.; Shirasu, K.; Okabe, T. Molecular-scale investigation on relationship between thermal conductivity and the structure of crosslinked epoxy resin. Int. J. Heat Mass Transf. 2022, 198, 123429. [Google Scholar] [CrossRef]
- Zhang, J.; Hu, C.; Chen, L.; Kong, Y. Structure, mechanical properties and thermal decomposition of carbon-alloyed TiAlN thin films: A first-principle study. Vacuum 2024, 221, 112952. [Google Scholar] [CrossRef]
- Correia, A.N.; Gaspar, B.M.; Cipriano, G.; Braga, D.F.O.; Baptista, R.; Infante, V. Thermo-Mechanical Characterization of Metal–Polymer Friction Stir Composite Joints—A Full Factorial Design of Experiments. Polymers 2024, 16, 602. [Google Scholar] [CrossRef]
- Elhannani, M.; Madani, K.; Chama, Z.; Legrand, E.; Touzain, S.; Feaugas, X. Influence of the presence of defects on the adhesive layer for the single-lap bonded joint—Part II: Probabilistic assessment of the critical state. Aerosp. Sci. Technol. 2017, 63, 372–386. [Google Scholar] [CrossRef]
- Lu, W.; Zhang, C.; Luan, S. Quantifying effect of overload-induced residual stress behind crack tip on fatigue crack growth. Eng. Fract. Mech. 2023, 292, 109593. [Google Scholar] [CrossRef]
- Mehdikhani, M.; Gorbatikh, L.; Verpoest, I.; Lomov, S.V. Voids in fiber-reinforced polymer composites: A review on their formation, characteristics, and effects on mechanical performance. J. Compos. Mater. 2019, 53, 1579–1669. [Google Scholar] [CrossRef]
- Ma, Y.; Wang, J.; Zhao, Y.; Wei, X.; Ju, L.; Chen, Y. A new vacuum pressure infiltration CFRP method and preparation experimental study of composite. Polymers 2020, 12, 419. [Google Scholar] [CrossRef] [PubMed]
- Chang, T.; Zhan, L.; Tan, W. Void content and interfacial properties of composite laminates under different autoclave cure pressure. Compos. Interfaces 2017, 24, 529–540. [Google Scholar] [CrossRef]


| Factors | Curing Pressure (MPa) | Heating Rate (°C/min) | Heat Preservation Temperature (°C) | Heat Preservation Time (min) | |
|---|---|---|---|---|---|
| Levels | |||||
| 1 | 0.0 | 0.5 | 140 | 120 | |
| 2 | 0.2 | 1 | 160 | 150 | |
| 3 | 0.4 | 3 | 180 | 180 | |
| 4 | 0.6 | 5 | 200 | 210 | |
| Defect Shape | Area Ratio of Defect (%) | Tensile Shear Strength (MPa) | Relative Tensile Shear Strength (%) |
|---|---|---|---|
| NA | NA | 16.33 | 100 |
| Circular | 1 | 15.71 | 96.20 |
| Elliptical | 1 | 15.57 | 95.35 |
| Rectangular | 1 | 15.22 | 93.20 |
| Triangular | 1 | 14.96 | 91.61 |
| Circular | 5 | 15.19 | 93.02 |
| Elliptical | 5 | 14.81 | 90.69 |
| Rectangular | 5 | 14.63 | 89.59 |
| Triangular | 5 | 14.25 | 87.26 |
| Circular | 10 | 15.16 | 92.84 |
| Elliptical | 10 | 14.69 | 89.96 |
| Rectangular | 10 | 14.32 | 87.69 |
| Triangular | 10 | 14.13 | 86.53 |
| Defect Shape | Area Ratio of Defect (%) | Defect Location | Tensile Shear Strength (MPa) | Relative Tensile Shear Strength (%) |
|---|---|---|---|---|
| NA | NA | NA | 16.33 | 100 |
| Circular | 5 | Edge | 13.39 | 82.00 |
| Circular | 5 | 0.25 L | 14.94 | 91.49 |
| Circular | 5 | 0.5 L | 15.19 | 93.02 |
| Circular | 5 | 0.5 D | 15.70 | 96.14 |
| Triangular | 5 | Edge | 13.10 | 80.22 |
| Triangular | 5 | 0.25 L | 13.31 | 81.51 |
| Triangular | 5 | 0.5 L | 14.25 | 87.26 |
| Triangular | 5 | 0.5 D | 14.81 | 90.69 |
| Defect Shape | Area Ratio of Defect in Total (%) | Defect Number | Tensile Shear Strength (MPa) | Relative Tensile Shear Strength (%) |
|---|---|---|---|---|
| NA | NA | NA | 16.33 | 100 |
| Circular | 5 | 1 | 15.19 | 93.02 |
| Circular | 5 | 2 | 14.43 | 88.36 |
| Circular | 5 | 3 | 14.16 | 86.53 |
| Circular | 5 | 4 | 12.74 | 78.02 |
| Triangular | 5 | 1 | 14.25 | 87.26 |
| Triangular | 5 | 2 | 11.92 | 72.99 |
| Triangular | 5 | 3 | 11.61 | 71.10 |
| Triangular | 5 | 4 | 10.87 | 66.56 |
| Defect Shape | Area Ratio of Defect in Total (%) | Penetrating or Non-Penetrating | Tensile Shear Strength (MPa) | Relative Tensile Shear Strength (%) |
|---|---|---|---|---|
| NA | NA | NA | 16.33 | 100 |
| Circular | 5 | penetrating | 14.90 | 91.24 |
| Elliptical | 5 | penetrating | 13.39 | 82.00 |
| Rectangular | 5 | penetrating | 11.37 | 69.63 |
| Triangular | 5 | penetrating | 9.42 | 57.69 |
| Test Number | Curing Pressure (MPa) | Heating Rate (°C/min) | Heat Preservation Temperature (°C) | Heat Preservation Time (min) | Tensile Shear Strength (MPa) |
|---|---|---|---|---|---|
| 1 | 1 | 1 | 1 | 1 | 12.65 |
| 2 | 1 | 2 | 2 | 2 | 15.65 |
| 3 | 1 | 3 | 3 | 3 | 15.25 |
| 4 | 1 | 4 | 4 | 4 | 10.62 |
| 5 | 2 | 1 | 2 | 3 | 16.39 |
| 6 | 2 | 2 | 1 | 4 | 16.45 |
| 7 | 2 | 3 | 4 | 1 | 11.15 |
| 8 | 2 | 4 | 3 | 2 | 15.45 |
| 9 | 3 | 1 | 3 | 4 | 16.61 |
| 10 | 3 | 2 | 4 | 3 | 13.98 |
| 11 | 3 | 3 | 1 | 2 | 14.25 |
| 12 | 3 | 4 | 2 | 1 | 15.89 |
| 13 | 4 | 1 | 4 | 2 | 14.22 |
| 14 | 4 | 2 | 3 | 1 | 17.62 |
| 15 | 4 | 3 | 2 | 4 | 17.34 |
| 16 | 4 | 4 | 1 | 3 | 16.32 |
| Mean value I | 13.54 | 14.97 | 14.92 | 14.33 | |
| Mean value II | 14.86 | 15.92 | 16.32 | 14.89 | |
| Mean value III | 15.18 | 14.50 | 16.23 | 15.49 | |
| Mean value IV | 16.37 | 14.57 | 12.49 | 15.25 | |
| Rang R | 2.83 | 1.35 | 3.82 | 1.16 |
| Factor | Deviation Sum of Squares | Degree | Mean Square | F Value | Salience |
|---|---|---|---|---|---|
| Curing pressure | 16.270 | 3 | 5.423 | 13.19 | 0.031 |
| Heating rate | 5.175 | 3 | 1.725 | 4.20 | 0.135 |
| Heat preservation temperature | 38.195 | 3 | 12.732 | 30.97 | 0.009 |
| Heat preservation time | 3.055 | 3 | 1.018 | 2.48 | 0.238 |
| Error | 1.233 | 3 | 0.411 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, X.; Zhang, M.; Zhan, L.; Ma, B.; Wu, X.; Liu, C.; Xiang, H. Effects of Curing Defects in Adhesive Layers on Carbon Fiber–Quartz Fiber Bonded Joint Performance. Polymers 2024, 16, 1406. https://doi.org/10.3390/polym16101406
Yang X, Zhang M, Zhan L, Ma B, Wu X, Liu C, Xiang H. Effects of Curing Defects in Adhesive Layers on Carbon Fiber–Quartz Fiber Bonded Joint Performance. Polymers. 2024; 16(10):1406. https://doi.org/10.3390/polym16101406
Chicago/Turabian StyleYang, Xiaobo, Miaomiao Zhang, Lihua Zhan, Bolin Ma, Xintong Wu, Cong Liu, and He Xiang. 2024. "Effects of Curing Defects in Adhesive Layers on Carbon Fiber–Quartz Fiber Bonded Joint Performance" Polymers 16, no. 10: 1406. https://doi.org/10.3390/polym16101406
APA StyleYang, X., Zhang, M., Zhan, L., Ma, B., Wu, X., Liu, C., & Xiang, H. (2024). Effects of Curing Defects in Adhesive Layers on Carbon Fiber–Quartz Fiber Bonded Joint Performance. Polymers, 16(10), 1406. https://doi.org/10.3390/polym16101406







