Analysis of Water-Based Polyurethane Properties in the Ballistic Behavior of Ultra-High Molecular Weight Polyethylene Fiber Composites
Abstract
1. Introduction
2. Materials and Methods
2.1. Materials
2.2. Methods
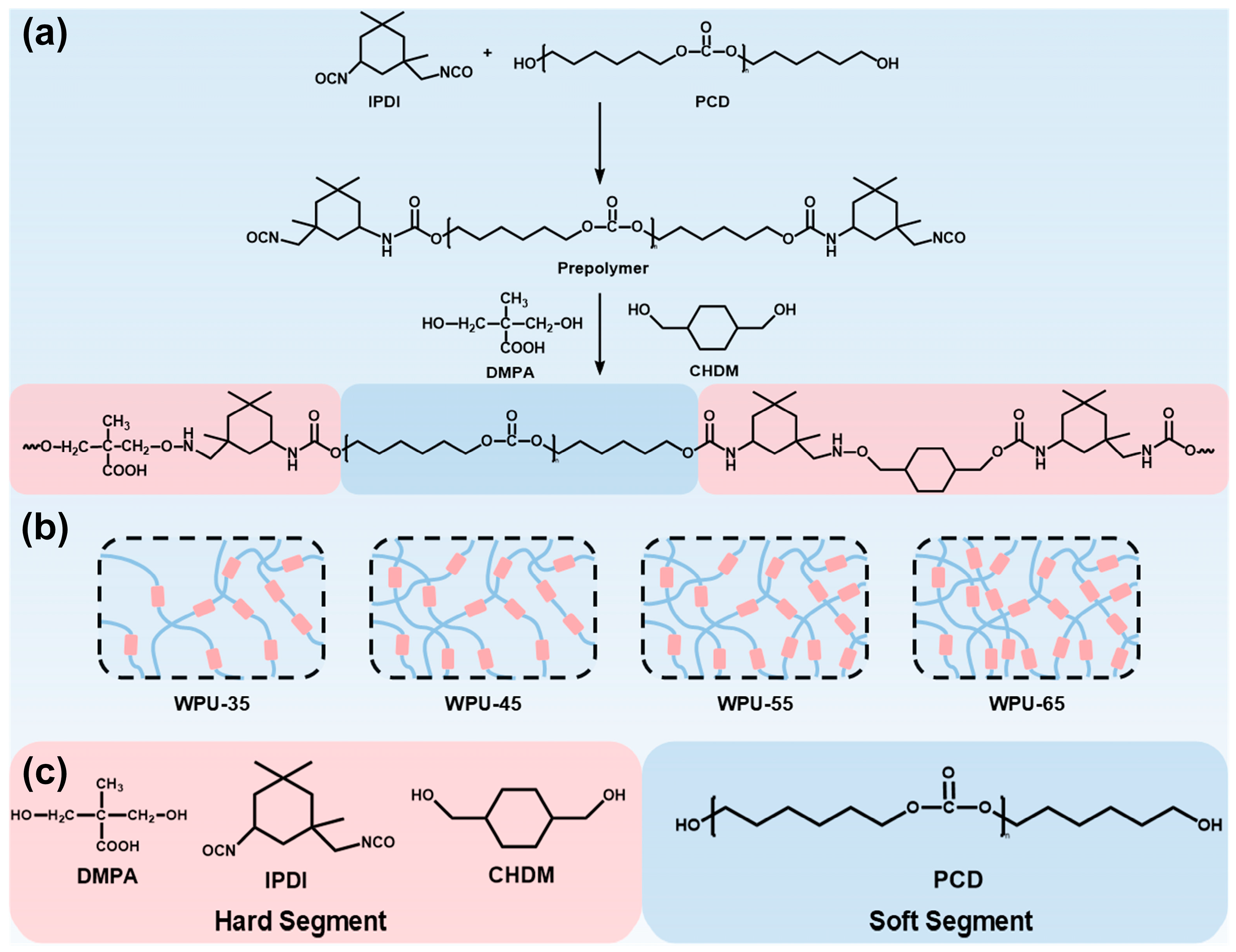
2.2.1. Preparation of WPU
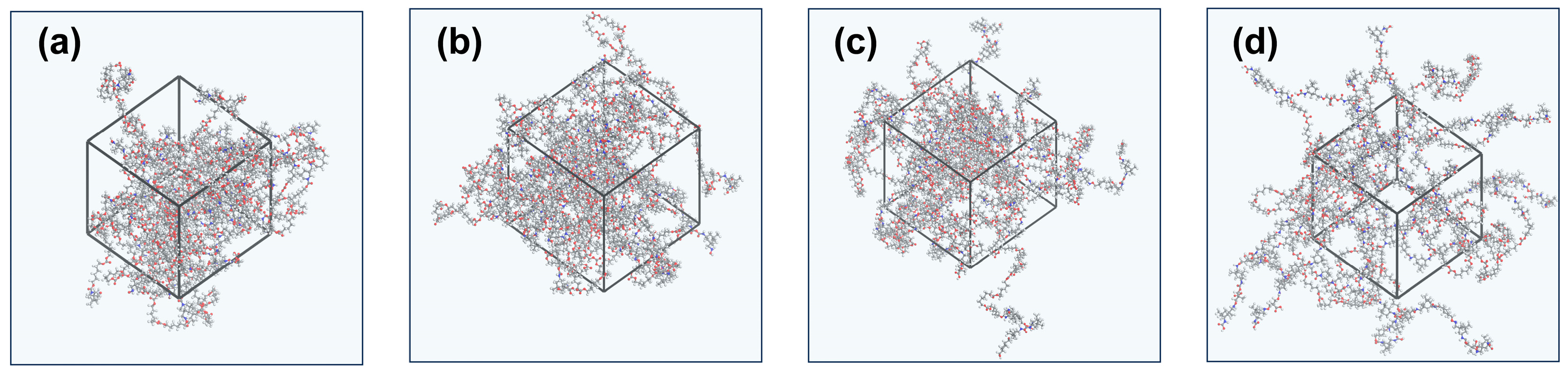
2.2.2. Molecular Dynamics Simulation of WPU-HS
2.2.3. Characterization of WPU-HS
2.2.4. Preparation of WPU-HS/UHMWPE Composites
2.2.5. Characterization of Quasi-Static Mechanical Properties of WPU-HS/UHMWPE Composites
2.2.6. Ballistic Performance Testing of WPU-HS/UHMWPE Composites
2.2.7. Morphological Analysis of WPU-HS/UHMWPE Composites
3. Results and Discussion
3.1. Molecular Dynamics Simulation Results of WPU-HS
3.2. Chemical Structure, Thermal, and Mechanical Properties of WPU-HS
3.2.1. Molecular Weight Testing of WPU-HS
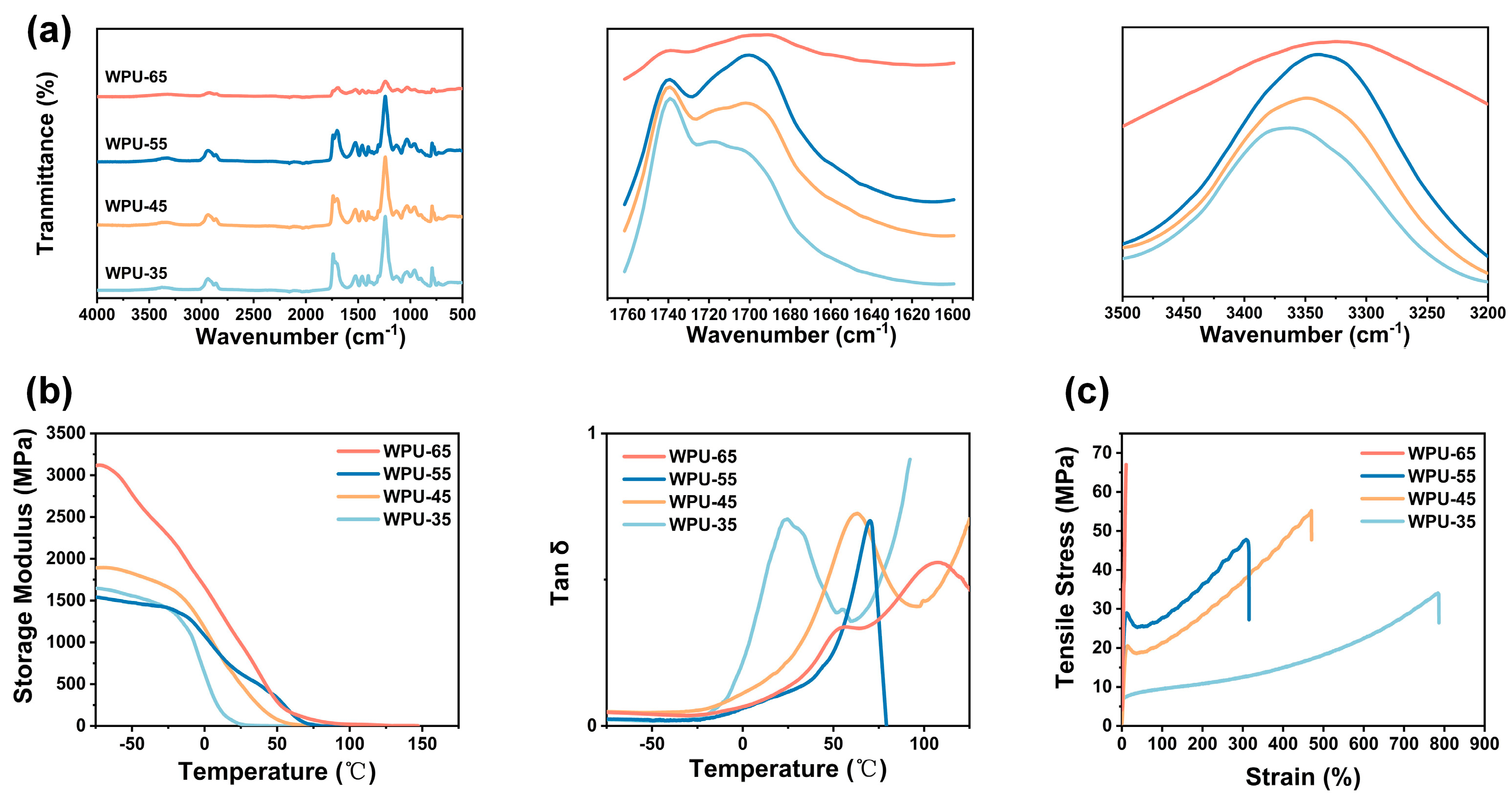
3.2.2. Fourier Transform Infrared Spectroscopy (FTIR) Analysis of WPU-HS
3.2.3. Differential Scanning Calorimetry (DSC) Analysis of WPU-HS
3.2.4. Dynamic Mechanical Analysis (DMA) of WPU-HS
3.2.5. Mechanical Property Analysis of WPU-HS
3.3. Quasi-Static Mechanical Properties of WPU-HS/UHMWPE Composites
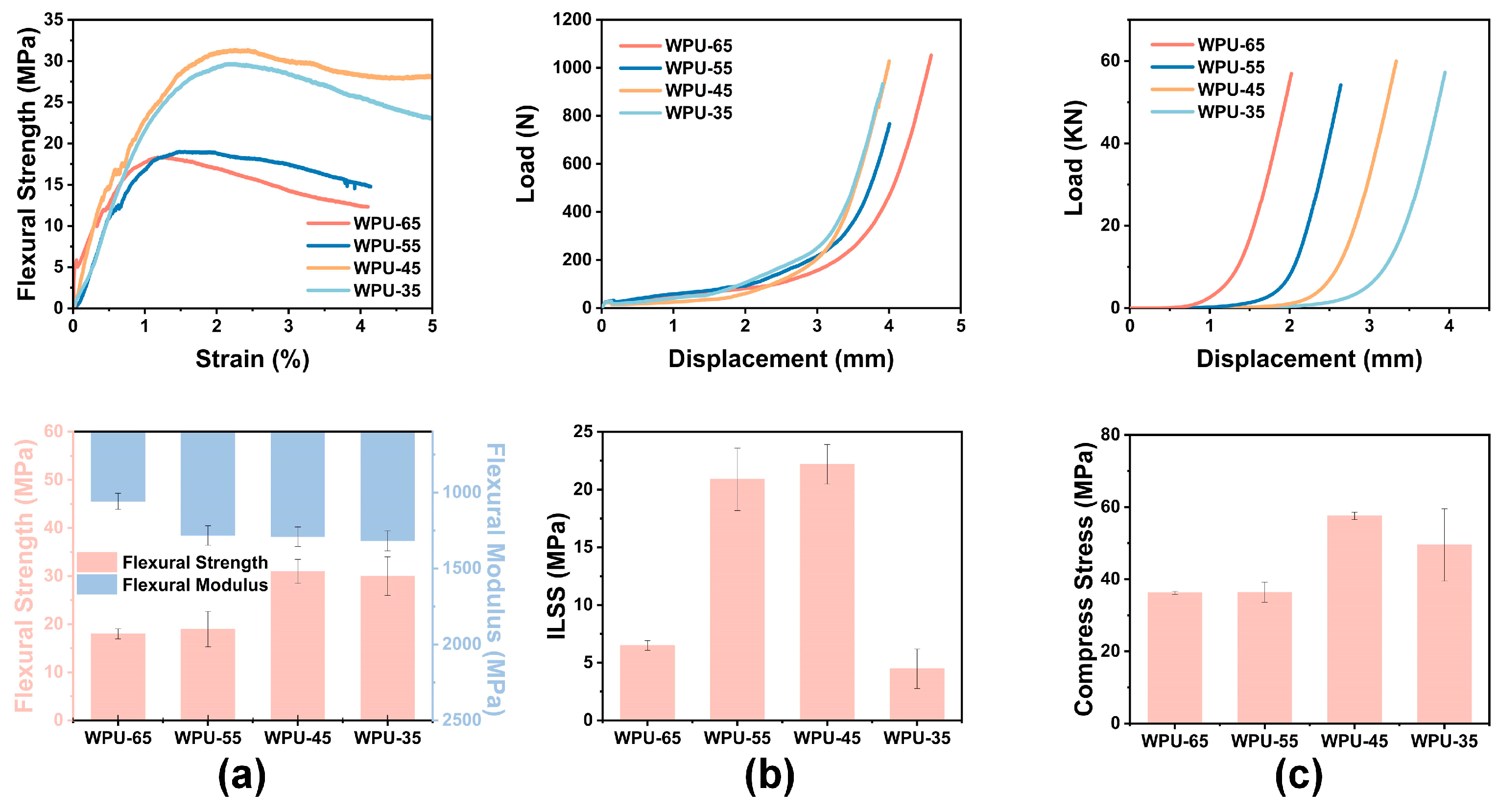
3.3.1. Quasi-Static Flexural Properties of WPU-HS/UHMWPE Composites
3.3.2. Interlaminar Shear Strength of WPU-HS/UHMWPE Composites
3.3.3. Compressive Strength of WPU-HS/UHMWPE Composites
3.3.4. Model-I Interlaminar Fracture Toughness of WPU-HS/UHMWPE Composites
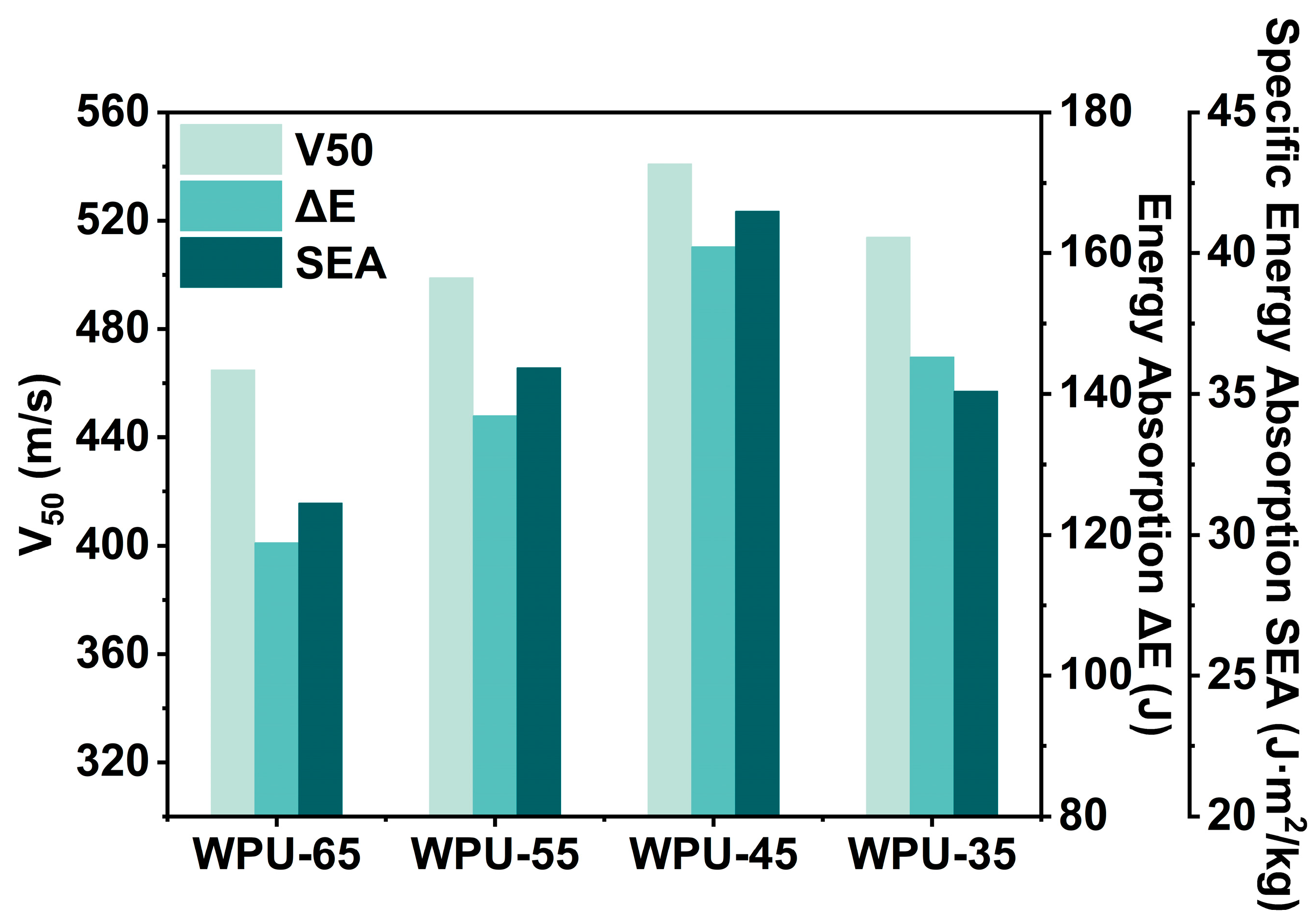
3.4. Ballistic Performance of WPU-HS/UHMWPE Composites
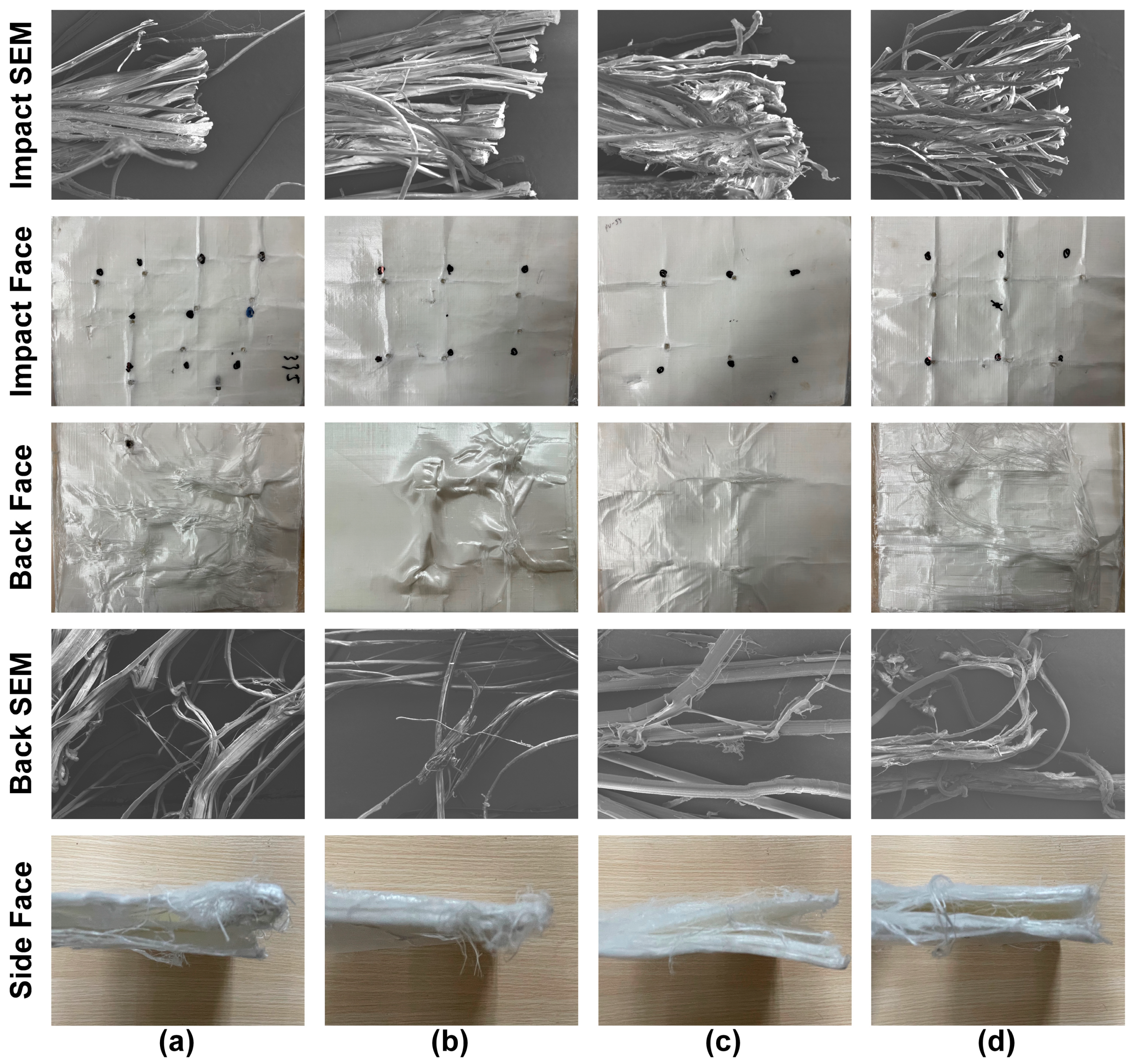
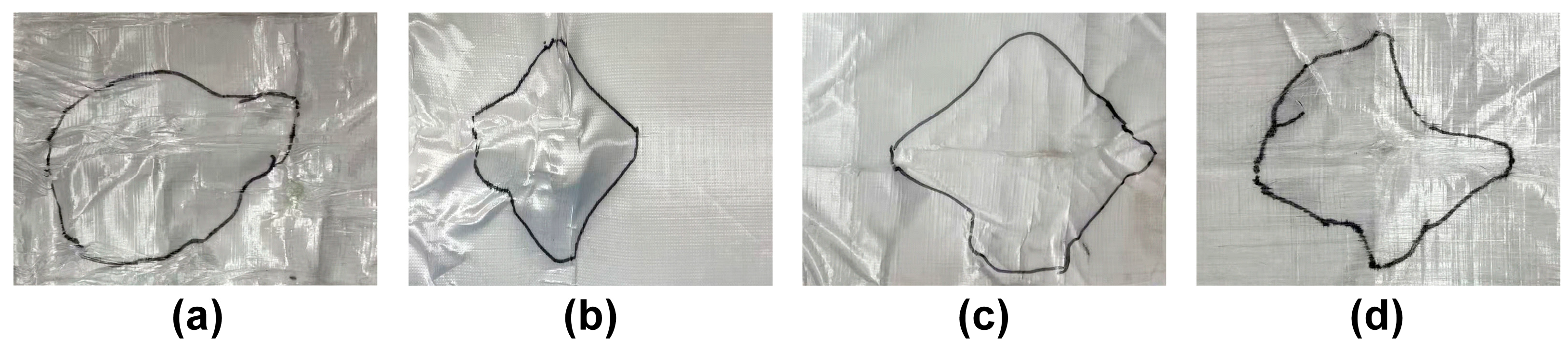
3.5. Microscopic Morphology Analysis of WPU-HS/UHMWPE Composites
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Jiang, Y.; Qian, K.; Zhang, Y.; Xia, Y.; Xiong, Z.; Zhang, Z.; Yu, K. Experimental characterisation and numerical simulation of ballistic penetration of columnar ceramic/fiber laminate composite armor. Mater. Des. 2022, 224, 111394. [Google Scholar]
- Ma, Y.; Wang, J.; Zhao, G.; Liu, Y. New insights into the damage assessment and energy dissipation weight mechanisms of ceramic/fiber laminated composites under ballistic impact. Ceram. Int. 2023, 49, 21966–21977. [Google Scholar]
- Pengcheng, H.; Yuansheng, C.; Pan, Z.; Jun, L.; Haifu, Y.; Jianyong, C. A metal/UHMWPE/SiC multi-layered composite armor against ballistic impact of flat-nosed projectile. Ceram. Int. 2021, 47, 22497–22513. [Google Scholar]
- Kwon, D.-J.; Kim, N.-S.-R.; Jang, Y.-J.; Choi, H.H.; Kim, K.; Kim, G.-H.; Kong, J.; Nam, S.Y. Impacts of thermoplastics content on mechanical properties of continuous fiber-reinforced thermoplastic composites. Compos. Part B Eng. 2021, 216, 108859. [Google Scholar]
- Claus, J.; Santos, R.A.M.; Gorbatikh, L.; Swolfs, Y. Effect of matrix and fibre type on the impact resistance of woven composites. Compos. Part B Eng. 2020, 183, 107736. [Google Scholar] [CrossRef]
- Yao, S.-S.; Jin, F.-L.; Rhee, K.Y.; Hui, D.; Park, S.-J. Recent advances in carbon-fiber-reinforced thermoplastic composites: A review. Compos. Part B Eng. 2018, 142, 241–250. [Google Scholar] [CrossRef]
- Boria, S.; Scattina, A.; Belingardi, G. Impact behavior of a fully thermoplastic composite. Compos. Struct. 2017, 167, 63–75. [Google Scholar]
- Crouch, I.G. Body armour—New materials, new systems. Def. Technol. 2019, 15, 241–253. [Google Scholar]
- Wang, Z.; Zhang, H.; Dong, Y.; Zhou, H.; Huang, G. Ballistic performance and protection mechanism of aramid fabric modified with polyethylene and graphene. Int. J. Mech. Sci. 2023, 237, 107772. [Google Scholar]
- Wang, M.; Zhong, L.; Cao, H.; Chen, H.; Huang, X. Research on Bending and Ballistic Performance of Three-Dimensional Ply-to-Ply Angle Interlock Kevlar/EP Armor Material. Materials 2022, 15, 6994. [Google Scholar] [CrossRef]
- Xiao, K.; Zhang, P.; Hu, D.; Huang, C.; Wu, X. Micron-Thick Interlocked Carbon Nanotube Films with Excellent Impact Resistance via Micro-Ballistic Impact. Small 2023, 19, 2302403. [Google Scholar] [CrossRef]
- Lei, X.; Xiao, K.; Wu, X.; Huang, C. Dynamic mechanical properties of several high-performance single fibers. Materials 2021, 14, 3574. [Google Scholar] [CrossRef] [PubMed]
- Fejdyś, M.; Kośla, K.; Kucharska-Jastrząbek, A.; Landwijt, M. Hybride composite armour systems with advanced ceramics and ultra-high molecular weight polyethylene (UHMWPE) fibres. Fibres Text. East. Eur. 2016, 24, 79–89. [Google Scholar] [CrossRef]
- Tabiei, A.; Nilakantan, G. Ballistic impact of dry woven fabric composites: A review. Appl. Mech. Rev. 2008, 61, 010801. [Google Scholar] [CrossRef]
- Wang, H.; Weerasinghe, D.; Hazell, P.J.; Mohotti, D.; Morozov, E.V.; Escobedo-Diaz, J.P. Ballistic impact response of flexible and rigid UHMWPE textile composites: Experiments and simulations. Def. Technol. 2023, 22, 37–53. [Google Scholar] [CrossRef]
- Wang, H.; Hazell, P.J.; Shankar, K.; Morozov, E.V.; Escobedo, J.P. Impact behaviour of Dyneema® fabric-reinforced composites with different resin matrices. Polym. Test. 2017, 61, 17–26. [Google Scholar] [CrossRef]
- Mia, R.; Sultana, S.; Bhuiyan, M.S.K.; Islam, M.M. Improving ballistic performance of Kevlar fabrics by resin treatment. J. Text. Inst. 2022, 113, 1603–1626. [Google Scholar] [CrossRef]
- Firouzi, D.; Foucher, D.A.; Bougherara, H. Nylon-Coated Ultra High Molecular Weight Polyethylene Fabric for Enhanced Penetration Resistance. J. Appl. Polym. Sci. 2014, 131, 40350. [Google Scholar] [CrossRef]
- Ahmad, M.R.; Hassim, N.; Ahmad, W.Y.W.; Samsuri, A.; Yahya, M.H.M. Preliminary Investigation on the Ballistic Limit of Ultra High Molecular Weight Polyethylene Unidirectional Coated Fabric System. Fibres Text. East. Eur. 2013, 21, 89–94. [Google Scholar]
- Yan, R.; Zhang, Q.; Shi, B.; Liu, S.; Qin, Z.; Jia, L. Investigation on low-velocity impact and interfacial bonding properties of weft-knitted UHMWPE reinforced composites. J. Ind. Text. 2022, 51, 5370S–5388S. [Google Scholar] [CrossRef]
- Han, F.; Wang, C.; Zhang, Y.; Wang, Z.; Yue, H.; Zong, L.; Wang, J.; Jian, X. Analysis of matrix properties on ballistic mechanisms of aramid fabric composites: From experiment to numerical simulation. Chem. Eng. J. 2023, 478, 146391. [Google Scholar]
- Lässig, T.R.; May, M.; Ulrich, H.; Werner, R. Effect of consolidation pressure on the impact behavior of UHMWPE composites. Compos. Part B Eng. 2018, 147, 47–55. [Google Scholar]
- Das, A.; Mahanwar, P. A brief discussion on advances in polyurethane applications. Adv. Ind. Eng. Polym. Res. 2020, 3, 93–101. [Google Scholar]
- Delebecq, E.; Pascault, J.-P.; Boutevin, B.; Ganachaud, F. On the Versatility of Urethane/Urea Bonds: Reversibility, Blocked Isocyanate, and Non-isocyanate Polyurethane. Chem. Rev. 2013, 113, 80–118. [Google Scholar] [PubMed]
- Islam, M.R.; Beg, M.D.H.; Jamari, S.S. Development of vegetable-oil-based polymers. J. Appl. Polym. Sci. 2014, 131, 40787. [Google Scholar]
- Lin, C.; Tian, Q.; Chen, K.; He, G.; Zhang, J.; Liu, S.; Almásy, L. Polymer bonded explosives with highly tunable creep resistance based on segmented polyurethane copolymers with different hard segment contents. Compos. Sci. Technol. 2017, 146, 10–19. [Google Scholar] [CrossRef]
- Wang, X.; Zhang, J.; Bao, L.; Yang, W.; Zhou, F.; Liu, W. Enhancement of the ballistic performance of aramid fabric with polyurethane and shear thickening fluid. Mater. Des. 2020, 196, 109015. [Google Scholar] [CrossRef]
- Li, X.; Lu, Y.; Wang, H.; Pöselt, E.; Eling, B.; Men, Y. Crystallization of hard segments in MDI/BD-based polyurethanes deformed at elevated temperature and their dependence on the MDI/BD content. Eur. Polym. J. 2017, 97, 423–436. [Google Scholar]
- Fang, Z.; Mu, H.; Sun, Z.; Zhang, K.; Zhang, A.; Chen, J.; Zheng, N.; Zhao, Q.; Yang, X.; Liu, F. 3D printable elastomers with exceptional strength and toughness. Nature 2024, 631, 783–788. [Google Scholar] [CrossRef]
- Sun, L.; Jiang, H. Design, Preparation and Properties of Polyurethane Dispersions via Prepolymer Method. Molecules 2023, 28, 625. [Google Scholar] [CrossRef]
- Xiang, D.; Liu, L.; Liang, Y. Effect of hard segment content on structure, dielectric and mechanical properties of hydroxyl-terminated butadiene-acrylonitrile copolymer-based polyurethane elastomers. Polymer 2017, 132, 180–187. [Google Scholar] [CrossRef]
- Ni, F.; Wang, G.; Zhan, H. Molecular and condition parameters dependent diffusion coefficient of water in poly(vinyl alcohol): A molecular dynamics simulation study. Colloid Polym. Sci. 2017, 295, 859–868. [Google Scholar] [CrossRef]
- Raskovalov, A. A new extension of classical molecular dynamics: An electron transfer algorithm. J. Comput. Chem. 2017, 38, 926–932. [Google Scholar] [CrossRef]
- Boddu, V.M.; Brenner, M.W.; Patel, J.S.; Kumar, A.; Mantena, P.R.; Tadepalli, T.; Pramanik, B. Energy dissipation and high-strain rate dynamic response of E-glass fiber composites with anchored carbon nanotubes. Compos. Part B Eng. 2016, 88, 44–54. [Google Scholar]
- ASTM D7264; Standard Test Method for Flexural Properties of Polymer Matrix Composite Materials. ASTM International: West Conshohocken, PA, USA, 2015.
- ASTM D2344; Standard Test Method for Short-Beam Strength of Polymer Matrix Composite Materials and Their Laminates. ASTM International: West Conshohocken, PA, USA, 2022.
- ASTM D5528; Standard Test Method for Mode I Interlaminar Fracture Toughness of Unidirectional Fiber-Reinforced Polymer Matrix Composites. ASTM International: West Conshohocken, PA, USA, 2007.
- Bolduc, M.; Jager, H. Summary of newly ratified NATO standard AEP 2920, ed. A, V1. In Proceedings of the Personal Armour Systems Symposium PASS 2016 Proceeding Book; NATO Army Armaments Group: Brussels, Belgium, 2016. [Google Scholar]
- Khodadadi, A.; Liaghat, G.; Ahmadi, H.; Bahramian, A.R.; Razmkhah, O. Impact response of Kevlar/rubber composite. Compos. Sci. Technol. 2019, 184, 107880. [Google Scholar] [CrossRef]
- Khodadadi, A.; Liaghat, G.; Bahramian, A.R.; Ahmadi, H.; Anani, Y.; Asemani, S.; Razmkhah, O. High velocity impact behavior of Kevlar/rubber and Kevlar/epoxy composites: A comparative study. Compos. Struct. 2019, 216, 159–167. [Google Scholar]
- Wang, H.; Li, Y.; Zhang, H.; Lang, X.; Wang, X.; Cao, L.; Zong, C. Effects of soft segment molecular weight on the properties of recyclable polyurethanes with low-temperature multiple shape memory. J. Polym. Sci. 2025, 63, 121–132. [Google Scholar]
- Paez-Amieva, Y.; Mateo-Oliveras, N.; Martín-Martínez, J.M. Polyurethanes Made with Blends of Polycarbonates with Different Molecular Weights Showing Adequate Mechanical and Adhesion Properties and Fast Self-Healing at Room Temperature. Materials 2024, 17, 5532. [Google Scholar] [CrossRef]
- Stepto, R.F.T. Dispersity in polymer science (IUPAC Recommendations 2009). Pure Appl. Chem. 2009, 81, 351–353. [Google Scholar]
- Jing, X.; Li, X.; Di, Y.; Zhao, Y.; Wang, J.; Kang, M.; Li, Q. Effect of the amide units in soft segment and urea units in hard segment on microstructures and physical properties of polyurethane elastomer. Polymer 2021, 233, 124205. [Google Scholar] [CrossRef]
- Hu, S.; Shou, T.; Zhao, X.; Wang, Z.; Zhang, S.; Qin, X.; Guo, M.; Zhang, L. Rational design of a novel NDI-based thermoplastic polyurethane elastomer with superior heat resistance. Polymer 2020, 205, 122764. [Google Scholar] [CrossRef]
- Han, F.; Shah, S.A.A.; Liu, X.; Zhao, F.; Xu, B.; Zhang, J.; Cheng, J. Preparation and properties of self-healing cross-linked polyurethanes based on blocking and deblocking reaction. React. Funct. Polym. 2019, 144, 104347. [Google Scholar] [CrossRef]
- McCreath, S.; Boinard, P.; Boinard, E.; Gritter, P.; Liggat, J.J. High clarity polyurethane laminating adhesives based on poly (propylene glycol). Effect of hard segment on microphase morphology, haze and adhesion. Int. J. Adhes. Adhes. 2022, 117, 103168. [Google Scholar] [CrossRef]
- Cao, Z.; Zhou, Q.; Jie, S.; Li, B.-G. High cis-1,4 Hydroxyl-Terminated Polybutadiene-Based Polyurethanes with Extremely Low Glass Transition Temperature and Excellent Mechanical Properties. Ind. Eng. Chem. Res. 2016, 55, 1582–1589. [Google Scholar] [CrossRef]
- Hu, S.; Shou, T.; Guo, M.; Wang, R.; Wang, J.; Tian, H.; Qin, X.; Zhao, X.; Zhang, L. Fabrication of New Thermoplastic Polyurethane Elastomers with High Heat Resistance for 3D Printing Derived from 3,3-Dimethyl-4,4′-diphenyl Diisocyanate. Ind. Eng. Chem. Res. 2020, 59, 10476–10482. [Google Scholar] [CrossRef]
- Pernas-Sánchez, J.; Artero-Guerrero, J.A.; Varas, D.; López-Puente, J. Experimental analysis of normal and oblique high velocity impacts on carbon/epoxy tape laminates. Compos. Part A Appl. Sci. Manuf. 2014, 60, 24–31. [Google Scholar] [CrossRef]
- Khazaie, M.; Eslami-Farsani, R.; Saeedi, A. Evaluation of repeated high velocity impact on polymer-based composites reinforced with basalt and Kevlar fibers. Mater. Today Commun. 2018, 17, 76–81. [Google Scholar] [CrossRef]
- Zhang, Y.; Liu, C.; Jia, H.; Qiao, Y.; Dang, X.; Weng, Z.; Chen, Y.; Zou, J.; Jian, X. Effect of electrospun PPENK nanofiber loaded with ZnO nanowires on the mode Ⅱ fracture toughness, flexural properties and ILSS of CF/poly(phthalazinone ether ketone) composites. Compos. Part B Eng. 2022, 244, 110172. [Google Scholar] [CrossRef]
- Jia, H.; Liu, C.; Qiao, Y.; Zhang, Y.; Dang, X.; Chen, Y.; Jian, X. Enhanced interfacial and mechanical properties of basalt fiber reinforced poly(aryl ether nitrile ketone) composites by amino-silane coupling agents. Polymer 2021, 230, 124028. [Google Scholar] [CrossRef]
- López-Puente, J.; Zaera, R.; Navarro, C. Experimental and numerical analysis of normal and oblique ballistic impacts on thin carbon/epoxy woven laminates. Compos. Part A Appl. Sci. Manuf. 2008, 39, 374–387. [Google Scholar] [CrossRef]
- Lyashenko-Miller, T.; Marom, G. Delamination fracture toughness of UHMWPE fibers/polyurethane laminates interleaved with carbon nanotube-reinforced polyurethane films. Polym. Adv. Technol. 2017, 28, 606–612. [Google Scholar]
- Li, W.; Li, Y.; Xiang, D.; Zhao, C.; Wang, B.; Li, H.; Han, H. Simultaneous enhancement of electrical conductivity and interlaminar shear strength of CF/EP composites through MWCNTs doped thermoplastic polyurethane film interleaves. J. Appl. Polym. Sci. 2019, 136, 47988. [Google Scholar] [CrossRef]




| Samples | CED (J/cm3) | Number-Average Molecular Weight (Mn) | Weight-Average Molecular Weight (Mw) | ĐM |
|---|---|---|---|---|
| WPU-35 | 351.7 | 1.22 × 104 | 3.74 × 104 | 3.06 |
| WPU-45 | 330.7 | 1.67 × 104 | 4.81 × 104 | 2.89 |
| WPU-55 | 319.8 | 1.17 × 104 | 2.81 × 104 | 2.39 |
| WPU-65 | 354.4 | 0.74 × 104 | 2.54 × 104 | 3.42 |
| Samples | TgDSC (°C) | TgDMA (°C) | Storage Modulus (25 °C) (MPa) | Tanδ (Max) | Tensile Strength (MPa) | Strain (%) | Young’s Modulus (MPa) |
|---|---|---|---|---|---|---|---|
| WPU-35 | 22.01 | 23.05 | 21.65 | 0.71 | 34.11 | 786.63 | 4.35 |
| WPU-45 | 57.75 | 62.81 | 496.11 | 0.73 | 55.22 | 470.11 | 11.75 |
| WPU-55 | 74.50 | 70.23 | 626.82 | 0.71 | 43.09 | 239.86 | 17.97 |
| WPU-65 | 104.75 | 106.65 | 985.93 | 0.56 | 67.05 | 10.55 | 636.77 |
| Samples | Thickness (mm) | Areal Density (kg/m2) | V50 (m/s) | Energy Absorption (J) | SEA (J·m2/kg) |
|---|---|---|---|---|---|
| WPU-65 | 4.47 | 3.82 | 465 | 118.92 | 31.13 |
| WPU-55 | 4.33 | 3.81 | 499 | 136.95 | 35.94 |
| WPU-45 | 4.27 | 3.88 | 541 | 160.97 | 41.49 |
| WPU-35 | 4.46 | 4.14 | 514 | 145.31 | 35.10 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, S.; Zhai, S.; Piao, M.; Wang, X.; Shi, H.; Li, C. Analysis of Water-Based Polyurethane Properties in the Ballistic Behavior of Ultra-High Molecular Weight Polyethylene Fiber Composites. Polymers 2025, 17, 837. https://doi.org/10.3390/polym17070837
Yang S, Zhai S, Piao M, Wang X, Shi H, Li C. Analysis of Water-Based Polyurethane Properties in the Ballistic Behavior of Ultra-High Molecular Weight Polyethylene Fiber Composites. Polymers. 2025; 17(7):837. https://doi.org/10.3390/polym17070837
Chicago/Turabian StyleYang, Shuhao, Shumao Zhai, Mingxing Piao, Xiao Wang, Haofei Shi, and Chaolong Li. 2025. "Analysis of Water-Based Polyurethane Properties in the Ballistic Behavior of Ultra-High Molecular Weight Polyethylene Fiber Composites" Polymers 17, no. 7: 837. https://doi.org/10.3390/polym17070837
APA StyleYang, S., Zhai, S., Piao, M., Wang, X., Shi, H., & Li, C. (2025). Analysis of Water-Based Polyurethane Properties in the Ballistic Behavior of Ultra-High Molecular Weight Polyethylene Fiber Composites. Polymers, 17(7), 837. https://doi.org/10.3390/polym17070837







