Atomistic Studies of Mechanical Properties of Graphene
Abstract
:1. Introduction
1.1. Brief Overview of Graphene
1.2. Current Status of Theoretical and Numerical Study
1.3. Organization of Review
2. Mechanical Response to In-Plane Tensile Load
2.1. Mechanical Behavior of Monolayer Graphene at Small Deformation


| Reference | Simulation Method | Elastic Modulus (TPa) | Poisson’s Ratio |
|---|---|---|---|
| Liu et al. [36] | DFT | 1.05 | 0.186 |
| Van Lier et al [38] | DFT | 1.11 | – |
| Konstantinova [39] | DFT | 1.24 ± 0.01 | – |
| Kudin et al [40] | DFT | 1.029 | 0.149 |
| Sanchez-Portal [59] | DFT | 1.07 | 0.14–0.19 |
| Hernández [60] | TB | 1.206 | – |
| Gupta [61] | MD (Brenner potential) | 1.272 | 0.147 |
| Bao [62] | MD(REBO) | 1.026 | – |
| Meo [63] | MM (Morse potential) | 0.945 | – |
| Chang [64] | MM (Morse potential) | 1.06 | 0.16 |
| Zhao [14] | MD (AIREBO) | 1.01 ± 0.03 | 0.21 ± 0.01 |
| Zhao [14] | TB | 0.91 | – |
| Hajgato [65] | DFT | 1.05 | – |
| Hemmasizadeh [66] | MM/CM | 0.939 | – |
| Terdalkar [67] | MM (AIREBO) | 0.84 | – |
| Zheng [16] | MD (AIREBO) | 0.99 ± 0.04 | – |
| Lu [30] | MD (REBO) | 0.725 | 0.398 |
| Shen [68] | MD (modified AIREBO) | 1.025 | – |
| Zhang [17] | MD (AIREBO) | 0.995 | – |
| Pei [55] | MD (AIREBO) | 0.893 (ac)/0.832 (zz) | – |
| Wei [35] | DFT | 1.039 | 0.169 |
| Cadelano [34] | TB | 0.931 | 0.31 |
| Reddy [47] | MM (Tersoff–Brenner) | 0.669 | 0.416 |
| Jiang [69] | MD (COMPASS) | 1.0322 | – |
| Neek-Amal [46] | MD, bilayer indentation (Brenner potential) | 0.8 | – |
| Neek-Amal [70] | MD indentation (Brenner potential) | 0.501 ± 0.032 | – |
| Zhou [21] | MM (COMPASS ) | 1.167 | 0.456 |
| Zhou [24] | MM indentation (COMPASS ) | 1.19 | – |
2.2. Mechanical Behavior of Monolayer Graphene at Large Deformation
2.3. Intrinsic Strength of Monolayer Graphene
| Reference | Method | Strength (N/m) σint | Strain εint |
|---|---|---|---|
| Lee et al. [4] | Experiment | 42 ± 4 | 0.25 |
| Liu et al. [36] | DFT | 31.1 (zz) 30.3 (ac) | 0.30 (zz) 0.21 (ac) |
| Zhao [14] | AIREBO | 34.5 (ac) 29.4 (zz) | 0.22 (zz) 0.14 (ac) |
| Marianetti [37] | DFT | 25.7 | – |
| Zheng [16] | REBO | 35.8 (zz) | 0.23 (zz) |
| Lu [19] | REBO | 28.1 (zz) 25.9 (ac) | 0.33 (zz) 0.2 (ac) |
| Zhang [17] | AIREBO | 35.2 (zz) 30.6 (ac) | 0.21 (zz) 0.14 (ac) |
| Pei [55] | AIREBO | 39.5 (zz) 35.0 (ac) | 0.30 (zz) 0.19 (ac) |
| Wei [35] | DFT | 31.0 (zz) 28.9 (ac) | 0.25 (zz) 0.19 (ac) |
| Wei [71] | FEM | 30.1 | 0.23 |
| Cadelano [34] | TB | 35.0 (zz) 51.8 (ac) | 0.22 (zz) 0.33 (ac) |



3. Mechanical Response to Free Standing Indentation Load

3.1. Mechanical Behavior of Monolayer Graphene to Small Deformation


3.2. Mechanical Behavior of Monolayer Graphene to Large Deformation


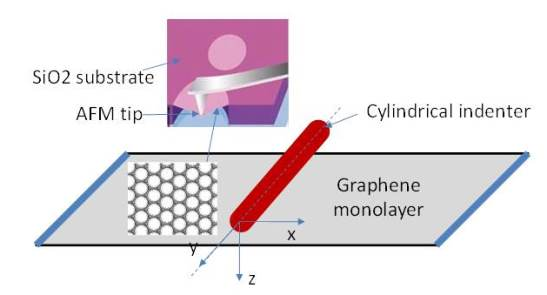
3.3. True Boundary Condition in Free Standing Indentation of Graphene


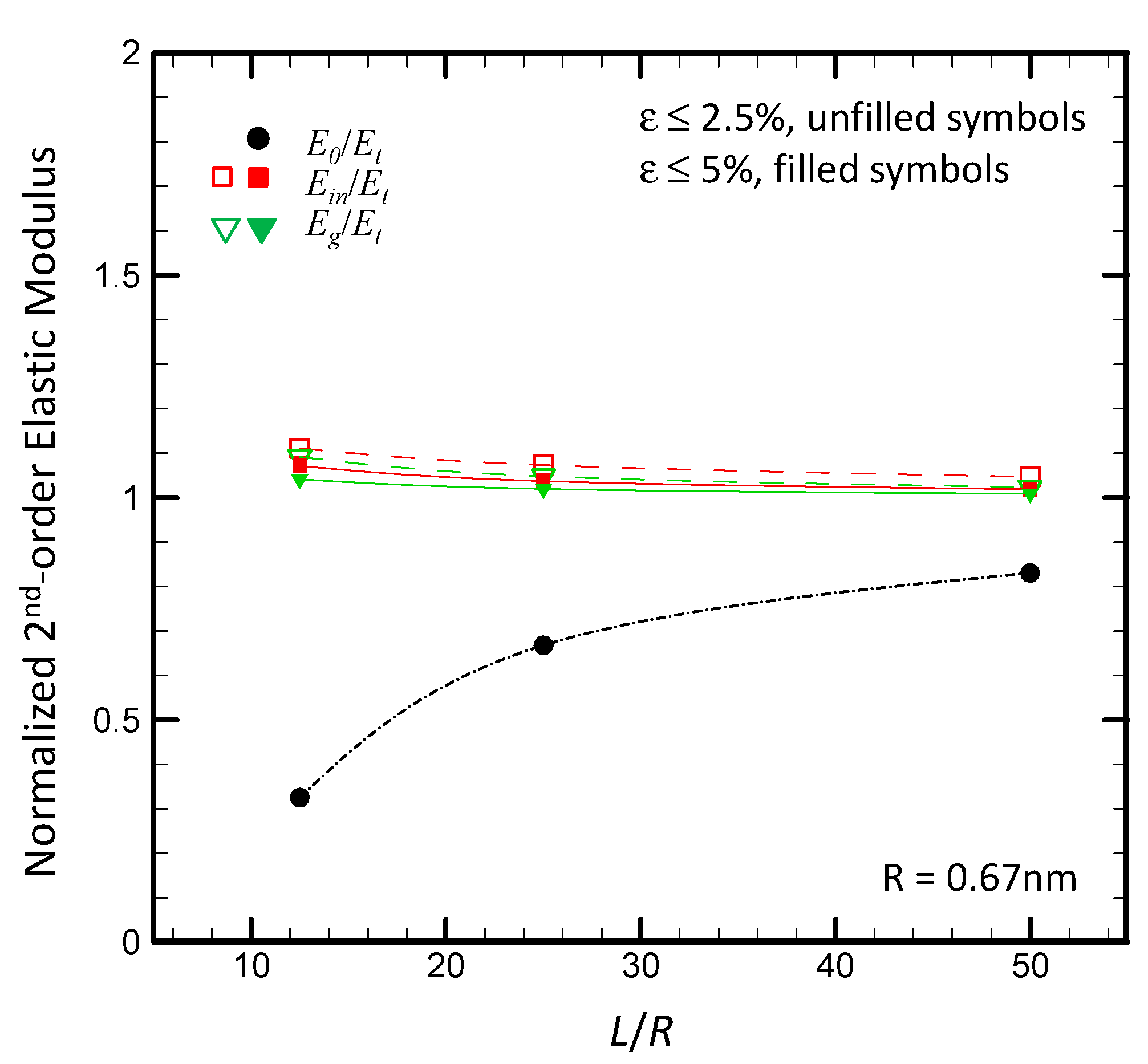
3.4. Pre-Strain Effect on the Indentation Response of Graphene


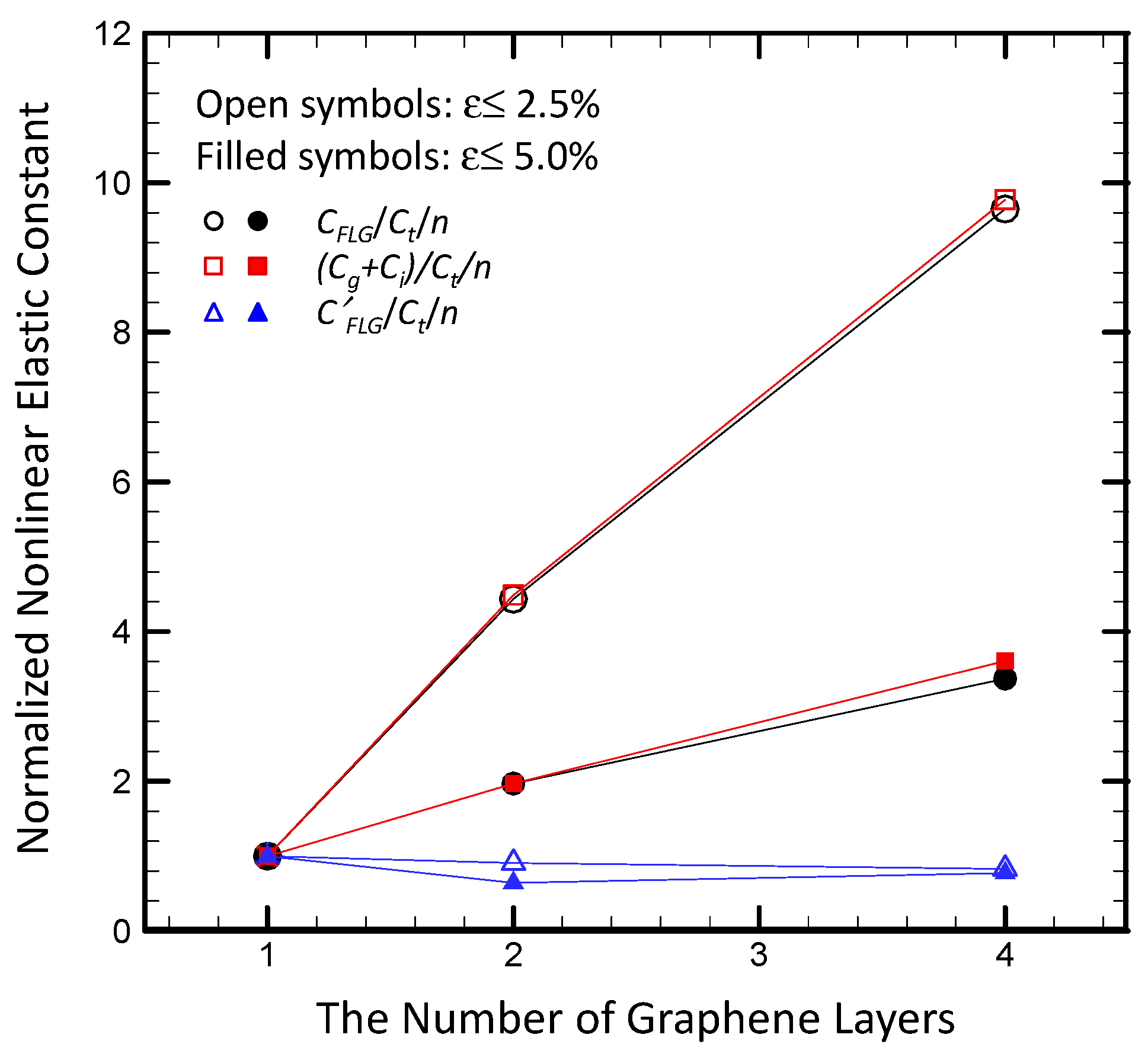
3.5. Indentation Response of Few-Layer Graphene



4. Other Effects on the Mechanical Properties of Graphene
5. Conclusions
Acknowledgments
Conflicts of Interest
References
- Balandin, A.A.; Ghosh, S.; Bao, W.Z.; Calizo, I.; Teweldebrhan, D.; Miao, F.; Lau, C.N. Superior thermal conductivity of single-layer graphene. Nano Lett. 2008, 8, 902–907. [Google Scholar]
- Bolotin, K.I.; Sikes, K.J.; Jiang, Z.; Klima, M.; Fudenberg, G.; Hone, J.; Kim, P.; Stormer, H.L. Ultrahigh electron mobility in suspended graphene. Solid State Commun. 2008, 146, 351–355. [Google Scholar] [CrossRef]
- Steurer, P.; Wissert, R.; Thomann, R.; Mulhaupt, R. Functionalized graphenes and thermoplastic nanocomposites based upon expanded graphite oxide. Macromol. Rapid Commun. 2009, 30, 316–327. [Google Scholar] [CrossRef] [PubMed]
- Lee, C.; Wei, X.D.; Kysar, J.W.; Hone, J. Measurement of the elastic properties and intrinsic strength of monolayer graphene. Science 2008, 321, 385–388. [Google Scholar] [CrossRef] [PubMed]
- Geim, A.K. Graphene: Status and prospects. Science 2009, 324, 1530–1534. [Google Scholar] [CrossRef] [PubMed]
- Novoselov, K.S.; Geim, A.K.; Morozov, S.V.; Jiang, D.; Katsnelson, M.I.; Grigorieva, I.V.; Dubonos, S.V.; Firsov, A.A. Two-dimensional gas of massless dirac fermions in graphene. Nature 2005, 438, 197–200. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Stankovich, S.; Dikin, D.A.; Dommett, G.H.B.; Kohlhaas, K.M.; Zimney, E.J.; Stach, E.A.; Piner, R.D.; Nguyen, S.T.; Ruoff, R.S. Graphene-based composite materials. Nature 2006, 442, 282–286. [Google Scholar] [CrossRef] [PubMed]
- Eda, G.; Fanchini, G.; Chhowalla, M. Large-area ultrathin films of reduced graphene oxide as a transparent and flexible electronic material. Nat. Nanotechnol. 2008, 3, 270–274. [Google Scholar] [CrossRef] [PubMed]
- Soldano, C.; Mahmood, A.; Dujardin, E. Production, properties and potential of graphene. Carbon 2010, 48, 2127–2150. [Google Scholar] [CrossRef]
- Frank, I.W.; Tanenbaum, D.M.; van der Zande, A.M.; McEuen, P.L. Mechanical properties of suspended graphene sheets. J. Vac. Sci. Technol. B 2007, 25, 2558–2561. [Google Scholar] [CrossRef]
- Zhang, Y.P.; Pan, C.X. Measurements of mechanical properties and number of layers of graphene from nano-indentation. Diam. Relat. Mater. 2012, 24, 1–5. [Google Scholar] [CrossRef]
- Lee, J.U.; Yoon, D.; Cheong, H. Estimation of young’s modulus of graphene by raman spectroscopy. Nano Lett. 2012, 12, 4444–4448. [Google Scholar] [CrossRef] [PubMed]
- Lindahl, N.; Midtvedt, D.; Svensson, J.; Nerushev, O.A.; Lindvall, N.; Isacsson, A.; Campbell, E.E.B. Determination of the bending rigidity of graphene via electrostatic actuation of buckled membranes. Nano Lett. 2012, 12, 3526–3531. [Google Scholar] [CrossRef] [PubMed]
- Zhao, H.; Min, K.; Aluru, N.R. Size and chirality dependent elastic properties of graphene nanoribbons under uniaxial tension. Nano Lett. 2009, 9, 3012–3015. [Google Scholar] [CrossRef] [PubMed]
- Zhao, H.; Aluru, N.R. Temperature and strain-rate dependent fracture strength of graphene. J. Appl. Phys. 2010, 108. [Google Scholar] [CrossRef]
- Zheng, Y.P.; Wei, N.; Fan, Z.Y.; Xu, L.Q.; Huang, Z.G. Mechanical properties of grafold: A demonstration of strengthened graphene. Nanotechnology 2011, 22. [Google Scholar] [CrossRef]
- Zhang, Y.Y.; Pei, Q.X.; Wang, C.M. Mechanical properties of graphynes under tension: A molecular dynamics study. Appl. Phys. Lett. 2012, 101. [Google Scholar] [CrossRef]
- Wang, L.; Zhang, Q. Elastic behavior of bilayer graphene under in-plane loadings. Curr. Appl. Phys. 2012, 12, 1173–1177. [Google Scholar] [CrossRef]
- Lu, Q.; Gao, W.; Huang, R. Atomistic simulation and continuum modeling of graphene nanoribbons under uniaxial tension. Model. Simul. Mater. Sci. Eng. 2011, 19. [Google Scholar] [CrossRef]
- Sakhaee-Pour, A. Elastic properties of single-layered graphene sheet. Solid State Commun. 2009, 149, 91–95. [Google Scholar] [CrossRef]
- Zhou, L.X.; Wang, Y.G.; Cao, G.X. Elastic properties of monolayer graphene with different chiralities. J. Phys. Condens. Mater. 2013, 25. [Google Scholar] [CrossRef]
- Zhou, L.X.; Wang, Y.G.; Cao, G.X. Van der waals effect on the nanoindentation response of free standing monolayer graphene. Carbon 2013, 57, 357–362. [Google Scholar] [CrossRef]
- Zhou, L.X.; Wang, Y.G.; Cao, G.X. Boundary condition and pre-strain effects on the free standing indentation response of graphene monolayer. J. Phys. Condens. Mater. 2013, 25. [Google Scholar] [CrossRef]
- Zhou, L.X.; Xue, J.M.; Wang, Y.G.; Cao, G.X. Molecular mechanics simulations of the deformation mechanism of graphene monolayer under free standing indentation. Carbon 2013, 63, 117–124. [Google Scholar] [CrossRef]
- Zhou, L.X.; Wang, Y.G.; Cao, G.X. Estimating the elastic properties of few-layer graphene from the free-standing indentation response. J. Phys. Condens. Mater. 2013, 25. [Google Scholar] [CrossRef]
- Sakhaee-Pour, A.; Ahmadian, M.T.; Naghdabadi, R. Vibrational analysis of single-layered graphene sheets. Nanotechnology 2008, 19. [Google Scholar] [CrossRef]
- Sakhaee-Pour, A. Elastic buckling of single-layered graphene sheet. Comput. Mater. Sci. 2009, 45, 266–270. [Google Scholar] [CrossRef]
- Lu, Z.X.; Dunn, M.L. Van der waals adhesion of graphene membranes. J. Appl. Phys. 2010, 107. [Google Scholar] [CrossRef]
- Wang, Q. Simulations of the bending rigidity of graphene. Phys. Lett. A 2010, 374, 1180–1183. [Google Scholar] [CrossRef]
- Lu, Q.; Huang, R. Nonlinear mechanics of single-atomic-layer graphene sheets. Int. J. Appl. Mech. 2009, 1, 443–467. [Google Scholar] [CrossRef]
- Neek-Amal, M.; Peeters, F.M. Graphene nanoribbons subjected to axial stress. Phys. Rev. B 2010, 82. [Google Scholar] [CrossRef]
- Min, K.; Aluru, N.R. Mechanical properties of graphene under shear deformation. Appl. Phys. Lett. 2011, 98. [Google Scholar] [CrossRef]
- Rajendran, S.; Reddy, C.D. Determination of elastic properties of graphene and carbon-nanotubes using brenner potential: The maximum attainable numerical precision. J. Comput. Theor. Nanosci. 2006, 3, 382–390. [Google Scholar]
- Cadelano, E.; Palla, P.L.; Giordano, S.; Colombo, L. Nonlinear elasticity of monolayer graphene. Phys. Rev. Lett. 2009, 102. [Google Scholar] [CrossRef]
- Wei, X.D.; Fragneaud, B.; Marianetti, C.A.; Kysar, J.W. Nonlinear elastic behavior of graphene: Ab initio calculations to continuum description. Phys. Rev. B 2009, 80. [Google Scholar] [CrossRef]
- Liu, F.; Ming, P.M.; Li, J. Ab initio calculation of ideal strength and phonon instability of graphene under tension. Phys. Rev. B 2007, 76. [Google Scholar] [CrossRef]
- Marianetti, C.A.; Yevick, H.G. Failure mechanisms of graphene under tension. Phys. Rev. Lett. 2010, 105. [Google Scholar] [CrossRef]
- Van Lier, G.; van Alsenoy, C.; van Doren, V.; Geerlings, P. Ab initio study of the elastic properties of single-walled carbon nanotubes and graphene. Chem. Phys. Lett. 2000, 326, 181–185. [Google Scholar] [CrossRef]
- Konstantinova, E.; Dantas, S.O.; Barone, P.M.V.B. Electronic and elastic properties of two-dimensional carbon planes. Phys. Rev. B 2006, 74. [Google Scholar] [CrossRef]
- Kudin, K.N.; Scuseria, G.E.; Yakobson, B.I. C2F, BN, and C nanoshell elasticity from ab initio computations. Phys. Rev. B 2001, 64. [Google Scholar] [CrossRef]
- Wei, Y.J.; Wang, B.L.; Wu, J.T.; Yang, R.G.; Dunn, M.L. Bending rigidity and gaussian bending stiffness of single-layered graphene. Nano Lett. 2013, 13, 26–30. [Google Scholar] [PubMed]
- Faccio, R.; Denis, P.A.; Pardo, H.; Goyenola, C.; Mombru, A.W. Mechanical properties of graphene nanoribbons. J. Phys.Condens. Mater. 2009, 21. [Google Scholar] [CrossRef]
- Porezag, D.; Frauenheim, T.; Kohler, T.; Seifert, G.; Kaschner, R. Construction of tight-binding-like potentials on the basis of density-functional theory-application to carbon. Phys. Rev. B. 1995, 51, 12947–12957. [Google Scholar] [CrossRef]
- Sun, H. Compass: An ab initio force-field optimized for condensed-phase applications—Overview with details on alkane and benzene compounds. J. Phys. Chem. B. 1998, 102, 7338–7364. [Google Scholar] [CrossRef]
- Zhu, J.; He, M.; Qiu, F. Effect of vacancy defects on the young’s modulus and fracture strength of graphene: A molecular dynamics study. Chin. J. Chem. 2012, 30, 1399–1404. [Google Scholar] [CrossRef]
- Neek-Amal, M.; Peeters, F.M. Nanoindentation of a circular sheet of bilayer graphene. Phys. Rev. B 2010, 81. [Google Scholar] [CrossRef]
- Reddy, C.D.; Rajendran, S.; Liew, K.M. Equilibrium configuration and continuum elastic properties of finite sized graphene. Nanotechnology 2006, 17. [Google Scholar] [CrossRef]
- Huang, Y.; Wu, J.; Hwang, K.C. Thickness of graphene and single-wall carbon nanotubes. Phys. Rev. B. 2006, 74. [Google Scholar] [CrossRef]
- Plimpton, S. Fast parallel algorithms for short-range molecular-dynamics. J. Comput. Phys. 1995, 117, 1–19. [Google Scholar] [CrossRef]
- Cao, G.X.; Chen, X. Buckling of single-walled carbon nanotubes upon bending: Molecular dynamics simulations and finite element method. Phys. Rev. B 2006, 73. [Google Scholar] [CrossRef]
- Cao, G.X.; Chen, X. The effects of chirality and boundary conditions on the mechanical properties of single-walled carbon nanotubes. Int. J. Solids Struct. 2007, 44, 5447–5465. [Google Scholar] [CrossRef]
- Cao, G.X.; Chen, X.; Kysar, J.W. Strain sensing of carbon nanotubes: Numerical analysis of the vibrational frequency of deformed single-wall carbon nanotubes. Phys. Rev. B 2005, 72. [Google Scholar] [CrossRef]
- Chen, X.; Cao, G.X. A structural mechanics study of single-walled carbon nanotubes generalized from atomistic simulation. Nanotechnology 2006, 17. [Google Scholar] [CrossRef]
- Shen, X.; Lin, X.Y.; Yousefi, N.; Jia, J.J.; Kim, J.K. Wrinkling in graphene sheets and graphene oxide papers. Carbon 2014, 66, 84–92. [Google Scholar] [CrossRef]
- Pei, Q.X.; Zhang, Y.W.; Shenoy, V.B. Mechanical properties of methyl functionalized graphene: A molecular dynamics study. Nanotechnology 2010, 21. [Google Scholar] [CrossRef]
- Arroyo, M.; Belytschko, T. Finite crystal elasticity of carbon nanotubes based on the exponential cauchy-born rule. Phys. Rev. B. 2004, 69. [Google Scholar] [CrossRef]
- Michel, K.H.; Verberck, B. Theory of the elastic constants of graphite and graphene. Phys. Status. Solidi B 2008, 245, 2177–2180. [Google Scholar] [CrossRef]
- Jiang, J.W.; Wang, J.S.; Li, B.W. Young’s modulus of graphene: A molecular dynamics study. Phys. Rev. B. 2009, 80. [Google Scholar] [CrossRef]
- Sanchez-Portal, D.; Artacho, E.; Soler, J.M.; Rubio, A.; Ordejon, P. Ab initio structural, elastic, and vibrational properties of carbon nanotubes. Phys. Rev. B 1999, 59, 12678–12688. [Google Scholar] [CrossRef]
- Hernandez, E.; Goze, C.; Bernier, P.; Rubio, A. Elastic properties of C and bxcynz composite nanotubes. Phys. Rev. Lett. 1998, 80, 4502–4505. [Google Scholar] [CrossRef]
- Gupta, S.; Dharamvir, K.; Jindal, V.K. Elastic moduli of single-walled carbon nanotubes and their ropes. Phys. Rev. B 2005, 72. [Google Scholar] [CrossRef]
- Bao, W.X.; Zhu, C.C.; Cui, W.Z. Simulation of young’s modulus of single-walled carbon nanotubes by molecular dynamics. Phys. B Condens. Matter. 2004, 352, 156–163. [Google Scholar] [CrossRef]
- Meo, M.; Rossi, M. Prediction of young’s modulus of single wall carbon nanotubes by molecular-mechanics based finite element modelling. Compos. Sci. Technol. 2006, 66, 1597–1605. [Google Scholar] [CrossRef]
- Chang, T.C.; Gao, H.J. Size-dependent elastic properties of a single-walled carbon nanotube via a molecular mechanics model. J. Mech. Phys. Solids 2003, 51, 1059–1074. [Google Scholar] [CrossRef]
- Hajgato, B.; Guryel, S.; Dauphin, Y.; Blairon, J.M.; Miltner, H.E.; van Lier, G.; de Proft, F.; Geerlings, P. Theoretical investigation of the intrinsic mechanical properties of single- and double-layer graphene. J. Phys. Chem. C 2012, 116, 22608–22618. [Google Scholar] [CrossRef]
- Hemmasizadeh, A.; Mahzoon, M.; Hadi, E.; Khandan, R. A method for developing the equivalent continuum model of a single layer graphene sheet. Thin Solid Films 2008, 516, 7636–7640. [Google Scholar] [CrossRef]
- Terdalkar, S.S.; Huang, S.; Yuan, H.Y.; Rencis, J.J.; Zhu, T.; Zhang, S.L. Nanoscale fracture in graphene. Chem. Phys. Lett. 2010, 494, 218–222. [Google Scholar] [CrossRef]
- Shen, Y.K.; Wu, H.A. Interlayer shear effect on multilayer graphene subjected to bending. Appl. Phys. Lett. 2012, 100. [Google Scholar] [CrossRef]
- Jing, N.N.; Xue, Q.Z.; Ling, C.C.; Shan, M.X.; Zhang, T.; Zhou, X.Y.; Jiao, Z.Y. Effect of defects on young’s modulus of graphene sheets: A molecular dynamics simulation. RSC Adv. 2012, 2, 9124–9129. [Google Scholar] [CrossRef]
- Neek-Amal, M.; Peeters, F.M. Linear reduction of stiffness and vibration frequencies in defected circular monolayer graphene. Phys. Rev. B 2010, 81. [Google Scholar] [CrossRef]
- Wei, X.D.; Kysar, J.W. Experimental validation of multiscale modeling of indentation of suspended circular graphene membranes. Int. J. Solids Struct. 2012, 49, 3201–3209. [Google Scholar] [CrossRef]
- Cao, G. Graphene: An anisotropic two-dimensional tensegrity. Carbon 2014, unpublished. [Google Scholar]
- Bunch, J.S.; Verbridge, S.S.; Alden, J.S.; van der Zande, A.M.; Parpia, J.M.; Craighead, H.G.; McEuen, P.L. Impermeable atomic membranes from graphene sheets. Nano Lett. 2008, 8, 2458–2462. [Google Scholar] [CrossRef] [PubMed]
- Han, J.; Ryu, S.; Sohn, D.; Im, S. Mechanical strength characteristics of asymmetric tilt grain boundaries in graphene. Carbon 2014, 68, 250–257. [Google Scholar] [CrossRef]
- Jhon, Y.I.; Zhu, S.E.; Ahn, J.H.; Jhon, M.S. The mechanical responses of tilted and non-tilted grain boundaries in graphene. Carbon 2012, 50, 3708–3716. [Google Scholar] [CrossRef]
- Ovid’ko, I.A.; Sheinerman, A.G. Cracks at disclinated grain boundaries in graphene. J. Phys. D Appl. Phys. 2013, 46. [Google Scholar] [CrossRef]
- Jhon, Y.I.; Chung, P.S.; Smith, R.; Min, K.S.; Yeom, G.Y.; Jhon, M.S. Grain boundaries orientation effects on tensile mechanics of polycrystalline graphene. RSC Adv. 2013, 3, 9897–9903. [Google Scholar] [CrossRef]
- Liu, T.H.; Gajewski, G.; Pao, C.W.; Chang, C.C. Structure, energy, and structural transformations of graphene grain boundaries from atomistic simulations. Carbon 2011, 49, 2306–2317. [Google Scholar] [CrossRef]
- Yi, L.J.; Yin, Z.N.; Zhang, Y.Y.; Chang, T.C. A theoretical evaluation of the temperature and strain-rate dependent fracture strength of tilt grain boundaries in graphene. Carbon 2013, 51, 373–380. [Google Scholar] [CrossRef]
- Wei, Y.J.; Wu, J.T.; Yin, H.Q.; Shi, X.H.; Yang, R.G.; Dresselhaus, M. The nature of strength enhancement and weakening by pentagon-heptagon defects in graphene. Nat. Mater. 2012, 11, 759–763. [Google Scholar] [CrossRef] [PubMed]
- He, L.C.; Guo, S.S.; Lei, J.C.; Sha, Z.D.; Liu, Z.S. The effect of stone-thrower-wales defects on mechanical properties of graphene sheets—A molecular dynamics study. Carbon 2014, 75, 124–132. [Google Scholar] [CrossRef]
- Yazyev, O.V.; Louie, S.G. Topological defects in graphene: Dislocations and grain boundaries. Phys. Rev. B 2010, 81. [Google Scholar] [CrossRef]
- Lee, G.H.; Cooper, R.C.; An, S.J.; Lee, S.; van der Zande, A.; Petrone, N.; Hammerherg, A.G.; Lee, C.; Crawford, B.; Oliver, W.; et al. High-strength chemical-vapor deposited graphene and grain boundaries. Science 2013, 340, 1073–1076. [Google Scholar] [CrossRef] [PubMed]
- Zhang, J.F.; Zhao, J.J.; Lu, J.P. Intrinsic strength and failure behaviors of graphene grain boundaries. ACS Nano 2012, 6, 2704–2711. [Google Scholar] [CrossRef] [PubMed]
© 2014 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution license (http://creativecommons.org/licenses/by/3.0/).
Share and Cite
Cao, G. Atomistic Studies of Mechanical Properties of Graphene. Polymers 2014, 6, 2404-2432. https://doi.org/10.3390/polym6092404
Cao G. Atomistic Studies of Mechanical Properties of Graphene. Polymers. 2014; 6(9):2404-2432. https://doi.org/10.3390/polym6092404
Chicago/Turabian StyleCao, Guoxin. 2014. "Atomistic Studies of Mechanical Properties of Graphene" Polymers 6, no. 9: 2404-2432. https://doi.org/10.3390/polym6092404
APA StyleCao, G. (2014). Atomistic Studies of Mechanical Properties of Graphene. Polymers, 6(9), 2404-2432. https://doi.org/10.3390/polym6092404