A Simple Application for Computing Reference Evapotranspiration with Various Levels of Data Availability—ETo Tool
Abstract
:1. Introduction
2. Conceptual Model and Accuracy Indicators
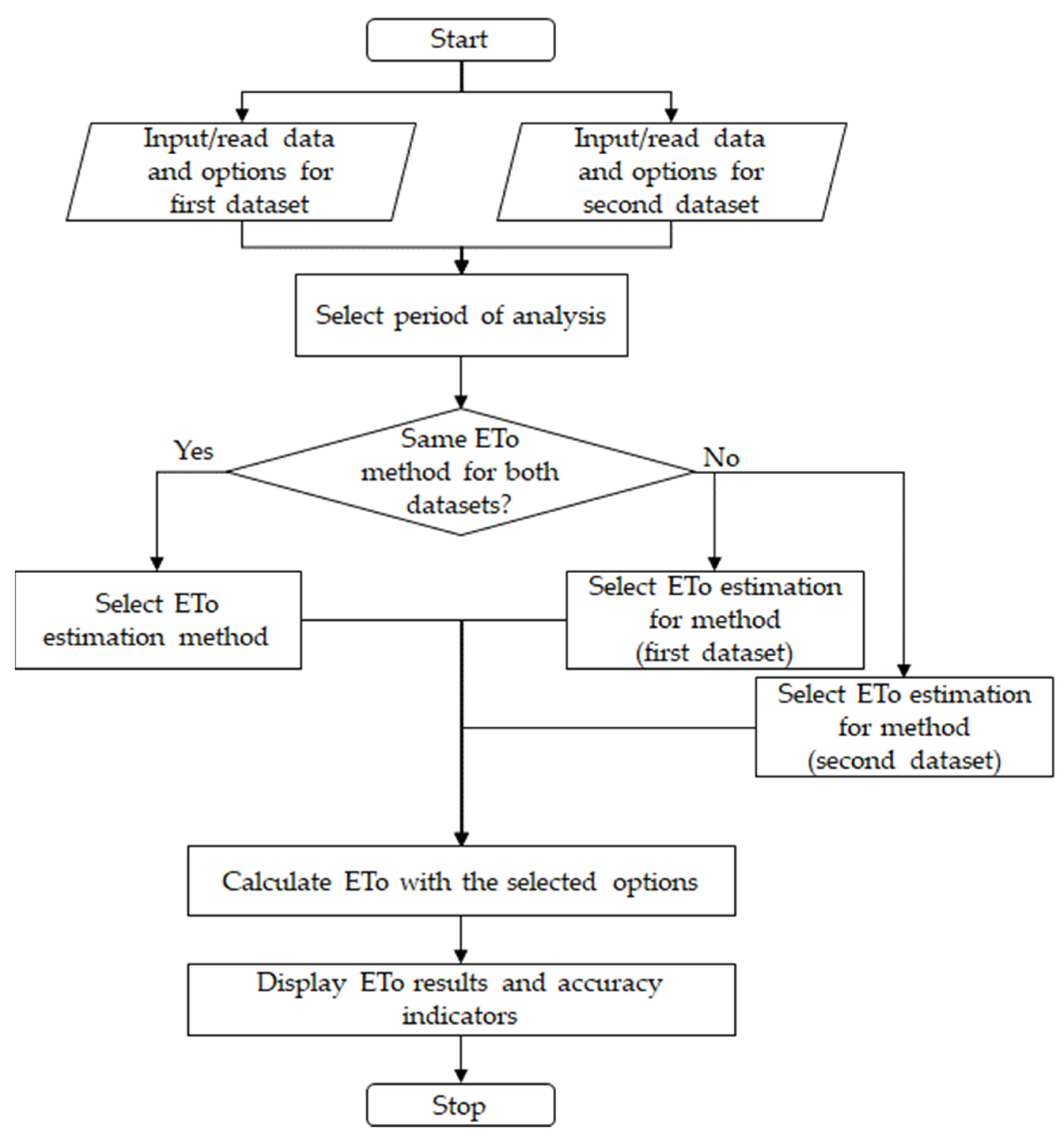
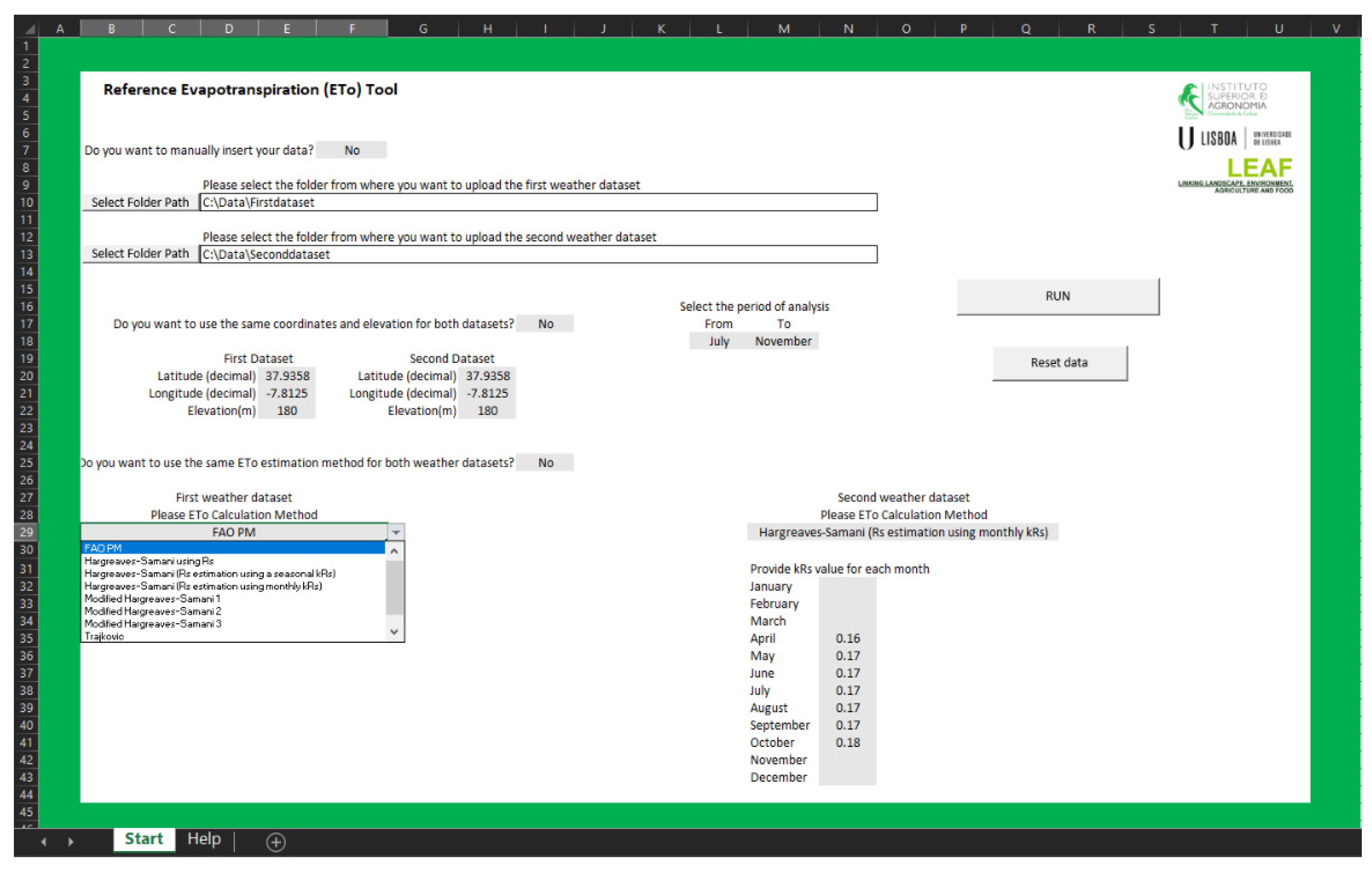
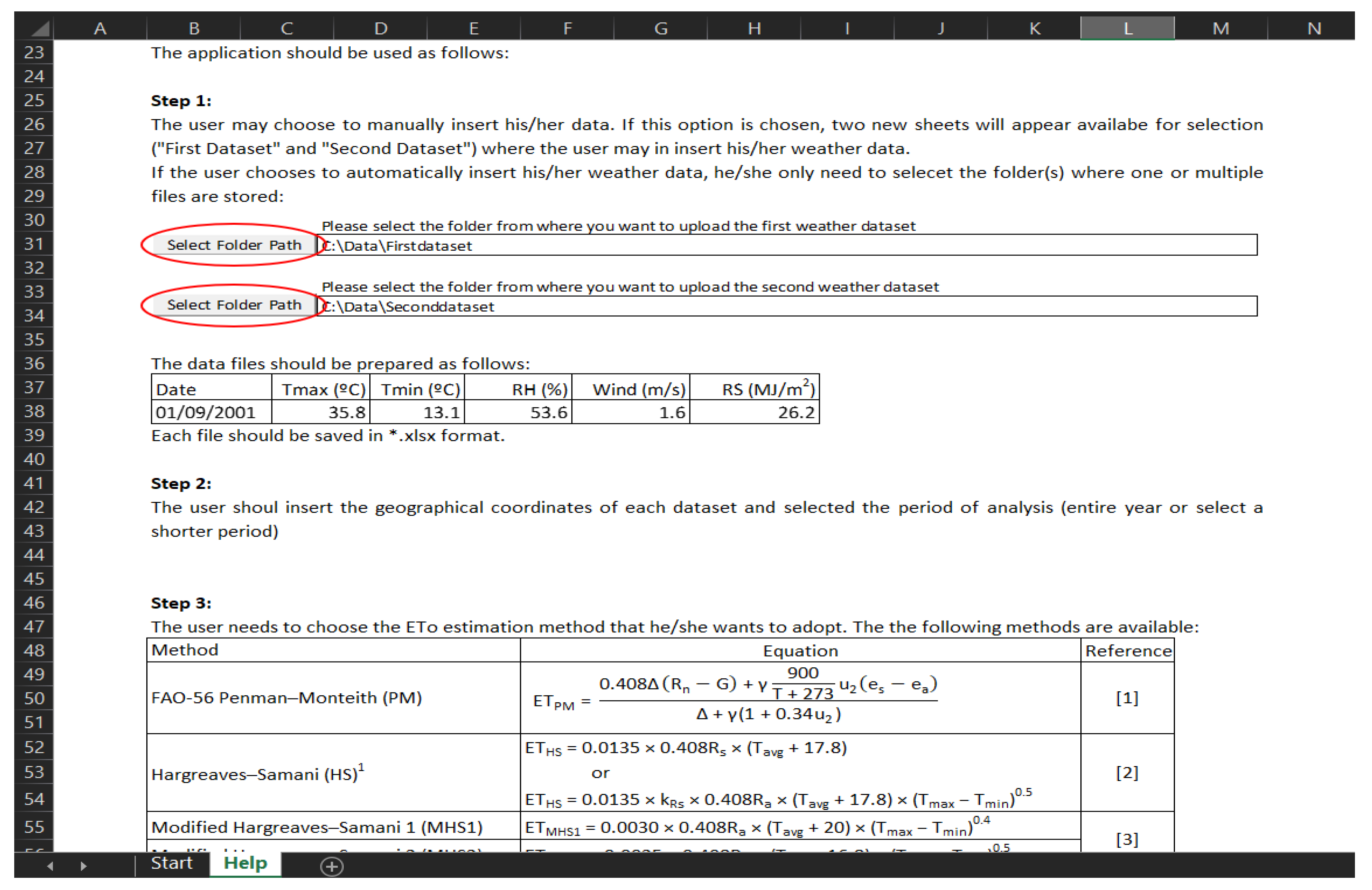
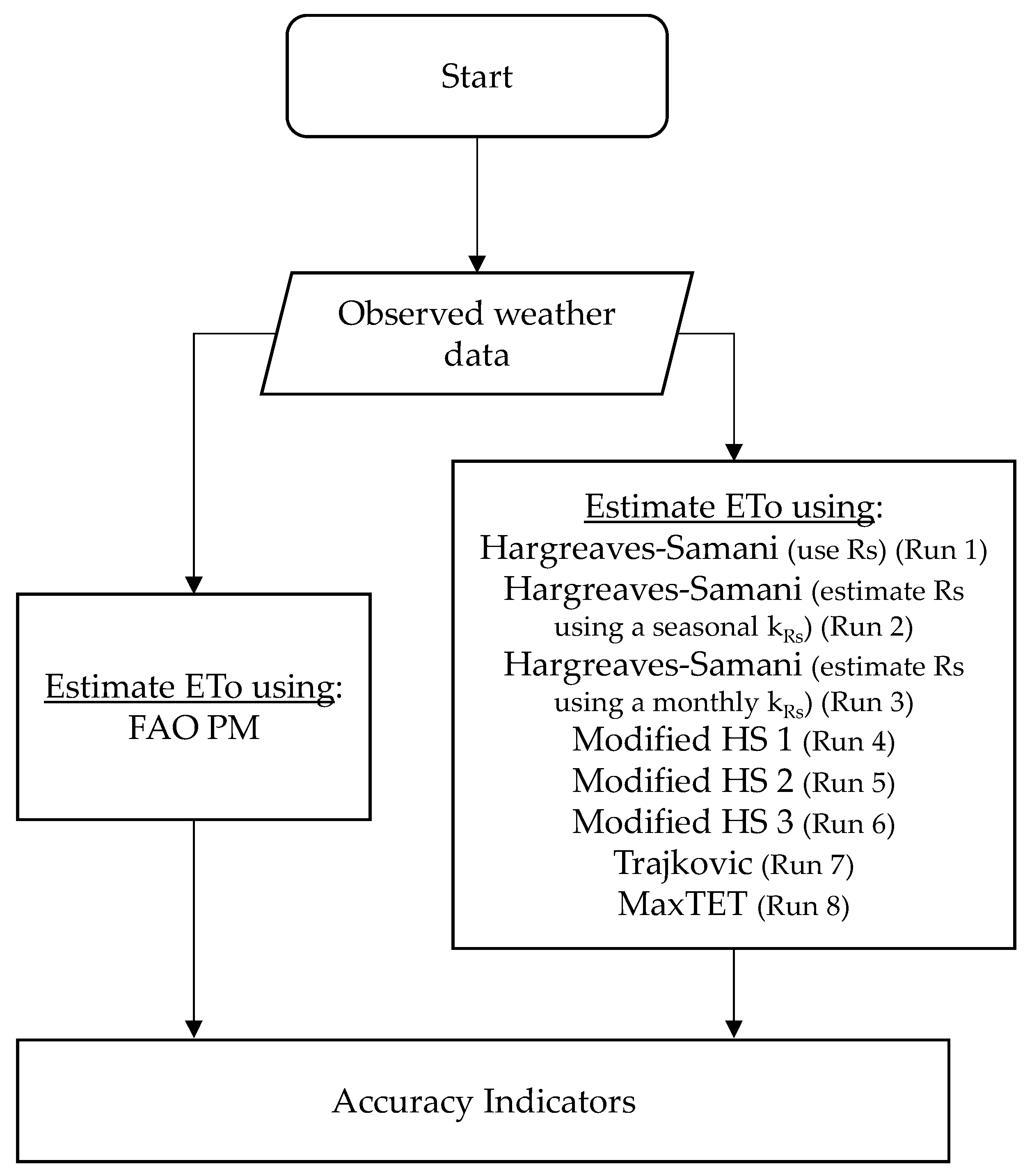
2.1. App Concept
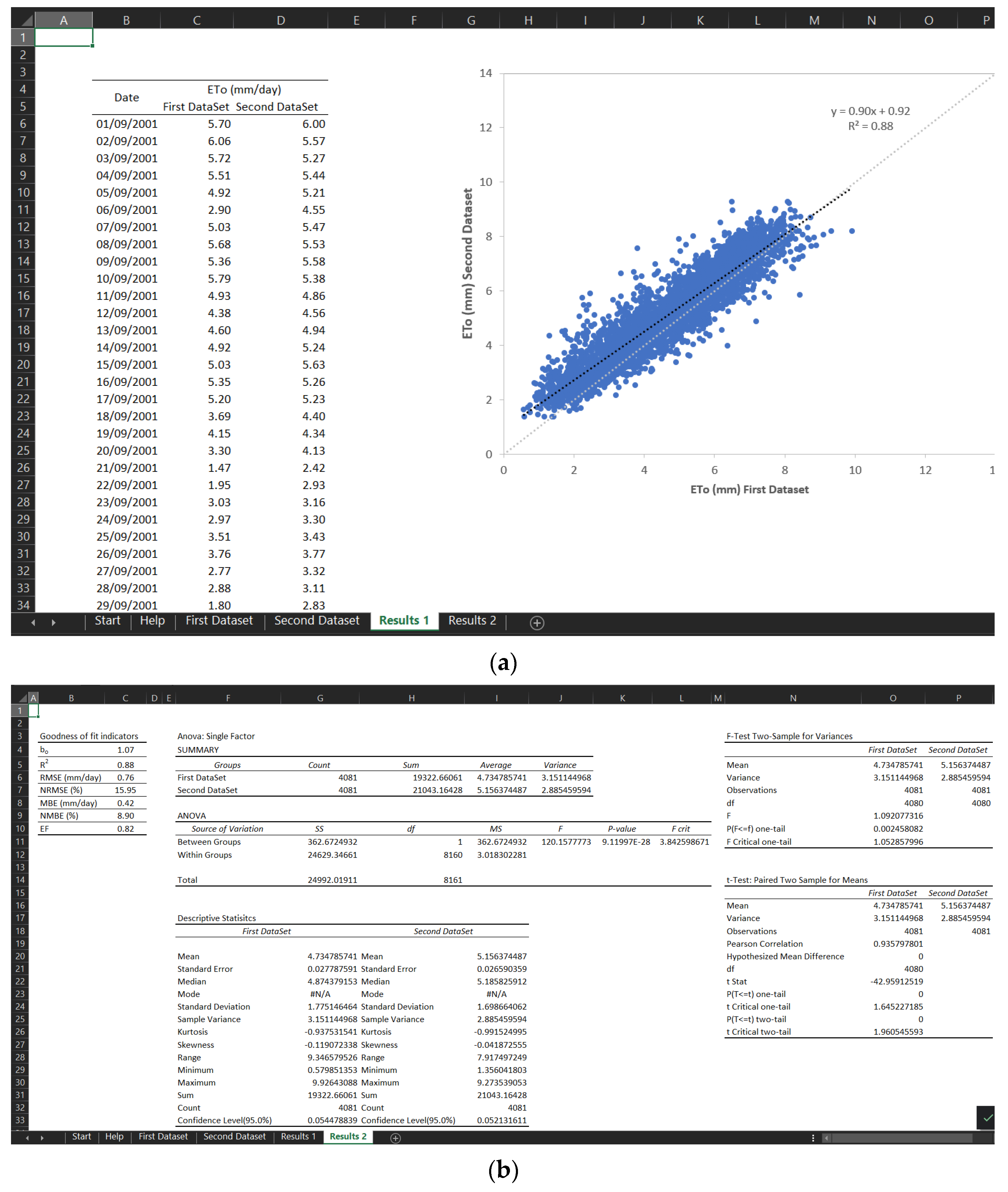
2.2. Accuracy Indicators
- The coefficients of regression and determination, relating the first and second dataset, b and R2, respectively, are defined as:
- The root mean square error, RMSE and its normalization, NRMSE, which characterizes the variance of the estimation error can be defined as:
- The mean bias error, MBE, and its normalization, NMBE, that measures the systematic error between the second dataset and first dataset values can be defined as:
- The Nash and Sutcliffe [36] modelling efficiency, EF, that is the ratio of the mean square error to the variance of the first dataset, subtracted from unity, can be defined as:
3. Example Case
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Doorenbos, J.; Pruitt, W.O. Guidelines for predicting crop-water requirements. In FAO Irrigation and Drainage Paper No. 24, 2nd ed.; FAO: Rome, Italy, 1977; p. 156. [Google Scholar]
- Wright, J.L.; Jensen, M.E. Development and Evaluation of Evapotranspiration Models for Irrigation Scheduling. Trans. ASAE 1978, 21, 0088–0091. [Google Scholar] [CrossRef]
- Jensen, M.E.; Burman, R.D.; Allen, R.G. Evapotranspiration and Irrigation Water Requirements; ASCE Manuals and Reports on Engineering Practices No. 70; American Society of Civil Engineers: New York, NY, USA, 1990; 360p. [Google Scholar]
- Allen, R.G.; Pereira, L.S.; Raes, D.; Smith, M. Crop Evapotranspiration—Guidelines for Computing Crop Water Requirements. Irrigation and Drainage Paper 56; FAO: Rome, Italy, 1998; p. 300. [Google Scholar]
- Allen, R.G.; Wright, J.L.; Pruitt, W.O.; Pereira, L.S.; Jensen, M.E. Water Requirements. In Design and Operation of Farm Irrigation Systems, 2nd ed.; Hoffman, G.J., Evans, R.G., Jensen, M.E., Martin, D.L., Elliot, R.L., Eds.; ASABE: Joseph, MI, USA, 2007; pp. 208–288. [Google Scholar]
- Howell, T.A.; Evett, S.; Tolk, J.A.; Schneider, A.D. Evapotranspiration of Full-, Deficit-Irrigated, and Dryland Cotton on the Northern Texas High Plains. J. Irrig. Drain. Eng. 2004, 130, 277–285. [Google Scholar] [CrossRef]
- Steduto, P.; Hsiao, T.C.; Raes, D.; Fereres, E. AquaCrop—The FAO crop model to simulate yield response to water: I. Concepts and underlying principles. Agron. J. 2009, 101, 426–437. [Google Scholar] [CrossRef] [Green Version]
- Rodrigues, G.C.; Pereira, L.S. Assessing economic impacts of deficit irrigation as related to water productivity and water costs. Biosyst. Eng. 2009, 103, 536–551. [Google Scholar] [CrossRef] [Green Version]
- Paredes, P.; Rodrigues, G.; Alves, I.; Pereira, L. Partitioning evapotranspiration, yield prediction and economic returns of maize under various irrigation management strategies. Agric. Water Manag. 2014, 135, 27–39. [Google Scholar] [CrossRef]
- Allen, R.G.; Clemmens, A.J.; Burt, C.M.; Solomon, K.; O’Halloran, T. Prediction accuracy for project wide evapotranspiration using crop coefficients and reference evapotranspiration. J. Irrig. Drain. Eng. 2005, 131, 24–36. [Google Scholar] [CrossRef] [Green Version]
- Allen, R.G.; Pruitt, W.O.; Wright, J.L.; Howell, T.A.; Ventura, F.; Snyder, R.; Itenfisu, D.; Steduto, P.; Berengena, J.; Yrisarry, J.B.; et al. A recommendation on standardized surface resistance for hourly calculation of reference ETo by the FAO56 Penman-Monteith method. Agric. Water Manag. 2006, 81, 1–22. [Google Scholar] [CrossRef]
- Trajkovic, S. Temperature-Based Approaches for Estimating Reference Evapotranspiration. J. Irrig. Drain. Eng. 2005, 131, 316–323. [Google Scholar] [CrossRef]
- Adeboye, O.B.; Osunbitan, J.A.; Adekalu, K.O.; Okunade, D.A. Evaluation of FAO-56 Penman-Monteith and temperature based models in estimating reference evapotranspiration using complete and limited data, application to Nigeria. Agric. Eng. Int. CIGR J. 2009, XI, 1–25. [Google Scholar]
- Sentelhas, P.C.; Gillespie, T.J.; Santos, E.A. Evaluation of FAO Penman–Monteith and alternative methods for estimating reference evapotranspiration with miss-ing data in Southern Ontario, Canada. Agr. Water Manag. 2010, 97, 635–644. [Google Scholar] [CrossRef]
- Mohawesh, O.E.; Talozi, S.A. Comparison of Hargreaves and FAO56 equations for estimating monthly evapotranspiration for semi-arid and arid environments. Arch. Agron. Soil Sci. 2012, 58, 321–334. [Google Scholar] [CrossRef]
- Cobaner, M.; Citakoğlu, H.; Haktanir, T.; Kisi, O. Modifying Hargreaves–Samani equation with meteorological variables for estimation of reference evapotranspiration in Turkey. Hydrol. Res. 2016, 48, 480–497. [Google Scholar] [CrossRef]
- Song, X.; Lu, F.; Xiao, W.; Zhu, K.; Zhou, Y.; Xie, Z. Performance of 12 reference evapotranspiration estimation methods compared with the Penman-Monteith method and the potential influences in northeast China. Meteorol. Appl. 2019, 26, 83–96. [Google Scholar] [CrossRef] [Green Version]
- Paredes, P.; Fontes, J.C.; Azevedo, E.B.; Pereira, L.S. Daily reference crop evapotranspiration in the humid environments of Azores islands using reduced data sets: Accuracy of FAO-PM temperature and Hargreaves-Samani methods. Theor. Appl. Clim. 2018, 134, 595–611. [Google Scholar] [CrossRef]
- Hargreaves, G.H.; Samani, Z.A. Reference Crop Evapotranspiration from Temperature. Appl. Eng. Agric. 1985, 1, 96–99. [Google Scholar] [CrossRef]
- Droogers, P.; Allen, R.G. Estimating Reference Evapotranspiration Under Inaccurate Data Conditions. Irrig. Drain. Syst. 2002, 16, 33–45. [Google Scholar] [CrossRef]
- Berti, A.; Tardivo, G.; Chiaudani, A.; Rech, F.; Borin, M. Assessing reference evapotranspiration by the Hargreaves method in north-eastern Italy. Agric. Water Manag. 2014, 140, 20–25. [Google Scholar] [CrossRef]
- Trajkovic, S. Hargreaves versus Penman-Monteith under Humid Conditions. J. Irrig. Drain. Eng. 2007, 133, 38–42. [Google Scholar] [CrossRef]
- Tabari, H.; Talaee, P.H. Local Calibration of the Hargreaves and Priestley-Taylor Equations for Estimating Reference Evapotranspiration in Arid and Cold Climates of Iran Based on the Penman-Monteith Model. J. Hydrol. Eng. 2011, 16, 837–845. [Google Scholar] [CrossRef]
- Raziei, T.; Pereira, L.S. Estimation of ETo with Hargreaves–Samani and FAO-PM temperature methods for a wide range of climates in Iran. Agric. Water Manag. 2013, 121, 1–18. [Google Scholar] [CrossRef]
- Valipour, M.; Eslamian, S. Analysis of potential evapotranspiration using 11 modified temperature-based models. Int. J. Hydrol. Sci. Technol. 2014, 4, 192. [Google Scholar] [CrossRef]
- Valipour, M. Temperature analysis of reference evapotranspiration models. Meteorol. Appl. 2015, 22, 385–394. [Google Scholar] [CrossRef]
- Akhavan, S.; Kanani, E.; Dehghanisanij, H. Assessment of different reference evapotranspiration models to estimate the actual evapotranspiration of corn (Zea mays L.) in a semiarid region (case study, Karaj, Iran). Theor. Appl. Clim. 2018, 137, 1403–1419. [Google Scholar] [CrossRef]
- Rodrigues, G.; Braga, R. Estimation of Reference Evapotranspiration during the Irrigation Season Using Nine Temperature-Based Methods in a Hot-Summer Mediterranean Climate. Agriculture 2021, 11, 124. [Google Scholar] [CrossRef]
- Allen, R.G. REF-ET: Reference Evapotranspiration Calculation Software for FAO and ASCE Standardized Equations; University of Idaho: Moscow, Russia, 2000. [Google Scholar]
- Hess, T.M. Potential Evapotranspiration [DAILYET]; Silsoe College: Cranfield, UK, 1996. [Google Scholar]
- George, B.A.; Reddy, B.R.S.; Raghuwanshi, N.S.; Wallender, W.W. Decision Support System for Estimating Reference Evapotranspiration. J. Irrig. Drain. Eng. 2002, 128, 1–10. [Google Scholar] [CrossRef]
- Rodrigues, G.C.; Braga, R.P. Estimation of Daily Reference Evapotranspiration from NASA POWER Reanalysis Products in a Hot Summer Mediterranean Climate. Agronomy 2021, 11, 2077. [Google Scholar] [CrossRef]
- Rodrigues, G.; Braga, R. A Simple Procedure to Estimate Reference Evapotranspiration during the Irrigation Season in a Hot-Summer Mediterranean Climate. Sustainability 2021, 13, 349. [Google Scholar] [CrossRef]
- Henseler, J.; Ringle, C.; Sinkovics, R. The use of partial least squares path modeling in international marketing. Adv. Int. Mark. 2009, 20, 277–320. [Google Scholar]
- Willmott, C.J.; Matsuura, K. On the use of dimensioned measures of error to evaluate the performance of spatial interpolators. Int. J. Geogr. Inf. Sci. 2006, 20, 89–102. [Google Scholar] [CrossRef]
- Nash, J.E.; Sutcliffe, J.V. River flow forecasting through conceptual models part I—A discussion of principles. J. Hydrol. 1970, 10, 282–290. [Google Scholar] [CrossRef]
- Legates, D.R.; McCabe, G.J., Jr. Evaluating the use of goodness-of-fit measures in hydrologic and hydroclimatic model validation. Water Resour. Res. 1999, 35, 233–241. [Google Scholar] [CrossRef]
- Martinez, C.J.; Thepadia, M. Estimating Reference Evapotranspiration with Minimum Data in Florida. J. Irrig. Drain. Eng. 2010, 136, 494–501. [Google Scholar] [CrossRef]
- Estévez, J.; Gavilán, P.; Berengena, J. Sensitivity analysis of a Penman-Monteith type equation to estimate reference evapotranspiration in southern Spain. Hydrol. Process. 2009, 23, 3342–3353. [Google Scholar] [CrossRef]
| Weather Station | Latitude (N) | Longitude (W) | Elevation (m) | Distance to the Sea (km) |
|---|---|---|---|---|
| Beja | 38°02′15′′ | 07°53′06′′ | 206 | 79 |
| Elvas | 38°54′56′′ | 07°05′56′′ | 202 | 160 |
| Odemira | 37°30′06′′ | 08°45′12′′ | 92 | 4 |
| Year | ETo Estimation Method | Accuracy Indicators | |||||
|---|---|---|---|---|---|---|---|
| b | R2 | NRMSE (%) | NMBE (%) | EF | |||
| Humid | HS | Using Rs | 1.29 | 0.73 | 34.81 | 30.60 | −0.98 |
| Using Seasonal kRs | 1.12 | 0.62 | 22.82 | 12.60 | 0.15 | ||
| Using Monthly kRs | 1.08 | 0.61 | 20.55 | 9.40 | 0.31 | ||
| MHS1 | 1.20 | 0.63 | 28.60 | 21.97 | −0.33 | ||
| MHS2 | 1.18 | 0.62 | 28.01 | 19.31 | −0.28 | ||
| MHS3 | 0.98 | 0.62 | 17.33 | −1.25 | 0.51 | ||
| Tr | 0.93 | 0.63 | 16.92 | −6.43 | 0.53 | ||
| MaxTET | 1.07 | 0.60 | 18.51 | 8.89 | 0.44 | ||
| Average | HS | Using Seasonal kRs | 1.18 | 0.84 | 24.00 | 18.72 | 0.31 |
| Using Monthly kRs | 0.99 | 0.75 | 14.58 | 1.01 | 0.75 | ||
| Using Seasonal kRs | 0.96 | 0.73 | 15.13 | −1.99 | 0.73 | ||
| MHS1 | 1.08 | 0.75 | 17.80 | 10.43 | 0.62 | ||
| MHS2 | 1.05 | 0.75 | 16.27 | 6.94 | 0.68 | ||
| MHS3 | 0.87 | 0.75 | 18.73 | −11.53 | 0.58 | ||
| Tr | 0.83 | 0.75 | 21.78 | −15.57 | 0.43 | ||
| MaxTET | 0.95 | 0.67 | 17.27 | −1.81 | 0.64 | ||
| Dry | HS | Using Rs | 1.17 | 0.80 | 22.95 | 17.07 | 0.29 |
| Using Seasonal kRs | 1.01 | 0.75 | 14.58 | 1.52 | 0.71 | ||
| Using Monthly kRs | 0.97 | 0.72 | 14.85 | −1.58 | 0.70 | ||
| MHS1 | 1.09 | 0.78 | 17.00 | 10.38 | 0.61 | ||
| MHS2 | 1.07 | 0.74 | 17.02 | 7.57 | 0.61 | ||
| MHS3 | 0.88 | 0.74 | 17.78 | −11.02 | 0.57 | ||
| Tr | 0.84 | 0.77 | 20.29 | −15.40 | 0.44 | ||
| MaxTET | 0.96 | 0.73 | 14.44 | −1.66 | 0.72 | ||
| Year | ETo Estimation Method | Accuracy Indicators | |||||
|---|---|---|---|---|---|---|---|
| b | R2 | NRMSE (%) | NMBE (%) | EF | |||
| Humid | HS | Using Rs | 1.01 | 0.99 | 8.13 | 1.58 | 0.99 |
| Using Seasonal kRs | 1.05 | 0.88 | 14.16 | 6.15 | 0.84 | ||
| Using Monthly kRs | 1.05 | 0.88 | 13.96 | 5.97 | 0.85 | ||
| MHS1 | 1.09 | 0.88 | 16.70 | 10.89 | 0.78 | ||
| MHS2 | 1.11 | 0.88 | 18.55 | 12.67 | 0.73 | ||
| MHS3 | 0.93 | 0.88 | 14.03 | −6.39 | 0.85 | ||
| Tr | 0.85 | 0.88 | 19.54 | −13.95 | 0.70 | ||
| MaxTET | 1.04 | 0.82 | 16.65 | 6.68 | 0.78 | ||
| Average | HS | Using Rs | 1.04 | 0.91 | 12.61 | 4.83 | 0.89 |
| Using Seasonal kRs | 1.08 | 0.85 | 18.32 | 10.34 | 0.77 | ||
| Using Monthly kRs | 1.08 | 0.85 | 18.32 | 10.22 | 0.77 | ||
| MHS1 | 1.11 | 0.85 | 21.17 | 14.94 | 0.70 | ||
| MHS2 | 1.14 | 0.85 | 23.32 | 17.20 | 0.63 | ||
| MHS3 | 0.95 | 0.85 | 15.36 | −2.67 | 0.84 | ||
| Tr | 0.87 | 0.85 | 19.50 | −10.65 | 0.74 | ||
| MaxTET | 1.08 | 0.82 | 19.96 | 11.51 | 0.73 | ||
| Dry | HS | Using Rs | 1.01 | 0.95 | 8.75 | 1.22 | 0.94 |
| Using Seasonal kRs | 1.04 | 0.87 | 14.66 | 5.79 | 0.84 | ||
| Using Monthly kRs | 1.04 | 0.88 | 14.34 | 5.55 | 0.85 | ||
| MHS1 | 1.08 | 0.87 | 17.11 | 10.48 | 0.78 | ||
| MHS2 | 1.11 | 0.87 | 18.83 | 12.37 | 0.74 | ||
| MHS3 | 0.92 | 0.87 | 14.85 | −6.72 | 0.84 | ||
| Tr | 0.84 | 0.87 | 20.32 | −14.18 | 0.70 | ||
| MaxTET | 1.05 | 0.83 | 16.98 | 7.83 | 0.79 | ||
| Year | ETo Estimation Method | Accuracy Indicators | |||||
|---|---|---|---|---|---|---|---|
| b | R2 | NRMSE (%) | NMBE (%) | EF | |||
| Humid | HS | Using Rs | 1.03 | 0.94 | 9.93 | 4.50 | 0.93 |
| Using Seasonal kRs | 1.07 | 0.83 | 18.39 | 9.35 | 0.76 | ||
| Using Monthly kRs | 1.04 | 0.82 | 17.18 | 5.74 | 0.79 | ||
| MHS1 | 1.17 | 0.83 | 25.91 | 20.47 | 0.52 | ||
| MHS2 | 1.21 | 0.83 | 29.22 | 23.41 | 0.39 | ||
| MHS3 | 1.00 | 0.83 | 15.86 | 2.57 | 0.82 | ||
| Tr | 0.91 | 0.83 | 17.19 | −6.26 | 0.79 | ||
| MaxTET | 1.04 | 0.80 | 18.43 | 7.29 | 0.76 | ||
| Average | HS | Using Rs | 0.97 | 0.92 | 10.82 | −2.14 | 0.91 |
| Using Seasonal kRs | 1.02 | 0.79 | 17.28 | 4.35 | 0.77 | ||
| Using Monthly kRs | 0.98 | 0.80 | 16.22 | 0.79 | 0.80 | ||
| MHS1 | 1.11 | 0.79 | 22.59 | 15.18 | 0.61 | ||
| MHS2 | 1.15 | 0.79 | 24.89 | 17.70 | 0.53 | ||
| MHS3 | 0.95 | 0.79 | 16.90 | −2.13 | 0.78 | ||
| Tr | 0.87 | 0.79 | 20.36 | −10.49 | 0.69 | ||
| MaxTET | 0.99 | 0.79 | 17.07 | 2.07 | 0.78 | ||
| Dry | HS | Using Rs | 0.90 | 0.86 | 17.00 | −8.98 | 0.81 |
| Using Seasonal kRs | 0.95 | 0.75 | 19.39 | −2.28 | 0.75 | ||
| Using Monthly kRs | 0.92 | 0.77 | 19.25 | −5.75 | 0.75 | ||
| MHS1 | 1.04 | 0.76 | 20.48 | 7.56 | 0.72 | ||
| MHS2 | 1.07 | 0.75 | 22.30 | 10.29 | 0.66 | ||
| MHS3 | 0.89 | 0.75 | 21.10 | −8.33 | 0.70 | ||
| Tr | 0.81 | 0.76 | 25.69 | −16.28 | 0.55 | ||
| MaxTET | 0.93 | 0.79 | 18.36 | −4.09 | 0.77 | ||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rodrigues, G.C.; Braga, R.P. A Simple Application for Computing Reference Evapotranspiration with Various Levels of Data Availability—ETo Tool. Agronomy 2021, 11, 2203. https://doi.org/10.3390/agronomy11112203
Rodrigues GC, Braga RP. A Simple Application for Computing Reference Evapotranspiration with Various Levels of Data Availability—ETo Tool. Agronomy. 2021; 11(11):2203. https://doi.org/10.3390/agronomy11112203
Chicago/Turabian StyleRodrigues, Gonçalo C., and Ricardo P. Braga. 2021. "A Simple Application for Computing Reference Evapotranspiration with Various Levels of Data Availability—ETo Tool" Agronomy 11, no. 11: 2203. https://doi.org/10.3390/agronomy11112203
APA StyleRodrigues, G. C., & Braga, R. P. (2021). A Simple Application for Computing Reference Evapotranspiration with Various Levels of Data Availability—ETo Tool. Agronomy, 11(11), 2203. https://doi.org/10.3390/agronomy11112203







