Modeling and Experimental Validation of the Atomization Efficiency of a Rotary Atomizer for Aerial Spraying
Abstract
:1. Introduction
2. Measurement, Analysis, and Modeling of Atomization Efficiency
2.1. Analysis of Droplet Break-Up
2.2. Theory of the Atomization Efficiency Model
3. Materials and Methods
3.1. Composition of Atomization Experimental Platform
3.2. Experimental Methods
4. Results and Analysis
- (1)
- When the wind speed remained constant and the angle of the atomizer fan blade increased, the size of the droplets increased;
- (2)
- As the wind speed increased by the same value, the decrease in the droplet size depended on the fan blade angle. The smaller the fan blade angle, the smaller the droplet size; the larger the change in wind speed, the larger the change in droplet size;
- (3)
- The traction force was directly proportional to the wind speed. The higher the wind speed, the larger the traction force;
- (4)
- The traction force was dependent on the fan blade angle. At a wind speed of 50.11 m/s, the traction forces were 31.6, 30.4, 29.6, and 29.6 N at fan blade angles of 35°, 45°, 55°, and 65°, respectively. Thus, the smaller the fan blade angle, the higher the traction force of the atomizer. Hence, there was a clear correlation between the fan blade angle and traction force. However, as the fan blade angle increased, the rate of change in traction force decreased;
- (5)
- The traction power increased as the wind speed increased, and it was proportional to the wind speed.
5. Conclusions
- (1)
- The atomization efficiency decreased as the fan blade angle of the atomizer increased. When the fan blade angle was 35°, the atomization efficiency was optimal, regardless of the wind speed. In contrast, when the fan blade angle was 65°, the efficiency degraded, regardless of the wind speed;
- (2)
- Variations in the flow rate did not affect the trends in the atomization efficiency of the atomizer. At flow rates of 5.0 and 10.8 L/min, the model equation was valid, and the visualization curves for the data measured experimentally showed the same regularity;
- (3)
- In this study, an AU5000 atomizer was used to simulate a rotary atomizer in use by a manned aerial vehicle. The variable quantity regularity also applied when the flow rate extended beyond the range of this experiment;
- (4)
- At present, studies have only been performed in the wind tunnel of the laboratory platform. Due to financial and technical reasons, there is no field experiment in farmland. Therefore, this study does not include the comparison of spray atomization performance when the outcomes are tested with a field experiment. This study has not solved this work, and is currently moving towards this goal.
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Zhang, R.; Li, L.; Wen, Y.; Chen, L.; Tang, Q.; Yi, T.; Song, J. Fluorescent tracer analysis of the deposition characteristics of spray droplets in the plant protection UAV. Agric. Eng. J. 2020, 36, 47–55. [Google Scholar]
- Martin, D.; Singh, V.; Latheef, M.A.; Bagavathiannan, M. Spray deposition on weeds (palmer amaranth and morning glory) from a remotely piloted aerial application system and backpack sprayer. Drones 2020, 4, 59. [Google Scholar] [CrossRef]
- Faiçal, B.S.; Freitas, H.; Gomes, P.H.; Mano, L.Y.; Pessin, G.; de Carvalho, A.C.; Krishnamachari, B.; Ueyama, J. An adaptive approach for UAV-based pesticide spraying in dynamic environments. Comput. Electron. Agric. 2017, 138, 210–223. [Google Scholar] [CrossRef]
- Hooper, G.H.S.; Spurgin, P.A. Droplet size spectra produced by the atomization of a ULV formulation of fenitrothion with a Micronair AU5000 rotary atomizer. Crop Prot. 1995, 14, 27–30. [Google Scholar] [CrossRef]
- Tsai, M.Y.; Elgethun, K.; Ramaprasad, J.; Yost, M.G.; Felsot, A.S.; Hebert, V.R.; Fenske, R.A. The Washington aerial spray drift study: Modeling pesticide spray drift deposition from an aerial application. Atmos. Environ. 2005, 39, 6194–6203. [Google Scholar] [CrossRef]
- Teske, M.E.; Thistle, H.W. Aerial application model extension into the far field. Biosyst. Eng. 2004, 89, 29–36. [Google Scholar] [CrossRef]
- Ryan, S.D.; Gerber, A.G.; Holloway, A.G.L. A computational study of sprays produced by rotary cage atomizers. Trans. ASABE 2012, 55, 1133–1148. [Google Scholar] [CrossRef]
- Lebeau, F.; El Bahir, L.; Destain, M.F.; Kinnaert, M.; Hanus, R. Improvement of spray deposit homogeneity using a PWM spray controller to compensate horizontal boom speed variations. Comput. Electron. Agric. 2004, 43, 149–161. [Google Scholar] [CrossRef]
- Berenstein, R.; Edan, Y. Automatic Adjustable Spraying Device for Site-Specific Agricultural Application. IEEE Trans. Autom. Sci. Eng. 2018, 15, 4–15. [Google Scholar] [CrossRef]
- Van Deventer, H.; Houben, R.; Koldeweij, R. New atomization nozzle for spray drying. Dry. Technol. 2013, 31, 891–897. [Google Scholar] [CrossRef]
- Fabiano, G.; Ricardo, A.; Cícero, A.; Eduardo, S.; Nelson, H.; Marcelo, C. How much do adjuvant and nozzles models reduce the spraying drift? Drift in agricultural spraying. Am. J. Plant Sci. 2017, 8, 15–23. [Google Scholar]
- Weicai, Q.; Baijing, Q.; Xinyu, X. Droplet deposition and control effect of insecticides sprayed with an unmanned aerial vehicle against plant hoppers. Crop Prot. 2016, 85, 79–88. [Google Scholar]
- Zhang, B.; Tang, Q.; Chen, L.P.; Zhang, R.R.; Xu, M. Numerical simulation of spray drift and deposition from a crop spraying aircraft using a CFD approach. Biosyst. Eng. 2018, 166, 84–199. [Google Scholar] [CrossRef]
- Wen, Y.; Zhang, R.; Chen, L.; Huang, Y.; Yi, T.; Xu, G.; Li, L.; Hewitt, A.J. A new spray deposition pattern measurement system based on spectral analysis of a fluorescent tracer. Comput. Electron. Agric. 2019, 160, 14–22. [Google Scholar] [CrossRef]
- Zhang, T.; Dong, B.; Chen, X.; Qiu, Z.; Jiang, R.; Li, W. Spray characteristics of pressure-swirl nozzles at different nozzle diameters. Appl. Therm. Eng. 2017, 121, 984–991. [Google Scholar] [CrossRef]
- Dorr, G.J.; Hewitt, A.J.; Adkins, S.W.; Hanan, J.; Zhang, H.; Noller, B. A comparison of initial spray characteristics produced by agricultural nozzles. Crop Prot. 2013, 53, 109–117. [Google Scholar] [CrossRef]
- Craig, I.P.; Hewitt, A.; Terry, H. Rotary atomiser design requirements for optimum pesticide application efficiency. Crop Prot. 2014, 66, 34–39. [Google Scholar] [CrossRef]
- Guler, H.; Zhu, H.; Ozkan, H.E.; Ling, P. Characterization of hydraulic nozzles for droplet size and spray coverage. Atom. Sprays 2012, 22, 627–645. [Google Scholar] [CrossRef] [Green Version]
- Martin, D.E.; Carlton, J.B. Airspeed and orifice size affect spray droplet spectra from an aerial electrostatic nozzle for rotary-wing applications. Atom. Sprays 2012, 22, 997–1010. [Google Scholar] [CrossRef] [Green Version]
- Martin, D.E.; Latheef, M.A. Aerial electrostatic spray deposition and canopy penetration in cotton. J. Electrost. 2017, 90, 38–44. [Google Scholar] [CrossRef]
- Darwish, A.; Abubaker, A.; Salaimeh, A.; Akafuah, N. Schlieren visualization of shaping air during operation of an electrostatic rotary bell sprayer: Impact of shaping air on droplet atomization and transport. J. Coat. 2018, 8, 279. [Google Scholar] [CrossRef] [Green Version]
- Darwish, A.; Singh, B.; Doerre, M.; Abubaker, A.; Arabghahestani, M.; Salaimeh, A.; Akafuah, N. Spatial Positioning and Operating Parameters of a Rotary Bell Sprayer: 3D Mapping of Droplet Size Distributions. J. Fluids 2019, 4, 165. [Google Scholar] [CrossRef] [Green Version]
- Oswald, W.; Gödeke, L.; Ehrhard, P.; Willenbacher, N. Influence of the elongational flow resistance and pigmentation of coating fluids on high-speed rotary bell atomization. J. At. Sprays 2019, 29, 913–935. [Google Scholar] [CrossRef]
- Shen, B.; Ye, Q.; Guettler, N.; Tiedje, O.; Domnick, J. Primary breakup of a non-Newtonian liquid using a high-speed rotary bell atomizer for spray-painting processes. J. Coat. Technol. Res. 2019, 16, 1581–1596. [Google Scholar] [CrossRef]
- Pendar, M.; Páscoa, J. Atomization and spray characteristics around an ERBS using various operational models and conditions: Numerical investigation. Int. J. Heat Mass Transf. 2020, 161, 122–123. [Google Scholar] [CrossRef]
- Pendar, M.; Páscoa, J. Numerical analysis of charged droplets size distribution in the electrostatic coating process: Effect of different operational conditions. J. Phys. Fluids 2021, 33, 317. [Google Scholar] [CrossRef]
- Kumar, A.; Sahu, S. Liquid jet breakup unsteadiness in a coaxial air-blast atomizer. Int. J. Spray Combus. Dyn. 2018, 10, 211–230. [Google Scholar] [CrossRef] [Green Version]
- Makhnenko, I.; Alonzi, E.; Fredericks, S. A review of liquid sheet breakup: Perspectives from agricultural sprays. J. Aerosol Sci. 2021, 157, 105. [Google Scholar] [CrossRef]
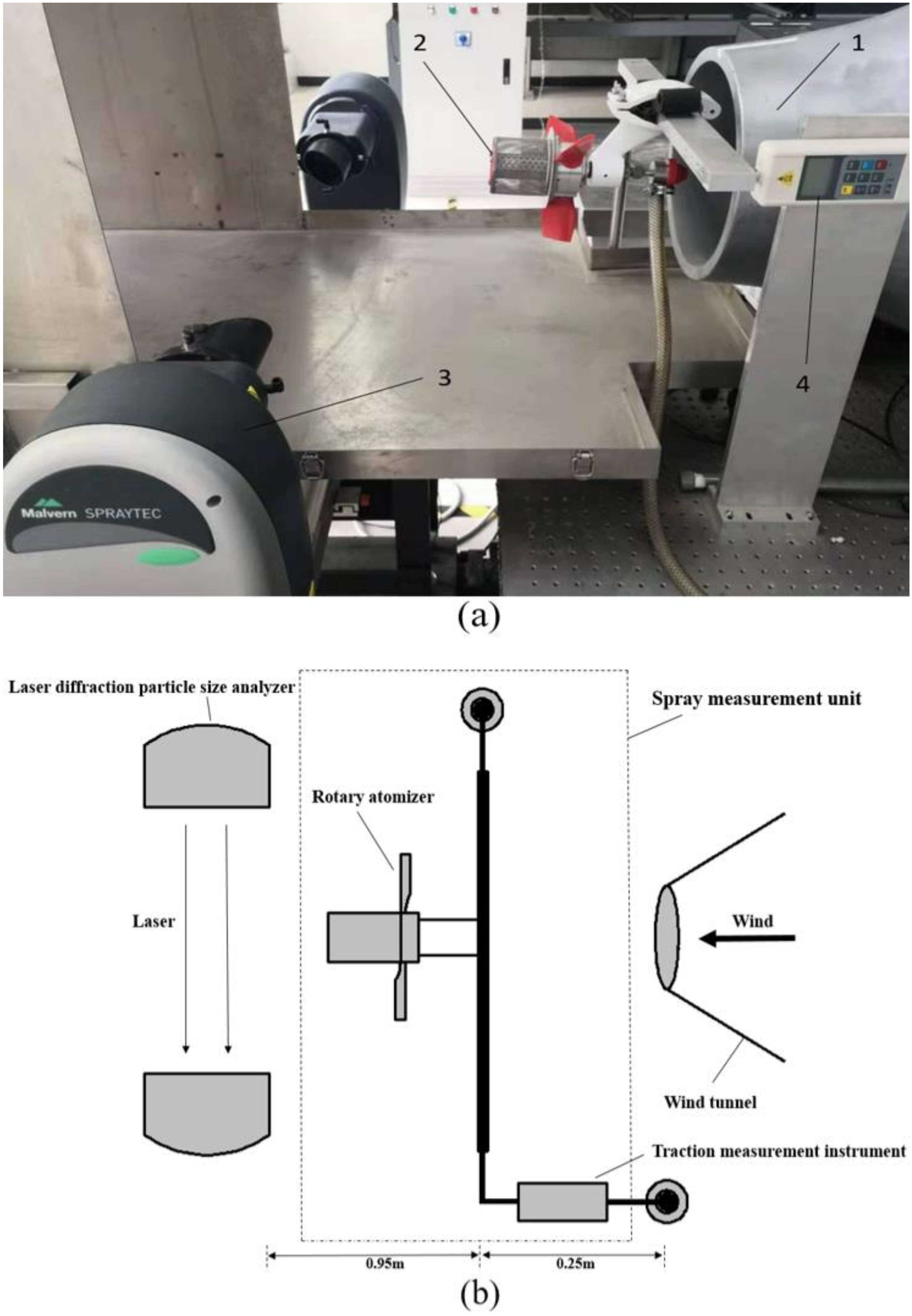
| Equipment | Specification | Parameter |
|---|---|---|
| Traction measurement instrument | Indicating error | ±0.5% |
| Wind tunnel | Wind speed | 6.70–98.0 m/s |
| Dynamic pressure stability coefficient | <2% | |
| Turbulence scale | <1.0% | |
| Mean airflow bias | <0.5% | |
| Rotary atomizer | Fan blade adjustable angle | 25–85° |
| Flow bearing range | 0–23 L/min | |
| Laser diffraction particle size analyzer | Particle size range | 0.1–3500.0 µm |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, G.; Chen, L.; Li, L.; Yi, T.; Ding, C.; Wang, J.; Zhao, C.; Zhang, R. Modeling and Experimental Validation of the Atomization Efficiency of a Rotary Atomizer for Aerial Spraying. Agronomy 2022, 12, 419. https://doi.org/10.3390/agronomy12020419
Li G, Chen L, Li L, Yi T, Ding C, Wang J, Zhao C, Zhang R. Modeling and Experimental Validation of the Atomization Efficiency of a Rotary Atomizer for Aerial Spraying. Agronomy. 2022; 12(2):419. https://doi.org/10.3390/agronomy12020419
Chicago/Turabian StyleLi, Gen, Liping Chen, Longlong Li, Tongchuan Yi, Chenchen Ding, Juan Wang, Chunjiang Zhao, and Ruirui Zhang. 2022. "Modeling and Experimental Validation of the Atomization Efficiency of a Rotary Atomizer for Aerial Spraying" Agronomy 12, no. 2: 419. https://doi.org/10.3390/agronomy12020419
APA StyleLi, G., Chen, L., Li, L., Yi, T., Ding, C., Wang, J., Zhao, C., & Zhang, R. (2022). Modeling and Experimental Validation of the Atomization Efficiency of a Rotary Atomizer for Aerial Spraying. Agronomy, 12(2), 419. https://doi.org/10.3390/agronomy12020419








