Analytical Design and Test of Licorice Harvester Based on DEM–MBD Coupling

Abstract

This study addresses the issues of high operating resistance, incomplete separation in ascending transport chains, and significant wear and tear in existing licorice harvesters. A new licorice harvester has been designed that incorporates a lift chain conveyor separation device, enabling excavation, separation, collection, and centralized stacking to be completed in a single operation. The paper describes the harvester’s overall structure and provides detailed analyses and designs of its key components, including the digging shovel, roller screen, conveying and separating screens, and soil-crushing roller. Multi-body dynamics (MBD) and discrete element models (DEM) for licorice and soil were developed, and the entire harvesting process was simulated using the coupled DEM–MBD method to analyze the trajectory and speed of the licorice. Field tests confirmed that the conveyor separation screen operates smoothly, effectively separates licorice rhizomes from soil, and minimizes damage to the licorice. Field test results show a net digging rate of 96.2%, a damage rate of 4.3%, and an average digging depth of 580 mm. The operational indexes meet the standards for harvesting root and stem Chinese herbal medicines. The machine operates stably and exhibits exceptional conveying and separating effects, demonstrating its suitability for mechanized harvesting of root and stem herbs.

1. Introduction

Licorice is a perennial dicotyledonous herb belonging to the genus Glycyrrhiza within the family Leguminosae. It features a stout rhizome with a brown outer skin and a yellowish inner skin, known for its sweet flavor. This herb is extensively utilized across various industries, including pharmaceuticals and food. Recently, the continuous advancement in the Chinese medicine and health sector has led to a significant increase in the licorice market, highlighting a growing disparity between supply and demand. Over-exploitation of wild licorice has resulted in diminishing wild resources and substantial environmental degradation [1]. To address the supply–demand imbalance, the development of an artificial licorice planting industry has become imperative [2].

Current agricultural equipment for harvesting root and tuber herbal medicines faces several challenges, including high operating resistance, incomplete separation of medicinal materials from soil during transportation, and severe wear and tear due to poorly designed lifting-chain structures. These issues increase labor costs and adversely impact farmers’ economic benefits. Consequently, there is a pressing need for further design and optimization of licorice harvesters [3,4].

In recent years, other countries have developed more comprehensive technology harvesting equipment for potatoes and carrots and other shallow-root crops. Japan’s Kubota company produces the CH-201C carrot combine harvester, with a maximum digging depth of 300 mm. Its mechanical performance is stable, with a fast operating speed, and its harvesting loss rate is low [5]. The 4150 from Sweden’s Greemo AB’s self-propelled potato harvester VENTOR has a working width of up to 3 m. In the process of operation, it can complete any angle of flexible turning [6]. It is worth noting that these machines utilize the principle of vibration to reduce energy consumption, thus improving the efficiency of the machine [7]. These harvesting machines are unable to give full play to their advantages for the hilly and mountainous regions in the northwest of China. The existing root herb harvesting machinery is based on the potato harvesting machine for improvement, but it is difficult to meet the deep-root herb harvesting. Therefore, in view of the growth characteristics of Chinese herbs and the terrain of the hilly mountainous areas in Northwest China, many domestic research teams have begun research and development of harvesting machinery for root and tuberous Chinese herbs [8]. For example, Xie Xinya et al. [9]. from Xinjiang Agricultural University have designed a licorice harvester suited for sandy soils in northern Xinjiang, incorporating a roller-type separator and conveyor with a 600 mm operating width. Additionally, the 4WGX-120/150-type root herb excavator, jointly developed by Gansu Province Agricultural Mechanisation Technology Extension General Station and Dingxi City Agricultural Mechanisation Technology Extension General Station [10], offers a digging depth of 400–500 mm, high efficiency, and good mobility. Zhang Shun et al. [11]. from Anhui Agricultural University have introduced a traction root and tuber harvester with a linear vibrating screen for separation and a second-order planar digging shovel, which meets strength requirements through finite element analysis.

Currently, the lift chain conveyor separator screen remains a crucial component for separating crops from soil during harvesting. However, due to the suboptimal design of the lifting-chain structure, improper operation parameters, and power configuration, problems such as incomplete conveying and separation, excessive wear of transmission parts, and high tractor traction resistance persist. Thus, in-depth research is needed to optimize the operation mechanism and parameters of the conveyor separation device in licorice harvesters.

Addressing these issues, this paper presents the design of a licorice harvester tailored to the planting modes and agronomic requirements of licorice in the hilly and mountainous regions of Northwest China. The harvester integrates excavation, separation of medicine and soil, conveying, collecting, and centralized stacking into a single operation. Firstly, the theoretical design of the structure of the digging shovel and the elevating-chain conveyor separating screen is carried out [12], then the whole harvesting process of the harvester is simulated using the EDEM–RecurDyn coupling method to analyze the conveying and separating effect of the separating screen for the medicine–soil mixture during the harvesting process, and, finally, the harvesting effect of the harvester is tested by the field test. It is believed that this study can provide a new reference for the innovative design and optimization of root herb harvesters.

2. Materials and Methods

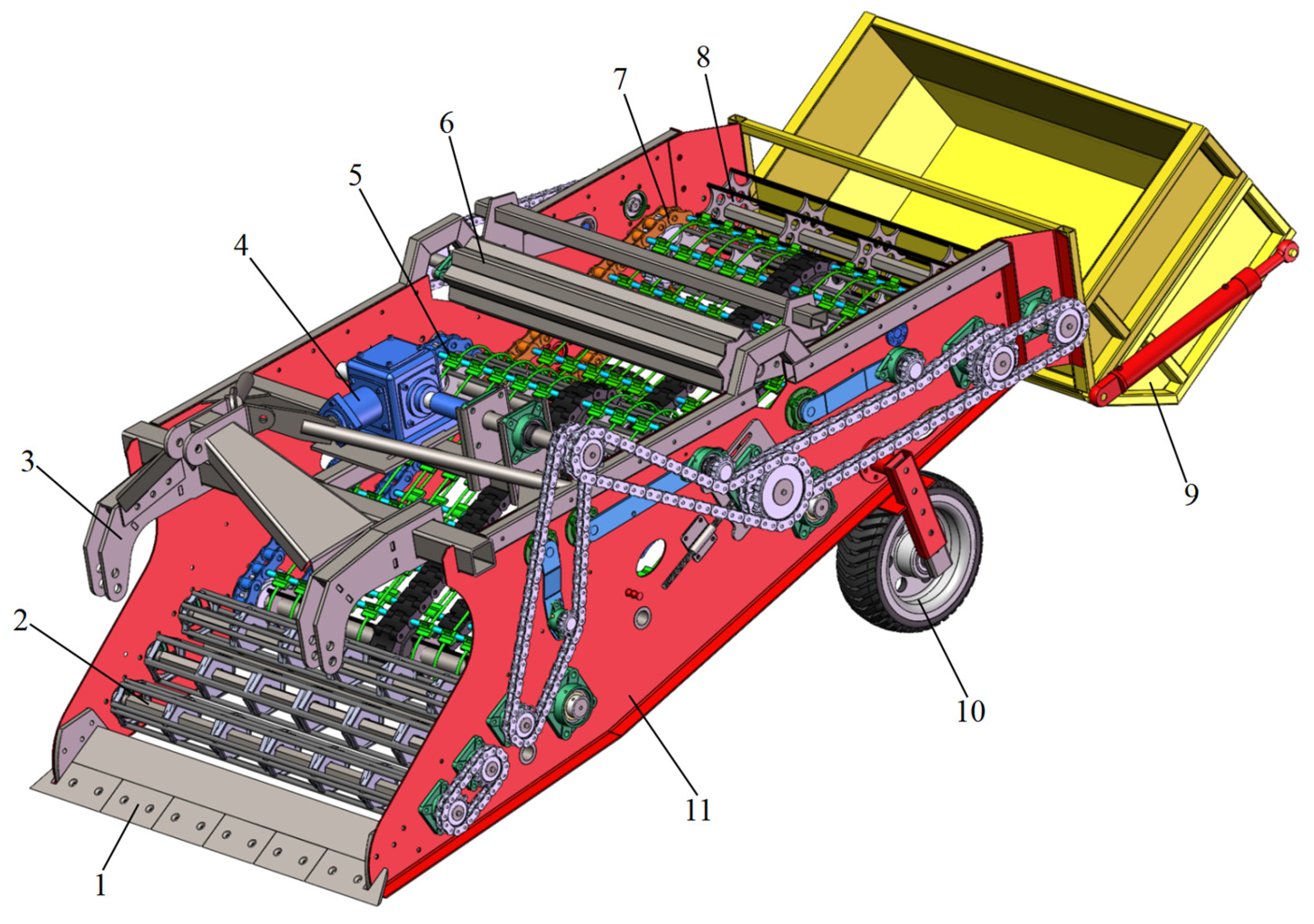
2.1. General Structure and Main Technical Parameters

The licorice harvester is mainly composed of a traction frame, frame, gearbox, digging-shovel assembly, roller screen, first-stage conveying screen, soil-crushing roller, second-stage conveying screen, rear support wheel, medicine collection box, depth-limiting wheel, etc. Its structural structure is shown in Figure 1.

The machine is designed in accordance with the agronomic requirements for standardized cultivation of licorice in the hilly and mountainous areas of Northwest China, and the main parameters are shown in

Table 1. Main design parameters.

Parameters	Numerical Value
Overall size (Length × Width × Height)	3450 × 1830 × 1410 (mm)
Motive force	65 kw
Working width	1200 mm
Digging depth	500–600 mm

.

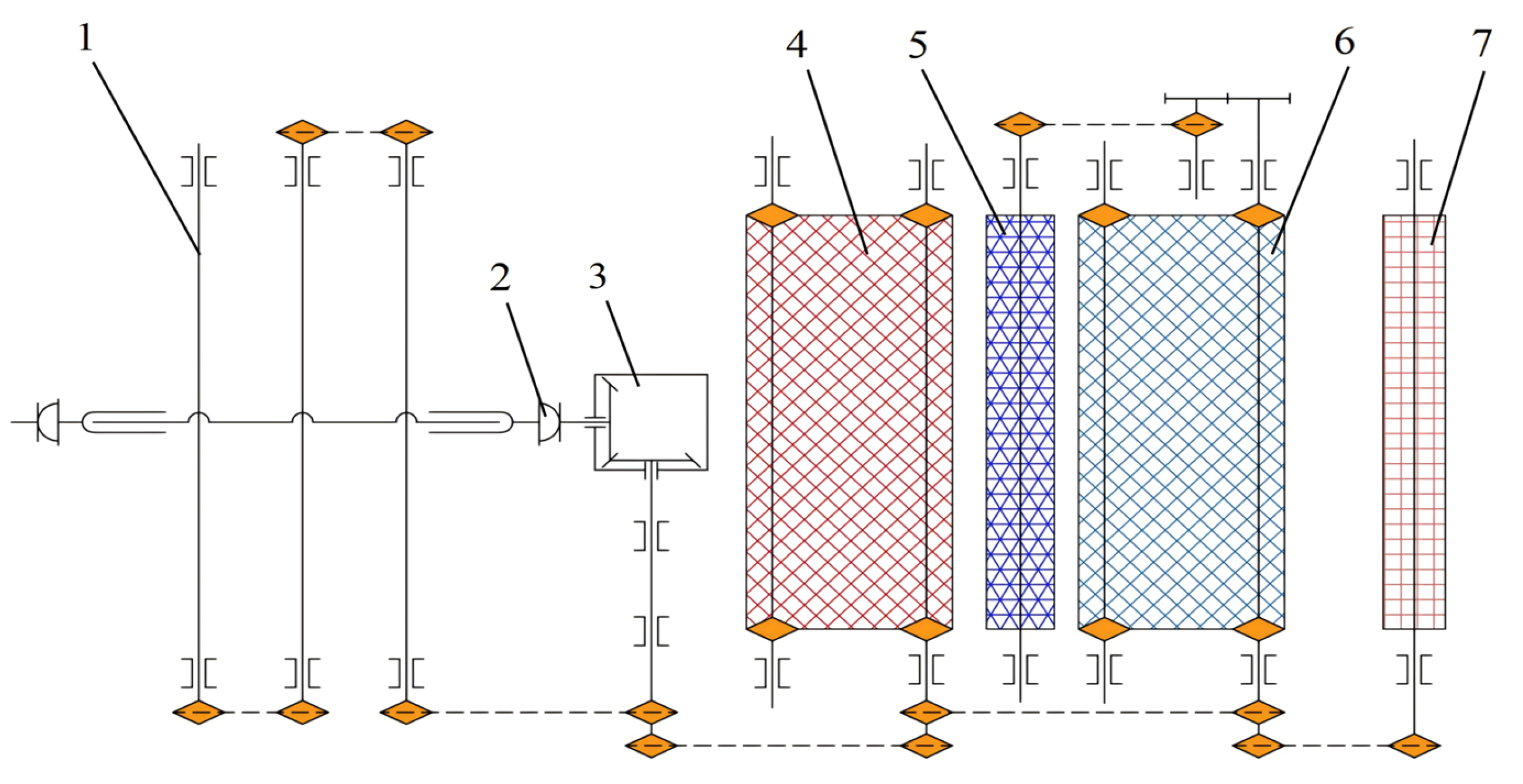
2.2. Working Principle

The licorice harvester is towed by a tractor and features a three-point suspension system. During operation, the tractor drives the traction frame forward, allowing the machine to adjust its working angle by repositioning the depth-limiting wheel’s positioning pin hole. The universal joint drive shaft at the rear of the tractor transfers power to the gearbox mounted above the traction frame. The gearbox’s output shaft powers three sets of soil-crushing rollers on the roller screen, two sets of conveyor separating screens, the rear paddle wheels, and the upper part of the machine.

The tractor suspension system moves the traction frame, causing the excavation shovel to dig into the ground and lift both soil and licorice. The soil and licorice are initially processed by three sets of loading rollers, which then feed the mixture to the first-stage conveyor chain. This chain performs the initial separation of finely crushed soil from medicinal herbs. The soil-crushing rollers further process the mixture, and the second-stage conveyor chain separates any remaining larger soil pieces. The separated licorice is collected in a medicine collection box at the rear, which is then emptied into a truck for centralized stacking when full.

3. Key Component Design

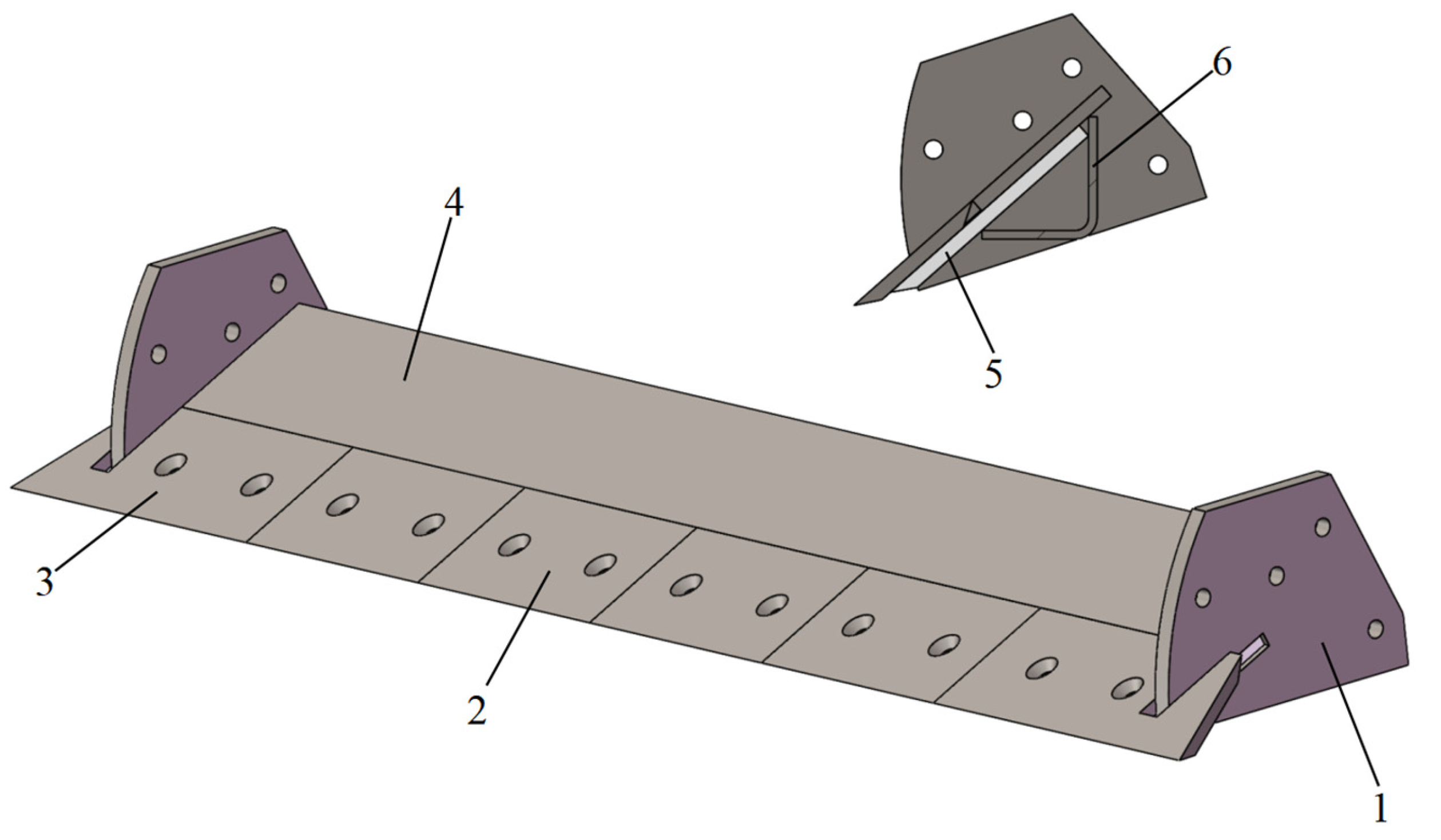
3.1. Excavating Shovel Structural Design

In accordance with the agronomic requirements for licorice harvesting, the soil characteristics, and the growing conditions of licorice, the digging shovel features a flat combined design [13]. This design is attached to the mounting plate with countersunk bolts and secured to the frame using digging-shovel fixing plates on both sides. This approach simplifies maintenance and reduces costs compared to an integral digging shovel [14].

The design includes six individual shovels to balance excessive deformation and material wastage during replacement. Additionally, to address potential driving errors caused by tractor traction, the central digging shovel and the side shovels have different structural designs. The structure of the digging-shovel assembly is illustrated in Figure 2.

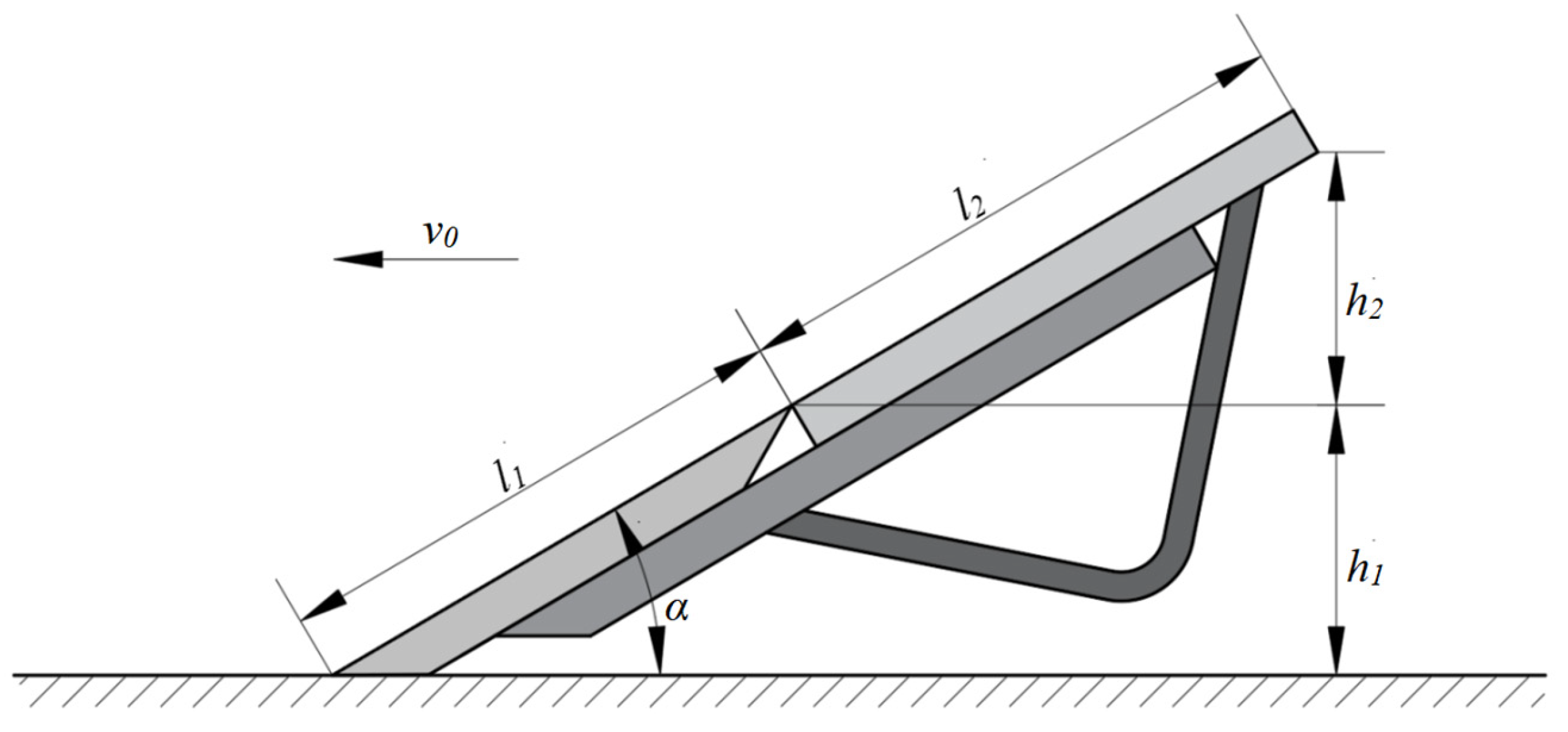
Determination of the Main Parameters of the Excavating Shovel

A force analysis was carried out on the medicinal soil mixture picked up by the excavating shovel, as shown in Figure 3.

• Inclination of shovel surface (angle of entry) α;

The equilibrium equation of the excavated mixture on the shovel surface at an angle of inclination of the shovel surface is given by

$$\left\{\begin{array}{l}Pcos \alpha -T-Gsin \alpha =0\\ R-Psin \alpha -Gcos \alpha =0\end{array}\right.$$

(1)

where P is the horizontal force on the soil mixture dug up by the excavating shovel, N; G is the gravitational force on the soil mixture dug up by the excavating shovel, N; R is the plane support force of the excavating shovel on the soil mixture dug up by the excavating shovel, N; T is the friction between the soil mixture dug up by the excavating shovel and the surface of the shovel, T = μR, N; and μ is the friction coefficient between the soil mixture dug up by the excavating shovel and the surface of the shovel.

The angle of inclination of the shovel surface is calculated as follows:

$$\alpha =arctan{\displaystyle \frac{P-\mu G}{\mu P+G}}$$

(2)

Typically, increasing the angle of shovel-surface inclination (α) enhances the soil-crushing effect. However, if the α angle is too large, the resistance of the excavation shovel during operation also increases [15], leading to higher power consumption by the tractor. Considering the overall working parameters of the machine, an α angle of 30° is chosen.

2.

Length of shovel surface

Length of shovel surface. The dimensions of the digging shovel are illustrated in Figure 4. Here, v₀ represents the forward speed of the unit, while the total length of the shovel surface comprises the length of the digging shovel (l₁) and the length of the digging-shovel fixing plate (l₂). These lengths can be determined based on their respective geometrical relationships.

The dimensions of the shovel face l₁: l₁ are determined by the mounting height h₁ of the shovel and the angle α. According to the geometrical relations,

$$l_1={\displaystyle \frac{h_1}{sin\alpha }}$$

(3)

where h₁ is the excavation shovel installation height, m.

The dimensions of the extension plate of the excavation shovel l₂. l₂ is the lifting section; the excavated object needs to overcome gravity and shovel surface friction to perform work during its movement from point B to point C. According to the law of conservation of energy,

$$\begin{array}{c}{\displaystyle \frac{1}{2}}m{v}_0^2=W_f+W_g\\ W_f=\mu mg{L}_{2}cos\alpha \\ W_g=mg{L}_{2}sin\alpha \\ \mu =tan\phi \end{array}$$

(4)

where v₀ is the forward speed of the machine, m/s; W_f is the work performed by the friction of the shovel surface, J; W_g is the work performed by gravity, J; μ is the friction coefficient between the excavated mixture and the shovel surface; m is the mass of the excavated mixture, kg; g is the acceleration of gravity, m/s²; α is the inclination angle of the shovel surface, °; and φ is the friction angle between the soil and the steel, °, taken as 34°.

The length of the extension plate of the excavation shovel can be obtained as follows:

$$L_2={\displaystyle \frac{v_0^{2}cos\phi }{2gsin\left(\alpha +\phi \right)}}$$

(5)

Then, the total length of the shovel can be obtained:

$$L=L_1+L_2={\displaystyle \frac{h_1}{sin\alpha }}+{\displaystyle \frac{v_0^{2}cos\phi }{2gsin\left(\alpha +\phi \right)}}$$

(6)

3.

Excavating shovel width B.

In order to facilitate dismantling and maintenance during the operation of the harvester, the width of a single shovel should not be too large. Combined with the whole machine operating width and digging-shovel fixed-plate thickness and other parameters, the single shovel width-calculation formula is as follows:

$$B={\displaystyle \frac{L-2b}{N}}$$

(7)

where L is the width of the harvester, m; b is the thickness of the digging-shovel fixed plate, m; and N is the number of combined shovels, n.

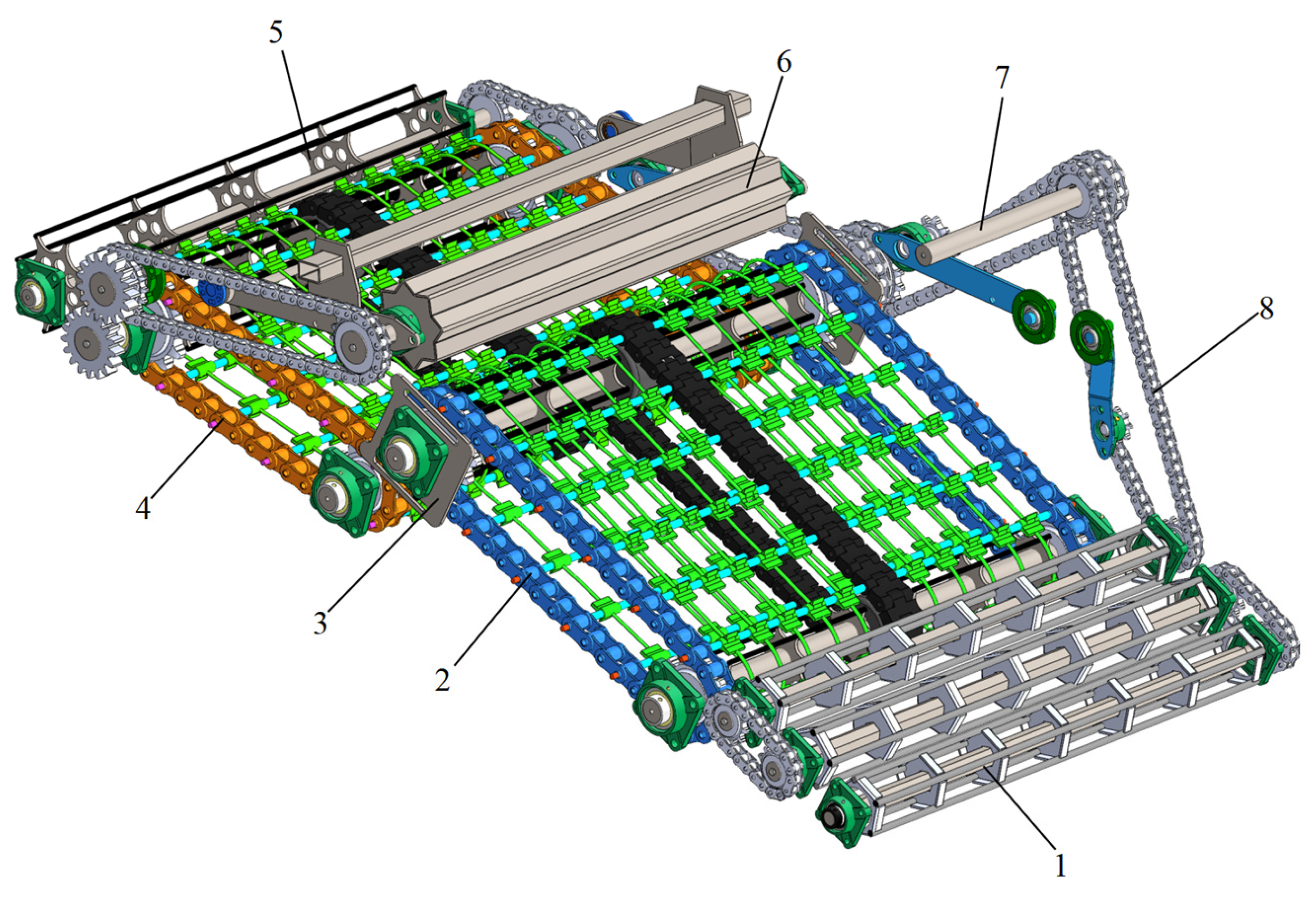
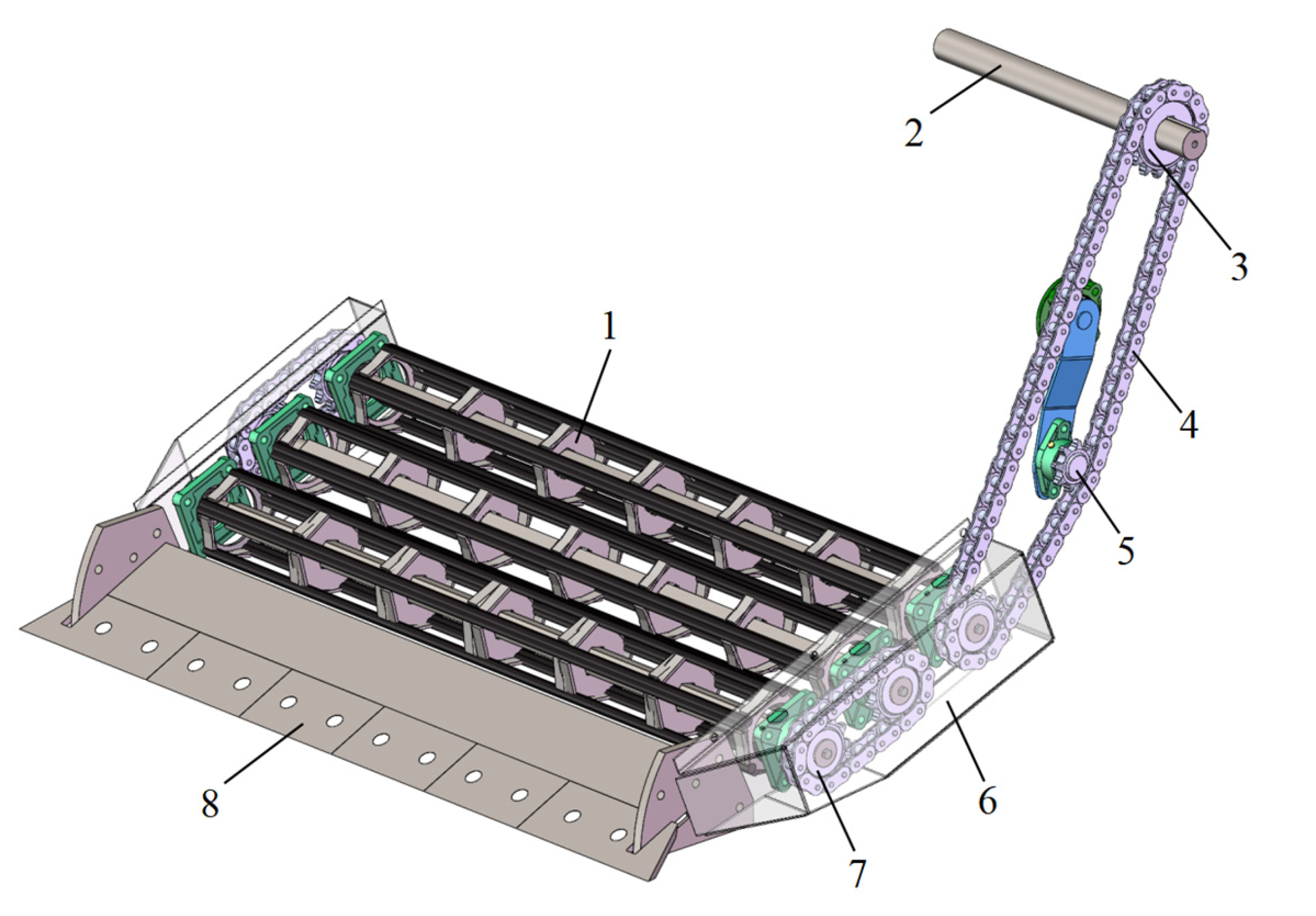
3.2. Structural Design of Conveying and Separating Units

The conveying and separating device is a critical component of root and tuber herb harvesters [16]. Currently, ascending-chain conveying and separating devices are commonly employed in herb harvesting machinery. Given that all moving parts interact with the soil during operation, chain transmission is used to ensure reliable performance of the entire machine. During licorice harvesting, the machine operates at significant digging depths, resulting in a large volume of soil and licorice mixture. As a single-stage screen is insufficient for complete separation, the conveyor separation device utilizes a multi-stage separation structure. Its overall configuration is depicted in Figure 5.

Three sets of first-stage conveyor chain rollers initially perform the preliminary separation of the medicine–soil mixture excavated by the shovel. This mixture is then fed into the second-stage screen, which further conveys and separates the licorice into the collection box. The soil-crushing rollers, positioned atop the frame, break down soil that has not passed through the first-stage screen. Force analysis is conducted for each component, and the optimal dimensions and operating parameters are determined through calculations.

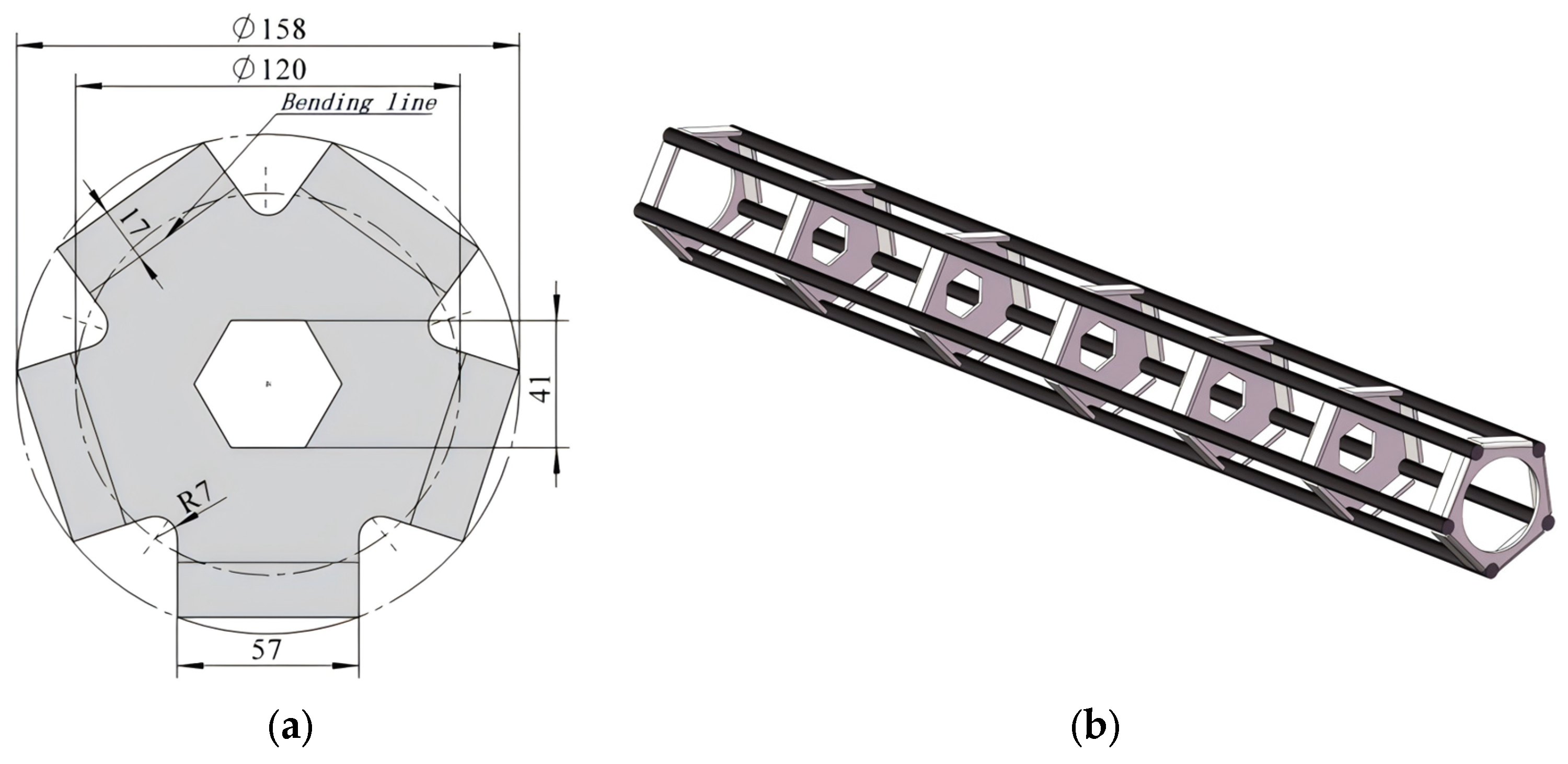
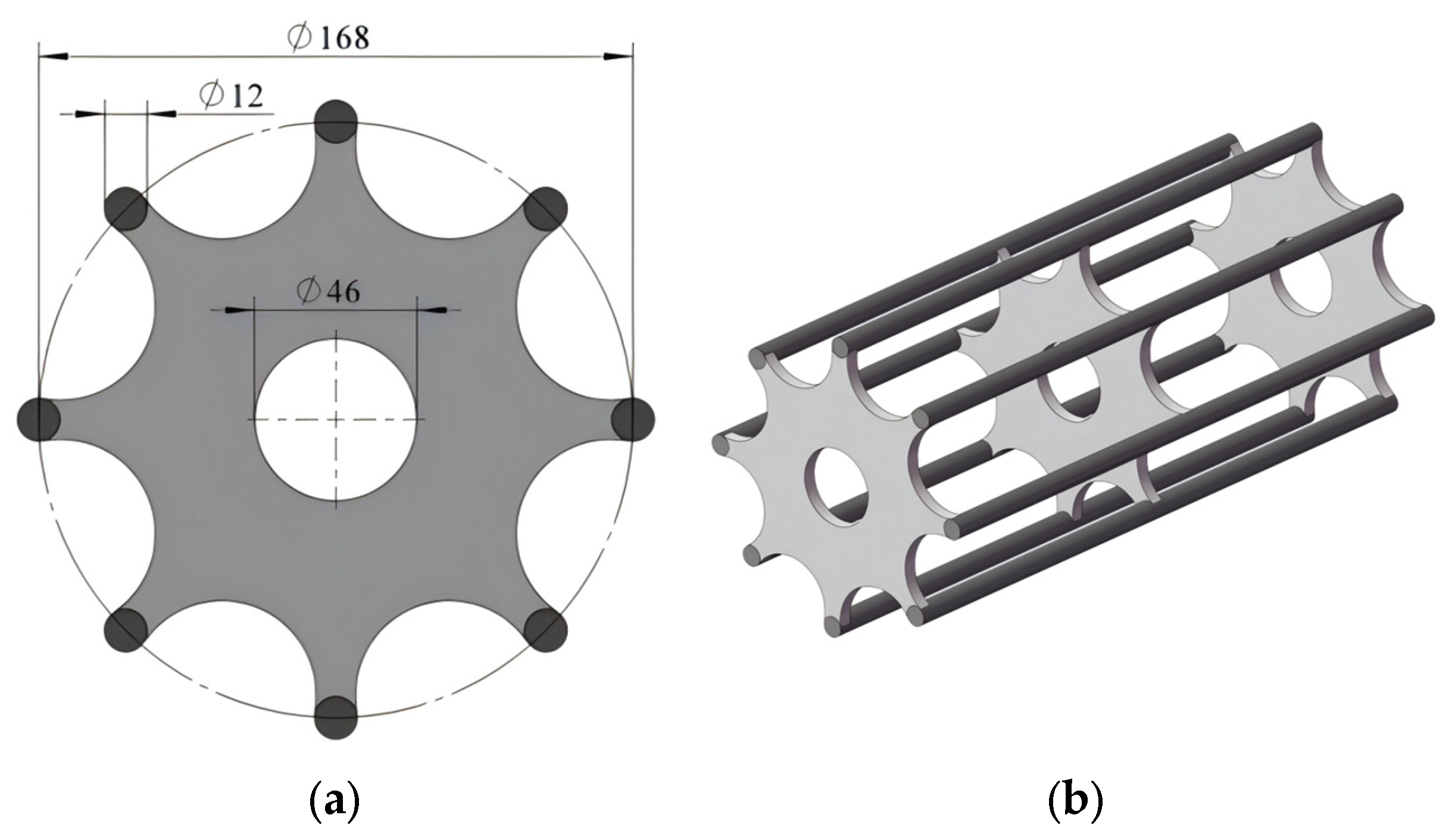
3.2.1. Design of Roller Screen Structure

• Structural design

During the tractor’s forward movement, the digging shovel lifts the medicine–soil mixture. This mixture moves backward along the digging shovel, where the rear feeding rollers break up cohesive soil pieces. Soil that falls through the gaps of the feeding rollers is left on the ground, while larger pieces of soil and licorice that do not pass through are conveyed to the screen by the rotating roller screen. Considering the operating environment, a chain drive is used for power transmission. The system, as shown in Figure 6, comprises an active sprocket, a tensioning wheel, a driven sprocket, and three sets of roller screens. Sprockets are installed at both ends of the second- and third-level feeding roller screens to transfer power. To prevent soil from affecting the chain at the ends of the feeding roller screens, a protective cover is installed, with oil injection holes on top for easy maintenance and inspection in the field.

Based on the designed working width and the overall machine structure, the feed roller screen is 1150 mm in length. Given that the feed roller rotates within the soil and makes contact through friction, the crossbar is constructed from 65Mn steel with a diameter of 14 mm. To ensure effective conveying and soil breaking, the feed roller screen features a pentagonal design. The cross-sectional dimensions are depicted in Figure 7a. The fixed plates and the five middle support plates are made from 5 mm thick 65Mn steel, cut and bent 90° along the bending line. The distance between the fixed plates is 184 mm, and the drive shaft is constructed from hexagonal steel with an opposite side distance of 40 mm. The crossbar is welded to both sides of the fixed plate and support plate, as well as to the hexagonal steel. The assembly is connected to the frame using seated bearings. The overall structure is illustrated in Figure 7b.

The power is transferred from the output shaft at the rear of the tractor to the commutator mounted above the frame. The bevel gear inside the commutator directs power to the left onto the power output shaft. The active sprocket on this shaft transmits power via a chain to the sprocket on the left side of the third-stage roller screen. The left sprocket then transfers power to the right sprocket, which is installed coaxially. This right sprocket passes power to the sprocket on the shaft of the second-stage feeder screen through another chain. The second-stage feeder roller shaft, which is connected to a left-side sprocket installed coaxially, further transmits power to the first-stage feeder screen. Concurrently, the right sprocket on the second-stage feeder screen sends power to the right sprocket on the shaft of the second-stage feeding roller screen. This power is then transferred to the left-side sprocket on the first-stage feeder screen, completing the power transmission cycle from the third stage to the first stage in a left-right-left sequence.

2.

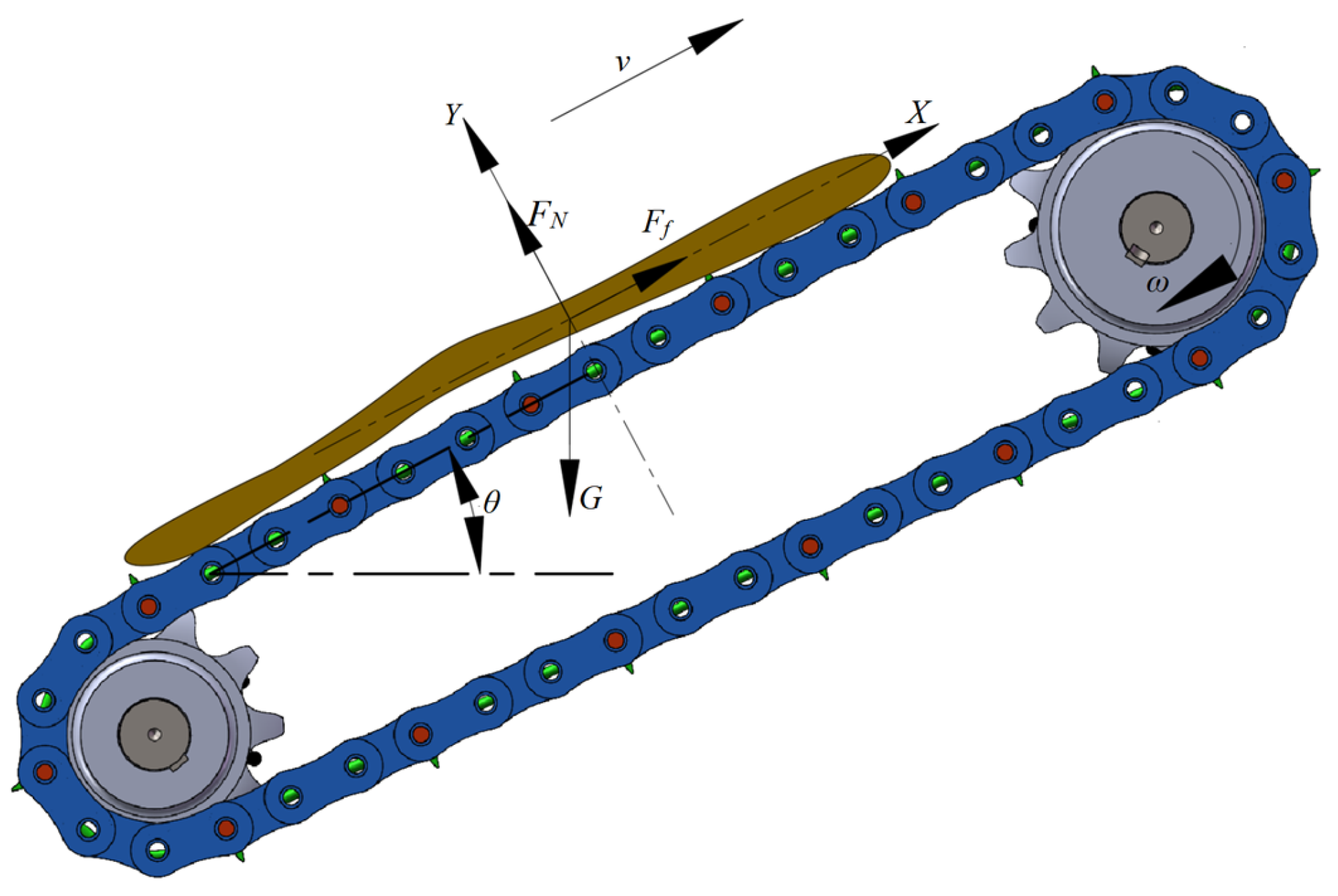
Force analysis of the roller screen

To ensure that the medicine–soil mixture excavated by the digging shovel is smoothly conveyed to the first-stage conveyor chain by the loading roller, a force analysis of the mixture during the loading roller conveyor process is conducted [17]. The analysis considers the soil and licorice excavated per unit time as individual particles. The force analysis diagram is presented in Figure 8.

Then, the equilibrium equation for the force on the drug–soil mixture on the roller screen is

$$\begin{array}{c}{F}_N-Gcos\theta =0\\ F_d-Gsin\theta -F_f-F_s=0\\ G=mg\end{array}$$

(8)

where F_N is the support force of the loading roller screen on the soil mixture, N; G is the gravity of the soil mixture, N; θ is the angle between the three sets of loading roller screen and the horizontal plane, °; F_d is the conveying force provided by the loading roller screen on the soil mixture, N; and F_f is the friction of the soil mixture in the process of movement, N. F_s is the friction force of the drug–soil mixture by the neck of the roller screen, N.

Among them,

$$F_N=Gcos \theta$$

(9)

$$F_d=m{\displaystyle \frac{v^2}{R}}=m{\omega }^{2}R$$

(10)

$$F_f={\displaystyle \frac{2kG}{D}}+\mu F_N$$

(11)

$$F_s={\displaystyle \frac{\left(zq+Gcos \theta /cos \gamma \right)\rho d}{D}}$$

(12)

where ω is the rotational speed of the loading roller screen; k is the rolling friction coefficient of the soil mixture and the loading roller screen, 0.05; μ is the friction coefficient between the soil mixture and the loading roller screen, 0.6; D is the diameter of the loading roller screen, mm; and z is the number of loading rollers screen in contact with the soil mixture, z = 3. q is the mass of the feeding roller screen, kg; γ is the sliding friction angle between the drug–soil mixture and the feeding roller screen, taking γ = 26.5°; ρ is the equivalent friction coefficient of the feeding roller screen, taking ρ = 0.2; and d is the diameter of the shaft of the feeding roller screen, mm.

The following conditions have to be fulfilled in order to smoothly convey the soil–medicine mixture picked up by the excavating shovel to the first-stage conveyor chain screen along the plane of the roller:

$$F_d\ge Gsin \theta +F_f+F_s$$

(13)

Then, the minimum angular velocity of the roller screen that enables the smooth upward conveying of the drug–soil mixture is calculated as follows:

$$\omega =\sqrt{{\displaystyle \frac{DGsin\theta +2kG+\left(zq+Gcos\theta /cos\gamma \right)\rho d}{m DR}}}$$

(14)

The rotational speed of roller screens is calculated as follows:

$$n={\displaystyle \frac{60\omega }{2\pi }}\ge {\displaystyle \frac{30}{\pi }}\sqrt{{\displaystyle \frac{DGsin\theta +2kG+\left(zq+Gcos \theta /cos \gamma \right)\rho d}{m DR}}}$$

(15)

The diameter of the roller screen is D = 120 mm, and the diameter of the roller screen neck is d = 36 mm. The angle between the working plane of the roller screen and the ground is θ = 30°. The mass of the soil mixture conveyed by the roller screen per unit time is q = 400 kg. The critical speed of the roller screen for conveying the soil mixture is calculated to be 143.6 rpm, and the operating speed is set to 150 rpm to accommodate different working terrains and digging depths.

3.2.2. Design of Sieve Screen

• Force of the soil–pharmaceutical mixture on the sieve screen

The licorice and soil mixture is fed into the first-stage conveyor chain by three sets of feed rollers [18]. The mixture is then conveyed diagonally upward along the screen and subsequently thrown diagonally at the end of the screen.

The drug–soil mixture undergoes an oblique upward motion in the working plane surface of the conveyor chain. Air resistance is neglected. The force analysis is shown in Figure 9. The equilibrium equation in this state is

$$\left\{\begin{array}{l}{F}_f-Gsin \theta =0\\ F_N-Gcos \theta =0\\ G=mg\end{array}\right.$$

(16)

where F_f is the friction force between the medicinal soil mixture and the conveyor chain, N; m is the mass of the medicinal soil mixture, kg; g is the acceleration of gravity, taken as 9.8 kg/s²; θ is the angle between the working surface of the conveyor chain and the horizontal plane, °; and F_N is the support force of the conveyor chain on the medicinal soil mixture, N.

The upward movement of the soil mixture along the screen relies on the friction between the soil mixture and the crossbars of the screen to provide upward momentum. If the inclination angle of the screen mesh is too steep, the soil mixture may slide downward. Therefore, optimizing the structure of the screen bars is essential to enhance the friction between the screen and the soil mixture.

2.

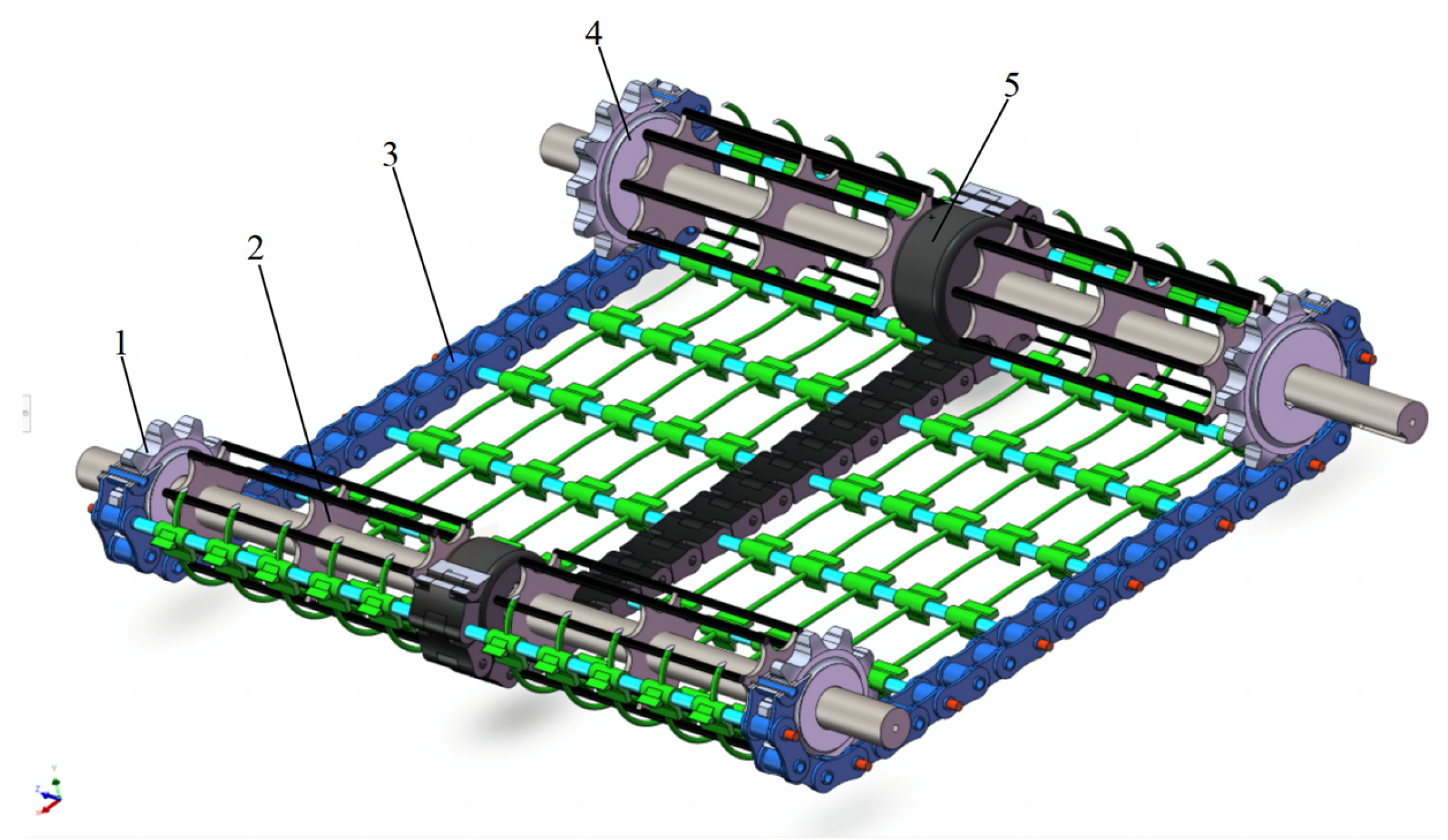
Structural design of lift chain conveyor separation screens

The harvester operates with a significant digging depth. Consequently, a model 32A sprocket and chain are utilized for power transmission in the screen system, with the chain transferring power at both ends. To enhance the strength of the screen, steel support wheels and rubber wheels have been added to the driving and driven shafts, respectively. The structure of the conveyor separation screen is illustrated in Figure 10.

The roller screen conveys licorice and larger clods of soil backward through rotation and then separates them using a conveyor separator mounted at its rear. In accordance with the design specifications and working environment of the screen, the screen’s sides are secured with chains, and power is transmitted via a sprocket drive. The middle section is reinforced with plastic links to enhance stability. The crossbar is made of 65Mn steel, with a diameter of 10 mm, a length of 1166 mm, and a spacing of 153 mm between crossbars. A rubber chain is installed on the crossbar to increase friction between the screen and the soil and licorice mixture, thereby improving the conveying and separation capabilities. The rubber chain is enclosed by a casing, 38 mm in length, to maintain consistent screen hole size throughout operation. The structure is shown in Figure 11.

The drive shaft is reinforced to handle the substantial load imposed on the screen during operation. A rubber wheel is positioned at the center of the drive shaft to support the middle of the screen mesh, while steel support wheels are mounted on both sides of the rubber wheel to provide additional support for the rubber chain. To ensure even distribution of forces on the steel wheels, the support wheels feature a positive octagonal design. The diameter of the steel support wheel on the drive shaft is 168 mm, while the diameter of the steel support wheel on the driven shaft is 145 mm. Eight 12 mm round steel rods are evenly welded onto each steel wheel. The dimensional parameters and structure are illustrated in Figure 12.

Power is transmitted from the power output shaft to the active sprocket on the output shaft. The active sprocket then transfers the power through the chain to drive the first-stage conveyor chain. This chain drives the first-stage conveyor, which in turn powers the second-stage conveyor chain through a sprocket mounted on the first-stage chain. The first-stage conveyor chain’s tension can be adjusted using an adjustment bolt on the frame. To prevent chain vibration caused by tension adjustments, a tensioning sprocket is installed on the two-stage transmission chain.

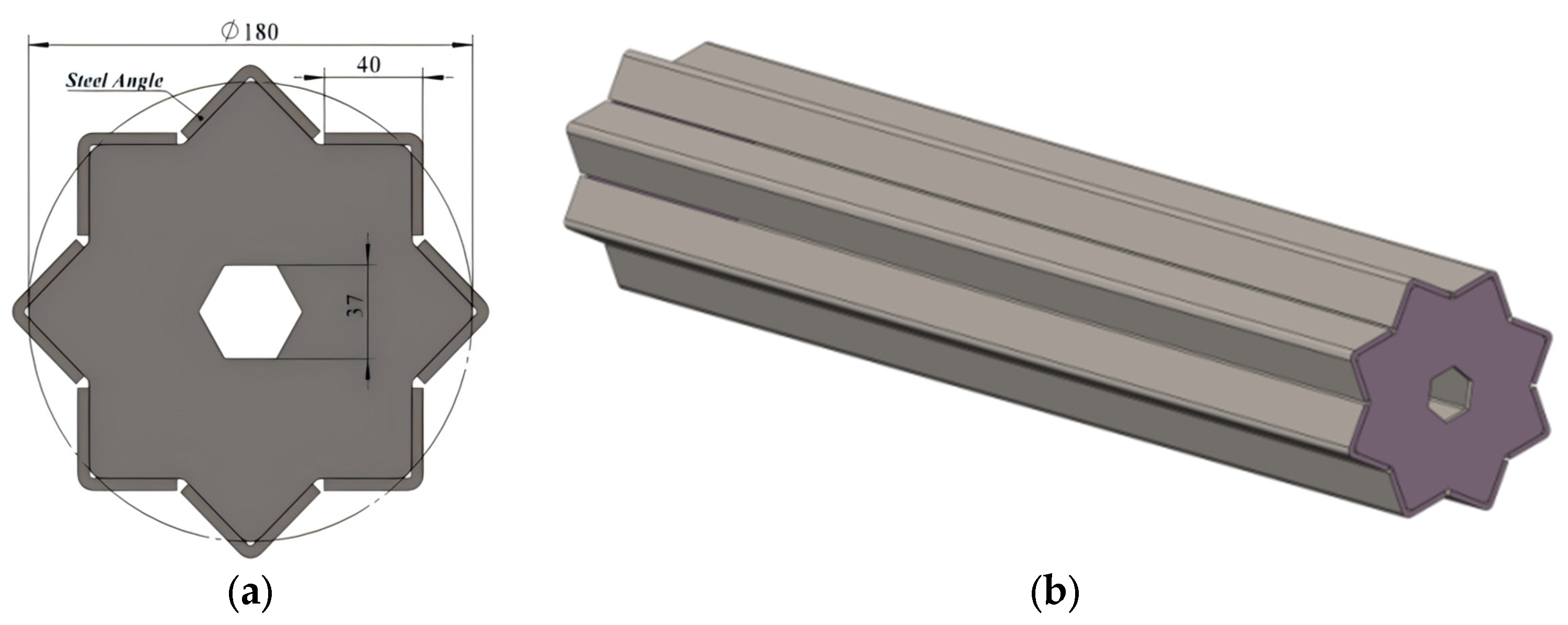
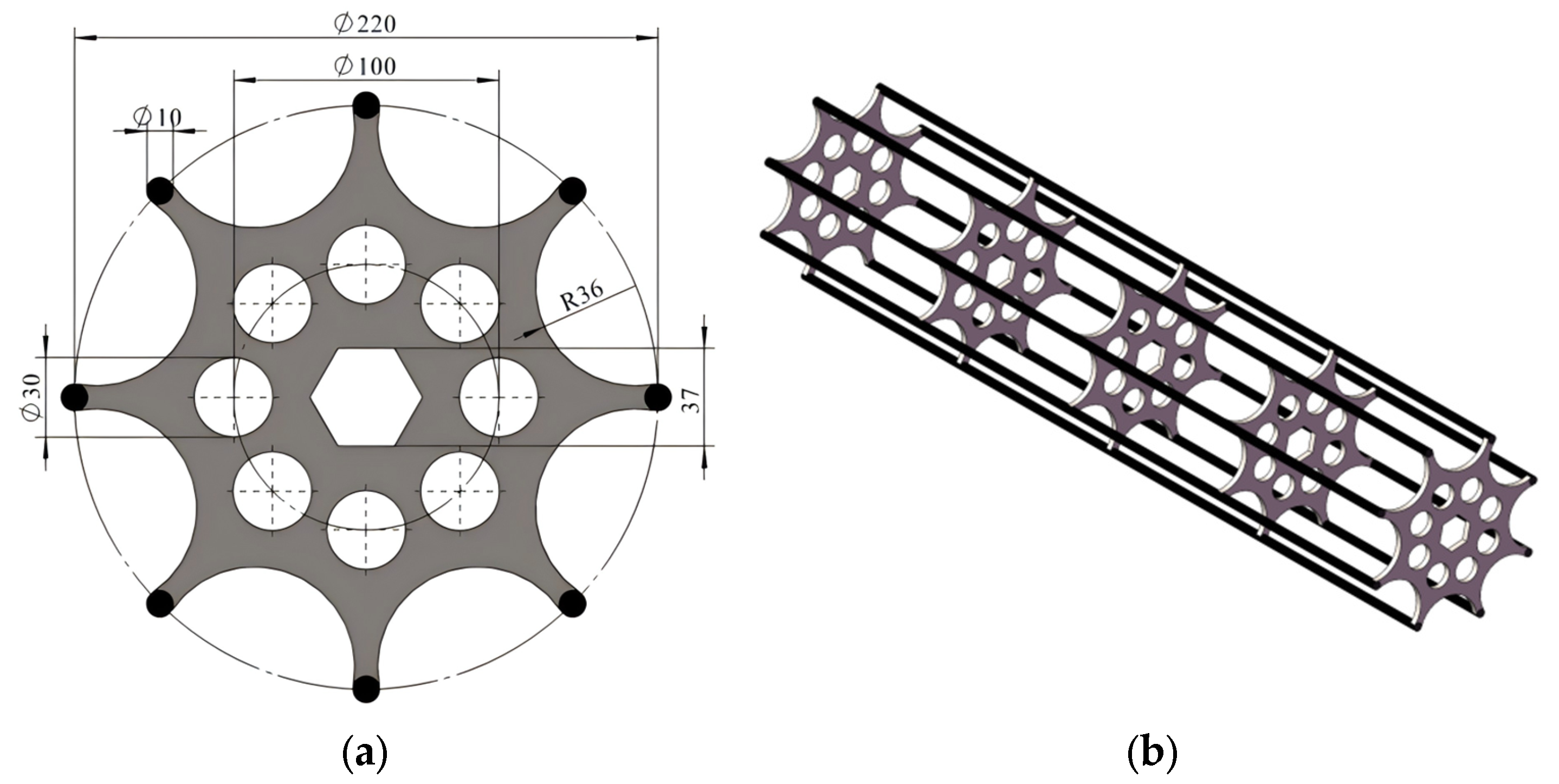
3.2.3. Design of Soil-Crushing Rollers

• Force analysis of soil-crushing rollers

The force diagram of the soil-crushing rollers is shown in Figure 13. To pass through the gap between the soil-crushing rollers and the first-stage conveyor chain, the soil must be crushed sufficiently. The soil, represented as a sphere with a diameter d, will only pass through if the friction between the soil and the soil-crushing rollers exceeds the friction between the soil and the first-stage conveyor chain. In other words, the frictional force exerted by the soil-crushing rollers on the soil must be greater than the frictional force exerted by the first-stage conveyor chain on the soil. That is,

$$F_f\ge \mu F_N$$

(17)

$$\mu \ge {\displaystyle \frac{sin \alpha }{cos \alpha }}$$

(18)

where F_f is the friction force of the soil-crushing rollers on the soil block, N; F_N is the support force of the soil-crushing rollers on the soil block, N; α is the angle between the line connecting the soil-crushing rollers and the first-stage conveyor chain rotating center and the geometrical centre of the soil, °; and μ is the friction coefficient between the soil-crushing rollers and the soil block, which is taken as 0.07.

According to the geometrical relations,

$$\cos \alpha ={\displaystyle \frac{D+H}{D+d}}$$

(19)

$$\sin \alpha =\sqrt{1-{\left({\displaystyle \frac{D+H}{D+d}}\right)}^2}$$

(20)

Combining Equations (19) and (20) with the inequality Equation (18) yields

$$\mu \ge \sqrt{{\displaystyle \frac{{\left(D+d\right)}^2-{\left(D+H\right)}^2}{{\left(D+H\right)}^2}}}$$

(21)

where D is the diameter of the soil-crushing rollers, mm, and d is the diameter of the soil block, mm.

The diameter of the soil-crushing rollers is calculated as follows:

$$D={\displaystyle \frac{d-H\sqrt{1+{\mu }^2}}{\sqrt{1+{\mu }^2}-1}}$$

(22)

According to the formula, the size of the soil-crushing rollers is influenced by the coefficient of friction (μ) between the crushing rollers and the clods, the diameter (d) of the clods, and the vertical distance (H) between the crushing rollers and the first-stage conveyor chain. The friction coefficient (μ) between the crushing rollers and soil blocks ranges from 0.5 to 0.7. The first-stage conveyor chain can handle soil blocks with a minimum size of 100 mm. To ensure that soil blocks crushed by the rollers can be effectively screened by the second-stage conveyor chain, the size of the crushing rollers and the first-stage conveyor chain should be less than 100 mm. Additionally, the vertical distance between the crushing rollers and the first-stage conveyor chain should be 80 mm. While a larger diameter of the crushing rollers improves the soil-crushing effect, it also increases the size of the entire machine, which can lead to higher power consumption by the tractor.

2.

Soil-crushing rollers’ structural design

The first-stage conveyor chain transports the soil and licorice mixture excavated by the shovel backward to the first-stage screen. Smaller soil particles fall through the screen holes, while slightly larger particles that cannot pass through are conveyed to the end of the first-stage screen [19]. At this point, the soil-crushing rollers, installed at the end of the first-stage conveyor chain, crush the larger soil particles into smaller pieces. These smaller pieces then enter the second-stage conveyor chain for further separation. The second-stage conveyor chain, which consists of sprockets, soil-crushing rollers, bearing seats, crossbars, connecting plates, and adjusting plates, handles this process.

For the soil-crushing rollers to effectively crush the soil, they must rotate in the opposite direction to the linear velocity of the first-stage conveyor chain. This is achieved by using an external meshing gear to change the direction of power rotation. The active gear is mounted on the active shaft of the second-stage conveyor screen, while the driven gear and soil-crushing rollers’ active sprocket are mounted coaxially. These components are fixed to the frame with pedestal bearings, and the active sprocket drives the soil-crushing rollers’ sprocket through a chain. The structure is illustrated in Figure 14.

The size, shape, and mounting position of the soil-crushing rollers significantly impact the quality of the soil crushed and the overall efficiency of the harvester. Cylindrical soil-crushing rollers have limited crushing capacity and can lead to congestion in the first-stage conveyor chain when handling large amounts of soil. The soil-crushing rollers are fixed on both sides of a plate, with an eight-sided cross-section and angle welds of 40 mm on each side. The drive shaft is constructed from hexagonal steel, with opposite sides spaced 36 mm apart. This design is simple, durable, and easy to manufacture. The soil-crushing rollers’ shaft is connected to the adjusting plate via a seated bearing. Additionally, the left and right connecting plates are attached to the crossbar above the soil-crushing rollers through the adjusting plate to enhance structural stability. The entire assembly is mounted on the frame through a bearing seat, allowing the soil-crushing rollers’ device to rotate around the bearing seat to adjust the spacing between the soil-crushing rollers and the first-stage conveyor chain. The structure of the soil crushing roller is shown in Figure 15:

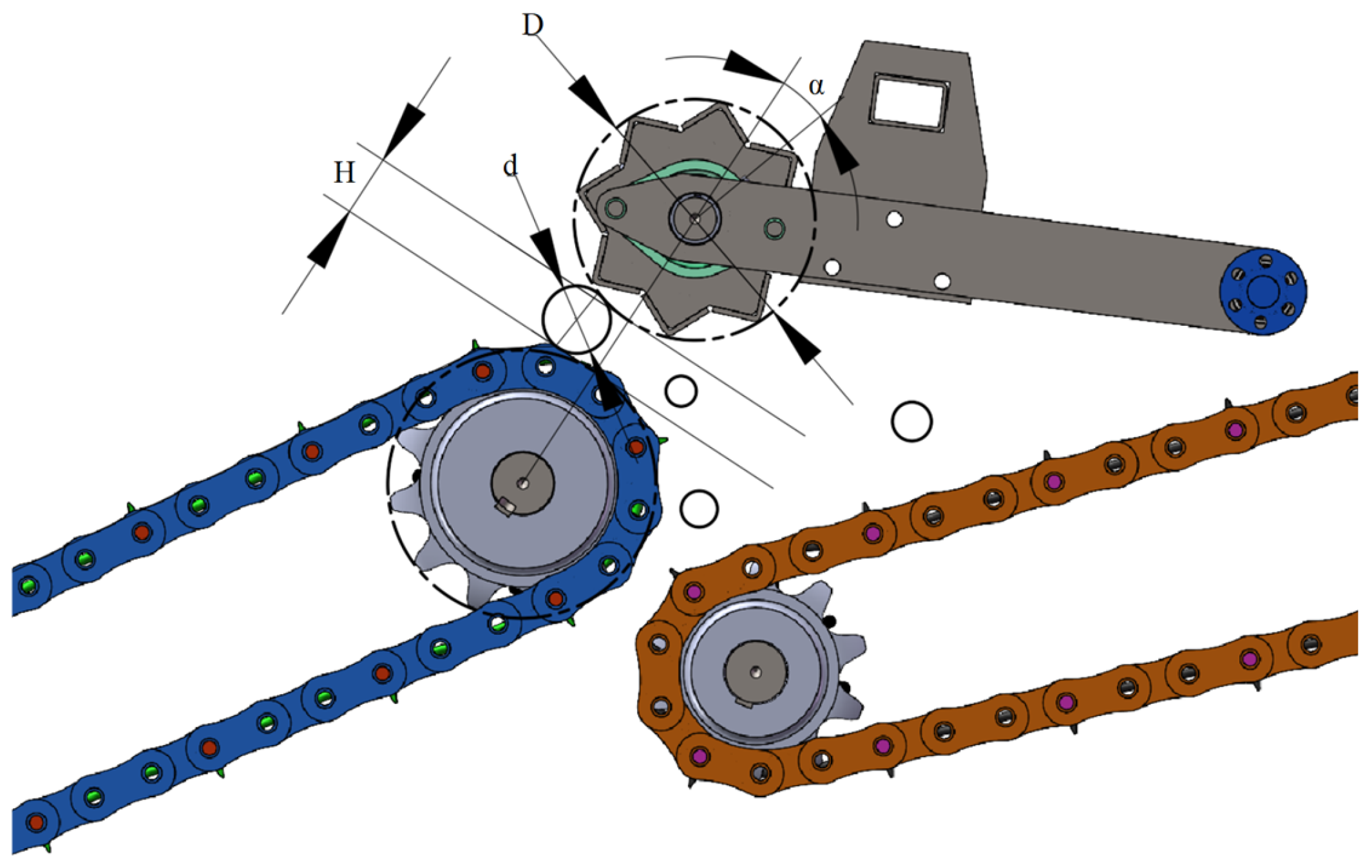
3.2.4. Structural Design of Rear Support Wheel

By analyzing the movement of the soil–medicine mixture from the moment it leaves the second-stage conveyor chain to when it falls into the medicine collection box, it becomes evident that the large differences in the geometrical structures of licorice and soil result in distinct movement behaviors and trajectories as they exit the screen. To address this, a pivoting wheel device can be introduced between the end of the second-stage conveyor chain and the collection box [20,21]. This device will facilitate the conveyance of licorice, which is in the form of long rods, to the collection box while allowing the soil to be sifted out through the gaps. The process of transferring licorice from the end of the second-stage conveyor chain to the collection box can be divided into four stages, as illustrated in Figure 16:

a.

Screen-Conveying Stage: When the top of the licorice begins to leave the screen, its center of gravity remains on the screen, and it continues to move upwards with the screen;

b.

Tilting and Touching the Wheel Stage: Once the center of gravity of the licorice leaves the plane of the screen, its head starts to fall. At this stage, the licorice contacts the rotating rear paddle wheel, which supports the tilting and falling licorice and lifts it upward through the rotation of the paddle wheel;

c.

Paddle Wheel Support Stage: As the rear paddle wheel rotates, it fully supports the licorice;

d.

Leaving the Wheel and Entering the Box Stage: The licorice exits the paddle wheel and falls into the collection box. Meanwhile, soil blocks, which are subject to an oblique throwing movement upon leaving the screen, will fall through the gaps between the crossbars of the paddle wheel and land on the ground.

• Analysis of soil oblique throwing motion

When the licorice and the unseparated clods of soil are conveyed together to the end of the second-stage conveyor chain, their velocity matches the linear velocity of the conveyor chain. Neglecting air resistance during the inclined throwing motion, the soil block exhibits uniform linear motion in the horizontal direction. Vertically, the soil block first moves with uniform deceleration until it reaches the highest point of its trajectory, after which it descends with uniform acceleration. This behavior is illustrated in Figure 17.

The soil block is thrown obliquely from the point where it leaves A. The velocity in the horizontal and vertical directions is calculated as follows:

$$\left\{\begin{array}{l}{v}_x=v_{0}cos\theta \\ v_y=v_{0}sin\theta \end{array}\right.$$

(23)

where v_x is the partial velocity of the soil block along the horizontal direction, m/s; v_y is the partial velocity of the soil block along the vertical direction, m/s; and v₀ is the linear velocity of the second-stage conveyor chain, m/s.

The velocity in the vertical direction is 0 when moving to point B according to the following formula:

$$\begin{array}{c}{v}_y=g{t}_1\\ -{v_y }^2=-2g{H}_1\end{array}$$

(24)

The time t₁ and displacement H₁ required for the block to move upwards to the highest point can be obtained by

$$t_1={\displaystyle \frac{v_{0}sin\theta }{g}}$$

(25)

$$H_1={\displaystyle \frac{{\left(v_{0}sin\theta \right)}^2}{2g}}$$

(26)

where g is the acceleration of gravity, 9.81 m/s².

The soil block leaves point B and makes a parabolic motion in the section BC, according to the following equation:

$$\left\{\begin{array}{c}{H}_0=R_{1}cos\theta \\ H_{max}={\displaystyle \frac{1}{2}}g{t_2 }^2\\ H_{max}=H_1+H_0\end{array}\right.$$

(27)

where R₁ is the second-stage conveyor chain active sprocket teeth’s top circle diameter, mm.

The time taken by the soil block in the section BC can be obtained by the following:

$$t_2=\sqrt{{\displaystyle \frac{2R_{1}cos\theta +{\left(v_{0}sin\theta \right)}^2}{g^2}}}$$

(28)

Total time taken for the movement of the soil block to be thrown obliquely can be calculated by the following equation:

$$t=t_1+t_2={\displaystyle \frac{v_{0}sin\theta }{g}}+\sqrt{{\displaystyle \frac{2R_{1}cos\theta +{\left(v_{0}sin\theta \right)}^2}{g^2}}}$$

(29)

Then, the maximum displacement of the soil block in the maximum horizontal direction after it is thrown obliquely can be calculated by

$$X=v_{x}t={\displaystyle \frac{{v_0 }^{2}sin2\theta }{2g}}+v_{0}cos\theta \sqrt{{\displaystyle \frac{2R_{1}cos\theta }{g}}+{\displaystyle \frac{{\left(v_{0}sin\theta \right)}^2}{g^2}}}$$

(30)

In order to prevent the soil from entering the collector box after being thrown diagonally at the end of the second-stage conveyor chain, the minimum distance between the collector box and the main shaft of the second-stage conveyor chain can be calculated by the following equation:

$$X_1=X-R_{1}sin\theta$$

(31)

Then, the maximum diameter of the rear support wheel can be calculated by

$$D_b=X-R_1\left(1+sin\theta \right)$$

(32)

2.

Rear support wheel structure design

The direction of rotation of the toggle wheel when it works is the same as the direction of rotation of the screen’s main wheel, so its power source is the second-stage conveyor chain driven by the main shaft of the screen. The transmission device is shown in Figure 18.

The support part is made of five fixed plates made of steel plate with a diameter of 220 mm and a thickness of 8 mm, its structure can be simplified in order to reduce its mass, and the working crossbars with a diameter of 10 mm are welded at equal distances on the eight vertices of the fixed plates. The drive shaft is made of hexagonal steel, with a distance of 36 mm from the opposite side. The dimensional parameters are shown in Figure 19a. The intermediate support plates are spaced at 280 mm. The structure is shown in Figure 19b.

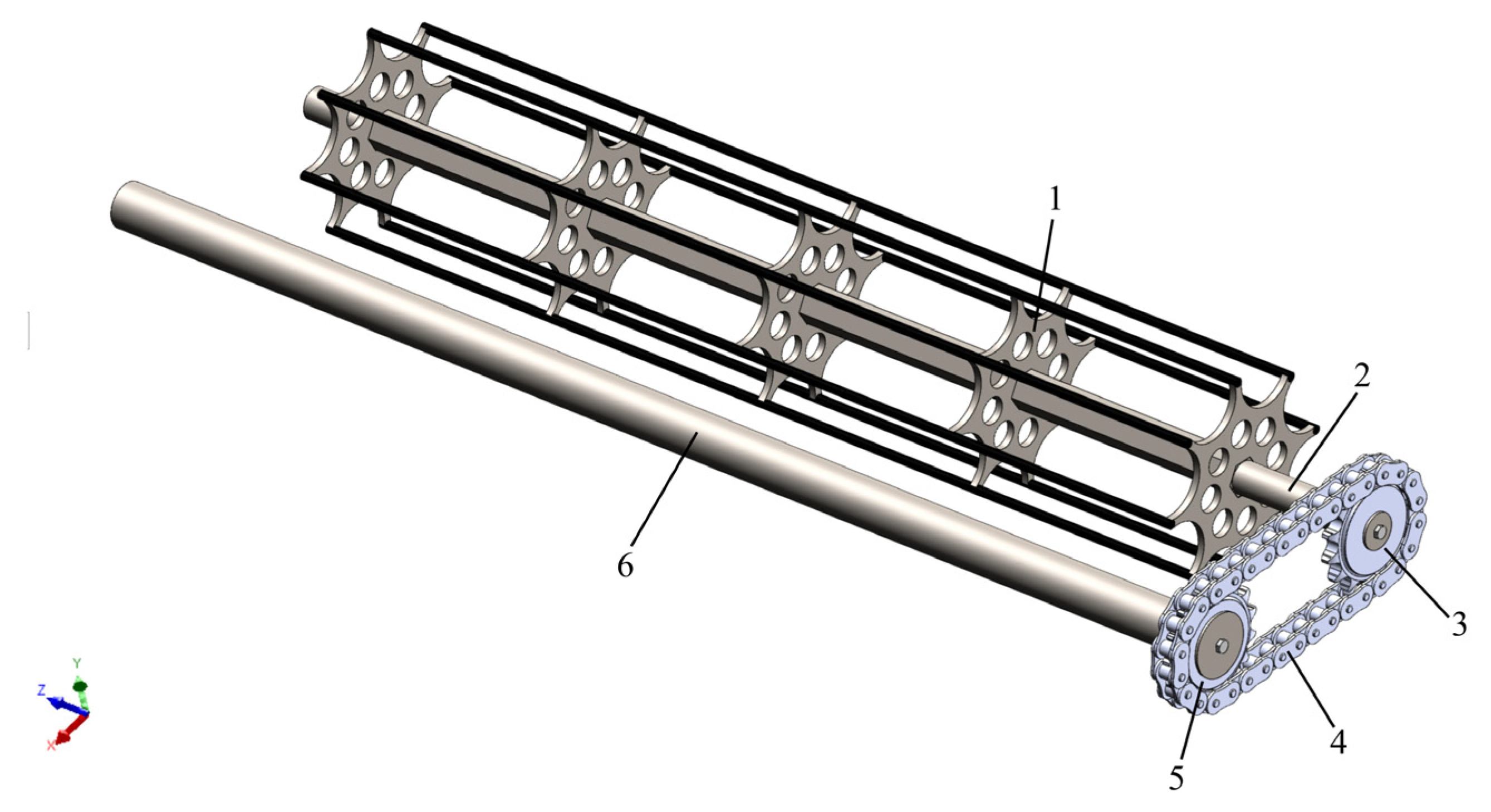
3.3. Structural Design of Power Transmission Part

The transmission system of the harvester mainly adopts chain drive, and the tractor transmits the power to the gearbox on the top of the frame through the universal joint drive shaft, and then the power is transmitted to the front roller screen and the rear conveyor chain through the output shaft of the gearbox. The structural diagram of the transmission system is shown in Figure 20.

The rated rotational speed of the tractor engine output shaft is n. The rotational speeds of the sprockets at all levels in the chain drive can be calculated by the following formula:

$${\displaystyle \frac{n_z}{n_c}}={\displaystyle \frac{Z_z}{Z_c}}$$

(33)

where n_z is the rotational speed of the active sprocket, r/min; n_c is the rotational speed of the driven sprocket, r/min; Z_z is the number of teeth of the active sprocket; and Z_c is the number of teeth of the driven sprocket.

The linear velocity of each component can be calculated from the following equation by means of the speed of the drive shaft of the main wheel at each level:

$$v_d=n_z\times \pi d_z$$

(34)

where d_z is the main sprocket indexing circle diameter, mm.

4. Results

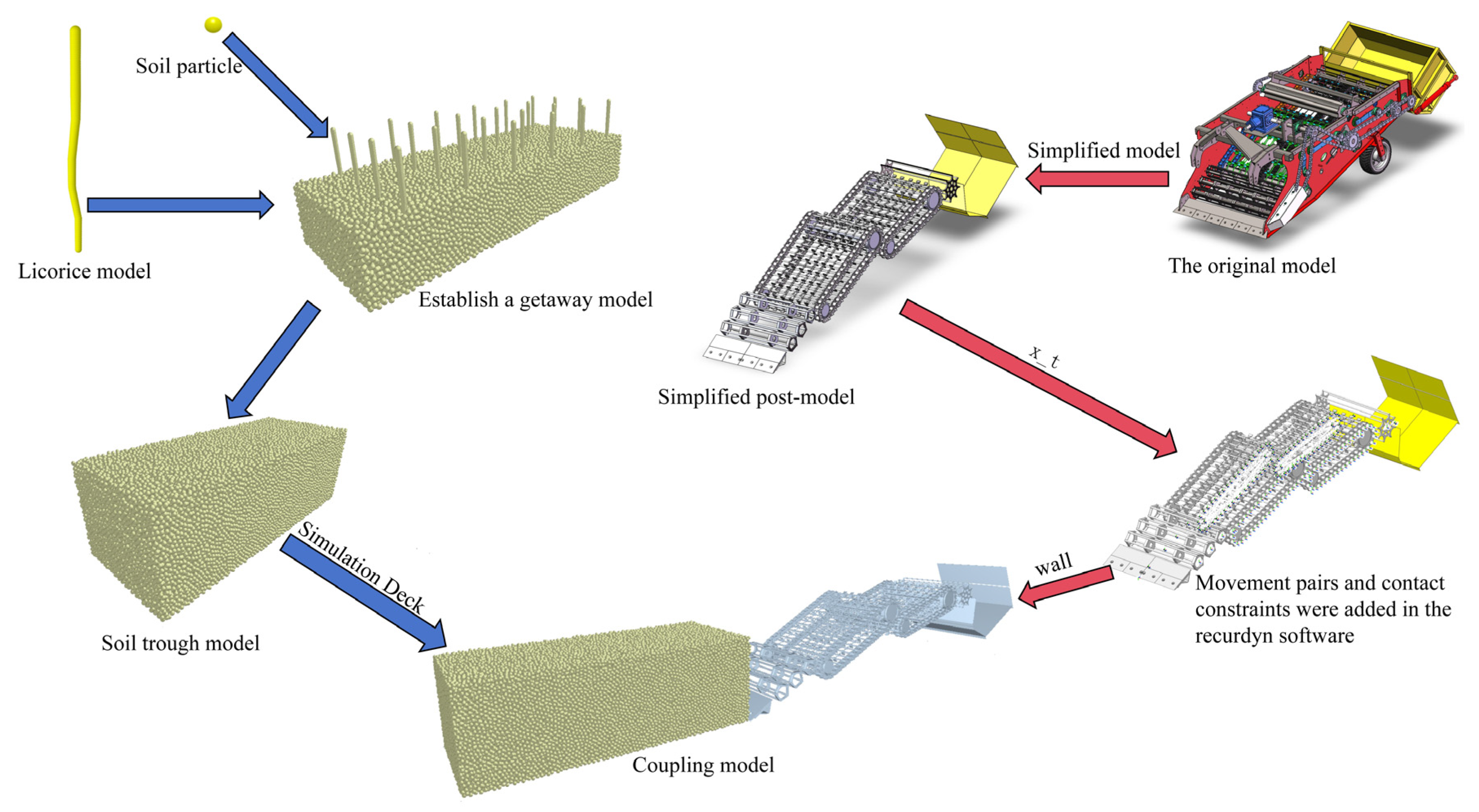
4.1. EDEM Modelling

4.1.1. Contact Model Selection and Parameterization

The discrete element simulation parameters are set as shown in

Table 2. Basic parameters of discrete element simulation.

Materials	Poisson’s Ratio	Shear Modulus/pa	Densities (kg/m3)
soil	0.3	5 × 107	2600
licorice	0.416	1.668 × 107	840
steel	0.3	7.9 × 1010	7860

and

Table 3. Contact parameters of discrete element simulation model.

Contact Type	Coefficient of Static Friction	Coefficient of Rolling Friction	Coefficient of Restitution
Soil–soil	0.68	0.27	0.21
Soil–licorice	0.453	0.17	0.587
Soil–steel	0.31	0.13	0.54
Licorice–licorice	0.453	0.086	0.587
Licorice–steel	0.349	0.074	0.509

[22]. Some of the unknown parameters are measured according to the method shown in the literature [23], and the Hertz–Mindlin (no-slip) model is used for the contact model between particles.

4.1.2. Modeling of Licorice Soils

Licorice grows vertically in the soil. To ensure that the simulation process accurately reflects the actual working conditions of the licorice harvester, the licorice model in the simulation is designed to mimic the characteristics of real licorice. The model uses 100 particles, with the largest particles having a radius of 25 mm, the smallest particles having a radius of 8 mm, and an overall length of 525 mm. For the soil particles, taking into account the actual soil conditions and the computational limits of the software, the model uses 8 mm single-sphere particles.

A discrete element model was established as shown in Figure 21. A soil tank with dimensions of 2000 mm (length) × 710 mm (width) × 1000 mm (height) was created. Particle factories for generating both the licorice model and soil particles were set up. In EDEM, the orientation of the licorice model was controlled to be parallel to the Y-axis, and the row spacing of the licorice model was adjusted according to agronomic requirements for licorice cultivation. The generated licorice model was then fully covered with loam particles to a thickness of 600 mm, which accurately reflects the natural growing conditions of licorice. This setup was used for harvesting test simulations.

4.2. Coupling Modeling

The original model was simplified using SolidWorks software 2023 and saved as an x_t file. This file was then imported into RecurDyn 2023 software, where motion subsystems and constraints were added to build a multi-body dynamics model. A wall file was generated and saved. The soil tank model was exported to the simulation deck in the post-processing module of EDEM 2022.3. After opening the file, the generated wall file was imported, and the relative position between the two models was adjusted. The coupled separation simulation model is shown in Figure 22.

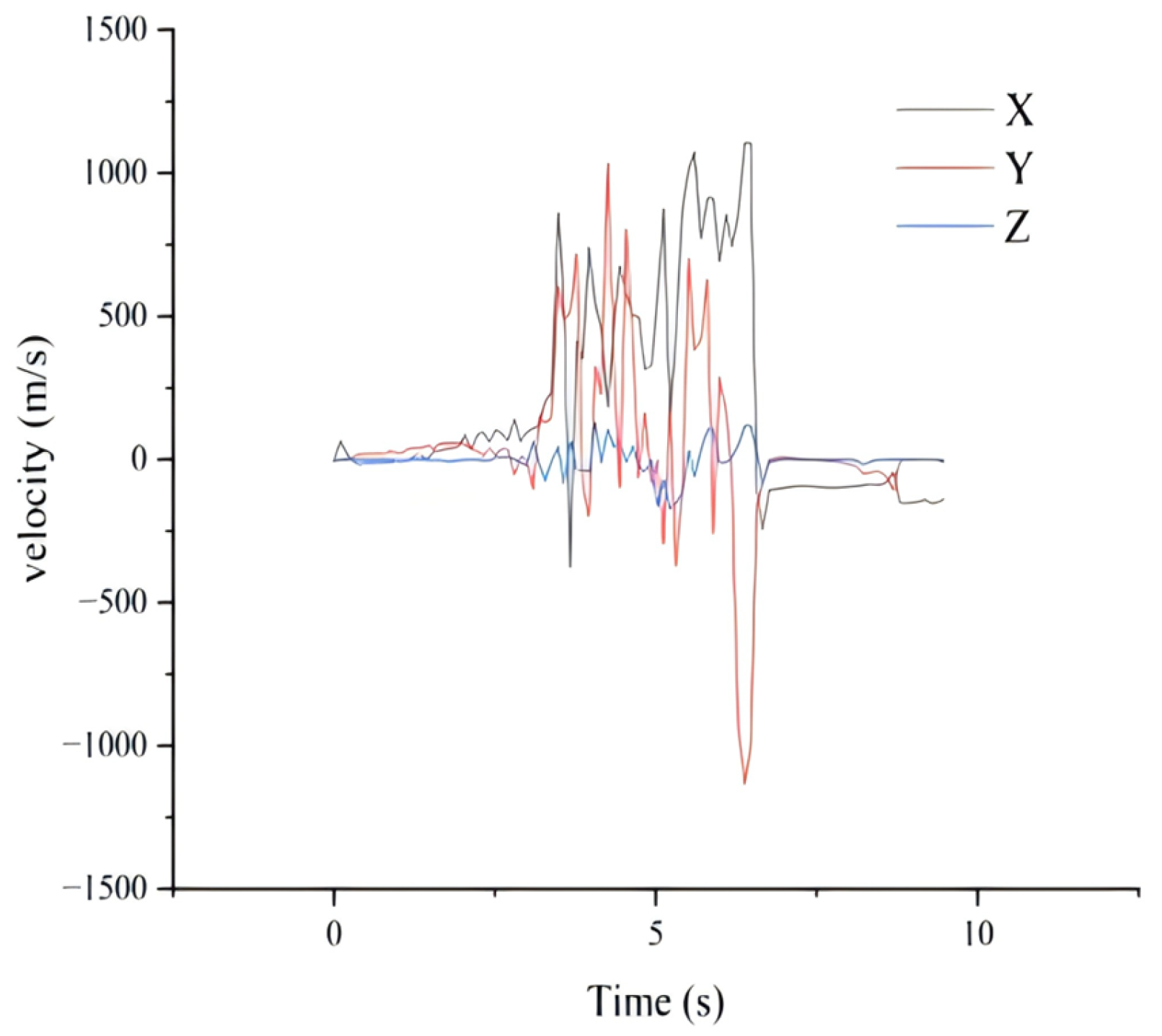
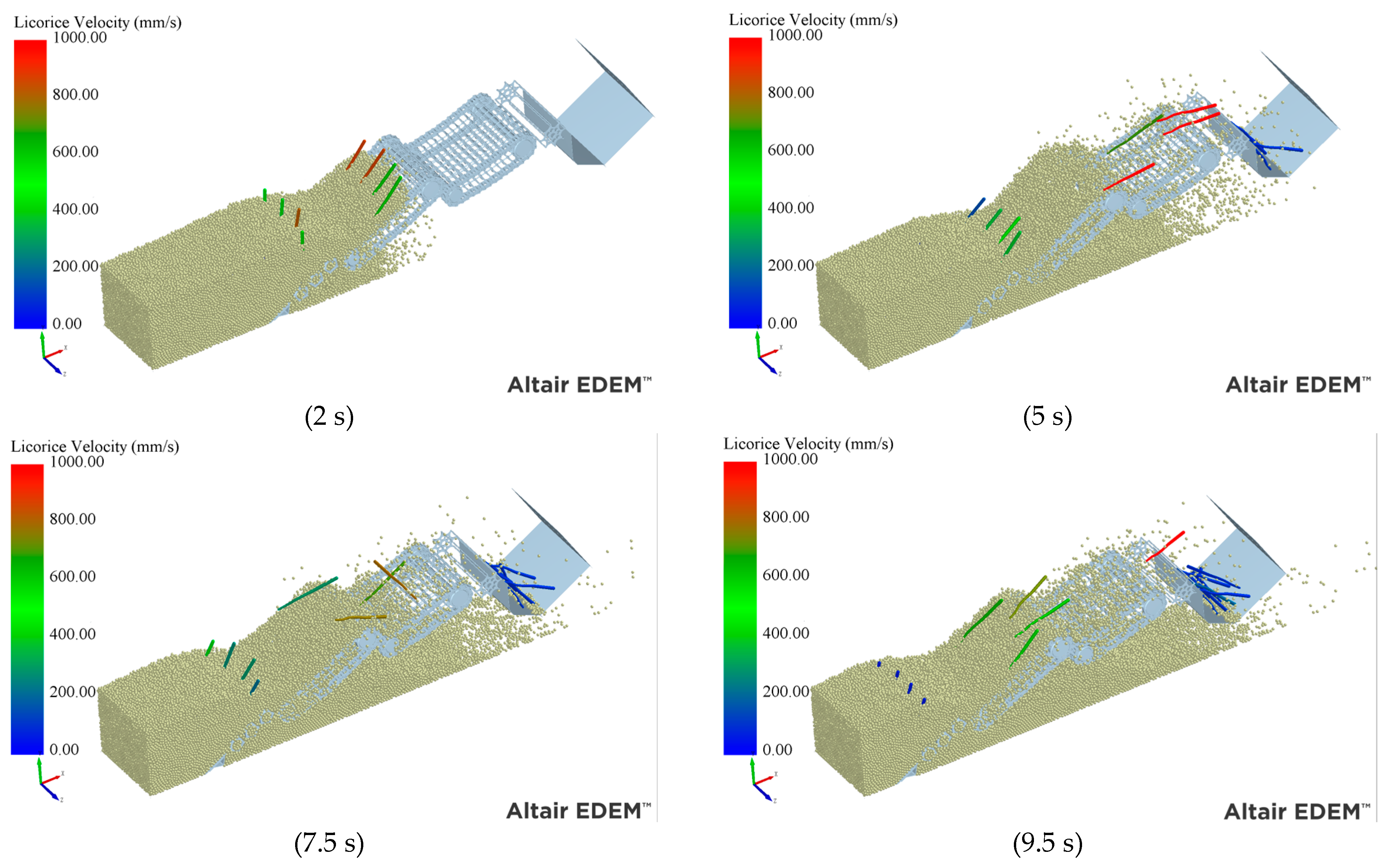
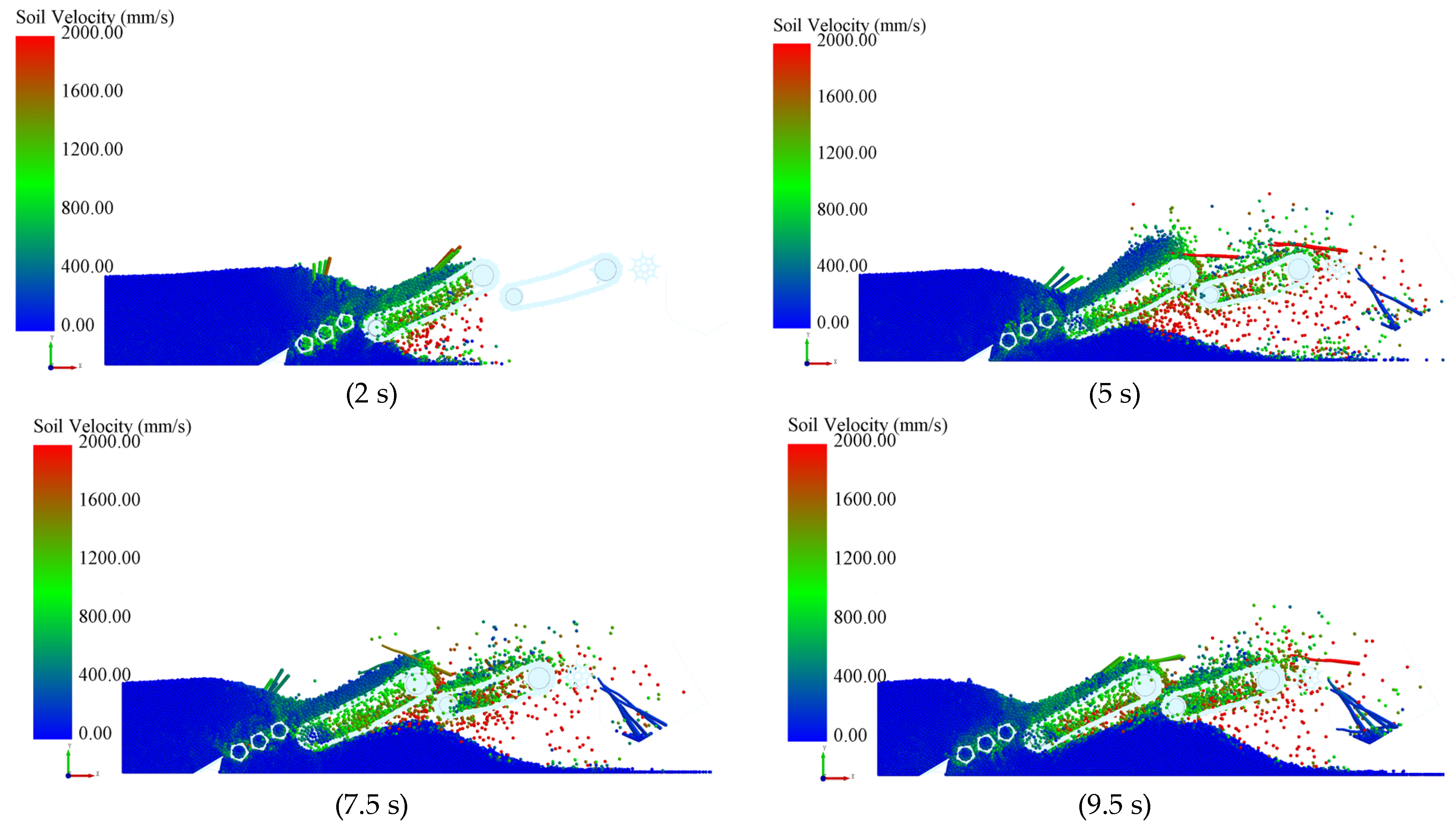
4.3. Simulation Results and Analysis

The simulation process revealed significant variations in the speed of licorice when it interacts with the loading roller screen and the screen itself during harvesting. To analyze these variations, two licorice models were selected in EDEM 2022.3 post-processing to examine their trajectories and velocities [24]. The velocity curves for four adjacent licorice models were plotted, as shown in Figure 23. The motion trajectories are illustrated in Figure 24.

The trajectory analysis during the simulation shows that the movement trend of each licorice model is generally consistent throughout the harvesting process. Figure 25 illustrates the velocity distribution of the licorice during the simulation. Initially, at 0.1 s, the digging shovel lifts the licorice along the shovel surface, causing a change in its velocity. As soil particles around the licorice model are progressively removed by the loading roller, the licorice begins to tilt towards the roller. At 1.9 s, when the licorice model contacts the loading roller, friction between the roller and the licorice causes the model to accelerate upward. By 3.35 s, as the licorice model leaves the loading roller and contacts the first-stage conveyor chain, it encounters a high density of soil particles. This causes irregular fluctuations in the model’s velocity between 3.35 and 4.6 s, as the soil particles slide between the model and the conveyor chain. As the soil particles are gradually removed from the first-stage conveyor chain, the licorice begins to make better contact with the sieve and accelerates, though its velocity remains below that of the first-stage conveyor chain. At 4.8 s, the model contacts the second-stage conveyor chain. Due to the reduced soil-particle density on this chain, the licorice accelerates more quickly compared to the first-stage conveyor chain. By 6.2 s, the licorice model leaves the second-stage conveyor chain and contacts the rear pivot wheel, which further accelerates it. The licorice enters the medicine collection box at 6.5 s and achieves a consistent speed with the forward motion of the machine by 6.7 s.

The velocity distribution of soil particles during the simulation is depicted in Figure 26. Initially, as the machine moves forward, soil particles are shoveled up and begin to contact the loading roller at 0.3 s. The rotation of the loading roller causes these particles to fall through the gaps in the crossbars. By 0.95 s, the soil particles come into contact with the first-stage conveyor screen. As the sieve mesh operates, the soil particles are progressively sifted. At 3.2 s, after the first-stage conveyor chain has been running, some of the drug–soil mixture remains unseparated, leading to a minor accumulation. This mixture continues onto the second-stage conveyor chain for further separation. By 4.67 s, the first licorice model starts falling into the collection box, while some of the drug–soil mixture is still being separated by vibration on the screen. After 7.5 s, under continuous separation by the conveyor chain, the mass of soil particles on the first-stage conveyor chain reaches a dynamic equilibrium, and no further accumulation occurs.

The X-axis coordinate values of the licorice model at different moments are shown in Figure 27. At the moment when the first licorice model fell into the medicine collection box, its displacement in the X-direction was 2098 mm, and its vertical displacement was 660 mm. This indicates a clear separation effect. Throughout the separation and conveying process, the licorice and soil moved together, driven by the conveyor chain, with minimal contact and abrasion between the model and the sieve mesh. The medicine–soil mixture was effectively transported backward by the conveyor separator screen, with no significant accumulation observed on the screen during the simulation. At the end of the simulation, only a few soil particles remained in the drug collection box. The conveyor separator screen fulfilled the actual working requirements. The simulation test results demonstrate that the designed conveyor separation device operates stably, with a clear and effective separation of soil and licorice. There was no accumulation of the drug–soil mixture at the first-stage conveyor chain, and the damage to the licorice rhizome was minimal. The licorice was efficiently collected into the drug collection box, meeting the design requirements for drug–soil separation.

4.4. Determination of Optimum Working Parameters

The simulation results reveal that, due to the large excavation depth of the machine, a significant amount of soil particles remain on the first-stage conveyor chain after the drug–soil mixture has passed through the first-stage conveyor chain and screen. These residual soil particles are then conveyed to the second-stage conveyor chain along with the movement of the first-stage conveyor chain. Some of the soil particles are removed by the first-stage conveyor chain during this process. Soil particles entering the second-stage conveyor chain are subsequently screened again, with those not screened out being thrown diagonally into the collection box. Therefore, the number of soil particles present on the second-stage conveyor chain directly influences the number of soil particles that end up in the collection box, affecting the overall screening efficiency.

To assess this, the effects of the unit’s working angle and the speed of the first-stage conveyor chain on conveying performance were first explored. Subsequently, the impact of the machine’s forward speed and screen speed on separation efficiency was investigated. The test measures the conveying rate and screening rate. The conveying rate is defined as the percentage of licorice models conveyed to the collection box by the two-stage screen relative to the total number of licorice models entering the screen per unit time. The screening rate is the percentage of the mass of actual sieved material compared to the mass of material that should have been sieved.

Further investigation into the factors affecting the machine’s efficiency was conducted through interaction tests. These tests varied the conveyor chain speed, unit working inclination, and forward speed of the unit, with the results being measured as the mass of the drug–soil mixture on the second-stage conveyor chain at different moments.

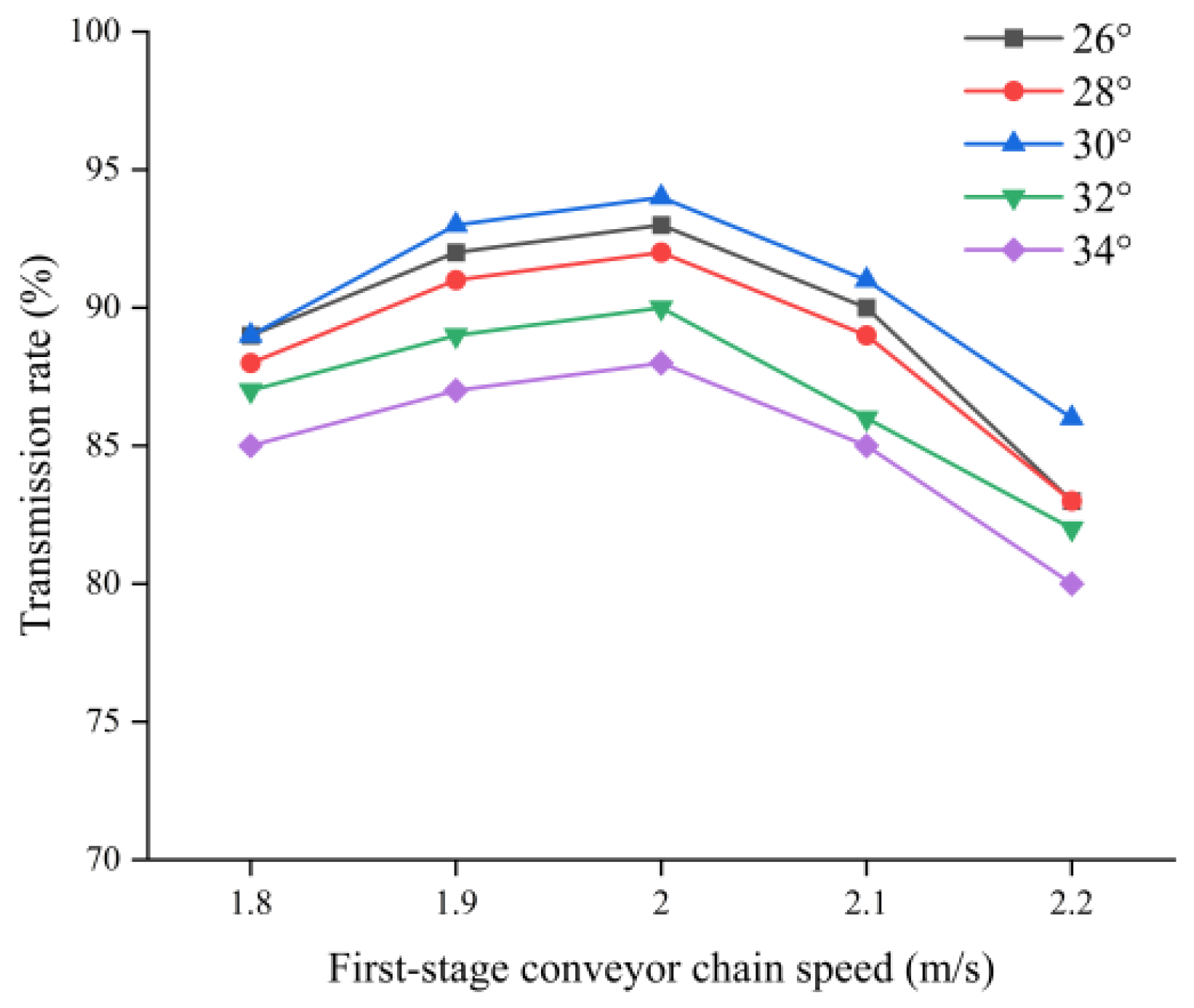
4.4.1. The Effect of First-Stage Conveyor Chain Speed and Unit Operating Angle on the Overall Conveyor Rate

The forward speed of the unit was set to 0.15 m/s, and the machine’s working angle was varied at 26°, 28°, 30°, 32°, and 34°. The speed of the first-stage conveyor chain was simulated within the range of 1.8 to 2.2 m/s for each angle. The resulting data were used to plot the curve of the first-stage conveyor chain speed versus the conveying rate at different working angles, as shown in Figure 28.

As the speed of the first-stage conveyor chain increases, the curve exhibits a pattern of initially rising and then falling. This pattern occurs because excessive screen speed can cause slight vibrations, which are detrimental to licorice conveying. Additionally, if the first-stage conveyor chain angle is too steep, friction between the licorice and the crossbar of the screen is reduced, leading to slippage and a decreased conveying rate. Conversely, if the working angle of the machine is too shallow, the digging depth may fail to meet design requirements, potentially causing damage to the licorice roots and reducing both yield and quality.

The test results indicate that the optimal conveying effect is achieved when the first-stage conveyor chain speed is set to 2 m/s. Both excessively large and small angles of the first-stage conveyor chain are unfavorable for licorice conveying. The most effective conveying rate is observed within the angle range of 30° to 34°.

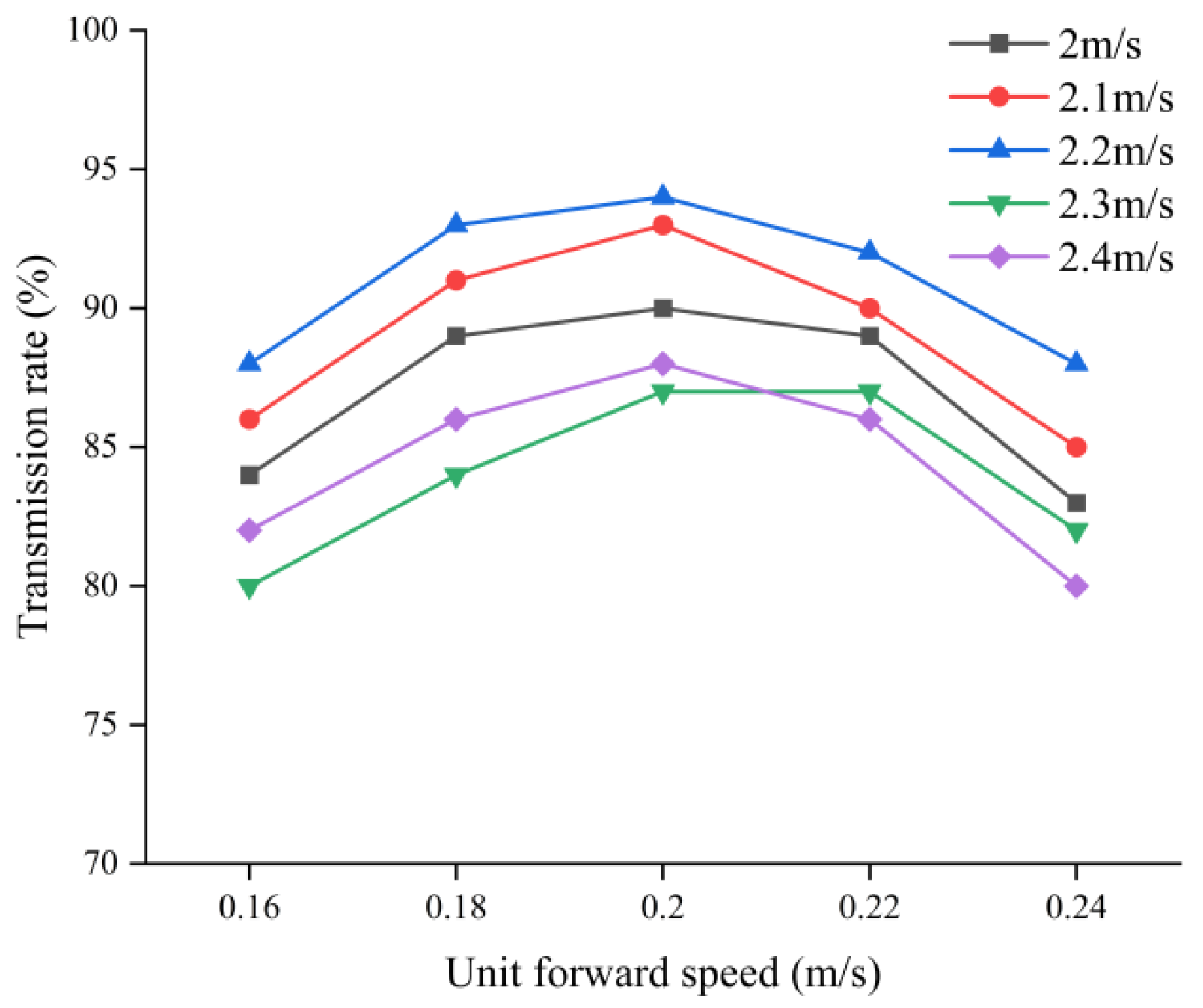
4.4.2. The Effect of Unit’s Forward Speed and Second-Stage Conveyor Chain Speed on the Overall Conveyor Rate

The speed of first-stage conveyor chain was set to 2 m/s. The simulation was carried out in the range of a second-stage conveyor chain speed of 1.8–2.2 m/s, and the forward speed of the unit was in the range of 0.16–0.24 m/s, respectively, so as to obtain the influence law of the forward speed of the unit on the conveying rate in the case of a different rotational speed of the second-stage conveyor chain, as shown in Figure 29.

As the forward speed of the unit increases, the curve first rises and then falls. This trend occurs because, at lower forward speeds, the amount of the drug–soil mixture excavated is limited, resulting in a generally low conveying rate. As the forward speed increases, the volume of the drug–soil mixture that can be handled by the two-stage screen reaches an optimal level, leading to a maximum conveying rate. However, if the forward speed continues to increase, the excavating shovel cannot effectively transfer the soil mixture to the first-stage conveyor chain in time, causing a decrease in conveying efficiency. Additionally, if the soil-crushing rollers cannot crush the large soil particles promptly, it may lead to congestion on the first-stage conveyor chain, which is detrimental to harvester performance.

The test results indicate that the optimal conveying effect of the second-stage conveyor chain is achieved at a speed of 2.2 m/s. Both excessively high and low forward speeds adversely affect licorice conveying, with the best conveying rate observed within the range of 0.18 m/s to 0.22 m/s.

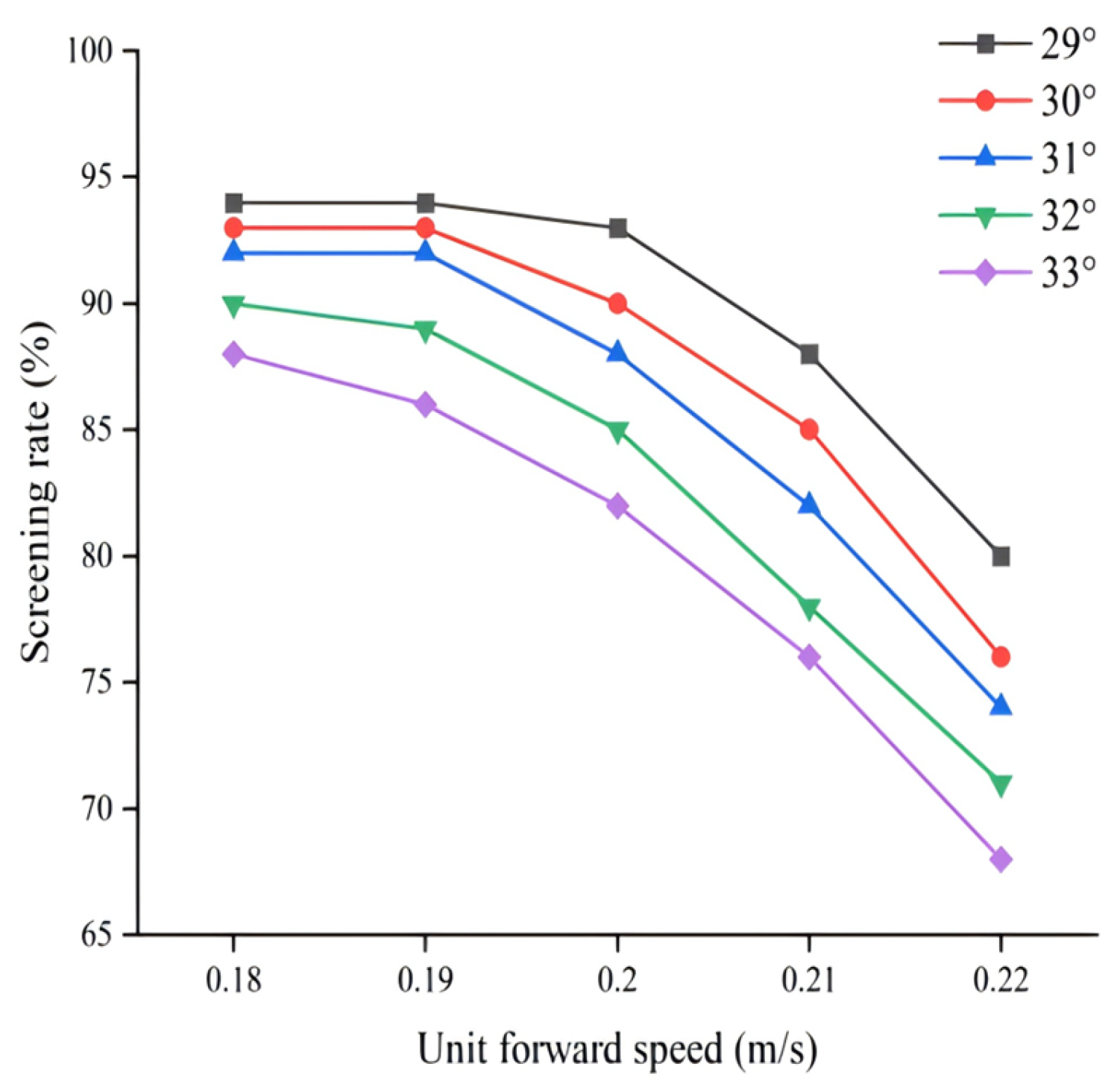
4.4.3. The Effect of the Forward Speed of the Unit and the Speed of the Second-Stage Conveyor Chain on the Overall Screening Rate

The first-stage conveyor chain speed was set to 2 m/s, and the second-stage conveyor chain speed was 2.2 m/s. The simulation test was carried out in the range of 0.18–0.22 m/s for the unit’s forward speed and a machine working angle of 32–35° to obtain the influence law of the unit’s forward speed on the sieve’s clean rate under the condition of different working angles of the unit, as shown in Figure 30.

With the increase in the forward speed of the machine and the tilt angle, the sieving rate exhibited a positive correlation with these two variables, showing a decreasing trend. This occurs because, given a fixed transport separation capacity of the two-stage screen, a smaller forward speed and working angle result in a lower volume of soil mixture being shoveled up by the excavating shovel per unit time. This enhances the separation efficiency of the soil and licorice through the two-stage screen, leading to a higher sieving rate. Conversely, as the forward speed and tilt angle increase, the volume of the drug–soil mixture shoveled up per unit time grows, which increases the workload on the two-stage screen. This reduced effectiveness in separating licorice and soil results in more unseparated material being transported into the collection box with the second-stage conveyor chain, thereby decreasing the sieving rate.

The test results indicate that the optimal separation effect of the sieve occurs when the forward speed of the machine is 0.18 m/s and the working angle is 30°.

4.5. Field Experiment

To verify the conveying effect and working performance of the licorice harvester, a field test was conducted in Longxi County, Dingxi City, Gansu Province. The test field was flat with loose soil, and the main test equipment used was the licorice harvester. The field test setup is illustrated in Figure 31.

The test primarily assessed the field performance of the licorice harvester by measuring the digging net rate, damage rate, and digging depth. A test area measuring 60 m in length and 12 m in width was selected. The harvester made four round trips across the field, with five measurement areas randomly chosen for each trip. Each measurement area was 3 m long and 1.2 m wide. During the test, the actual number of licorice roots, the number of harvested licorice, and the number of damaged licorice were recorded. These measurements follow industry standards [25,26]. The calculations for the digging net rate and damage rate were performed using the following methods:

$$L_1={\displaystyle \frac{Q_1}{Q}}\times 100\%$$

(35)

where L₁ is the digging net rate, %; Q₁ is the mass of rhizomes harvested by the excavator, kg; and Q is the total mass of rhizomes collected by the harvester, kg.

$$L_2={\displaystyle \frac{Q_2}{Q}}\times 100\%$$

(36)

where L₂ is the damage rate, %; and Q₂ is the mass of rhizomes damaged by the excavator during harvesting, kg.

The depth of excavation was calculated by measuring the vertical distance from the bottom of the excavation trench to the surface of the ground at 11 points per stroke after the operation, and the depth of excavation was measured over the width of the work at each point.

$$H={\displaystyle \frac{{\displaystyle \sum _{i=1}^n{H}_i}}{n}}$$

(37)

where H is the average value of excavation depth, mm; n is the number of measurement points, n; and H_i is the excavation depth of the ith point, mm.

The test operational performance, test results of the licorice harvester are shown in

Table 4. Performance test results of licorice harvester.

Determination Index	Technical Index	Measured Mean Value
Excavation rate/%	≥95	96.2
Wound rate/%	≤5	4.3
Digging depth/mm	≥500	580

.

The field tests demonstrated that the licorice harvester met all national and industry standards for key performance indicators, including digging clean rate, injury rate, and digging depth. The harvester effectively separates and conveys licorice, making it suitable for efficient licorice-harvesting operations.

5. Conclusions

(1)

According to the agronomic requirements of licorice harvesting, a licorice harvester was designed, which can complete the operations of digging, conveying, separating and collecting at one time. The harvesting efficiency of the whole machine is 0.26–0.48 hm/s², which is four–five times higher than that of the same amount of manual labor. The whole machine is easy to operate and runs smoothly. Compared with traditional manual digging and harvesting, it can reduce the labor intensity of manual labor, reduce the damage to Chinese herbs, improve production efficiency, and is of great significance to increasing the economic benefits for farmers.

(2)

After analyzing and designing the structure of the excavating shovel and the conveying separating device, the excavating shovel adopted a plane combination shovel. Three sets of roller screens were used to convey the shoveled soil–medicine mixture to the conveying and separating device, and the optimal rotational speed of the roller screens was 150 rpm. The two-stage conveying and separating screens were of the lifting-chain-type conveying and separating structure. Through theoretical analysis, it is concluded that the screen crossbar spacing is 153 mm, the diameter is 10 mm, the spacing of the finger-like rubber chain is 85 mm, and the crushing-roller diameter is 200 mm, which has the best crushing effect on the soil that has not passed through the first stage of the screen.

(3)

The harvesting process of the harvester was simulated using EDEM 2022.3 and RecurDyn 2023. The simulation results demonstrated that the conveying and separating screen operated smoothly throughout the process, effectively handling the medicine–soil mixture. The separation was efficient, with the simulation showing complete separation of soil and licorice. During the simulation, the maximum horizontal displacement of the licorice was 2098 mm, and the maximum vertical displacement was 660 mm. The process caused minimal damage to the licorice rhizome, and the licorice was effectively collected into the collection box.

(4)

The field test shows that after the operation of the licorice harvester, the net digging rate of licorice is 96.2%, the injury rate is 4.3%, the average digging depth is 580 mm, and the machine operates smoothly during the operation period, which meets the harvesting requirements of mechanization of harvesting of root and stem Chinese herbal medicines. The test indicators have reached the national industry standards.