Study on Flow Field Characteristics in Sandstorm Conditions Using Wind Tunnel Test
Abstract
:1. Introduction
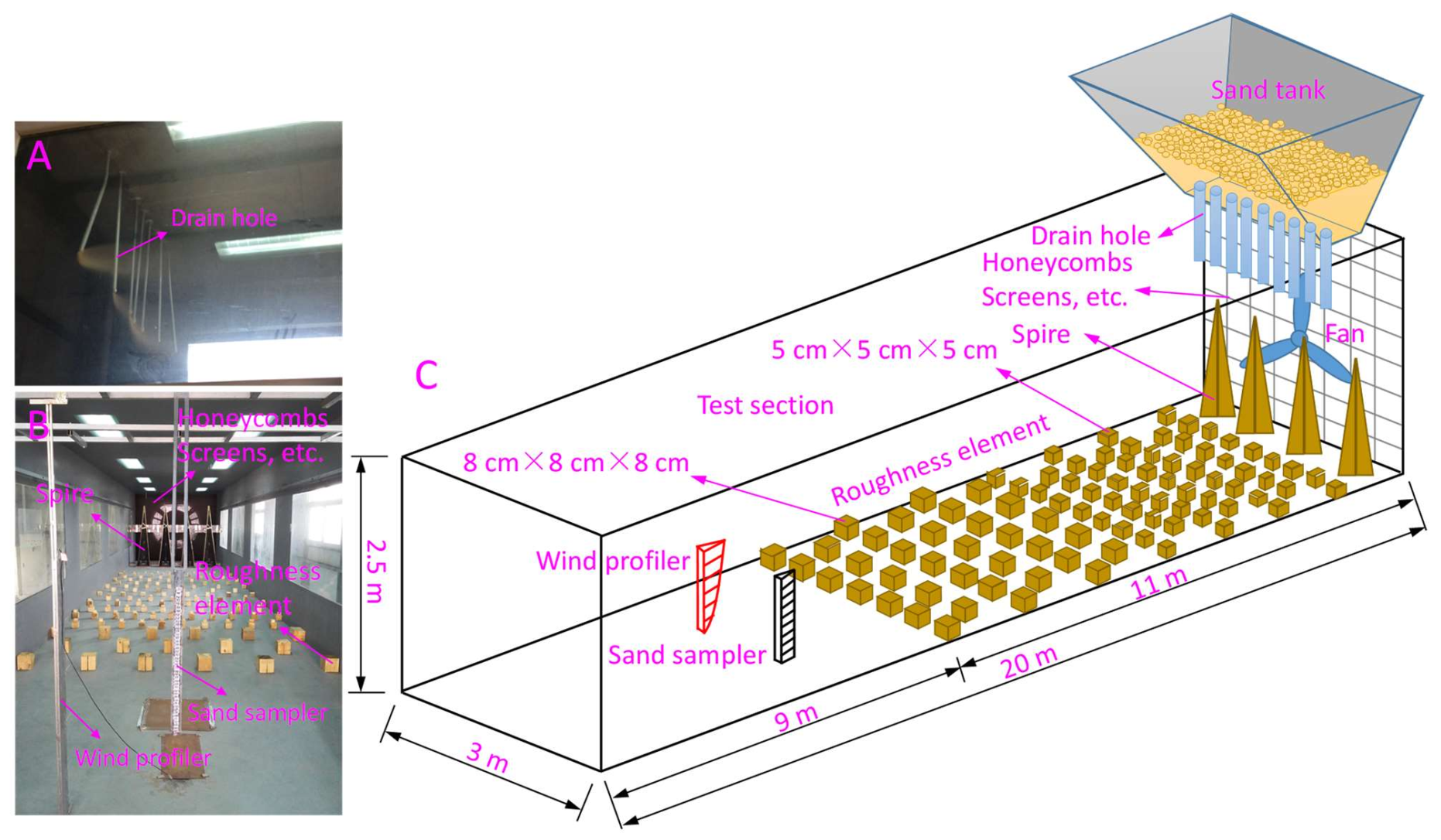
2. Test Methods and Apparatus
3. Test Results and Discussions
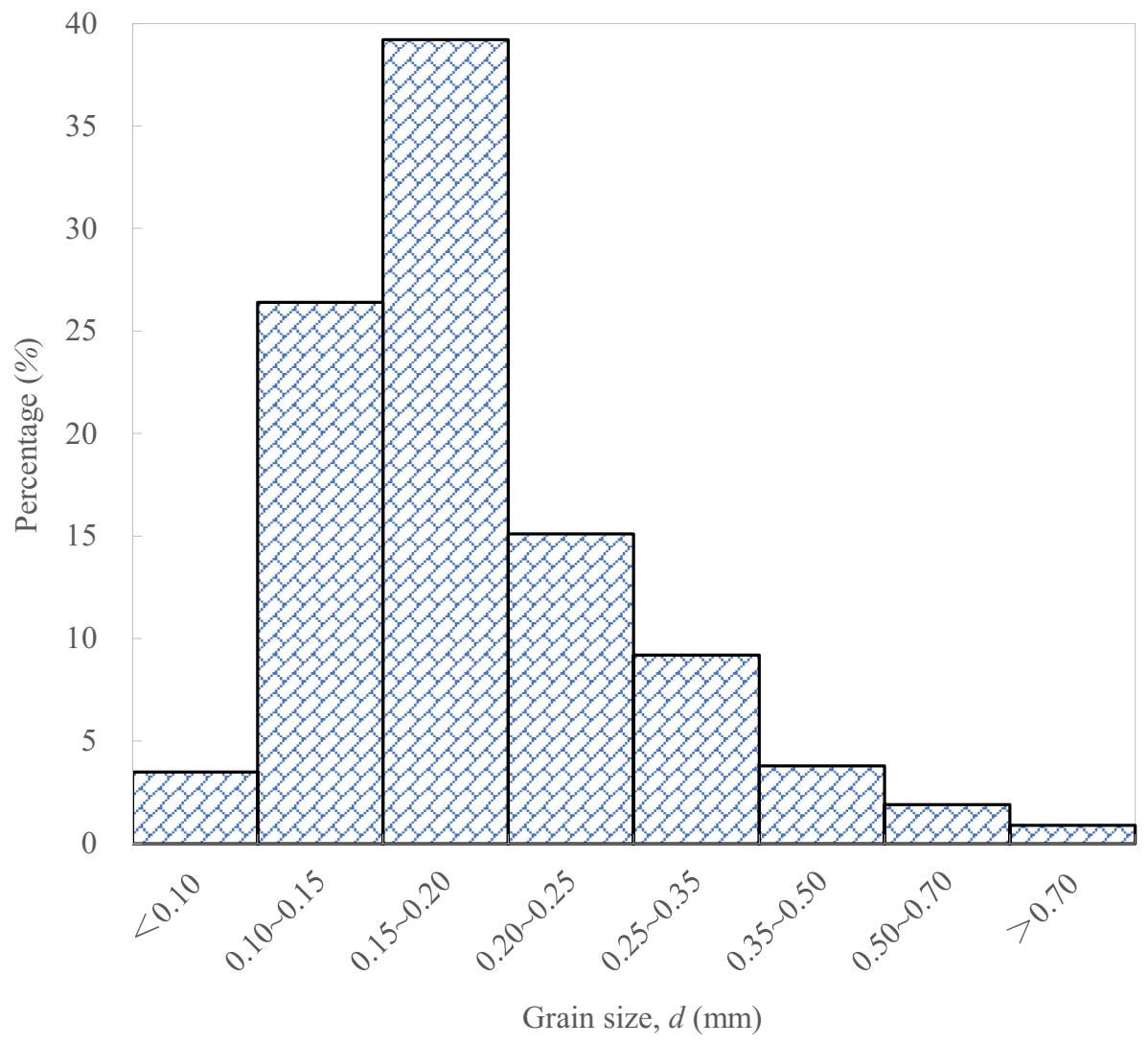
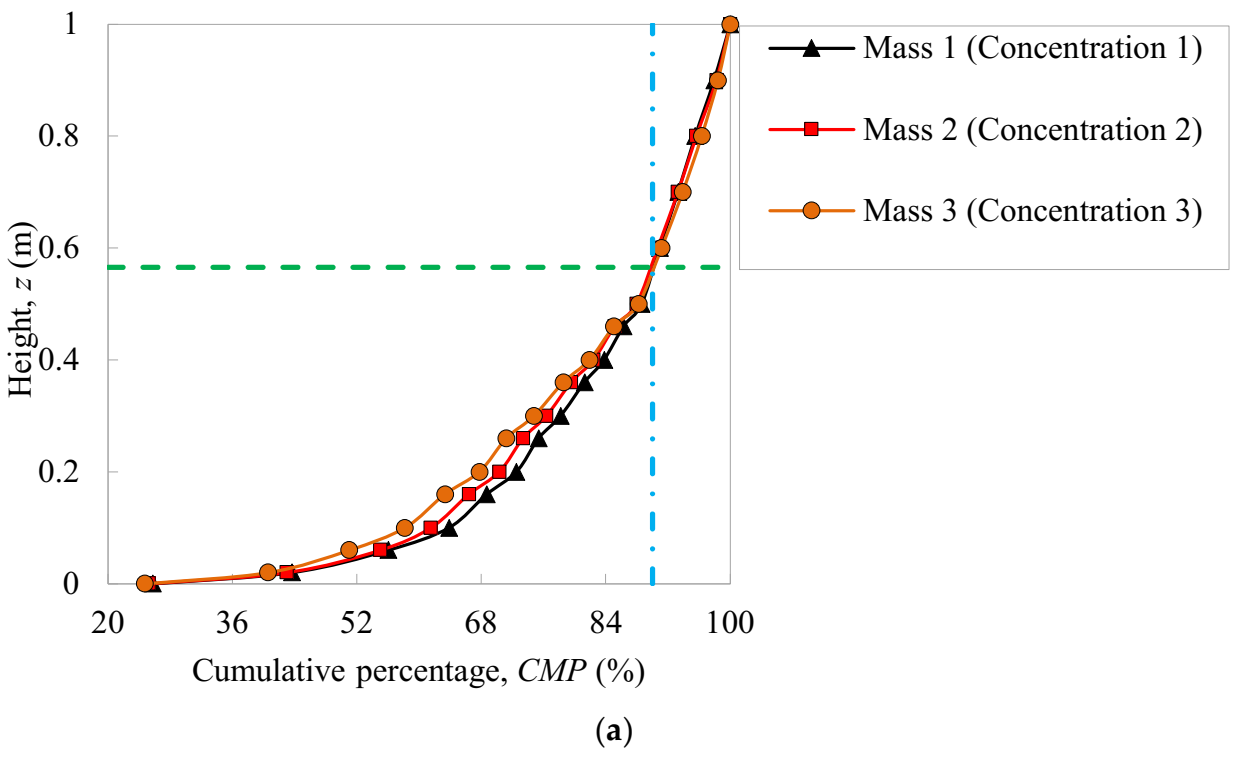
3.1. Cumulative Mass Curve of Sand Grains in the Sandstorm Flow Field
3.2. Distribution Characteristics of Grain Concentration in the Sandstorm Flow Field
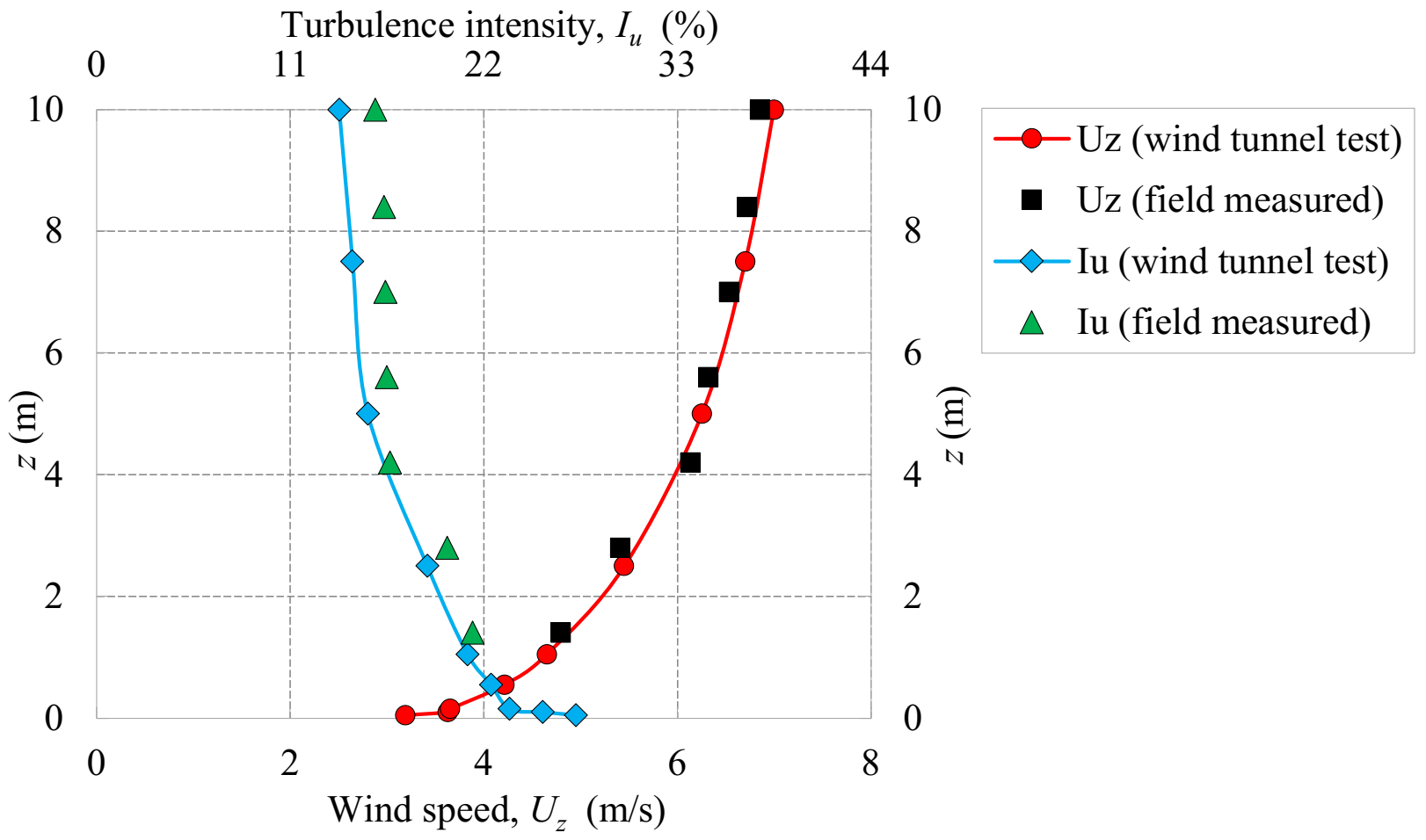
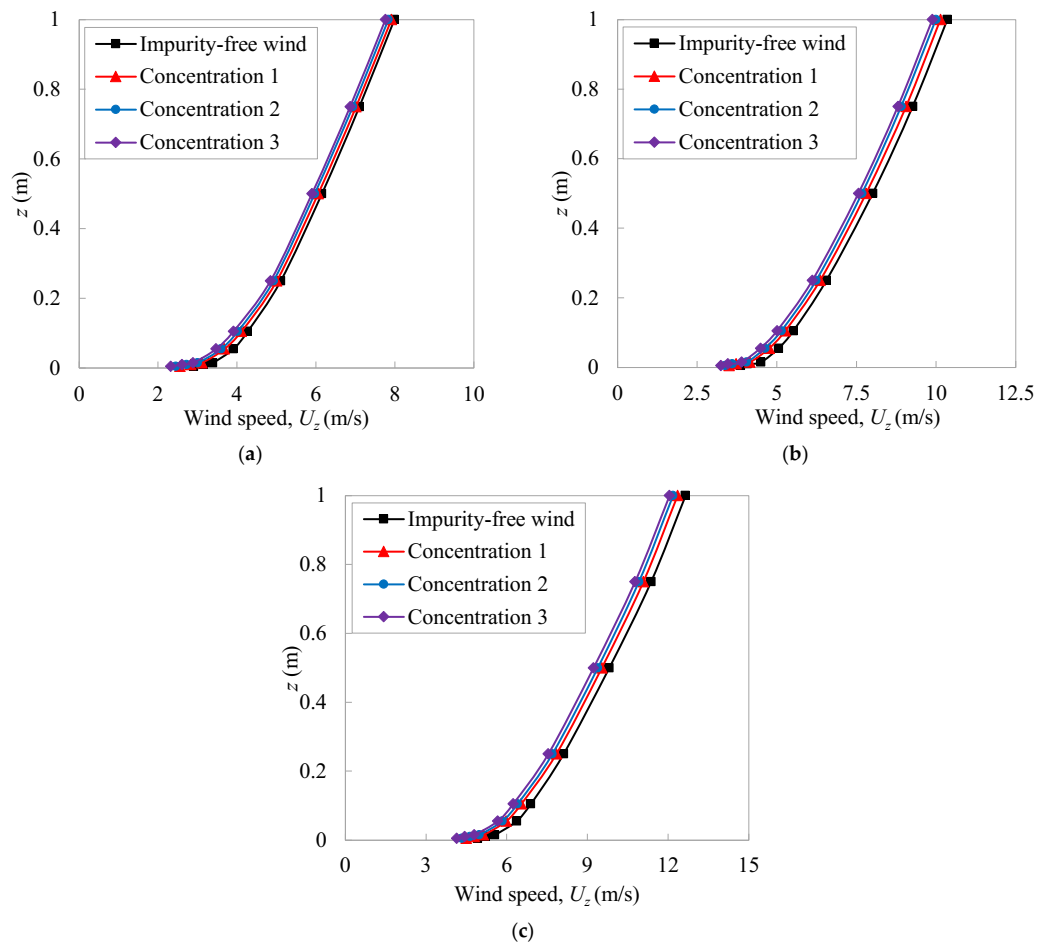
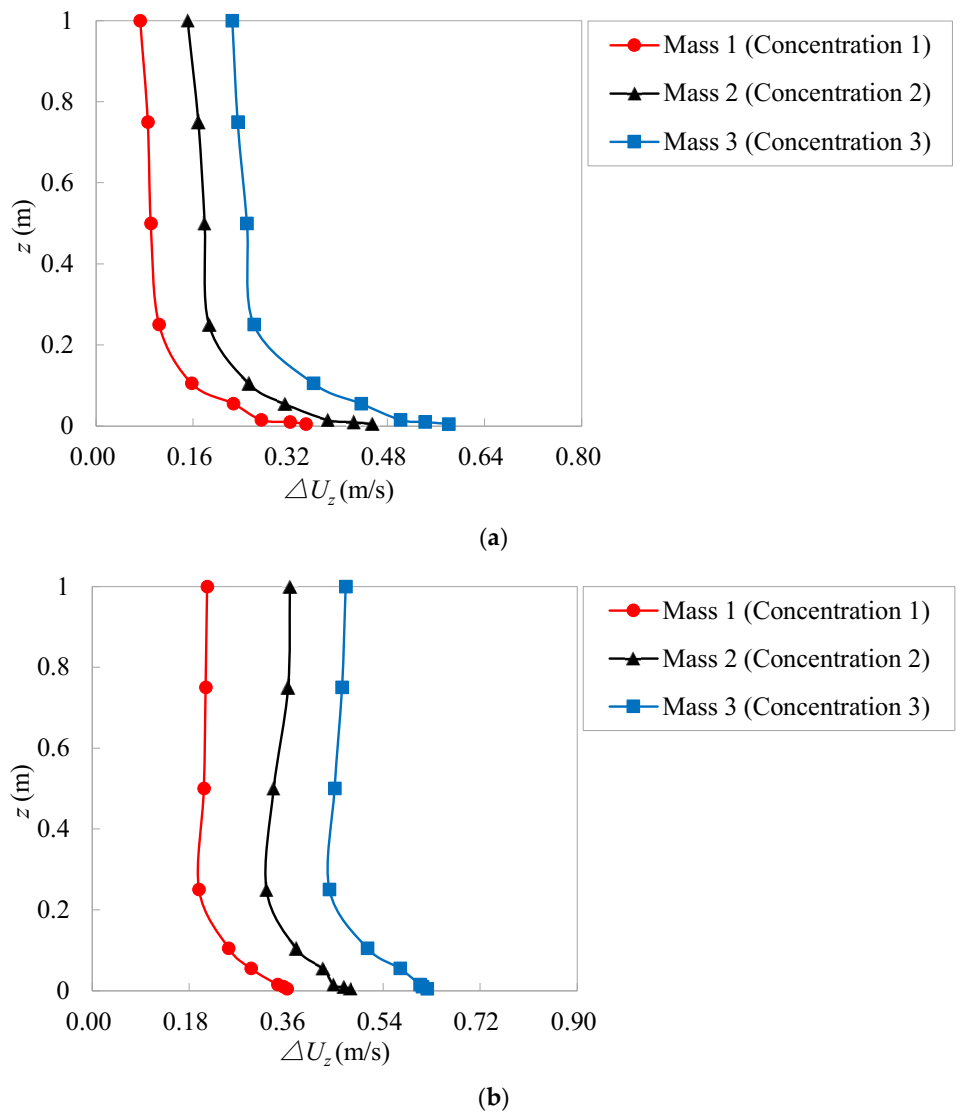
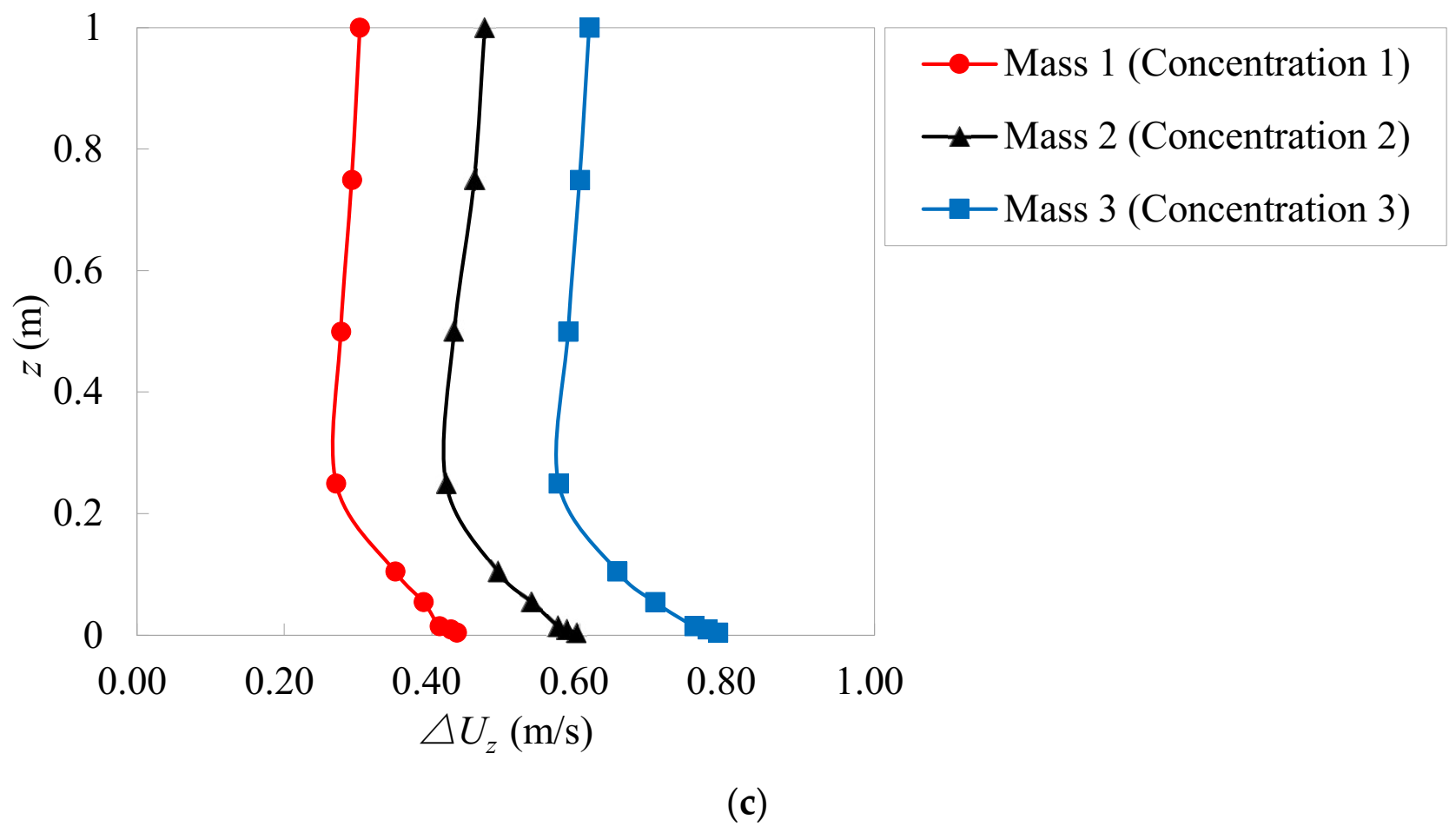
3.3. Wind Speed Profile in the Sandstorm Flow Field
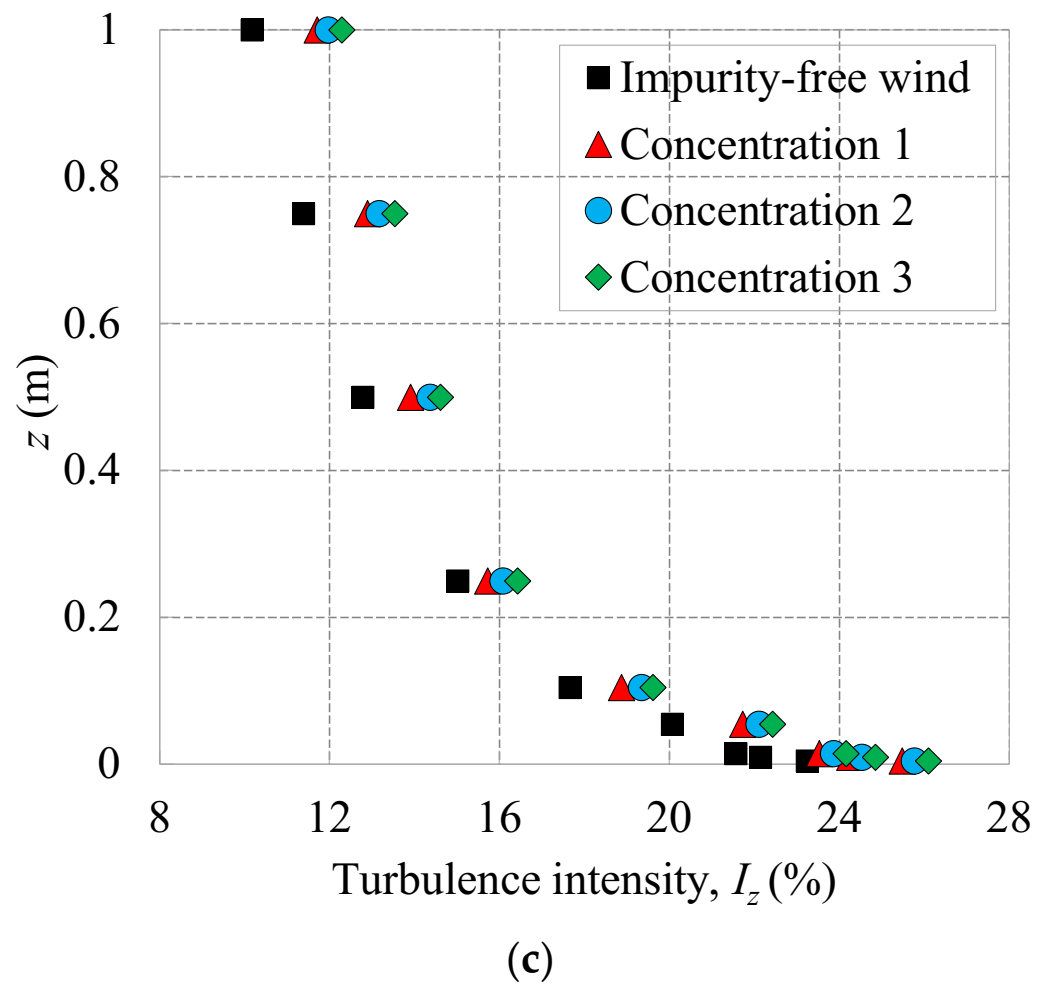
3.4. Turbulence Intensity in the Sandstorm Flow Field
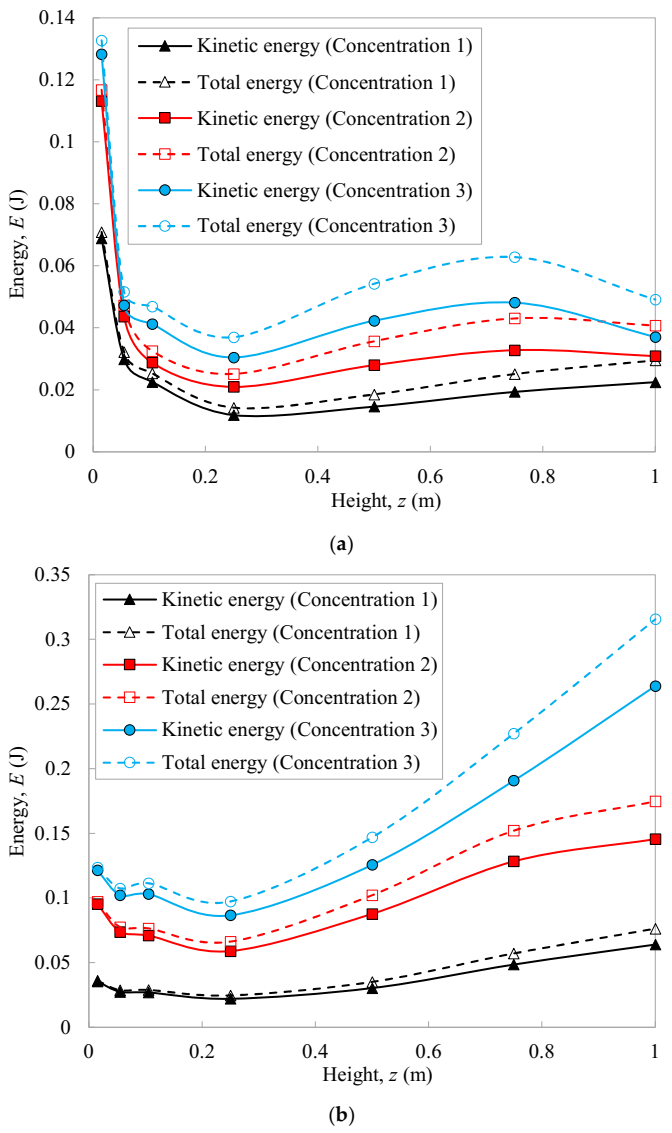
3.5. Energy Distribution of Grains in the Sandstorm Flow Field
4. Concluding Remarks
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| d | grain size |
| z | height |
| CMP | accumulative mass percentage |
| Z90 | the height corresponding to the cumulative mass percentage of 90% |
| Fc | creep percentage |
| ρs | grain concentration |
| Q | the collected sand grain mass |
| A | the inlet area of the sand sampler |
| t | the sand sampling time |
| Uz | the mean wind speed |
| the wind speed impact value | |
| the wind speed impact factor | |
| the airflow speeds in the impurity-free wind flow field | |
| the airflow speeds in the sandstorm flow field | |
| Iz | turbulence intensity |
| turbulence intensity impact value | |
| turbulence intensity impact factor | |
| the turbulence intensities in the impurity-free wind flow field | |
| the turbulence intensities in the sandstorm flow field | |
| Ek | the kinetic energy of sand grains in sandstorm conditions |
| Ep | the potential energy of sand grains in sandstorm conditions |
| Et | the total energy of sand grains in sandstorm conditions |
| the sand grain speed in sandstorm conditions | |
| the airflow speed in sandstorm conditions | |
| g | the gravitational acceleration |
References
- Huang, B.; Li, Z.N.; Cong, S.; Zhou, L.F.; Chen, C.; Hui, Y. State of the progress and prospect of research on wind-sand flow and wind-sand load of buildings. J. Nat. Disasters 2016, 25, 9–19. [Google Scholar]
- Yao, Z.Y.; Xiao, J.H.; Jiang, F.Q. Characteristics of daily extreme-wind gusts along the Lanxin Railway in Xinjiang, China. Aeolian Res. 2012, 6, 31–40. [Google Scholar] [CrossRef]
- Dong, Z.B.; Chen, G.T.; He, X.D.; Han, Z.W.; Wang, X.M. Controlling blown sand along the highway crossing the Taklimakan Desert. J. Arid Environ. 2004, 57, 329–344. [Google Scholar] [CrossRef]
- Cheng, J.J.; Xue, C.X. The sand-damage-prevention engineering system for the railway in the desert region of the Qinghai-Tibet plateau. J. Wind Eng. Ind. Aerodyn. 2014, 125, 30–37. [Google Scholar] [CrossRef]
- Cheng, J.J.; Jiang, F.Q.; Xue, C.X.; Xin, G.W.; Li, K.C.; Yang, Y.H. Characteristics of the disastrous wind-sand environment along railways in the Gobi area of Xinjiang, China. Atmos. Environ. 2015, 102, 344–354. [Google Scholar] [CrossRef]
- Alghamdi, A.A.A.; Al-Kahtani, N.S. Sand control measures and sand drift fences. J. Perform. Constr. Facil. 2005, 19, 295–299. [Google Scholar] [CrossRef]
- Holze, C.; Brucks, A. Accelerated lifetime modeling on the basis of wind tunnel analysis and sand storm aging. Energy Procedia 2014, 49, 1692–1699. [Google Scholar] [CrossRef] [Green Version]
- Bofah, K.K.; Al-Hinai, K.G. Field tests of porous fences in the regime of sand-laden wind. J. Wind Eng. Ind. Aerodyn. 1986, 23, 309–319. [Google Scholar] [CrossRef]
- Zhang, M. Numerical Simulation of Wind-Blown-Sand Two Phase Flow Field around the Building Based on Fluent. Master’s Thesis, Harbin Institute of Technology, Harbin, China, 2008. [Google Scholar]
- Wang, Y.P.; Gong, Z.; Wang, Q.C. Solid-particle erosion of concrete bridge piers and protective material under blown sand environment. Bull. Chin. Ceram. Soc. 2015, 34, 1941–1946. [Google Scholar]
- Mejia, F.; Kleissl, J.; Bosch, J.L. The effect of dust on solar photovoltaic systems. Energy Procedia 2014, 49, 2370–2376. [Google Scholar] [CrossRef] [Green Version]
- Gong, B.; Wang, Z.; Wei, Z. Design wind and sandstorm loads on trough collectors in fields. In Proceedings of the Solarpaces: International Conference on Concentrating Solar Power & Chemical Energy Systems, Santiago, Chile, 26–29 September 2017. [Google Scholar]
- Zhang, J.X.; Zhang, M.J.; Li, Y.L.; Jiang, F.Y.; Wu, L.H.; Guo, D.P. Comparison of wind characteristics in different directions of deep-cut gorges based on field measurements. J. Wind Eng. Ind. Aerodyn. 2021, 212, 104595. [Google Scholar] [CrossRef]
- He, J.Y.; He, Y.G.; Li, Q.S.; Chan, P.W.; Zhang, L.; Yang, H.L.; Li, L. Observational study of wind characteristics, wind speed and turbulence profiles during Super Typhoon Mangkhut. J. Wind Eng. Ind. Aerodyn. 2020, 206, 104362. [Google Scholar] [CrossRef]
- Wang, M.G.; Cao, S.Y.; Cao, J.X. Tornado-like-vortex-induced wind pressure on a low-rise building with opening in roof corner. J. Wind Eng. Ind. Aerodyn. 2020, 205, 104308. [Google Scholar] [CrossRef]
- Liu, J.Y.; Hui, Y.; Yang, Q.S.; Tamura, Y. Flow field investigation for aerodynamic effects of surface mounted ribs on square-sectioned high-rise buildings. J. Wind Eng. Ind. Aerodyn. 2021, 211, 104551. [Google Scholar] [CrossRef]
- Liu, J.Y.; Hui, Y.; Wang, J.X.; Yang, Q.S. LES study of windward-face-mounted-ribs’ effects on flow fields and aerodynamic forces on a square cylinder. Build. Environ. 2021, 200, 107950. [Google Scholar] [CrossRef]
- Jiang, C.W.; Dong, Z.B.; Wang, X.Y. Analysis of the mass flux profiles of an aeolian saltating cloud: Wind tunnel measurements by high-speed photography. J. Desert Res. 2016, 36, 1230–1237. [Google Scholar]
- Lancaster, N. Geomorpholgy of Desert Dunes; Routledge: London, UK, 1995; pp. 15–38. [Google Scholar]
- Liu, X.W. Experimental Wind-Sand Flow and Sand Drift Control Engineering; Science Press: Beijing, China, 1995; pp. 1–210. [Google Scholar]
- Livingstone, I.; Warren, A. Aeolian Geomorphology: An Introduction; Addison Wesley Longman: Upper Saddle River, NJ, USA, 1996; pp. 1–25. [Google Scholar]
- Pye, K.; Tsoar, H. Aeolian Sand and Sand Deposits; Unwin Hyman: London, UK, 1990; pp. 3–59. [Google Scholar]
- Shao, Y.; Leslie, L.M. Wind erosion prediction over the Australian continent. J. Geophys. Res. Atmos. 1997, 102, 30091–30105. [Google Scholar] [CrossRef] [Green Version]
- Zobeck, T.M.; Scott, V.P.R. Wind induced dust generation and transport mechanics on a bare agriculture field. J. Hazard. Mater. 2006, 132, 26–38. [Google Scholar] [CrossRef]
- Shi, F.; Huang, N. Measurement and simulation of sand saltation movement under fluctuating wind in a natural field environment. Phys. A Stat. Mech. Its Appl. 2012, 391, 474–484. [Google Scholar] [CrossRef]
- Zhang, Z.C.; Dong, Z.B.; Zhao, A.G. The characteristics of aeolian sediment flux profiles in the south-eastern Tengger Desert. Sedimentology 2011, 58, 1884–1894. [Google Scholar] [CrossRef]
- Liu, X.P.; Dong, Z.B.; Wang, X.M. Wind tunnel modeling and measurements of the flux of wind-blown sand. J. Arid Environ. 2006, 66, 657–672. [Google Scholar] [CrossRef]
- Dong, Z.B.; Liu, X.P.; Wang, H.T.; Zhao, A.G.; Wang, X.M. The flux profile of a blowing sand cloud: A wind tunnel investigation. Geomorphology 2002, 49, 219–230. [Google Scholar] [CrossRef]
- Dong, Z.B.; Wang, H.T.; Liu, X.P.; Wang, X.M. The blown sand flux over a sandy surface: A wind tunnel investigation on the fetch effect. Geomorphology 2004, 57, 117–127. [Google Scholar] [CrossRef]
- Zou, X.Y.; Wang, Z.L.; Hao, Q.Z.; Zhang, C.L.; Liu, Y.Z.; Dong, G.R. The distribution of velocity and energy of saltating sand grains in a wind tunnel. Geomorphology 2001, 36, 155–165. [Google Scholar] [CrossRef]
- Ma, G.S.; Zheng, X.J. The fluctuation property of blown sand particles and the wind-sand flow evolution studied by numerical method. Eur. Phys. J. E 2011, 34, 54. [Google Scholar] [CrossRef]
- Raffaele, L.; Bruno, L.; Fransos, D.; Pellerey, F. Incoming windblown sand drift to civil infrastructures: A probabilistic evaluation. J. Wind Eng. Ind. Aerodyn. 2017, 166, 37–47. [Google Scholar] [CrossRef] [Green Version]
- Huang, B.; Li, Z.N.; Zhao, Z.F.; Wu, H.H.; Zhou, H.F.; Cong, S. Near-ground impurity-free wind and wind-driven sand of photovoltaic power stations in a desert area. J. Wind Eng. Ind. Aerodyn. 2018, 179, 483–502. [Google Scholar] [CrossRef]
- Zhang, Z.C.; Dong, Z.B.; Wu, G.X. Field observations of sand transport over the crest of a transverse dune in northwestern China Tengger Desert. Soil Tillage Res. 2017, 166, 67–75. [Google Scholar] [CrossRef]
- Huang, B.; Li, Z.N.; Zhang, Z.T.; Zhao, Z.F.; Gong, B. Wind Tunnel Test on Windblown Sand Two-Phase Flow Characteristics in Arid Desert Regions. Appl. Sci. 2021, 11, 11349. [Google Scholar] [CrossRef]
- Bagnold, R.A. The Physics of Blown Sand and Desert Dunes; Methuen: London, UK, 1941; pp. 18–60. [Google Scholar]
- Jiang, F.Q.; Li, Y.; Li, K.C.; Cheng, J.J.; Xue, C.X.; Ge, S.C. Study on structural characteristics of Gobi wind sand flow in 100 km wind area along Lan-Xin Railway. J. China Railw. Soc. 2010, 32, 105–110. [Google Scholar]
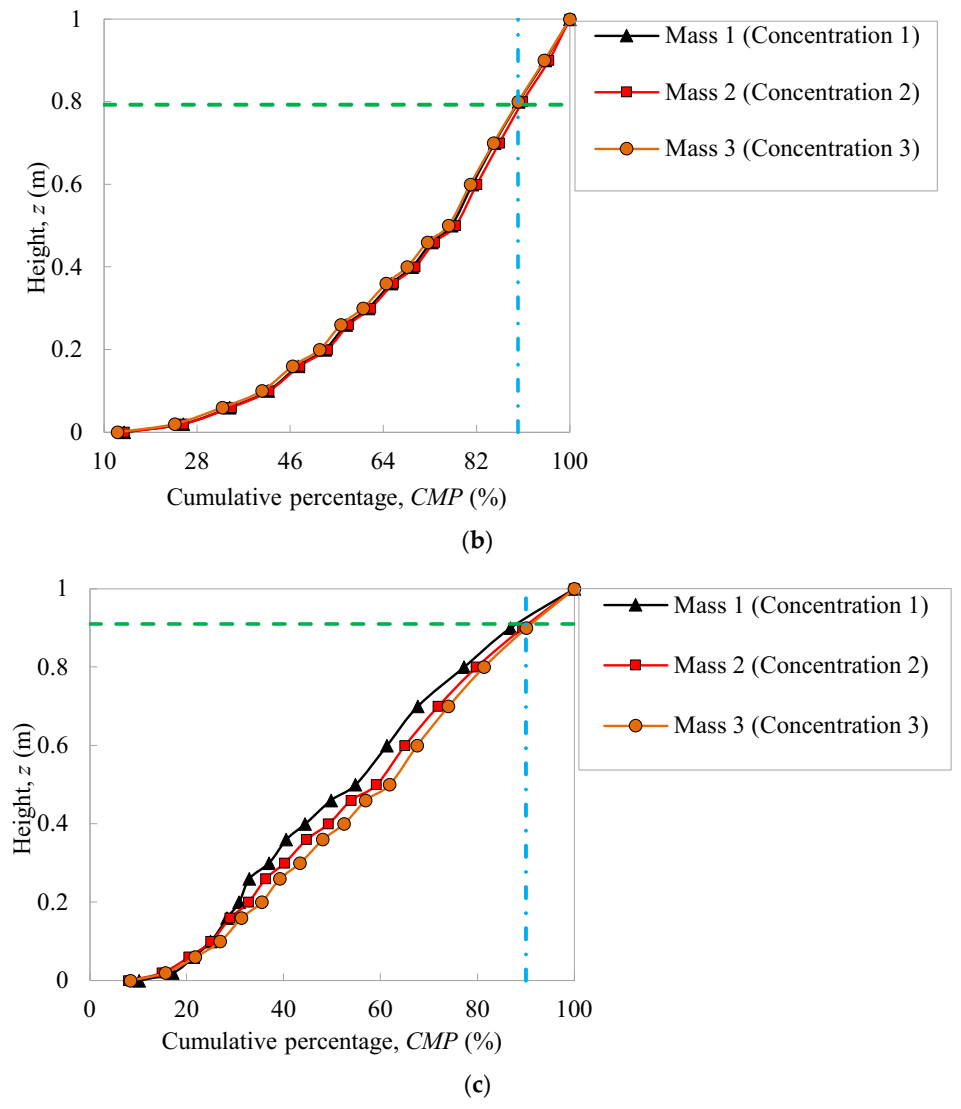
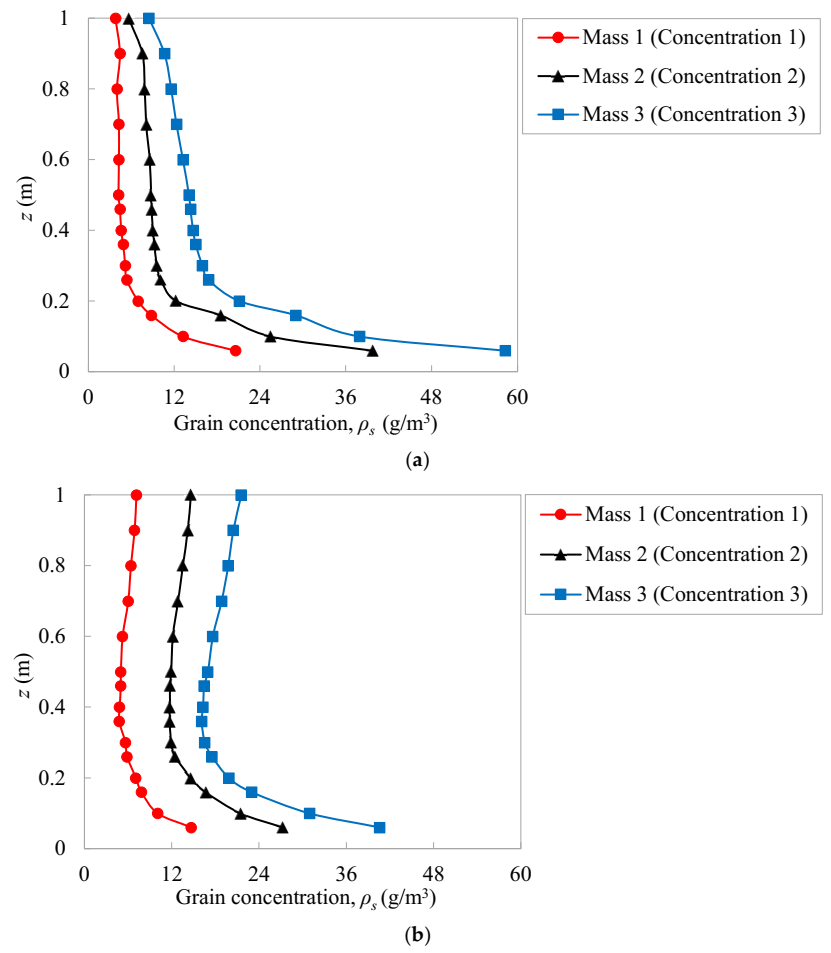

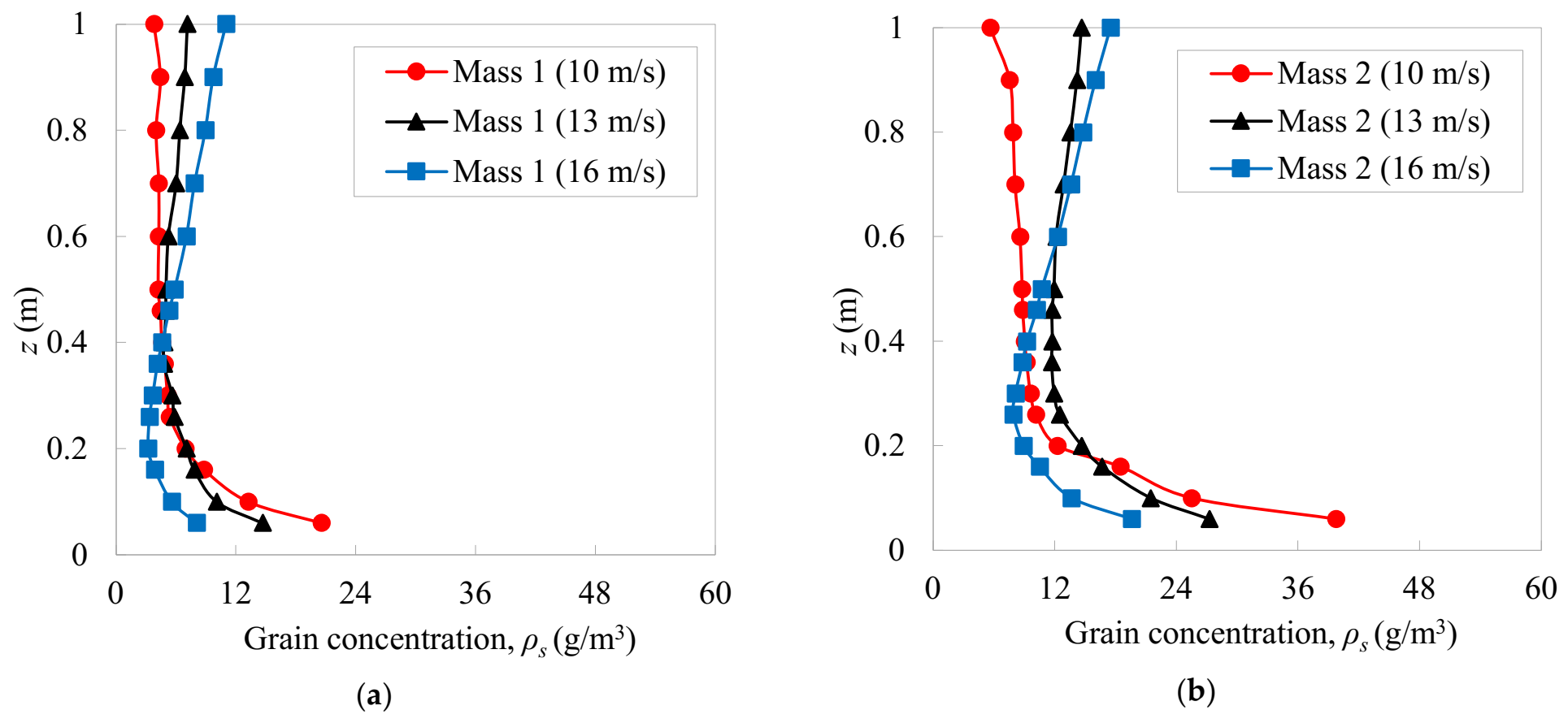
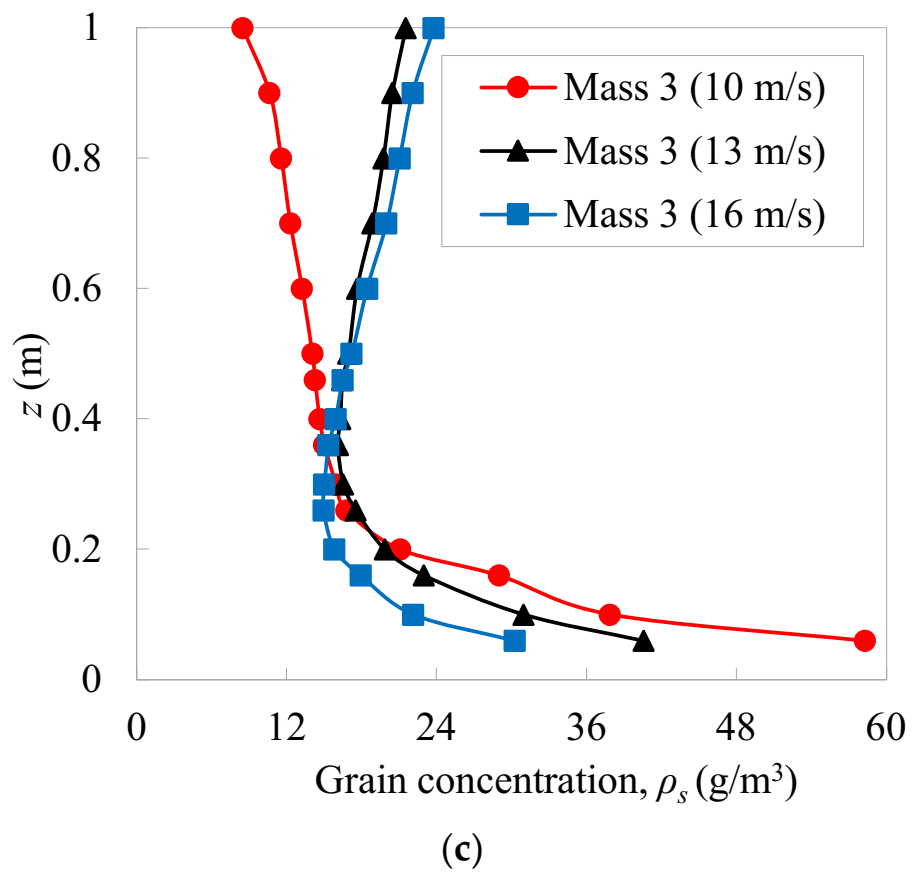

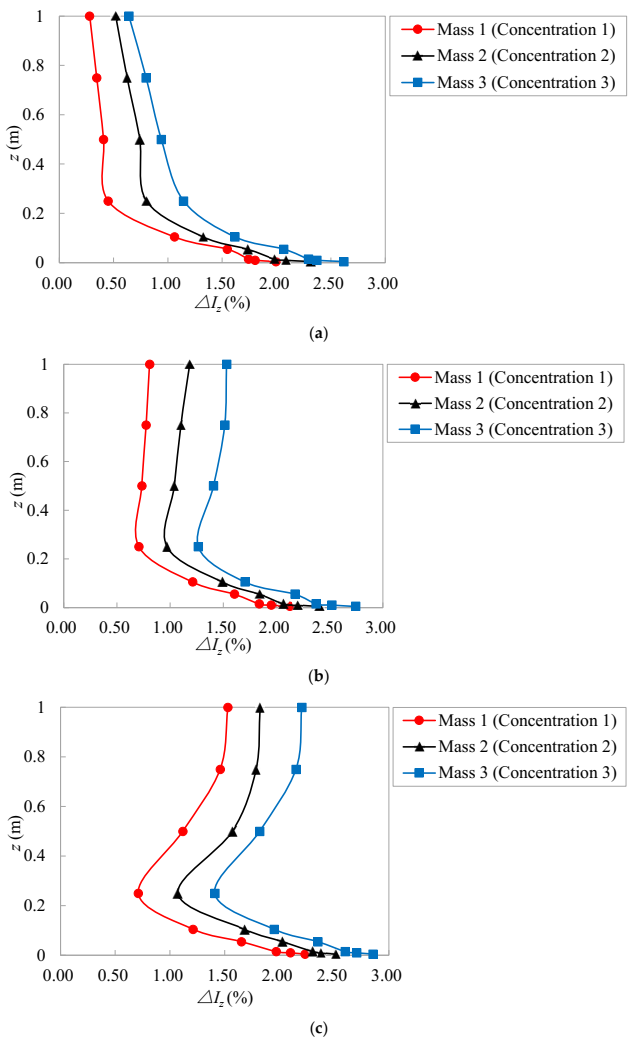

| Wind Speed (m/s) | Grain Concentration | 1 Z90 (cm) | Mean Z90 (cm) | 2 Fc (%) | Mean Fc (%) |
|---|---|---|---|---|---|
| 10 | Concentration 1 (0.5 S) | 56.0 | 56.6 | 25.7 | 25.2 |
| Concentration 2 (1.0 S) | 57.6 | 25.2 | |||
| Concentration 3 (1.5 S) | 56.1 | 24.7 | |||
| 13 | Concentration 1 (0.5 S) | 79.8 | 79.3 | 13.9 | 13.5 |
| Concentration 2 (1.0 S) | 78.1 | 13.9 | |||
| Concentration 3 (1.5 S) | 79.9 | 12.6 | |||
| 16 | Concentration 1 (0.5 S) | 92.4 | 91.0 | 10.2 | 8.9 |
| Concentration 2 (1.0 S) | 90.6 | 8.0 | |||
| Concentration 3 (1.5 S) | 89.9 | 8.4 |
| Wind Speed (m/s) | Height (cm) | Mass 1 (Concentration 1) | Mass 2 (Concentration 2) | Mass 3 (Concentration 3) | |||
|---|---|---|---|---|---|---|---|
| (m/s) | (m/s) | (m/s) | |||||
| 10 | 0.5 | 0.35 | 0.88 | 0.46 | 0.84 | 0.58 | 0.80 |
| 1 | 0.32 | 0.90 | 0.42 | 0.87 | 0.54 | 0.83 | |
| 1.5 | 0.27 | 0.92 | 0.38 | 0.89 | 0.50 | 0.85 | |
| 5.5 | 0.23 | 0.94 | 0.31 | 0.92 | 0.44 | 0.89 | |
| 10.5 | 0.16 | 0.96 | 0.25 | 0.94 | 0.36 | 0.92 | |
| 25 | 0.10 | 0.98 | 0.19 | 0.96 | 0.26 | 0.95 | |
| 50 | 0.09 | 0.98 | 0.18 | 0.97 | 0.25 | 0.96 | |
| 75 | 0.08 | 0.99 | 0.17 | 0.98 | 0.23 | 0.97 | |
| 100 | 0.07 | 0.99 | 0.15 | 0.98 | 0.22 | 0.97 | |
| 13 | 0.5 | 0.36 | 0.91 | 0.48 | 0.88 | 0.62 | 0.84 |
| 1 | 0.36 | 0.91 | 0.47 | 0.89 | 0.61 | 0.85 | |
| 1.5 | 0.34 | 0.92 | 0.45 | 0.90 | 0.61 | 0.87 | |
| 5.5 | 0.30 | 0.94 | 0.43 | 0.91 | 0.57 | 0.89 | |
| 10.5 | 0.25 | 0.95 | 0.38 | 0.93 | 0.51 | 0.91 | |
| 25 | 0.20 | 0.97 | 0.32 | 0.95 | 0.44 | 0.93 | |
| 50 | 0.21 | 0.97 | 0.34 | 0.96 | 0.45 | 0.94 | |
| 75 | 0.21 | 0.98 | 0.36 | 0.96 | 0.46 | 0.95 | |
| 100 | 0.22 | 0.98 | 0.36 | 0.97 | 0.47 | 0.96 | |
| 16 | 0.5 | 0.43 | 0.91 | 0.60 | 0.88 | 0.79 | 0.84 |
| 1 | 0.42 | 0.92 | 0.58 | 0.89 | 0.77 | 0.85 | |
| 1.5 | 0.41 | 0.93 | 0.57 | 0.90 | 0.76 | 0.86 | |
| 5.5 | 0.39 | 0.94 | 0.54 | 0.92 | 0.70 | 0.89 | |
| 10.5 | 0.35 | 0.95 | 0.49 | 0.93 | 0.65 | 0.91 | |
| 25 | 0.27 | 0.97 | 0.42 | 0.95 | 0.57 | 0.93 | |
| 50 | 0.28 | 0.97 | 0.43 | 0.96 | 0.59 | 0.94 | |
| 75 | 0.29 | 0.97 | 0.45 | 0.96 | 0.60 | 0.95 | |
| 100 | 0.29 | 0.98 | 0.46 | 0.97 | 0.59 | 0.95 | |
| Wind Speed (m/s) | Height (cm) | Mass 1 (Concentration 1) | Mass 2 (Concentration 2) | Mass 3 (Concentration 3) | |||
|---|---|---|---|---|---|---|---|
| (%) | (%) | (%) | |||||
| 10 | 0.5 | 1.99 | 1.08 | 2.31 | 1.10 | 2.62 | 1.11 |
| 1 | 1.80 | 1.08 | 2.09 | 1.10 | 2.37 | 1.11 | |
| 1.5 | 1.74 | 1.08 | 1.98 | 1.09 | 2.29 | 1.11 | |
| 5.5 | 1.55 | 1.08 | 1.73 | 1.09 | 2.07 | 1.10 | |
| 10.5 | 1.06 | 1.06 | 1.32 | 1.08 | 1.62 | 1.09 | |
| 25 | 0.45 | 1.03 | 0.80 | 1.05 | 1.14 | 1.07 | |
| 50 | 0.41 | 1.03 | 0.74 | 1.05 | 0.94 | 1.06 | |
| 75 | 0.35 | 1.03 | 0.62 | 1.05 | 0.80 | 1.06 | |
| 100 | 0.28 | 1.02 | 0.52 | 1.05 | 0.64 | 1.06 | |
| 13 | 0.5 | 2.13 | 1.09 | 2.41 | 1.10 | 2.74 | 1.12 |
| 1 | 1.95 | 1.09 | 2.20 | 1.10 | 2.52 | 1.12 | |
| 1.5 | 1.84 | 1.09 | 2.06 | 1.10 | 2.37 | 1.11 | |
| 5.5 | 1.60 | 1.08 | 1.84 | 1.09 | 2.17 | 1.11 | |
| 10.5 | 1.21 | 1.07 | 1.49 | 1.09 | 1.70 | 1.10 | |
| 25 | 0.71 | 1.05 | 0.97 | 1.07 | 1.26 | 1.09 | |
| 50 | 0.73 | 1.06 | 1.04 | 1.08 | 1.41 | 1.11 | |
| 75 | 0.77 | 1.07 | 1.10 | 1.09 | 1.51 | 1.13 | |
| 100 | 0.81 | 1.08 | 1.08 | 1.11 | 1.53 | 1.15 | |
| 16 | 0.5 | 2.23 | 1.10 | 2.52 | 1.11 | 2.85 | 1.12 |
| 1 | 2.10 | 1.10 | 2.38 | 1.11 | 2.71 | 1.12 | |
| 1.5 | 1.97 | 1.09 | 2.30 | 1.11 | 2.60 | 1.12 | |
| 5.5 | 1.65 | 1.08 | 2.03 | 1.10 | 2.35 | 1.12 | |
| 10.5 | 1.21 | 1.07 | 1.68 | 1.09 | 1.95 | 1.11 | |
| 25 | 0.71 | 1.05 | 1.07 | 1.07 | 1.41 | 1.09 | |
| 50 | 1.12 | 1.09 | 1.57 | 1.12 | 1.82 | 1.14 | |
| 75 | 1.51 | 1.13 | 1.79 | 1.16 | 2.15 | 1.19 | |
| 100 | 1.53 | 1.15 | 1.79 | 1.18 | 2.10 | 1.21 | |
| Wind Speed (m/s) | Grain Concentration | Mean Ek (10−3 J) | Mean Ep (10−3 J) | Mean Et (10−3 J) |
|---|---|---|---|---|
| 10 | Concentration 1 (0.5 S) | 27 | 4 | 31 |
| Concentration 2 (1.0 S) | 43 | 6 | 49 | |
| Concentration 3 (1.5 S) | 54 | 8 | 62 | |
| 13 | Concentration 1 (0.5 S) | 36 | 5 | 41 |
| Concentration 2 (1.0 S) | 94 | 12 | 106 | |
| Concentration 3 (1.5 S) | 142 | 19 | 161 | |
| 16 | Concentration 1 (0.5 S) | 109 | 12 | 121 |
| Concentration 2 (1.0 S) | 167 | 18 | 185 | |
| Concentration 3 (1.5 S) | 252 | 26 | 278 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Huang, B.; Li, Z.; Zhang, Z.; Zhao, Z.; Gong, B.; Xiao, T. Study on Flow Field Characteristics in Sandstorm Conditions Using Wind Tunnel Test. Atmosphere 2022, 13, 446. https://doi.org/10.3390/atmos13030446
Huang B, Li Z, Zhang Z, Zhao Z, Gong B, Xiao T. Study on Flow Field Characteristics in Sandstorm Conditions Using Wind Tunnel Test. Atmosphere. 2022; 13(3):446. https://doi.org/10.3390/atmos13030446
Chicago/Turabian StyleHuang, Bin, Zhengnong Li, Zhitian Zhang, Zhefei Zhao, Bo Gong, and Tianyin Xiao. 2022. "Study on Flow Field Characteristics in Sandstorm Conditions Using Wind Tunnel Test" Atmosphere 13, no. 3: 446. https://doi.org/10.3390/atmos13030446
APA StyleHuang, B., Li, Z., Zhang, Z., Zhao, Z., Gong, B., & Xiao, T. (2022). Study on Flow Field Characteristics in Sandstorm Conditions Using Wind Tunnel Test. Atmosphere, 13(3), 446. https://doi.org/10.3390/atmos13030446








