Extreme Value Analysis and Modelling of Wet Snow Accretion on Overhead Lines in Hungary
Abstract
:1. Introduction
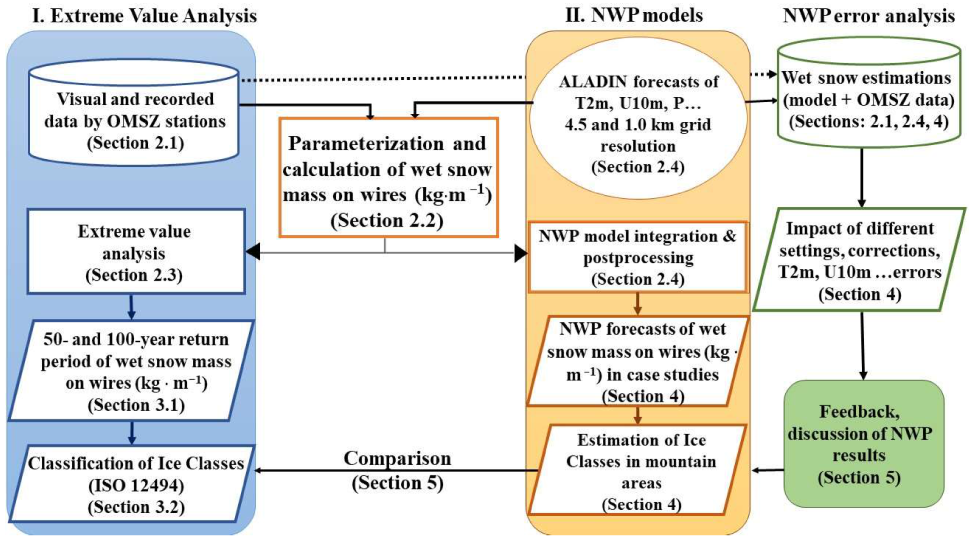
2. Materials and Methods
2.1. Input Data for Calculating the 50- and 100-Year Return Periods of Wet Snow Mass
- The temperature at 2 m was between −0.5 and +2 °C;
- Snowfall was recorded at the time of observation (synoptic code: 70, 71, 72, 73, 74, 75);
- End of the event: if the temperature rose above +2 °C and/or precipitation ceased and no precipitation was registered for 6 h thereafter.
2.2. Parameterization of Wet Snow Accretion
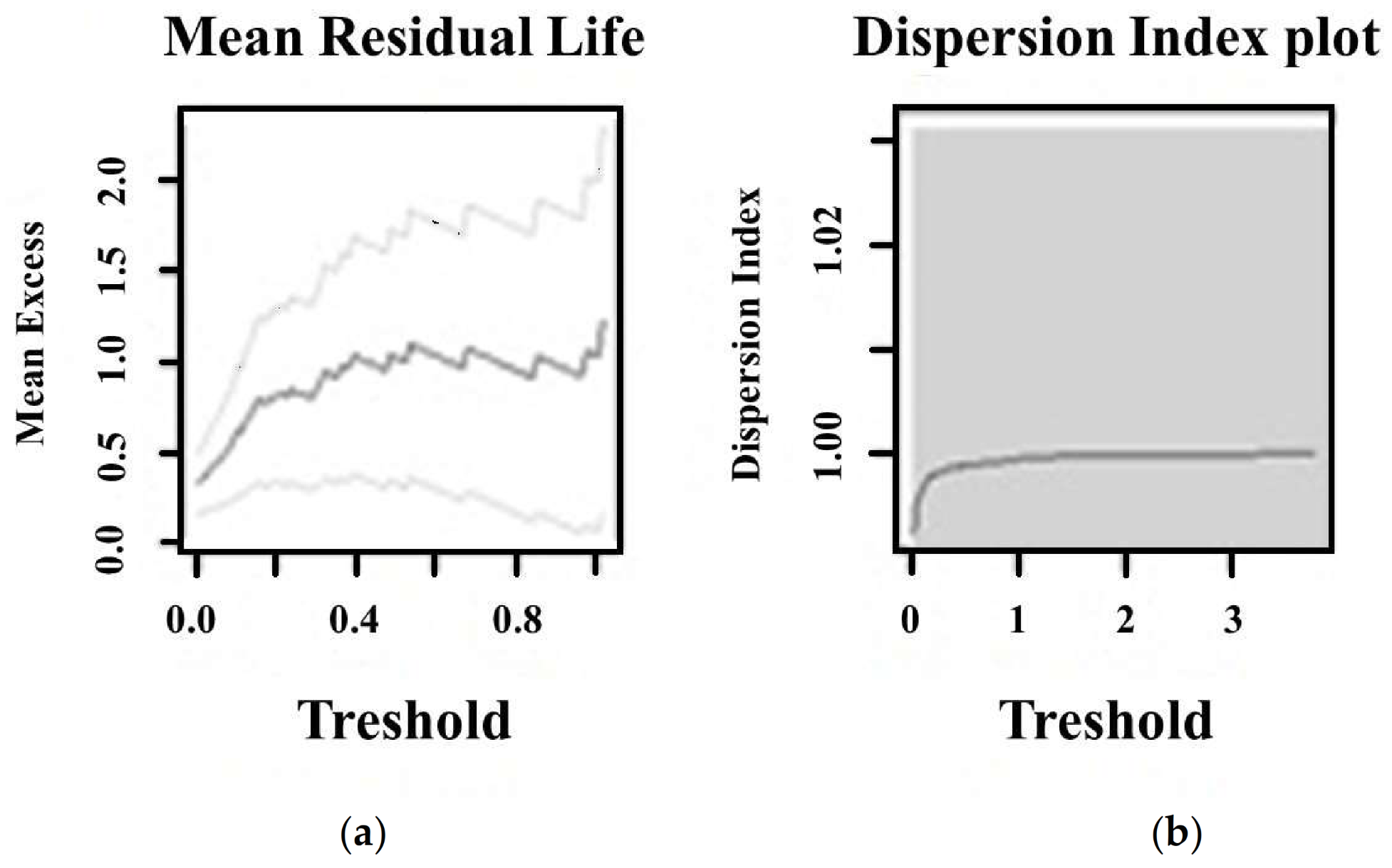
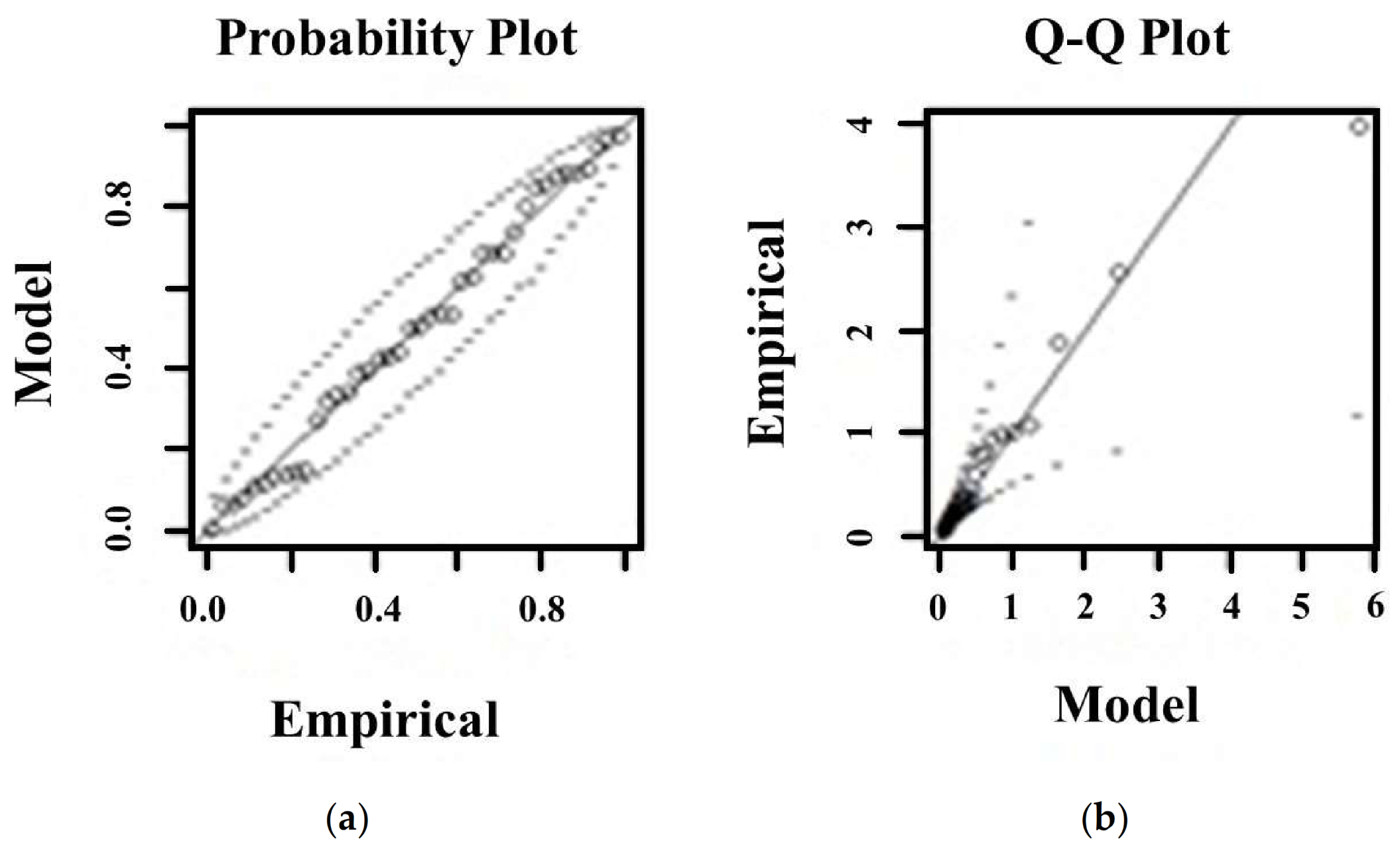
2.3. Extreme Value Analysis with the Peaks over Threshold Method
2.4. Description of the Numerical Weather Prediction Models and Analysis of Forecast Accuracy
3. Results
3.1. Return Period of Wet Snow Events
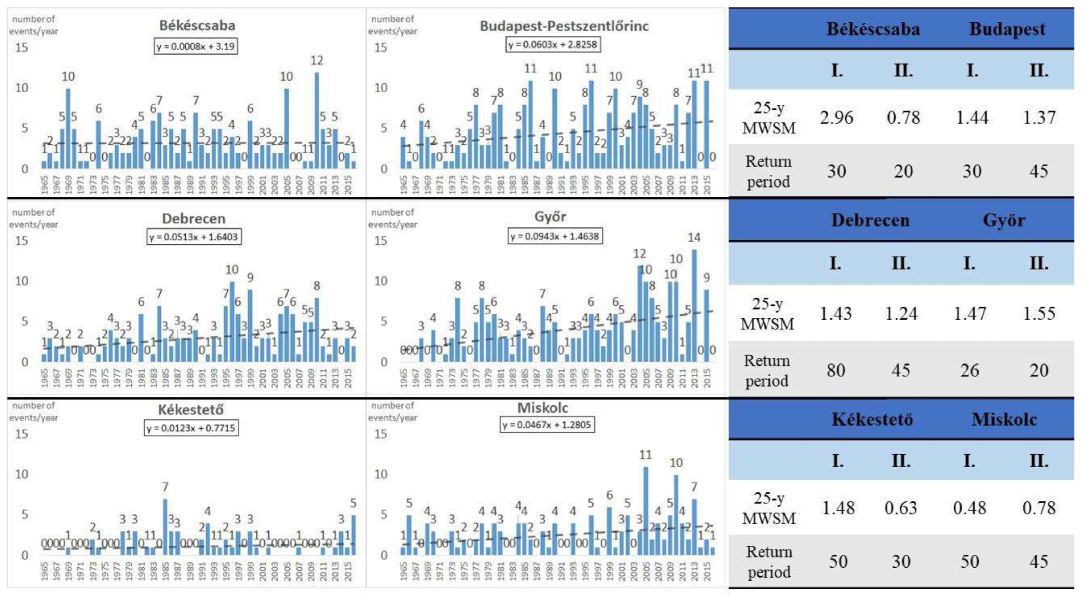
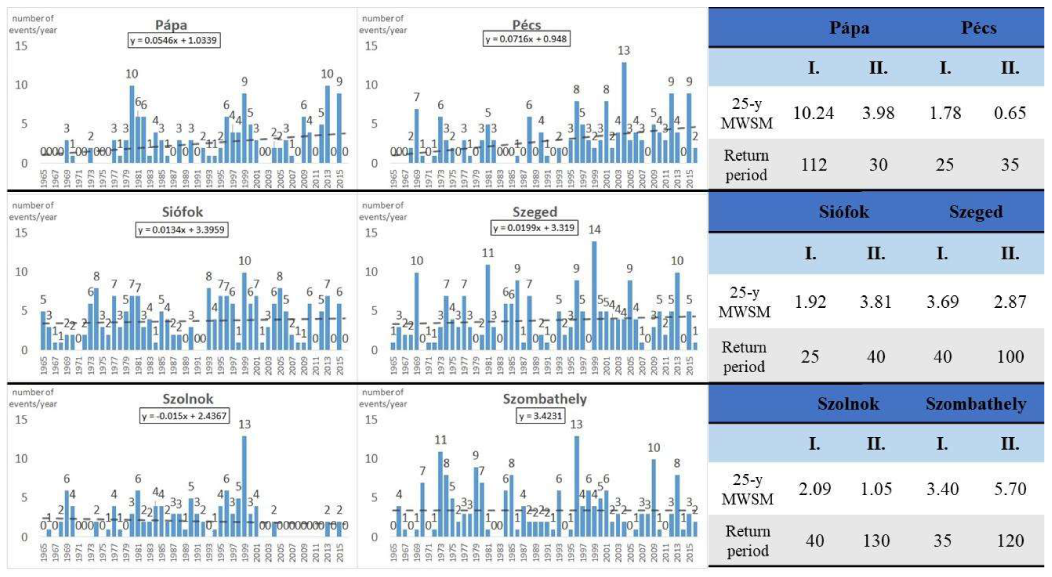
3.1.1. The Annual Frequency and 25-Year Maxima of Wet Snow Events
3.1.2. Return Period between 1965–1990 and 1991–2016
3.2. Classification of Ice Classes
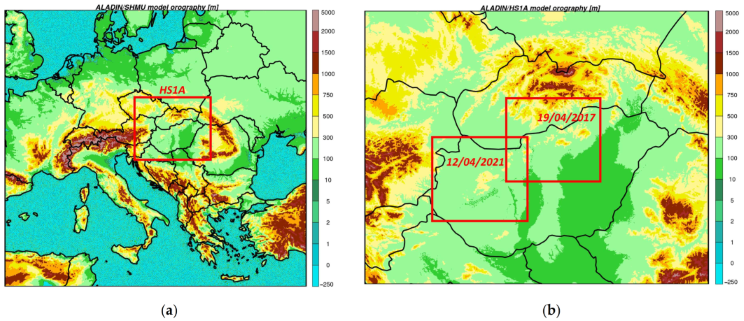
4. Case Studies
5. Discussion
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Wakahama, G.; Kuroiwa, D.; Goto, K. Snow Accretion on Electric Wires and its Prevention. J. Glaciol. 1977, 19, 479–487. [Google Scholar] [CrossRef] [Green Version]
- Sakamoto, Y. Snow accretion on overhead wires. Philos. Trans. R. Soc. London. Ser. A Math. Phys. Eng. Sci. 2000, 358, 2941–2970. [Google Scholar] [CrossRef]
- Makkonen, L.; Wichura, B. Simulating wet-snow loads on power line cables by a simple model. Cold Reg. Sci. Technol. 2010, 61, 73–81. [Google Scholar] [CrossRef]
- Dalle, B.; Admirat, P. Wet snow accretion on overhead lines with French report of experience. Cold Reg. Sci. Technol. 2011, 65, 43–51. [Google Scholar] [CrossRef]
- Yamamoto, K.; Matsumiya, H.; Sato, K.; Nemoto, M.; Togashi, K.; Matsushima, H.; Sugimoto, S. Evaluation for characteristics of wet snow accretion on transmission lines—Establishment of an experimental method using a vertical plate. Cold Reg. Sci. Technol. 2020, 174, 103014. [Google Scholar] [CrossRef]
- Poots, G.; Skelton, P.L.I. Thermodynamic models of wet-snow accretion: Axial growth and liquid water content on a fixed conductor. Int. J. Heat Fluid Flow 1995, 16, 43–49. [Google Scholar] [CrossRef]
- Tóth, K.; Kolláth, K. A tapadó hóteher mennyiségi előrejelzése. Légkör 2009, 54, 10–14. (In Hungarian) [Google Scholar]
- Bonelli, P.; Lacavalla, M.; Marcacci, P.; Mariani, G.; Stella, G. Wet snow hazard for power lines: A forecast and alert system applied in Italy. Nat. Hazard Earth Sys. 2011, 11, 2419–2431. [Google Scholar] [CrossRef]
- Somfalvi-Tóth, K.; Simon, A.; Kolláth, K.; Dezső, Z. Forecasting of wet- and blowing snow in Hungary. Időjárás 2015, 119, 277–306. [Google Scholar]
- Laforte, J.L.; Allaire, M.A.; Laflamme, J. State-of-the-art on power line de-icing. Atmos. Res. 1998, 46, 143–158. [Google Scholar] [CrossRef]
- Paul, S.; Chang, J. Design of novel electromagnetic energy harvester to power a deicing robot and monitoring sensors for transmission lines. Energy Convers. Manag. 2009, 197, 111868. [Google Scholar] [CrossRef]
- Kollár, L.E.; Farzaneh, M. Modeling Sudden Ice Shedding from Conductor Bundles. IEEE Trans. Power Deliv. 2013, 28, 604–611. [Google Scholar] [CrossRef]
- Somfalvi-Tóth, K. A Nagyfeszültségű Felsővezeték Hálózatot Érő Veszélyes Tapadó Hó Felhalmozódások Vizsgálata, Modellezése És Integrálása Az Előrejelzői Gyakorlatba. Ph.D. Thesis, ELTE, Budapest, Hungary, 2019. (In Hungarian). [Google Scholar]
- Sakakibara, D.; Nakamura, Y.; Kawashima, K.; Miura, S. Experimental result for snow accretion characteristics of communications cable. In Proceedings of the International Wire and Cable Symposium, Lake Buena Vista, FL, USA, 21–23 May 2007. [Google Scholar]
- Fikke, S.; Ronsten, G.; Heimo, A.; Kunz, S.; Ostrozlik, M.; Persson, P.E.; Sabata, J.; Wareing, B.; Wichura, B.; Chum, J.; et al. COST 727: Atmospheric Icing on Structures Measurements and Data Collection in Icing: State of the Art, Veröffentlichung MeteoSchweiz Nr. 75, Bundesamt Für Meteorologie und Klimatologie; Meteo Schweiz: Zurich, Switzerland, 2007; pp. 1–115. Available online: https://www.meteoswiss.admin.ch/dam/jcr:070f7439-3435-4966-8cbd-db30bbf59137/cost727atmosphericicingonstructures.pdf (accessed on 13 December 2022).
- Lacavalla, M.; Marcacci, P.; Freddo, A. Wet-snow activity research in Italy. In Proceedings of the International Workshop on Atmospheric Icing and Structures, Uppsala, Sweden, 28 June–3 July 2015. [Google Scholar]
- Csomor, M. A zúzmara megfigyelése. Légkör 1975, 3, 70–71. (In Hungarian) [Google Scholar]
- Szűcs, M.; Sepsi, P.; Simon, A. Hungary’s use of ECMWF ensemble boundary conditions. ECMWF Newsl. 2016, 148, 24–30. [Google Scholar] [CrossRef]
- Tóth, K.; Lakatos, M.; Kolláth, K.; Fülöp, R.; Simon, A. Climatology and forecasting of severe wet snow icing in Hungary. In Proceedings of the 13th International Workshop on Atmospheric Icing of Structures, IWAIS XIII, Andermatt, Switzerland, 8–11 September 2009. [Google Scholar]
- Radnóti, G.; Ajjaji, R.; Bubnová, R.; Caian, M.; Cordoneanu, E. The spectral limited area model Arpègè-Aladin. PWPR Rep. Ser. 1995, 7, 111–117. [Google Scholar]
- Horányi, A.; Ihász, I.; Radnóti, G. ARPEGE-ALADIN: A numerical weather prediction model for Central Europe with the participation of the Hungarian Meteorological Service. Időjárás 1996, 100, 277–300. [Google Scholar]
- ISO 12494; Atmospheric Icing of Structures. International Standardization Organisation (ISO): Geneva, Switzerland, 2001.
- Farzaneh, M. Atmospheric Icing of Power Networks, 1st ed.; Springer: Dordrecht, The Netherlands, 2008; pp. 1–373. [Google Scholar]
- Tozer, B.; Sandwell, D.T.; Smith, W.H.F.; Olson, C.; Beale, J.R.; Wessel, P. Global bathymetry and topography at 15 arc sec: SRTM15+. Earth Space Sci. 2019, 6, 1847–1864. [Google Scholar] [CrossRef]
- Nikolov, D.; Makkonen, L. Testing Six Wet Snow Models by 30 Years of Observations in Bulgaria. In Proceedings of the International Workshop on Atmospheric Icing and Structures, Uppsala, Sweden, 28 June–3 July 2015. [Google Scholar]
- Admirat, P. Wet Snow Accretion on Overhead lines. In Atmospheric Icing of Power Networks, 1st ed.; Farzaneh, M., Ed.; Springer: Dordrecht, The Netherlands, 2008; Volume 4, pp. 119–169. [Google Scholar] [CrossRef]
- Finstad, K.; Fikke, J.; Ervik, M. A comprehensive deterministic model for transmission line icing applied to laboratory and field observations. In Proceedings of the International Workshop on Atmospheric Icing and Structures, Paris, France, 4–7 September 1988. [Google Scholar]
- Sakamoto, Y.; Miura, A. Comparative study of wet snow models for estimating snow load on power lines based on general meteorological parameters. In Proceedings of the International Workshop on Atmospheric Icing and Structures, Budapest, Hungary, 20–23 September 1993. [Google Scholar]
- Nygaard, B.E.K.; Ágústsson, H.; Somfalvi-Tóth, K. Modeling Wet Snow Accretion on Power Lines: Improvements to Previous Methods Using 50 Years of Observations. J. Appl. Meteor. Climatol. 2013, 52, 2189–2203. [Google Scholar] [CrossRef]
- Rasmussen, R.; Baker, B.; Kochendorfer, J.; Myers, T.; Landolt, S.; Fisher, A.; Black, J.; Theriault, J.; Kucera, P.; Gochis, D.; et al. How well are we measuring snow? The NOAA/FAA/NCAR winter precipitation test bed. Bull. Am. Meteorol. Soc. 2012, 93, 811–829. [Google Scholar] [CrossRef] [Green Version]
- Goodison, B.E.; Louie, P.Y.T.; Yang, D. WMO Solid Precipitation Measurement Intercomparison—Final Report. In Instruments and Observing Reports, 1st ed.; Goodison, B.E., Ed.; World Meteorological Organization: Geneva, Italy, 1998; Available online: https://library.wmo.int/index.php?lvl=notice_display&id=6441#.Y2Yzy3bMLIU (accessed on 30 December 2022).
- Deneau, V.; Guillot, P. Wet-snow accretion on power lines: Cartographic examination of risks throughout France. Houille Blanche. 1984, 6, 465–474. [Google Scholar] [CrossRef] [Green Version]
- Admirat, P.; Sakamoto, Y.; DeGoncourt, B. Calibration of a snow accumulation model based on actual cases in Japan and France. In Proceedings of the International Workshop on Atmospheric Icing and Structures, Paris, France, 4–7 September 1988. [Google Scholar]
- Admirat, P.; Sakamoto, Y. Calibration of a wet-snow model on real cases in Japan and France. In Proceedings of the International Workshop on Atmospheric Icing and Structures, Paris, France, 4–7 September 1988. [Google Scholar]
- Le Du, M.; Laurent, C. T-Return Period Values of Meteorological Design Parameters. In Proceedings of the International Workshop on Atmospheric Icing and Structures, Montreal, QC, Canada, 12–16 June 2005. [Google Scholar]
- Lakatos, M.; Bihari, Z. Hóteher a távvezetékeken. A 2009. január 27–28-án kialakult időjárási helyzet elemzése Vas és Zala megye területén. Légkör 2009, 54, 6–9. (In Hungarian) [Google Scholar]
- Coles, S. An Introduction to Statistical Modeling of Extreme Values, 1st ed.; Springer: London, UK, 2001; Volume 4, pp. 74–91. [Google Scholar] [CrossRef]
- Lakatos, M.; Matyasovszky, I. Analysis of the extremity of precipitation intensity using the POT method. Időjárás 2004, 108, 155–208. [Google Scholar]
- Ribatet, M. POT: Modelling peaks over a threshold. R News 2007, 7, 34–36. [Google Scholar]
- Termonia, P.; Fischer, C.; Bazile, E.; Bouyssel, F.; Brožková, R.; Bénard, P.; Bochenek, B.; Degrauwe, D.; Derková, M.; El Khatib, R.; et al. The ALADIN System and its Canonical Model Configurations AROME CY41T1 and ALARO CY40T1. Geosci. Model Dev. 2018, 11, 257–281. [Google Scholar] [CrossRef] [Green Version]
- El Khatib, R. Fullpos Users Guide for Arpege/Aladin Cycle 25T1; Météo France—CNRM/GMAP: Toulouse, France, 2002; p. 68. Available online: https://confluence.ecmwf.int/download/attachments/19661682/fullpos_usersguide_cy25y1.pdf (accessed on 12 December 2022).
- Derková, M.; Vivoda, J.; Belluš, M.; Španiel, O.; Dian, M.; Neštiak, M.; Zehnal, R. Recent Improvements in the ALADIN/SHMU Operational System. Meteorol. J. 2017, 20, 45–52. Available online: https://www.shmu.sk/File/ExtraFiles/MET_CASOPIS/2017-2__MC.pdf (accessed on 5 November 2022).
- Simon, A.; Belluš, M.; Čatlošová, K.; Derková, M.; Dian, M.; Imrišek, M.; Kaňák, J.; Méri, L.; Neštiak, M.; Vivoda, J. Numerical simulations of June 7, 2020 convective precipitation over Slovakia using deterministic, probabilistic, and convection-permitting approaches. Idojaras 2021, 125, 571–607. [Google Scholar] [CrossRef]
- Taillefer, F.; CANARI Technical Documentation. Based on ARPEGE Cycle CY25T1 (AL25T1 for ALADIN); CNRM/GMAP Meteo France. 2002. Available online: http://www.cnrm.meteo.fr/gmapdoc/spip.php?article3 (accessed on 5 November 2022).
- Courtier, P.; Geleyn, J.-F. A global numerical weather prediction model with variable resolution: Application to the shallow model equations. Quart. J. Roy. Meteor. Soc. 1988, 114, 1321–1346. [Google Scholar] [CrossRef]
- Courtier, P.; Freydier, C.; Geleyn, J.-F.; Rabier, F.; Rochas, M. The ARPEGE project at Météo-France. In Proceedings of the 1991 ECMWF Seminar on Numerical Methods in Atmospheric Models, ECMWF, Reading, UK, 9–13 September 1991; pp. 193–231. [Google Scholar]
- Simmons, A.J.; Burridge, D. An energy and angularmomentum conserving vertical finite-difference scheme and hybrid vertical coordinates. Mon. Weather Rev. 1981, 109, 758–766. [Google Scholar] [CrossRef]
- Hortal, M. The development and testing of a new two-time-level semi-Lagrangian scheme (SETTLS) in the ECMWF forecast model. Quart. J. Roy. Meteorol. Soc. 2002, 128, 1671–1687. [Google Scholar] [CrossRef]
- Bénard, P.; Vivoda, J.; Mašek, J.; Smolíková, P.; Yessad, K.; Smith, C.; Brožková, R.; Geleyn, J.F. Dynamical kernel of the Aladin–NH spectral limited-area model: Revised formulation and sensitivity experiments. Quart. J. Roy. Meteor. Soc. 2010, 136, 155–169. [Google Scholar] [CrossRef]
- Ďurán, I.B.; Geleyn, J.; Váňa, F. A Compact Model for the Stability Dependency of TKE Production–Destruction–Conversion Terms Valid for the Whole Range of Richardson Numbers. J. Atmos. Sci. 2014, 71, 3004–3026. [Google Scholar] [CrossRef]
- Ďurán, I.B.; Geleyn, J.; Váňa, F.; Schmidli, J.; Brožková, R. A Turbulence Scheme with Two Prognostic Turbulence Energies. J. Atmos. Sci. 2018, 75, 3381–3402. [Google Scholar] [CrossRef]
- Gerard, L.; Piriou, J.-M.; Brožková, R.; Geleyn, J.-F.; Banciu, D. Cloud and precipitation parameterization in a meso-gamma scale operational weather prediction model. Mon. Weather Rev. 2009, 137, 3960–3977. [Google Scholar] [CrossRef]
- Lopez, P. Implementation and validation of a new prognostic large-scale cloud and precipitation scheme for climate and data assimilation purposes. Quart. J. Roy. Meteor. Soc. 2002, 128, 229–257. [Google Scholar] [CrossRef]
- Noilhan, J.; Planton, S. A simple parameterization of land surface processes for meteorological models. Mon. Weather Rev. 1989, 117, 536–549. [Google Scholar] [CrossRef]
- Masson, V.; Champeaux, J.-L.; Chauvin, F.; Meriguet, C.; Lacaze, R. A Global Database of Land Surface Parameters at 1-km Resolution in Meteorological and Climate Models. J. Clim. 2003, 16, 1261–1282. [Google Scholar] [CrossRef]
- Faroux, S.; Masson, V.; Roujean, J.-L. ECOCLIMAP-II: A climatologic global data base of ecosystems and land surface parameters at 1 km based on the analysis of time series of VEGETATION data. In Proceedings of the 2007 IEEE International Geoscience and Remote Sensing Symposium, Barcelona, Spain, 23–28 July 2007; pp. 1008–1011. [Google Scholar] [CrossRef]
- Brožková, R.; Bučánek, A.; Mašek, J.; Smolíková, P.; Trojáková, A. Nová provozní konfigurace modelu Aladin ve vysokém rozlišení. Meteorol. Zprávy 2019, 72, 129–139. Available online: http://www.cmes.cz/sites/default/files/chmu_mz_5-19_129-139.pdf (accessed on 5 November 2022).
- Brožková, R.; Bučánek, A.; Mašek, J.; Němec, D.; Smolíková, P.; Šábik, F.; Trojáková, A. Numerical Weather Prediction Activities at CHMI. In Proceedings of the ACCORD 1st AWS, Video Conference, 12–16 April 2021; Available online: http://www.umr-cnrm.fr/accord/IMG/pdf/poster_asw_2021_cz.pdf (accessed on 5 November 2022).
- Huai, B.; Broeke, M.R.; Reijmer, C.H.; Cappellen, J. Quantifying Rainfall in Greenland: A Combined Observational and Modeling Approach. J. Appl. Meteor. Climatol. 2021, 60, 1171–1188. [Google Scholar] [CrossRef]
- Ducloux, H.; Nygaard, B.E. 50-year return-period wet-snow load estimation based on weather station data for overhead line design in France. Nat. Hazards Earth Syst. Sci. 2014, 14, 3031–3041. [Google Scholar] [CrossRef] [Green Version]
- Gulyás, K. A Tapadó Hó Statisztikus Klimatológiai Vizsgálata És Előrejelzési Lehetőségei. Master’s Thesis, ELTE, Budapest, Hungary, 2012. [Google Scholar]
- OMSZ. Magyarország Időjárása (Daily Weather Report of 20 April 2017, In Hungarian); Országos Meteorológiai Szolgálat. Available online: https://owww.met.hu/idojaras/aktualis_idojaras/napijelentes_2005-2019/main.php?ts=1492646400&ful=napijelentes_szoveg (accessed on 5 November 2022).
- Erdődiné Molnár, Z.; (OMSZ, Bükkszentkereszt, Hungary); Simon, A.; (OMSZ, Budapest, Hungary). Personal communication, 2017.
- OMSZ. Időjárás Napijelentés (Daily Weather Report of 14 April 2021, In Hungarian); Országos Meteorológiai Szolgálat. Available online: https://met.hu/downloads.php?id=20&file=20210414&type=1 (accessed on 5 November 2022).
- Makkonen, L.; Lehtonen, P.; Helle, L. Anemometry in Icing Conditions. J. Atmos. Oceanic Technol. 2001, 18, 1457–1469. [Google Scholar] [CrossRef]
- Tóth, R.; (OMSZ, Budapest, Hungary); Simon, A.; (OMSZ, Budapest, Hungary). Personal communication, 2022.
- Lacavalla, M.; Sperati, S.; Bonanno, R.; Marcacci, P. A Revision of Wet Snow Load Map for the Italian Power Lines with a New High Resolution Reanalysis Dataset. In Proceedings of the International Workshop on Atmopheric Icing of Structures, IWAIS, Reykjavík, Iceland, 23–28 June 2019; Available online: https://iwais2019.is/images/Papers/012_IWAIS2019_complete_paper_Lacavalla.pdf (accessed on 13 December 2022).
- Simon, A.; Somfalvi-Tóth, K.; Szűcs, M. Probabilistic forecasting of freezing rain and wet snow in Hungary. In Proceedings of the EMS Annual Meeting: European Conference for Applied Meteorology and Climatology, Budapest, Hungary, 2–7 September 2018. [Google Scholar]
- Lancz, D.; Jávorné Radnóczi, K.; Szanyi, K.; Szepszo, G.; Szintai, B.; Tóth, B.; Tóth, G.; Tóth, H.; Várkonyi, A. NWP activities at the Hungarian Meteorological Service. In Proceedings of the 44th EWGLAM–29th SRNWP Workshop, Brussels, Belgium, 26–29 September 2022. [Google Scholar]
- Ablonczy, D. How Do We Measure the Rain? How Strong is the Sun Now? How Does the Anemometer Work? Meteorological Instruments Simply in Practice. Légkör 2018, 63, 163–177. [Google Scholar]
- Gascón, E.; Hewson, T.; Haiden, T. Improving Predictions of Precipitation Type at the Surface: Description and Verification of Two New Products from the ECMWF Ensemble. Wea. Forecast. 2018, 33, 89–108. [Google Scholar] [CrossRef]
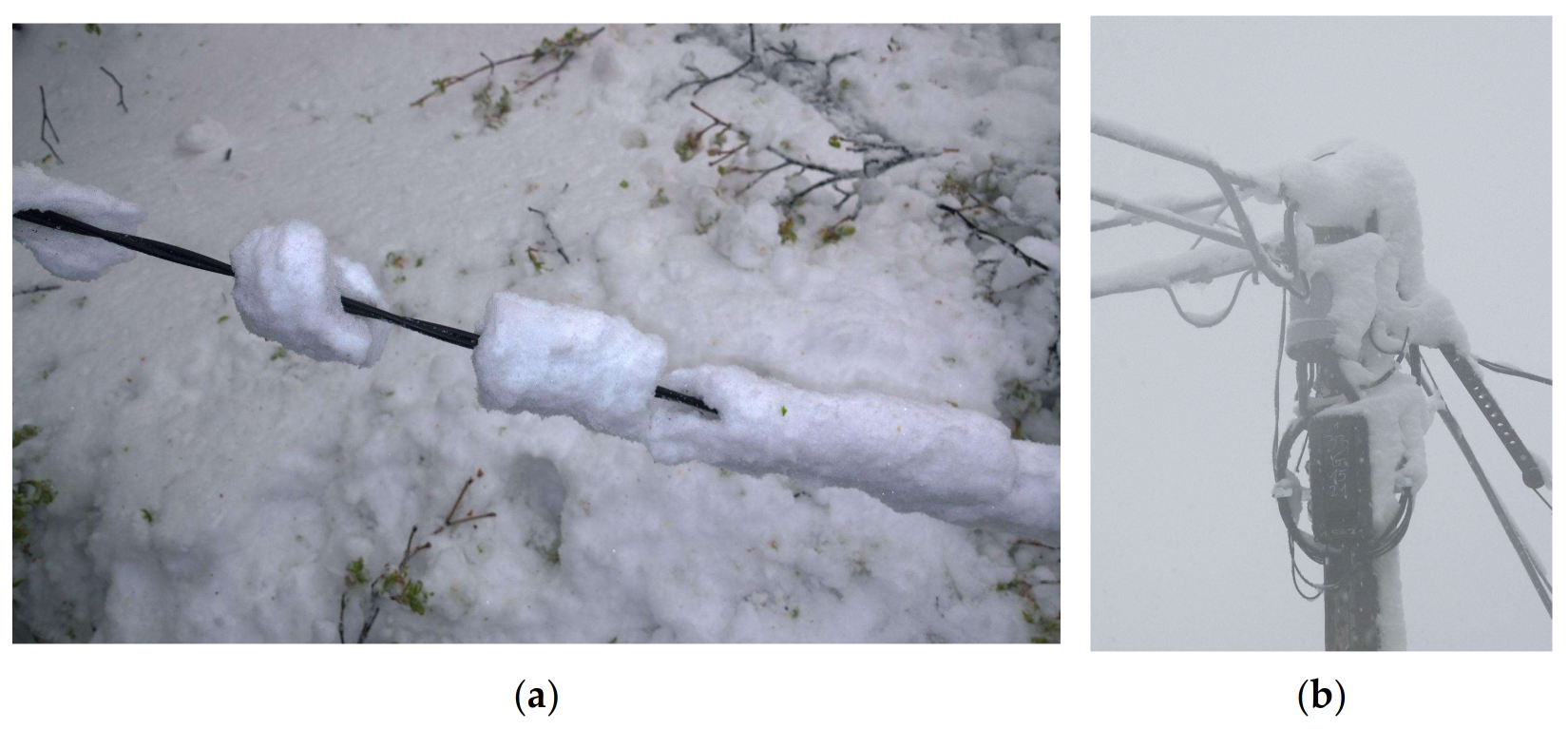
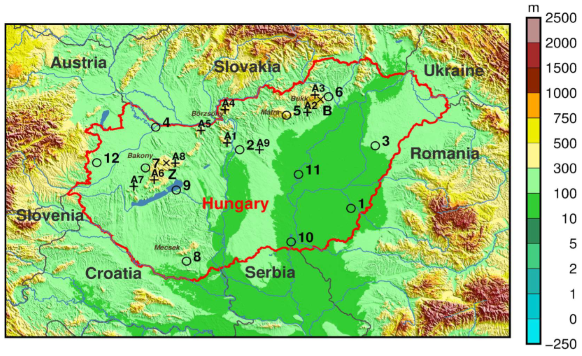



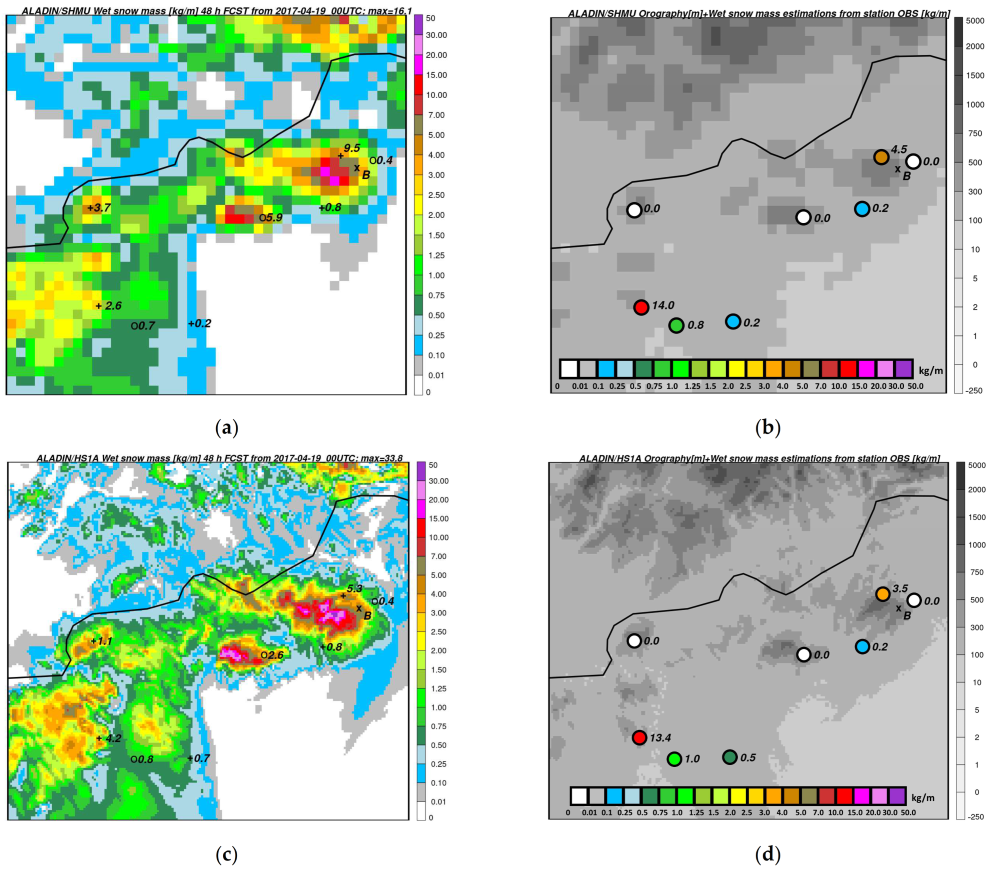
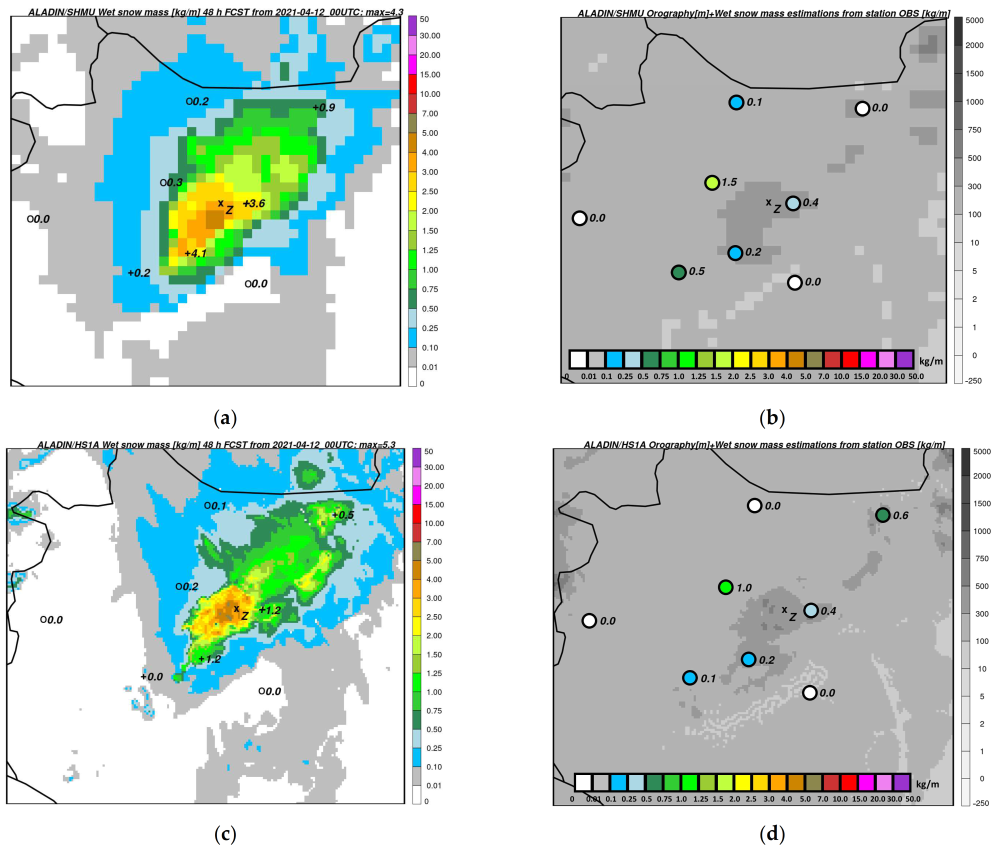

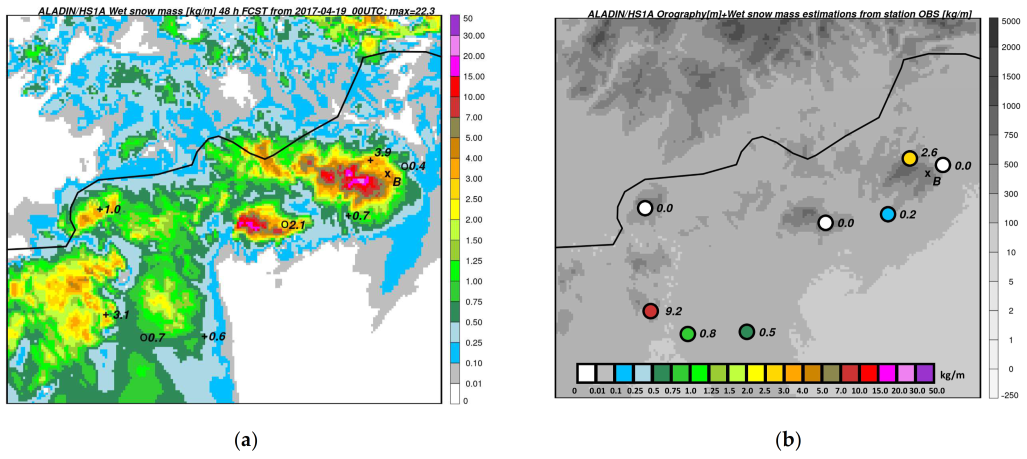
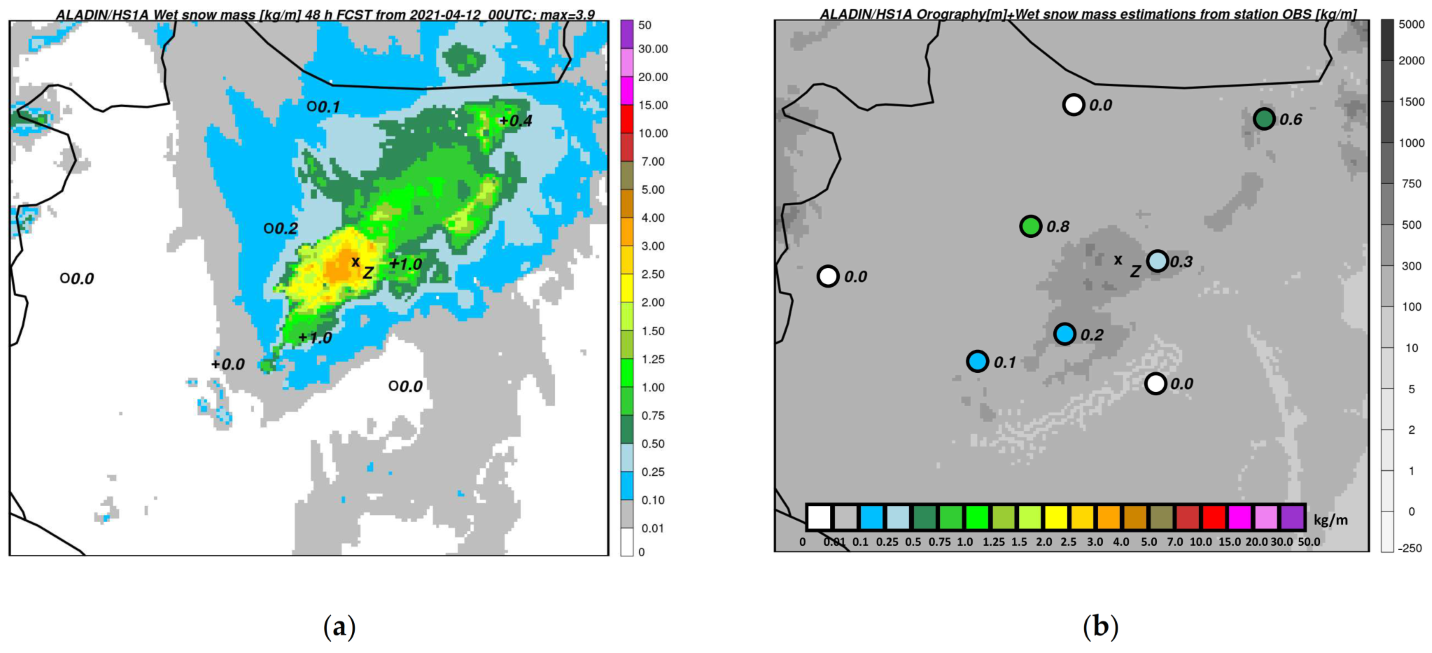
| Station Name | Label | Latitude | Longitude | Height a.s.l. [m] |
|---|---|---|---|---|
| Békéscsaba | 1 | 46.7 | 21.1 | 89 |
| Budapest-Pestszentlőrinc | 2 | 47.4 | 19.3 | 139 |
| Debrecen | 3 | 47.5 | 21.6 | 121 |
| Győr | 4 | 47.6 | 17.6 | 108 |
| Kékestető | 5 | 47.9 | 20.0 | 1012 |
| Miskolc | 6 | 48.1 | 20.7 | 162 |
| Pápa | 7 | 47.3 | 17.4 | 200 |
| Pécs | 8 | 46.0 | 18.2 | 153 |
| Siófok | 9 | 46.9 | 18.1 | 124 |
| Szeged | 10 | 46.2 | 20.1 | 76 |
| Szolnok | 11 | 47.1 | 20.2 | 68 |
| Szombathely | 12 | 47.2 | 16.6 | 209 |
| Label (Figure 3) | Station | Station Height (m) | NWP Model Height | |
|---|---|---|---|---|
| ALADIN/ SHMU | ALADIN/ HS1A | |||
| A1 | Budapest János-hegy | 516 | 394 | 446 |
| A2 | Eger | 225 | 214 | 202 |
| A3 | Miskolc Szentlélek | 752 | 724 | 754 |
| A4 | Nagy Hideg hegy | 855 | 568 | 793 |
| A5 | Gerecse tető | 620 | 373 | 584 |
| A6 | Kab-hegy | 595 | 485 | 525 |
| A7 | Sümeg | 195 | 174 | 176 |
| A8 | Tés | 472 | 464 | 467 |
| A9 | Sülysáp | 181 | 159 | 190 |
| A10 | Budapest Zugliget | 421 | 394 | 405 |
| Model Version | ALADIN/SHMU | ALADIN/HS1A |
|---|---|---|
| Status | operational | experimental |
| Horizontal resolution | 4.5 km | 1.0 km |
| Number of vertical levels | 63 | 73 |
| Domain size | 2813 × 2592 km | 720 × 576 km |
| Coupling model | ARPEGE, 3 h coupling frequency | ALADIN/SHMU, 3 h coupling frequency |
| Initialization | none | none |
| Data assimilation | optimal interpolation CANARI [44] | none (dynamical and LBC downscaling) |
| Dynamics | hydrostatic, spectral, semi-implicit, 2 time level semi-lagrangian scheme | non-hydrostatic, spectral, semi-implicit 2 time level iterative lefted implicit scheme |
| Physics | ALARO-1vB | ALARO-1vB, adapted for convection-permitting scales (after [57,58]) |
| ISO International Ice Classes [22] | Snow Mass on the Wire (kg·m−1) | Diameter of Snow Sleeve (cm) | Calculated Intervals (kg·m−1) [60] | |
|---|---|---|---|---|
| (Diameter of Wire is 30 mm) | ||||
| Snow Density (kg·m−3) | ||||
| 300 | 500 | |||
| R1 | 0.5 | 5.5 | 4.7 | 0–0.70 |
| R2 | 0.9 | 6.9 | 5.6 | 0.71–1.26 |
| R3 | 1.6 | 8.8 | 7.1 | 1.27–2.25 |
| R4 | 2.8 | 11.3 | 9.0 | 2.26–3.95 |
| R5 | 5.0 | 14.9 | 11.7 | 3.96–7.05 |
| R6 | 8.9 | 19.7 | 15.4 | 7.06–12.55 |
| R7 | 16 | 26.2 | 20.4 | 12.56–22.55 |
| R8 | 28 | 34.6 | 26.9 | 22.56–39.48 |
| R9 | 50 | 46.2 | 35.8 | 39.49–70.50 |
| R10 | Extreme icing events | >70.51 | ||
| Station | NC (1965–2016) | Trend Analysis of ANC t-Value (p = 0.05) | Trend Analysis of MWSM t-Value (p = 0.05) |
|---|---|---|---|
| Békéscsaba | 167 | 0.78 | −2.24 |
| Budapest-Pestszentlőrinc | 230 | 77.6 | −0.19 |
| Debrecen | 156 | 47.73 | 0.006 |
| Győr | 206 | 127.36 | 0.67 |
| Kékestető | 57 | 6.69 | −0.03 |
| Miskolc | 131 | 42.98 | −0.34 |
| Pápa | 129 | 57.08 | −4.35 |
| Pécs Pogány | 148 | 80.25 | −1.08 |
| Siófok | 195 | 13.13 | 0.73 |
| Szeged | 200 | 23.62 | −2.1 |
| Szolnok | 106 | −13.09 | −0.73 |
| Szombathely | 178 | 0 | −1.69 |
| Station | Label | Ice Class 1965–1990 | Ice Class 1991–2016 |
|---|---|---|---|
| Békéscsaba | 1 | R5 | R3 |
| Budapest | 2 | R3 | R3 |
| Debrecen | 3 | R3 | R3 |
| Győr | 4 | R3 | R4 |
| Kékestető | 5 | R3 | R2 |
| Miskolc | 6 | R2 | R2 |
| Pápa | 7 | R5 | R5 |
| Pécs | 8 | R4 | R2 |
| Siófok | 9 | R4 | R5 |
| Szeged | 10 | R5 | R3 |
| Szolnok | 11 | R4 | R2 |
| Szombathely | 12 | R5 | R4 |
| Parameter | 2 m Temperature | 10 m Wind | Total Precipitation | Wet Snow Mass | ||||
|---|---|---|---|---|---|---|---|---|
| SHMU | HS1A | SHMU | HS1A | SHMU | HS1A | SHMU | HS1A | |
| BIAS | 1.16 | 0.65 | 1.42 | 1.44 | −29.41 | −25.5 | 0.3 | −0.36 |
| MAE | 1.21 | 0.73 | 2.8 | 2.17 | 29.86 | 26 | 3.13 | 1.85 |
| Parameter | 2 m Temperature | 10 m Wind | Total Precipitation | Wet Snow Mass | ||||
|---|---|---|---|---|---|---|---|---|
| SHMU | HS1A | SHMU | HS1A | SHMU | HS1A | SHMU | HS1A | |
| BIAS | 0.71 | 0.77 | 1.32 | 1.37 | −0.27 | −5.1 | 0.84 | 0.1 |
| MAE | 0.75 | 0.77 | 2.27 | 2.41 | 9.1 | 10.1 | 1.22 | 0.37 |
| Observation Replaced by Model Data | Temperature | Wind | Precipitation | All | ||||
|---|---|---|---|---|---|---|---|---|
| SHMU | HS1A | SHMU | HS1A | SHMU | HS1A | SHMU | HS1A | |
| BIAS | 7.06 | 2.32 | 0.21 | −0.04 | −1.49 | −1.43 | 0.39 | −0.37 |
| MAE | 7.85 | 2.48 | 2.68 | 1.75 | 1.58 | 1.56 | 3.15 | 1.79 |
| Observation Replaced by Model Data | Temperature | Wind | Precipitation | All | ||||
|---|---|---|---|---|---|---|---|---|
| SHMU | HS1A | SHMU | HS1A | SHMU | HS1A | SHMU | HS1A | |
| BIAS | 1.42 | 1.07 | 0.06 | 0.07 | −0.11 | −0.15 | 0.84 | 0.1 |
| MAE | 1.62 | 1.14 | 0.08 | 0.09 | 0.23 | 0.19 | 1.22 | 0.37 |
| Label | Name | Height a.s.l. [m] | Pw [mm] NC | Pw [mm] C, N.U.L | Pw [mm] C, Umax | M [kg m−1] NC | M [kg m−1] C, N.U.L | M [kg m−1] C, Umax |
|---|---|---|---|---|---|---|---|---|
| 2 | Budapest-Pestszentlőrinc | 139 | 11.07 | 13.55 | 13.55 | 0.74 | 0.98 | 0.98 |
| 6 | Miskolc | 162 | 0 | 0 | 0 | 0 | 0 | 0 |
| A9 | Sülysáp | 181 | 5.64 | 7.5 | 7.5 | 0.36 | 0.52 | 0.52 |
| A2 | Eger | 225 | 3.27 | 3.72 | 3.72 | 0.18 | 0.21 | 0.21 |
| A10 | Budapest Zugliget | 421 | 26 | 26 | 26 | 4.26 | 4.26 | 4.26 |
| A1 | Budapest János-hegy | 516 | 17.44 | 41.22 | 38.57 | 3.09 | 13.38 | 11.8 |
| A3 | Miskolc Szentlélek | 752 | 22.72 | 31.16 | 31.16 | 2.14 | 3.53 | 3.53 |
| A4 | Nagy-Hideg hegy | 855 | 0.45 | 0.6 | 0.6 | 0.02 | 0.03 | 0.03 |
| 5 | Kékestető | 1012 | 0 | 0 | 0 | 0 | 0 | 0 |
| Label | Name | Height a.s.l. [m] | Pw [mm] NC | Pw [mm] C, N.U.L | Pw [mm] C, Umax | M [kg m−1] NC | M [kg m−1] C, N.U.L | M [kg m−1] C, Umax |
|---|---|---|---|---|---|---|---|---|
| 9 | Siófok | 108 | 0 | 0 | 0 | 0 | 0 | 0 |
| 4 | Győr | 117 | 0.73 | 0.82 | 0.82 | 0.04 | 0.04 | 0.04 |
| 7 | Pápa | 145 | 4.9 | 8.76 | 8.68 | 0.46 | 1.02 | 1.00 |
| A7 | Sümeg | 195 | 0.95 | 1.52 | 1.52 | 0.06 | 0.10 | 0.10 |
| 12 | Szombathely | 201 | 0 | 0 | 0 | 0 | 0 | 0 |
| A8 | Tés | 472 | 2.35 | 4.42 | 4.42 | 0.17 | 0.36 | 0.36 |
| A6 | Kab-hegy | 595 | 0.88 | 2.39 | 2.05 | 0.08 | 0.24 | 0.20 |
| A5 | Gerecse tető | 620 | 4.74 | 7.87 | 7.87 | 0.33 | 0.65 | 0.65 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Somfalvi-Tóth, K.; Simon, A. Extreme Value Analysis and Modelling of Wet Snow Accretion on Overhead Lines in Hungary. Atmosphere 2023, 14, 81. https://doi.org/10.3390/atmos14010081
Somfalvi-Tóth K, Simon A. Extreme Value Analysis and Modelling of Wet Snow Accretion on Overhead Lines in Hungary. Atmosphere. 2023; 14(1):81. https://doi.org/10.3390/atmos14010081
Chicago/Turabian StyleSomfalvi-Tóth, Katalin, and André Simon. 2023. "Extreme Value Analysis and Modelling of Wet Snow Accretion on Overhead Lines in Hungary" Atmosphere 14, no. 1: 81. https://doi.org/10.3390/atmos14010081
APA StyleSomfalvi-Tóth, K., & Simon, A. (2023). Extreme Value Analysis and Modelling of Wet Snow Accretion on Overhead Lines in Hungary. Atmosphere, 14(1), 81. https://doi.org/10.3390/atmos14010081





