Turbulence with Magnetic Helicity That Is Absent on Average
Abstract
:1. Introduction
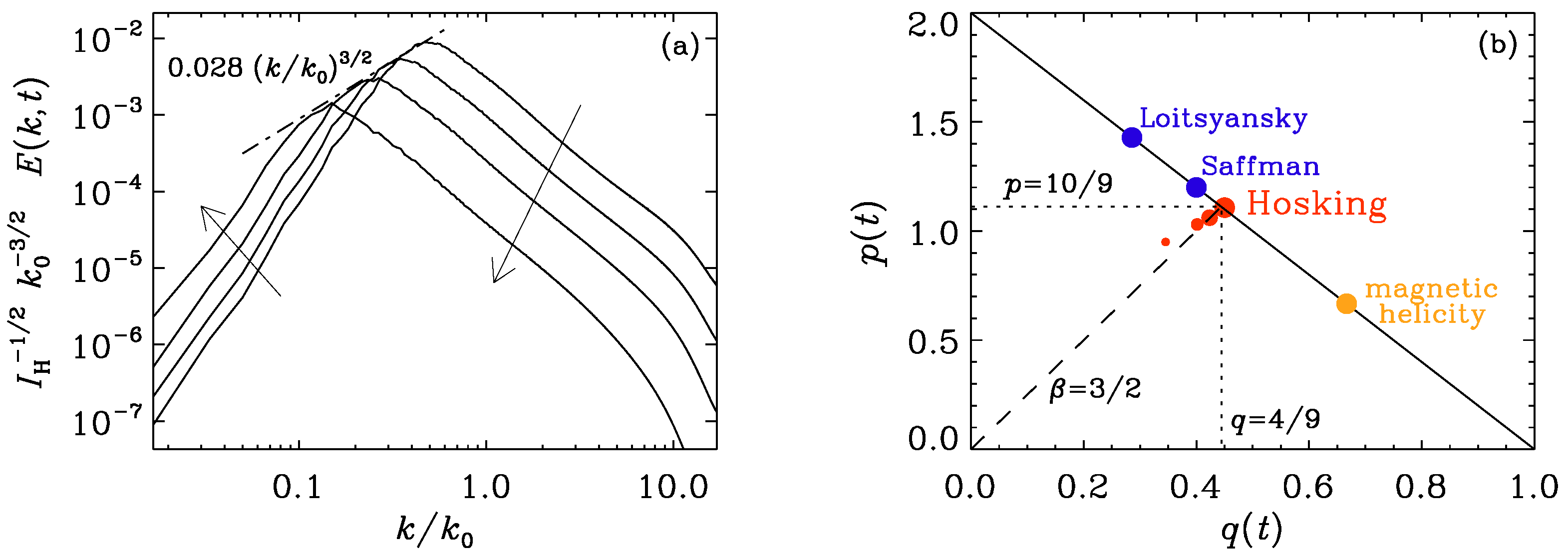
2. Nonhelical Turbulence and the Hosking Integral
2.1. Nonhelical Inverse Cascading and Scaling Relations
2.2. The Loitsyansky and Saffman Integrals in Hydrodynamics
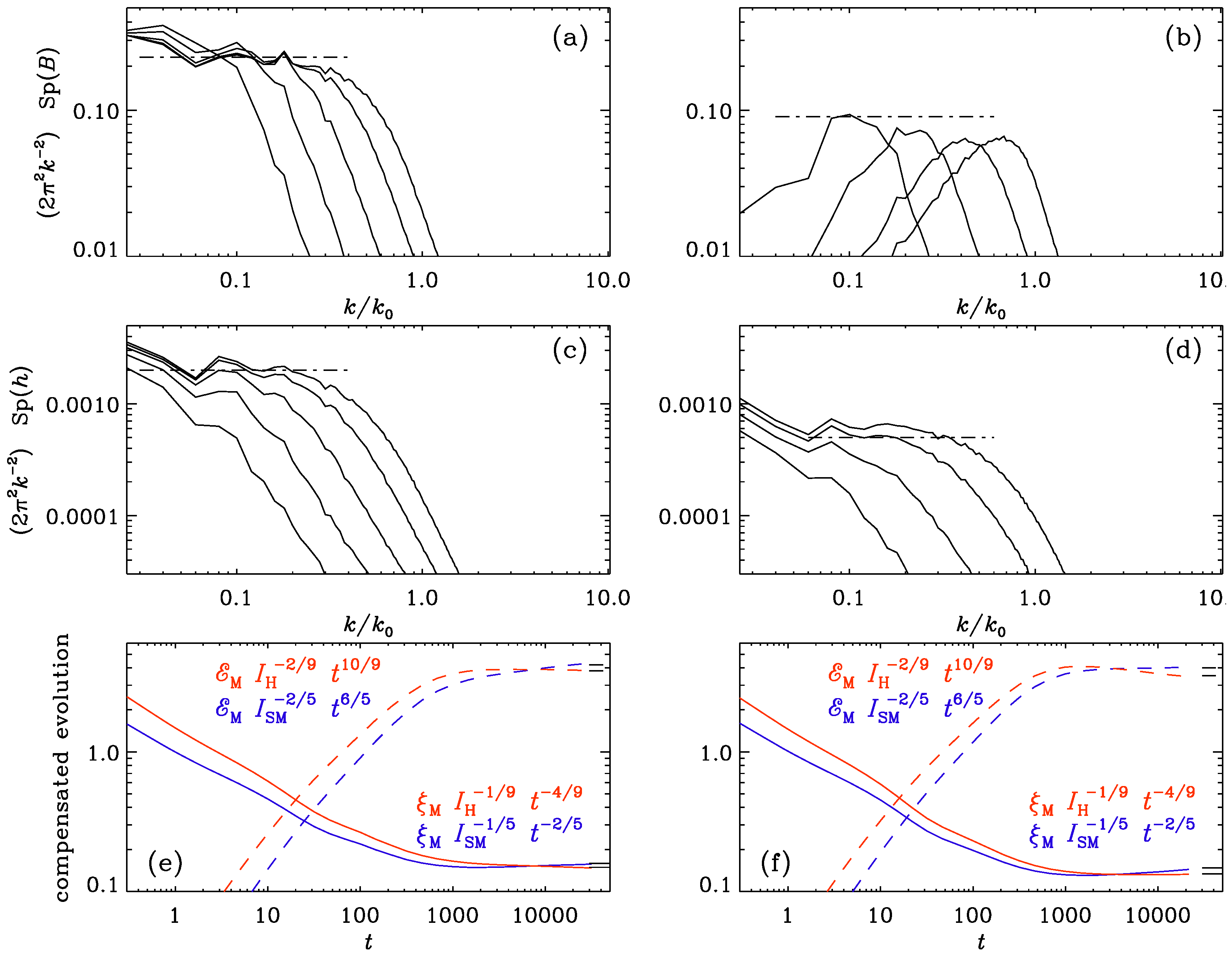
2.3. The Magnetic Saffman Integral: Comparison with the Hosking Integral
2.4. The Effect of Rotation
3. Extensions of the Hosking Idea
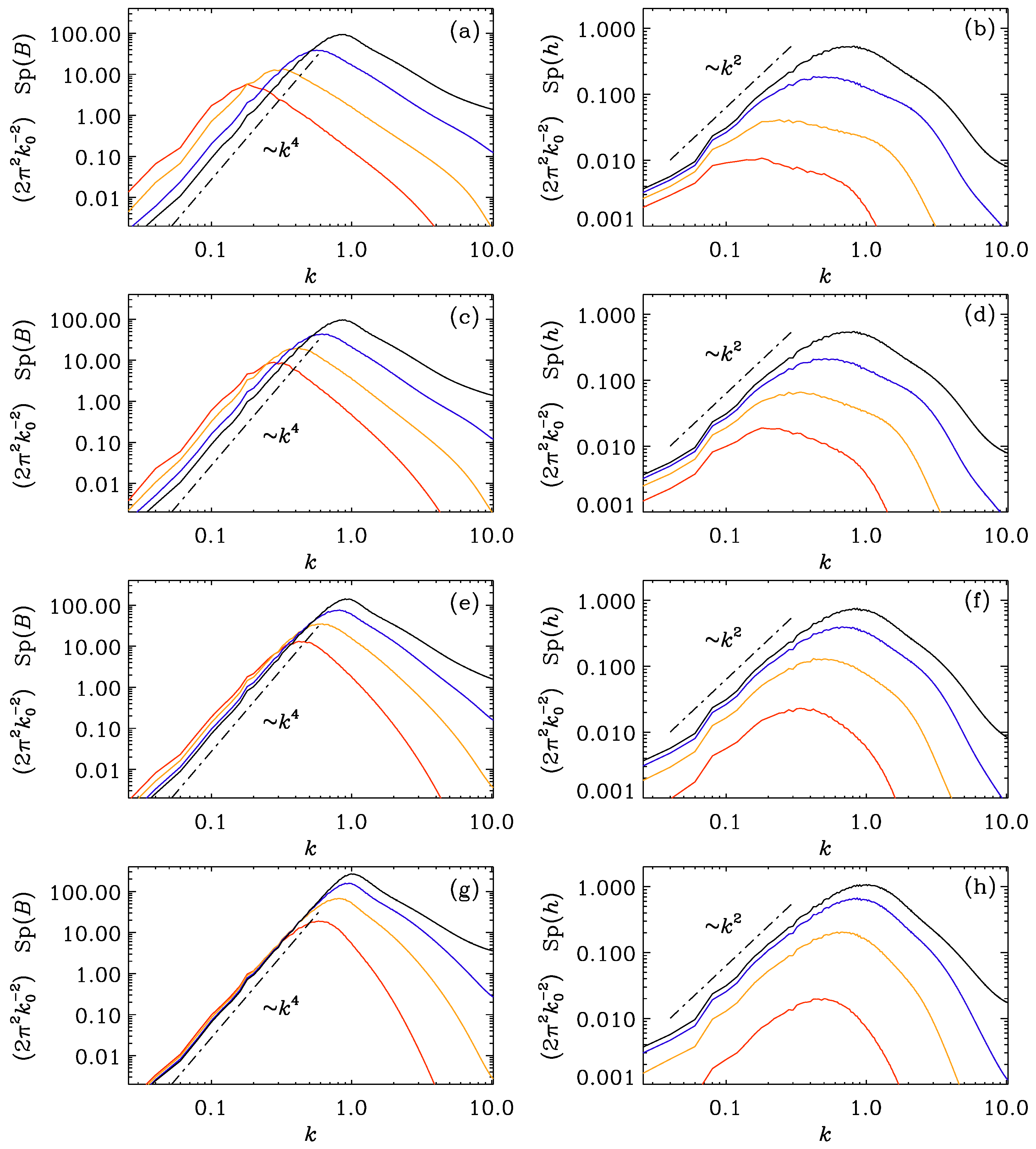
3.1. Hall Effect
3.2. Ambipolar Diffusion
3.3. Chiral MHD
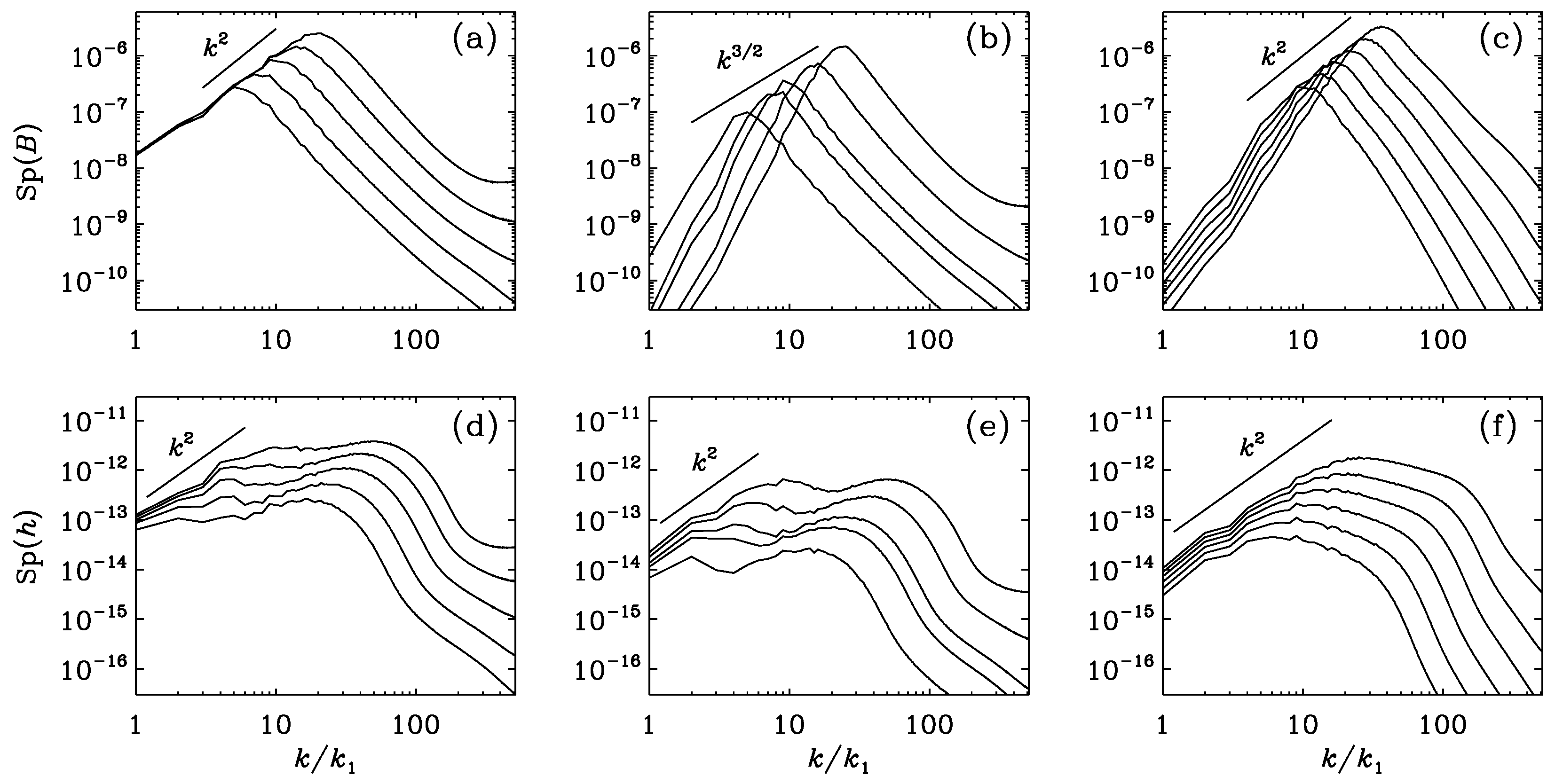
4. Hosking Integral in Shell Models of Chiral MHD
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Herring, J.R.; Licht, A.L. Effect of the solar wind on the lunar atmosphere. Science 1959, 130, 266. [Google Scholar] [CrossRef] [PubMed]
- Herring, J.; Kyle, L. Density in a planetary exosphere. J. Geophys. Res. 1961, 66, 1980–1982. [Google Scholar] [CrossRef] [Green Version]
- Arking, A.; Herring, J. The contribution of absorption lines to the opacity of matter in stellar interiors. Publ. Astron. Soc. Pac. 1963, 75, 226. [Google Scholar] [CrossRef]
- Parker, E.N. Dynamics of the interplanetary gas and magnetic fields. Astrophys. J. 1958, 128, 664. [Google Scholar] [CrossRef]
- Henyey, L.G.; Forbes, J.E.; Gould, N.L. A new method of automatic computation of stellar evolution. Astrophys. J. 1964, 139, 306. [Google Scholar] [CrossRef]
- Pouquet, A.; Frisch, U.; Leorat, J. Strong MHD helical turbulence and the nonlinear dynamo effect. J. Fluid Mech. 1976, 77, 321–354. [Google Scholar] [CrossRef] [Green Version]
- Pouquet, A.; Rosenberg, D.; Stawarz, J.E.; Marino, R. Helicity dynamics, inverse, and bidirectional cascades in fluid and magnetohydrodynamic turbulence: A brief review. Earth Space Sci. 2019, 6, 351–369. [Google Scholar] [CrossRef] [Green Version]
- Moffatt, H.K. Magnetic Field Generation in Electrically Conducting Fluids; Cambridge University Press: Cambridge, UK, 1978. [Google Scholar]
- Parker, E.N. Cosmical Magnetic Fields: Their Origin and Their Activity; Clarendon Press: Oxford, UK, 1979. [Google Scholar]
- Krause, F.; Rädler, K.H. Mean-Field Magnetohydrodynamics and Dynamo Theory; Pergamon Press: Oxford, UK, 1980. [Google Scholar]
- Matthaeus, W.H.; Goldstein, M.L. Measurement of the rugged invariants of magnetohydrodynamic turbulence in the solar wind. J. Geophys. Res. 1982, 87, 6011–6028. [Google Scholar] [CrossRef]
- Brandenburg, A.; Subramanian, K. Astrophysical magnetic fields and nonlinear dynamo theory. Phys. Rep. 2005, 417, 1–209. [Google Scholar] [CrossRef] [Green Version]
- Rust, D.M.; Kumar, A. Evidence for helically kinked magnetic flux ropes in solar eruptions. Astrophys. J. Lett. 1996, 464, L199. [Google Scholar] [CrossRef]
- Brandenburg, A.; Subramanian, K.; Balogh, A.; Goldstein, M.L. Scale dependence of magnetic helicity in the solar wind. Astrophys. J. 2011, 734, 9. [Google Scholar] [CrossRef] [Green Version]
- Christensson, M.; Hindmarsh, M.; Brandenburg, A. Inverse cascade in decaying three-dimensional magnetohydrodynamic turbulence. Phys. Rev. E 2001, 64, 056405. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kahniashvili, T.; Tevzadze, A.G.; Brandenburg, A.; Neronov, A. Evolution of primordial magnetic fields from phase transitions. Phys. Rev. D 2013, 87, 083007. [Google Scholar] [CrossRef] [Green Version]
- Durrer, R.; Kahniashvili, T.; Yates, A. Microwave background anisotropies from Alfvén waves. Phys. Rev. D 1998, 58, 123004. [Google Scholar] [CrossRef] [Green Version]
- Durrer, R.; Caprini, C. Primordial magnetic fields and causality. J. Cosmol. Astropart. Phys. 2003, 2003, 010. [Google Scholar] [CrossRef] [Green Version]
- Reppin, J.; Banerjee, R. Nonhelical turbulence and the inverse transfer of energy: A parameter study. Phys. Rev. E 2017, 96, 053105. [Google Scholar] [CrossRef] [Green Version]
- Brandenburg, A.; Kahniashvili, T.; Mandal, S.; Pol, A.R.; Tevzadze, A.G.; Vachaspati, T. Evolution of hydromagnetic turbulence from the electroweak phase transition. Phys. Rev. D 2017, 96, 123528. [Google Scholar] [CrossRef] [Green Version]
- Brandenburg, A.; Kahniashvili, T.; Tevzadze, A.G. Nonhelical inverse transfer of a decaying turbulent magnetic field. Phys. Rev. Lett. 2015, 114, 075001. [Google Scholar] [CrossRef] [Green Version]
- Zrake, J. Inverse cascade of nonhelical magnetic turbulence in a relativistic fluid. Astrophys. J. Lett. 2014, 794, L26. [Google Scholar] [CrossRef] [Green Version]
- Hosking, D.N.; Schekochihin, A.A. Reconnection-controlled decay of magnetohydrodynamic turbulence and the role of invariants. Phys. Rev. X 2021, 11, 041005. [Google Scholar] [CrossRef]
- Zhou, H.; Sharma, R.; Brandenburg, A. Scaling of the Hosking integral in decaying magnetically dominated turbulence. J. Plasma Phys. 2022, 88, 905880602. [Google Scholar] [CrossRef]
- Schekochihin, A.A. MHD turbulence: A biased review. J. Plasma Phys. 2022, 88, 155880501. [Google Scholar] [CrossRef]
- Uchida, F.; Fujiwara, M.; Kamada, K.; Yokoyama, J. New description of the scaling evolution of the cosmological magneto-hydrodynamic system. arXiv 2022, arXiv:2212.14355. [Google Scholar] [CrossRef]
- Sharma, R.; Brandenburg, A. Low frequency tail of gravitational wave spectra from hydromagnetic turbulence. Phys. Rev. D 2022, 106, 103536. [Google Scholar] [CrossRef]
- Brandenburg, A.; Kahniashvili, T. Classes of hydrodynamic and magnetohydrodynamic turbulent decay. Phys. Rev. Lett. 2017, 118, 055102. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Davidson, P.A. Was Loitsyansky correct? A review of the arguments. J. Turbulence 2000, 1, 6. [Google Scholar] [CrossRef]
- Davidson, P.A. The role of angular momentum conservation in homogeneous turbulence. J. Fluid Mech. 2009, 632, 329. [Google Scholar] [CrossRef]
- Brandenburg, A.; Boldyrev, S. The turbulent stress spectrum in the inertial and subinertial ranges. Astrophys. J. 2020, 892, 80. [Google Scholar] [CrossRef]
- Pencil Code Collaboration; Brandenburg, A.; Johansen, A.; Bourdin, P.; Dobler, W.; Lyra, W.; Rheinhardt, M.; Bingert, S.; Haugen, N.; Mee, A.; et al. The Pencil Code, a modular MPI code for partial differential equations and particles: Multipurpose and multiuser-maintained. J. Open Source Softw. 2021, 6, 2807. [Google Scholar] [CrossRef]
- Brandenburg, A.; Kamada, K.; Schober, J. Decay law of magnetic turbulence with helicity balanced by chiral fermions. Phys. Rev. Res. 2023, 5, L022028. [Google Scholar] [CrossRef]
- Cho, J. Magnetic helicity conservation and inverse energy cascade in electron magnetohydrodynamic wave packets. Phys. Rev. Lett. 2011, 106, 191104. [Google Scholar] [CrossRef] [Green Version]
- Goldreich, P.; Reisenegger, A. Magnetic field decay in isolated neutron stars. Astrophys. J. 1992, 395, 250. [Google Scholar] [CrossRef] [Green Version]
- Brandenburg, A. Hosking integral in nonhelical Hall cascade. J. Plasma Phys. 2023, 89, 175890101. [Google Scholar] [CrossRef]
- Brandenburg, A. Hall cascade with fractional magnetic helicity in neutron star crusts. Astrophys. J. 2020, 901, 18. [Google Scholar] [CrossRef]
- Gary, S.P.; Saito, S.; Li, H. Cascade of whistler turbulence: Particle-in-cell simulations. Geophys. Res. Lett. 2008, 35, L02104. [Google Scholar] [CrossRef]
- Sarin, N.; Brandenburg, A.; Haskell, B. Confronting the neutron star population with inverse cascades. arXiv 2023, arXiv:2305.14347. [Google Scholar] [CrossRef]
- Smith, C.W.; Hamilton, K.; Vasquez, B.J.; Leamon, R.J. Dependence of the dissipation range spectrum of interplanetary magnetic fluctuations on the rate of energy cascade. Astrophys. J. Lett. 2006, 645, L85–L88. [Google Scholar] [CrossRef] [Green Version]
- Rogachevskii, I.; Ruchayskiy, O.; Boyarsky, A.; Fröhlich, J.; Kleeorin, N.; Brandenburg, A.; Schober, J. Laminar and turbulent dynamos in chiral magnetohydrodynamics. I. Theory. Astrophys. J. 2017, 846, 153. [Google Scholar] [CrossRef] [Green Version]
- Brandenburg, A.; Kamada, K.; Mukaida, K.; Schmitz, K.; Schober, J. Chiral magnetohydrodynamics with zero total chirality. arXiv 2023, arXiv:2304.06612. [Google Scholar] [CrossRef]
- Plunian, F.; Stepanov, R.; Frick, P. Shell models of magnetohydrodynamic turbulence. Phys. Rep. 2013, 523, 1–60. [Google Scholar] [CrossRef] [Green Version]
- Kadanoff, L.; Lohse, D.; Wang, J.; Benzi, R. Scaling and dissipation in the GOY shell model. Phys. Fluids 1995, 7, 617–629. [Google Scholar] [CrossRef] [Green Version]
- Brandenburg, A.; Enqvist, K.; Olesen, P. Large-scale magnetic fields from hydromagnetic turbulence in the very early universe. Phys. Rev. D 1996, 54, 1291–1300. [Google Scholar] [CrossRef] [Green Version]
- Frick, P.; Sokoloff, D. Cascade and dynamo action in a shell model of magnetohydrodynamic turbulence. Phys. Rev. E 1998, 57, 4155–4164. [Google Scholar] [CrossRef]
- Basu, A.; Sain, A.; Dhar, S.K.; Pandit, R. Multiscaling in models of magnetohydrodynamic turbulence. Phys. Rev. Lett. 1998, 81, 2687–2690. [Google Scholar] [CrossRef] [Green Version]
- Brandenburg, A.; Enqvist, K.; Olesen, P. The effect of Silk damping on primordial magnetic fields. Phys. Lett. B 1997, 392, 395–402. [Google Scholar] [CrossRef]
- Lessinnes, T.; Plunian, F.; Carati, D. Helical shell models for MHD. Theor. Comp. Fluid Dyn. 2009, 23, 439–450. [Google Scholar] [CrossRef]
- Olesen, P. Inverse cascades and primordial magnetic fields. Phys. Lett. B 1997, 398, 321–325. [Google Scholar] [CrossRef] [Green Version]
- Kazantsev, A.P. Enhancement of a magnetic field by a conducting fluid. Sov. J. Exp. Theor. Phys. 1968, 26, 1031. [Google Scholar]

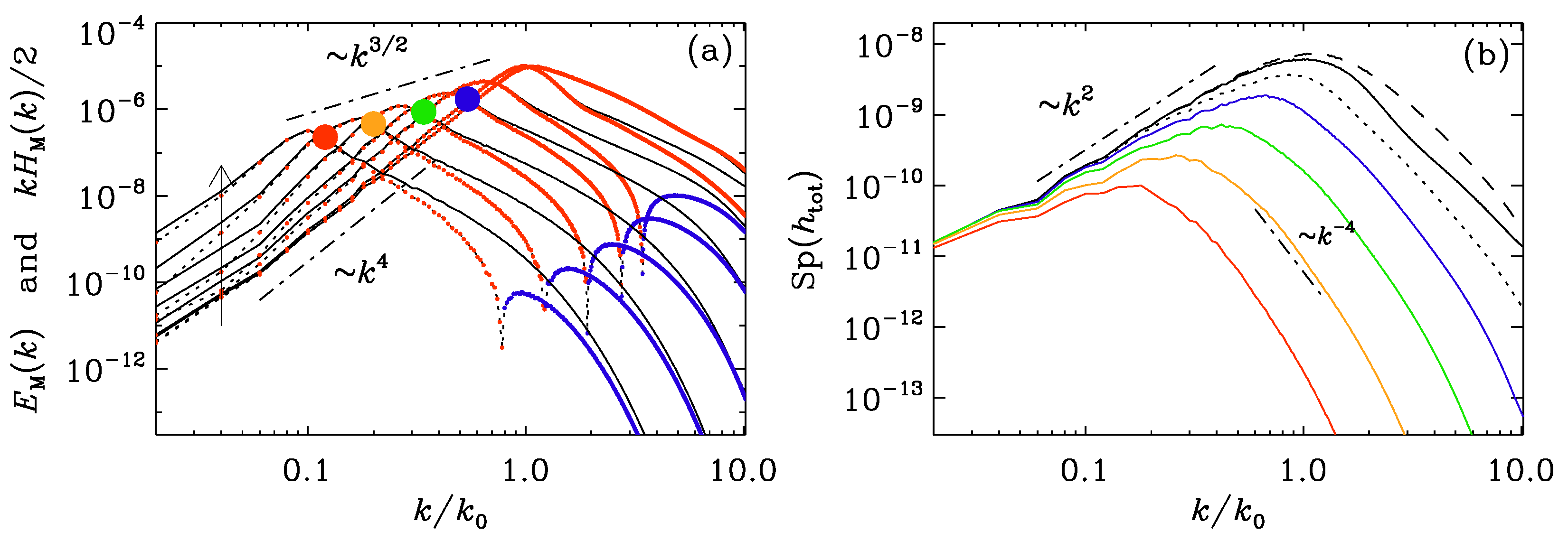

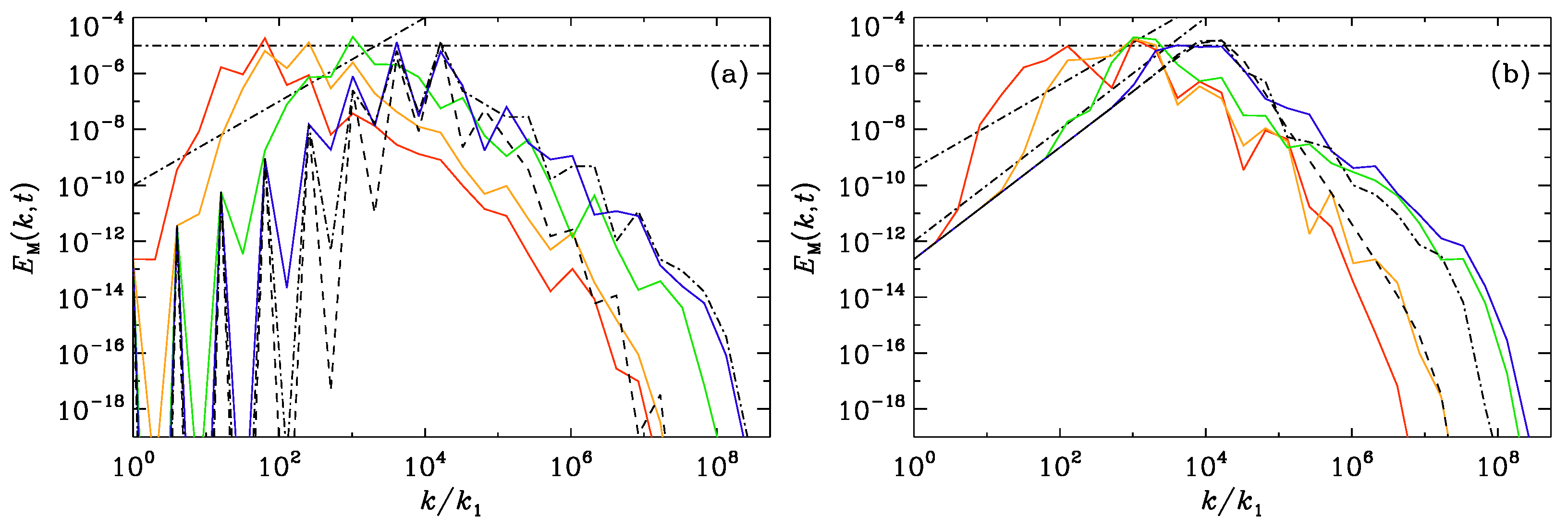
| 2 | 2 | 0.16 | 0.15 | 4.2 | 3.8 | 0.025 | (0.05) |
| 4 | 3/2 | 0.15 | 0.13 | 4.0 | 3.5 | (0.02) | 0.037 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Brandenburg, A.; Larsson, G. Turbulence with Magnetic Helicity That Is Absent on Average. Atmosphere 2023, 14, 932. https://doi.org/10.3390/atmos14060932
Brandenburg A, Larsson G. Turbulence with Magnetic Helicity That Is Absent on Average. Atmosphere. 2023; 14(6):932. https://doi.org/10.3390/atmos14060932
Chicago/Turabian StyleBrandenburg, Axel, and Gustav Larsson. 2023. "Turbulence with Magnetic Helicity That Is Absent on Average" Atmosphere 14, no. 6: 932. https://doi.org/10.3390/atmos14060932
APA StyleBrandenburg, A., & Larsson, G. (2023). Turbulence with Magnetic Helicity That Is Absent on Average. Atmosphere, 14(6), 932. https://doi.org/10.3390/atmos14060932








