Charged Atmospheric Aerosols from Charged Saltating Dust Aggregates
Abstract
:1. Introduction
2. Materials and Methods
3. Results and Discussion
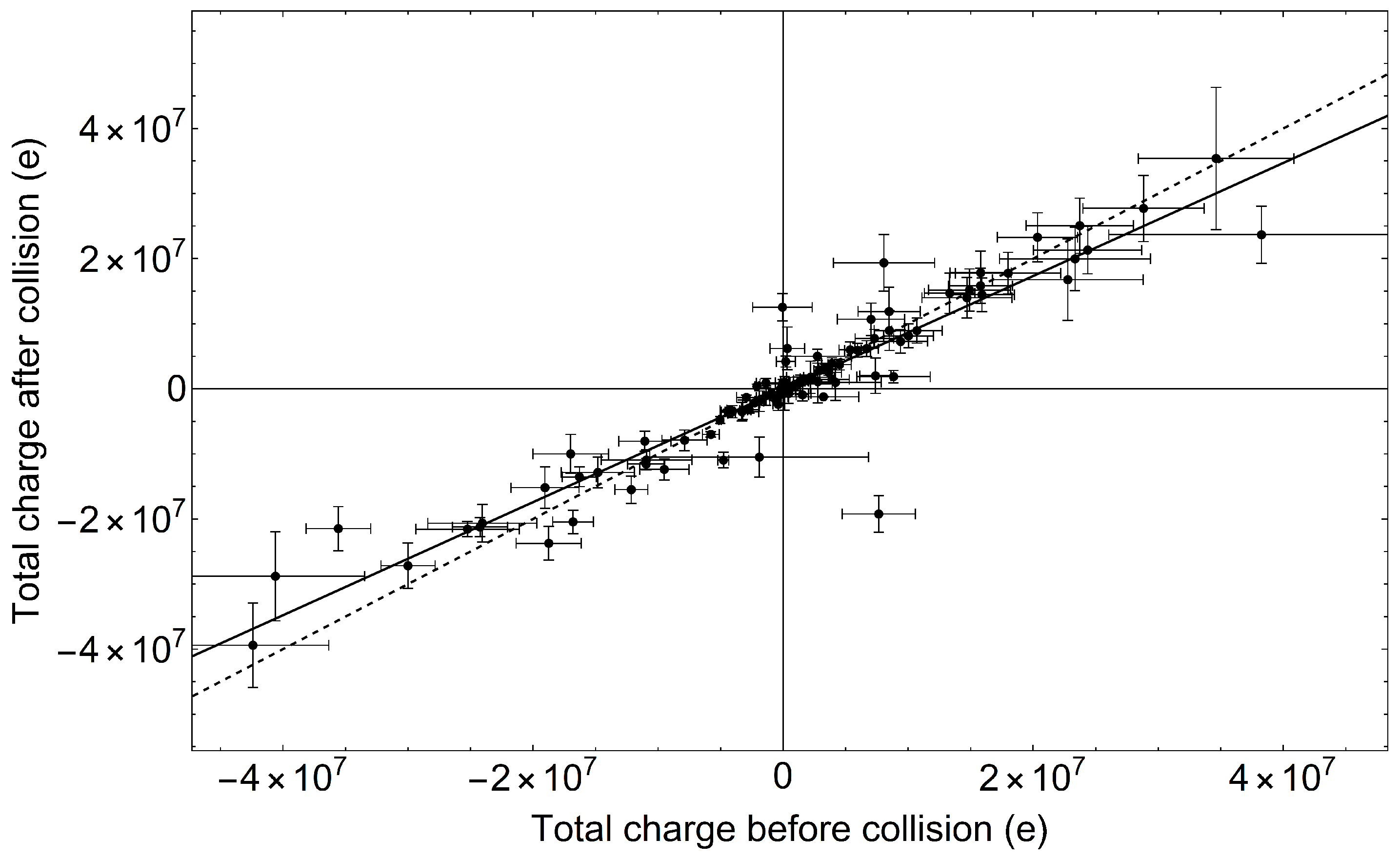
3.1. Collisions of Basalt Beads
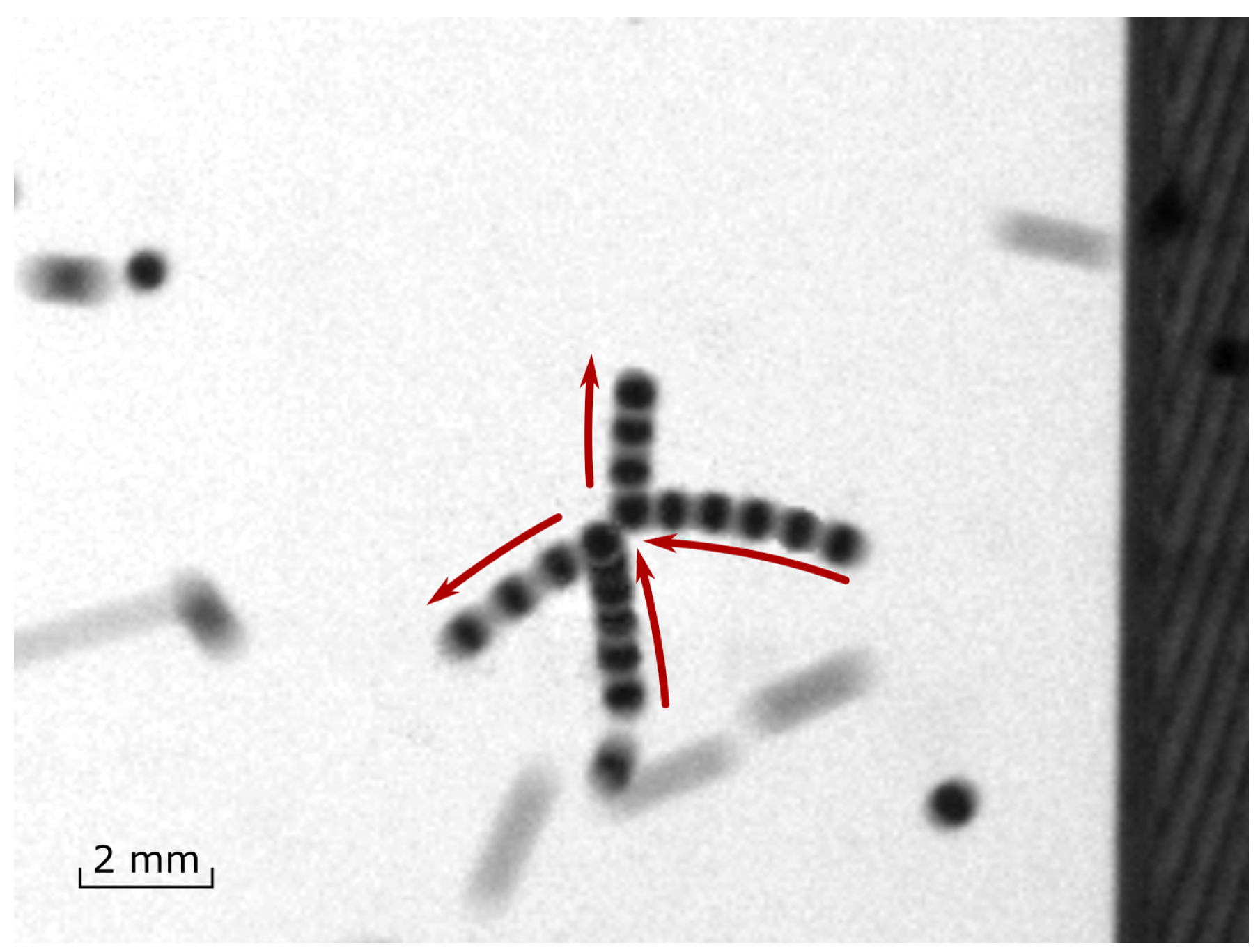
3.2. Collisions of Basalt Dust Aggregates
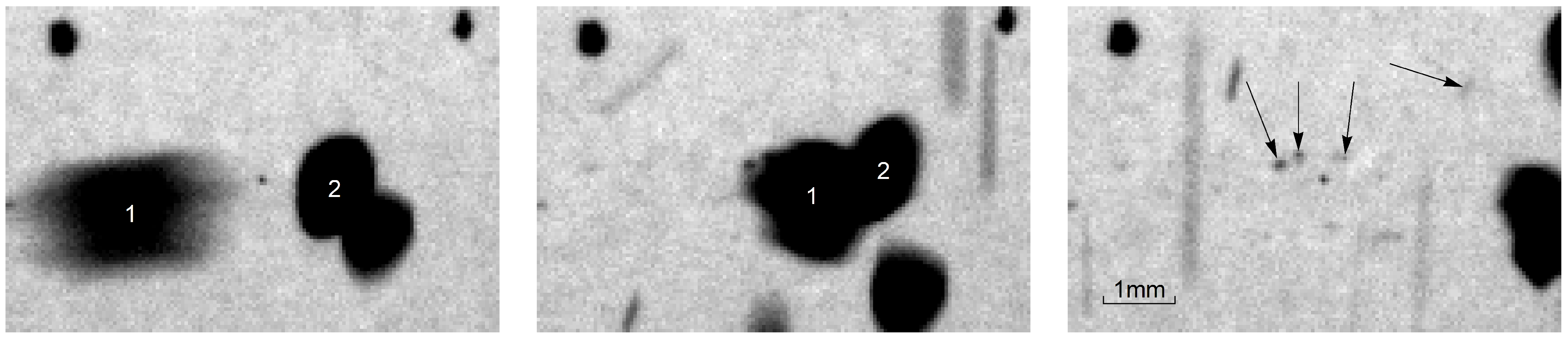
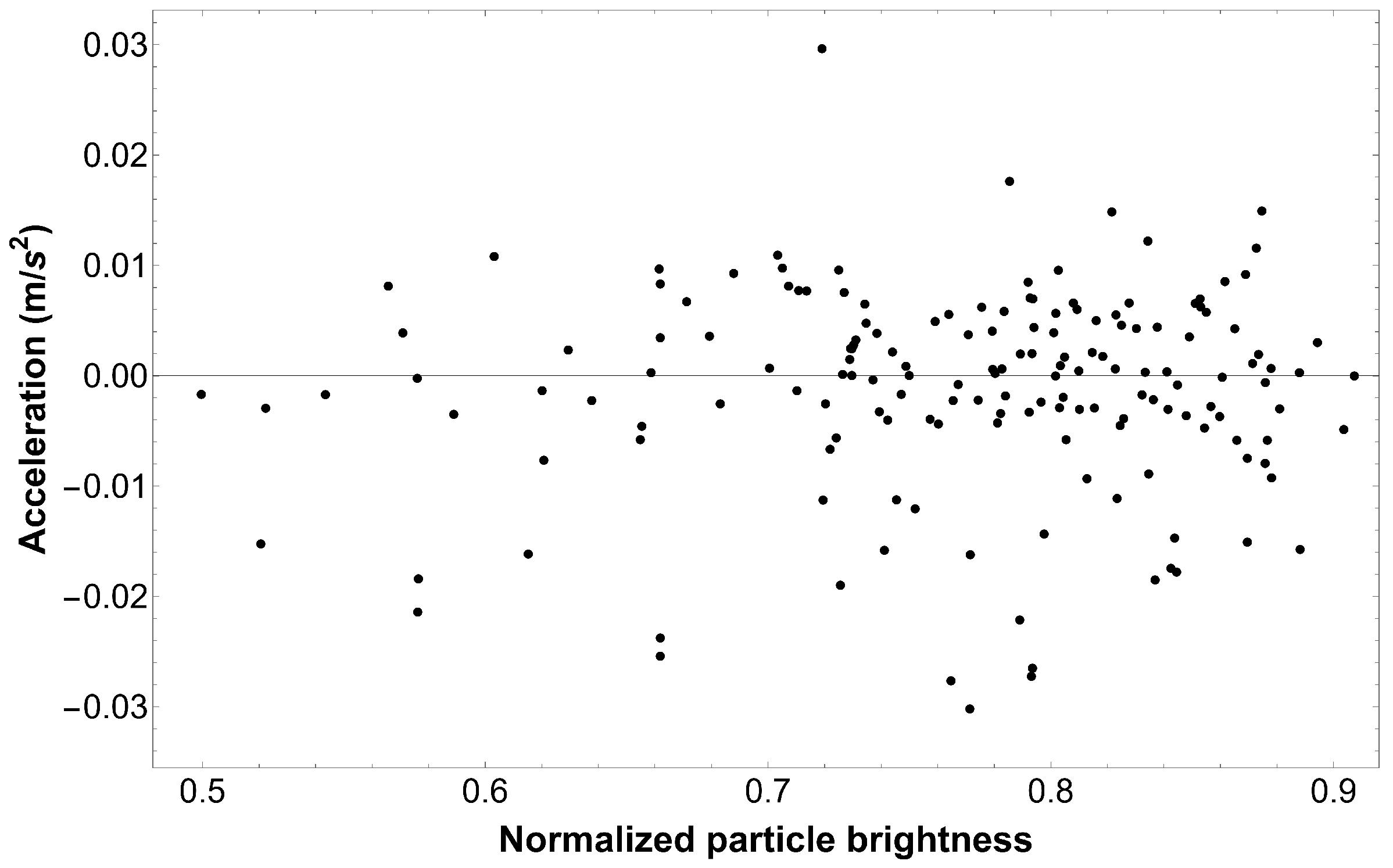
3.3. Loss of Small Grains
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Greeley, R.; Iversen, J.D. Wind as a Geological Process on Earth, Mars, Venus and Titan; Cambridge University Press: New York, NY, USA, 1985. [Google Scholar] [CrossRef]
- Tegen, I.; Lacis, A.A. Modeling of particle size distribution and its influence on the radiative properties of mineral dust aerosol. J. Geophys. Res. Atmos. 1996, 101, 19237–19244. [Google Scholar] [CrossRef] [Green Version]
- Lemmon, M.T.; Guzewich, S.D.; McConnochie, T.; de Vicente-Retortillo, A.; Martínez, G.; Smith, M.D.; Bell, J.F.; Wellington, D.; Jacob, S. Large Dust Aerosol Sizes Seen During the 2018 Martian Global Dust Event by the Curiosity Rover. Geophys. Res. Lett. 2019, 46, 9448–9456. [Google Scholar] [CrossRef]
- Chen-Chen, H.; Pérez-Hoyos, S.; Sánchez-Lavega, A. Dust particle size and optical depth on Mars retrieved by the MSL navigation cameras. Icarus 2019, 319, 43–57. [Google Scholar] [CrossRef] [Green Version]
- Becker, T.; Teiser, J.; Jardiel, T.; Peiteado, M.; Muñoz, O.; Martikainen, J.; Martin, J.C.G.; Wurm, G. Releasing Atmospheric Martian Dust in Sand Grain Impacts. Planet. Sci. J. 2022, 3, 195. [Google Scholar] [CrossRef]
- Waza, A.; Kjer, J.; Peiteado, M.; Jardiel, T.; Iversen, J.; Rasmussen, K.; Merrison, J. Aeolian dust resuspension on Mars studied using a recirculating environmental wind tunnel. Planet. Space Sci. 2023, 227, 105638. [Google Scholar] [CrossRef]
- Waitukaitis, S.R.; Lee, V.; Pierson, J.M.; Forman, S.L.; Jaeger, H.M. Size-Dependent Same-Material Tribocharging in Insulating Grains. Phys. Rev. Lett. 2014, 112, 218001. [Google Scholar] [CrossRef] [Green Version]
- Haeberle, J.; Schella, A.; Sperl, M.; Schröter, M.; Born, P. Double origin of stochastic granular tribocharging. Soft Matter 2018, 14, 4987–4995. [Google Scholar] [CrossRef] [Green Version]
- Lacks, D.J.; Shinbrot, T. Long-standing and unresolved issues in triboelectric charging. Nat. Rev. Chem. 2019, 3, 465–476. [Google Scholar] [CrossRef]
- Harrison, R.G.; Barth, E.; Esposito, F.; Merrison, J.; Montmessin, F.; Aplin, K.L.; Borlina, C.; Berthelier, J.J.; Déprez, G.; Farrell, W.M.; et al. Applications of Electrified Dust and Dust Devil Electrodynamics to Martian Atmospheric Electricity. Space Sci. Rev. 2016, 203, 299–345. [Google Scholar] [CrossRef] [Green Version]
- Cimarelli, C.; Alatorre-Ibarguengoitia, M.A.; Kueppers, U.; Scheu, B.; Dingwell, D.B. Experimental generation of volcanic lightning. Geology 2014, 42, 79–82. [Google Scholar] [CrossRef] [Green Version]
- Schmidt, D.S.; Schmidt, R.A.; Dent, J.D. Electrostatic force on saltating sand. J. Geophys. Res. Atmos. 1998, 103, 8997–9001. [Google Scholar] [CrossRef]
- Zheng, X.J.; Huang, N.; Zhou, Y.H. Laboratory measurement of electrification of wind-blown sands and simulation of its effect on sand saltation movement. J. Geophys. Res. (Atmos.) 2003, 108, 4322. [Google Scholar] [CrossRef]
- Zhang, H.; Zheng, X.J.; Bo, T. Electrification of saltating particles in wind-blown sand: Experiment and theory. J. Geophys. Res. (Atmos.) 2013, 118, 12086–12093. [Google Scholar] [CrossRef]
- Kok, J.F.; Renno, N.O. Enhancement of the emission of mineral dust aerosols by electric forces. Geophys. Res. Lett. 2006, 33, L19S10. [Google Scholar] [CrossRef] [Green Version]
- Esposito, F.; Molinaro, R.; Popa, C.I.; Molfese, C.; Cozzolino, F.; Marty, L.; Taj-Eddine, K.; Di Achille, G.; Franzese, G.; Silvestro, S.; et al. The role of the atmospheric electric field in the dust-lifting process. Geophys. Res. Lett. 2016, 43, 5501–5508. [Google Scholar] [CrossRef] [Green Version]
- Onyeagusi, F.C.; Jungmann, F.; Teiser, J.; Wurm, G. Electrostatic Repulsion of Dust from Planetary Surfaces. Planet. Sci. J. 2023, 4, 13. [Google Scholar] [CrossRef]
- Matsuyama, T. A discussion on maximum charge held by a single particle due to gas discharge limitation. In Proceedings of the 1st International Conference and Exhibition on Powder Technology Indonesia (ICePTi) 2017, Jatinangor, Indonesia, 8–9 August 2017; American Institute of Physics Conference Series. 2018; Volume 1927, p. 020001. [Google Scholar] [CrossRef]
- Wurm, G.; Schmidt, L.; Steinpilz, T.; Boden, L.; Teiser, J. A challenge for Martian lightning: Limits of collisional charging at low pressure. Icarus 2019, 331, 103–109. [Google Scholar] [CrossRef] [Green Version]
- Schoenau, L.; Steinpilz, T.; Teiser, J.; Wurm, G. Corona discharge of a vibrated insulating box with granular medium. Granul. Matter 2021, 23, 1–6. [Google Scholar] [CrossRef]
- Jungmann, F.; van Unen, H.; Teiser, J.; Wurm, G. Violation of triboelectric charge conservation on colliding particles. Phys. Rev. E 2021, 104, L022601. [Google Scholar] [CrossRef]
- Schindelin, J.; Arganda-Carreras, I.; Frise, E.; Kaynig, V.; Longair, M.; Pietzsch, T.; Preibisch, S.; Rueden, C.; Saalfeld, S.; Schmid, B.; et al. Fiji: An open-source platform for biological-image analysis. Nat. Methods 2012, 9, 676–682. [Google Scholar] [CrossRef] [Green Version]
- McCarty, L.S.; Winkleman, A.; Whitesides, G.M. Ionic Electrets: Electrostatic Charging of Surfaces by Transferring Mobile Ions upon Contact. J. Am. Chem. Soc. 2007, 129, 4075–4088. [Google Scholar] [CrossRef]
- Lee, V.; James, N.M.; Waitukaitis, S.R.; Jaeger, H.M. Collisional charging of individual submillimeter particles: Using ultrasonic levitation to initiate and track charge transfer. Phys. Rev. Mater. 2018, 2, 035602. [Google Scholar] [CrossRef]
- Harris, I.A.; Lim, M.X.; Jaeger, H.M. Temperature dependence of nylon and PTFE triboelectrification. Phys. Rev. Mater. 2019, 3, 085603. [Google Scholar] [CrossRef] [Green Version]
- Jungmann, F.; Onyeagusi, F.C.; Teiser, J.; Wurm, G. Charge transfer of pre-charged dielectric grains impacting electrodes in strong electric fields. J. Electrost. 2022, 117, 103705. [Google Scholar] [CrossRef]
- Steinpilz, T.; Jungmann, F.; Joeris, K.; Teiser, J.; Wurm, G. Measurements of dipole moments and a Q-patch model of collisionally charged grains. New J. Phys. 2020, 22, 093025. [Google Scholar] [CrossRef]




Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Onyeagusi, F.C.; Meyer, C.; Teiser, J.; Becker, T.; Wurm, G. Charged Atmospheric Aerosols from Charged Saltating Dust Aggregates. Atmosphere 2023, 14, 1065. https://doi.org/10.3390/atmos14071065
Onyeagusi FC, Meyer C, Teiser J, Becker T, Wurm G. Charged Atmospheric Aerosols from Charged Saltating Dust Aggregates. Atmosphere. 2023; 14(7):1065. https://doi.org/10.3390/atmos14071065
Chicago/Turabian StyleOnyeagusi, F. Chioma, Christian Meyer, Jens Teiser, Tim Becker, and Gerhard Wurm. 2023. "Charged Atmospheric Aerosols from Charged Saltating Dust Aggregates" Atmosphere 14, no. 7: 1065. https://doi.org/10.3390/atmos14071065
APA StyleOnyeagusi, F. C., Meyer, C., Teiser, J., Becker, T., & Wurm, G. (2023). Charged Atmospheric Aerosols from Charged Saltating Dust Aggregates. Atmosphere, 14(7), 1065. https://doi.org/10.3390/atmos14071065






