Equivalent Small Hydro Power: A Simple Method to Evaluate Energy Production by Small Turbines in Collective Irrigation Systems

Abstract

The exploitation of water flows in collective irrigation networks is promising in view of enhancing renewable energy production in agriculture. To this goal, a simplified method to estimate the electricity production of small hydro power (SHP) plants integrated in existing irrigation systems is proposed. This method schematizes the water network by an “equivalent” system, consisting of a single pipeline with homogeneous diameter and material. The proposed method only requires as input data the altimetry and the maps of the irrigated areas instead of the materials and diameters of all the conduits of a common water network (often unknown by irrigation managers). The feasibility of the proposed method has been verified to size SHP plants in seven collective irrigation systems of Calabria (Southern Italy). This application has highlighted a mean error of 20% in estimating the SHP power with a more detailed model, previously developed by the same authors and verified in the same context; these estimates are more accurate for SHP plants not exceeding 150–175 kW of electrical power. These results suggest the applicability of the proposed method for feasibility studies or large-scale projects of small SHP plants.

1. Introduction

Hydropower currently represents, worldwide, a significant source of electrical energy [1]. It contributes to one-fifth of the total power worldwide and it is the only domestic source for electricity generation in many countries [2,3]. Moreover, from the environmental point of view, hydropower is more sustainable compared to the other hydroelectrical energy production systems. It avoids the heavy impacts of large dams on the natural ecosystem [4] and, more generally, the constraints of human-controlled flow regulation works, affecting freshwater conditions [5].

The use of small hydro power (SHP) plants—each part of a hydroelectric plant containing a hydraulic turbine with a nominal power of less than 10 MW [6,7]—is also an optimal solution for rural electrification. Thanks to simple technology and low installation and management costs [8], SHP adoption has been suggested in marginal areas with difficult accessibility, where public electrical networks are often absent. These areas are often devoted to agricultural activities and served by collective irrigation systems. These water networks generally consist of a water diversion structure, dominating the irrigated areas, and pressured pipelines for water distribution. The potential energy deriving from the hydraulic heads and water flows of the irrigation network can be exploited for electrical energy production by SHP plants [9]. However, despite its economic convenience and environmental sustainability, this opportunity is often neglected and, thus, the SHP potential energy is practically wasted [10]. However, the analysis of the potential recovered energy has not been performed deeply yet. Preliminary studies were made [11], but these studies have calculated the theoretical recovered energy in some pipe branches of a network (i.e., in line, in hydrant or in irrigation point) [12], thus, without a thorough energy analysis at water network level.

The net electrical power (henceforth indicated as “P_n”) is the most important parameter to evaluate in feasibility projects of SHP plants [8]. Thus, reliable calculation methods of P_n, starting from input parameters of the water network, are needed to assess the technical and economical convenience of the SHP plant installation in existing collective irrigation networks, at least in the preliminary design stages before the executive planning and implementation [13]. As reviewed in the paper of Perez-Sanchez et al. [14], the existing literature reports several methods to evaluate the best SHP plant configuration to adopt from the technical and economic points of view [15,16,17,18,19,20]. However, these methods are mainly suitable for SHP integration in common water systems (e.g., aqueducts for civil and industrial uses), whose hydraulic schemes and functioning can be different from collective irrigation systems [9,12]. Most of these works have focused on urban water supply networks and not on the irrigation sector, which usually has higher fluctuations in water demand, as well as different seasonal and daily demand patterns [21]. As regards the energy recovery in irrigation networks, Pérez-Sánchez et al. [12] have proposed a methodology to estimate the energy dissipated by friction losses, the energy required for irrigation, and the recoverable energy based on the variation of flow following the random demand of the users and the real irrigation allocations. The same authors have developed an optimization strategy for increasing the energy efficiency in pressurized irrigation networks by energy recovering, considering different objective functions including feasibility index [22].

However, the literature methods seem to be more useful for detailed installation projects rather than for a preliminary feasibility assessment, because they require many input variables, whose determination may be difficult and time consuming at the preliminary design stage. Even though the models adopted to analyze energy systems have become in the years more and more complex and accurate, the same attention has not always been paid to the model input data [9]. As regards the simplest methods specifically targeted to integrate SHP plants in irrigation networks, we cite the previous study of Zema et al. [23] who have recently proposed an original method allowing sizing and positioning SHP plants in pressured irrigation network and the related analysis of economic profitability.

In many irrigation districts, served by collective systems, most of the input data needed by the SHP plant design methods—that is, the morphological and hydraulic parameters of the water networks—are often not available and/or their measurements are not possible (e.g., diameters and materials of the underground conduits). We hypothesized that some of these collective irrigation systems (often having a complicated layout) can be schematized by more simple water networks, consisting only of a diversion structure, a main pipeline—with homogenous diameter and material—and an outlet; for these water networks, the irrigated areas (always known by the managers of the water user associations) can be adopted as input parameter of the original method proposed by Zema et al. [23]—hereinafter “original method”(OM) —in replacement of the different diameters and materials of the actual water network.

This study evaluates the accuracy of SHP power estimates provided by applying the simplified methodology (hereinafter “simplified method” (SM), called “Equivalent Small Hydro Power”) to seven collective irrigation systems selected in water user associations (WUAs) of Calabria (Southern Italy), adopted as case studies. If the adoption of few morphological and hydraulic parameters of the water network leads to comparable results, a reliable, specific, but simpler, design tool can be made available for small turbine installation in collective irrigation networks. When a significant number of irrigation systems (no less than 5–8), in which the irrigated areas and the corresponding diameters of the individual pipelines are known, a regression equation can be used to estimate P_n of the turbines of the Equivalent Small Hydro Power (ESHP) network form the irrigated areas.

2. Material and Methods

2.1. Outlines on the Original Method (OM)

The complete description of the OM to evaluate the technical and economic feasibility of SHP in existing irrigation systems is reported in the cited paper of Zema et al. [23]. Here, we summarize the main design steps.

First, the mapping of the water network of the collective irrigation system allows the definition of layout and longitudinal profile and identification of supply and distribution lines and nodes. The difference in height in the nodes is taken as the gross hydraulic heads.

Then, the possibility of adjustments in the existing water network is examined to exploit as much as possible the available potential energy and/or to reduce the installation costs (e.g., replacing turbines of small size, discarding turbines of the smallest sizes, increasing sections of supplying lines).

Finally, the hydraulic calculations of the water network, having gross hydraulic head (ΔH_g), and pipeline diameter, and length (D and L), respectively, provides P_n of the SHP plant (whose efficiency is η) and the corresponding Q* (see list of abbreviations), as follows.

Previously, the hydraulic gradient J is calculated by a common monomial equation, such as Hazen-Williams’ formula:

J = kQ^αD⁻ⁿ,

(1)

where D is the pipeline internal diameter, α = 1.852, n = 4.870, and k = (10.675 × C^−α). The latter is a roughness coefficient, which depends on the pipeline material.

P_n and Q* can be calculated by the Equations (2) and (3), respectively:

P_n = ηγQ(ΔH_g − kQ^αD⁻ⁿL),

(2)

Q* = [(ΔH_gDⁿ)/(1 + α)kL] ^1/α,

(3)

where Q* is the water discharge maximizing the SHP power.

Since collective irrigation systems are long water networks (that is, L/D > 1000), in Equations (2) and (3) local hydraulic head losses are ignored.

Discarding pipelines with P_n lower than a certain threshold is generally advisable (e.g., 5 kW, Zema et al. [23]), because of their low economic viability.

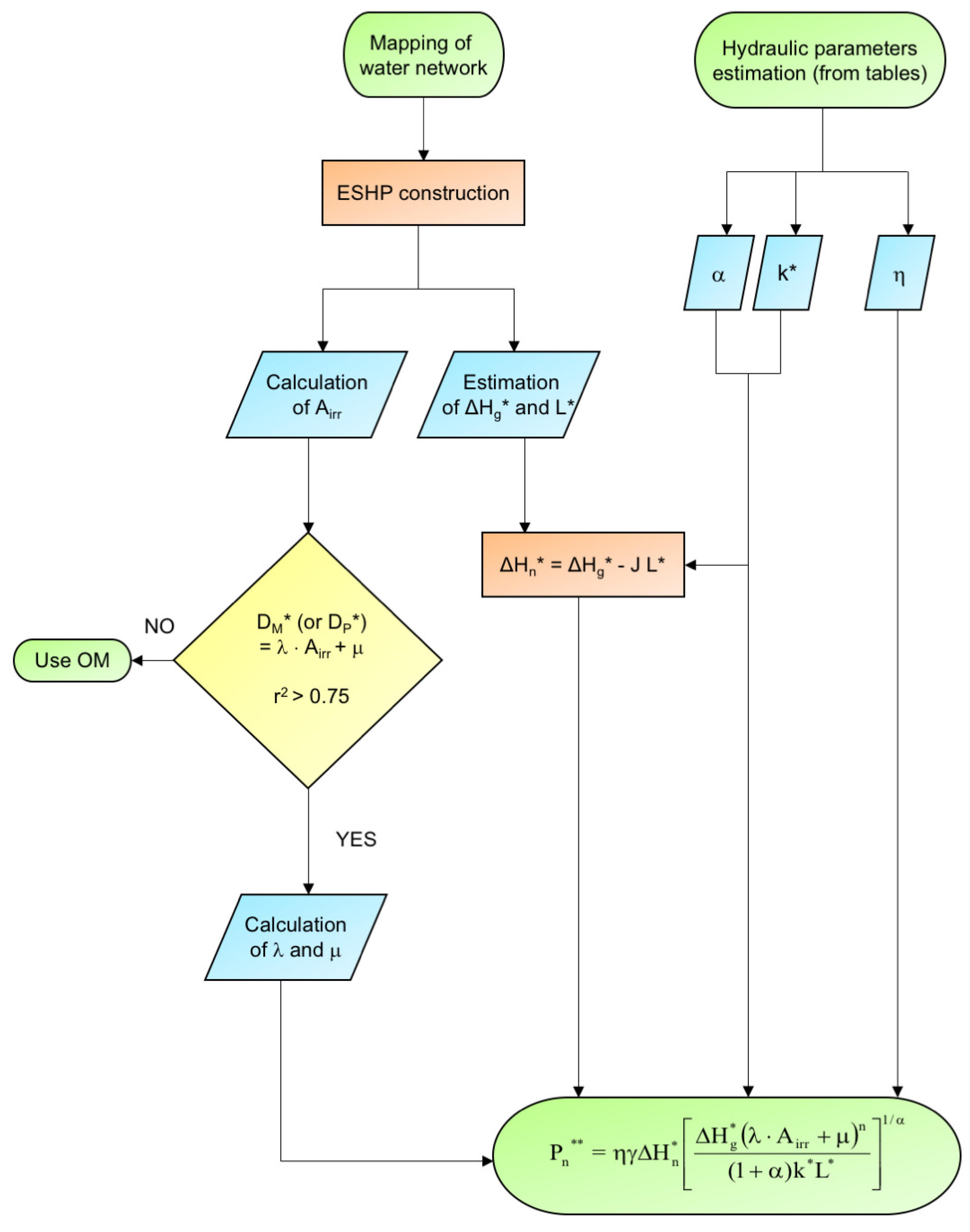
2.2. Description of the Simplified Method (SM)

In SM the actual water network is replaced by an “equivalent” SHP (equivalent small hydro power, henceforth “ESHP”) system, which is simpler to calculate. The ESHP system consists of:

• − A single pressured pipeline with constant diameter (D*) and roughness coefficient (k*);
• − A single turbine (SHP).

The basic hypothesis of SM is that the integration of the single SHP plant into the single pipeline of the ESHP system provides an “equivalent” electrical power, that is, equal to P_n estimated using OM.

To calculate k* of ESHP pipeline, two options can be adopted:

a)

k* (henceforth k_P) equal to k of the material with prevalent length of the actual water network;

b)

k* (henceforth k_M) equal to the arithmetic mean of the roughness coefficients (k) of all materials of the actual water network.

Two new parameters are introduced for ESHP:

• − L*, equal to the total length of the main pipelines of the water network (that is, the feeders);
• − ΔH_g* (gross hydraulic head), estimated as the difference between the altitudes of the water supply point (reservoir or river section) and the most depressed point of the irrigated areas.

Hereinafter, D_P* indicates the ESHP diameter estimated for k* = k_P, while D_M* is the ESHP diameter for k* = k_M.

Replacing ΔH_g*, L* and D_P* or D_M* in Equation (2), Q** (that is, the optimal discharge of the ESHP network) is obtained as follows:

Q** = [(ΔH_g*D*ⁿ)/(1 + α)k*L*]^1/α.

(4)

Again, replacing these parameters in Equation (2) P_n of the ESHP can be estimated:

P_n* = ηγΔH_n*[(ΔH_g*D*ⁿ)/(1 + α)k*L*]^1/α.

(5)

In Equation (5) ΔH_n* is the difference between ΔH_g* and the distributed head losses (JL), and J is calculated by Equation (3), being J = f(α, k*). In Equation (5) P_n* is a function of ΔH_g*, L*, α, k*, n, and D* only, where the first five parameters are known, but not D*.

Finally, if P_n* (net electrical power of ESHP system) is equaled to P_n (calculated by OM for the actual SHP system), then it is possible to calculate D_P* or D_M* by Equation (5), using, for example, a non-linear optimization algorithm, such as the objective function of Microsoft^® Excel^®.

As mentioned above, often (D) of a single pipeline of the actual water network is not known. Therefore, generally the higher D, the higher Q delivered to the served irrigated area (A_irr), which leads to suppose the existence of a correlation between D_P* (or D_M*) and A_irr (which is, instead, generally known by WUA managers). If this correlation exists and is significant (for instance, hypothesizing a linear regression with r² > 0.75), then it is possible to estimate D** of the ESHP (that is, the pipeline internal diameter of the ESHP network) only from A_irr. Therefore, P_n** (the net electrical power of the turbines of the ESHP network, estimated by the SM) can easily be calculated:

P_n* = ηγΔH_n*[(ΔH_g*(λ⋅A_irr + μ)ⁿ(/(1 + α)k*L*]^1/α,

(6)

where λ and μ are the slope and intercept of a linear correlation between D_M* or D_P* (dependent variable) and A_irr (independent variable):

D_M* (or D_P*) = λ⋅A_irr + μ.

(7)

Of course, this correlation should result from a significant number of irrigation systems (no less than 5–8), in which the irrigated areas and the corresponding diameters of the individual pipelines are known.

A flow chart of the SM evaluating SHP energy production in collective irrigation systems is shown in Figure 1.

2.3. SM Verification in Seven Case Studies of Calabria (Southern Italy)

In Calabria (Southern Italy) collective irrigation is mainly managed by water user associations (WUAs), which operate several water networks [24,25,26]. Under a morphological and hydrological point of view, Calabria shows many suitable sites (water bodies at high altitude with significant supplied water volumes) for SHP plant installation in the existing collective irrigation systems [27]. Therefore, in this region the energy potential from SHP plants could be exploited at sustainable costs and the annual income of these organizations or associates could be integrated with additional profits [10].

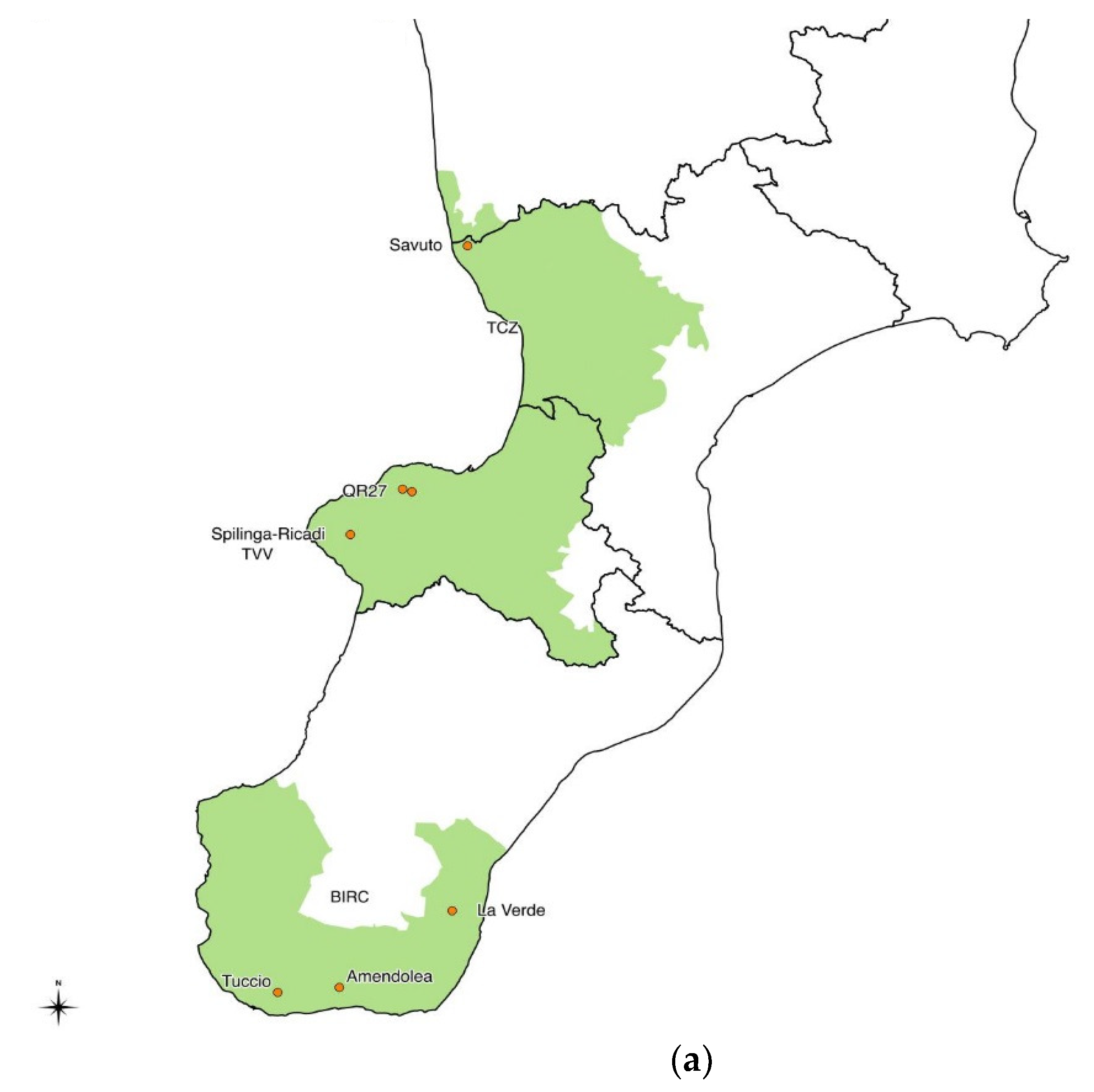
2.3.1. Study Area

The SM and OM were applied in seven collective irrigation systems located in three WUAs of Calabria: “Spilinga-Ricadi”, “Murria” and “QR27” (WUA “Tirreno Vibonese”—TVV; “La Verde”, “Amendolea” and “Tuccio” (WUA “Basso Ionio Reggino”—BIRC; “Savuto” (WUA “Tirreno Catanzarese—TCZ) (Figure 2).

In the seven irrigation systems, high river discharge occurs outside of the irrigation season (from May to September). The WUA wants to exploit the energy production capacity of the existing geodetic heads through SHP system.

2.3.2. Analysis of the Collective Irrigation Systems and SHP Plants

For each of the seven collective irrigation systems, the map and longitudinal profile of the water networks were schematized by a 1:2000 map, drawn from the last available aerial view (2016). Field surveys, operated on a longitudinal 100-m step, and an analysis of water network design provided the characteristics of the existing pipelines (namely lengths, materials, and diameters). The irrigable areas of each irrigation system were identified by subtracting the urban areas from the areas equipped by the water network and thus potentially served by collective irrigation.

Based on the OM, the turbines were located in the irrigation systems using some of the water network adjustments proposed by Zema et al. [23]. Then, by hydraulic calculations, the (Q*) and the (P_n) of the turbines were estimated in the SHP plants. In the calculations, the value of 0.85 was taken for the turbine efficiency (η) in Equation (2), according to the optimal values suggested by Dragu et al. [6] and Paish [7]. Being L/D > 1000, concentrated head losses have been ignored; thus, the net hydraulic head (ΔH_n) was simply the difference between ΔH and the continuous losses (JL).

Table 1. Coefficients of Hazen-Williams’ formula used in the methods for evaluating SHP energy production in seven irrigation systems of Calabria (Southern Italy).

Coefficient	Pipeline material
	Concrete or Asbestos-Concrete	Steel	Cast Iron	Plastic (HDPE, PVC)
C	100	120	130	150
kP 1	0.00211	0.00151	0.0013	0.00099
kM 2	0.00148	0.00148	0.00148	0.00148

¹ k_P = k of the prevalent material; ² k_M = k averaged among the different materials.

reports the coefficients of Hazen-Williams’ Equation (3) used to calculate J in the analyzed water networks.

3. Results and Discussions

3.1. Analysis of the Seven Irrigation Systems and Calculation of the SHP Potential by the OM

About the “Spilinga-Ricadi” irrigation system, a preliminary analysis of the water network evidenced a more complex configuration compared to the other analyzed systems, due to its intrinsic morphology of the irrigable areas. As a matter of fact, in the irrigation system “Spilinga-Ricadi” water is supplied from several sources, individually feeding different parts of the water network, which can be practically considered as independent each other. Therefore, for the subsequent analysis this water network was split in three sub-systems (Spilinga I, Spilinga II, and Spilinga III).

Table 2. Hydraulic parameters and turbine electrical power calculated by the original method for evaluating SHP energy production in seven irrigation systems of Calabria (Southern Italy).

Irrigation System	Pipeline Characteristics		Airr (ha)	Number of Turbines	ΔHg (m)	L (m)	Q* (L/s)	Pn (kW)	Total Pn (kW)
	Material	D (mm)
Spilinga-Ricadi	Steel	80, 125, 150, 200, 600	463	7	57–145	844–2892	31–64	10–47	171
	Plastic (PVC)	200, 250, 315
Murria	Steel	250, 300, 400, 450	282	4	21–102	2337–2786	165–280	29–122	206
	Plastic (HDPE)	200, 280, 315
	Plastic (PVC)	250
QR27	Steel	250, 300, 400	393	4	18–118	1555–2227	334	33–82	200
	Concrete	600
	Plastic (PVC)	250
La Verde	Steel	350	463	7	47–57	622–1515	756	180–181	365
	Concrete	400
	Asbestos-concrete	200, 225, 350, 400
	Cast iron	600
	Plastic (HDPE)	110, 140
	Plastic (PVC)	200, 250, 315
Amendolea	Steel	100, 250, 300	642	4	24–66	1549– 3276	51–619	7–95	164
	Asbestos-concrete	175, 300, 350, 400
	Cast iron	600
	Plastic (HDPE)	110, 140, 160, 280, 315
Tuccio	Concrete	400	725	4	13–196	927–4054	161–235	25–64	207
Savuto	Steel	600	975	3	18–35	674–4308	291–427	28–70	168
	Concrete	600

^{Note: see the List of abbreviations for symbol meaning.}

reports the main hydraulic parameters of the analyzed irrigation systems. It can be noticed that all the water networks were not homogeneous in terms of pipeline materials and mainly diameters (up to nine sections for “La Verde” system, respectively) (Table 2).

Based on output data of the water network analysis and hydraulic calculations, three (“Savuto” system) to seven (“Spilinga-Ricadi” and “La Verde” systems) turbines were sized and located. According to the smalls hydro association classification, ΔH_g was low (18 m, “QR27” and “Savuto” systems) to high (196 m, “Tuccio” system). The pipeline length varied from 622 (“La Verde” system) to 4308 m (“Savuto”) (Table 2).

The OM implementation allowed the estimation of the optimal discharge Q* (from 31, “Spilinga-Ricadi” system, to 756, “La Verde”, L/s) as well as P_n of the individual turbines (from 7, “Amendolea” system, to 181 kW, “La Verde”). Overall, the total P_n was in the range 164–365 kW (for “Amendolea” and “La Verde” systems, respectively) (Table 2).

3.2. Schematization and Calculation of the ESHP by the SM

Subsequently, the SHP plants, planned by the OM, were schematized using the SM in as many ESHP systems, whose main hydraulic parameters are reported in

Table 3. Hydraulic parameters calculated by the simplified method for evaluating SHP energy production in seven irrigation systems of Calabria (Southern Italy).

ESHP		Hydraulic Parameters
		ΔHg* (m)	L* (m)	JL* (m)	ΔHn* (m)	kP (-)	DP* (mm)	kM (-)	DM* (mm)	Q** (L/s)
Spilinga	I	240	9763	84.2	156	0.001	211	0.0015	229	54
	II	222	5859	77.8	144	0.001	199	0.0015	215	58
	III	312	7961	109.4	203	0.001	162	0.0015	176	34
Murria		224	12,042	78.5	145	0.002	377	0.0015	376	170
QR27		246	7417	86.3	160	0.001	294	0.0015	319	150
La Verde		176	22,000	61.7	114	0.001	559	0.0015	606	379
Amendolea		155	23,695	54.3	101	0.001	453	0.0015	491	195
Tuccio		215	12,752	75.4	140	0.002	419	0.0015	390	177
Savuto		85	15,635	29.8	55	0.002	649	0.0015	646	364

Note: see the Table of abbreviations for symbol meaning.

. As it can be noticed, the SM replaced the SHP plants with equivalent water networks with higher ΔH_g* (up to 246 m, “QR27” system) and L* (up to about 24 km, “Amendolea” system). Figure 3 reports the comparison between the SHP and the ESHP plants in the sample irrigation system “QR27”.

3.3. Replacement of D of the SHP Plants with D_P*/D_M* in the ESHP Plants

By using the two hypothesized values of the Hazen-Williams roughness coefficient (that is, k_P and k_M) separately in Equation (5) and equaling the potential P_n of both SHP and ESHP systems, the (D_P* and D_M*) were estimated. These diameters were very similar for two SHP systems (“Murria” and “Savuto”); for the other systems, the D_P* and D_M* calculated considering k* = k_P were 7–8% lower than in the case k* = k_M (Table 3). Equation (4) provided the estimation of the optimal discharge Q** of the ESHP, ranging from 34 (“Spilinga III” sub-system) to 379 (“La Verde”) L/s.

3.4. Analysis of Differences in Hydraulic Parameters of the Water Networks Between OM and SM

The differences between the net hydraulic head (ΔH_n and ΔH_n*) calculated by SM and OM were lower than 20% for seven out of the nine SHP plants analyzed; only for “La Verde” system this difference was higher than 50%. The pipeline lengths (L and L*) estimated by the SM were much more different from the corresponding values calculated by the OM (on average +170% with a maximum of +930% for “La Verde” system (

Table 4. Comparison of the net hydraulic head (ΔH_n and ΔH_n*) and pipeline length (L and L*) given by the original (OM) and simplified (SM) methods, respectively, for evaluating SHP energy production in seven irrigation systems of Calabria (Southern Italy).

ESHP		ΔHn vs. ΔHn*			L vs. L*
		OM (m)	SM (m)	Difference (%)	OM (m)	SM (m)	Difference (%)
Spilinga	I	140	156	−0.2	4942	9763	−48.2
	II	158	144	0.6	4687	5859	−11.7
	III	174	203	16.7	2655	7961	−53.1
Murria		205	145	0.6	10,449	12,042	−15.9
QR27		156	160	−0.0	7770	7417	3.5
La Verde		73	114	−0.4	2137	22,000	−198.6
Amendolea		105	101	0.0	9461	23,695	−142.3
Tuccio		130	140	−0.1	9028	12,752	−37.2
Savuto		54	55	0.0	9776	15,635	−58.6

). Moreover, the differences ΔH_n vs. ΔH_n* and L vs. L* were well correlated each other (r² = 0.75, p < 0.05 by t-test). Of course, these errors in ESHP hydraulic parameters estimation were reflected in the accuracy of P_n calculation, as it will be detailed in Section 3.6.

3.5. Analysis of the Correlations between D_P*/D_M* and A_irr in the ESHP Plants

Plotting D_P* or D_M* against A_irr of ESHP in each irrigation system (Figure 4), the following linear regression equations were obtained (the related coefficients of determination are reported in brackets):

D_P* = 0.540 A_irr + 126.75    (r² = 0.88, p < 0.05),

(8)

and:

D_M* = 0.530 A_irr + 145.04    (r² = 0.84, p < 0.05),

(9)

In both cases, the dependent variable (A_irr) was strongly correlated to the independent variable (D_P* or D_M*, respectively). The highest accuracy was achieved when k_P was adopted as k* coefficient of Hazen-Williams’ equation of the ESHP network (r² = 0.88, p < 0.05, against a value of 0.84, p < 0.05, in the correlation D_M*–A_irr). Moreover, if two of the analyzed ESHP systems (“La Verde” and “Tuccio”) were excluded from the interpolation, both coefficients of determination even increased to 0.94. The accuracy of these interpolation equations leads to think that in the majority of ESHP plants the equivalent pipeline diameter can be simply estimated by the irrigated area of the system.

3.6. Analysis of the Reliability of Turbine Power Estimates by SM

As mentioned in Section 2, the correlations A_irr–D_P* and A_irr–D_M* allowed the estimation of the pipeline diameter (D**) of the ESHP plant and, based on these estimates, of its electrical power (P_n**).

The comparison between the results of the two estimation methods (OM and SM) confirmed that the adoption k = k_P* for calculating the equivalent diameter (D** as a function of k_P*) was the most appropriate choice. In this case the average error in estimating the ESHP power (P_n**) was about 18% compared to the parameter estimation provided by the OM and the maximum inaccuracy was lower than 50% (“Spilinga III” system). For three ESHP plants estimates of P_n** were practically equal to those produced by the OM (“Spinga II”, “Murria” and “Savuto” systems) (

Table 5. Differences between the original and simplified methods OM and SM in estimating the net electrical power (P_n) of SHP plants in seven irrigation systems of Calabria (Southern Italy).

SHP/ESHP Plant		Pn (by OM) (kW)	k = kP* 1			k = kM* 2
			D** (mm)	Pn** (by SM) (kW)	Difference Pn**, Pn (%)	D** (mm)	Pn** (by SM) (kW)	Difference Pn**, Pn (%)
Spilinga	I	70.0	243	100.8	43.9	259	96.5	37.8
	II	69.5	199	69.6	0.1	216	69.8	0.4
	III	58.1	189	87.0	49.8	206	88.4	52.2
Murria		95.0	279	93.3	−1.8	295	108.6	14.4
QR27		200.4	339	291.7	45.5	353	263.1	31.3
La Verde		361.6	467	224.9	−37.8	479	194.4	−46.2
Amendolea		163.7	473	184.3	12.6	485	159.1	−2.8
Tuccio		206.5	482	297.4	44.0	494	384.3	86.1
Savuto		163.7	653	170.3	1.7	662	178.2	8.9
Mean					17.6			20.2

¹ k_P = k of the prevalent material; ² k_M = k averaged among the different materials.

).

In the other case (k* = k_M) this mean error in P_n** estimation was slightly higher (20%), while only in two SHP systems (“Tuccio” and “Spilinga III”) the overestimation is over 50% (Table 5).

The SM was less accurate in estimating P_n** for “Tuccio”, “QR27”, “Spilinga I” and “Spilinga III” irrigation systems (errors higher than 40% compared to the estimates by the OM). However, it should be noticed that three of these irrigation systems (“Tuccio”, “Spilinga I” and “Spilinga III”) noticeably differ from the other SHPs analyzed in this study. In more detail, for “Spilinga” sub-systems, in the calculation of the electrical power using OM, a large part of the main pipelines (the feeders) were not considered, due to the very limited geodetic differences. In the “Tuccio” system there is a particular distribution of the irrigable areas: since about 50% of the served areas lay on the flat coast, the geodetic heads cannot be exploited for hydro-electrical production, differently from the other irrigation systems.

Overall, for some of the SHP plants analyzed in this study (those with P_n less than 150–175 kW, Figure 5), the error in estimating the electrical power of the equivalent ESHP plant was very limited (in four cases below 10–15%). For such irrigation systems the simplified methodology proposed in this study can provide a rough estimate of the hydro-electrical energy that can be produced. These water networks hosting SHP plants correspond to the simplest irrigation systems from the plano-altimetric point of view—the most widespread in Calabria—consisting of single intake and supplying pipeline distributing the irrigation water by the main and, from these latter, secondary pipelines (for example the “Murria” system, Figure 2), which are typically very close to the scheme of the ESHP.

Finally, it can also be noticed that: (i) the overestimation (or, in some cases, the underestimation) of the pipeline length of the ESHP plant (calculated by the SM) did not influence P_n estimates provided by the OM; (ii) the estimate of ΔH_n* by the same SM gave values that are practically exact, since they were equal to the corresponding heads calculated by OM (Table 5).

4. Conclusions

To calculate electrical power of SHP plants integrated in collective irrigation systems, this study has proposed and verified a simple methodology, requiring input data that are easily available at the Water User Associations managing the related water networks. The working hypothesis has adopted a hydraulic scheme of the water network (“equivalent system”) consisting of a single pressured conduit with homogeneous diameter and material. The proposed method basically requires only the altimetry and the maps of the irrigated areas.

The comparison between the electrical power of the equivalent SHP plants (estimated using the proposed method) and the corresponding estimates provided by the method proposed by the same authors [23,27] has given errors of about 18–20% with a maximum value of 50%. The simplified method has provided the highest accuracy in electrical power estimates of smaller SHP plants (not exceeding 150–175 kW).

Excluding its use for detailed projects (for which the method may produce large errors), this simplified method could support the technicians and decision makers in roughly estimating the hydro-electric potential of smaller SHP plants integrated in existing irrigation systems. This could be useful in order to assess whether or not to proceed with subsequent detailed studies (executive projects) without requiring large efforts to acquire other input parameters (such as material and diameter of the water network pipelines). Further methodological and in-depth developments could improve the reliability of the estimates provided by the proposed method, in order to increase its usefulness and consolidate its practical use.