Inherent Relationship between Flow Duration Curves at Different Time Scales: A Perspective on Monthly Flow Data Utilization in Daily Flow Duration Curve Estimation
Abstract
:1. Introduction
2. Methodology
2.1. M-FDC-P Method
2.2. E-FDC-R Method
- Estimation of the empirical FDCs. An empirical FDC is constructed by ranking flows at specific time scales from all recorded years and plotting them against an estimate of their exceedance probability, known as a plotting position [1]. The first step in empirical FDC construction is to sort flow data from highest to lowest. For the probability with which each flow is exceeded, the Weibull plotting position is then used, as it provides an unbiased estimate of exceedance probability, regardless of the underlying probability distribution of the ranked observations [1]. The Weibull plotting position is described as follows:where p is the exceedance probability for the mth flow data, and N is the number of the total flow records. Plot p on x axis and the corresponding flow (the mth flow value) on y axis. The plotted dots and the x and y axis form the empirical FDC.
- Calculation of the ratios between empirical FDCs. Firstly, sample a series of flow with pre-selected exceedance probabilities of empirical FDCs at different time scales, and then calculate the ratios of flow values at different time scales with given exceedance probabilities. Thus, the quantitative relationship of FDCs at different time scales is achieved. It should be noted that the number of pre-selected exceedance probabilities needs to be large enough to sufficiently represent the ratio relation of FDCs. Secondly, the quantitative relationship is analyzed in order to find a certain function to represent the quantitative relationship between FDCs.
- Evaluation of Modelled FDC. Once the specific function is obtained, the FDC at smaller time scale can be derived via the empirical FDC at larger time scale. To evaluate the performance of FDC at smaller time scale to reproduce observations, a measure of the standardized mean square error commonly referred to as Nash–Sutcliffe efficiency (NSE) is used. The description of NSE is introduced in [9], and hence not reproduced herein.
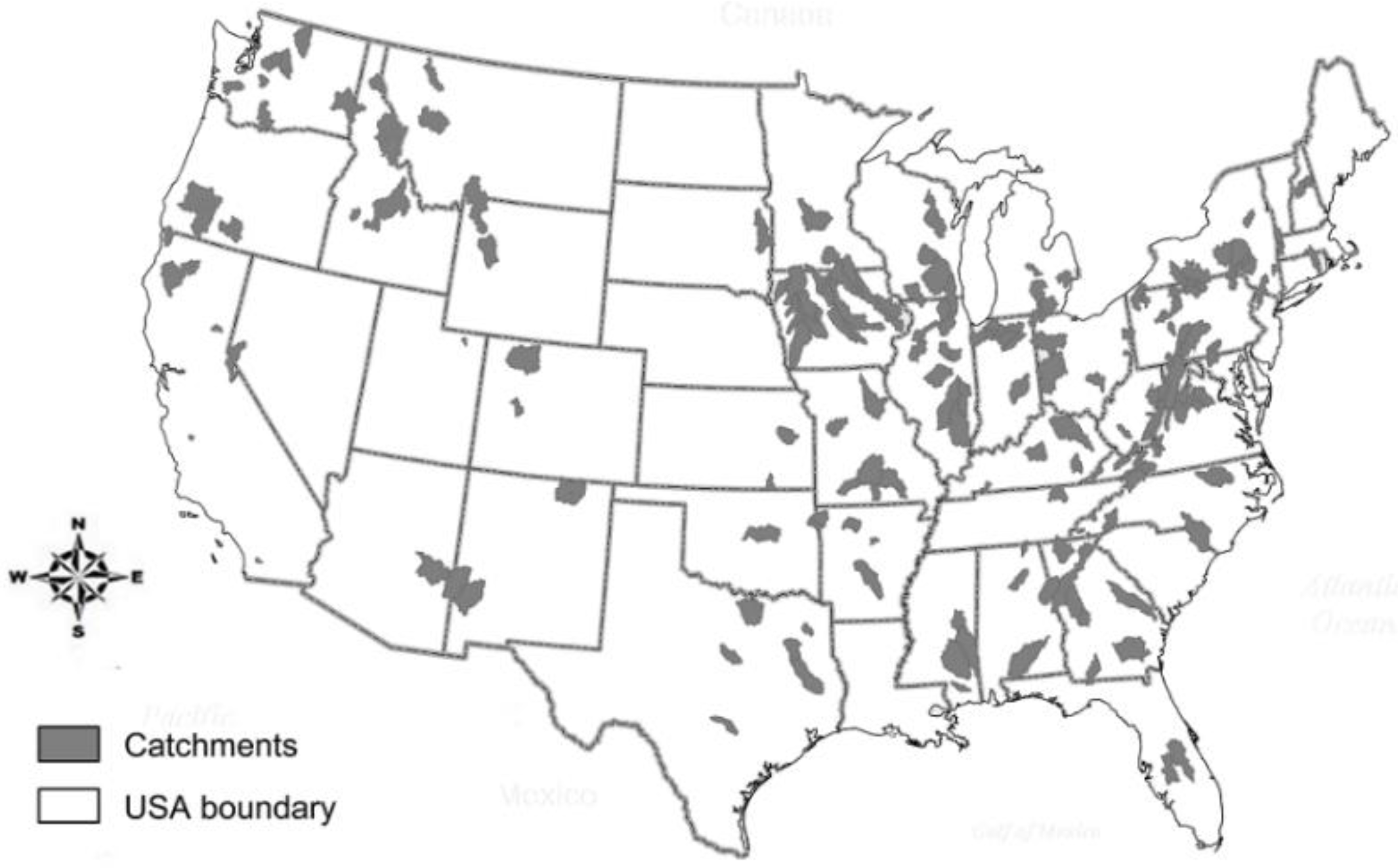
3. Study Area and Data
4. Results and Analysis
4.1. Empirical FDCs Variation with Different Time Scales
4.2. Relationships of FDCs Derived via M-FDC-P
4.3. Relationships of FDCs Derived via E-FDC-R
5. Discussion
6. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Vogel, R.M.; Fennessey, N.M. L moment diagrams should replace product moment diagrams. Water Resour. Res. 1993, 29, 1745–1752. [Google Scholar] [CrossRef]
- LeBoutillier, D.W.; Waylen, P.R. A stochastic model of flow duration curves. Water Resour. Res. 1993, 29, 3535–3541. [Google Scholar] [CrossRef]
- Cheng, L.; Yaeger, M.; Viglione, A.; Coopersmith, E. Exploring the physical controls of regional patterns of flow duration curves—Part 1: Insights from statistical analyses. Hydrol. Earth Syst. Sci. 2012, 9, 4435–4446. [Google Scholar] [CrossRef] [Green Version]
- Zhang, Q.; Xu, C.Y.; Chen, Y.D.; Yu, Z. Multifractal detrended fluctuation analysis of streamflow series of the Yangtze River basin, China. Hydrol. Process. 2008, 22, 4997–5003. [Google Scholar] [CrossRef]
- Sivakumar, B. Chaos theory in geophysics: Past, present and future. Chaos Soliton Fract. 2004, 19, 441–462. [Google Scholar] [CrossRef]
- Wang, W.; Vrijling, J.K.; van Gelder, P.H.A.J.M.; Ma, J. Testing for nonlinearity of streamflow processes at different timescales. J. Hydrol. 2006, 322, 247–268. [Google Scholar] [CrossRef]
- Tung, W.W.; Gao, J.; Hu, J.; Yang, L. Detecting chaos in heavy-noise environments. Phys. Rev. E 2011, 83, 046210. [Google Scholar] [CrossRef] [PubMed]
- Bowers, M.C.; Tung, W.W.; Gao, J.B. On the distributions of seasonal river flows: Lognormal or power law? Water Resour. Res. 2012, 48, 5536. [Google Scholar] [CrossRef]
- Blum, A.G.; Archfield, S.A.; Vogel, R.M. On the probability distribution of daily streamflow in the United States. Hydrol. Earth Syst. Sci. 2017, 21, 3093–3103. [Google Scholar] [CrossRef]
- Castellarin, A.; Botter, G.; Hughes, D.A.; Liu, S.; Ouarda, T.B.M.J.; Parajka, J.; Post, D.A.; Sivapalan, M.; Spence, C.; Viglione, A.; et al. Prediction of flow duration curves ungauged basins. In Runoff Prediction in Ungauged Basins: Synthesis Across Processes, Places and Scales; Blöschl, G., Sivapalan, M., Wagener, T., Viglione, A., Savenije, H., Eds.; Cambridge University Press: Cambridge, UK, 2013; p. 465. [Google Scholar]
- Hsu, N.S.; Huang, C.J. Estimation of Flow Duration Curve at Ungauged Locations in Taiwan. J. Hydrol. Eng. 2017, 22, 05017009. [Google Scholar] [CrossRef]
- Chiang, S.M. Hydrologic Regionalization of Watersheds. II: Applications. J. Water Res. Plan. Manag. 2002, 128, 12–20. [Google Scholar] [CrossRef]
- Mohamoud, Y. Prediction of daily flow duration curves and streamflow for ungauged catchments using regional flow duration curves. Hydrol. Sci. J. 2008, 53, 706–724. [Google Scholar] [CrossRef] [Green Version]
- Hope, A.; Bart, R. Synthetic monthly flow duration curves for the Cape Floristic Region, South Africa. Water SA 2012, 38, 191–200. [Google Scholar] [CrossRef]
- Shu, C.; Ouarda, T.B.M.J. Improved methods for daily streamflow estimates at ungauged sites. Water Resour. Res. 2012, 48, 2523. [Google Scholar] [CrossRef]
- Castellarin, A.; Galeati, G.; Brandimarte, L.; Montanari, A.; Brath, A. Regional flow-duration curves: Reliability for ungauged basins. Adv. Water Resour. 2004, 27, 953–965. [Google Scholar] [CrossRef]
- Li, M.; Shao, Q.; Zhang, L.; Chiew, F.H.S. A new regionalization approach and its application to predict flow duration curve in ungauged basins. J. Hydrol. 2010, 389, 137–145. [Google Scholar] [CrossRef]
- Mendicino, G.; Senatore, A. Evaluation of parametric and statistical approaches for the regionalization of flow duration curves in intermittent regimes. J. Hydrol. 2013, 480, 19–32. [Google Scholar] [CrossRef]
- Yu, P.S.; Yang, T.C.; Liu, C.W. A regional model of low flow for southern Taiwan. Hydrol. Process. 2010, 16, 2017–2034. [Google Scholar] [CrossRef]
- Pugliese, A.; Farmer, W.H.; Castellarin, A.; Archfield, S.A.; Vogel, R.M. Regional flow duration curves: Geostatistical techniques versus multivariate regression. Adv. Water Resour. 2016, 96, 11–22. [Google Scholar] [CrossRef]
- Chokmani, K.; Ouarda, T.B.M.J. Physiographical space-based kriging for regional flood frequency estimation at ungauged sites. Water Resour. Res. 2004, 40, 1–13. [Google Scholar] [CrossRef]
- Skoien, J.O.; Merz, R.; Bloschl, G. Top-kriging-geostatistics on stream networks. Hydrol. Earth Syst. Sci. 2006, 2, 2253–2286. [Google Scholar] [CrossRef]
- Castiglioni, S.; Castellarin, A.; Montanari, A. Prediction of low-flow indices in ungauged basins through physiographical space-based interpolation. J. Hydrol. 2009, 378, 272–280. [Google Scholar] [CrossRef]
- Archfield, S.A.; Pugliese, A.; Castellarin, A.; Skøien, J.O. Topological and canonical kriging for design-flood prediction in ungauged catchments: An improvement over a traditional regional regression approach? Hydrol. Earth Syst. Sci. 2013, 9, 1575–1588. [Google Scholar] [CrossRef]
- Castellarin, A.; Camorani, G.; Brath, A. Predicting annual and long-term flow-duration curves in ungauged basins. Adv. Water Resour. 2007, 30, 937–953. [Google Scholar] [CrossRef]
- Hosking, J.R.M.; Wallis, J.R. Regional Frequency Analysis: An Approach Based on L-Moments; Cambridge University Press: Cambridge, UK, 1997; p. 244. [Google Scholar]
- Hosking, J.R.M. L-moments: Analysis and estimation of distributions using linear combinations of order statistics. J. R. Stat. Soc. B Methodol. 1990, 52, 105–124. [Google Scholar]
- Kjeldsen, T.R.; Ahn, H.; Prosdocimi, I. On the use of a four-parameter kappa distribution in regional frequency analysis. Hydrol. Sci. J. 2017, 62, 1–10. [Google Scholar] [CrossRef]
- Duan, Q.; Schaakeb, J.; Andréassian, V.; Franks, S.; Gotetie, G.; Gupta, H.V.; Gusev, Y.M.; Habet, F.; Hall, A.; Hay, L.; et al. Model Parameter Estimation Experiment (MOPEX): An overview of science strategy and major results from the second and third workshops. J. Hydrol. 2006, 320, 3–17. [Google Scholar]
- Slack, J.R.; Lumb, A.M.; Landwehr, J.M. Hydro-climatic data network (HCDN): A U.S. Geological Survey streamflow data set for the United States for the study of climate variation, 1874–1988. J. Phys. Chem. C 1992, 113, 2538–2544. [Google Scholar]
- Wang, D.; Hejazi, M. Quantifying the relative contribution of the climate and direct human impacts on mean annual streamflow in the contiguous United States. Water Resour. Res. 2011, 47, 411. [Google Scholar] [CrossRef]
- Kroll, C.N.; Croteau, K.E.; Vogel, R.M. Hypothesis tests for hydrologic alteration. J. Hydrol. 2015, 530, 117–126. [Google Scholar] [CrossRef]
- Smakhtin, V.Y.; Watkins, D.A. Low Flow Estimation in South Africa; Report No 494/1/97; Water Research Commission: Pretoria, South Africa, 1997. [Google Scholar]
- Parida, B.P. Modelling of Indian Summer Monsoon Rainfall Using a Four-parameter Kappa Distribution. Int. J. Climatol. 1999, 19, 1389–1398. [Google Scholar] [CrossRef]
- Smakhtin, V.U. Estimating daily flow duration curves from monthly streamflow data. Water SA 2000, 26, 13–18. [Google Scholar]
- Sugiyama, H.; Vudhivanich, V.; Whitaker, A.; Lorsirirat, K. Stochastic Flow Duration Curves for Evaluation of Flow Regimes in Rivers. J. Am. Water Resour. Assoc. 2007, 39, 47–58. [Google Scholar] [CrossRef]







| Parameter | ξ | α | k | h | ||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Time Scale | 1 Day | 7 Day | 15 Day | 30 Day | 1 Day | 7 Day | 15 Day | 30 Day | 1 Day | 7 Day | 15 Day | 30 Day | 1 Day | 7 Day | 15 Day | 30 Day |
| 1 day | 1 | 0.959 | 0.901 | 0.858 | 1 | 0.953 | 0.893 | 0.835 | 1 | 0.867 | 0.654 | 0.475 | 1 | 0.987 | 0.967 | 0.940 |
| 7 day | 0.959 | 1 | 0.982 | 0.954 | 0.953 | 1 | 0.981 | 0.941 | 0.867 | 1 | 0.931 | 0.817 | 0.987 | 1 | 0.992 | 0.973 |
| 15 day | 0.901 | 0.982 | 1 | 0.990 | 0.893 | 0.981 | 1 | 0.985 | 0.654 | 0.931 | 1 | 0.962 | 0.967 | 0.992 | 1 | 0.992 |
| 30 day | 0.858 | 0.954 | 0.990 | 1 | 0.835 | 0.941 | 0.985 | 1 | 0.475 | 0.817 | 0.962 | 1 | 0.940 | 0.973 | 0.992 | 1 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ye, L.; Ding, W.; Zeng, X.; Xin, Z.; Wu, J.; Zhang, C. Inherent Relationship between Flow Duration Curves at Different Time Scales: A Perspective on Monthly Flow Data Utilization in Daily Flow Duration Curve Estimation. Water 2018, 10, 1008. https://doi.org/10.3390/w10081008
Ye L, Ding W, Zeng X, Xin Z, Wu J, Zhang C. Inherent Relationship between Flow Duration Curves at Different Time Scales: A Perspective on Monthly Flow Data Utilization in Daily Flow Duration Curve Estimation. Water. 2018; 10(8):1008. https://doi.org/10.3390/w10081008
Chicago/Turabian StyleYe, Lei, Wei Ding, Xiaofan Zeng, Zhuohang Xin, Jian Wu, and Chi Zhang. 2018. "Inherent Relationship between Flow Duration Curves at Different Time Scales: A Perspective on Monthly Flow Data Utilization in Daily Flow Duration Curve Estimation" Water 10, no. 8: 1008. https://doi.org/10.3390/w10081008
APA StyleYe, L., Ding, W., Zeng, X., Xin, Z., Wu, J., & Zhang, C. (2018). Inherent Relationship between Flow Duration Curves at Different Time Scales: A Perspective on Monthly Flow Data Utilization in Daily Flow Duration Curve Estimation. Water, 10(8), 1008. https://doi.org/10.3390/w10081008





