Improving the Performance of Water Distribution Networks Based on the Value Index in the System Dynamics Framework
Abstract
:1. Introduction
2. Materials and Methods
2.1. Environmental Assessment
2.1.1. Goal and Scope Definition
2.1.2. Life Cycle Inventory
2.1.3. Life Cycle Impact Assessment
2.1.4. Environmental Performance Index
2.2. Hydraulic Assessment
2.2.1. Leakage Estimation
2.2.2. Hydraulic Performance Index
2.3. Value Index
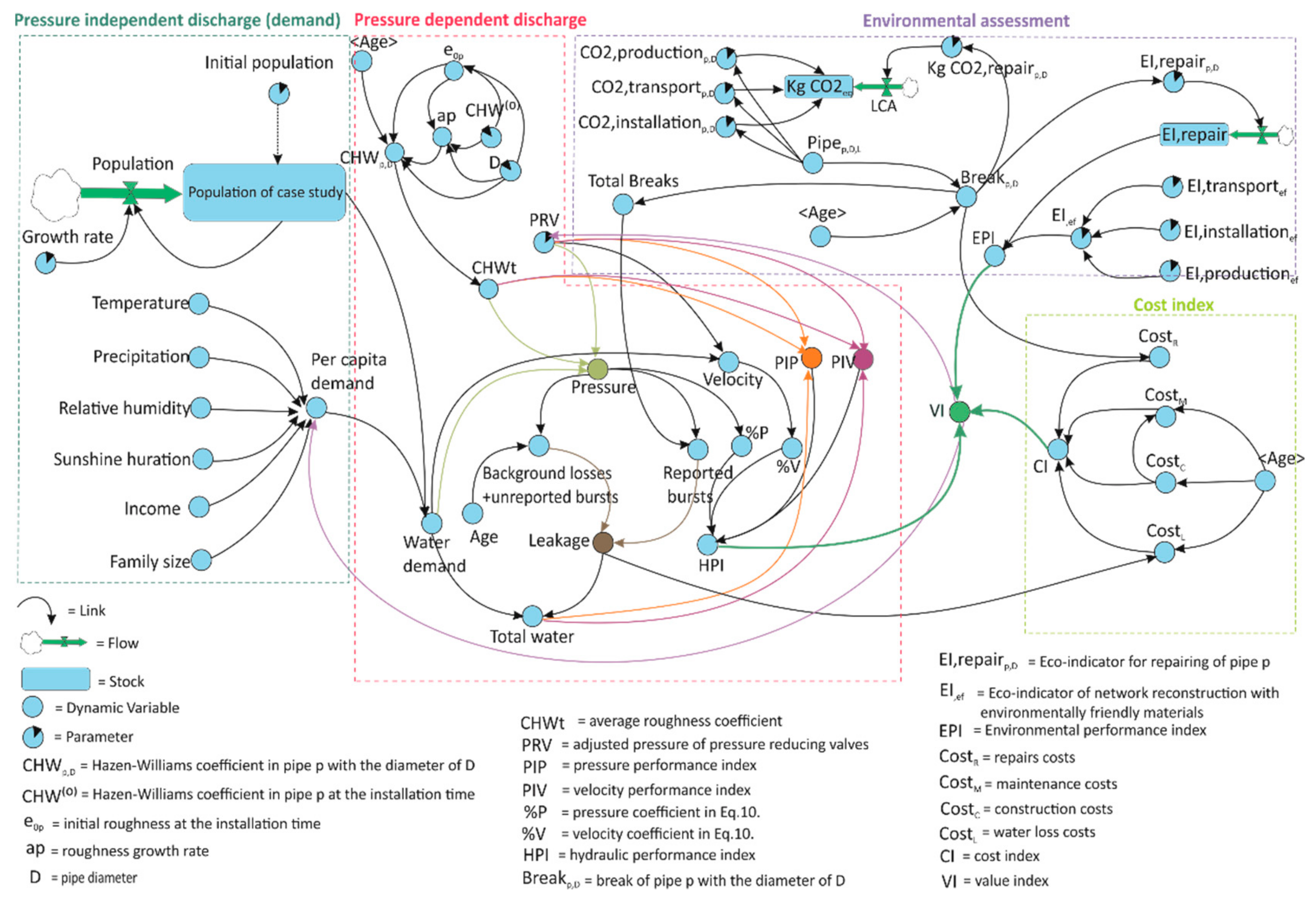
2.4. System Dynamics
- Pipe roughness coefficient: hydraulic capacity of pipes decreases over time. In general, reducing hydraulic capacity is the result of increasing roughness in pipes. As roughness of a pipe increases, roughness coefficient decreases. Thus, according to the Hazen–Williams Equation (14), overall head loss increases and pressure decreases as a result. Therefore, the pipes’ roughness coefficients are directly related to the pressure of WDNs. In the SD model, Equation (15) is used to consider the changes in Hazen–Williams coefficient in each pipe [44]. Average roughness coefficient of a network (CHWt) as the representative of the whole system roughness is calculated by weighting the coefficient of each diameter based on its length.
- Demand changes: The pressure independent discharge (demand) is the second variable on which the pressure and velocity of WDNs are dependent. Increasing the demand results in increasing the consumption which reduces the pressure of the nodes and increases the flow velocity in the pipes.
- Adjusted pressure of pressure-reducing valves (PRV): adjusted pressure of PRV valves can have a significant effect on the pressure and velocity of the system.
- Discharge of the background losses and unreported bursts: it is considered as the function of the average pressure and average age of the system (Equation (18)).
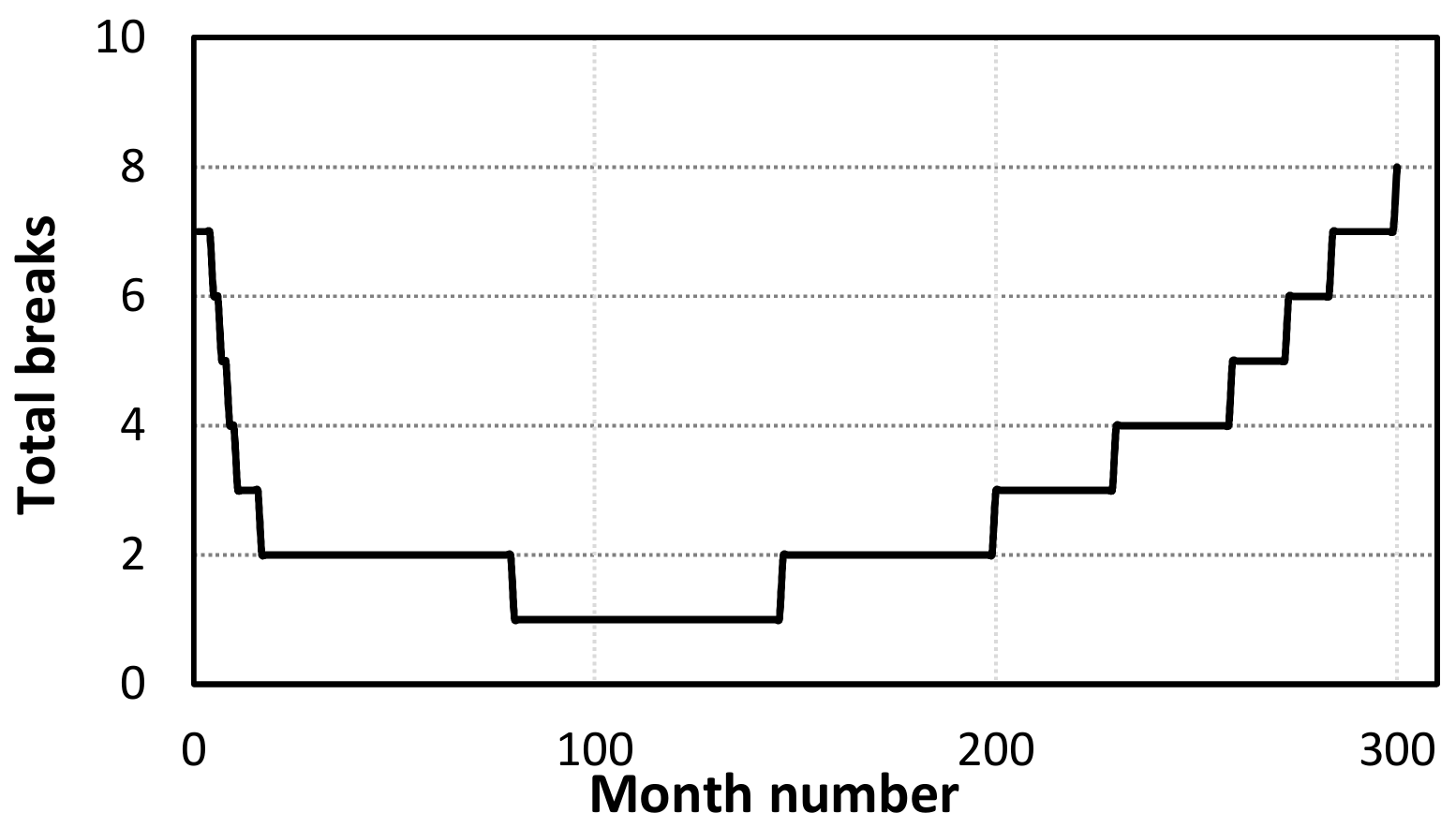
- Discharge of the reported bursts: regarding the guidelines [41], it is considered as the function of total breaks, the flow rate of burst, the average pressure of the system, and the duration of the total breaks (Equation (19)). The break rate of the pipes is calculated based on Equations (20) and (21) [45]. According to the previous studies and guidelines [41,46], the flow rate of burst () is considered 12 . The parameters of A, B, C in Equation (18) and t (the total break duration) in Equation (19) are determined by calibration of these equations with the leakage calculated based on MNF method. For this purpose, OptQuest calibration module [47,48] in AnyLogic software is used. This module minimizes the difference between the simulated and observed leakage. This process is basically an optimization process which its objective function is defined in accordance with Equation (22).
3. Case Study
4. Results and Discussion
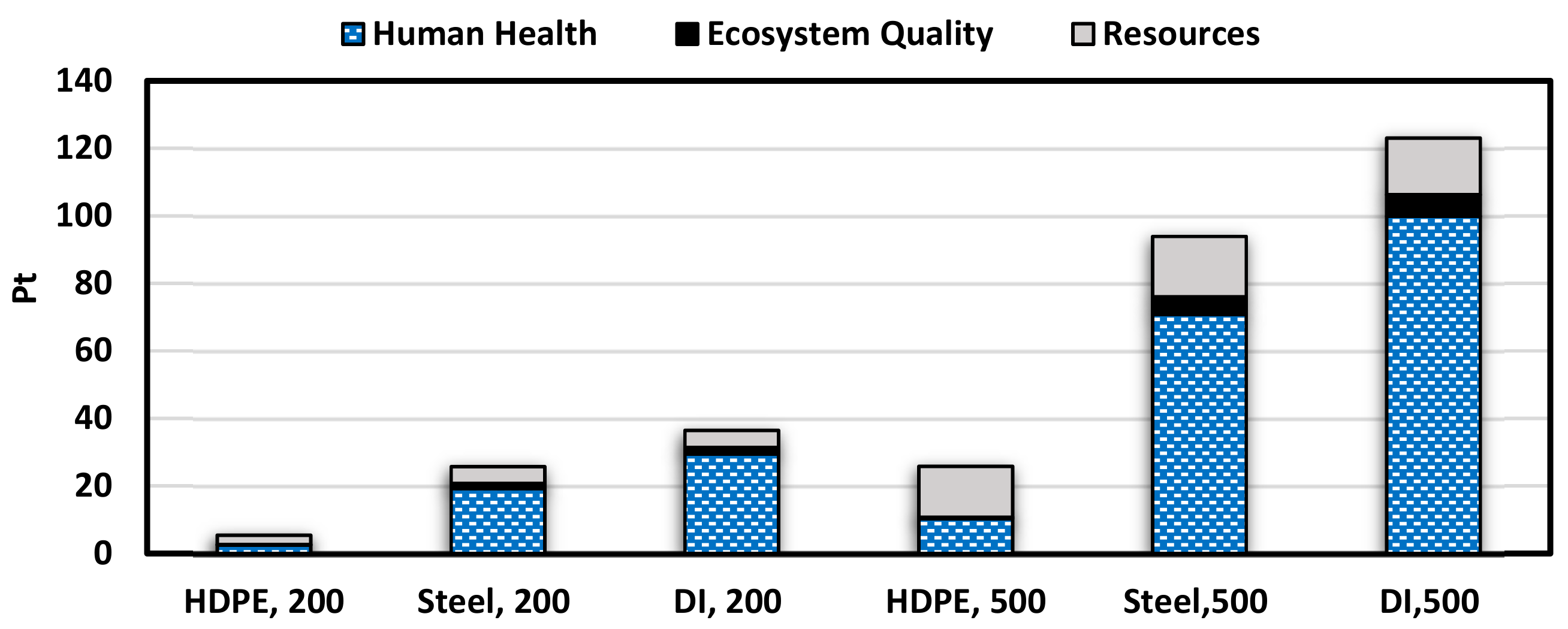
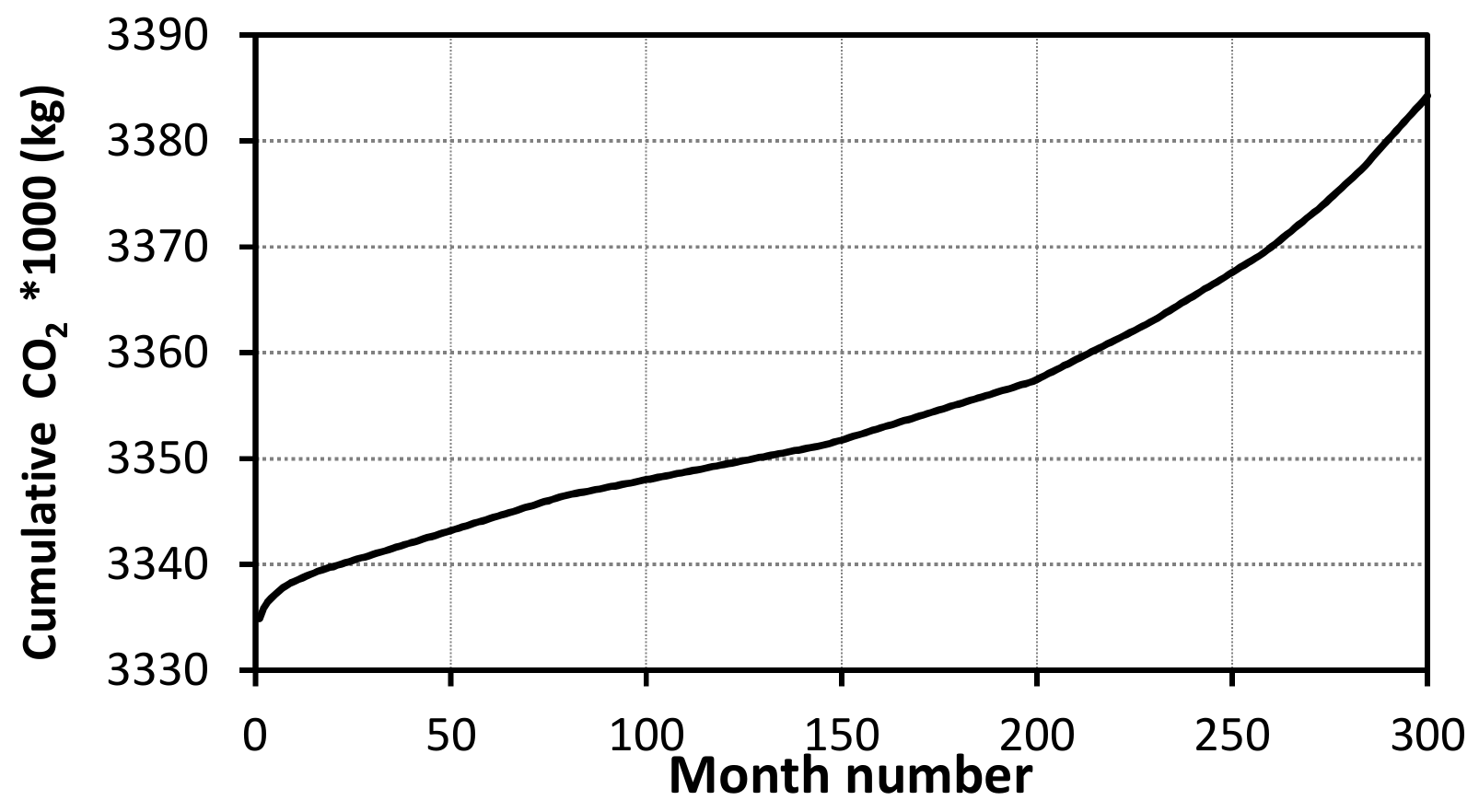
4.1. Environmental Impacts Assessment
4.2. Calibration of the Leakage Equation
4.3. Correlation between the Simulated and Observed Inflow
4.4. Evaluation of the Leakage, Pressure, and Inflow of the WDN
4.5. Evaluation of the Leakage, Pressure, and Inflow of the WDN
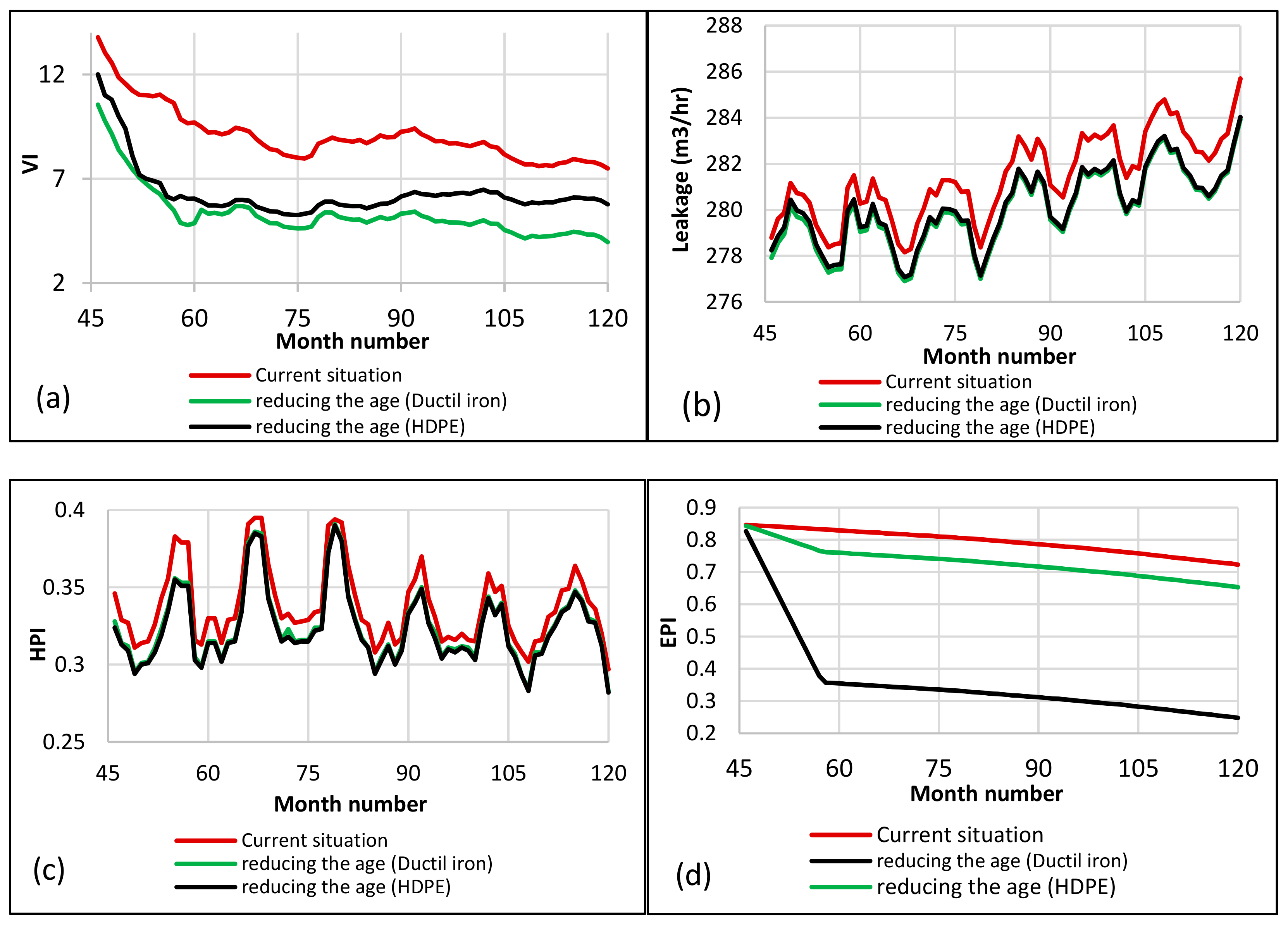
4.6. Evaluating VI of the System
4.7. Scenarios for Improvement of VI
4.7.1. Scenario 1: Reducing the Per Capita Water Demand
4.7.2. Scenario 2: Reducing the Average Pressure
4.7.3. Scenario 3: Reducing the Age of the System by Renewing Pipe
4.7.4. Scenario 4: Reducing the Per Capita Water Demand and Average Pressure
4.7.5. Comparing the Best Scenarios
5. Summary and Conclusions
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- United Nations Educational Scientific and Cultural Organization (UNESCO). The United Nations World Water Development Report 4: Managing Water Under Uncertainty and Risk; United Nations Educational Scientific and Cultural Organization (UNESCO): Paris, France, 2012; Volume 1. [Google Scholar]
- Veldkamp, T.I.E.; Wada, Y.; Aerts, J.; Döll, P.; Gosling, S.N.; Liu, J.; Masaki, Y.; Oki, T.; Ostberg, S.; Pokhrel, Y. Water scarcity hotspots travel downstream due to human interventions in the 20th and 21st century. Nat. Commun. 2017, 8, 15697. [Google Scholar] [CrossRef] [PubMed]
- Grigg, N.S. Main Break Prediction, Prevention, and Control; American Water Works Association: Denver, CO, USA, 2007; Volume 75. [Google Scholar]
- Sterman, J.D. System dynamics modeling: Tools for learning in a complex world. Calif. Manag. Rev. 2001, 43, 8–25. [Google Scholar] [CrossRef]
- Zarghami, M.; Akbariyeh, S. System dynamics modeling for complex urban water systems: Application to the city of Tabriz, Iran. Resour. Conserv. Recycl. 2012, 60, 99–106. [Google Scholar] [CrossRef]
- Ahmad, S.; Simonovic, S.P. Spatial system dynamics: New approach for simulation of water resources systems. J. Comput. Civ. Eng. 2004, 18, 331–340. [Google Scholar] [CrossRef]
- Ahmad, S.; Simonovic, S.P. An intelligent decision support system for management of floods. Water Resour. Manag. 2006, 20, 391–410. [Google Scholar] [CrossRef]
- Madani, K.; Mariño, M.A. System dynamics analysis for managing Iran’s Zayandeh-Rud river basin. Water Resour. Manag. 2009, 23, 2163–2187. [Google Scholar] [CrossRef]
- Rusuli, Y.; Li, L.; Ahmad, S.; Zhao, X. Dynamics model to simulate water and salt balance of Bosten Lake in Xinjiang, China. Environ. Earth Sci. 2015, 74, 2499–2510. [Google Scholar] [CrossRef]
- Sharawat, I.; Dahiya, R.; Dahiya, R.P.; Sreekrishnan, T.R.; Kumari, S. Policy options for managing the water resources in rapidly expanding cities: A system dynamics approach. Sustain. Water Resour. Manag. 2019, 5, 1201–1215. [Google Scholar] [CrossRef]
- Sun, B.; Yang, X. Simulation of water resources carrying capacity in Xiong’an New Area based on system dynamics model. Water 2019, 11, 1085. [Google Scholar] [CrossRef]
- Qaiser, K.; Ahmad, S.; Johnson, W.; Batista, J.R. Evaluating water conservation and reuse policies using a dynamic water balance model. Environ. Manag. 2013, 51, 449–458. [Google Scholar] [CrossRef]
- Venkatesan, A.K.; Ahmad, S.; Johnson, W.; Batista, J.R. Systems dynamic model to forecast salinity load to the Colorado River due to urbanization within the Las Vegas Valley. Sci. Total Environ. 2011, 409, 2616–2625. [Google Scholar] [CrossRef] [PubMed]
- Neto, A.D.C.L.; Legey, L.F.L.; González-Araya, M.C.; Jablonski, S. A system dynamics model for the environmental management of the Sepetiba Bay watershed, Brazil. Environ. Manag. 2006, 38, 879–888. [Google Scholar] [CrossRef] [PubMed]
- Zhang, F.-Y.; Li, L.-H.; Ahmad, S.; Li, X.-M. Using path analysis to identify the influence of climatic factors on spring peak flow dominated by snowmelt in an alpine watershed. J. Mt. Sci. 2014, 11, 990–1000. [Google Scholar] [CrossRef]
- Simonovic, S.P.; Li, L. Methodology for assessment of climate change impacts on large-scale flood protection system. J. Water Resour. Plan. Manag. 2003, 129, 361–371. [Google Scholar] [CrossRef]
- Stojkovic, M.; Simonovic, S.P. System Dynamics Approach for Assessing the Behaviour of the Lim Reservoir System (Serbia) under Changing Climate Conditions. Water 2019, 11, 1620. [Google Scholar] [CrossRef]
- Salvitabar, A.; Zarghami, M.; Abrishamchi, A. System Dynamic Model in Tehran Urban Water Management. J. Water Wastewater 2006, 17, 12–28. [Google Scholar]
- Karamouz, M.; Goharian, E.; Nazif, S. Reliability assessment of the water supply systems under uncertain future extreme climate conditions. Earth Interact. 2013, 17, 1–27. [Google Scholar] [CrossRef]
- Dawadi, S.; Ahmad, S. Evaluating the impact of demand-side management on water resources under changing climatic conditions and increasing population. J. Environ. Manag. 2013, 114, 261–275. [Google Scholar] [CrossRef]
- Wu, G.; Li, L.; Ahmad, S.; Chen, X.; Pan, X. A dynamic model for vulnerability assessment of regional water resources in arid areas: A case study of Bayingolin, China. Water Resour. Manag. 2013, 27, 3085–3101. [Google Scholar] [CrossRef]
- Wei, T.; Lou, I.; Yang, Z.; Li, Y. A system dynamics urban water management model for Macau, China. J. Environ. Sci. 2016, 50, 117–126. [Google Scholar] [CrossRef]
- Chen, C.; Ahmad, S.; Kalra, A.; Xu, Z. A dynamic model for exploring water-resource management scenarios in an inland arid area: Shanshan County, Northwestern China. J. Mt. Sci. 2017, 14, 1039–1057. [Google Scholar] [CrossRef]
- Zarghami, S.A.; Gunawan, I.; Schultmann, F. System Dynamics Modelling Process in Water Sector: A Review of Research Literature. Syst. Res. Behav. Sci. 2018, 35, 776–790. [Google Scholar] [CrossRef]
- Karamouz, M.F.; Elyasi, A.; Ahmadi, A. An Algorithm for Evaluation of Water Resources Development. World Environ. Water Resour. Congr. 2008, 2008, 1–10. [Google Scholar]
- Li, C.; Liu, S.; Tao, T. Value engineering-based methodology of evaluating water distribution systems. Tongji Daxue Xuebao/J. Tongji Univ. Sci. 2006, 34, 646–650. [Google Scholar]
- Cuimei, L.; Suiqing, L. Water Distribution Systems Evaluating Method Based on Value Engineering: Case Study. In Water Distribution Systems Analysis Symposium 2006; American Society of Civil Engineers: Reston, VA, USA, 2008; pp. 1–11. [Google Scholar]
- Askarzadeh Farahani, M. Performance Evaluation of Water Distribution Networks Based on Value Engineering and Fuzzy Sets. Master’s Thesis, University of Tehran, Tehran, Iran, 2014. [Google Scholar]
- Li, C.; Li, S.; Wang, J.; Wang, H.; Zhang, S. The Comparison of the Value Engineering Method and Hydraulic Method on Water Distribution Systems Performance Evaluation. In World Environmental and Water Resources Congress 2011; American Society of Civil Engineers: Reston, VA, USA, 2011; pp. 4514–4522. [Google Scholar]
- International Organization for Standardization (ISO). ISO 14044—Environmental Management—Life Cycle Assessment—Requirements and Guidance; International Organization for Standardization: London, UK, 2006. [Google Scholar]
- Tehran Province Water and Wastewater Company (TPWWC). Statistics Obtained from the Director of Operation; Accessed on January 2017; Tehran Province Water and Wastewater Company (TPWWC): Tehran, Iran, 2017. [Google Scholar]
- Ecoinvent Swiss Centre for Life Cycle Inventories. Ecoinvent Database v3.0. Available online: http://www.ecoinvent.ch/ (accessed on 21 November 2019).
- Pre’ Consultants. SimaPro Database Manual; Pre’ Consultants: Amersfoort, The Nederlands, 2014. [Google Scholar]
- Roghani, B. Estimating the Sewerage Network Performance based on Value Engineering and Environmental Issues. Master’s Thesis, University of Tehran, Tehran, Iran, 2013. [Google Scholar]
- Tabesh, M.; Yekta, A.H.A.; Burrows, R. An integrated model to evaluate losses in water distribution systems. Water Resour. Manag. 2009, 23, 477–492. [Google Scholar] [CrossRef]
- Nazif, S.; Karamouz, M.; Tabesh, M.; Moridi, A. Pressure management model for urban water distribution networks. Water Resour. Manag. 2010, 24, 437–458. [Google Scholar] [CrossRef]
- Rossman, L.A. EPANET2 Users Manual National Risk Management Research Laboratory; US Environmental Protection Agency: Cincinnati, OH, USA, 2000.
- Lambert, A. Pressure Management/Leakage Relationships: Theory, Concepts and Practical Applications; IQPC Seminar: London, UK, 1997. [Google Scholar]
- Germanopoulos, G. A technical note on the inclusion of pressure dependent demand and leakage terms in water supply network models. Civ. Eng. Syst. 1985, 2, 171–179. [Google Scholar] [CrossRef]
- Tabesh, M.; Zia, A. Dynamic management of water distribution networks based on hydraulic performance analysis of the system. Water Sci. Technol. Water Supply 2003, 3, 95–102. [Google Scholar] [CrossRef]
- IRIVSPS (Islamic Republic of Iran Vice presidency for Strategic Planning and Suppervision). Guidline for Determining Effective Parameters an Unaccounted for Water and Water Losses Reduction Schemes; IRIVSPS: Tehran, Iran, 2012. [Google Scholar]
- Goodman, A.S.; Hastak, M. Infrastructure Planning Handbook: Planning, Engineering, and Economics; ASCE: Reston, VA, USA, 2006. [Google Scholar]
- Management and Planning Organization of Iran (MPO). Unit Price List of Water Distribution Network; Management and Planning Organization of Iran (MPO): Tehran, Iran, 2007. [Google Scholar]
- Sharp, W.W.; Walski, T.M. Predicting internal roughness in water mains. J. Am. Water Works Assoc. 1988, 80, 34–40. [Google Scholar] [CrossRef]
- Wang, Y.; Zayed, T.; Moselhi, O. Prediction Models for Annual Break Rates of Water Mains. J. Perform. Constr. Facil. 2009, 23, 47–54. [Google Scholar] [CrossRef]
- Lambert, A.O.; McKenzie, R.D. Practical experience in using the Infrastructure Leakage Index. In Proceedings of the IWA Conference–Leakage Management: A Practical Approach; Water Board of Lemesos: Lemesos, Cyprus, 2002. [Google Scholar]
- Kleijnen, J.P.C.; Wan, J. Optimization of simulated systems: OptQuest and alternatives. Simul. Model. Pract. Theory 2007, 15, 354–362. [Google Scholar] [CrossRef]
- Laguna, M. Optimization of Complex Systems with OptQuest; A White Paper; OptTek Systems Inc.: Boulder, CO, USA, 1997; pp. 1–13. [Google Scholar]
- Tabesh, M. Advanced Modeling of Water Distribution Networks; University of Tehran Press: Tehran, Iran, 2016. [Google Scholar]
- Friedrich, E.; Pillay, S.; Buckley, C.A. Carbon footprint analysis for increasing water supply and sanitation in South Africa: A case study. J. Clean. Prod. 2009, 17, 1–12. [Google Scholar] [CrossRef]
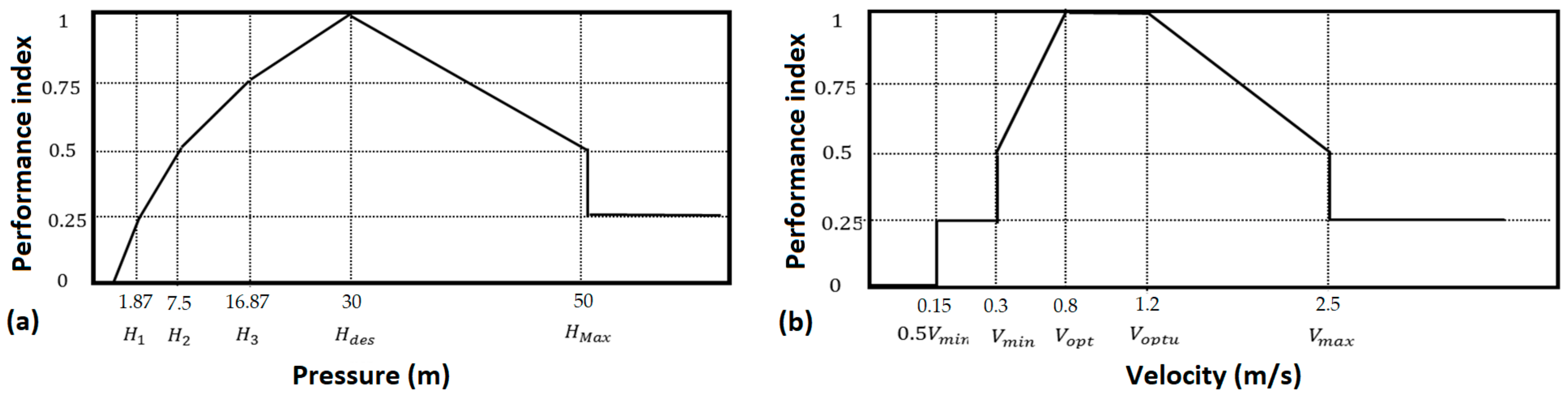











| Factors | Empirical Equations | Equation | |
|---|---|---|---|
| Head loss (H) | , | (14) | |
| Remark | H is head loss (m), is flow rate (), L is length of pipe (m), D is diameter (m), and CHW is Hazen–Williams coefficient. | ||
| Roughness coefficient () | , [44] | (15) | |
| Remark | is Hazen–Williams coefficient in pipe p at year t, is initial roughness at the installation time (unit of length), is roughness growth rate in pipe p (unit of length per year), is the diameter of pipe p (unit of length), is the age of pipe p at the present time (year) and t is annual time. , and are calculated using Equations (16) and (17). | ||
| Initial roughness | , | (16) | |
| roughness growth rate () | , | (17) | |
| Remark | is Hazen–Williams coefficient in pipe p at the installation time. | ||
| Background losses + unreported bursts | , | (18) | |
| Remark | is the average pressure in the SD model (m), is the average age (year), A, B, and C are undetermined coefficients. | ||
| Reported bursts | , [41] | (19) | |
| Remark | is the total bursts of pipes in each time step, is the flow rate of burst in the pressure of 50 m (), is the average pressure (m), is the power term of pressure, and is the total time of break (h). | ||
| Break rate of ductile iron and steel pipes | , [45] | (20) | |
| Break rate of plastic pipes | , [45] | (21) | |
| Remark | is the rate of break (), is the length of pipe (m), is the age of pipe (year), and S is pipe diameter (mm). | ||
| Objective function of the calibration module | (22) | ||
| Remark | is the leakage which is calculated based on the MNF, is the leakage which is obtained from the sum of Equations (18) and (19). | ||
| Factors | Regression Equations | Equation | |
| Per capita demand | , | 0.943 | (23) |
| Remark | All the variables are calculated based on the average monthly amount. | ||
| Average pressure | , | 0.997 | (24) |
| Average velocity | , | 0.992 | (25) |
| Remark | is the average monthly demand (), is the average roughness coefficient and is adjusted pressure of the PRV valves (m). | ||
| Pressure performance index (PIP) | , | 0.993 | (26) |
| Velocity performance index (PIV) | , | 0.951 | (27) |
| Remark | is total inflow to the network (), is the average roughness coefficient, and is the pressure of the PRV valves (m). |
| Parameters | Remark | Optimum Value |
|---|---|---|
| A | Coefficient of the average pressure | 4.472 |
| B | Coefficient of the average age | 0.507 |
| C | Constant number | 99.939 |
| T | Total breaks duration (h) | 24 |
| Simulated Phase | R | RS | |
|---|---|---|---|
| After calibration | 0.9516 | 0.97 | 24.4 |
| Validation | 0.9073 | 0.94 | 27.5 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hajibabaei, M.; Nazif, S.; Sitzenfrei, R. Improving the Performance of Water Distribution Networks Based on the Value Index in the System Dynamics Framework. Water 2019, 11, 2445. https://doi.org/10.3390/w11122445
Hajibabaei M, Nazif S, Sitzenfrei R. Improving the Performance of Water Distribution Networks Based on the Value Index in the System Dynamics Framework. Water. 2019; 11(12):2445. https://doi.org/10.3390/w11122445
Chicago/Turabian StyleHajibabaei, Mohsen, Sara Nazif, and Robert Sitzenfrei. 2019. "Improving the Performance of Water Distribution Networks Based on the Value Index in the System Dynamics Framework" Water 11, no. 12: 2445. https://doi.org/10.3390/w11122445
APA StyleHajibabaei, M., Nazif, S., & Sitzenfrei, R. (2019). Improving the Performance of Water Distribution Networks Based on the Value Index in the System Dynamics Framework. Water, 11(12), 2445. https://doi.org/10.3390/w11122445






