Energy Reduction and Uniformity of Low-Pressure Online Drip Irrigation Emitters in Field Tests
Abstract
:1. Introduction
2. Materials and Methods
2.1. Experimental Sites
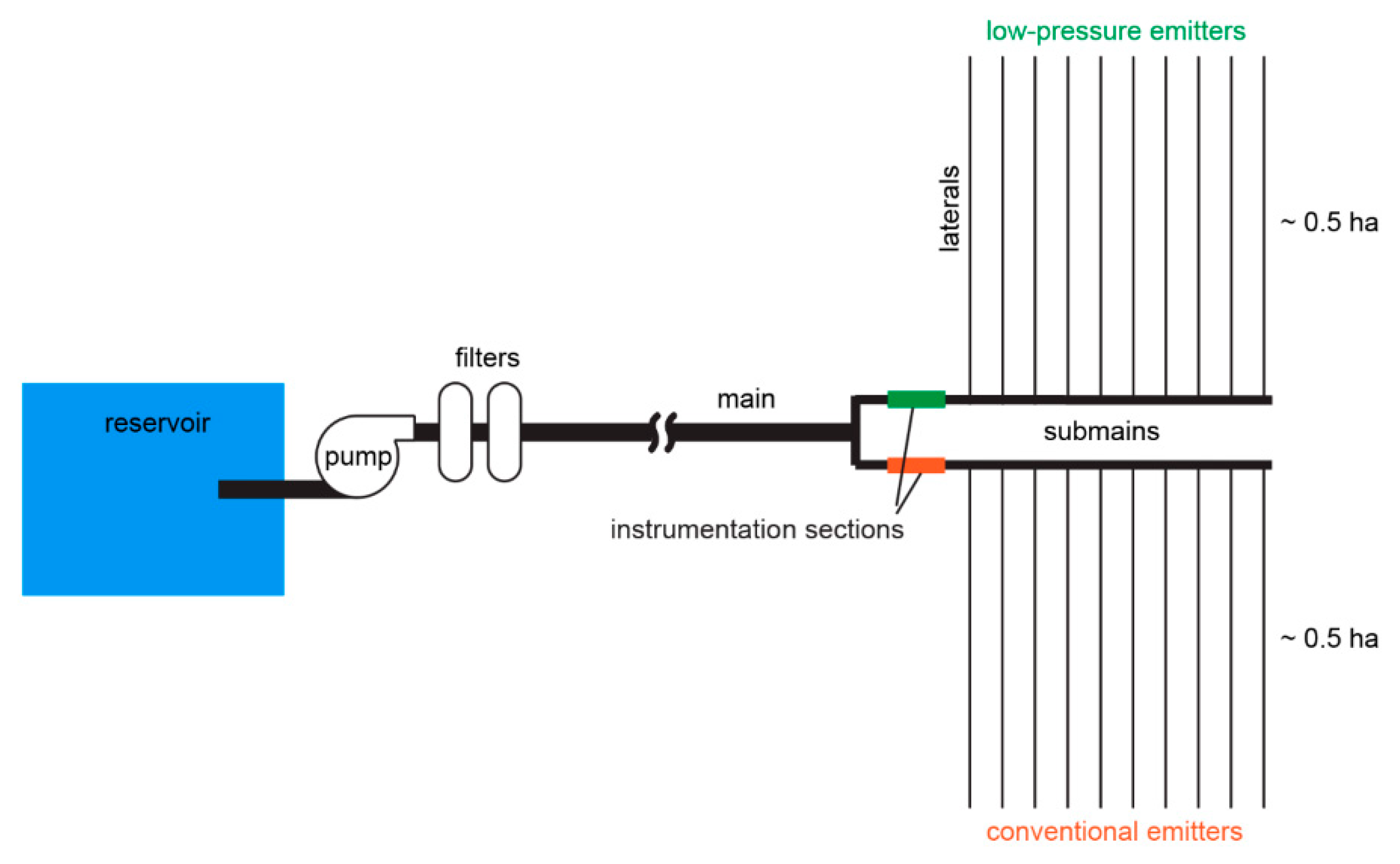
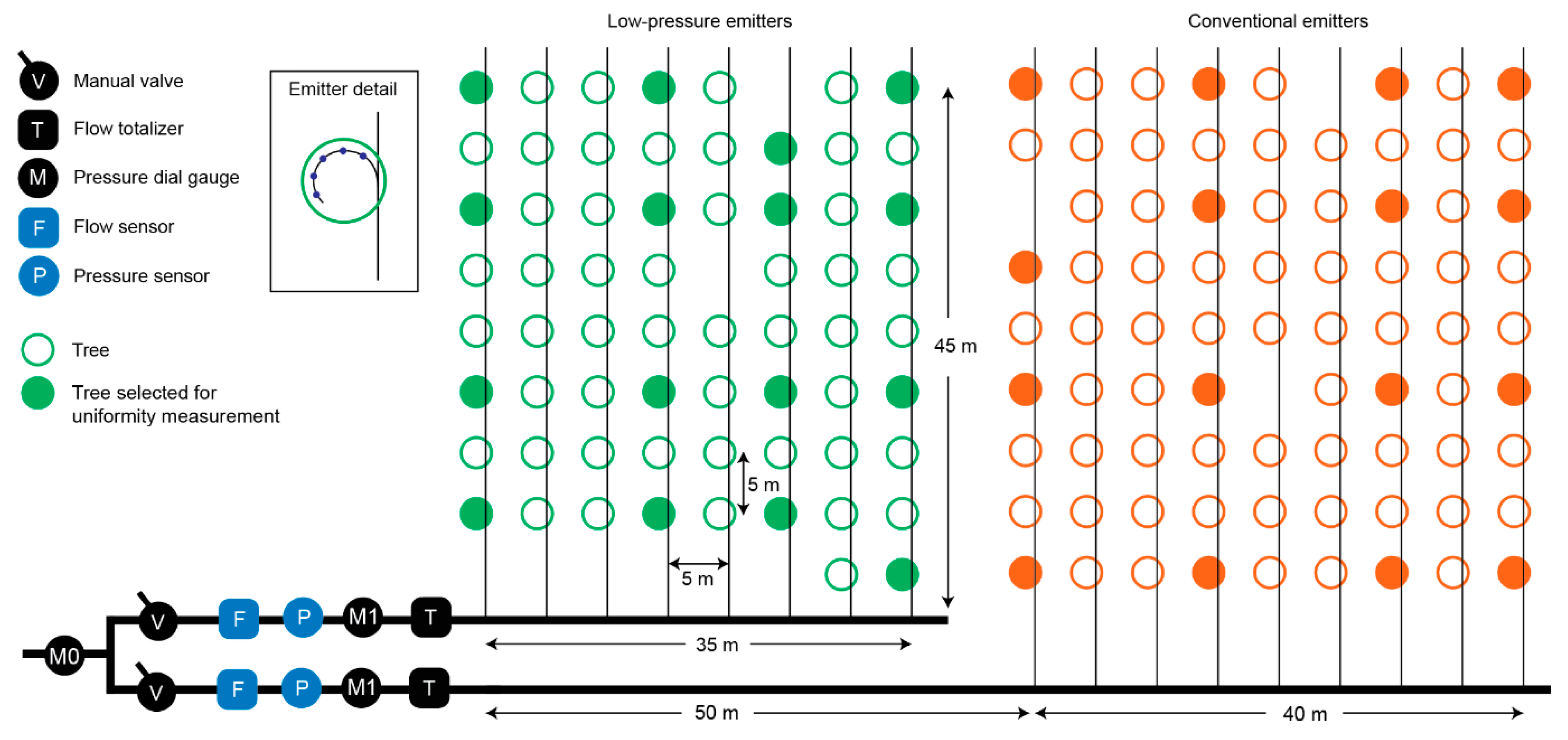
2.2. Experimental Setup for Measurement of Emitter Performance with Freshwater
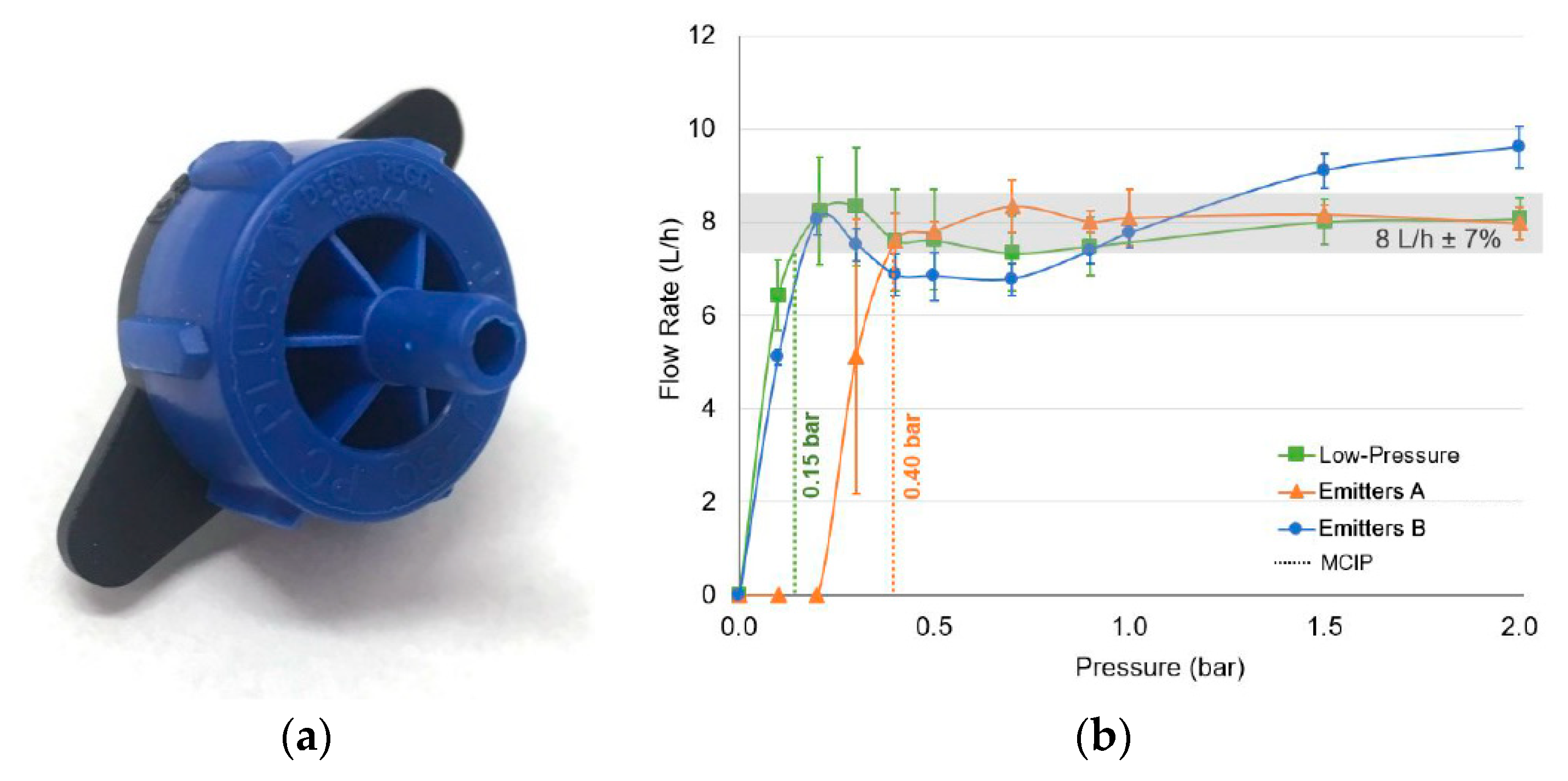
- Conventional emitters: The submain pressure was reduced until the pressure at the end of the farthest lateral was above the minimum emitter operating pressure, as recommended by the emitter manufacturer.
- Low-pressure emitters: The submain pressure was reduced until the average flow rate from the farthest five emitters was within ±10% of the nominal flow rate. Nominal flow rate was deemed an appropriate metric because these emitters are not sold commercially and, hence, do not have a manufacturer-recommended operating pressure.
2.3. Experimental Setup for Measurement of Emitter Performance with Treated Wastewater
2.4. Emitter Characteristics
2.5. Experimental Measurements
2.5.1. Pressure and Flow Sensor Measurements
2.5.2. Uniformity Measurement
2.5.3. Water Quality Measurement
2.6. Data Processing
- Aggregation: Data recorded by the data logger in CSV files and data transmitted to the server were merged, sorted by timestamp, and cleared of duplicates. Irregular time intervals were standardized by resampling all data into 10-minute bins.
- Cleaning: Any data points that were outside the possible range of sensor outputs were excluded. Periods when any of the sensors were not functioning properly due to mechanical failure were also excluded.
- Filtering: The average pressure during each irrigation event was compared to the minimum operating pressure (pset) for the plot (Table 3, column Pressure setting). The average pressure during an irrigation event was estimated using a robust linear least-square fit to a constant, in order to minimize the influence of large pressure spikes during system start-up. Irrigation events during which the average pressure was less than 80% of pset were excluded from further analysis. During such events, operating the emitters at water pressures below setpoint led to flow rates dropping below the nominal flow rate, thus delivering less water than expected at artificially low pressure and hydraulic power.
3. Results
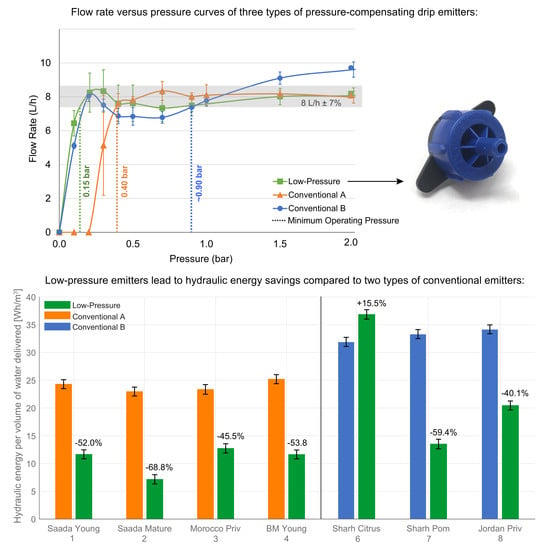
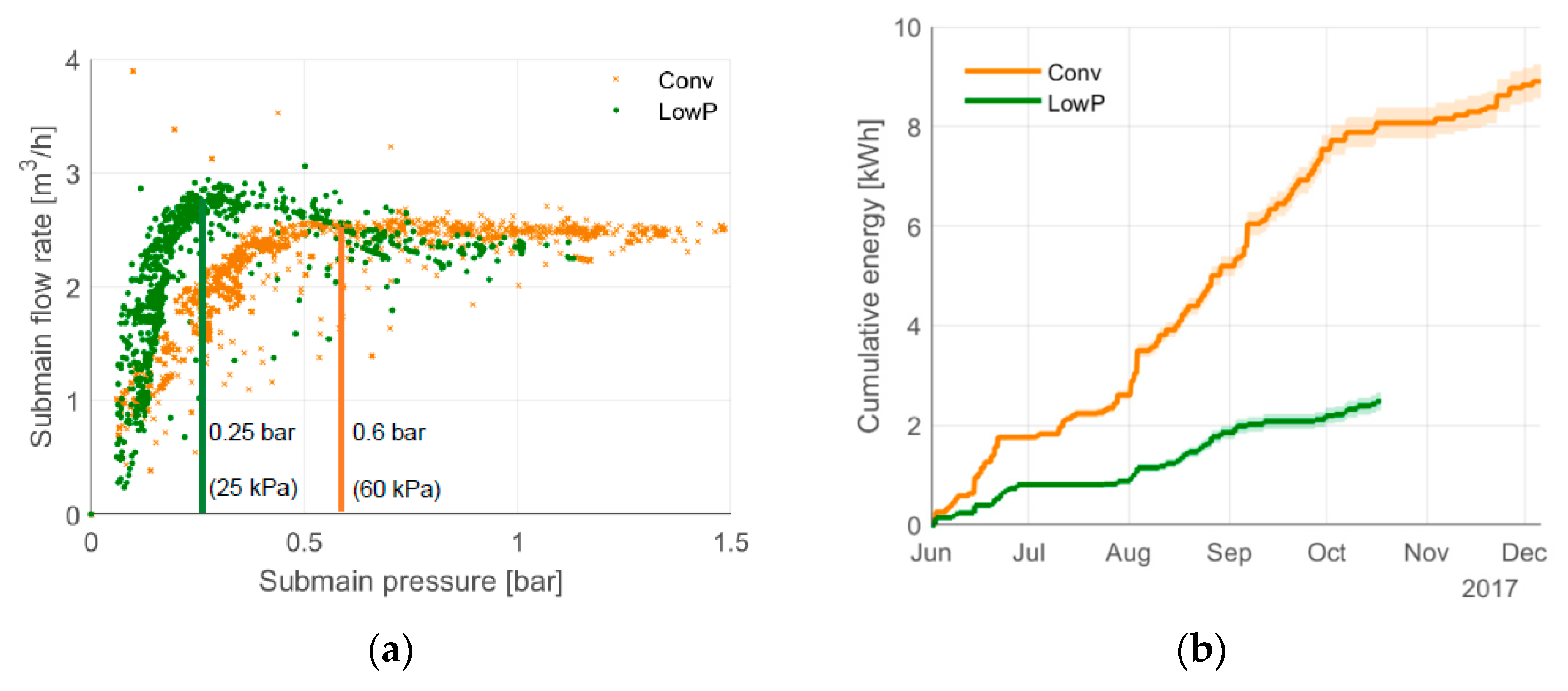
3.1. Flow, Pressure, and Hydraulic Energy
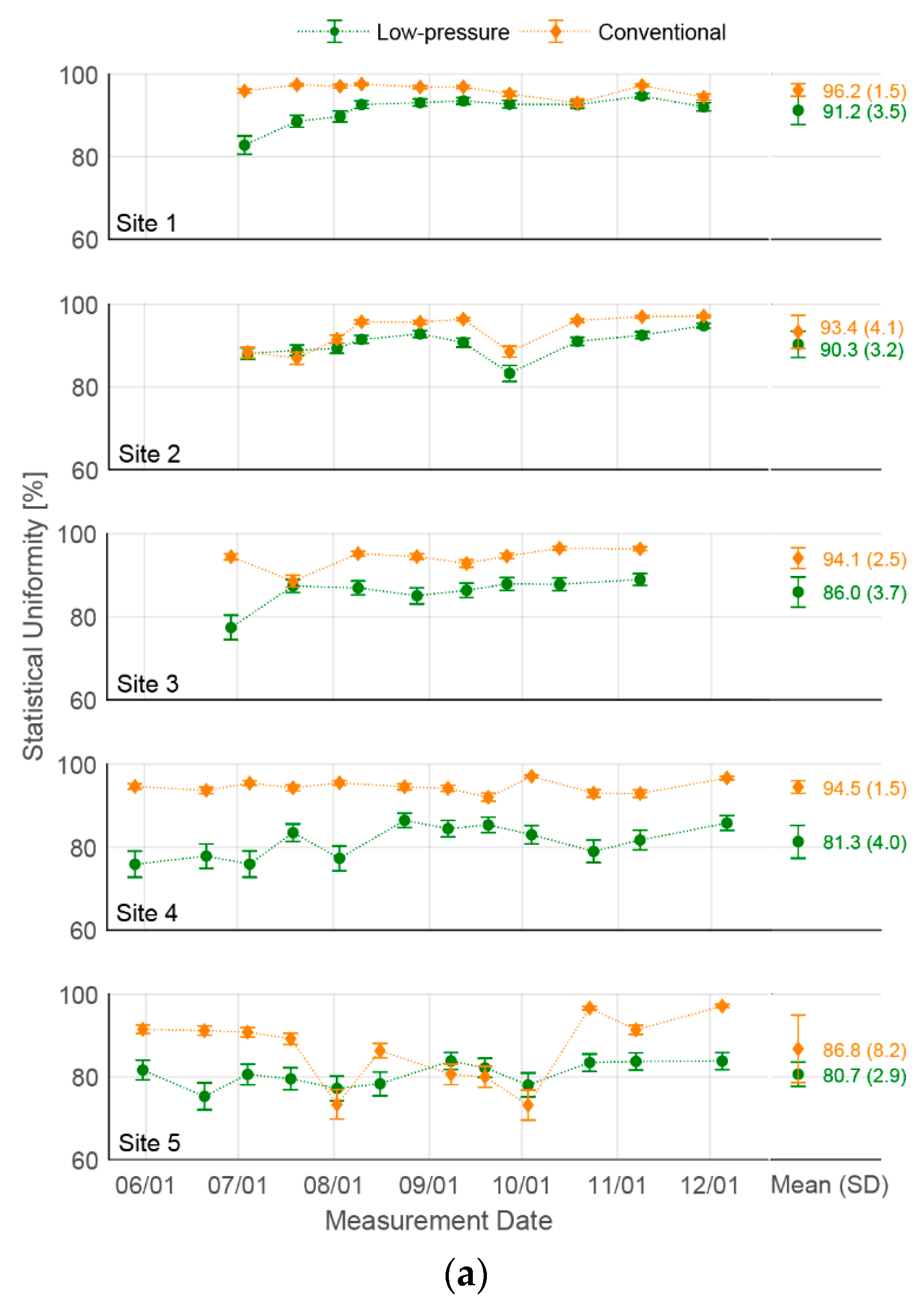
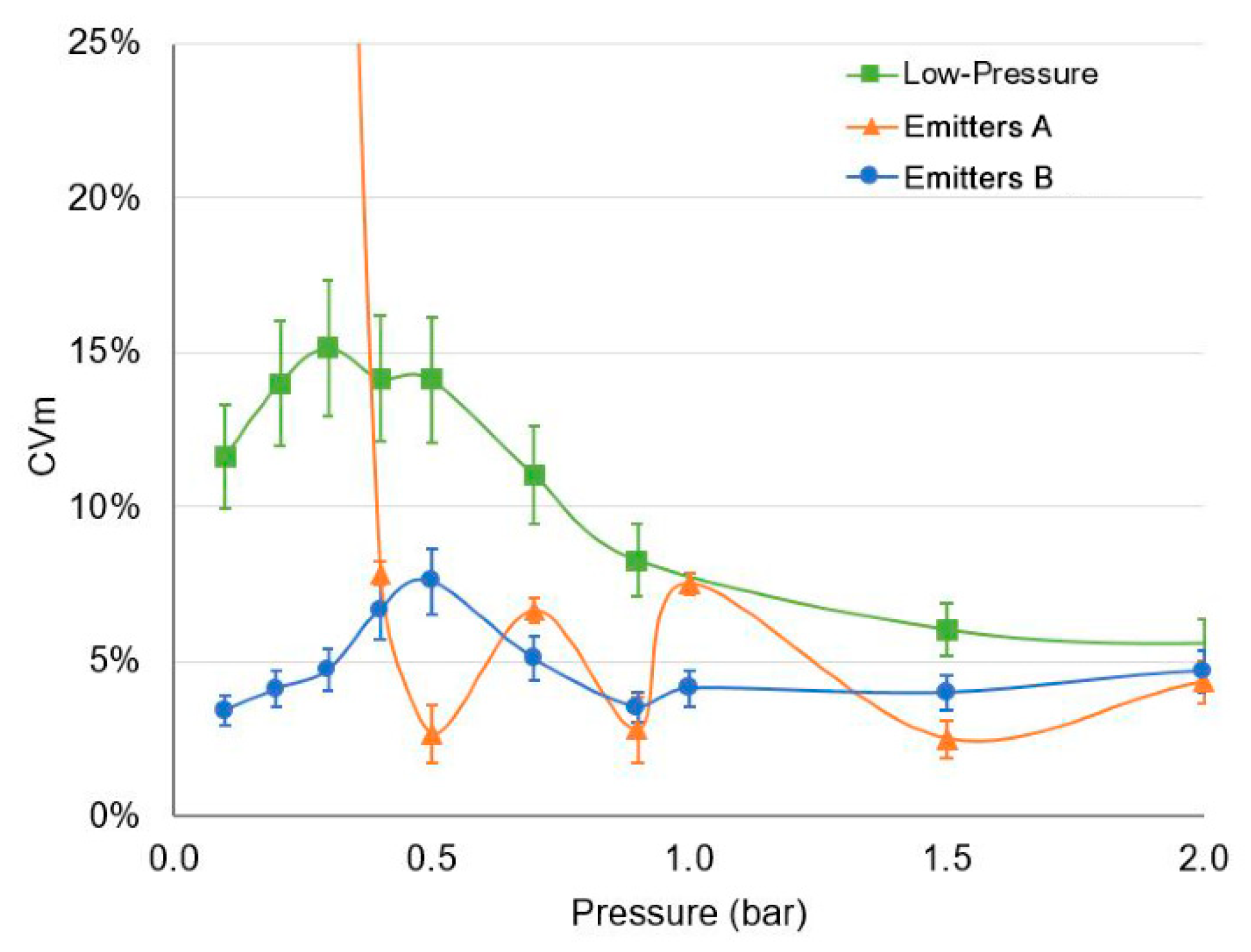
3.2. Uniformity and Clogging
4. Discussion
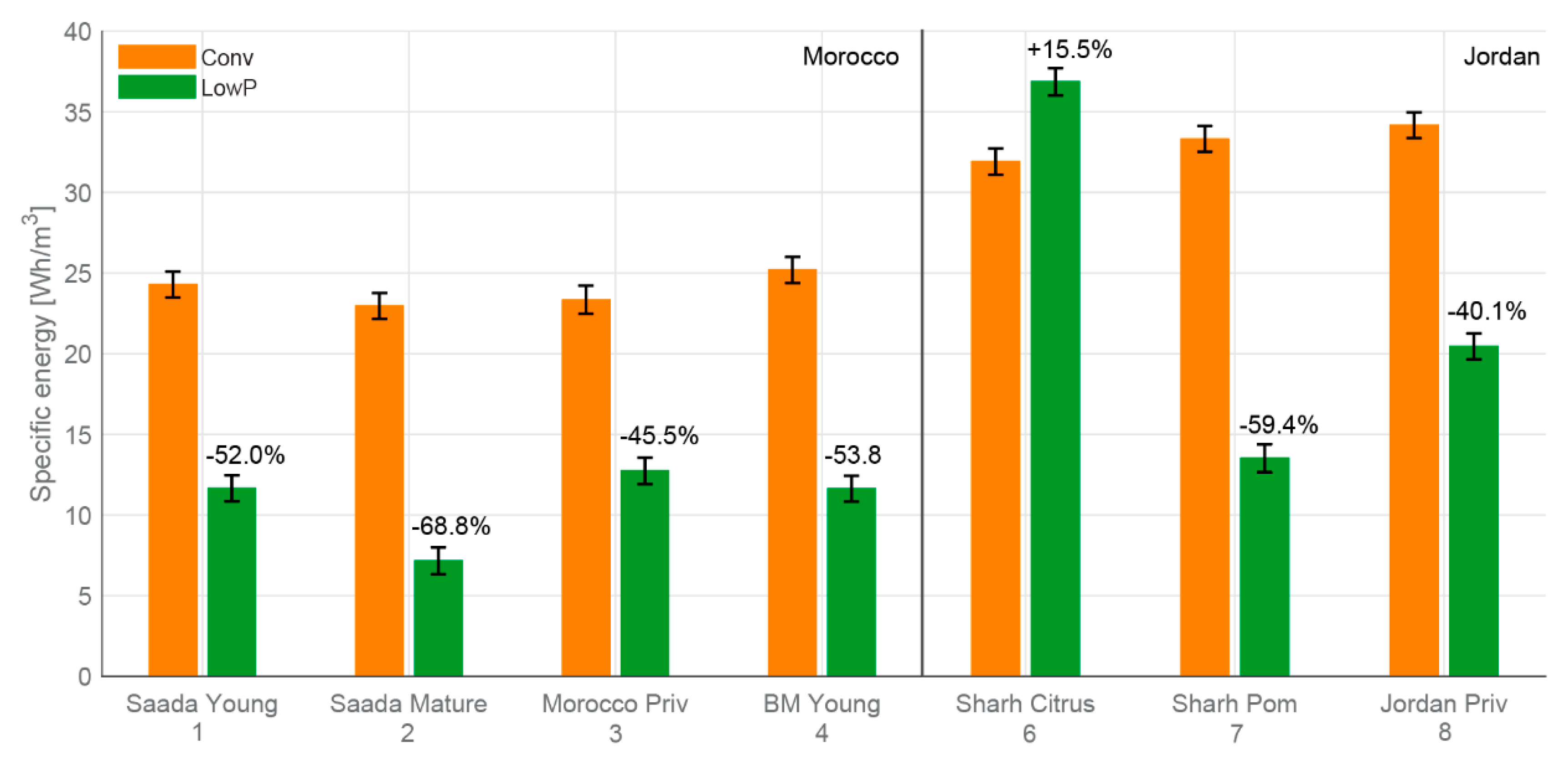
4.1. Energy and Cost Savings
4.2. Uniformity and Clogging
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A. Supplementary Methods
Appendix A.1. Irrigation System Maintenance at Experimental Sites
Appendix A.2. Pressure and Flow Sensor Calibration
Appendix B. Supplementary Tables and Figures



Appendix C. Drip Irrigation System Model Description
Hydraulics Module
Agronomy Module
System Operating Time and Cost Module
References
- FAO (Food and Agriculture Organization of the United Nations). The State of Food and Agriculture: Leveraging Food Systems for Inclusive Rural Transformation; FAO: Rome, Italy, 2017. [Google Scholar]
- Postel, S.; Polak, P.; Gonzales, F.; Keller, J. Drip irrigation for small farmers: A new initiative to alleviate hunger and poverty. Water Int. 2001, 26, 3–13. [Google Scholar] [CrossRef]
- Wang, X.; Huo, Z.; Guan, H.; Guo, P.; Qu, Z. Drip irrigation enhances shallow groundwater contribution to crop water consumption in an arid area. Hydrol. Process. 2018, 32, 747–758. [Google Scholar] [CrossRef]
- Narayanamoorthy, A. State of Adoption of Drip Irrigation for Crops Cultivation in Maharashtra. In Micro Irrigation Systems in India; Viswanathan, P., Kumar, M., Narayanamoorthy, A., Eds.; India Studies in Business and Economics; Springer: Singapore, 2016. [Google Scholar]
- Ibragimov, N.; Evett, S.R.; Esanbekov, Y.; Kamilov, B.S.; Mirzaev, L.; Lamers, J.P.A. Water use efficiency of irrigated cotton in Uzbekistan under drip and furrow irrigation. Agric. Water Manag. 2007, 90, 112–120. [Google Scholar] [CrossRef]
- ICID (International Commission on Irrigation and Drainage). Agricultural Water Management for Sustainable Rural Development: Annual Report 2016–2017; ICID: New Delhi, India, 2017. [Google Scholar]
- Shamshery, P.; Winter, A.G. Shape and form optimization of on-line pressure-compensating drip emitters to achieve lower activation pressure. J. Mech. Des. 2018, 140, 035001. [Google Scholar] [CrossRef]
- Shamshery, P.; Wang, R.-Q.; Tran, D.V.; Winter, A.G. Modeling the future of irrigation: A parametric description of pressure compensating drip irrigation emitter performance. PLoS ONE 2017, 12, e0175241. [Google Scholar] [CrossRef] [PubMed]
- Joffé, G. The Impending Water Crisis in the MENA Region. Int. Spect. 2016, 51, 55–66. [Google Scholar] [CrossRef]
- Tekken, V.; Costa, L.; Kropp, J.P. Increasing pressure, declining water and climate change in north-eastern Morocco. J. Coast. Conserv. 2013, 17, 379–388. [Google Scholar] [CrossRef]
- Alaoui, M. Water sector in Morocco: Situation and perspectives. J. Water Resour. Ocean Sci. 2013, 2, 108–114. [Google Scholar]
- Berrada, A. Assessment of Drip Irrigation in Morocco with Particular Emphasis on the Plain of Tadla; Colorado State University: Fort Collins, CO, USA, 2009. [Google Scholar]
- Kalpakian, J.; Legrouri, A.; Ejekki, F.; Doudou, K.; Ouardaoui, A.; Kettani, D. Obstacles facing the diffusion of drip irrigation technology in the Middle Atlas region of Morocco. Int. J. Environ. Stud. 2014, 71, 63–75. [Google Scholar] [CrossRef]
- MAPM (Ministry of Agriculture, Fisheries, Rural Development, Water and Forests). Maroc Vert. Available online: http://www.agriculture.gov.ma/en/pages/strategy (accessed on 24 January 2017).
- FAO. AQUASTAT Country Fact Sheet: Jordan. Available online: http://www.fao.org/nr/water/aquastat/ data/cf/readPdf.html?f=JOR-CF_eng.pdf (accessed on 1 July 2018).
- Ministry of Water and Irrigation. Jordan Water Sector Facts & Figures; Ministry of Water and Irrigation: Amman, Jordan, 2015.
- USAID (United States Agency for International Development). Water Resources and Environment. Available online: https://www.usaid.gov/jordan/water-and-wastewater-infrastructure (accessed on 1 July 2018).
- Nakayama, F.S.; Bucks, D.A. Water quality in drip/trickle irrigation: A review. Irrig. Sci. 1991, 12, 187–192. [Google Scholar] [CrossRef]
- Harris, I.; Jones, P.D.; Osborn, T.J.; Lister, D.H. Updated high-resolution grids of monthly climatic observations—The CRU TS3.10 Dataset. Int. J. Climatol. 2014, 34, 623–642. [Google Scholar] [CrossRef]
- Meteorological Department of Jordan. Sharhabeel (Wadi El-Rayyan) and Ramtha Stations; Meteorological Department of Jordan: Amman, Jordan, 2019.
- ISO (International Organization for Standardization). ISO Standard 9261:2004(E), Agricultural Irrigation Equipment—Emitters and Emitting Pipe—Specification and Test Methods; ISO: Geneva, Switzerland, 2004. [Google Scholar]
- Lamm, F.R.; Storlie, C.A.; Pitts, D.J. Revision of EP458: Field evaluation of microirrigation systems. In Proceedings of the ASAE Annual International Meeting, American Society of Agricultural Engineers, Minneapolis, MN, USA, 10–14 August 1997. [Google Scholar]
- Bralts, V.F.; Wu, I.-P.; Gitlin, H. Drip Irrigation Uniformity Considering Emitter Plugging. Trans. ASAE 1981, 24, 1234–1240. [Google Scholar] [CrossRef]
- Bralts, V.F. Field Performance and Evaluation. In Trickle Irrigation for Crop Production: Design, Operation, and Management; American Society of Agricultural Engineers: St. Joseph, MI, USA, 1986; pp. 216–240. [Google Scholar]
- United States Salinity Laboratory Staff. Diagnosis and Improvement of Saline and Alkali Soils; US Department of Agriculture: Washington, DC, USA, 1954.
- MathWorks, Inc. MATLAB R2017b. 2017. Available online: https://www.mathworks.com/products/matlab.html (accessed on 1 January 2017).
- Sokol, J.; Sheline, C.; Grant, F.; Winter, A.G. Development of a System Model for Low-Cost, Solar-Powered Drip Irrigation Systems in the MENA Region. In Proceedings of the ASME 2018 International Design Engineering Technical Conferences and Computers and Information in Engineering Conference, Quebec City, QC, Canada, 26–29 August 2018; Volume 2B. [Google Scholar]
- White Box Technologies. Weather Data for Energy Calculations. Available online: http://weather.whiteboxtechnologies.com (accessed on 1 May 2017).
- FAO. Irrigation and Drainage Paper 56: Crop Evapotranspiration-Guidelines for Computing Crop Water Requirements; FAO: Rome, Italy, 1998. [Google Scholar]
- NaanDanJain. Pomegranate. Available online: http://naandanjain.com/solutions/pomegrante/ (accessed on 7 August 2018).
- Pentax Industries. CH Catalogue. 2018. Available online: https://admin.pentax-pumps.it/WebResource/Pentax/Serie/PdfCatalogo/CH_50HZ.pdf (accessed on 1 July 2018).
- Pentax Industries. CS Catalogue. 2018. Available online: https://admin.pentax-pumps.it/WebResource/Pentax/Serie/PdfCatalogo/CS_50HZ.pdf (accessed on 1 July 2018).
- NEPCO. Electricity Tariff in Jordan. Available online: http://www.nepco.com.jo/en/ electricity_tariff_en.aspx (accessed on 1 July 2018).
- Curto, J.D.; Pinto, J.C. The coefficient of variation asymptotic distribution in the case of non-iid random variables. J. Appl. Stat. 2009, 36, 21–32. [Google Scholar] [CrossRef]
- Bralts, V.F.; Wu, I.-P.; Gitlin, H. Manufacturing Variation and Drip Irrigation Uniformity. Trans. ASAE 1981, 24, 113–119. [Google Scholar] [CrossRef]
- Oliver, M.M.H.; Hewa, G.A.; Pezzaniti, D. Thermal variation and pressure compensated emitters. Agric. Water Manag. 2016, 176, 29–39. [Google Scholar] [CrossRef]
- White, F.M. Fluid Mechanics, 7th ed.; McGraw-Hill: New York, NY, USA, 2003. [Google Scholar]
- Swamee, P.K.; Jain, A.K. Explicit equations for pipe flow problems. J. Hydraul. Div. ASCE 1976, 107, 657–664. [Google Scholar]





| Location | Weather | Water Quality | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Country | Site Name | Region | Mean Annual Temp (°C) | Mean Annual Rainfall (mm) | Weather Data Time Period | Water Source | Irrigation Filters | pH | EC (μS/cm) | Ca2+ (meq/L) | Water Analysis Date(s) | Clogging Potential |
| Morocco | Saada Research Station | Marrakech | 19.6 | 273 | 1980–2016 | Canal water | Sand, Disk | 6.8 ± 0.3 | 0.93 ± 0.23 | 4.6 ± 0.7 | 4/18/2017 8/01/2017 9/10/2017 | Moderate |
| Morocco private farm | Marrakech | 19.6 | 273 | 1980–2016 | Groundwater | Sand, Disk | 7.0 ± 0.1 | 3.33 ± 0.40 | 8.2 ± 0.9 | 4/18/2017 8/01/2017 9/10/2017 | Severe | |
| Beni Mellal Research Station | Beni Mellal | 18.0 | 268 | 1970–2007 | Canal water | Sand, Disk | 7.9 ± 0.5 | 0.63 ± 0.23 | 5.6 ± 2.9 | 4/18/2017 8/01/2017 9/10/2017 | Moderate | |
| Jordan | Sharhabeel Research Station | North Jordan Valley | 22.3 | 310 | 1961–2003 | Groundwater & canal mix | Sand, Disk | 8.2 ± 0.2 | 1.23 ± 0.01 | 4.2 ± 0.1 | 6/11/2017 9/17/2017 | Severe |
| Jordan private farm | Al-Mafraq | 17.0 | 133 | 1980–2016 | Groundwater | None | 8.3 | 1.35 | 3.4 | 7/20/2017 | Severe | |
| Ramtha Research Station | Ramtha | 17.4 | 229 | 1961–2003 | Treated wastewater | Sand, Disk | 8.3 ± 0.6 | 2.50 ± 0.18 | 3.8 ± 0.8 | 7/10/2017 9/17/2017 10/04/2017 | Severe | |
| Country | Site Name | Site # | Crop | Emitter Type | Plot Area (ha) | Number of Trees | Number of Emitters | Submain Pressure Setting (kPa) | Measurements Conducted | Sensor Data Logging Period |
|---|---|---|---|---|---|---|---|---|---|---|
| Morocco | Saada Research Station | 1 | Olives, young | Low-pressure | 0.52 | 90 | 360 | 25 | S, U | 6/1/2017–10/18/2017 |
| Conventional (A) | 0.52 | 90 | 360 | 60 | S, U | 6/1/2017–12/20/2017 | ||||
| 2 | Olives, mature | Low-pressure | 0.56 | 78 | 936 | 25 | S, U | 7/20/2017–12/26/2017 | ||
| Conventional (A) | 0.56 | 76 | 912 | 60 | S, U | 9/20/2017–10/23/2017 | ||||
| Morocco private farm | 3 | Olives | Low-pressure | 0.62 | 126 | 840 | 35 | S, U | 6/1/2017–10/1/2017 | |
| Conventional (A) | 0.63 | 142 | 936 | 70 | S, U | 9/18/2017–11/1/2017 | ||||
| Beni Mellal Research Station | 4 | Citrus, young | Low-pressure | 0.76 | 395 | 790 | 35 | U | -- | |
| Conventional (A) | 0.76 | 395 | 790 | 65 | U | -- | ||||
| 5 | Citrus, mature | Low-pressure | 0.63 | 165 | 660 | 25 | S, U | 6/1/2017–12/26/2017 | ||
| Conventional (A) | 0.63 | 160 | 640 | 65 | S, U | 6/1/2017–12/26/2017 | ||||
| Jordan | Sharhabeel Research Station | 6 | Citrus | Low-pressure | 0.16 | 64 | 320 | 25 | S, U | 9/26/2017–12/18/2017 |
| Conventional (B) | 0.18 | 78 | 390 | 120 | S, U | 9/26/2017–12/18/2017 | ||||
| 7 | Pomegranates | Low-pressure | 0.26 | 135 | 675 | 55 | S, U | 6/1/2017–12/26/2017 | ||
| Conventional (B) | 0.28 | 141 | 705 | 120 | S, U | 6/1/2017–12/26/2017 | ||||
| Jordan private farm | 8 | Olives | Low-pressure | 0.40 | 95 | 475 | 25 | S, U | 6/1/2017–12/26/2017 | |
| Conventional (B) | 0.40 | 103 | 515 | 140 | S, U | 6/1/2017–12/26/2017 | ||||
| Ramtha Research Station | 9 | N/A | Low-pressure | 0.09 | 0 | 640 | 25, 50, 100 | S, U | 9/27/2017–12/18/2017 | |
| Conventional (B) | 0.09 | 0 | 640 | 50, 100 | S, U | 9/27/2017–12/18/2017 |
| Country | Site Name | Site # | Emitter Type | Total Num. of Irrigation Events | Filtered Num. of Irrigation Events | Pressure Setting (kPa) | Mean Pressure (kPa) | Mean Flow Rate (m3/h) | Total Volume Delivered (m3) | Total Hydraulic Energy (kWh) | Specific Hydraulic Energy (Wh/m3) | % Difference in Specific Hydraulic Energy |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Morocco | Saada Research Station | 1 | Low-pressure | 88 | 43 | 25 | 43 ± 27 | 2.51 ± 0.45 | 212.60 ± 0.66 | 2.48 ± 0.18 | 11.66 ± 0.81 | −52.0 ± 5.0% |
| Conventional (A) | 109 | 81 | 60 | 91 ± 34 | 2.46 ± 0.37 | 366.54 ± 0.25 | 8.91 ± 0.34 | 24.30 ± 0.80 | ||||
| 2 | Low-pressure | 15 | 4 | 25 | 26 ± 10 | 7.88 ± 1.78 | 46.83 ± 0.16 | 0.34 ± 0.04 | 7.16 ± 0.84 | −68.8 ± 5.6% | ||
| Conventional (A) | 91 | 48 | 60 | 86 ± 28 | 6.58 ± 1.33 | 691.57 ± 0.55 | 15.88 ± 0.64 | 22.96 ± 0.80 | ||||
| Morocco private farm | 3 | Low-pressure | 8 | 6 | 35 | 44 ± 19 | 6.84 ± 1.46 | 101.97 ± 0.30 | 1.30 ± 0.09 | 12.73 ± 0.83 | −45.5 ± 5.4% | |
| Conventional (A) | 44 | 14 | 70 | 78 ± 30 | 7.64 ± 2.29 | 240.19 ± 0.48 | 5.61 ± 0.24 | 23.35 ± 0.87 | ||||
| Beni Mellal Research Station | 4 | Low-pressure | 41 | 34 | 35 | 44 ± 26 | 5.43 ± 0.57 | 898.90 ± 0.57 | 10.46 ± 0.75 | 11.63 ± 0.80 | −53.8 ± 4.9% | |
| Conventional (A) | 39 | 25 | 65 | 93 ± 34 | 5.48 ± 0.93 | 636.83 ± 0.48 | 16.04 ± 0.61 | 25.19 ± 0.82 | ||||
| Jordan | Sharhabeel Research Station | 6 | Low-pressure | 52 | 49 | 25 | 131 ± 65 | 3.30 ± 1.09 | 395.53 ± 0.31 | 14.58 ± 0.45 | 36.86 ± 0.85 | +15.5 ± 3.7% |
| Conventional (B) | 36 | 12 | 120 | 117 ± 34 | 3.38 ± 0.74 | 82.12 ± 0.14 | 2.62 ± 0.09 | 31.91 ± 0.82 | ||||
| 7 | Low-pressure | 83 | 55 | 55 | 47 ± 33 | 5.85 ± 1.13 | 674.24 ± 0.51 | 9.12 ± 0.61 | 13.52 ± 0.86 | −59.4 ± 3.8% | ||
| Conventional (B) | 82 | 28 | 120 | 124 ± 35 | 5.71 ± 1.10 | 270.22 ± 0.32 | 9.00 ± 0.28 | 33.31 ± 0.80 | ||||
| Jordan private farm | 8 | Low-pressure | 92 | 79 | 25 | 76 ± 46 | 4.46 ± 0.83 | 389.49 ± 0.34 | 7.97 ± 0.35 | 20.46 ± 0.80 | −40.1 ± 3.4% | |
| Conventional (B) | 53 | 15 | 140 | 128 ± 47 | 3.41 ± 1.55 | 147.75 ± 0.20 | 5.05 ± 0.16 | 34.17 ± 0.80 |
| Case | Emitters | Emitter Price US$ | Pump Model | Pump Size kW (HP) | Pump Price US$ |
|---|---|---|---|---|---|
| 1 | Conventional (B) | 0.07 | Pentax CH-310 | 2.2 (3.0) | 350 |
| 2 | Conventional (A) | 0.15 | Pentax CH-210 | 1.5 (2.0) | 310 |
| 3 | Low-pressure | 0.06 | Pentax CSB-150/2 | 1.1 (1.5) | 290 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sokol, J.; Amrose, S.; Nangia, V.; Talozi, S.; Brownell, E.; Montanaro, G.; Abu Naser, K.; Bany Mustafa, K.; Bahri, A.; Bouazzama, B.; et al. Energy Reduction and Uniformity of Low-Pressure Online Drip Irrigation Emitters in Field Tests. Water 2019, 11, 1195. https://doi.org/10.3390/w11061195
Sokol J, Amrose S, Nangia V, Talozi S, Brownell E, Montanaro G, Abu Naser K, Bany Mustafa K, Bahri A, Bouazzama B, et al. Energy Reduction and Uniformity of Low-Pressure Online Drip Irrigation Emitters in Field Tests. Water. 2019; 11(6):1195. https://doi.org/10.3390/w11061195
Chicago/Turabian StyleSokol, Julia, Susan Amrose, Vinay Nangia, Samer Talozi, Elizabeth Brownell, Gianni Montanaro, Khaled Abu Naser, Khalil Bany Mustafa, Abdeljabar Bahri, Bassou Bouazzama, and et al. 2019. "Energy Reduction and Uniformity of Low-Pressure Online Drip Irrigation Emitters in Field Tests" Water 11, no. 6: 1195. https://doi.org/10.3390/w11061195
APA StyleSokol, J., Amrose, S., Nangia, V., Talozi, S., Brownell, E., Montanaro, G., Abu Naser, K., Bany Mustafa, K., Bahri, A., Bouazzama, B., Bouizgaren, A., Mazahrih, N., Moussadek, R., Sikaoui, L., & Winter, A. G. (2019). Energy Reduction and Uniformity of Low-Pressure Online Drip Irrigation Emitters in Field Tests. Water, 11(6), 1195. https://doi.org/10.3390/w11061195