Drought in the Upper Hron Region (Slovakia) between the Years 1984–2014
Abstract
:1. Introduction
- Find out the trends of SPI and SPEI along the studied area’s altitudinal gradient.
- Detect possible abrupt changes in temporal trends of the SPI and SPEI.
- Analyze the trends of the above indices in individual months.
- Try to spatially identify drought-prone areas based on the SPI and SPEI time series’ temporal evolution.
2. Materials and Methods
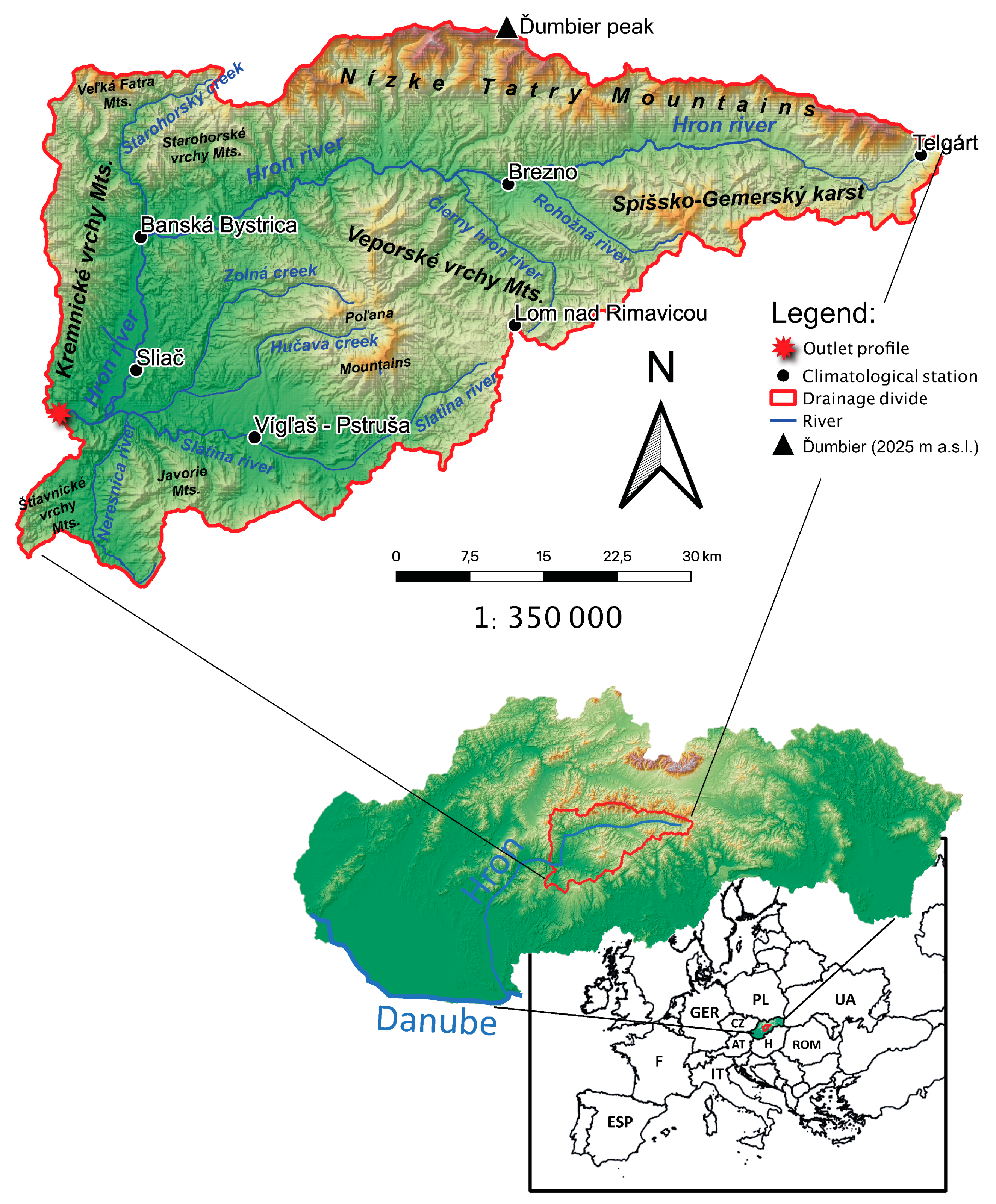
2.1. Study Area
2.2. Climate of the Area
2.3. Climate Data
2.4. Drought Indices
2.4.1. Standardised Precipitation Index (SPI)
2.4.2. Standardised Precipitation Evapotranspiration Index (SPEI)
2.5. Trend Analyses
- (i)
- Mann–Kendall trend test (MK) and
- (ii)
- Cumulative sum of Rank Difference test (CRD).
- significance level set to 5%
- time scale representing a moving average of 60 (60 months = 5 years)
- the initial block set to 10
- number of Monte Carlo runs for resampling set to 1000 (default setting)
2.6. Spatial Identification of the Drought-Prone Area
3. Results
3.1. Trends of SPI and SPEI within the Period 1984–2014
3.1.1. Trends of the SPI within the Studied Period
3.1.2. Trends of the SPEI within the Studied Period
3.2. Detection of Abrupt Changes in Temporal Trends of SPI and SPEI within the Period 1984–2014
3.3. Trend Analyses of SPI and SPEI for Individual Months in the Period 1984–2014
3.4. Spatial Identification of the Drought-Prone Areas
4. Discussion
Author Contributions
Funding
Conflicts of Interest
References
- Lindner, M.; Fitzgerald, J.B.; Zimmermann, N.E.; Reyer, C.; Delzon, S.; Van Der Maaten, E.; Schelhaas, M.-J.; Lasch, P.; Eggers, J.; Van Der Maaten-Theunissen, M.; et al. Climate change and European forests: What do we know, what are the uncertainties, and what are the implications for forest management? J. Environ. Manag. 2014, 146, 69–83. [Google Scholar] [CrossRef] [Green Version]
- Andrade, C.; Leite, S.M.; Santos, J.A. Temperature extremes in Europe: Overview of their driving atmospheric patterns. Nat. Hazards Earth Syst. Sci. 2012, 12, 1671–1691. [Google Scholar] [CrossRef] [Green Version]
- Blauhut, V.; Stahl, K.; Stagge, J.H.; Tallaksen, L.M.; De Stefano, L.; Vogt, J.V. Estimating drought risk across Europe from reported drought impacts, drought indices, and vulnerability factors. Hydrol. Earth Syst. Sci. 2016, 20, 2779–2800. [Google Scholar] [CrossRef] [Green Version]
- Gobiet, A.; Kotlarski, S.; Beniston, M.; Heinrich, G.; Rajczak, J.; Stoffel, M. 21st century climate change in the European Alps—A review. Sci. Total. Environ. 2014, 493, 1138–1151. [Google Scholar] [CrossRef]
- Ruffault, J.; Martin-StPaul, N.K.; Duffet, C.; Goge, F.; Mouillot, F. Projecting future drought in Mediterranean forests: Bias correction of climate models matters! Theor. Appl. Clim. 2013, 117, 113–122. [Google Scholar] [CrossRef]
- Stagge, J.H.; Kingston, D.G.; Tallaksen, L.M.; Hannah, D.M. Observed drought indices show increasing divergence across Europe. Sci. Rep. 2017, 7, 14045. [Google Scholar] [CrossRef] [PubMed]
- Vicente-Serrano, S.M.; Lopez-Moreno, J.-I.; Beguería, S.; Lorenzo-Lacruz, J.; Sanchez-Lorenzo, A.; García-Ruiz, J.M.; Azorin-Molina, C.; Morán-Tejeda, E.; Revuelto, J.; Trigo, R.; et al. Evidence of increasing drought severity caused by temperature rise in southern Europe. Environ. Res. Lett. 2014, 9, 044001. [Google Scholar] [CrossRef]
- Trnka, M.; Balek, J.; Štěpánek, P.; Zahradníček, P.; Možný, M.; Eitzinger, J.; Žalud, Z.; Formayer, H.; Turňa, M.; Nejedlík, P.; et al. Drought trends over part of Central Europe between 1961 and 2014. Clim. Res. 2016, 70, 143–160. [Google Scholar] [CrossRef] [Green Version]
- Spinoni, J.; Naumann, G.; Vogt, J. Spatio-temporal seasonal drought patterns in Europe from 1950 to 2015. EGU Gen. Assem. 2016, 18, 12268. [Google Scholar]
- Alfieri, L.; Burek, P.; Feyen, L.; Forzieri, G. Global warming increases the frequency of river floods in Europe. Hydrol. Earth Syst. Sci. 2015, 19, 2247–2260. [Google Scholar] [CrossRef] [Green Version]
- Dai, A. Increasing drought under global warming in observations and models. Nat. Clim. Chang. 2012, 3, 52–58. [Google Scholar] [CrossRef]
- Twardosz, R.; Cezak, U.K. Thermal anomalies in the Mediterranean and in Asia Minor (1951–2010). Int. J. Glob. Warm. 2019, 18, 304. [Google Scholar] [CrossRef]
- Vicente-Serrano, S.M.; García-Herrera, R.; Barriopedro, D.; Azorin-Molina, C.; López-Moreno, J.I.; Martín-Hernández, N.; Tomás-Burguera, M.; Gimeno, L.; Nieto, R. The Westerly Index as complementary indicator of the North Atlantic oscillation in explaining drought variability across Europe. Clim. Dyn. 2015, 47, 845–863. [Google Scholar] [CrossRef] [Green Version]
- Vido, J.; Nalevanková, P.; Valach, J.; Šustek, Z.; Tadesse, T. Drought Analyses of the Horné Požitavie Region (Slovakia) in the Period 1966–2013. Adv. Meteorol. 2019, 2019, 1–10. [Google Scholar] [CrossRef]
- Škvarenina, J.; Tomlain, J.; Hrvol’, J.; Škvareninová, J.; Nejedlík, P. Progress in dryness and wetness parameters in altitudinal vegetation stages of West Carpathians: Time-series analysis 1951–2007. Idojaras 2009, 113, 47–54. [Google Scholar]
- Miklós, L.; Hrnčiarová, T. Atlas Krajiny Slovenskej Republiky [Landscape Atlas of the Slovak Republic]; Ministry of Environment of the Slovak Republic, Slovak Agency of Environment: Basnská Bystrica, Slovak, 2002. [Google Scholar]
- Zeleňáková, M.; Purcz, P.; Blišťan, P.; Vranayová, Z.; Hlavatá, H.; Diaconu, D.C.; Portela, M.M. Trends in Precipitation and Temperatures in Eastern Slovakia (1962–2014). Water 2018, 10, 727. [Google Scholar] [CrossRef] [Green Version]
- Bartholy, J.; Pongrácz, R.; Gelybó, G.; Kern, A. What Climate Can We Expect in Central/Eastern Europe by 2071–2100? BT-Bioclimatology and Natural Hazards. In Bioclimatology and Natural Hazards; Střelcová, K., Mátyás, C., Kleidon, A., Lapin, M., Matejka, F., Blaženec, M., Škvarenina, J., Holécy, J., Eds.; Springer: Dordrecht, The Netherlands, 2009; pp. 3–14. [Google Scholar]
- Škvarenina, J.; Tomlain, J.; Hrvol’, J.; Škvareninová, J. Occurrence of Dry and Wet Periods in Altitudinal Vegetation Stages of West Carpathians in Slovakia: Time-Series Analysis 1951–2005 BT-Bioclimatology and Natural Hazards. In Bioclimatology and Natural Hazards; Střelcová, K., Mátyás, C., Kleidon, A., Lapin, M., Matejka, F., Blaženec, M., Škvarenina, J., Holécy, J., Eds.; Springer: Dordrecht, The Netherlands, 2009; pp. 97–106. [Google Scholar]
- Melo, M.; Lapin, M.; Kapolková, H.; Pecho, J.; Kružicová, A. Climate Trends in the Slovak Part of the Carpathians BT-The Carpathians: Integrating Nature and Society Towards Sustainability. In Bioclimatology and Natural Hazards; Kozak, J., Ostapowicz, K., Bytnerowicz, A., Wyżga, B., Eds.; Springer: Berlin/Heidelberg, Germany, 2013; pp. 131–150. [Google Scholar]
- Melo, M.; Lapin, M.; Damborská, I. Shifts in Climatic Regions in Mountain Parts of Slovakia. Sustain. Dev. Bioclimate 2009, 42–43. [Google Scholar] [CrossRef] [Green Version]
- Zlatník, A. Lesnícka Fytológia Forestry Phytology; SZN: Praha, Czech Republic, 1976. [Google Scholar]
- Vilček, J.; Škvarenina, J.; Vido, J.; Nalevanková, P.; Kandrík, R.; Škvareninová, J. Minimal change of thermal continentality in Slovakia within the period 1961–2013. Earth Syst. Dyn. 2016, 7, 735–744. [Google Scholar] [CrossRef] [Green Version]
- Zeleňáková, M.; Vido, J.; Portela, M.C.A.S.; Purcz, P.; Blišťan, P.; Hlavatá, H.; Hluštík, P. Precipitation Trends over Slovakia in the Period 1981–2013. Water 2017, 9, 922. [Google Scholar] [CrossRef] [Green Version]
- Briedoň, V. Ein Beitrag zum Problem der Niederschlagesabhängigkeit von der Seehohe im Tschechoslowakischen Karpatengebiet. In Príspevok k Meteorológii Karpát Contribution to Carpathian Meteorology; Konček, M., Ed.; Slovenská Akadémia Vied Slovak Academy of Sciences: Bratislava, Slovakia, 1961; pp. 212–220. [Google Scholar]
- Bochníček, O. Klimatický atlas Slovenska Climate atlas of Slovakia; Slovenský Hydrometeorologický ústav Slovak Hydrometeorological Institue: Bratislava, Slovakia, 2015. [Google Scholar]
- Škvarenina, J.; Vido, J.; Minďaš, J.; Střelcová, K.; Škvareninová, J.; Fleischer, P.; Bošeľa, M. Globálne zmeny klímy a lesné Ekosystémy Climate Change and Forest Ecosystems; Technická univerzita vo Zvolene Technical University in Zvolen: Zvolen, Slovakia, 2018. [Google Scholar]
- SHMI. Report Containing Additional In-Formation with Respect to the Implementation of the GCOS Plan, Following the Established Reporting Guidelines FCCC/SBSTA/2007/L.14; Slovak Hydrometeorological Institute: Bratislava, Slovakia, 2008; p. 210. [Google Scholar]
- Vido, J.; Střelcová, K.; Nalevanková, P.; Leštianska, A.; Kandrík, R.; Pástorová, A.; Škvarenina, J.; Tadesse, T. Identifying the relationships of climate and physiological responses of a beech forest using the Standardised Precipitation Index: A case study for Slovakia. J. Hydrol. Hydromech. 2016, 64, 246–251. [Google Scholar] [CrossRef] [Green Version]
- Vido, J.; Tadesse, T.; Šustek, Z.; Kandrík, R.; Hanzelová, M.; Škvarenina, J.; Škvareninová, J.; Hayes, M. Drought Occurrence in Central European Mountainous Region (Tatra National Park, Slovakia) within the Period 1961–2010. Adv. Meteorol. 2015, 2015, 1–8. [Google Scholar] [CrossRef] [Green Version]
- Šustek, Z.; Vido, J.; Škvareninová, J.; Skvarenina, J.; Surda, P. Drought impact on ground beetle assemblages (Coleoptera, Carabidae) in Norway spruce forests with different management after windstorm damage—A case study from Tatra Mts. (Slovakia). J. Hydrol. Hydromech. 2017, 65, 333–342. [Google Scholar] [CrossRef] [Green Version]
- McKee, T.B.; Doesken, N.J.; Kleist, J. The relationship of drought frequency and duration to time scales. In Proceedings of the 8th Conference on Applied Climatology, Anaheim, CA, USA, 17–22 January 1993; pp. 179–183. [Google Scholar]
- Edwards, D.C. Characteristics of 20th Century Drought in the United States at Multiple Time Scales; Climatology Report No. 97-2. Atmospheric Science Paper No. 634; Colorado State University: Fort Collins, CO, USA, 1997; p. 155. 155p. [Google Scholar]
- Hayes, M.J.; Svoboda, M.D.; Wilhite, D.A.; Vanyarkho, O.V. Monitoring the 1996 Drought Using the Standardized Precipitation Index. Bull. Am. Meteorol. Soc. 1999, 80, 430–438. Available online: http://digitalcommons.unl.edu/droughtfacpubhttp://digitalcommons.unl.edu/droughtfacpub/31 (accessed on 6 June 2020). [CrossRef] [Green Version]
- Bak, B.; Labedzki, L. Assessing drought severity with the relative precipitation index [RPI] and the standardised precipitation index [SPI]. J. Water Land Dev. 2002, 6, 89–105. [Google Scholar]
- Tirivarombo, S.; Osupile, D.; Eliasson, P. Drought monitoring and analysis: Standardised Precipitation Evapotranspiration Index (SPEI) and Standardised Precipitation Index (SPI). Phys. Chem. Earth, Parts A/B/C 2018, 106, 1–10. [Google Scholar] [CrossRef]
- Heim, R.R. A Review of Twentieth-Century Drought Indices Used in the United States. Bull. Am. Meteorol. Soc. 2002, 83, 1149–1166. [Google Scholar] [CrossRef] [Green Version]
- Vicente-Serrano, S.M.; Beguería, S.; López-Moreno, J.I. A Multiscalar Drought Index Sensitive to Global Warming: The Standardized Precipitation Evapotranspiration Index. J. Clim. 2010, 23, 1696–1718. [Google Scholar] [CrossRef] [Green Version]
- Thornthwaite, C.W. An approach toward a rational classification of climate. Geogr. Rev. 1948, 38, 55–94. [Google Scholar] [CrossRef]
- Yue, S.; Wang, C. The Mann-Kendall Test Modified by Effective Sample Size to Detect Trend in Serially Correlated Hydrological Series. Water Resour. Manag. 2004, 18, 201–218. [Google Scholar] [CrossRef]
- Onyutha, C. Influence of Hydrological Model Selection on Simulation of Moderate and Extreme Flow Events: A Case Study of the Blue Nile Basin. Adv. Meteorol. 2016, 2016, 1–28. [Google Scholar] [CrossRef] [Green Version]
- Onyutha, C. Statistical Uncertainty in Hydrometeorological Trend Analyses. Adv. Meteorol. 2016, 2016, 1–26. [Google Scholar] [CrossRef] [Green Version]
- Gudmundsson, L.; I Seneviratne, S. Anthropogenic climate change affects meteorological drought risk in Europe. Environ. Res. Lett. 2016, 11, 044005. [Google Scholar] [CrossRef]
- Lapin, M.; Gera, M.; Hrvol’, J.; Melo, M.; Tomlain, J. Possible impacts of climate change on hydrologic cycle in Slovakia and results of observations in 1951–2007. Biologia 2009, 64, 454–459. [Google Scholar] [CrossRef]
- Ionita, M.; Tallaksen, L.M.; Kingston, D.G.; Stagge, J.H.; Laaha, G.; Van Lanen, H.A.J.; Scholz, P.; Chelcea, S.M.; Haslinger, K. The European 2015 drought from a climatological perspective. Hydrol. Earth Syst. Sci. 2017, 21, 1397–1419. [Google Scholar] [CrossRef] [Green Version]
- Hríbik, M.; Majlingová, A.; Škvarenina, J.; Kyselová, D. Winter Snow Supply in Small Mountain Watershed as a Potential Hazard of Spring Flood Formation BT-Bioclimatology and Natural Hazards. In Bioclimatology and Natural Hazards; Střelcová, K., Mátyás, C., Kleidon, A., Lapin, M., Matejka, F., Blaženec, M., Škvarenina, J., Holécy, J., Eds.; Springer: Dordrecht, The Netherlands, 2009; pp. 119–128. [Google Scholar]
- Sleziak, P.; Szolgay, J.; Hlavčová, K.; Parajka, J. The Impact of the Variability of Precipitation and Temperatures on the Efficiency of a Conceptual Rainfall-Runoff Model. Slovak J. Civ. Eng. 2016, 24, 1–7. [Google Scholar] [CrossRef] [Green Version]
- Marzen, M.; Iserloh, T.; De Lima, J.L.; Fister, W.; Ries, J.B. Impact of severe rain storms on soil erosion: Experimental evaluation of wind-driven rain and its implications for natural hazard management. Sci. Total. Environ. 2017, 590–591, 502–513. [Google Scholar] [CrossRef]
- Hlavčová, K.; Kohnová, S.; Borga, M.; Horvát, O.; Šťastný, P.; Pekárová, P.; Majerčáková, O.; Danáčová, Z. Post-event analysis and flash flood hydrology in Slovakia. J. Hydrol. Hydromech. 2016, 64, 304–315. [Google Scholar] [CrossRef] [Green Version]
- Millan, M.M. Extreme hydrometeorological events and climate change predictions in Europe. J. Hydrol. 2014, 518, 206–224. [Google Scholar] [CrossRef]
- Šiška, B.; Takáč, J. Drought analyses of agricultural regions as influenced by climatic conditions in the Slovak Republic. Idojárás 2009, 13, 135–143. [Google Scholar]
- Střelcová, K.; Kučera, J.; Fleischer, P.; Giorgi, S.; Gömöryová, E.; Škvarenina, J.; Ditmarová, L. Canopy transpiration of mountain mixed forest as a function of environmental conditions in boundary layer. Biologia 2009, 64, 507–511. [Google Scholar] [CrossRef] [Green Version]
- Solberg, S. Summer drought: A driver for crown condition and mortality of Norway spruce in Norway. For. Pathol. 2004, 34, 93–104. [Google Scholar] [CrossRef]
- Ďurský, J.; Škvarenina, J.; Minďáš, J.; Miková, A. Regional analysis of climate change impact on Norway spruce (Picea abies L. Karst.) growth in Slovak mountain forests. J. For. Sci. 2006, 52, 306–315. Available online: http://www.scopus.com/inward/record.url?eid=2-s2.0-33746599991&partnerID=40 (accessed on 6 June 2020).







| Station Name | Jan. | Feb. | Mar. | Apr. | May | Jun. | Jul. | Aug. | Sep. | Oct. | Nov. | Dec. | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Sliač | WMO index 11903; | Altitude 313 m a.s.l.; | Latitude [φ] 48°38′33″; | Longitude [λ] 19°08′31″ | |||||||||
| Precipitation [mm] | 44.7 | 41.6 | 42.5 | 47.7 | 65.2 | 83.5 | 72.6 | 67.5 | 54.7 | 54.0 | 63.8 | 56.0 | |
| Temperature [°C] | −3.3 | −1.2 | 3.3 | 9.1 | 14.0 | 17.2 | 18.9 | 18.1 | 13.7 | 8.6 | 3.4 | −1.9 | |
| Vígľaš- | WMO index 11904; | Altitude 368 m a.s.l.; | Latitude [φ] 48°32′39″; | Longitude [λ] 19°19′19″ | |||||||||
| Pstruša | Precipitation [mm] | 31.5 | 30.9 | 31.6 | 46.1 | 70.1 | 85.4 | 73.1 | 62.1 | 50.8 | 47.3 | 53.6 | 44.2 |
| Temperature [°C] | −3.4 | −1.2 | 3.2 | 8.7 | 13.5 | 16.5 | 18.1 | 17.6 | 13.4 | 8.3 | 3.3 | −1.9 | |
| Banská | WMO index 11898; | Altitude 427 m a.s.l.; | Latitude [φ] 48°44′01″; | Longitude [λ] 19°07′01″ | |||||||||
| Bystrica | Precipitation [mm] | 55.9 | 51.4 | 52.4 | 55.9 | 82.1 | 89.3 | 81.4 | 71.0 | 62.2 | 66.0 | 80.4 | 71.1 |
| Temperature [°C] | −2.5 | −0.6 | 3.3 | 9.1 | 13.8 | 16.8 | 18.4 | 17.8 | 13.5 | 8.6 | 3.6 | −1.3 | |
| Brezno | WMO index 11917; | Altitude 487 m a.s.l.; | Latitude [φ] 48°48′06″; | Longitude [λ] 19°38′14″ | |||||||||
| Precipitation [mm] | 41.7 | 39.6 | 45.3 | 51.4 | 88.4 | 98.7 | 96.1 | 81.0 | 59.5 | 56.8 | 56.5 | 50.1 | |
| Temperature [°C] | −3.7 | −2.0 | 2.3 | 8.3 | 13.3 | 16.4 | 18.2 | 17.3 | 12.6 | 7.7 | 2.7 | −2.5 | |
| Telgárt | WMO index 11938; | Altitude 901 m a.s.l.; | Latitude [φ] 48°50′55″; | Longitude [λ] 20°11′21″ | |||||||||
| Precipitation [mm] | 33.6 | 40.6 | 41.9 | 63.1 | 113 | 121 | 92.6 | 87.1 | 60.6 | 63.3 | 70.7 | 42.2 | |
| Temperature [°C] | −5.3 | −4.0 | −0.5 | 4.5 | 9.6 | 12.6 | 14.3 | 13.6 | 10.2 | 5.7 | 0.3 | −4.0 | |
| Lom nad | WMO index 11910; | Altitude 1018 m a.s.l.; | Latitude [φ] 48°39′38″; | Longitude [λ] 19°39′57″ | |||||||||
| Rimavicou | Precipitation [mm] | 48.7 | 56.4 | 51.8 | 64.2 | 100 | 124 | 99.5 | 96.4 | 64.6 | 74.8 | 87.2 | 66.8 |
| Temperature [°C] | −5.4 | −4.1 | −0.6 | 4.3 | 9.7 | 12.6 | 14.5 | 13.9 | 10.3 | 5.6 | 0.3 | −4.0 | |
| Station Name | SPI 1m | SPI 12m | SPEI 1m | SPEI 12m |
|---|---|---|---|---|
| Sliač | – | ▲ | – | – |
| p-value | 0.249 | 0.000 | 0.989 | 0.71 |
| Vígľaš-Pstruša | – | ▲ | – | – |
| p-value | 0.145 | <0.0001 | 0.946 | 0.862 |
| Banská Bystrica | – | ▲ | – | – |
| p-value | 0.109 | <0.0001 | 0.752 | 0.083 |
| Brezno | – | ▲ | – | – |
| p-value | 0.183 | <0.0001 | 0.823 | 0.217 |
| Telgárt | ▲ | ▲ | – | ▲ |
| p-value | 0.021 | <0.0001 | 0.161 | <0.0001 |
| Lom nad Rimavicou | ▲ | ▲ | – | ▲ |
| p-value | 0.023 | <0.0001 | 0.198 | <0.0001 |
| SPI (Standardised Precipitation Index) | ||||||||||||
| Station Name | Jan. | Feb. | Mar. | Apr. | May | Jun. | Jul. | Aug. | Sep. | Oct. | Nov. | Dec. |
| Sliač | ▲ | – | – | ▼ | ▼ | ▲ | ▲ | – | ▼ | – | ▼ | ▲ |
| p-value | 0.083 | 0.773 | 0.946 | 0.812 | 0.341 | 0.333 | 0.011 | 0.812 | 0.760 | 0.986 | 0.658 | 0.496 |
| Vígľaš-Pstruša | ▲ | ▲ | – | ▼ | ▼ | – | ▲ | – | – | ▲ | – | ▲ |
| p-value | 0.074 | 0.234 | 0.932 | 0.227 | 0.454 | 0.865 | 0.006 | 0.671 | 0.812 | 0.386 | 0.367 | 0.395 |
| Banská Bystrica | ▲ | ▲ | ▲ | ▼ | ▼ | – | ▲ | – | – | – | ▼ | ▲ |
| p-value | 0.004 | 0.683 | 0.518 | 0.529 | 0.292 | 0.540 | 0.004 | 0.671 | 1.000 | 0.878 | 0.734 | 0.465 |
| Brezno | ▲ | – | – | ▼ | ▼ | – | ▲ | ▲ | – | ▲ | ▼ | ▲ |
| p-value | 0.043 | 1.000 | 0.946 | 0.276 | 0.529 | 0.905 | 0.025 | 0.598 | 1.000 | 0.646 | 0.598 | 0.367 |
| Telgárt | ▲ | – | – | ▼ | ▼ | ▲ | ▲ | ▲ | ▲ | ▲ | – | ▲ |
| p-value | 0.110 | 0.799 | 0.812 | 0.434 | 0.405 | 0.118 | 0.004 | 0.359 | 0.825 | 0.367 | 0.852 | 0.385 |
| Lom nad Rimavicou | ▲ | ▲ | ▲ | ▼ | ▼ | – | ▲ | – | ▲ | ▲ | – | ▲ |
| p-value | 0.036 | 0.773 | 0.308 | 0.496 | 0.316 | 1.000 | 0.024 | 0.878 | 0.734 | 0.563 | 1.000 | 0.316 |
| SPEI (Standardised Precipitation Evapotranspiration Index) | ||||||||||||
| Station Name | Jan. | Feb. | Mar. | Apr. | May | Jun. | Jul. | Aug. | Sep. | Oct. | Nov. | Dec. |
| Sliač | ▲ | – | – | ▼ | ▼ | ▲ | ▲ | ▼ | ▼ | – | ▼ | ▲ |
| p-value | 0.096 | 0.919 | 0.812 | 0.234 | 0.292 | 0.892 | 0.069 | 0.465 | 0.622 | 0.973 | 0.191 | 0.454 |
| Vígľaš-Pstruša | ▲ | ▲ | ▲ | ▼ | ▼ | – | ▲ | – | – | – | – | ▲ |
| p-value | 0.004 | 0.683 | 0.518 | 0.529 | 0.292 | 0.540 | 0.004 | 0.671 | 1.000 | 0.878 | 0.734 | 0.465 |
| Banská Bystrica | ▲ | ▲ | – | ▼ | ▼ | ▼ | ▲ | ▼ | ▼ | – | ▼ | ▲ |
| p-value | 0.004 | 0.598 | 0.892 | 0.234 | 0.227 | 0.507 | 0.040 | 0.518 | 0.825 | 0.919 | 0.865 | 0.529 |
| Brezno | ▲ | ▲ | ▼ | ▼ | ▼ | ▼ | ▲ | ▲ | ▲ | – | ▼ | ▲ |
| p-value | 0.034 | 0.886 | 0.844 | 0.058 | 0.643 | 0.682 | 0.087 | 0.872 | 0.592 | 0.901 | 0.225 | 0.605 |
| Telgárt | ▲ | – | ▲ | ▼ | ▼ | ▲ | ▲ | ▲ | – | ▲ | ▼ | ▲ |
| p-value | 0.110 | 0.773 | 0.878 | 0.341 | 0.350 | 0.350 | 0.014 | 0.658 | 0.959 | 0.444 | 0.760 | 0.385 |
| Lom nad Rimavicou | ▲ | ▲ | ▲ | ▼ | ▼ | ▼ | ▲ | – | – | ▲ | – | ▲ |
| p-value | 0.036 | 0.721 | 0.563 | 0.158 | 0.341 | 0.575 | 0.045 | 0.658 | 0.747 | 0.696 | 0.721 | 0.316 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Vido, J.; Nalevanková, P. Drought in the Upper Hron Region (Slovakia) between the Years 1984–2014. Water 2020, 12, 2887. https://doi.org/10.3390/w12102887
Vido J, Nalevanková P. Drought in the Upper Hron Region (Slovakia) between the Years 1984–2014. Water. 2020; 12(10):2887. https://doi.org/10.3390/w12102887
Chicago/Turabian StyleVido, Jaroslav, and Paulína Nalevanková. 2020. "Drought in the Upper Hron Region (Slovakia) between the Years 1984–2014" Water 12, no. 10: 2887. https://doi.org/10.3390/w12102887





