Investigation of Local Scouring around Hydrodynamic and Circular Pile Groups under the Influence of River Material Harvesting Pits
Abstract
:1. Introduction
2. Dimensional Analysis
3. Numerical Model
3.1. Flow Field Simulation
- A.
- The fluid is continuous and the stress is linearly related to the strain rate;
- B.
- The fluid is isotropic, i.e., the properties of the fluid are independent of direction. As a result, the law of deformation is independent of the chosen axis;
- C.
- When the strain rate is zero, the law of deformation is reduced to hydrostatic pressure.
3.2. Turbulence Model
3.3. Sediment Scour Model
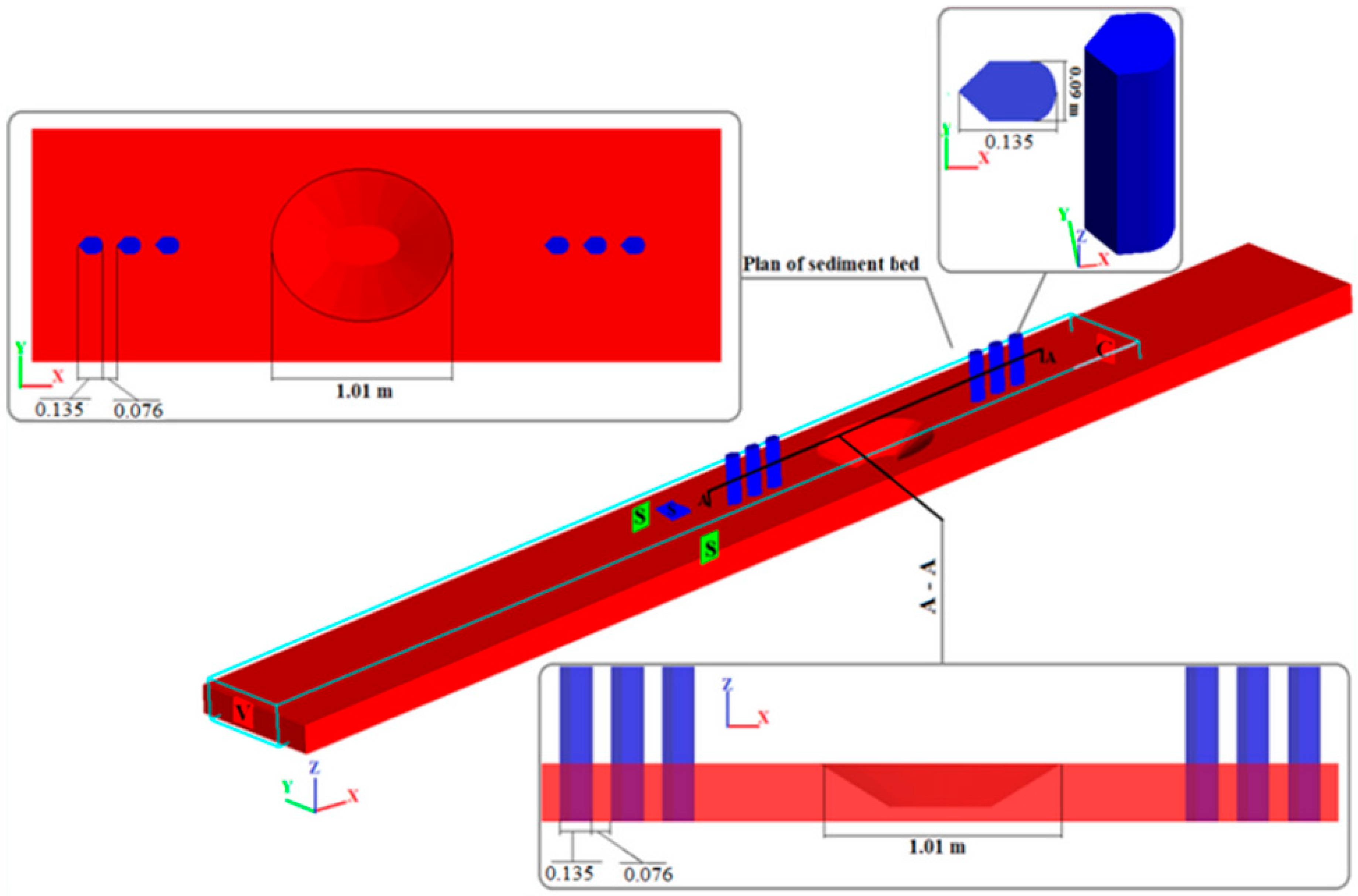
3.4. Description of the Laboratory Experiment and Numerical Setup
3.5. Effect of the Computational Mesh on the Scour Depth Results
3.6. Validation of the Numerical Simulation
3.7. Simulation of Local Scouring around the Hydrodynamic Pile Group
4. Results
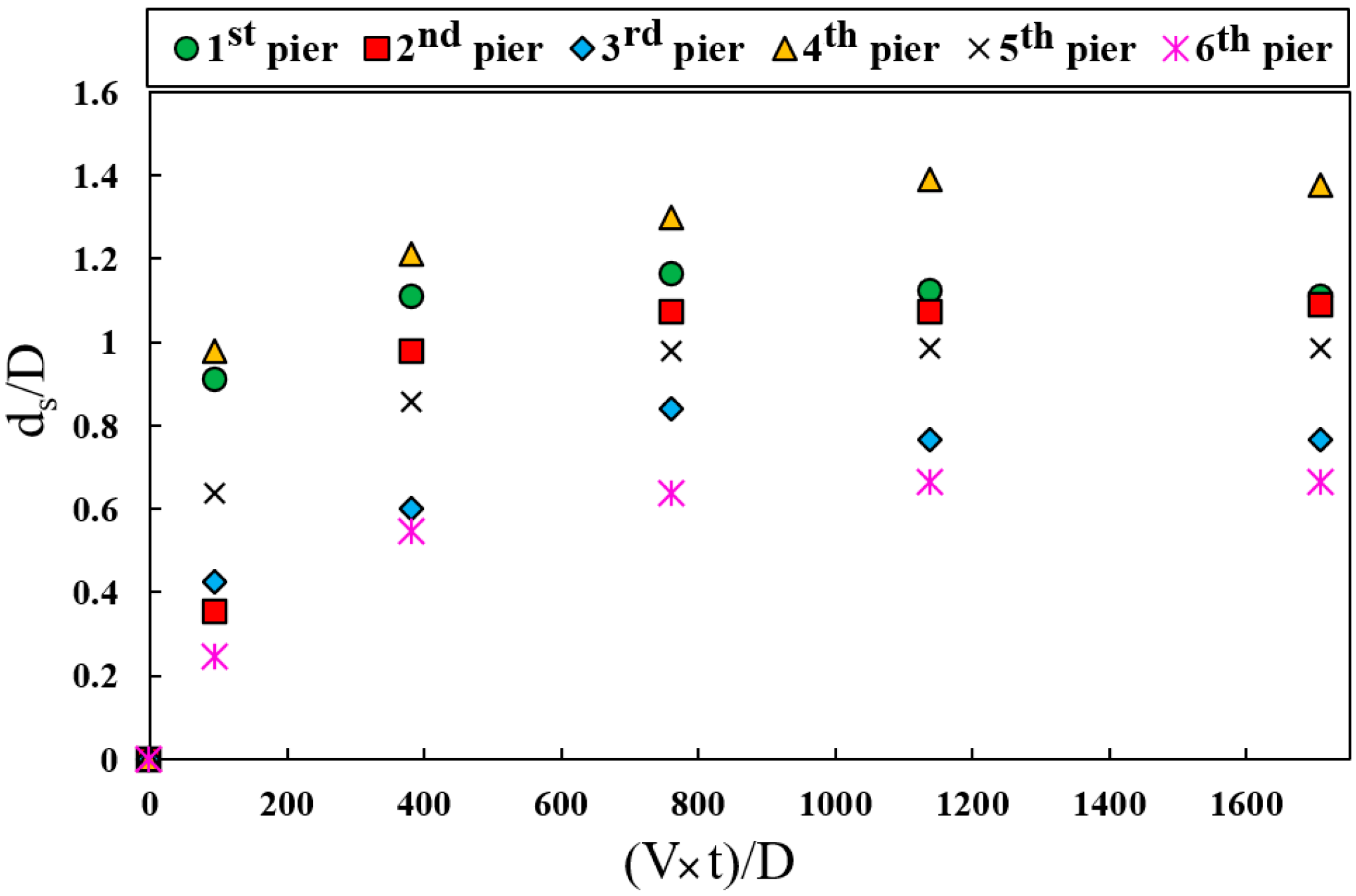
4.1. Results of Laboratory Study
4.2. Comparison of Scour for the Hydrodynamic and Circular Pile Groups
4.3. Flow Patterns around the Hydrodynamic and Circular Pile Groups
4.4. Investigation of the Material Harvest Pit Changes
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Koehnken, L.; Rintoul, M.S.; Goichot, M.; Tickner, D.; Loftus, A.C.; Acreman, M.C. Impacts of riverine sand mining on freshwater ecosystems: A review of the scientific evidence and guidance for future research. River Res. Appl. 2020, 36, 362–370. [Google Scholar] [CrossRef]
- Ataei-Ashtiani, B.; Beheshti, A.A. Experimental investigation of clear-water local scour at pile groups. J. Hydraul. Eng. 2006, 10, 1100–1104. [Google Scholar] [CrossRef] [Green Version]
- Zhou, Y.; Mahbub, A.M. Wake of two interacting circular cylinders: A review. Int. J. Heat Fluid Flow 2016, 62, 510–537. [Google Scholar] [CrossRef]
- Liang, F.; Wang, C.; Huang, M.; Wang, Y. Experimental observations and evaluations of formulae for local scour at pile groups in steady currents. Mar. Georesources Geotechnol. 2017, 35, 245–255. [Google Scholar] [CrossRef]
- Solaimani, N.; Amini, A.; Banejad, H.; Taherei Ghazvinei, P. The effect of pile spacing and arrangement on bed formation and scour hole dimensions in pile groups. Int. J. River Basin Manag. 2017, 15, 219–225. [Google Scholar] [CrossRef]
- Vaghefi, M.; Motlagh, M.J.T.N.; Hashemi, S.S.; Moradi, S. Experimental study of bed topography variations due to placement of a triad series of vertical piers at different positions in a 180 bend. Arab. J. Geosci. 2018, 11, 102. [Google Scholar] [CrossRef]
- Namaee, M.R.; Sui, J. Local scour around two side-by-side cylindrical bridge piers under ice-covered conditions. Int. J. Sediment Res. 2019, 34, 355–367. [Google Scholar] [CrossRef]
- Malik, R.; Setia, B. Interference between pier models and its effects on scour depth. SN Appl. Sci. 2020, 2, 68. [Google Scholar] [CrossRef] [Green Version]
- Yang, Y.; Qi, M.; Wang, X.; Li, J. Experimental study of scour around pile groups in steady flows. Ocean. Eng. 2020, 195, 106651. [Google Scholar] [CrossRef]
- Decker, C.; Keyes, J.; Jackson, C.R.; Shelton, J.; Jackson, B. The Effects of Sand Dredging on Channel Morphology, Habitat, and Water Quality in Urban DeKalb County Streams; Georgia Institute of Technology: Atlanta, GA, USA, 1999. [Google Scholar]
- Lee, H.Y.; Fu, D.T.; Song, M.H. Migration of rectangular mining pit composed of uniform sediment. J. Hydraul. Eng. 1993, 119, 64–80. [Google Scholar] [CrossRef]
- Cao, Z.; Pender, G. Numerical modeling of alluvial rivers subjected to interactive sediment mining and feeding. Adv. Water Resour. 2004, 27, 533–546. [Google Scholar] [CrossRef]
- Barman, B.; Kumar, B.; Sarma, A.K. Turbulent flow structures and geomorphic characteristics of a mining affected alluvial channel. Earth Surf. Proces. Landf. 2018, 43, 1811–1824. [Google Scholar] [CrossRef]
- Barman, B.; Kumar, B.; Sarma, A.K. Dynamic characterization of the migration of a mining pit in an alluvial channel. Int. J. Sediment Res. 2019, 34, 155–165. [Google Scholar] [CrossRef]
- Barman, B.; Sharma, A.; Kumar, B.; Sarma, A.K. Multiscale characterization of migrating sand wave in mining induced alluvial channel. Ecol. Eng. 2017, 102, 199–206. [Google Scholar] [CrossRef]
- Neyshabouri, S.A.A.S.; Farhadzadeh, A.; Amini, A. Experimental and field study of mining pit migration. Int. J. Sedim. Res. 2002, 17, 323–333. [Google Scholar]
- Barman, B.; Kumar, B.; Sarma, A.K. Impact of sand mining on alluvial channel flow characteristics. Ecol. Eng. 2019, 135, 36–44. [Google Scholar] [CrossRef]
- Lade, A.D.; Deshpande, V.; Kumar, B.; Oliveto, G. On the Morphodynamic Alterations around Bridge Piers under the Influence of Instream Mining. Water 2019, 11, 1676. [Google Scholar] [CrossRef] [Green Version]
- Majedi-Asl, M.; Daneshfaraz, R.; Abraham, J.; Valizadeh, S. Effects of Hydraulic Characteristics, Sedimentary Parameters, and Mining of Bed Material on Scour Depth of Bridge Pier Groups. J. Perform. Constr. Facil. 2020, 35, 04020148. [Google Scholar] [CrossRef]
- Daneshfaraz, R.; Sattariyan Karajabad, M.; Alinejad, B.; Majedi-Asl, M. Experimental Investigation of the Effects of Flow Discharge on the Scour Rate around the Groups of Bridge Piers with a Rough Surface in the Presence of Aggregate Extraction Pits. JWSS 2021, 24, 111–125. [Google Scholar]
- Mastbergen, D.R.; van den Berg, J.H. Breaching in fine sands and the generation of sustained turbidity currents in submarine canyons. Sedimentology 2003, 50, 625–637. [Google Scholar] [CrossRef]
- Chiew, Y.M.; Melville, B.W. Local scour around bridge piers. J. Hydraul. Res. 1987, 25, 15–26. [Google Scholar] [CrossRef]
- Melville, B.W.; Chiew, Y.M. Time scale for local scour at bridge piers. J. Hydraul. Eng. 1999, 125, 59–65. [Google Scholar] [CrossRef]
- Ghaderi, A.; Daneshfaraz, R.; Torabi, M.; Abraham, J.; Azamathulla, H.M. Experimental investigation on effective scouring parameters downstream from stepped spillways. Water Supply 2020, 20, 1988–1998. [Google Scholar] [CrossRef]
- Flow Science Inc. FLOW-3D V 11.2 User’s Manual; Flow Science, Inc.: Santa Fe, NM, USA, 2016. [Google Scholar]
- Abbasi, S.; Fatemi, S.; Ghaderi, A.; Di Francesco, S. The Effect of Geometric Parameters of the Antivortex on a Triangular Labyrinth Side Weir. Water 2021, 13, 14. [Google Scholar] [CrossRef]
- Daneshfaraz, R.; Ghaderi, A.; Akhtari, A.; Di Francesco, S. On the Effect of Block Roughness in Ogee Spillways with Flip Buckets. Fluids 2020, 5, 182. [Google Scholar] [CrossRef]
- Baykal, C.; Sumer, B.M.; Fuhrman, D.R.; Jacobsen, N.G.; Fredsøe, J. Numerical simulation of scour and backfilling processes around a circular pile in waves. Coast. Eng. 2017, 122, 87–107. [Google Scholar] [CrossRef] [Green Version]
- Li, Y.; Ong, M.C.; Fuhrman, D.R.; Larsen, B.E. Numerical investigation of wave-pluscurrent induced scour beneath two submarine pipelines in tandem. Coast. Eng. 2020, 156, 103619. [Google Scholar] [CrossRef]
- Ghaderi, A.; Abbasi, S. CFD simulation of local scouring around airfoil-shaped bridge piers with and without collar. Sādhanā 2019, 44, 216. [Google Scholar] [CrossRef] [Green Version]
- Li, Y.; Ong, M.C.; Fuhrman, D.R. CFD investigations of scour beneath a submarine pipeline with the effect of upward seepage. Coast. Eng. 2020, 156, 103624. [Google Scholar] [CrossRef]
- Ghaderi, A.; Dasineh, M.; Aristodemo, F.; Aricò, C. Numerical Simulations of the Flow Field of a Submerged Hydraulic Jump over Triangular Macroroughnesses. Water 2021, 13, 674. [Google Scholar] [CrossRef]
- Fuhrman, D.R.; Schløer, S.; Sterner, J. RANS-based simulation of turbulent wave boundary layer and sheet-flow sediment transport processes. Coast. Eng. 2013, 73, 151–166. [Google Scholar] [CrossRef]
- Choufu, L.; Abbasi, S.; Pourshahbaz, H.; Taghvaei, P.; Tfwala, S. Investigation of Flow, Erosion, and Sedimentation Pattern around Varied Groynes under Different Hydraulic and Geometric Conditions: A Numerical Study. Water 2019, 11, 235. [Google Scholar] [CrossRef] [Green Version]
- Ghaderi, A.; Daneshfaraz, R.; Dasineh, M.; Di Francesco, S. Energy Dissipation and Hydraulics of Flow over Trapezoidal–Triangular Labyrinth Weirs. Water 2020, 12, 1992. [Google Scholar] [CrossRef]
- Ghaderi, A.; Daneshfaraz, R.; Dasineh, M. Evaluation and prediction of the scour depth of bridge foundations with HEC-RAS numerical model and empirical equations (Case Study: Bridge of Simineh Rood Miandoab, Iran). Eng. J. 2019, 23, 279–295. [Google Scholar] [CrossRef]
- Daneshfaraz, R.; Aminvash, E.; Ghaderi, A.; Kuriqi, A.; Abraham, J. Three-Dimensional Investigation of Hydraulic Properties of Vertical Drop in the Presence of Step and Grid Dissipators. Symmetry 2021, 13, 895. [Google Scholar] [CrossRef]
- Kirkil, G.; Constantinescu, G.; Ettema, R. Detached eddy simulation investigation of turbulence at a circular pier with scour hole. J. Hydraul. Eng. 2009, 135, 888–901. [Google Scholar] [CrossRef]
- McCoy, A.; Constantinescu, G.; Weber, L.J. Numerical investigation of flow hydrodynamics in a channel with a series of groynes. J. Hydraul. Eng. 2008, 134, 157–172. [Google Scholar] [CrossRef]
- Koken, M.; Constantinescu, G. An investigation of the flow and scour mechanisms around isolated spur dikes in a shallow open channel: 1. Conditions corresponding to the initiation of the erosion and deposition process. Water Resour. Res. 2008, 44. [Google Scholar] [CrossRef]
- Soulsby, R. Chapter 9: Bedload transport. In Dynamics of Marine Sands; Thomas Telford Publications: London, UK, 1997. [Google Scholar]
- Omara, H.; Elsayed, S.M.; Abdeelaal, G.M.; Abd-Elhamid, H.F.; Tawfik, A. Hydromorphological numerical model of the local scour process around bridge piers. Arab. J. Sci. Eng. 2019, 44, 4183–4199. [Google Scholar] [CrossRef]
- Ghaderi, A.; Abbasi, S. Experimental and Numerical Study of the Effects of Geometric Appendance Elements on Energy Dissipation over Stepped Spillway. Water 2021, 13, 957. [Google Scholar] [CrossRef]
- Raudkivi, A.J.; Ettema, R. Clear-water scour at cylindrical piers. J. Hydraul. Eng. 1983, 109, 338–350. [Google Scholar] [CrossRef]











| Test No. | Q (L/s) | y0 (m) | u (m/s) | Fr | Re | u/ucr | d50 (m) | Gs | σg |
|---|---|---|---|---|---|---|---|---|---|
| T1 | 45 | 0.132 | 0.284 | 0.25 | 25,600 | 0.72 | 0.00086 | 2.65 | 1.32 |
| Model | Number of Cells | ds of the 1st Pier Upstream (m) | ds of the 1st Pier Downstream (m) | ||
|---|---|---|---|---|---|
| Physical model | - | 0.1 | - | 0.124 | - |
| Mesh size 0.02 m | 307,360 | 0.016 | 84 | 0.024 | 80 |
| Mesh size 0.015 m | 707,762 | 0.047 | 53 | 0.056 | 54 |
| Mesh size 0.009 m | 3,332,516 | 0.107 | 6.7 | 0.135 | 8.8 |
| Pier Number | The Dimensionless Scour Depth at the End of the Laboratory Model | The Dimensionless Scour Depth at the End of the Numerical Model | E | |||
|---|---|---|---|---|---|---|
| 1st | 1.1226 | 1.1876 | 0.99 | 0.05 | 0.06 | 0.06 |
| 2nd | 1.0989 | 0.9404 | 0.99 | 0.10 | 0.13 | 0.15 |
| 3rd | 0.7647 | 0.9826 | 0.93 | 0.10 | 0.14 | 0.21 |
| 4th | 1.405 | 1.5009 | 0.92 | 0.14 | 0.21 | 0.07 |
| 5th | 0.9940 | 1.020 | 0.95 | 0.08 | 0.15 | 0.03 |
| 6th | 0.6710 | 1.0792 | 0.98 | 0.14 | 0.21 | 0.36 |
| Pier Model | 1st Pier | 2nd Pier | 3rd Pier | 4th Pier | 5th Pier | 6th Pier |
|---|---|---|---|---|---|---|
| Scour depth around the hydrodynamic piers (m) | 0.0868 | 0.0821 | 0.0734 | 0.1083 | 0.0643 | 0.0880 |
| Scour depth around the circular piers (m) | 0.1067 | 0.0838 | 0.0883 | 0.1349 | 0.0915 | 0.0968 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Daneshfaraz, R.; Ghaderi, A.; Sattariyan, M.; Alinejad, B.; Asl, M.M.; Di Francesco, S. Investigation of Local Scouring around Hydrodynamic and Circular Pile Groups under the Influence of River Material Harvesting Pits. Water 2021, 13, 2192. https://doi.org/10.3390/w13162192
Daneshfaraz R, Ghaderi A, Sattariyan M, Alinejad B, Asl MM, Di Francesco S. Investigation of Local Scouring around Hydrodynamic and Circular Pile Groups under the Influence of River Material Harvesting Pits. Water. 2021; 13(16):2192. https://doi.org/10.3390/w13162192
Chicago/Turabian StyleDaneshfaraz, Rasoul, Amir Ghaderi, Maryam Sattariyan, Babak Alinejad, Mahdi Majedi Asl, and Silvia Di Francesco. 2021. "Investigation of Local Scouring around Hydrodynamic and Circular Pile Groups under the Influence of River Material Harvesting Pits" Water 13, no. 16: 2192. https://doi.org/10.3390/w13162192
APA StyleDaneshfaraz, R., Ghaderi, A., Sattariyan, M., Alinejad, B., Asl, M. M., & Di Francesco, S. (2021). Investigation of Local Scouring around Hydrodynamic and Circular Pile Groups under the Influence of River Material Harvesting Pits. Water, 13(16), 2192. https://doi.org/10.3390/w13162192








