A Study of Drag Reduction on Cylinders with Different V-Groove Depths on the Surface
Abstract
:1. Introduction
2. Mathematical Model Parameter Settings and Experimental Equipment
2.1. Control Equation
2.2. Turbulence Model
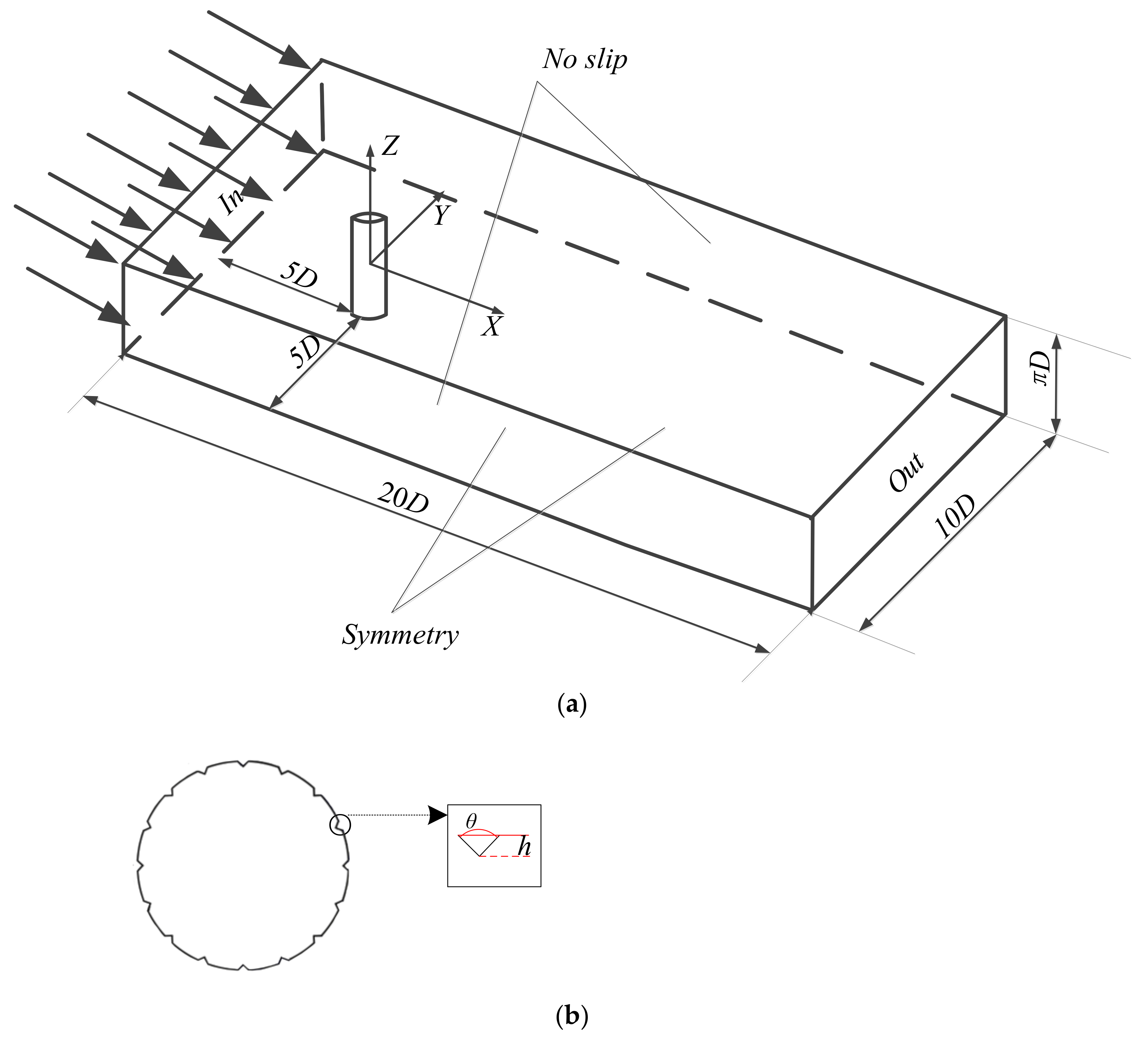
2.3. Geometric Models and Boundary Conditions
2.4. Experimental Equipment
3. Model Verification
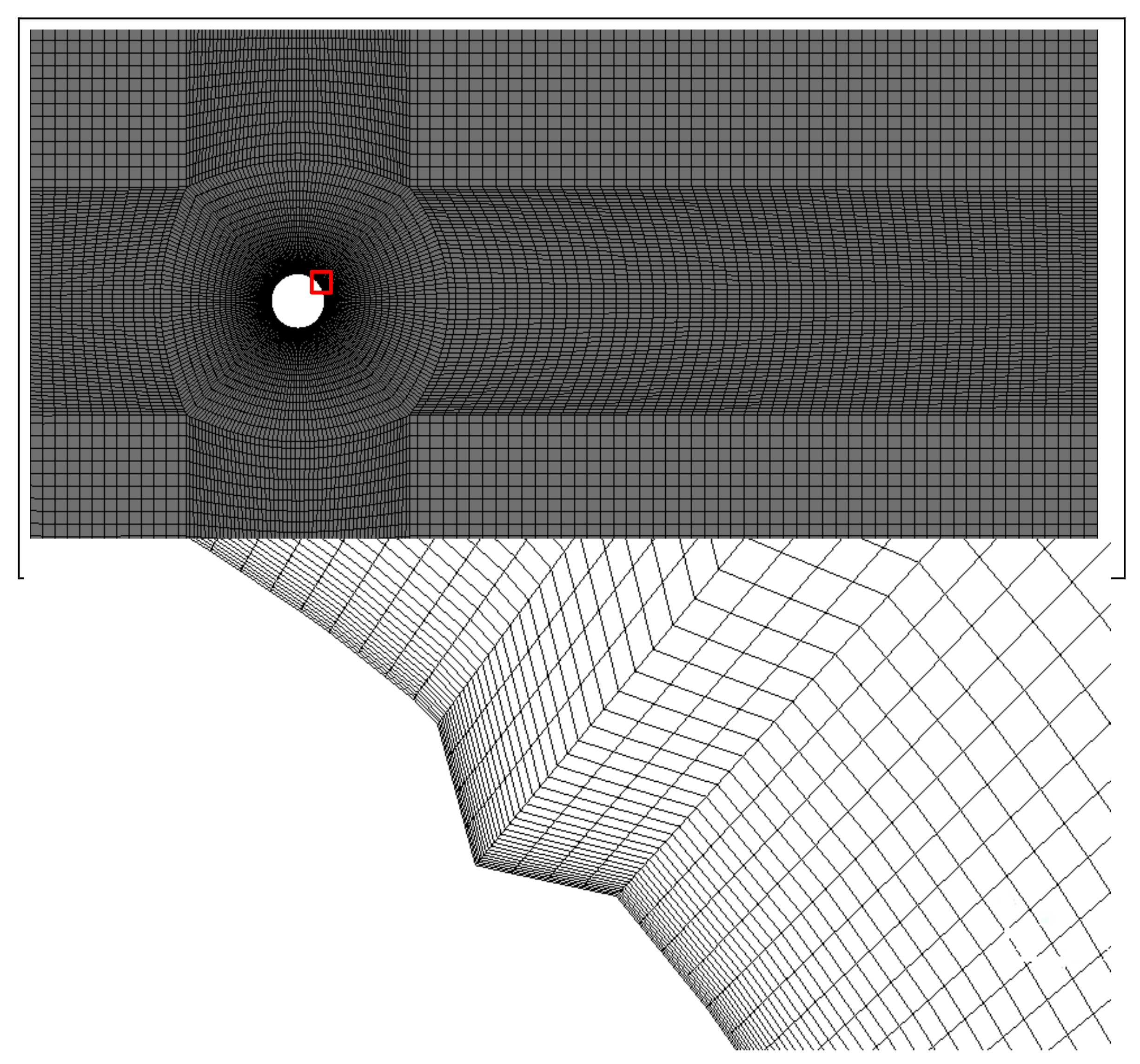
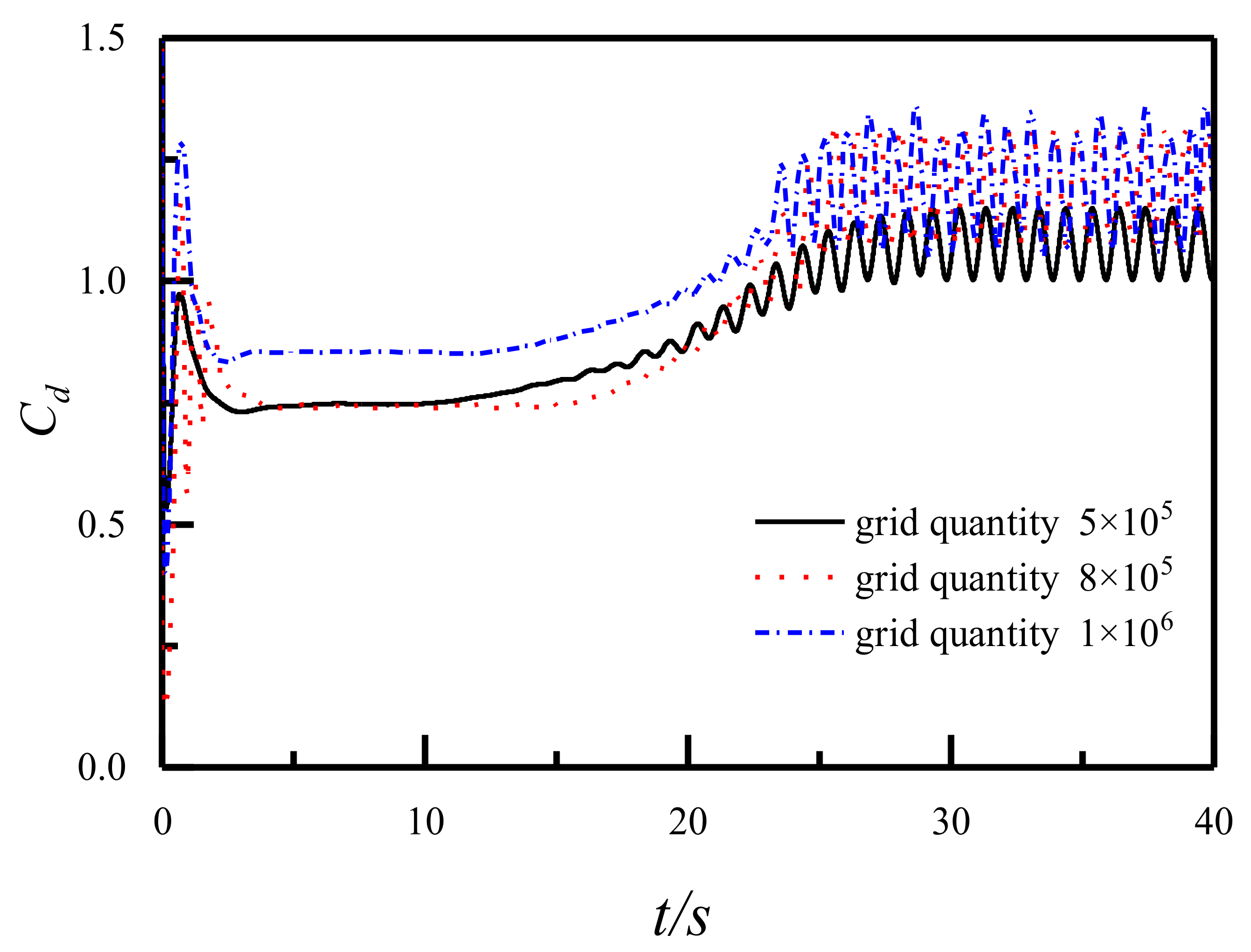
3.1. Grid-Independent Verification
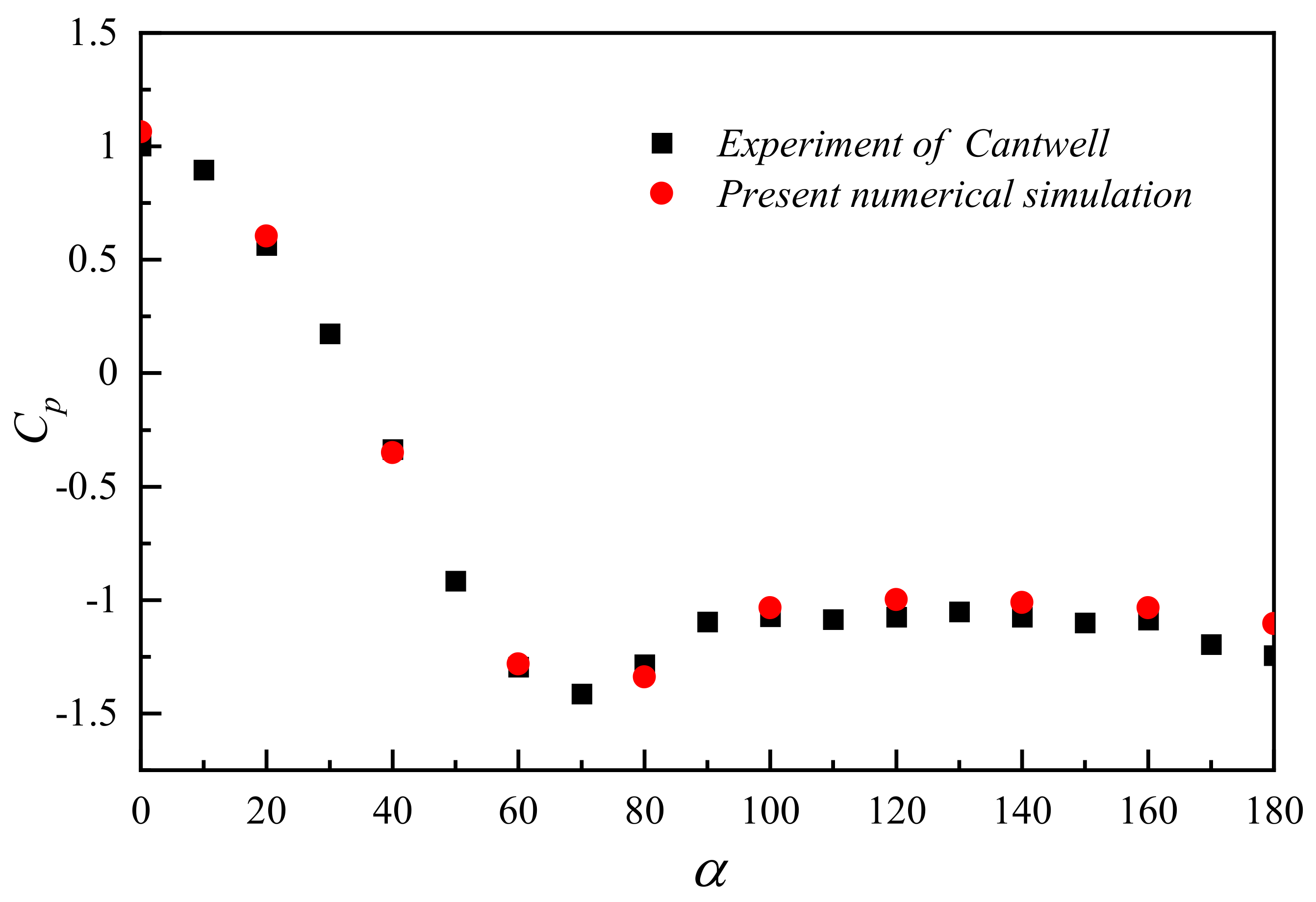
3.2. Parameter Verification
4. Numerical Simulation and Analysis
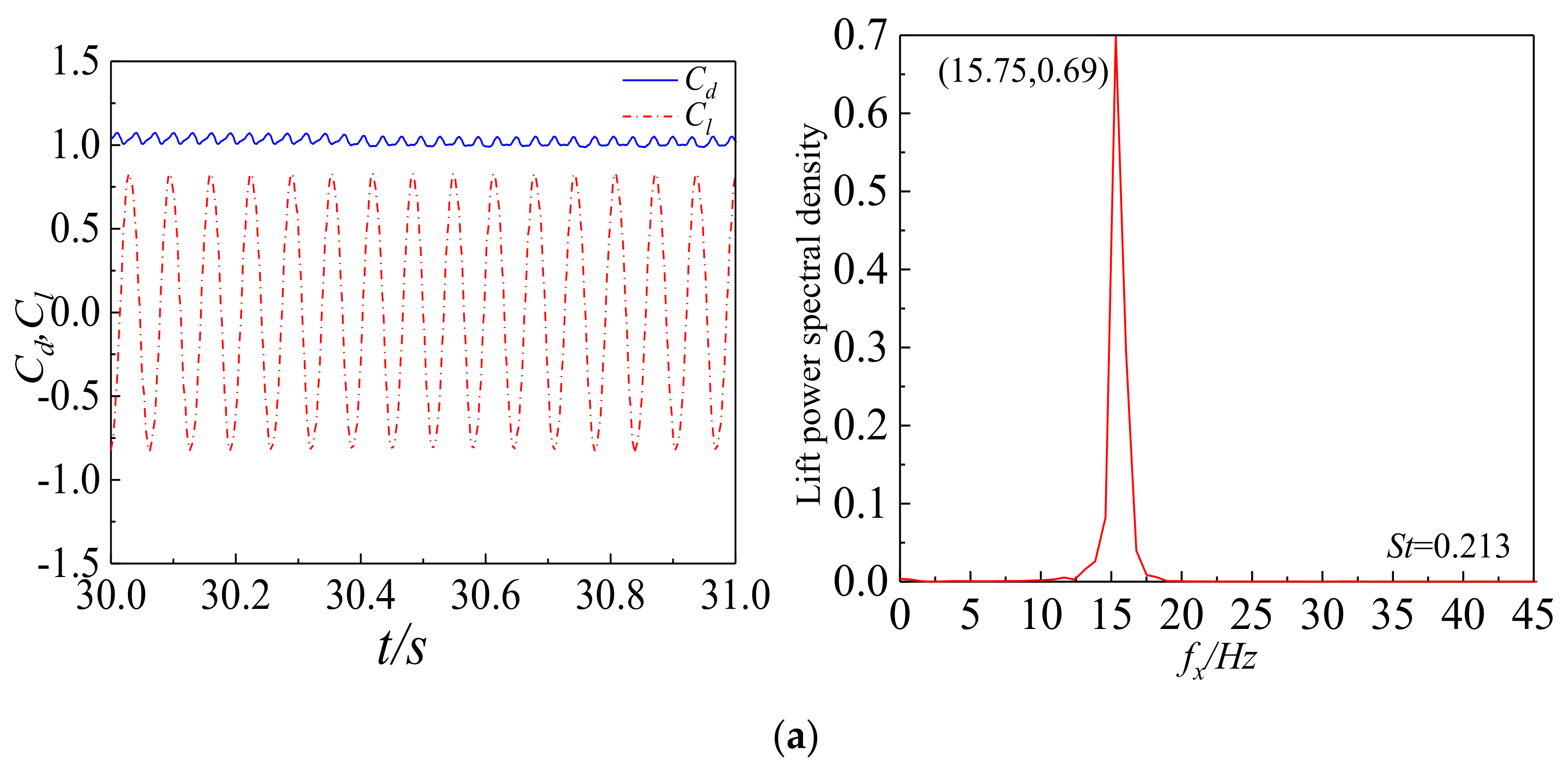
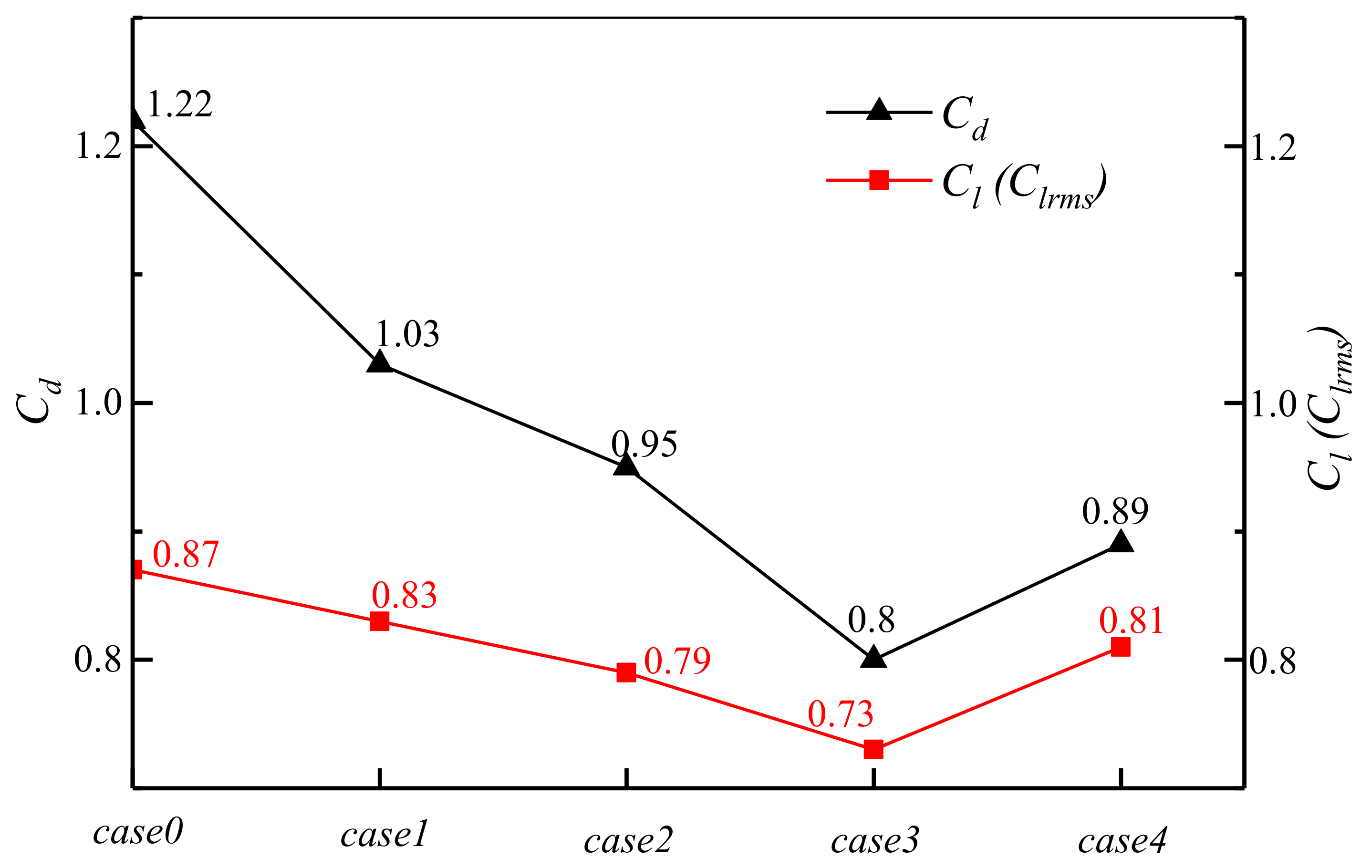
4.1. Lift Coefficient and Drag Coefficient
4.2. Pressure Coefficient
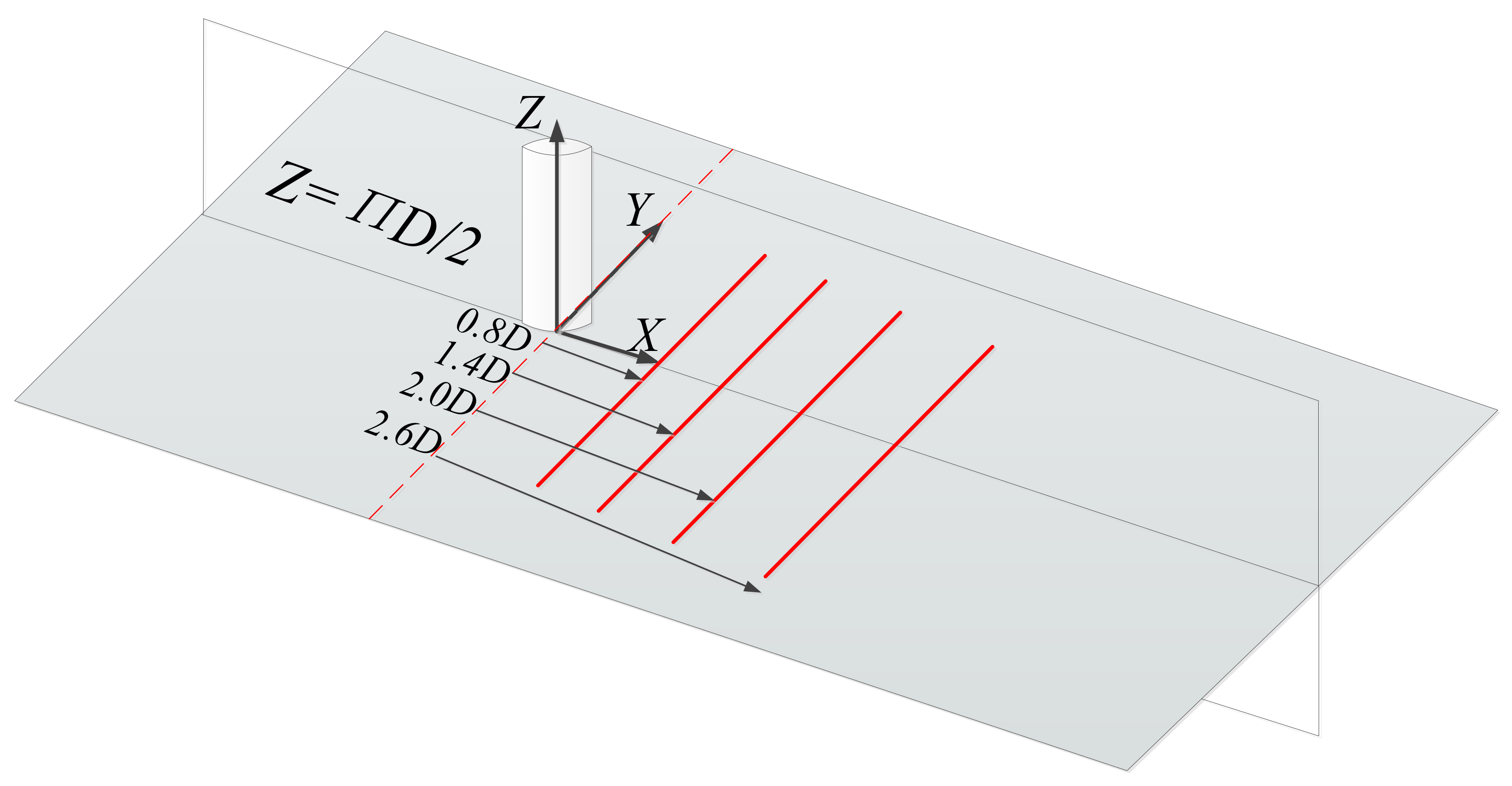
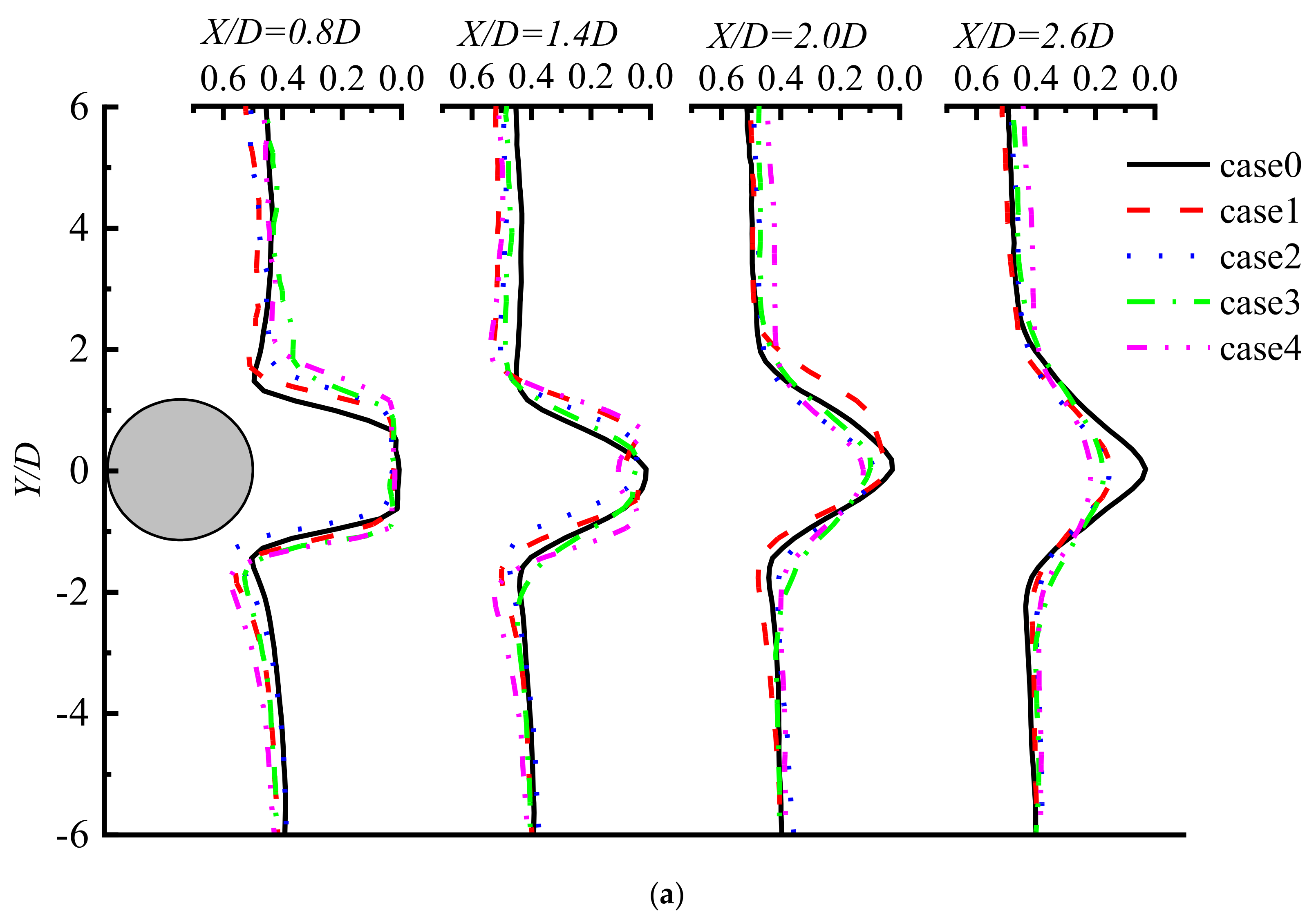
4.3. Time-Averaged Velocity of Flow Field
5. Experimental Results and Discussions
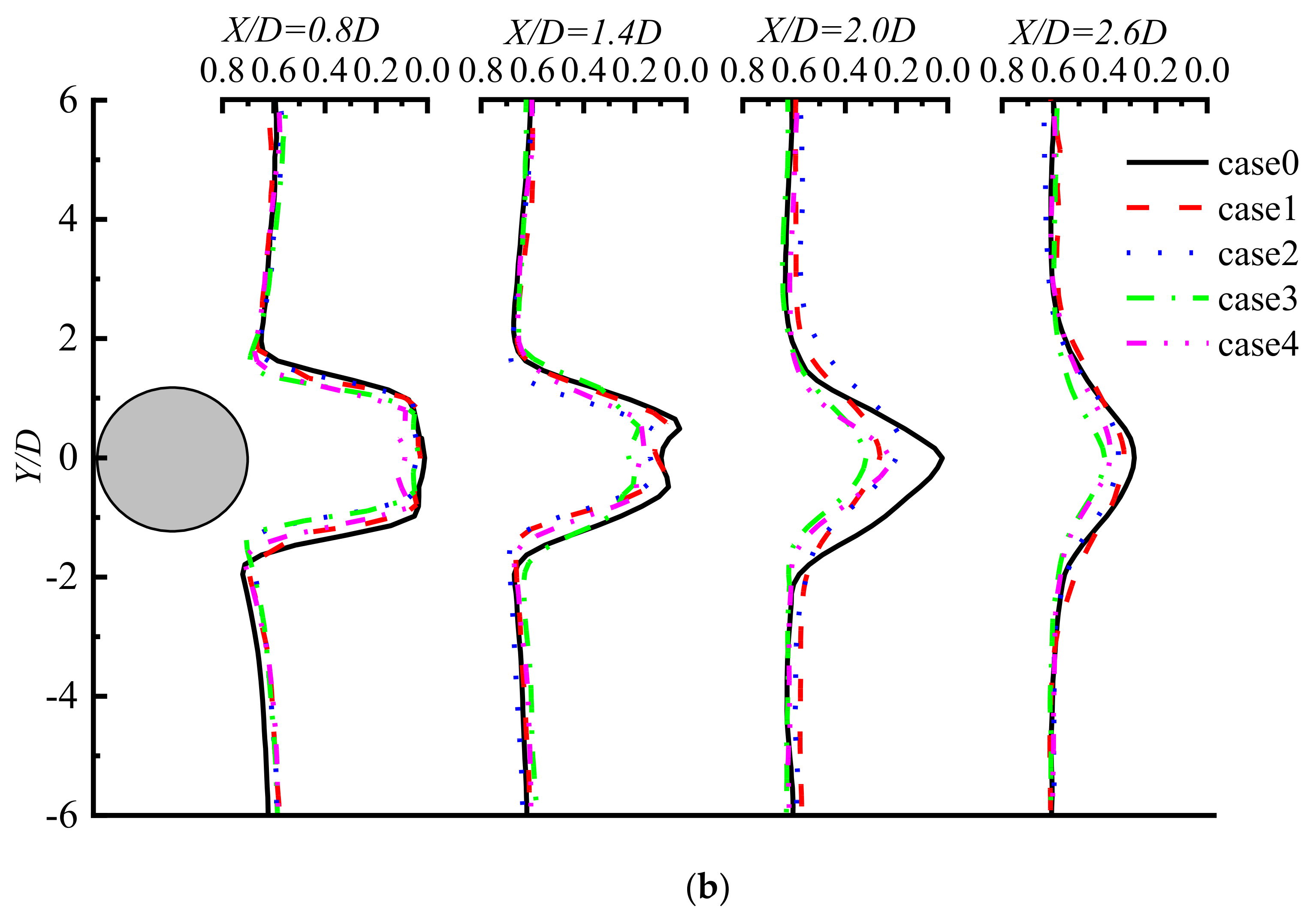
5.1. Velocity Field
5.2. Vortex Structure
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| D | the diameter of the cylinder, mm. |
| h | the depth of groove, mm. |
| U∞ | the speed inlet, m/s. |
| P | the pressure outlet, pa. |
| the fluid density. | |
| the fluid viscosity. | |
| Fd | the drag of the cylinder |
| A | the windward area. |
| Fl | the lift of the cylinder. |
| fx | the vortex shedding frequency. |
| Cd | the drag coefficient. |
| Cl | the lift coefficient. |
References
- Søreide, M. Experimental investigation of in line and cross-flow VIV. In Proceedings of the 13th International Offshore and Polar Engineering Conference, Honolulu, HI, USA, 25–30 May 2003. [Google Scholar]
- Chen, L. Two-dimensional numerical simulation of flow around a cylinder and a circular cylinder. Eng. Struct. 2020, 40, 196–199. [Google Scholar]
- Mario, A.A.L.; Filiberto, H.Z. Simulating the flow around a baseball: Study of a 2D-cylinder with a single bump. Comput. Math. Appl. 2019, 78, 3105–3116. [Google Scholar]
- Xu, Y.H.; Chen, S.S. Analysis of flow characteristics around a rotating cylinder. Chin. J. Theor. Appl. Mech. 2021, 55, 1900–1910. [Google Scholar]
- Yan, F.; Yang, H.F. Study on the drag reduction characteristics of circular cylinder with the dimpled surface. Water 2021, 13, 197. [Google Scholar] [CrossRef]
- Farhana, A.; Muhammad, A.R. Sharif. Numerical study of cross-flow around a circular cylinder with differently shaped span-wise surface grooves at low Reynolds numbe. Eur. J. Mech. B Fluids 2021, 91, 203–218. [Google Scholar]
- Baek, H.; Karniadakis, G.E. Suppressing vortex-induced vibrations via passive means. J. Fluid Struct. 2009, 25, 848–866. [Google Scholar] [CrossRef]
- Kakimpa, B.; Hargreaves, D.M.; Owen, J.S.; Martinez-Vazquez, P.; Quinn, A.D. CFD modelling of free-flight and auto-rotation of plate-type debris. Wind Struct. 2010, 13, 169–189. [Google Scholar] [CrossRef]
- Kakimpa, B.; Hargreaves, D.M.; Owen, J.S. An investigation of plate-type windborne debris flight using coupled CFD–RBD models. Part II: Free and constrained flight. J. Wind Eng. Ind. Aerodyn. 2012, 111, 104–116. [Google Scholar] [CrossRef] [Green Version]
- Zhu, R.Q.; Zhang, Y.L. Study on the suppression of vortex-induced vibration of cylinder by moving wave wall. J. Ship. Mech. 2011, 15, 1203–1209. [Google Scholar]
- El-Makdah, A.M.; Oweis, G.F. The flow past a cactus-inspired grooved cylinder. Exp. Fluids 2013, 54, 1464–1479. [Google Scholar] [CrossRef]
- Oruc, V.; Akilli, H.; Sahin, B. PIV measurements on the passive control of flow past a circular cylinder. Exp. Therm. Fluid Sci. 2016, 70, 283–291. [Google Scholar] [CrossRef]
- Xu, W.H.; Luan, Y.S.; Liu, L.Q.; Wu, Y.X. Influences of the helical strake cross-section shape on vortex-induced vibrations suppression for a long flexible cylinder. China Ocean Eng. 2017, 31, 438–446. [Google Scholar] [CrossRef]
- Yu, D.Y.; Zhao, J.H.; Huang, D.Y.; Zhong, Y.C. Numerical simulation and hydrodynamic analysis of flow around cylinders with different chamfering radii. Ocean Eng. 2018, 36, 5–15. [Google Scholar]
- Li, E.T.; Ji, Q.F.; Pang, M.J. Research on drag reduction mechanism of wall microstructure flow control technology. J. Hydraul. Eng. 2018, 6, 767–774. [Google Scholar]
- Menter, F.R. Two-equation eddy-viscosity turbulence models for engineering applications. AIAA J. 1994, 32, 1598–1605. [Google Scholar] [CrossRef] [Green Version]
- Lei, J.M.; Tan, C.M. Numerical study of high Reynolds number cylinder flow around transition based on Transition SST model. J. Beijing Univ. Aeronaut. Astronaut. 2017, 43, 207–217. [Google Scholar]
- Adrian, R.J. Scattering particle characteristics and their effect on pulsed laser measurements of fluid flow: Speckle velocimetry vsparticle image velocimetry. J. Appl. Optics 1984, 23, 1690–1691. [Google Scholar] [CrossRef]
- Wang, C.Y.; Gao, Q.; Wang, H.P. Divergence-free smoothing for volumetric PIV data. J. Exp. Fluids 2016, 57, 1–23. [Google Scholar] [CrossRef]
- Szepessy, S.; Bearman, P.W. Aspect ratio and end plate effects on vortex shedding from a circular cylinder. Fluid Mech. 1992, 234, 191–217. [Google Scholar] [CrossRef]
- Schewe, G. On the force fluctuations acting on a circular cylinder in crossflow from subcritical up to transcritical Reynolds number. J. Fluid Mech. 1983, 133, 265–285. [Google Scholar]
- Zdravkovich, M.M. Review and classification of various aerodynamic and hydrodynamic means for suppressing vortex shedding. J. Wind Eng. Ind. Aerod. 1981, 7, 145–189. [Google Scholar] [CrossRef]
- Cantwall, B.J. A Flying Hot Wire Study of the Turbulent Near Wake of a Circular Cylinder at A Re Number of 14,000; Pasadena California Institute of Technology: Pasadena, CA, USA, 1976. [Google Scholar]
- Richter, A.; Naudascher, E. Fluctuating forces on a rigid circular cylinder in confined flow. J. Fluid Mech. 1976, 78, 561. [Google Scholar] [CrossRef]
- Achenbach, E. Distribution of local pressure and skin friction around a circular cylinder in cross-flow up to Re = 5 × 106. J. Fluid Mech. 1968, 34, 625–639. [Google Scholar] [CrossRef]
- Aguedal, L.; Semmar, D.; Berrouk, A.S. 3D vortex structure investigation using large eddy simulation of flow around a rotary oscillating circular cylinder. Eur. J. Mech. B Fluids 2018, 71, 113–125. [Google Scholar] [CrossRef]
- Babu, P.; Mahesh, K. Aerodynamic loads on cactus-shaped cylinders at low Reynolds numbers. J. Phys. Fluids 2008, 20, 035112. [Google Scholar] [CrossRef] [Green Version]
- Liu, Y.Z.; Shi, L.L.; Yu, J. TR-PIV measurement of the wake behind a grooved cylinder at low Reynolds number. J. Fluids Struct. 2011, 27, 394–407. [Google Scholar] [CrossRef]











| Parameter | Symbol | Unit | Numerical Value |
|---|---|---|---|
| Speed inlet | U∞ | m/s | 1.5 |
| Pressure outlet | P | Pa | 1 |
| Time Step | - | s | 1 × 10−3 |
| Kinematic viscosity number | m2/s | 1.01 × 10−6 | |
| density | ρ | kg/m3 | 1025 |
| Residual accuracy | - | - | 1.0 × 10−5 |
| Cylindrical diameter | D | mm | 20 |
| Source of Literature | Cd | St | Experiment or Simulation |
|---|---|---|---|
| Schewe | 1.18 | 0.21 | experiment |
| Zdravkovich | 1.2 | 0.20 | experiment |
| This article | 1.21 | 0.23 | simulation |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Qi, J.; Qi, Y.; Chen, Q.; Yan, F. A Study of Drag Reduction on Cylinders with Different V-Groove Depths on the Surface. Water 2022, 14, 36. https://doi.org/10.3390/w14010036
Qi J, Qi Y, Chen Q, Yan F. A Study of Drag Reduction on Cylinders with Different V-Groove Depths on the Surface. Water. 2022; 14(1):36. https://doi.org/10.3390/w14010036
Chicago/Turabian StyleQi, Jiyang, Yue Qi, Qunyan Chen, and Fei Yan. 2022. "A Study of Drag Reduction on Cylinders with Different V-Groove Depths on the Surface" Water 14, no. 1: 36. https://doi.org/10.3390/w14010036
APA StyleQi, J., Qi, Y., Chen, Q., & Yan, F. (2022). A Study of Drag Reduction on Cylinders with Different V-Groove Depths on the Surface. Water, 14(1), 36. https://doi.org/10.3390/w14010036





