Influence of Tubular Turbine Runaway for Back Pressure Power Generation on the Stability of Circulating Cooling Water System
Abstract
:1. Introduction
2. Numerical Calculation Method
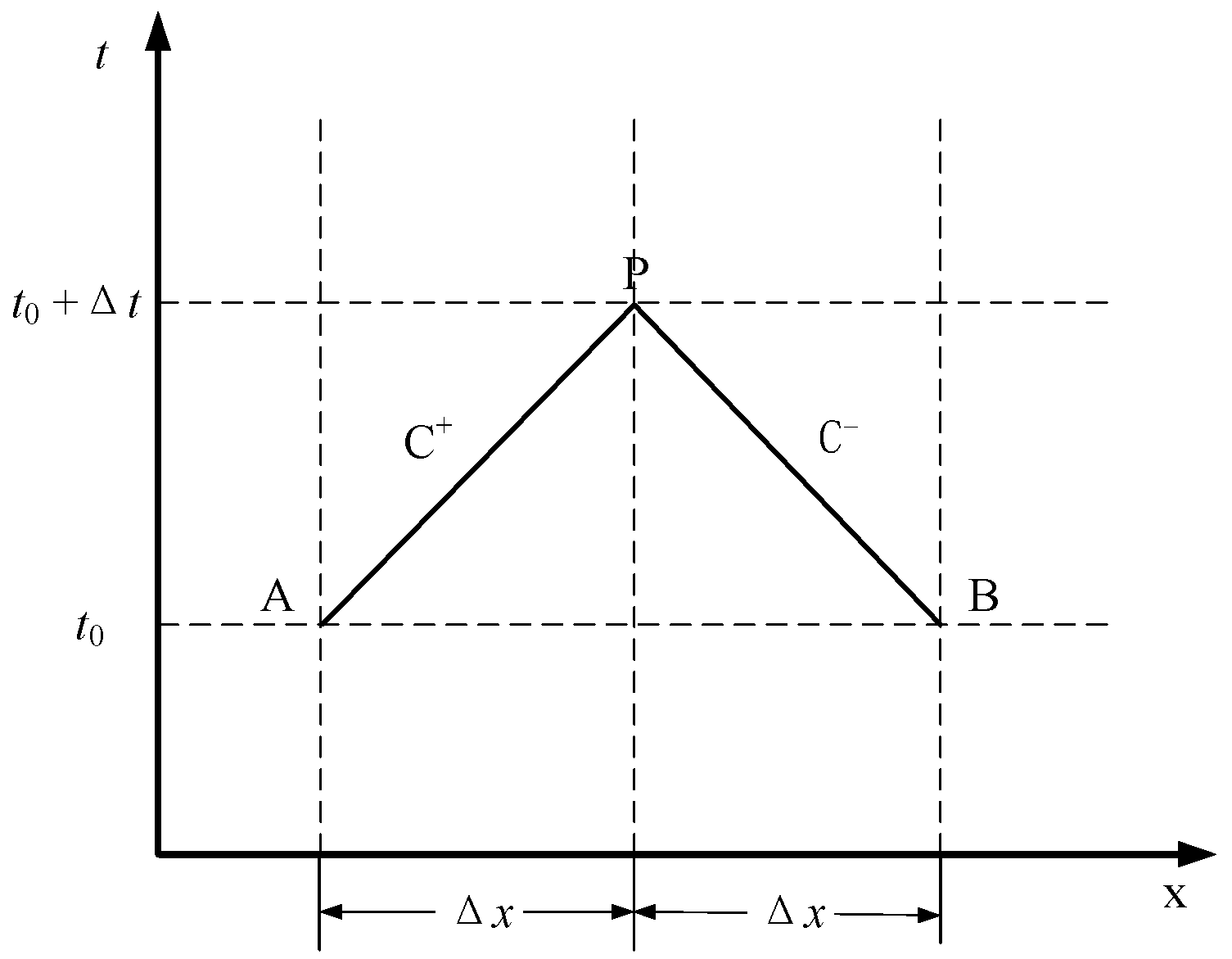
2.1. CCWS 1D MOC Method
2.2. 3D CFD Method for a Tubular Turbine
2.3. 1D MOC–3D CFD Coupled Simulation Method
3. Computational Model and Reliability Verification
3.1. System Coupling Model
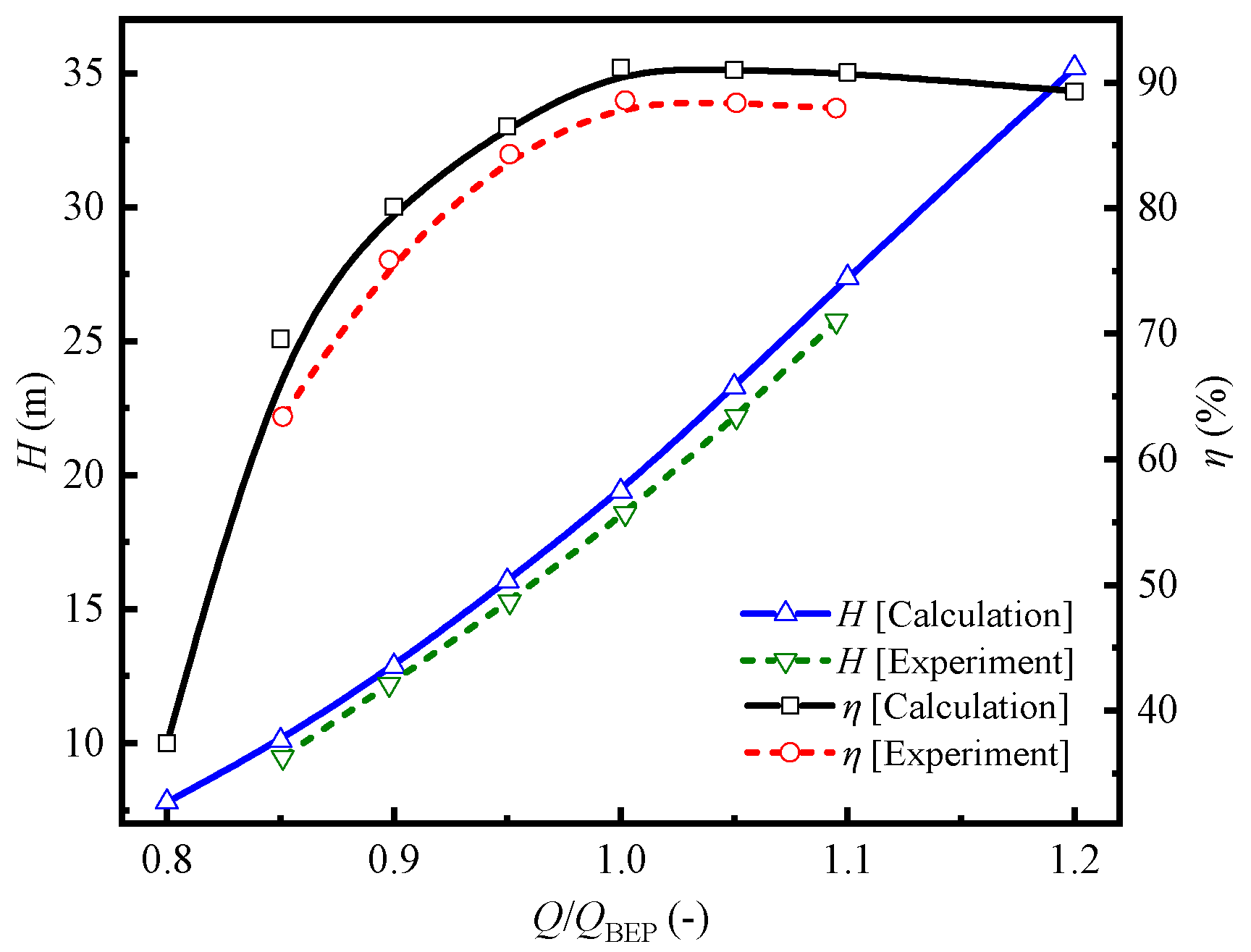
3.2. Comparative Analysis of the Coupling Algorithm and 1D MOC Calculation Results
4. Results and Discussion
4.1. Analysis of the Influence of the Runaway Process of the Turbine on the Main Parameters of the System
4.2. Analysis of the Pressure Pulsation of the Turbine and the Force Characteristics of the Runner during the Runaway Process
4.2.1. Influence of the Initial Flow Rate on the Runaway Process
4.2.2. Analysis of the Pressure Pulsation Characteristics
4.2.3. Analysis of the Force Characteristics of the Runner
4.3. Analysis on the Opening and Closing Law of the Control Valve during the Shutdown Process of the Turbine Runaway State
4.3.1. Process Analysis of Separate Closure of Tandem Valves
4.3.2. Analysis of the Separate Opening Process of the Parallel Valve
4.3.3. Synergistic Control Analysis of the Series and Parallel Valves
5. Conclusions
- (1)
- After the tubular turbine entered the runaway process, it did not cause major water hammer problems to the system. Before and after the runaway of the fault turbine, when the initial steady-state flow rate of the turbine is 0.85QBEP and 1.1QBEP, the return water pressure of the system decreases by 3% and 9%, the system flow rate increases by 0.5% and 1.8%, and the flow rate of the turbine in parallel with the fault turbine decreases by 4% and 8% respectively.
- (2)
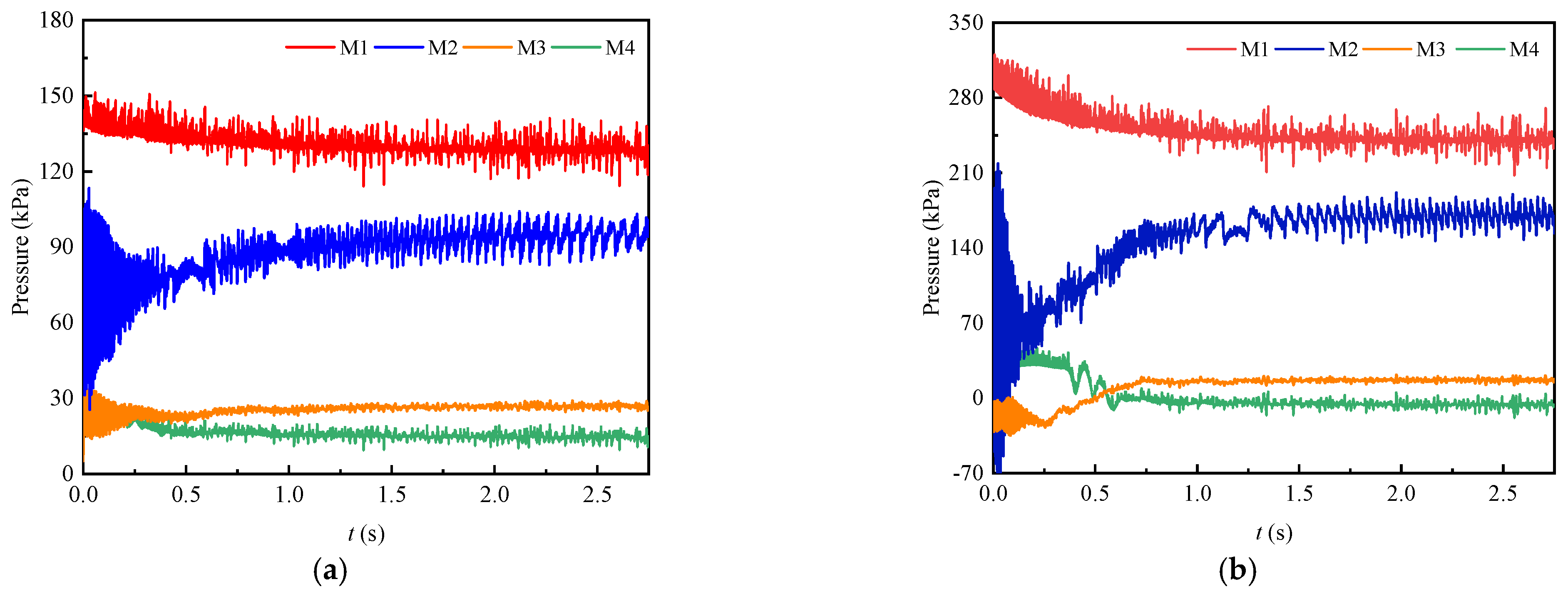
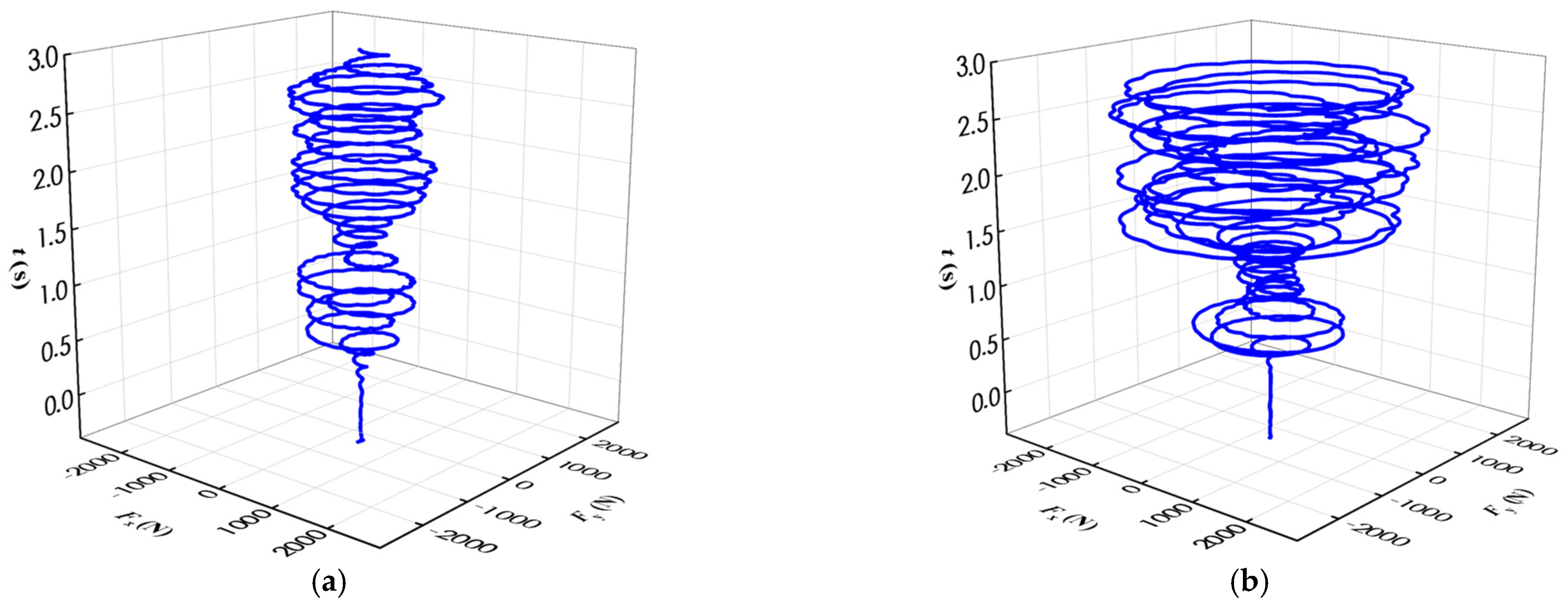
- When the tubular turbine enters the runaway process from the steady-state condition, the fault turbine shows the characteristics of increasing speed and flow rate. The change rates of speed and flow rate are great, and the time from the initial state to the runaway state is short with the increase in the initial flow rate. When the initial flow rate is 0.8QBEP, the runaway speed and flow rate of the turbine are 1.2 and 1.1 times the initial steady-state condition, respectively. When the initial flow rate is 1.1QBEP, the runaway speed and flow rate of the turbine are 1.7 and 1.2 times the initial steady-state condition, respectively. At the beginning of the runaway process, the pressure at the monitoring points in the runner area greatly fluctuated. The pressure oscillation amplitude of the monitoring points in the runner area increases with the increase in the initial steady-state flow rate, but the duration decreases. In addition, the radial force of the fault turbine runner in the runaway state greatly increases and shows violent oscillation compared with the initial steady-state condition. When the initial steady-state flow rate is 0.85QBEP and 1.1QBEP, the maximum radial force is 7 and 37 times the initial steady-state conditions, which will aggravate the shafting wear. Therefore, the runaway of hydraulic turbine in CCWS may lead to serious safety accidents, and it is necessary to shut it down as soon as possible.
- (3)
- During the shutdown of tubular turbine from runaway state, improper valve control scheme will cause severe positive and negative water hammer in the pipe network and seriously threaten the stable operation of the system. For the research system: Under different flow rate conditions, when the opening time of the parallel valve of the fault turbine is too short compared with the closing time of the series valve, the system return water pressure will produce a negative pressure wave, and the valley value of the negative pressure wave is about 69%~82% of the target pressure; On the contrary, when the opening time of the parallel valve is too long compared with the the closing time of the series valve, the return water pressure of the system will produce a positive pressure wave, and the peak value of the positive pressure wave is about 138%~160% of the target pressure; When the opening time of the parallel valve is 60% of the closing time of the series valve, the operating pressure of the system will return to the initial steady state smoothly.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Zhu, X.; Niu, D.; Wang, X.; Wang, F.; Jia, M. Comprehensive energy saving evaluation of circulating cooling water system based on combination weighting method. Appl. Therm. Eng. 2019, 157, 113735. [Google Scholar] [CrossRef]
- Wang, P.; Lu, J.; Cai, Q.; Chen, S.; Luo, X. Analysis and Optimization of Cooling Water System Operating Cost under Changes in Ambient Temperature and Working Medium Flow. Energies 2021, 14, 6903. [Google Scholar] [CrossRef]
- Gao, W.; Feng, X. The power target of a fluid machinery network in a circulating water system. Appl. Energy 2017, 205, 847–854. [Google Scholar] [CrossRef]
- Ma, J.; Wang, Y.; Feng, X. Energy recovery in cooling water system by hydro turbines. Energy 2017, 139, 329–340. [Google Scholar] [CrossRef]
- Li, Y.; Yuan, S.; Che, D. Utilization of residual energy of industrial circulating cooling water and its key technologies. J. Drain. Irrig. Mech. Eng. 2015, 7, 667–673. (In Chinese) [Google Scholar]
- Chen, H.; Zhou, D.; Kan, K.; Xu, H.; Zheng, Y.; Binama, M.; Xu, Z.; Feng, J. Experimental investigation of a model bulb turbine under steady state and load rejection process. Renew. Energy 2021, 169, 254–265. [Google Scholar] [CrossRef]
- Aponte, R.D.; Teran, L.A.; Grande, J.F.; Coronado, J.J.; Ladino, J.A.; Larrahondo, F.J.; Rodríguez, S.A. Minimizing erosive wear through a CFD multi-objective optimization methodology for different operating points of a Francis turbine. Renew. Energy 2020, 145, 2217–2232. [Google Scholar] [CrossRef]
- Whitacker, L.H.L.; Tomita, J.T.; Bringhenti, C. Effect of tip clearance on cavitating flow of a hydraulic axial turbine applied in turbopump. Int. J. Mech. Sci. 2022, 213, 106855. [Google Scholar] [CrossRef]
- Zhang, H.; Chen, D.; Wu, C.; Wang, X.; Lee, J.-M.; Jung, K.-H. Dynamic modeling and dynamical analysis of pump-turbines in S-shaped regions during runaway operation. Energy Convers. Manag. 2017, 138, 375–382. [Google Scholar] [CrossRef] [Green Version]
- Zhan, J. Analysis and treatment of runaway fault of unit in Feilaixia Hydropower Plant. Guide Get. Rich Sci. Technol. 2010, 2, 165–318. (In Chinese) [Google Scholar]
- Li, H.; Guo, L. Accident treatment of broken pin of bulb tubular turbine. Eng. Technol. 2015, 43, 116. (In Chinese) [Google Scholar]
- Feng, J.; Li, W.; Luo, X.; Zhu, G. Numerical analysis of transient characteristics of a bulb hydraulic turbine during runaway transient process. Proc. Inst. Mech. Eng. Part E J. Process Mech. Eng. 2018, 233, 813–823. [Google Scholar] [CrossRef]
- Chen, M.; Guo, J.; Sun, J. Influence of blade opening on runaway process of tubular turbine. Hydropower Energy Sci. 2022, 40, 4. (In Chinese) [Google Scholar]
- Ghidaoui, M.S.; Zhao, M.; McInnis, D.A.; Axworthy, D.H. A Review of Water Hammer Theory and Practice. Appl. Mech. Rev. 2005, 58, 49–76. [Google Scholar] [CrossRef]
- Lister, M. The numerical solution of hyperbolic partial differential equations by the method of characteristics. Math. Methods Digit. Comput. 1960, 1, 165–179. [Google Scholar]
- Singhal, A.K.; Athavale, M.M.; Li, H.; Jiang, Y. Mathematical Basis and Validation of the Full Cavitation Model. J. Fluids Eng. 2002, 124, 617–624. [Google Scholar] [CrossRef]
- Wang, W.; Pavesi, G.; Pei, J.; Yuan, S. Transient simulation on closure of wicket gates in a high-head Francis-type reversible turbine operating in pump mode. Renew. Energy 2020, 145, 1817–1830. [Google Scholar] [CrossRef]
- Li, Y.; Song, G.; Yan, Y. Transient hydrodynamic analysis of the transition process of bulb hydraulic turbine. Adv. Eng. Softw. 2015, 90, 152–158. [Google Scholar] [CrossRef]
- Yang, S.; Chen, X.; Wu, D.; Yan, P. Dynamic analysis of the pump system based on MOC–CFD coupled method. Ann. Nucl. Energy 2015, 78, 60–69. [Google Scholar] [CrossRef]
- Li, Z.; Bi, H.; Karney, B.; Wang, Z.; Yao, Z. Three-dimensional transient simulation of a prototype pump-turbine during normal turbine shutdown. J. Hydraul. Res. 2017, 55, 520–537. [Google Scholar] [CrossRef]
- Liu, D.; Zhang, X.; Yang, Z.; Liu, K.; Cheng, Y. Evaluating the pressure fluctuations during load rejection of two pump-turbines in a prototype pumped-storage system by using 1D-3D coupled simulation. Renew. Energy 2021, 171, 1276–1289. [Google Scholar] [CrossRef]
- Yang, Z.; Cheng, Y.; Xia, L.; Meng, W.; Liu, K.; Zhang, X. Evolutions of flow patterns and pressure fluctuations in a prototype pump-turbine during the runaway transient process after pump-trip. Renew. Energy 2020, 152, 1149–1159. [Google Scholar] [CrossRef]
- Fu, X.; Li, D.; Wang, H.; Li, Z.; Zhao, Q.; Wei, X. One- and three-dimensional coupling flow simulations of pumped-storage power stations with complex long-distance water conveyance pipeline system. J. Clean. Prod. 2021, 315, 128228. [Google Scholar] [CrossRef]
- Zhang, W. Partial Differential Equation Finite Difference Method in Scientific Calculation; Higher Education Press: Beijing, China, 2006. (In Chinese) [Google Scholar]
- Wang, L.; Xu, X. The Mathematical Basis of Finite Element Method; Science Press: Beijing, China, 2004. (In Chinese) [Google Scholar]
- Tan, W. Computational Shallow Water Dynamics: Application of Finite Volume Method; Tsinghua University Press: Beijing, China, 1998. (In Chinese) [Google Scholar]
- Ye, W.; Geng, C.; Luo, X. Unstable flow characteristics in vaneless region with emphasis on the rotor-stator interaction for a pump turbine at pump mode using large runner blade lean. Renew. Energy 2022, 185, 1343–1361. [Google Scholar] [CrossRef]
- Zhang, X.; Cheng, Y.; Yang, Z.; Chen, Q.; Liu, D. Water column separation in pump-turbine after load rejection: 1D-3D coupled simulation of a model pumped-storage system. Renew. Energy 2020, 163, 685–697. [Google Scholar] [CrossRef]
- Zhang, X.X.; Cheng, Y.G. Simulation of hydraulic transients in hydropower systems using the 1-D-3-D coupling approach. J. Hydrodyn. Ser. B 2012, 24, 595–604. [Google Scholar] [CrossRef]
- Walski, T.M.; Daviau, J.L.; Coran, S. Effect of Skeletonization on Transient Analysis Results. In Proceedings of the World Water & Environmental Resources Congress, Salt Lake City, UT, USA, 27 June–1 July 2004. [Google Scholar]
- Castro, M.M.; Song, T.W.; Pinto, J.M. Minimization of Operational Costs in Cooling Water Systems. Chem. Eng. Res. Des. 2000, 78, 192–201. [Google Scholar] [CrossRef]
- Su, W.-T.; Li, X.-B.; Xia, Y.-X.; Liu, Q.-Z.; Binama, M.; Zhang, Y.-N. Pressure fluctuation characteristics of a model pump-turbine during runaway transient. Renew. Energy 2020, 163, 517–529. [Google Scholar] [CrossRef]
- Zhang, M.; Wang, H.; Tsukamoto, H. Numerical Analysis of Unsteady Hydrodynamic Forces on a Diffuser Pump Impeller Due to Rotor-Stator Interaction. In Proceedings of the ASME 2002 Joint U.S.-European Fluids Engineering Division Conference. Volume 2: Symposia and General Papers, Parts A and B, Montreal, QC, Canada, 14–18 July 2002; pp. 751–760. [Google Scholar]
- Wei, Z.; Yin, G.; Wang, J.; Wan, L.; Jin, L. Stability analysis and supporting system design of a high-steep cut soil slope on an ancient landslide during highway construction of Tehran–Chalus. Environ. Earth Sci. 2012, 67, 1651–1662. [Google Scholar] [CrossRef]
- Yang, Z.; Zhou, L.; Dou, H.; Lu, C.; Luan, X. Water hammer analysis when switching of parallel pumps based on contra-motion check valve. Ann. Nucl. Energy 2019, 139, 107275. [Google Scholar] [CrossRef]
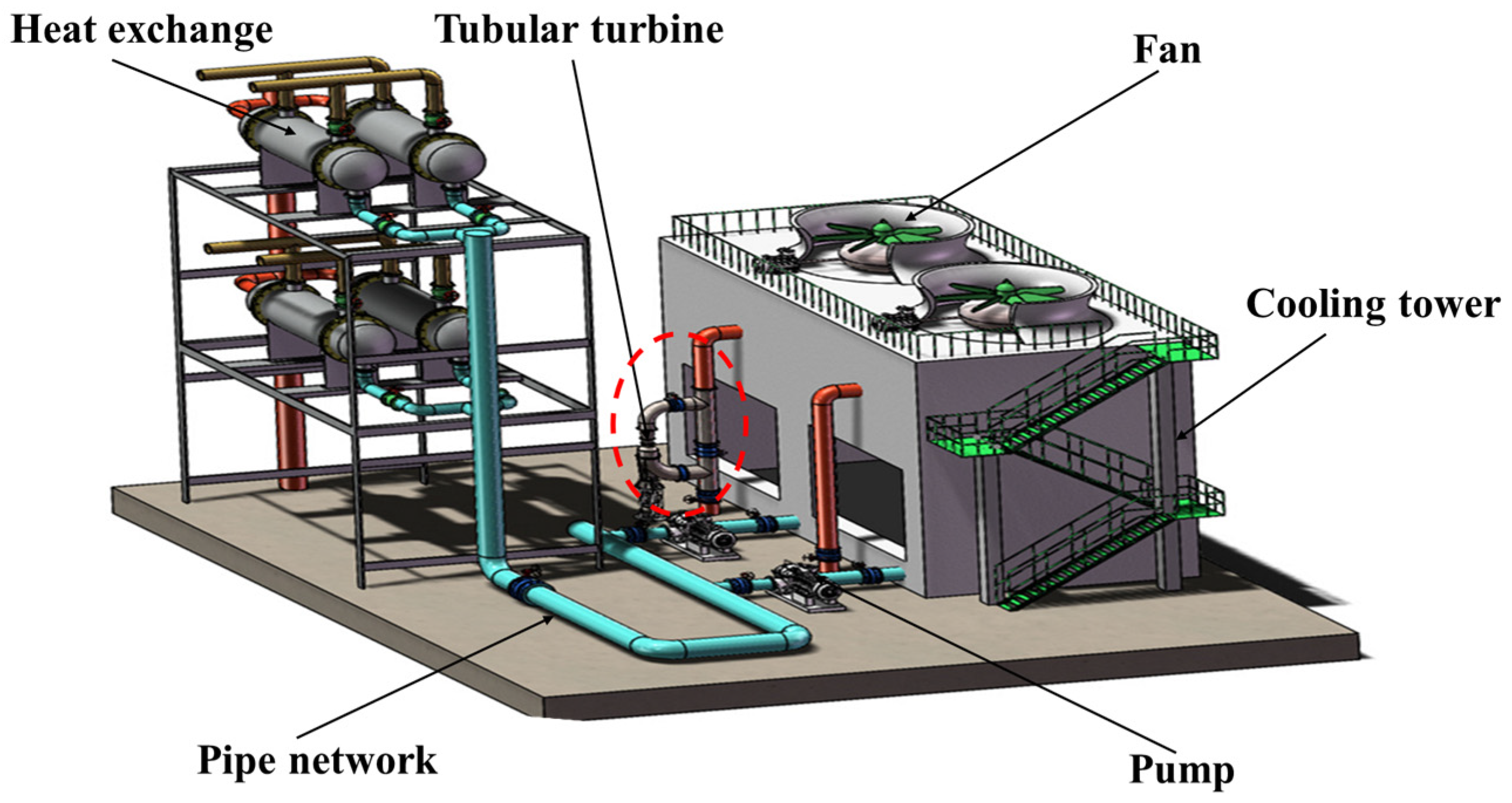
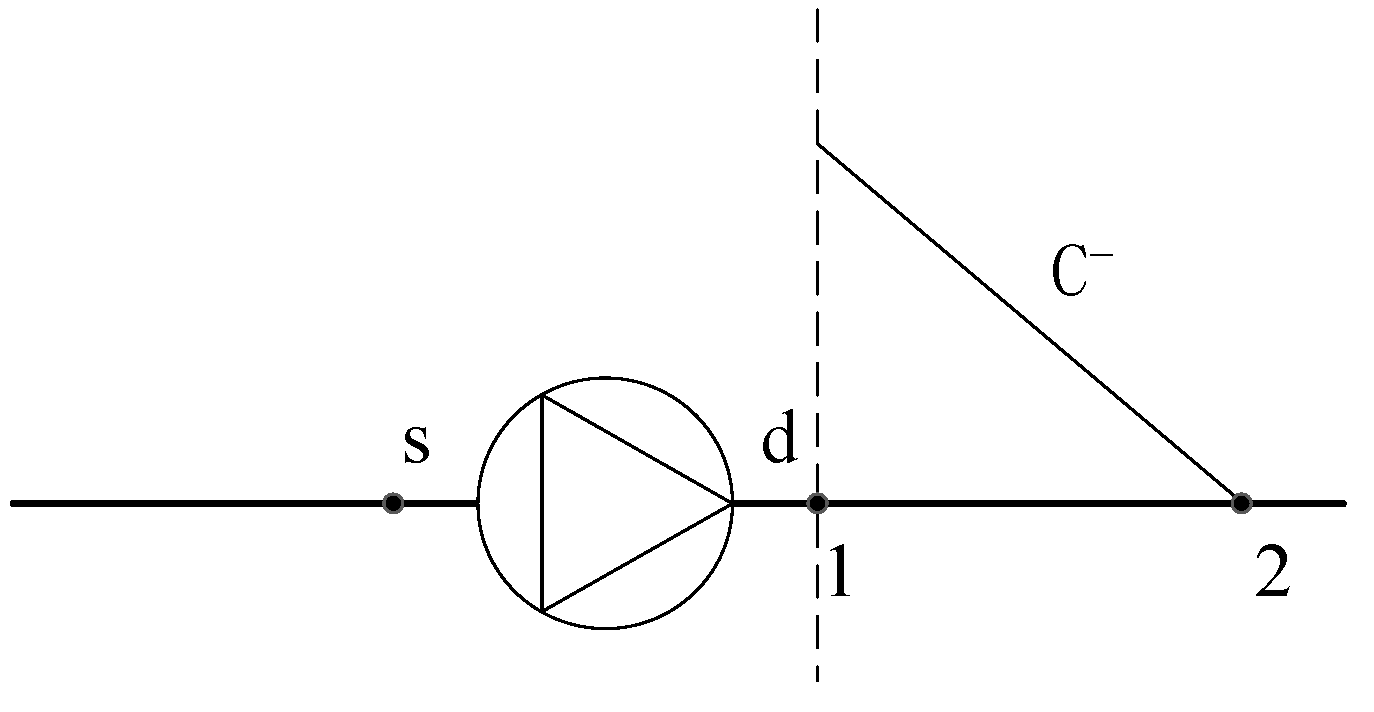













| Name | Date | Unit |
|---|---|---|
| Propeller blade | 5 | Piece |
| Vane | 12 | Piece |
| Blade opening | 21 | ° |
| Guide vane opening | 76 | ° |
| Runner speed | 1500 | r/min |
| Rotational inertia | 8 | Kg m2 |
| Runner diameter | 430 | mm |
| Bulb diameter | 450 | mm |
| Inlet diameter | 900 | mm |
| Outlet diameter | 800 | mm |
| Number of Mesh (Million) | Head (m) | Efficiency (%) |
|---|---|---|
| 298 | 19.18 | 91.09 |
| 382 | 19.35 | 91.14 |
| 456 | 19.36 | 91.15 |
| 533 | 19.36 | 91.15 |
| Runaway State | Q (m3/h) | H (m) | n (r/min) |
|---|---|---|---|
| 1D MOC | 5492 | 23.4 | 2539 |
| Coupling algorithm | 5893 | 22.1 | 2645 |
| Error rate (%) | 7.3 | 5.6 | 4.2 |
| Initial Steady State Condition Turbine Flow Rate | Fr Maximum (N) | |
|---|---|---|
| Initial Steady State Condition | Runaway State | |
| 0.85QBEP | 157 | 1290 |
| 1.1QBEP | 68 | 2510 |
| Scheme | V1 Valve | V2 Valve |
|---|---|---|
| Scheme 1 | Fully closed state | Linear off, 2 s fully off |
| Scheme 2 | Fully closed state | Linear off, 10 s fully off |
| Scheme 3 | Fully closed state | Linear off, 20 s fully off |
| Scheme | V1 Valve | V2 Valve |
|---|---|---|
| Scheme 1 | Linear opening, 2 s opening to resistance equivalent opening | Fully open |
| Scheme 2 | Linear opening, 10 s opening to resistance equivalent opening | Fully open |
| Scheme 3 | Linear opening, 20 s opening to resistance equivalent opening | Fully open |
| Scheme | V1 Valve | V2 Valve |
|---|---|---|
| Scheme 1 | Linear opening, 2 s opening to resistance equivalent opening | Linear off, 10 s fully off |
| Scheme 2 | Linear opening, 4 s opening to resistance equivalent opening | Linear off, 10 s fully off |
| Scheme 3 | Linear opening, 6 s opening to resistance equivalent opening | Linear off, 10 s fully off |
| Scheme 4 | Linear opening, 8 s opening to resistance equivalent opening | Linear off, 10 s fully off |
| Scheme 5 | Linear opening, 10 s opening to resistance equivalent opening | Linear off, 10 s fully off |
| Scheme 6 | Linear opening, 20 s opening to resistance equivalent opening | Linear off, 10 s fully off |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, P.; Luo, X.; Lu, J.; Gao, J.; Cai, Q. Influence of Tubular Turbine Runaway for Back Pressure Power Generation on the Stability of Circulating Cooling Water System. Water 2022, 14, 2294. https://doi.org/10.3390/w14152294
Wang P, Luo X, Lu J, Gao J, Cai Q. Influence of Tubular Turbine Runaway for Back Pressure Power Generation on the Stability of Circulating Cooling Water System. Water. 2022; 14(15):2294. https://doi.org/10.3390/w14152294
Chicago/Turabian StyleWang, Peng, Xingqi Luo, Jinling Lu, Jiawei Gao, and Qingsen Cai. 2022. "Influence of Tubular Turbine Runaway for Back Pressure Power Generation on the Stability of Circulating Cooling Water System" Water 14, no. 15: 2294. https://doi.org/10.3390/w14152294
APA StyleWang, P., Luo, X., Lu, J., Gao, J., & Cai, Q. (2022). Influence of Tubular Turbine Runaway for Back Pressure Power Generation on the Stability of Circulating Cooling Water System. Water, 14(15), 2294. https://doi.org/10.3390/w14152294





