Adsorption of Phosphate and Nitrate Ions on Oxidic Substrates Prepared with a Variable-Charge Lithological Material
Abstract
:1. Introduction
2. Materials and Methods
2.1. Oxidic Lithological Material
2.2. Chemical Analysis
2.3. Preparation of the Adsorbent Substrate and Activation of Positive Surface Charges
2.4. Adsorption Studies
2.5. Adsorption Isotherm Modeling
2.6. Adsorption Kinetic Modeling
3. Results and Discussion
3.1. pH, Electrical Conductivity (EC), and Cation Exchange Capacity (CEC)
3.2. BET Surface Area Studies
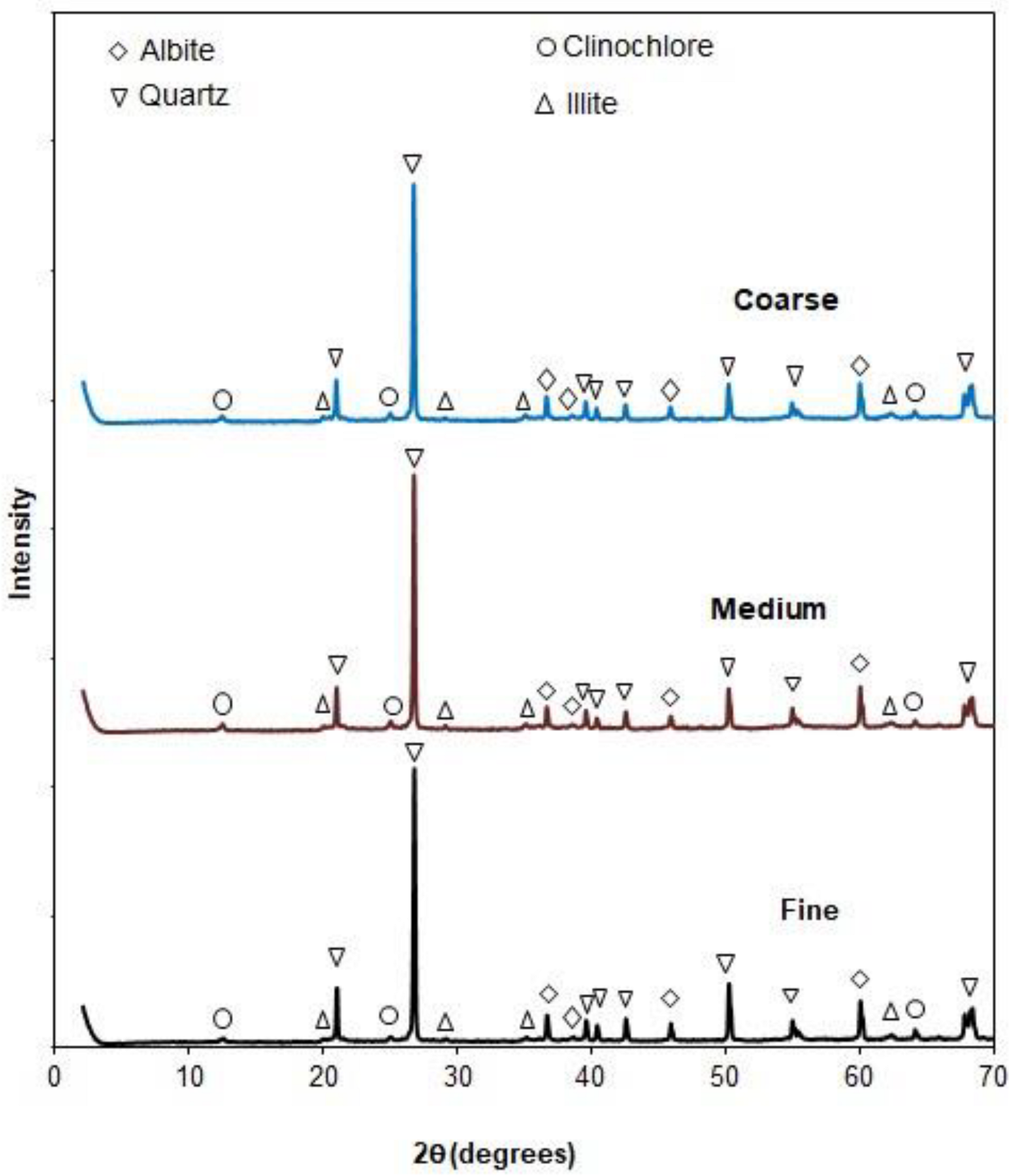
3.3. RX Diffraction
3.4. Differential Thermal Analysis (DTA)
3.5. Protonation Reaction on the Calcined Substrate; Activation Reaction
3.6. Phosphate Ion Adsorption
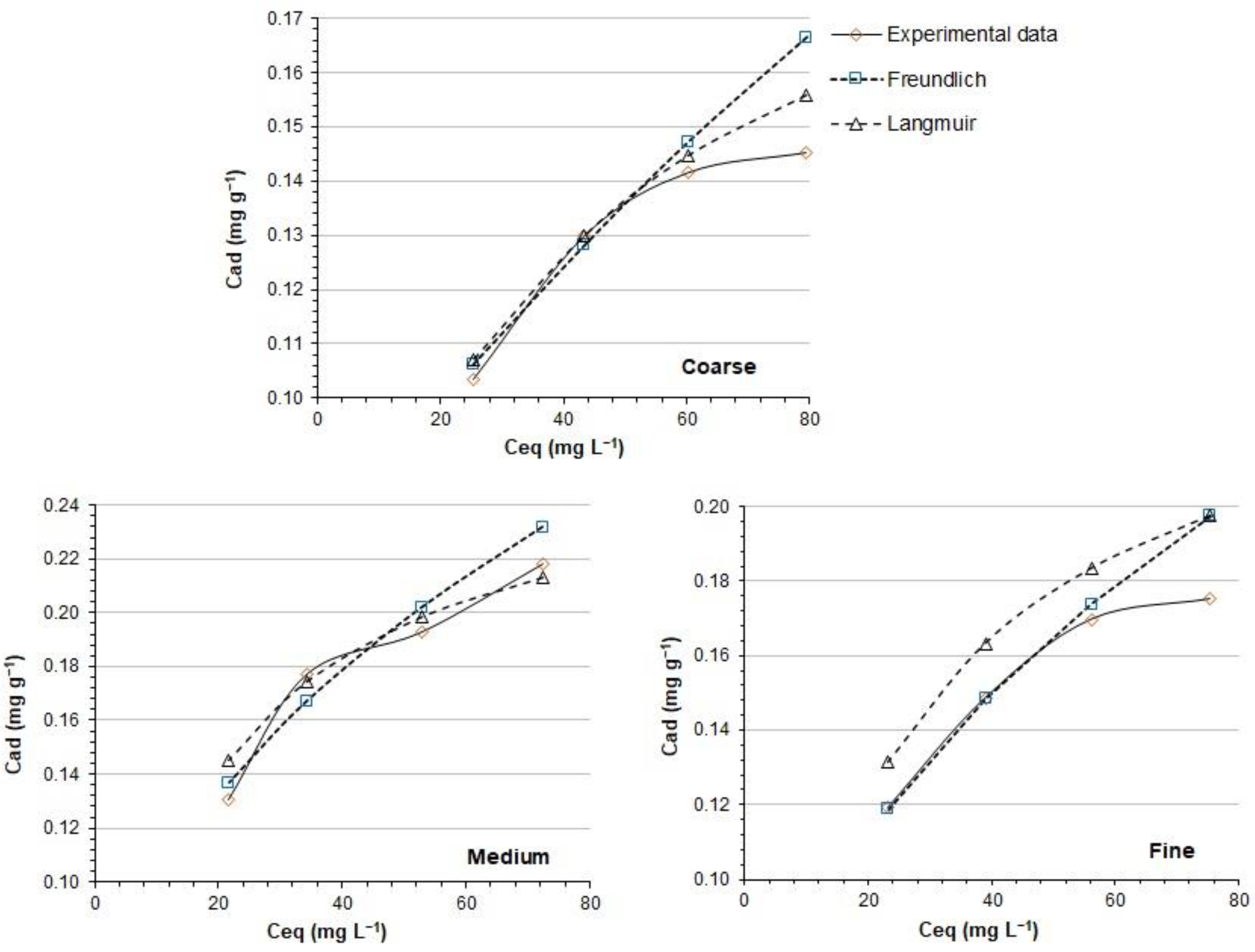
3.7. Phosphate Isotherms
3.8. Phosphate Kinetic
3.9. Nitrate Ion Adsorption
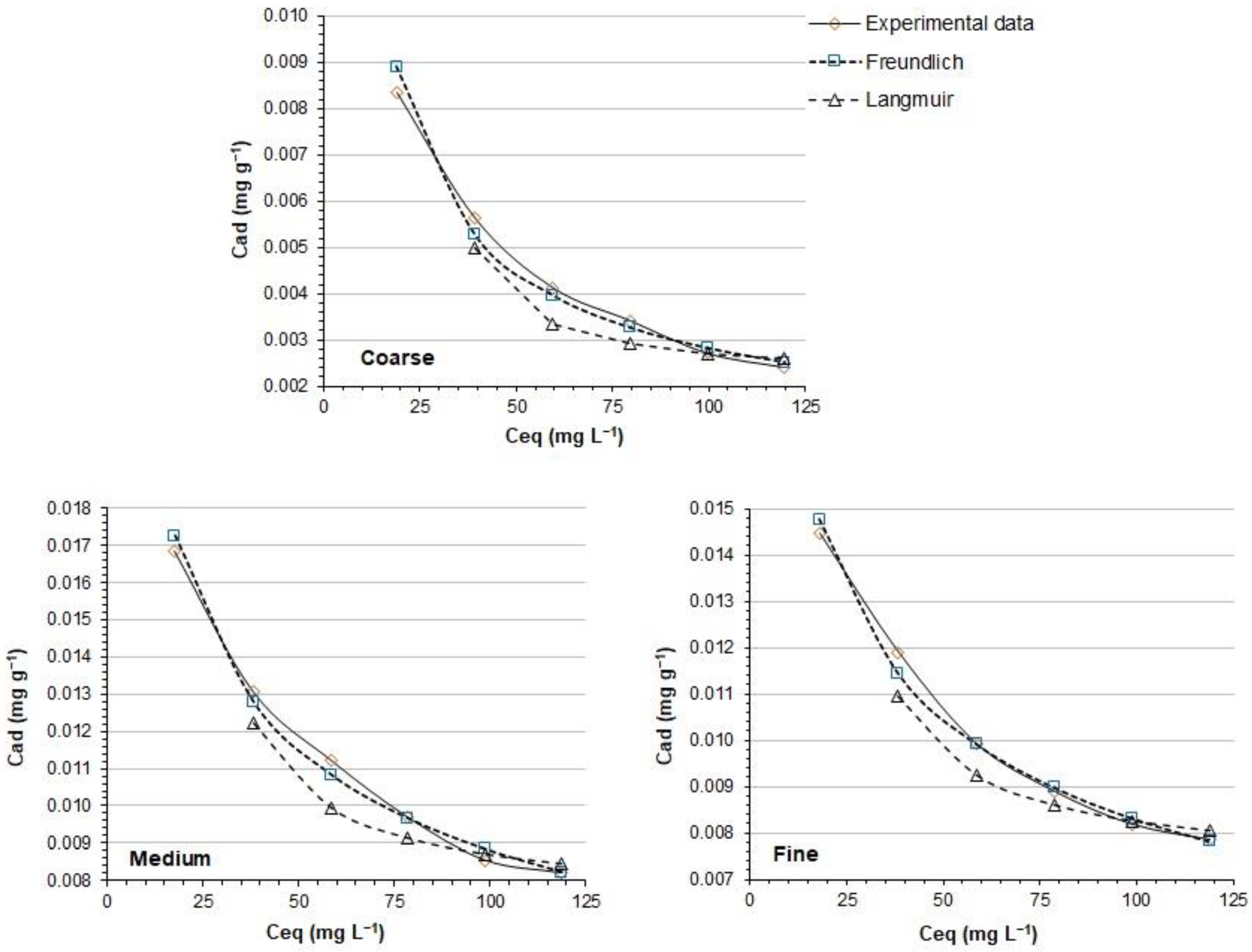
3.10. Nitrate Isotherms
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Payen, S.; Falconer, S.; Carlson, B.; Yang, W.; Ledgard, S. Eutrophication and Climate Change Impacts of a Case Study of New Zealand beef to the European Market. Sci. Total Environ. 2020, 710, 136120. [Google Scholar] [CrossRef]
- Singh, A.; Tripathi, A.; Kumar, A.; Singh, V. Nitrate and Phosphate Contamination in Ground Water of Varanasi, Uttar Pradesh, India. J. Ind. Res. Technol. 2012, 2, 26–32. [Google Scholar]
- Vicente-Martínez, Y.; Caravaca, M.; Soto-Meca, A.; Martín-Pereira, M.Á.; García-Onsurbe, M.d.C. Adsorption Studies on Magnetic Nanoparticles Functionalized with Silver to Remove Nitrates from Waters. Water 2021, 13, 1757. [Google Scholar] [CrossRef]
- Fanfan, L.; Yungen, L.; Yan, W.; Silin, Y.; Rong, M. Preparation of Structured Biochar, its Adsorption Capacity of N and P and its Characterization. Water Sci. Technol. 2022, 85, 2443–2462. [Google Scholar] [CrossRef]
- Huang, W.; Zhang, Y.; Li, D. Adsorptive Removal of Phosphate from Water using Mesoporous Materials: A Review. J. Environ. Manag. 2017, 193, 470–482. [Google Scholar] [CrossRef]
- Liang, Q.; Fu, X.; Wang, P.; Li, X.; Zheng, P. Dynamic Adsorption Characteristics of Phosphorus Using MBCQ. Water 2022, 14, 508. [Google Scholar] [CrossRef]
- Fan, T.; Wang, M.; Wang, X.; Chen, Y.; Wang, S.; Zhan, H.; Chen, X.; Lu, A.; Zha, S. Experimental Study of the Adsorption of Nitrogen and Phosphorus by Natural Clay Minerals. Adsorp. Sci. Technol. 2021, 2021, 4158151. [Google Scholar] [CrossRef]
- Wang, Y.; He, H.; Zhang, N.; Shimizu, K.; Lei, Z.; Zhang, Z. Efficient Capture of Phosphate from Aqueous Solution using Acid Activated Akadama Clay and Mechanisms Analysis. Water Sci. Technol. 2018, 78, 1603–1614. [Google Scholar] [CrossRef]
- Millán, F.; Prato, J.G.; García, O.; Díaz, I.; Sánchez, M. Adsorción de Iones Cu2+ y Zn2+ por Materiales Litológicos de Carga Variable Provenientes de Suelos del Estado Mérida, Venezuela. Rev. Técnica Fac. Ing. Univ. Zulia 2013, 36, 195–201. [Google Scholar]
- Yang, L.; Yang, M.; Xu, P.; Zhao, X.; Bai, H.; Li, H. Characteristics of Nitrate Removal from Aqueous Solution by Modified Steel Slag. Water 2017, 9, 757. [Google Scholar] [CrossRef] [Green Version]
- Prato, J.G.; González-Ramírez, L.C.; Pérez, M.C.; Rodríguez, M.E. Adsorción de la Dureza del Agua sobre Lechos de Rocas Volcánicas de Ecuador. Inf. Tecnol. 2021, 32, 51–60. [Google Scholar] [CrossRef]
- Xu, R.K.; Qafoku, N.P.; Van Ranst, E.; Li, J.Y.; Jiang, J. Adsorption Properties of Subtropical and Tropical Variable Soils: Implication from Climate Change and Biochard Amendment. Adv. Agron. 2016, 135, 1–58. [Google Scholar]
- Gu, S.; Kang, X.; Wang, L.; Lichtfouse, E.; Wang, C. Clay Mineral Adsorbents for Heavy Metal Removal from Wastewater: A Review. Environ. Chem. Lett. 2019, 17, 629–654. [Google Scholar] [CrossRef]
- Millán, F.; Prato, J.G.; González, L.C.; Márquez, A.; Djabayan, P. Cu (II) Chemisorption on Calcined Substrates made with an Oxidic Refractory Variable Charges Lithological Material. Rev. Técnica Fac. Ing. Univ. Zulia 2019, 32, 10–18. [Google Scholar] [CrossRef] [Green Version]
- Qafoku, N.P.; Van Ranst, E.; Noble, A.; Baert, G. Variable Charge Coils: Their Mineralogy, Chemistry and Management. Adv. Agron. 2004, 84, 159–214. [Google Scholar]
- Prato, J.G.; Millán, F.; González, L.C.; Ríos, I.; Márquez, A.; Sánchez, J.; Palomares, A.E.; Díaz, J.I. Evaluación de Materiales Litológicos Oxídicos como Adsorbentes para el Tratamiento de Efluentes y Aguas Residuales. Novasinergia 2021, 4, 93–110. [Google Scholar]
- Wang, Y.; Jiang, J.; Xu, R.K.; Tiwari, D. Phosphate Adsorption at Variable Charge Soils/water Interfaces as Influenced by Ionic Strength. Aust. J. Soil Res. 2009, 47, 529–536. [Google Scholar] [CrossRef]
- Prato, J.G.; Millán, F.; Ríos, A.; González-Ramírez, L.C. Uso de Materiales Litológicos Oxídicos para la Reducción de la Dureza en Aguas Naturales. Inf. Technol. 2022, 33, 145–156. [Google Scholar] [CrossRef]
- Xu, R.; Wang, Y.; Tiwari, D.; Wang, H. Effect of Ionic Strength on Adsorption of As(III) and As(V) on variable charge soils. J. Environ. Sci. 2009, 21, 927–932. [Google Scholar] [CrossRef]
- Agarwal, M.; Singh, K. Heavy Metal Removal from Wastewater using Various Adsorbents: A Review. J. Water Reuse Desal. 2017, 7, 387–419. [Google Scholar]
- Márquez, A.; Millán, F.; Prato, J.G.; La Cruz, C. Adsorción de Iones Cr(VI) sobre Lechos Adsorbentes Calcinados con Superficie de Carga Variable Químicamente Modificada. Rev. Técnica Fac. Ing. Univ. Zulia 2020, 43, 72–81. [Google Scholar] [CrossRef]
- Pansu, M.; Gautheyrou, J. Handbook of Soil Analysis; Springer: Berlin/Heidelberg, Germany, 2006; pp. 551–656. [Google Scholar]
- McKean, S.J. Manual de Análisis de Suelo y Tejido Vegetal; Centro Internacional de Agricultura Tropical: Bogotá, Colombia, 1993; pp. 95–99. [Google Scholar]
- Rice, E.W.; Baird, R.D.; Eaton, A.D. Standard Methods for the Examination of Water and Wastewater, 23rd ed.; American Public Health Association, American Water Works Association, Water Environment Federation: Washington, DC, USA, 2017. [Google Scholar]
- Márquez, C.O.; García, V.J.; Guaypatin, J.R.; Fernández-Martínez, F.; Ríos, A.C. Cationic and Anionic Dye Adsorption on a Natural Clayey Composite. Appl. Sci. 2021, 11, 5127. [Google Scholar] [CrossRef]
- Foo, K.Y.; Hameed, B.H. Insights into the Modeling of Adsorption Isotherm Systems. Chem. Eng. J. 2010, 156, 2–10. [Google Scholar] [CrossRef]
- Al-Ghouti, M.A.; Da’ana, D.A. Guidelines for the use and Interpretation of Adsorption Isotherm Models: A Review. J. Hazard. Mater. 2020, 393, 122383. [Google Scholar] [CrossRef]
- Kalam, S.; Abu-Khamsin, S.A.; Kamal, M.S.; Shirish Patil, S. Surfactant Adsorption Isotherms: A Review. ACS Omega 2021, 6, 32342–32348. [Google Scholar] [CrossRef]
- Tran, H.N.; You, S.J.; Hosseini-Bandegharaei, A.; Chao, H.P. Mistakes and Inconsistencies Regarding Adsorption of Contaminants from Aqueous Solutions: A Critical Review. Water Res. 2017, 120, 88–116. [Google Scholar] [CrossRef]
- Plazinski, W.; Rudzinski, W.; Plazinska, A. Theoretical Models of Sorption Kinetics Including a Surface Reaction Mechanism: A Review. Adv. Colloid Interface Sci. 2009, 152, 2–13. [Google Scholar] [CrossRef]
- Guaya, D.; Valderrama, C.; Farran, A.; Armijos, C.; Cortina, J.L. Simultaneous Phosphate and Ammonium Removal from Aqueous Solution by a Hydrated Alumium Oxide Modified Natural Zeolite. Chem. Eng. J. 2015, 271, 204–213. [Google Scholar] [CrossRef] [Green Version]
- Blanchard, G.; Maunaye, M.; Martin, G. Removal of Heavy Metals from Waters by Means of Natural Zeolites. Water Res. 1984, 18, 1501–1507. [Google Scholar] [CrossRef]
- Ho, Y.S. Review of Second-order Models for Adsorption Systems. J. Hazard. Mater. 2006, 136, 681–689. [Google Scholar] [CrossRef] [Green Version]
- Tan, K.H. Principles of Soil Chemistry, 4th ed.; CRC Press: Boca Raton, FL, USA, 2011; pp. 133–241. [Google Scholar]
- Kwon, S.; Hwang, H.; Lee, Y. Effect of Pressure Treatment on the Specific Surface Area in Kaolin Group Minerals. Crystals 2019, 9, 528. [Google Scholar] [CrossRef] [Green Version]
- Manoharan, C.; Sutharsan, P.; Dhanapandian, S.; Venkatachalapathy, R. Characteristics of Some Clay Materials from Tamilnadu, India, and their Possible Ceramic Uses. Ceramica 2012, 58, 412–418. [Google Scholar] [CrossRef] [Green Version]
- Húlan, T.; Trník, A.; Medved, I. Kinetics of Thermal Expansion of Illite-based Ceramics in the Dehydroxylation Region during Heating. J. Therm. Anal. Calorim. 2017, 127, 291–298. [Google Scholar] [CrossRef]
- Gasparini, E.; Serena, C.; Tarantino, S.C.; Ghigna, P.; Pia Riccardi, M.; Cedillo-González, E.I.; Siligardi, C.; Zema, M. Thermal Dehydroxylation of Kaolinite under Isothermal Conditions. Appl. Clay Sci. 2013, 80–81, 417–425. [Google Scholar] [CrossRef]
- Rahman, M.A.; Lamb, D.; Kunhikrishnan, A.; Rahman, M.M. Kinetics, Isotherms and Adsorption–Desorption Behavior of Phosphorus from Aqueous Solution Using Zirconium–Iron and Iron Modified Biosolid Biochars. Water 2021, 13, 3320. [Google Scholar] [CrossRef]
- Munar-Florez, D.A.; Varón-Cárdenas, D.A.; Ramírez-Contreras, N.E.; García-Núñez, J.A. Adsorption of Ammonium and Phosphates by Biochar Produced from Oil Palm Shells: Effects of Production Conditions. Result. Chem. 2021, 3, 100119. [Google Scholar] [CrossRef]
- Frišták, V.; Pipíška, M.; Turčan, V.; Bell, S.M.; Laughinghouse, H.D., IV; Ďuriška, L.; Soja, G. Preparation and Characterization of Novel Magnesium Composite/Walnut Shells-Derived Biochar for As and P Sorption from Aqueous Solutions. Agriculture 2021, 11, 714. [Google Scholar] [CrossRef]
- Luo, X.; Wang, X.; Bao, S.; Liu, X.; Zhang, W.; Fang, T. Adsorption of Phosphate in Water using One-step Synthesised Zirconium-loaded Reduced Graphene Oxide. Sci. Rep. 2016, 6, 38824. [Google Scholar] [CrossRef] [Green Version]
- Mahdavi, S.; Akhzari, D. The Removal of Phosphate from Aqueous Solutions using Two Nano-structures: Copper Oxide and Carbon Tubes. Clean Technol. Environ. Policy 2016, 18, 817–827. [Google Scholar] [CrossRef]
- Almanassra, I.W.; Al-Ansari, T.; Kochkodan, V.; Mckay, G.; Ali Atieh, M. Review of Phosphate Removal from Water by Carbonaceous Sorbents. J. Environ. Manag. 2021, 287, 112245. [Google Scholar] [CrossRef]
- Adamson, A.; Gast, A. Physical Chemistry of Surfaces, 6th ed.; John and Wiley and Sons: Hoboken, NJ, USA, 1997; pp. 1–808. [Google Scholar]
- Kang, J.K.; Kim, J.H.; Kim, S.B.; Lee, S.H.; Choi, J.W.; Lee, C.G. Ammonium-functionalized Mesoporous Silica MCM-41 for Phosphate Removal from Aqueous Solutions. Desalination Water Treat. 2016, 53, 10839–10849. [Google Scholar] [CrossRef]
- Ayawei, N.; Ebelegi, A.N.; Wankasi, D. Modelling and Interpretation of Adsorption Isotherms. J. Chem. 2017, 2017, 3039817. [Google Scholar] [CrossRef]
- Goss, M.; Goorahoo, D. Nitrate Contamination of Groundwater: Measurement and Prediction. Fertil. Res. 1995, 42, 331–338. [Google Scholar] [CrossRef]
- Hong, B.D.; Lee, K.S.; Lee, D.S.; Rhie, J.H.; Bae, H.S.; Seo, I.H.; Song, S.G.; Chung, D.Y. Competitive Adsorption and Subsequent Desorption of Sulfate in the Presence of Various Anions in Soils. Korean J. Soil Sci. Fert. 2016, 49, 541–547. [Google Scholar] [CrossRef] [Green Version]
- Chintala, R.; Mollinedo, J.; Schumacher, T.E.; Papiernik, S.K.; Malo, D.D.; Clay, D.E.; Kumar, S.; Gulbrandson, D.W. Nitrate Sorption and Desorption in Biochars from Fast Pyrolysis. Microporous Mesoporous Mater. 2013, 179, 250–257. [Google Scholar] [CrossRef]
- Gai, X.; Wang, H.; Liu, J.; Zhai, L.; Liu, S.; Ren, T.; Liu, H. Effects of Feedstock and Pyrolysis Temperature on Biochar Adsorption of Ammonium and Nitrate. PLoS ONE 2014, 9, e113888. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Fidel, R.B.; Laird, D.A.; Spokas, K.A. Sorption of Ammonium and Nitrate to Biochars is Electrostatic and pH-dependent. Sci. Rep. 2018, 8, 17627. [Google Scholar] [CrossRef]
- Lazaratoua, C.V.; Vayenasb, D.V.; Papoulis, D. The Role of Clays, Clay Minerals and Clay-based Materials for Nitrate Removal from Water Systems: A Review. Appl. Clay Sci. 2020, 185, 105377. [Google Scholar] [CrossRef]
- Haan, F.A.M. The negative Adsorption of Anions (anion exclusion) in Systems Interacting Double Layers. J. Phys. Chem. 1964, 68, 2970–2977. [Google Scholar] [CrossRef]
- Mouat, M.C.H. Negative Adsorption of Phosphate by Plant Roots. N. Z. J. Agric. Res. 1983, 26, 489–492. [Google Scholar] [CrossRef]





| Fraction | pH | EC (dS m−1) | CEC cmol(+) kg−1 |
|---|---|---|---|
| Coarse | 5.0 | 0.01 | 54.9 |
| Medium | 5.0 | 0.02 | 56.3 |
| Fine | 4.8 | 0.01 | 49.8 |
| Fraction | External Surface Area (m2 g−1) | BET Surface Area (m2 g−1) | Micropore Area (m2 g−1) | Pore Volume (mm3 g−1) | Micropore Volume (mm3 g−1) | Pore Diameter (Å) |
|---|---|---|---|---|---|---|
| Coarse | 3.094 | 5.281 | 2.085 | 45.37 | 1.034 | 72.27 |
| Medium | 5.345 | 9.747 | 6.375 | 80.65 | 2.805 | 75.12 |
| Fine | 13.941 | 21.505 | 7.564 | 80.78 | 3.721 | 78.67 |
| Fraction | Treatment | Percolated Volume (mL) | mg Input | mg Output | mg Adsorbed | % Reaction | mg Ads/100 g Bed |
|---|---|---|---|---|---|---|---|
| Coarse | Non-activated | 140 | 14.000 | 13.511 | 0.489 | 3.5 | 0.387 |
| HCl | 200 | 20.000 | 14.914 | 5.086 | 25.4 | 3.150 | |
| H2SO4 | 160 | 16.000 | 11.998 | 4.002 | 25.0 | 2.429 | |
| Medium | Non-activated | 140 | 14.000 | 13.493 | 0.507 | 3.6 | 0.398 |
| HCl | 200 | 20.000 | 14.014 | 5.976 | 29.9 | 3.628 | |
| H2SO4 | 160 | 16.000 | 11.461 | 4.539 | 28.4 | 2.783 | |
| Fine | Non-activated | 140 | 14.000 | 13.504 | 0.496 | 3.5 | 0.390 |
| HCl | 200 | 20.000 | 14.856 | 5.128 | 25.7 | 3.193 | |
| H2SO4 | 160 | 16.000 | 11.988 | 4012 | 25.1 | 2.443 |
| Fraction | Freundlich | Langmuir | |||||
|---|---|---|---|---|---|---|---|
| KA | n | r | qm | bA | r | ||
| HCl | Coarse | 0.031 | 0.448 | 0.927 | 0.321 | 0.026 | 0.917 |
| Medium | 0.039 | 0.456 | 0.990 | 0.266 | 0.050 | 0.985 | |
| Fine | 0.035 | 0.419 | 0.975 | 0.263 | 0.057 | 0.964 | |
| H2SO4 | Coarse | 0.031 | 0.381 | 0.984 | 0.195 | 0.048 | 0.995 |
| Medium | 0.036 | 0.438 | 0.990 | 0.266 | 0.055 | 0.994 | |
| Fine | 0.030 | 0.433 | 0.970 | 0.255 | 0.046 | 0.964 | |
| Fraction | qe, exp (mg g−1) | Pseudo-First-Order | Pseudo-Second-Order | |||||
|---|---|---|---|---|---|---|---|---|
| K1 (min−1) | qe (mg g−1) | r | K2 (g mg−1 min−1) | qe (g mg−1) | r | |||
| HCl | Coarse | 0.1663 | 0.0191 | 0.0043 | 0.876 | 0.2465 | 0.1683 | 0.994 |
| Medium | 0.2152 | 0.0092 | 0.1271 | 0.899 | 0.1744 | 0.2191 | 0.993 | |
| Fine | 0.1799 | 0.0108 | 0.1023 | 0.821 | 0.2361 | 0.1852 | 0.996 | |
| H2SO4 | Coarse | 0.1615 | 0.0207 | 0.0039 | 0.882 | 0.2766 | 0.1639 | 0.995 |
| Medium | 0.1955 | 0.0067 | 0.1001 | 0.875 | 0.2185 | 0.1957 | 0.988 | |
| Fine | 0.1716 | 0.0085 | 0.0954 | 0.875 | 0.2743 | 0.1719 | 0.994 | |
| Fraction | Treatment | Percolated Volume (mL) | mg Input | mg Output | mg Adsorbed | % Reaction | mg Ads/100 g Bed |
|---|---|---|---|---|---|---|---|
| Non-activated | 160 | 4.800 | 4.782 | 0.018 | 0.37 | 0.014 | |
| Coarse | HCl | 200 | 6.000 | 5.863 | 0.137 | 2.28 | 0.085 |
| H2SO4 | 200 | 6.000 | 5.961 | 0.039 | 0.64 | 0.023 | |
| Non-activated | 160 | 4.800 | 4.780 | 0.020 | 0.41 | 0.015 | |
| Medium | HCl | 200 | 6.000 | 5.796 | 0.204 | 3.41 | 0.126 |
| H2SO4 | 200 | 6.000 | 5.934 | 0.066 | 1.11 | 0.041 | |
| Non-activated | 160 | 4.800 | 4.781 | 0.019 | 0.39 | 0.014 | |
| Fine | HCl | 200 | 6.000 | 5.906 | 0.094 | 1.56 | 0.058 |
| H2SO4 | 160 | 4.800 | 4.761 | 0.039 | 0.81 | 0.024 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Prato, J.G.; Millán, F.C.; González, L.C.; Ríos, A.C.; López, E.; Ríos, I.; Navas, S.; Márquez, A.; Carrero, J.C.; Díaz, J.I. Adsorption of Phosphate and Nitrate Ions on Oxidic Substrates Prepared with a Variable-Charge Lithological Material. Water 2022, 14, 2454. https://doi.org/10.3390/w14162454
Prato JG, Millán FC, González LC, Ríos AC, López E, Ríos I, Navas S, Márquez A, Carrero JC, Díaz JI. Adsorption of Phosphate and Nitrate Ions on Oxidic Substrates Prepared with a Variable-Charge Lithological Material. Water. 2022; 14(16):2454. https://doi.org/10.3390/w14162454
Chicago/Turabian StylePrato, José Gregorio, Fernando Carlos Millán, Luisa Carolina González, Anita Cecilia Ríos, Esteban López, Iván Ríos, Siboney Navas, Andrés Márquez, Julio César Carrero, and Juan Isidro Díaz. 2022. "Adsorption of Phosphate and Nitrate Ions on Oxidic Substrates Prepared with a Variable-Charge Lithological Material" Water 14, no. 16: 2454. https://doi.org/10.3390/w14162454
APA StylePrato, J. G., Millán, F. C., González, L. C., Ríos, A. C., López, E., Ríos, I., Navas, S., Márquez, A., Carrero, J. C., & Díaz, J. I. (2022). Adsorption of Phosphate and Nitrate Ions on Oxidic Substrates Prepared with a Variable-Charge Lithological Material. Water, 14(16), 2454. https://doi.org/10.3390/w14162454







