Dynamic Changes in Groundwater Level under Climate Changes in the Gnangara Region, Western Australia
Abstract
:1. Introduction
2. Materials and Methods
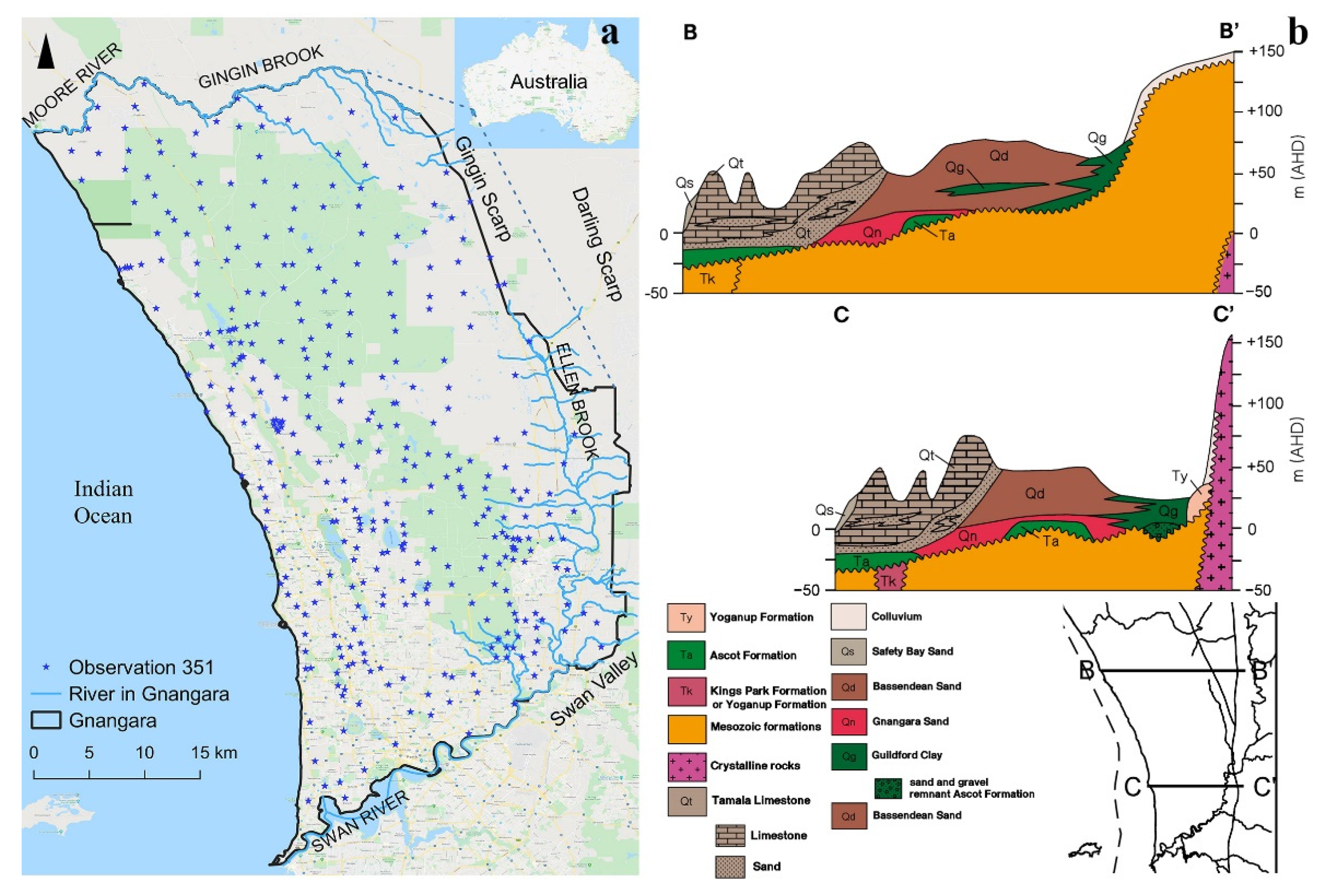
2.1. Site Location
2.2. Data Sources
2.3. Methods
2.3.1. Non-Parameter Kendall Test
2.3.2. Sen’s Slope Estimator
2.3.3. Innovative Trend Analysis
2.3.4. HARTT Model
3. Results and Discussion
3.1. Intra-Annual Variation in Groundwater Level
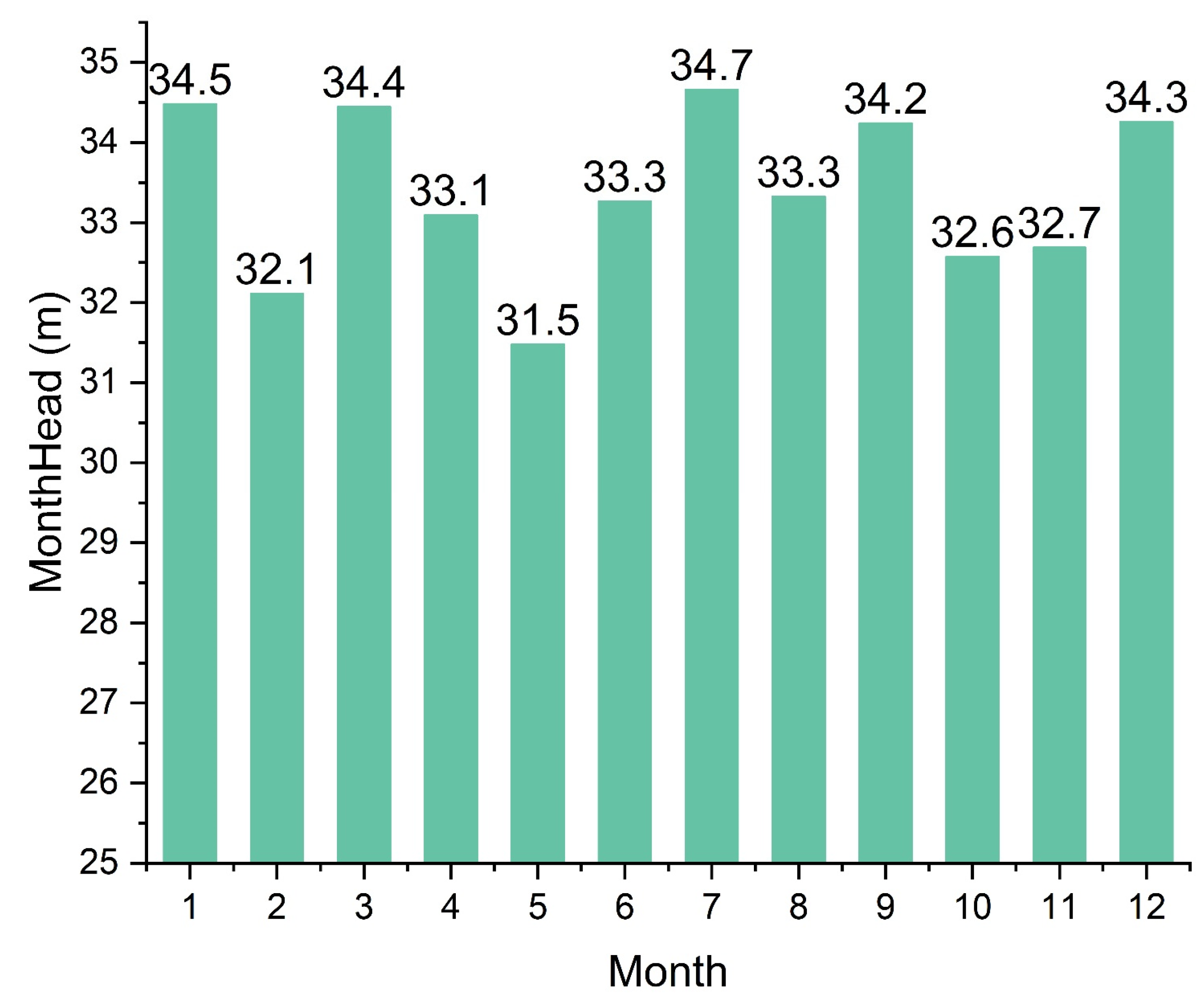
3.1.1. Variation in Average Monthly Groundwater Level
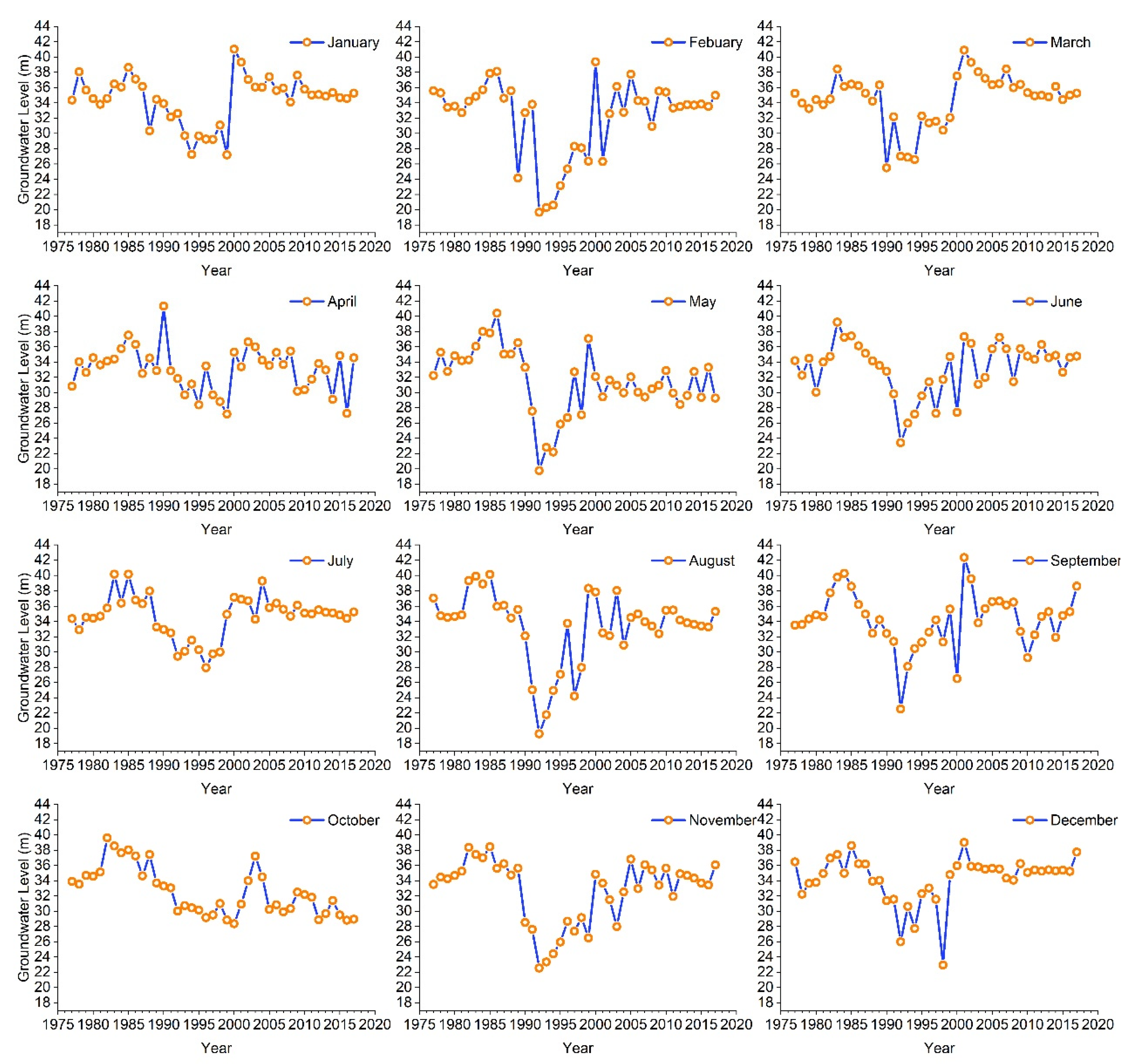
3.1.2. Long-Term Variation in Monthly Groundwater Level
3.2. Inter-Annual Variation in Groundwater Level
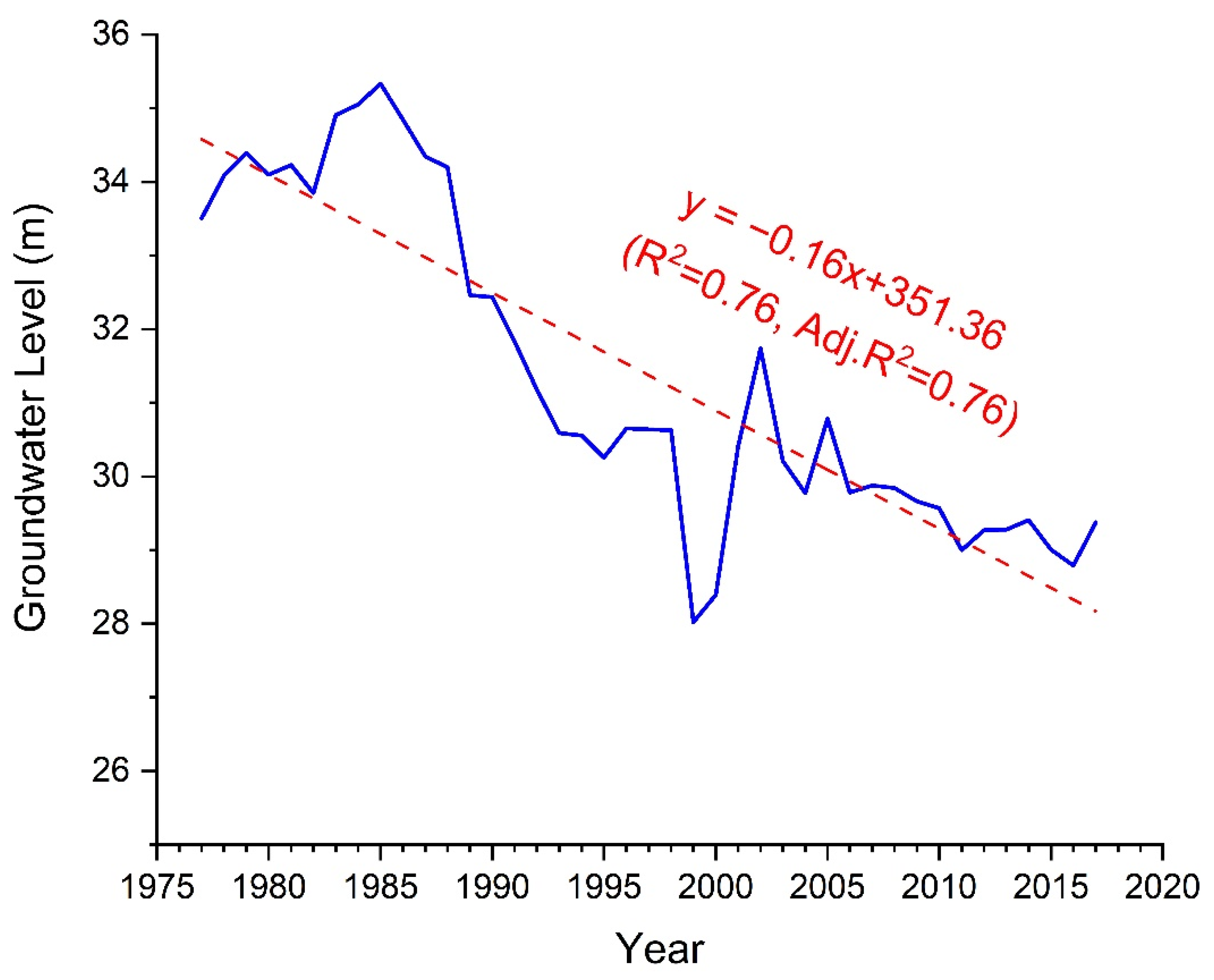
3.2.1. Long-Term Variation in Annual Mean Groundwater Level
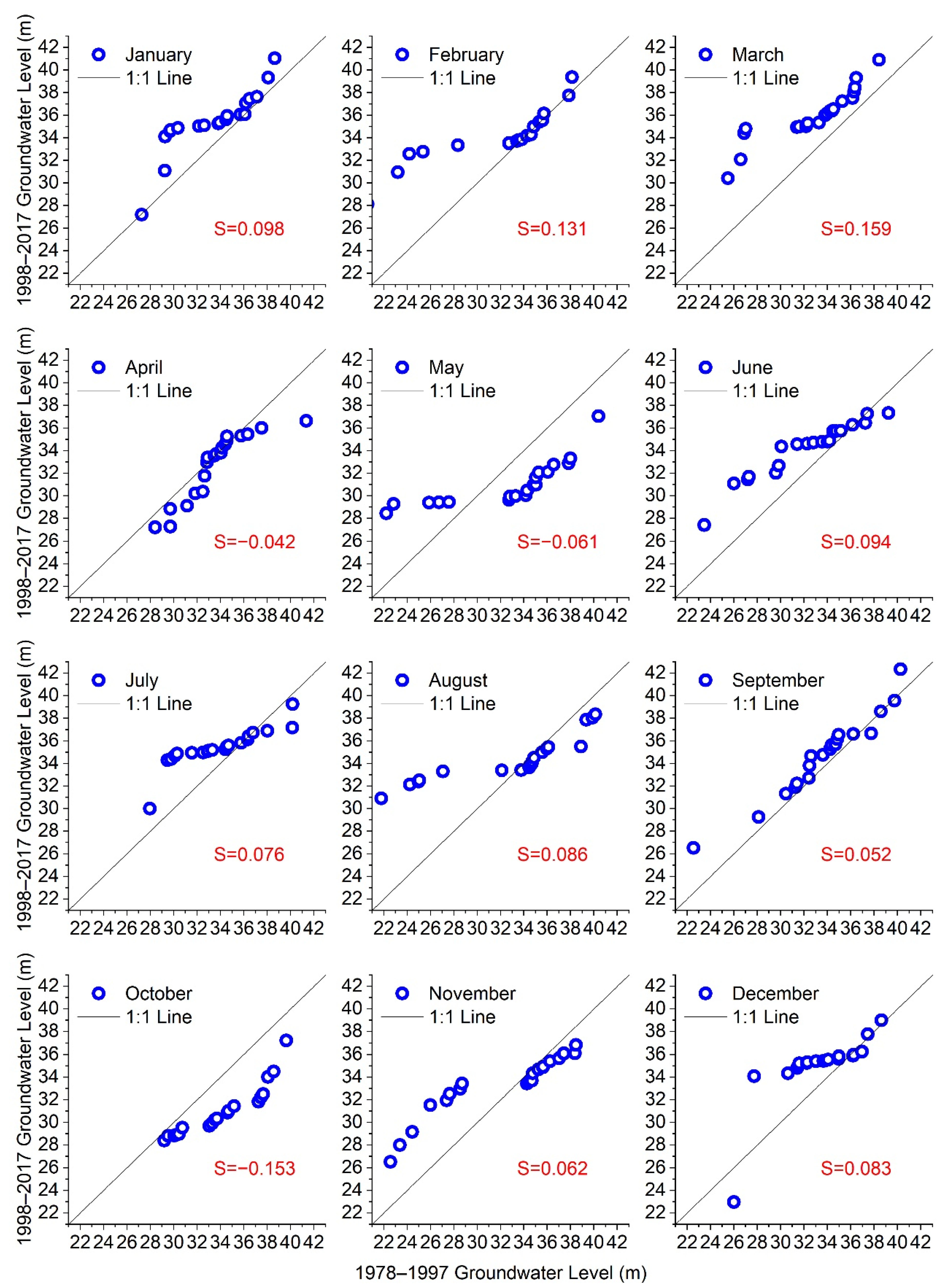
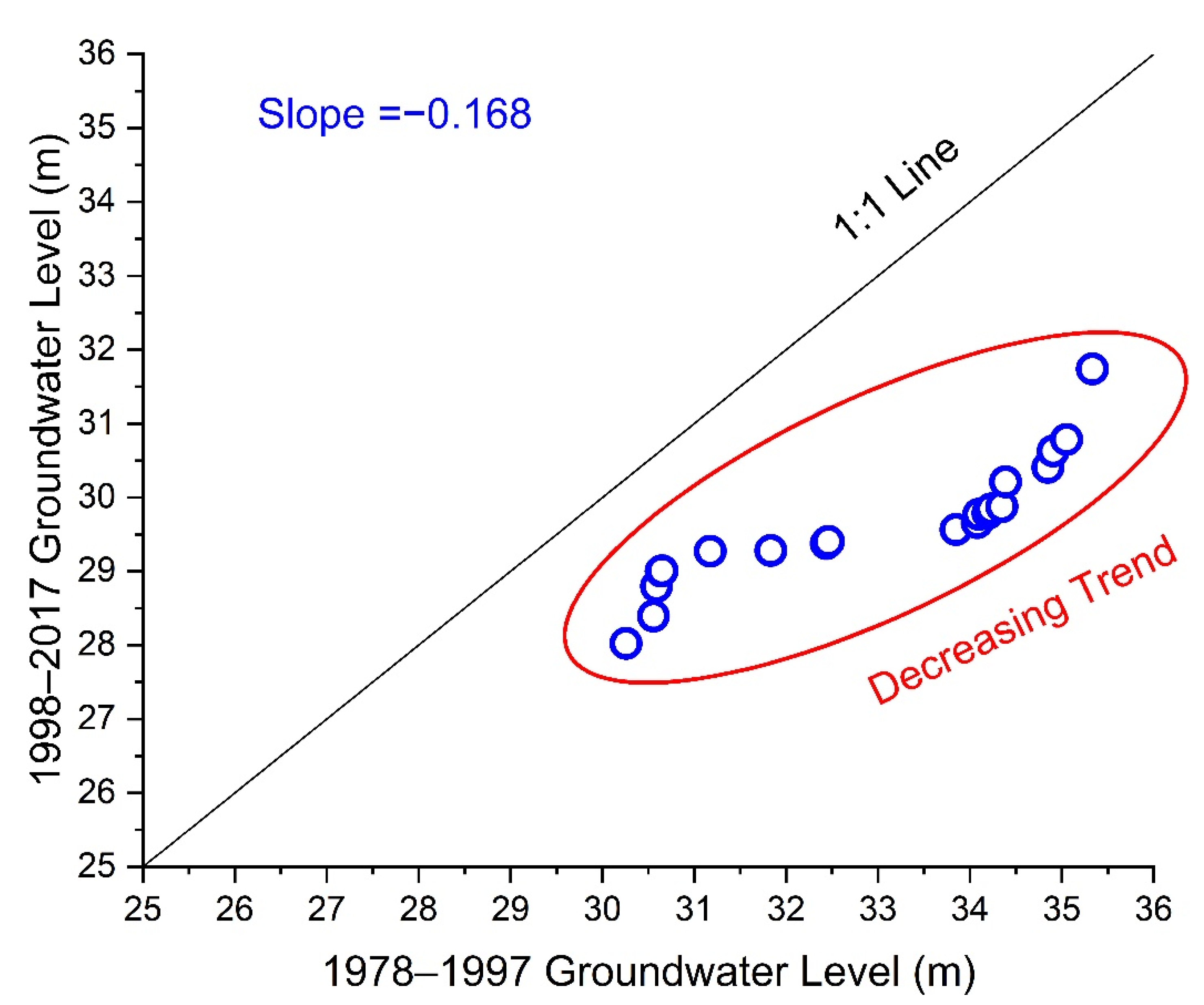
3.2.2. Variation in Annual Mean Groundwater Level in Different Periods
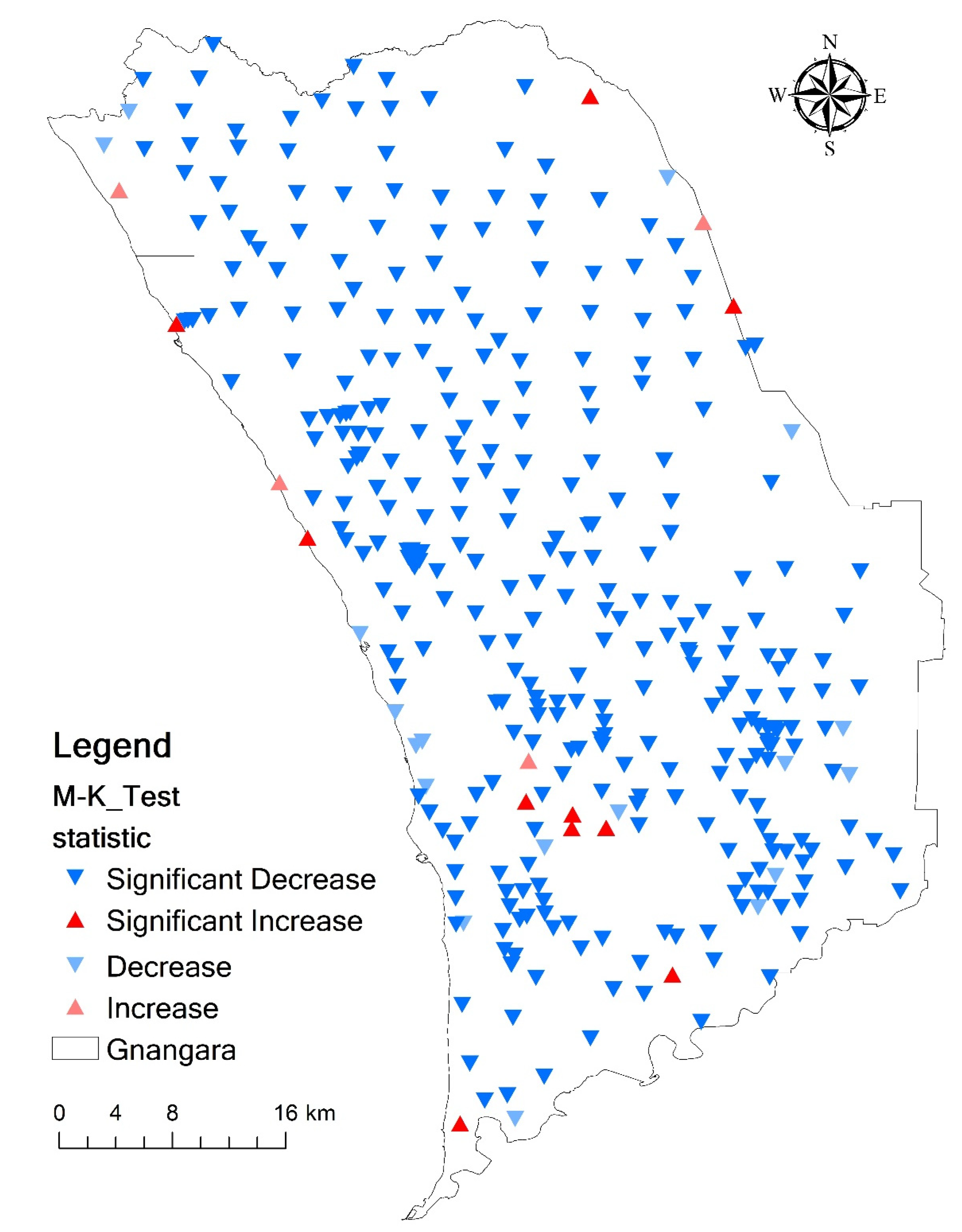
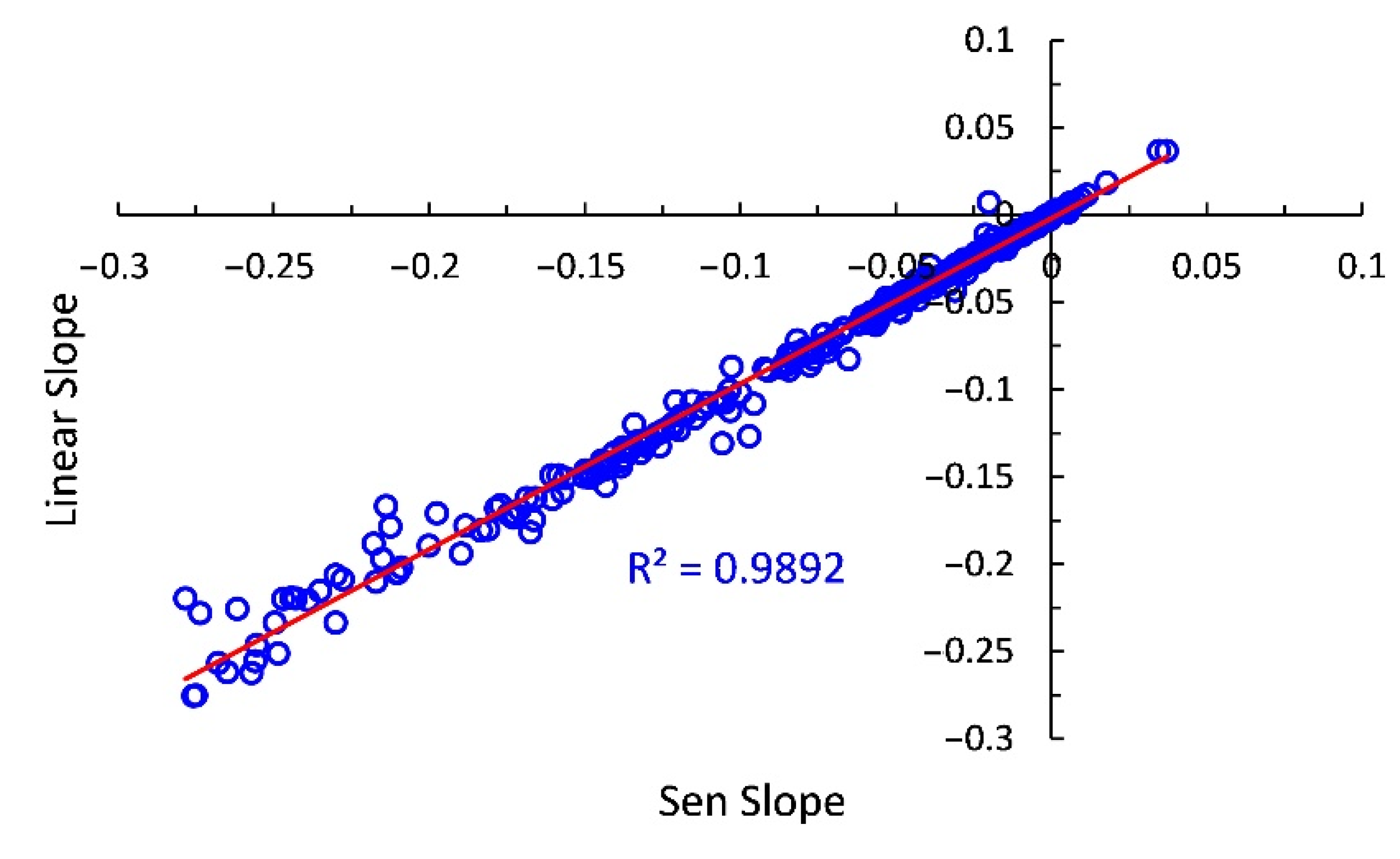
3.3. Spatial Variation in Groundwater Level
3.4. Relationship between Rainfall and Groundwater Level
3.5. Rainfall Impacts on the Groundwater Level Based on the HARRT Model
3.5.1. Rainfall Patterns
3.5.2. Rainfall Impacts
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
| Bore ID | Start Date of Record | Longitude | Latitude | Date of Well Construction | Well Depth (m) |
|---|---|---|---|---|---|
| bore61610005 | 1978-06 | 115.761 | −31.993 | 1978-06 | 23 |
| bore61610006 | 1978-06 | 115.763 | −31.917 | 1978-06 | 21 |
| bore61610007 | 1953-05 | 115.759 | −31.866 | 1953-05 | 0 |
| bore61610012 | 1967-10 | 115.759 | −31.850 | 1967-10 | 0 |
| bore61610013 | 1974-09 | 115.758 | −31.833 | 1974-09 | 51 |
| bore61610015 | 1946-10 | 115.777 | −31.979 | 0 | |
| bore61610016 | 1978-06 | 115.768 | −31.955 | 1978-06 | 26 |
| bore61610020 | 1970-07 | 115.797 | −31.990 | 1970-07 | 3.9 |
| bore61610021 | 1978-06 | 115.792 | −31.975 | 1978-06 | 30 |
| bore61610029 | 1978-06 | 115.795 | −31.926 | 1978-06 | 17 |
| bore61610031 | 1974-07 | 115.794 | −31.892 | 1974-07 | 18.3 |
| bore61610032 | 1972-03 | 115.796 | −31.886 | 1972-03 | 0 |
| bore61610033 | 1974-07 | 115.790 | −31.883 | 1974-07 | 23.9 |
| bore61610036 | 1974-07 | 115.789 | −31.871 | 1974-07 | 21.4 |
| bore61610037 | 1974-07 | 115.799 | −31.864 | 1974-07 | 17.1 |
| bore61610038 | 1974-07 | 115.793 | −31.855 | 1974-07 | 20.9 |
| bore61610039 | 1974-07 | 115.791 | −31.846 | 1974-07 | 16.2 |
| bore61610040 | 1967-09 | 115.786 | −31.834 | 1967-09 | 0 |
| bore61610045 | 1978-06 | 115.815 | −31.963 | 1978-06 | 21 |
| bore61610050 | 1974-07 | 115.810 | −31.900 | 1974-07 | 21.8 |
| bore61610100 | 1974-07 | 115.815 | −31.859 | 1974-07 | 16 |
| bore61610106 | 1974-07 | 115.802 | −31.845 | 1974-07 | 24.7 |
| bore61610107 | 1974-07 | 115.815 | −31.851 | 1974-07 | 28.4 |
| bore61610110 | 1974-07 | 115.811 | −31.842 | 1972-04 | 35.6 |
| bore61610111 | 1971-06 | 115.805 | −31.829 | 1972-06 | 0 |
| bore61610112 | 1965-09 | 115.815 | −31.818 | 0 | |
| bore61610142 | 1974-07 | 115.821 | −31.869 | 1974-07 | 19.8 |
| bore61610143 | 1974-07 | 115.830 | −31.866 | 1974-07 | 24.5 |
| bore61610161 | 1978-06 | 115.844 | −31.939 | 1978-06 | 15.8 |
| bore61610163 | 1974-07 | 115.838 | −31.882 | 1974-07 | 37.1 |
| bore61610171 | 1978-06 | 115.859 | −31.908 | 1978-05 | 15 |
| bore61610172 | 1974-01 | 115.852 | −31.875 | 1974-01 | 62.2 |
| bore61610200 | 1946-08 | 115.879 | −31.911 | 1946-08 | 7 |
| bore61610204 | 1947-11 | 115.876 | −31.891 | 1947-11 | 8 |
| bore61610277 | 1969-04 | 115.897 | −31.898 | 1969-04 | 0 |
| bore61610283 | 1981-12 | 115.899 | −31.874 | 0 | |
| bore61610284 | 1952-10 | 115.892 | −31.872 | 0 | |
| bore61610381 | 1922-03 | 115.915 | −31.928 | 1922-03 | 0 |
| bore61610474 | 1947-08 | 115.923 | −31.889 | 0 | |
| bore61610475 | 1974-01 | 115.919 | −31.872 | 1974-01 | 12.8 |
| bore61610493 | 1977-07 | 115.932 | −31.820 | 1977-07 | 18 |
| bore61610510 | 1974-01 | 115.941 | −31.856 | 1974-01 | 13.2 |
| bore61610511 | 1977-01 | 115.951 | −31.856 | 1900-01 | 4 |
| bore61610513 | 1976-02 | 115.937 | −31.846 | 1975-06 | 48 |
| bore61610517 | 1976-06 | 115.951 | −31.846 | 1976-06 | 5 |
| bore61610522 | 1976-05 | 115.943 | −31.838 | 1976-05 | 6 |
| bore61610525 | 1977-03 | 115.952 | −31.832 | 1977-06 | 15 |
| bore61610543 | 1978-06 | 115.958 | −31.900 | 1978-05 | 17.5 |
| bore61610546 | 1977-01 | 115.966 | −31.856 | 1977-01 | 5 |
| bore61610550 | 1976-05 | 115.957 | −31.846 | 1976-05 | 6 |
| bore61610559 | 1977-03 | 115.961 | −31.820 | 1977-03 | 17 |
| bore61610561 | 1977-08 | 115.969 | −31.820 | 1977-06 | 15 |
| bore61610563 | 1974-06 | 115.959 | −31.813 | 1974-06 | 14 |
| bore61610570 | 1977-01 | 115.978 | −31.851 | 1977-01 | 6 |
| bore61610572 | 1977-10 | 115.981 | −31.840 | 1977-10 | 12 |
| bore61610575 | 1977-02 | 115.979 | −31.827 | 1977-06 | 14 |
| bore61610576 | 1977-01 | 115.985 | −31.819 | 1977-06 | 17 |
| bore61610577 | 1976-04 | 115.979 | −31.813 | 1976-06 | 25 |
| bore61610582 | 1975-08 | 115.669 | −31.558 | 1975-08 | 16.8 |
| bore61610584 | 1974-12 | 115.709 | −31.624 | 1974-06 | 56 |
| bore61610585 | 1974-12 | 115.716 | −31.602 | 1974-06 | 58 |
| bore61610586 | 1974-12 | 115.718 | −31.572 | 1974-06 | 52 |
| bore61610587 | 1976-10 | 115.707 | −31.556 | 1976-10 | 37.8 |
| bore61610591 | 1973-06 | 115.720 | −31.732 | 1973-06 | 43 |
| bore61610594 | 1976-10 | 115.731 | −31.587 | 1976-10 | 46 |
| bore61610595 | 1977-01 | 115.735 | −31.553 | 1977-01 | 73 |
| bore61610596 | 1974-08 | 115.740 | −31.778 | 1974-08 | 39 |
| bore61610599 | 1974-12 | 115.738 | −31.692 | 1974-06 | 59 |
| bore61610600 | 1976-12 | 115.752 | −31.660 | 1976-12 | 32.1 |
| bore61610601 | 1976-11 | 115.747 | −31.642 | 1976-11 | 43.4 |
| bore61610603 | 1976-10 | 115.739 | −31.608 | 1976-10 | 45.7 |
| bore61610608 | 1978-10 | 115.751 | −31.517 | 1978-05 | 62 |
| bore61610613 | 1976-11 | 115.762 | −31.626 | 1976-11 | 45.9 |
| bore61610615 | 1975-06 | 115.761 | −31.605 | 1975-05 | 75 |
| bore61610617 | 1975-06 | 115.762 | −31.587 | 1975-05 | 82 |
| bore61610619 | 1975-06 | 115.760 | −31.570 | 1975-06 | 83 |
| bore61610620 | 1975-07 | 115.757 | −31.561 | 1975-06 | 84 |
| bore61610622 | 1976-10 | 115.764 | −31.551 | 1976-10 | 41.3 |
| bore61610624 | 1978-10 | 115.755 | −31.534 | 1978-05 | 72 |
| bore61610627 | 1972-03 | 115.782 | −31.777 | 1972-06 | 0 |
| bore61610628 | 1981-09 | 115.784 | −31.726 | 1981-09 | 0 |
| bore61610632 | 1974-08 | 115.779 | −31.688 | 1974-08 | 70 |
| bore61610633 | 1976-12 | 115.771 | −31.669 | 1976-12 | 44.9 |
| bore61610642 | 1981-02 | 115.771 | −31.636 | 1973-06 | 68 |
| bore61610644 | 1976-10 | 115.778 | −31.578 | 1976-10 | 31.5 |
| bore61610645 | 1976-10 | 115.781 | −31.566 | 1976-10 | 33 |
| bore61610649 | 1982-05 | 115.781 | −31.538 | 1982-05 | 48 |
| bore61610652 | 1982-05 | 115.781 | −31.538 | 1982-05 | 48 |
| bore61610654 | 1977-07 | 115.777 | −31.505 | 1977-06 | 31 |
| bore61610661 | 1970-06 | 115.796 | −31.745 | 1970-06 | 0 |
| bore61610662 | 1975-02 | 115.788 | −31.725 | 1975-02 | 20.7 |
| bore61610664 | 1975-03 | 115.797 | −31.705 | 1975-03 | 40 |
| bore61610665 | 1975-02 | 115.795 | −31.687 | 1975-02 | 39.6 |
| bore61610667 | 1975-04 | 115.793 | −31.653 | 1975-04 | 74 |
| bore61610670 | 1986-04 | 115.792 | −31.610 | 0 | |
| bore61610671 | 1976-10 | 115.794 | −31.594 | 1976-10 | 25.9 |
| bore61610672 | 1976-10 | 115.802 | −31.573 | 1976-10 | 30.8 |
| bore61610673 | 1976-11 | 115.801 | −31.547 | 1976-11 | 36.1 |
| bore61610674 | 1978-11 | 115.802 | −31.526 | 1978-08 | 68 |
| bore61610676 | 1978-11 | 115.799 | −31.508 | 1978-10 | 66 |
| bore61610677 | 1972-10 | 115.809 | −31.806 | 1972-06 | 0 |
| bore61610678 | 1972-03 | 115.814 | −31.784 | 1900-01 | 68.6 |
| bore61610679 | 1978-11 | 115.803 | −31.788 | 1978-02 | 13.5 |
| bore61610683 | 1975-02 | 115.808 | −31.751 | 1975-02 | 41.4 |
| bore61610684 | 1975-02 | 115.811 | −31.734 | 1900-01 | 27.4 |
| bore61610685 | 1979-01 | 115.811 | −31.728 | 1979-01 | 9 |
| bore61610688 | 1978-12 | 115.810 | −31.722 | 1978-12 | 9 |
| bore61610690 | 1972-06 | 115.806 | −31.714 | 1972-06 | 58.5 |
| bore61610696 | 1965-08 | 115.808 | −31.673 | 1965-08 | 48.5 |
| bore61610697 | 1977-02 | 115.810 | −31.649 | 1977-02 | 14 |
| bore61610698 | 1976-12 | 115.819 | −31.628 | 1976-12 | 13.2 |
| bore61610712 | 1973-09 | 115.808 | −31.479 | 1973-06 | 62.4 |
| bore61610714 | 1973-09 | 115.832 | −31.805 | 1973-09 | 31.7 |
| bore61610715 | 1963-08 | 115.833 | −31.796 | 1963-08 | 72.3 |
| bore61610716 | 1975-02 | 115.827 | −31.771 | 1975-02 | 53.6 |
| bore61610720 | 1981-03 | 115.832 | −31.756 | 1973-06 | 93.2 |
| bore61610734 | 1979-05 | 115.823 | −31.733 | 1979-12 | 16 |
| bore61610736 | 1978-12 | 115.823 | −31.725 | 1978-12 | 9 |
| bore61610745 | 1979-05 | 115.836 | −31.725 | 1979-02 | 13 |
| bore61610750 | 1976-10 | 115.829 | −31.658 | 1976-10 | 13.5 |
| bore61610753 | 1965-10 | 115.830 | −31.635 | 1965-10 | 38.1 |
| bore61610756 | 1976-12 | 115.832 | −31.587 | 1976-12 | 20.8 |
| bore61610758 | 1975-08 | 115.852 | −31.782 | 1975-08 | 32.9 |
| bore61610761 | 1977-09 | 115.837 | −31.755 | 1977-09 | 30 |
| bore61610762 | 1977-09 | 115.852 | −31.752 | 1977-09 | 10.7 |
| bore61610764 | 1977-04 | 115.851 | −31.749 | 1977-03 | 27 |
| bore61610769 | 1977-04 | 115.852 | −31.729 | 1977-03 | 30 |
| bore61610789 | 1965-06 | 115.836 | −31.708 | 1965-05 | 40.5 |
| bore61610794 | 1975-02 | 115.853 | −31.686 | 1975-02 | 16 |
| bore61610803 | 1976-11 | 115.846 | −31.634 | 1976-11 | 13.4 |
| bore61610804 | 1976-11 | 115.843 | −31.612 | 1976-11 | 17.1 |
| bore61610805 | 1976-11 | 115.845 | −31.573 | 1976-11 | 19.5 |
| bore61610807 | 1971-03 | 115.845 | −31.543 | 1971-03 | 81.7 |
| bore61610808 | 1976-11 | 115.843 | −31.529 | 1976-11 | 29.1 |
| bore61610809 | 1977-07 | 115.840 | −31.507 | 1977-07 | 26 |
| bore61610810 | 1977-07 | 115.844 | −31.478 | 1977-06 | 32 |
| bore61610811 | 1974-06 | 115.854 | −31.805 | 1974-06 | 22.4 |
| bore61610815 | 1981-12 | 115.862 | −31.795 | 1900-01 | 21.1 |
| bore61610816 | 1963-09 | 115.866 | −31.765 | 1963-09 | 48.8 |
| bore61610817 | 1975-02 | 115.853 | −31.746 | 1975-02 | 17.7 |
| bore61610822 | 1977-04 | 115.853 | −31.738 | 1977-04 | 20 |
| bore61610832 | 1975-02 | 115.863 | −31.672 | 1975-02 | 13.1 |
| bore61610833 | 1975-02 | 115.855 | −31.655 | 1975-02 | 14.4 |
| bore61610834 | 1976-12 | 115.861 | −31.597 | 1976-12 | 19.1 |
| bore61610835 | 1973-09 | 115.875 | −31.804 | 1973-09 | 16.5 |
| bore61610843 | 1973-09 | 115.876 | −31.785 | 1973-09 | 25.6 |
| bore61610845 | 1975-02 | 115.881 | −31.752 | 1975-02 | 8.2 |
| bore61610855 | 1981-10 | 115.878 | −31.716 | 1981-05 | 19.8 |
| bore61610860 | 1975-02 | 115.879 | −31.692 | 1975-02 | 13.5 |
| bore61610861 | 1965-09 | 115.876 | −31.661 | 1965-09 | 32 |
| bore61610864 | 1976-11 | 115.881 | −31.631 | 1976-11 | 17.2 |
| bore61610869 | 1976-11 | 115.877 | −31.522 | 1976-11 | 23.1 |
| bore61610870 | 1977-08 | 115.877 | −31.510 | 1977-08 | 24.5 |
| bore61610871 | 1973-09 | 115.878 | −31.483 | 1973-07 | 57 |
| bore61610883 | 1973-09 | 115.898 | −31.786 | 1973-09 | 15.8 |
| bore61610884 | 1963-07 | 115.895 | −31.768 | 1963-07 | 33.5 |
| bore61610907 | 1983-05 | 115.894 | −31.683 | 1983-05 | 11 |
| bore61610908 | 1975-02 | 115.895 | −31.662 | 1975-02 | 14.9 |
| bore61610913 | 1983-03 | 115.895 | −31.618 | 1983-03 | 13.5 |
| bore61610914 | 1977-01 | 115.896 | −31.598 | 1977-01 | 21.2 |
| bore61610915 | 1971-03 | 115.891 | −31.571 | 1971-03 | 78.9 |
| bore61610917 | 1977-07 | 115.905 | −31.477 | 1977-07 | 13.7 |
| bore61610918 | 1974-01 | 115.918 | −31.804 | 1974-01 | 13.4 |
| bore61610933 | 1963-10 | 115.927 | −31.771 | 1963-10 | 39.6 |
| bore61610936 | 1983-05 | 115.910 | −31.701 | 1983-05 | 17.5 |
| bore61610941 | 1983-06 | 115.907 | −31.694 | 1983-06 | 19 |
| bore61610943 | 1975-02 | 115.907 | −31.692 | 1975-02 | 16.1 |
| bore61610946 | 1982-11 | 115.905 | −31.676 | 1982-11 | 11 |
| bore61610949 | 1983-05 | 115.917 | −31.667 | 1983-05 | 8.8 |
| bore61610951 | 1973-06 | 115.916 | −31.539 | 1973-06 | 23 |
| bore61610952 | 1977-08 | 115.910 | −31.507 | 1977-08 | 17 |
| bore61610953 | 1977-06 | 115.909 | −31.455 | 1977-06 | 14 |
| bore61610967 | 1984-01 | 115.930 | −31.759 | 1900-01 | 10 |
| bore61610978 | 1975-03 | 115.922 | −31.728 | 1975-03 | 16.7 |
| bore61610980 | 1984-01 | 115.929 | −31.720 | 1900-01 | 10 |
| bore61610982 | 1982-11 | 115.934 | −31.714 | 1982-11 | 8.3 |
| bore61610983 | 1975-02 | 115.930 | −31.694 | 1975-02 | 16.5 |
| bore61610984 | 1983-05 | 115.933 | −31.682 | 1983-05 | 11 |
| bore61610985 | 1977-07 | 115.935 | −31.473 | 1977-07 | 3 |
| bore61610989 | 1973-09 | 115.939 | −31.785 | 1973-09 | 15.8 |
| bore61611003 | 1981-04 | 115.950 | −31.791 | 1976-06 | 8 |
| bore61611010 | 1984-01 | 115.950 | −31.759 | 1900-01 | 10 |
| bore61611011 | 1984-01 | 115.940 | −31.740 | 1900-01 | 13 |
| bore61611014 | 1984-01 | 115.951 | −31.740 | 1900-01 | 23 |
| bore61611018 | 1984-01 | 115.948 | −31.722 | 1900-01 | 10 |
| bore61611019 | 1965-08 | 115.950 | −31.674 | 1900-01 | 31.1 |
| bore61611021 | 1983-03 | 115.941 | −31.647 | 1983-03 | 15 |
| bore61611022 | 1977-07 | 115.943 | −31.500 | 1977-07 | 13 |
| bore61611023 | 1978-06 | 115.949 | −31.499 | 1978-06 | 16 |
| bore61611025 | 1978-08 | 115.954 | −31.804 | 1978-08 | 16 |
| bore61611031 | 1973-10 | 115.957 | −31.762 | 1971-06 | 47 |
| bore61611033 | 1984-01 | 115.968 | −31.764 | 1900-01 | 14 |
| bore61611034 | 1971-06 | 115.957 | −31.752 | 1971-06 | 19.7 |
| bore61611035 | 1976-04 | 115.957 | −31.742 | 1971-06 | 157 |
| bore61611037 | 1984-01 | 115.961 | −31.742 | 1900-01 | 9 |
| bore61611041 | 1984-01 | 115.969 | −31.721 | 1900-01 | 10 |
| bore61611042 | 1983-05 | 115.957 | −31.696 | 1983-05 | 9 |
| bore61611043 | 1965-10 | 115.968 | −31.641 | 1965-09 | 25.1 |
| bore61611044 | 1970-02 | 115.959 | −31.586 | 1970-02 | 85.3 |
| bore61611047 | 1978-06 | 115.972 | −31.553 | 1978-06 | 15 |
| bore61611056 | 1984-01 | 115.972 | −31.742 | 1900-01 | 10 |
| bore61611064 | 1984-01 | 115.999 | −31.770 | 1900-01 | 12 |
| bore61611065 | 1984-01 | 115.994 | −31.742 | 1900-01 | 6 |
| bore61611068 | 1984-01 | 115.992 | −31.718 | 1900-01 | 8 |
| bore61611072 | 1978-06 | 116.006 | −31.670 | 1978-06 | 15 |
| bore61611074 | 1978-06 | 116.009 | −31.805 | 1978-05 | 17 |
| bore61611076 | 1984-01 | 116.009 | −31.772 | 1900-01 | 12 |
| bore61611079 | 1984-01 | 116.005 | −31.742 | 1900-01 | 8 |
| bore61611082 | 1984-01 | 116.015 | −31.716 | 1900-01 | 8 |
| bore61611083 | 1978-06 | 116.016 | −31.642 | 1978-06 | 18 |
| bore61611087 | 1977-07 | 115.872 | −31.448 | 1977-06 | 16 |
| bore61611088 | 1977-12 | 115.898 | −31.435 | 1977-12 | 13.6 |
| bore61611089 | 1977-07 | 115.916 | −31.420 | 1977-07 | 13 |
| bore61611092 | 1974-12 | 115.650 | −31.584 | 1974-06 | 40.9 |
| bore61611151 | 1989-01 | 115.822 | −31.621 | 0 | |
| bore61611175 | 1984-12 | 115.970 | −31.696 | 1900-01 | 8 |
| bore61611183 | 1984-01 | 115.992 | −31.699 | 1900-01 | 7 |
| bore61611211 | 1985-11 | 115.804 | −31.861 | 1985-01 | 18 |
| bore61611220 | 1989-12 | 115.734 | −31.631 | 1989-03 | 4.3 |
| bore61611223 | 1989-12 | 115.731 | −31.629 | 1989-03 | 13.4 |
| bore61611225 | 1989-11 | 115.728 | −31.629 | 1989-03 | 7.65 |
| bore61611228 | 1990-01 | 115.736 | −31.635 | 1989-03 | 8.4 |
| bore61611233 | 1989-12 | 115.733 | −31.639 | 1989-03 | 8.4 |
| bore61611234 | 1989-11 | 115.731 | −31.637 | 1989-03 | 13.4 |
| bore61611240 | 1989-12 | 115.737 | −31.630 | 1989-03 | 10.4 |
| bore61611244 | 1989-12 | 115.735 | −31.631 | 1989-03 | 26 |
| bore61611247 | 1990-01 | 115.729 | −31.633 | 1989-03 | 7.2 |
| bore61611270 | 2001-10 | 115.902 | −31.914 | 2001-07 | 7 |
| bore61611289 | 2001-12 | 115.869 | −31.711 | 2001-09 | 11.8 |
| bore61611303 | 1990-02 | 115.708 | −31.588 | 1990-01 | 6.6 |
| bore61611377 | 2012-05 | 115.863 | −31.790 | 2011-12 | 7 |
| bore61611652 | 1989-08 | 115.716 | −31.693 | 1989-08 | 61.5 |
| bore61611656 | 1989-08 | 115.720 | −31.702 | 1989-08 | 71 |
| bore61611663 | 1989-11 | 115.722 | −31.715 | 1989-08 | 59.5 |
| bore61611664 | 1989-08 | 115.725 | −31.669 | 1989-08 | 48 |
| bore61611666 | 1989-08 | 115.697 | −31.677 | 1989-08 | 45 |
| bore61611667 | 1989-06 | 115.686 | −31.615 | 1989-06 | 62.4 |
| bore61611668 | 1989-06 | 115.698 | −31.615 | 1989-06 | 68 |
| bore61611669 | 1989-06 | 115.664 | −31.621 | 1989-06 | 20 |
| bore61611670 | 1989-06 | 115.657 | −31.605 | 1989-06 | 26.5 |
| bore61611673 | 1989-07 | 115.688 | −31.599 | 1989-07 | 68.5 |
| bore61611674 | 1989-07 | 115.700 | −31.631 | 1989-07 | 63.5 |
| bore61611676 | 1989-08 | 115.675 | −31.634 | 1989-08 | 26 |
| bore61611677 | 1989-08 | 115.683 | −31.631 | 1989-08 | 51 |
| bore61611678 | 1989-08 | 115.713 | −31.654 | 1989-08 | 57 |
| bore61611680 | 1989-08 | 115.706 | −31.645 | 1989-08 | 44.5 |
| bore61611681 | 1989-09 | 115.683 | −31.647 | 1989-09 | 29.5 |
| bore61611682 | 1989-09 | 115.689 | −31.622 | 1989-09 | 73.5 |
| bore61611685 | 1989-09 | 115.668 | −31.596 | 1989-09 | 55 |
| bore61611702 | 1990-07 | 115.698 | −31.682 | 1990-06 | 8.85 |
| bore61611833 | 1996-06 | 115.760 | −32.003 | 1996-06 | 26 |
| bore61611941 | 1993-05 | 115.846 | −31.612 | 1993-05 | 8.72 |
| bore61611960 | 2010-09 | 115.807 | −31.945 | 2008-01 | 15 |
| bore61611974 | 2010-09 | 115.770 | −31.976 | 2008-03 | 28.6 |
| bore61611975 | 2010-09 | 115.768 | −31.906 | 2008-03 | 15 |
| bore61611976 | 2010-09 | 115.744 | −31.818 | 2008-03 | 17.6 |
| bore61611977 | 2010-09 | 115.759 | −31.886 | 2008-03 | 30.7 |
| bore61612100 | 1991-05 | 115.712 | −31.537 | 1991-05 | 61.3 |
| bore61612101 | 1991-05 | 115.703 | −31.539 | 1991-05 | 45 |
| bore61612102 | 1991-06 | 115.692 | −31.541 | 1991-05 | 33.1 |
| bore61612103 | 1991-05 | 115.689 | −31.542 | 1991-05 | 10.7 |
| bore61612104 | 1991-05 | 115.685 | −31.543 | 1991-05 | 8.96 |
| bore61612105 | 1991-05 | 115.697 | −31.555 | 1991-05 | 21.5 |
| bore61612106 | 1991-05 | 115.687 | −31.555 | 1991-05 | 15.2 |
| bore61612107 | 1991-05 | 115.696 | −31.570 | 1991-05 | 17.9 |
| bore61613200 | 1995-09 | 115.944 | −31.766 | 1995-08 | 9.55 |
| bore61613201 | 1995-11 | 115.957 | −31.750 | 1995-08 | 10 |
| bore61613202 | 1995-09 | 115.947 | −31.736 | 1995-08 | 9.97 |
| bore61613203 | 1995-09 | 115.827 | −31.687 | 1995-08 | 8.41 |
| bore61613204 | 1995-11 | 115.840 | −31.653 | 1995-08 | 12 |
| bore61613205 | 1995-10 | 115.819 | −31.611 | 1995-08 | 11.7 |
| bore61613206 | 1995-10 | 115.815 | −31.604 | 1995-08 | 13.1 |
| bore61613208 | 1995-09 | 115.699 | −31.568 | 1995-08 | 14.7 |
| bore61613209 | 1995-10 | 115.697 | −31.568 | 1995-08 | 14.1 |
| bore61613210 | 1995-10 | 115.718 | −31.508 | 1995-08 | 11.7 |
| bore61613211 | 1995-09 | 115.854 | −31.667 | 1995-08 | 12.8 |
| bore61613213 | 1995-09 | 115.964 | −31.704 | 1995-08 | 8.13 |
| bore61613214 | 1995-09 | 115.963 | −31.743 | 1995-09 | 2.91 |
| bore61613215 | 1995-09 | 115.959 | −31.752 | 1995-09 | 2.4 |
| bore61613217 | 1994-01 | 115.974 | −31.753 | 1995-10 | 0 |
| bore61613231 | 1999-02 | 115.915 | −31.705 | 1999-02 | 10.1 |
| bore61618432 | 1995-07 | 115.805 | −31.762 | 1995-04 | 19 |
| bore61618440 | 1996-06 | 115.874 | −31.790 | 1996-06 | 5.67 |
| bore61618441 | 1996-07 | 115.861 | −31.821 | 1996-06 | 25.4 |
| bore61618444 | 1997-07 | 115.962 | −31.836 | 1997-06 | 7.95 |
| bore61618499 | 1997-08 | 115.763 | −31.721 | 1996-11 | 70 |
| bore61618500 | 1997-03 | 115.690 | −31.575 | 1997-02 | 6.54 |
| bore61618513 | 1997-10 | 115.764 | −31.866 | 1997-09 | 13 |
| bore61618558 | 1998-10 | 115.975 | −31.662 | 1998-06 | 6.3 |
| bore61618559 | 1998-07 | 116.013 | −31.606 | 1998-06 | 18 |
| bore61618606 | 2000-03 | 115.995 | −31.795 | 0 | |
| bore61618607 | 2000-03 | 115.980 | −31.771 | 0 | |
| bore61619294 | 1988-09 | 116.041 | −31.845 | 1987-02 | 20.4 |
| bore61619296 | 1988-09 | 116.037 | −31.822 | 1987-02 | 12 |
| bore61619406 | 1990-08 | 115.591 | −31.482 | 1990-07 | 66 |
| bore61619455 | 1990-08 | 115.589 | −31.482 | 1979-05 | 61 |
| bore61619461 | 1985-07 | 115.581 | −31.484 | 1973-03 | 15 |
| bore61619464 | 1980-06 | 115.586 | −31.483 | 1980-06 | 55 |
| bore61619608 | 1985-07 | 116.007 | −31.830 | 1985-07 | 12 |
| bore61619611 | 1985-07 | 116.025 | −31.813 | 1985-07 | 17 |
| bore61619615 | 1985-07 | 115.978 | −31.873 | 1985-07 | 19 |
| bore61620100 | 1992-05 | 115.737 | −31.750 | 1992-05 | 53.7 |
| bore61620101 | 1992-05 | 115.735 | −31.785 | 1992-05 | 41.8 |
| bore61620102 | 1992-05 | 115.742 | −31.795 | 1992-05 | 48.3 |
| bore61620103 | 1992-05 | 115.750 | −31.807 | 1992-05 | 36.2 |
| bore61620104 | 1992-05 | 115.758 | −31.815 | 1992-05 | 59.7 |
| bore61620110 | 1992-05 | 115.772 | −31.784 | 1992-05 | 50.8 |
| bore61620111 | 1992-05 | 115.768 | −31.803 | 1992-05 | 58.6 |
| bore61620112 | 1992-05 | 115.733 | −31.753 | 1992-05 | 75.4 |
| bore61625172 | 1995-06 | 115.723 | −31.708 | 1994-08 | 42.1 |
| bore61710001 | 1978-06 | 115.535 | −31.372 | 1978-06 | 43.9 |
| bore61710002 | 1978-06 | 115.545 | −31.399 | 1978-06 | 46 |
| bore61710003 | 1974-12 | 115.551 | −31.350 | 1974-12 | 38.4 |
| bore61710006 | 1977-07 | 115.561 | −31.374 | 1977-07 | 37.6 |
| bore61710007 | 1978-05 | 115.560 | −31.329 | 1978-05 | 36 |
| bore61710008 | 1977-07 | 115.586 | −31.389 | 1977-05 | 55 |
| bore61710010 | 1978-05 | 115.586 | −31.349 | 1978-05 | 46 |
| bore61710011 | 1977-07 | 115.595 | −31.420 | 1977-04 | 63 |
| bore61710013 | 1977-08 | 115.596 | −31.329 | 1977-08 | 64.5 |
| bore61710014 | 1973-06 | 115.605 | −31.307 | 1973-06 | 38.3 |
| bore61710017 | 1978-07 | 115.620 | −31.373 | 1978-07 | 42 |
| bore61710018 | 1973-06 | 115.620 | −31.362 | 1973-06 | 80.9 |
| bore61710022 | 1977-07 | 115.653 | −31.375 | 1977-06 | 35 |
| bore61710023 | 1977-07 | 115.654 | −31.354 | 1977-04 | 15 |
| bore61710025 | 1974-04 | 115.665 | −31.545 | 1974-04 | 0 |
| bore61710026 | 1974-04 | 115.655 | −31.509 | 1974-04 | 0 |
| bore61710027 | 1977-07 | 115.655 | −31.479 | 1977-05 | 45 |
| bore61710028 | 1974-04 | 115.677 | −31.544 | 0 | |
| bore61710031 | 1974-01 | 115.684 | −31.476 | 1973-06 | 66.2 |
| bore61710033 | 1977-07 | 115.685 | −31.445 | 1977-06 | 41 |
| bore61710034 | 1977-06 | 115.704 | −31.506 | 1977-06 | 46 |
| bore61710036 | 1979-07 | 115.694 | −31.463 | 1978-10 | 69 |
| bore61710037 | 1977-06 | 115.714 | −31.480 | 1977-06 | 35 |
| bore61710040 | 1977-06 | 115.721 | −31.453 | 1977-06 | 44 |
| bore61710041 | 1978-10 | 115.738 | −31.502 | 1978-07 | 60.4 |
| bore61710047 | 1973-05 | 115.746 | −31.480 | 1973-06 | 91.4 |
| bore61710048 | 1977-08 | 115.739 | −31.480 | 1977-08 | 44.5 |
| bore61710052 | 1978-11 | 115.771 | −31.483 | 1978-10 | 56 |
| bore61710053 | 1977-07 | 115.763 | −31.466 | 1977-06 | 22 |
| bore61710055 | 1978-11 | 115.786 | −31.496 | 1978-09 | 64 |
| bore61710060 | 1977-07 | 115.812 | −31.450 | 1977-07 | 21 |
| bore61710061 | 1977-07 | 115.846 | −31.453 | 1977-06 | 20 |
| bore61710062 | 1977-06 | 115.659 | −31.426 | 1977-05 | 37 |
| bore61710063 | 1977-06 | 115.657 | −31.402 | 1977-05 | 30 |
| bore61710065 | 1977-06 | 115.687 | −31.402 | 1977-06 | 33 |
| bore61710066 | 1977-07 | 115.674 | −31.343 | 1977-04 | 17.5 |
| bore61710072 | 1981-09 | 115.690 | −31.376 | 1981-09 | 0 |
| bore61710073 | 1977-07 | 115.695 | −31.348 | 1977-04 | 17.6 |
| bore61710074 | 1977-07 | 115.694 | −31.321 | 1977-06 | 22 |
| bore61710075 | 1977-07 | 115.709 | −31.423 | 1977-06 | 31 |
| bore61710076 | 1977-07 | 115.720 | −31.400 | 1977-06 | 20 |
| bore61710077 | 1977-07 | 115.714 | −31.377 | 1977-05 | 18 |
| bore61710078 | 1977-05 | 115.717 | −31.348 | 1977-04 | 18.4 |
| bore61710079 | 1977-05 | 115.715 | −31.329 | 1977-04 | 23 |
| bore61710080 | 1977-07 | 115.745 | −31.446 | 1977-06 | 21 |
| bore61710082 | 1973-05 | 115.748 | −31.426 | 1973-06 | 79 |
| bore61710083 | 1977-07 | 115.749 | −31.404 | 1977-06 | 18 |
| bore61710086 | 1977-04 | 115.742 | −31.341 | 1977-04 | 18 |
| bore61710088 | 1977-07 | 115.776 | −31.425 | 1977-06 | 21 |
| bore61710089 | 1977-07 | 115.785 | −31.404 | 1977-06 | 17.5 |
| bore61710092 | 1977-07 | 115.790 | −31.374 | 1977-04 | 3.88 |
| bore61710093 | 1977-05 | 115.803 | −31.334 | 1977-04 | 4 |
| bore61710095 | 1973-09 | 115.809 | −31.424 | 1973-07 | 50 |
| bore61710097 | 1977-05 | 115.812 | −31.407 | 1977-04 | 18.5 |
| bore61710098 | 1977-05 | 115.816 | −31.385 | 1977-04 | 12 |
| bore61710103 | 1977-07 | 115.844 | −31.339 | 1977-06 | 15 |
| bore61710105 | 1977-07 | 115.850 | −31.406 | 1977-07 | 10 |
| bore61710107 | 1973-09 | 115.882 | −31.422 | 1973-08 | 39 |
| bore61710110 | 1977-07 | 115.893 | −31.392 | 1977-07 | 17 |
| bore61710116 | 1974-04 | 115.602 | −31.480 | 1974-04 | 67.1 |
| bore61710117 | 1978-12 | 115.616 | −31.522 | 1978-12 | 27.5 |
| bore61710118 | 1975-03 | 115.621 | −31.476 | 1975-03 | 30.5 |
| bore61710119 | 1977-08 | 115.617 | −31.450 | 1977-05 | 41 |
| bore61710123 | 1977-07 | 115.645 | −31.450 | 1977-06 | 21 |
| bore61710126 | 1992-07 | 115.633 | −31.437 | 1992-07 | 41.1 |
| bore61710127 | 1992-07 | 115.627 | −31.430 | 1992-07 | 65.6 |
| bore61710129 | 1992-07 | 115.614 | −31.414 | 1992-06 | 68.5 |
| bore61710131 | 1992-07 | 115.608 | −31.396 | 1992-06 | 71.2 |
| bore61710134 | 1992-07 | 115.590 | −31.371 | 1992-06 | 77.2 |
| bore61710136 | 2001-05 | 115.857 | −31.390 | 2001-03 | 8.5 |
| bore61710137 | 2001-05 | 115.870 | −31.409 | 2001-03 | 4.5 |
| bore61710508 | 2008-06 | 115.614 | −31.309 | 2008-06 | 8.73 |
| bore61710510 | 2008-06 | 115.613 | −31.314 | 2008-06 | 20.9 |
| bore61710512 | 2008-07 | 115.650 | −31.331 | 2008-07 | 8.93 |
| bore61710514 | 2008-07 | 115.665 | −31.338 | 2008-07 | 12.7 |
| bore61710518 | 2008-07 | 115.762 | −31.300 | 2008-07 | 11.7 |
| bore61710520 | 2008-08 | 115.775 | −31.294 | 2008-08 | 8.95 |
| bore61710522 | 2008-08 | 115.807 | −31.295 | 2008-08 | 8.94 |
| bore61710526 | 2008-09 | 115.850 | −31.329 | 2008-08 | 9 |
| bore61710543 | 2008-09 | 115.746 | −31.334 | 2008-08 | 17 |
| bore61710546 | 2008-08 | 115.803 | −31.418 | 2008-07 | 50 |
| bore61710552 | 2008-08 | 115.772 | −31.395 | 2008-07 | 11 |
| bore61710561 | 2008-08 | 115.785 | −31.437 | 2008-08 | 47 |
| bore61710566 | 2008-09 | 115.832 | −31.408 | 2008-08 | 23 |
| bore61710569 | 2008-08 | 115.802 | −31.455 | 2008-07 | 56 |
| bore61710573 | 2008-04 | 115.701 | −31.322 | 2007-06 | 11.4 |
| bore61710582 | 2008-09 | 115.733 | −31.353 | 2008-08 | 20 |
| bore61710596 | 2009-11 | 115.824 | −31.396 | 2009-11 | 10 |
| bore61710702 | 2010-08 | 115.893 | −31.455 | 2010-04 | 12 |
References
- Döll, P. Vulnerability to the impact of climate change on renewable groundwater resources: A global-scale assessment. Environ. Res. Lett. 2009, 4, 035006. [Google Scholar] [CrossRef]
- Famiglietti, J.S. The global groundwater crisis. Nat. Clim. Chang. 2014, 4, 945–948. [Google Scholar] [CrossRef]
- Guo, C.; Liu, T.; Niu, Y.; Liu, Z.; Pan, X.; De Maeyer, P. Quantitative analysis of the driving factors for groundwater resource changes in arid irrigated areas. Hydrol. Processes 2020, 35, e13967. [Google Scholar] [CrossRef]
- Feng, W.; Zhong, M.; Lemoine, J.-M.; Biancale, R.; Hsu, H.-T.; Xia, J. Evaluation of groundwater depletion in North China using the Gravity Recovery and Climate Experiment (GRACE) data and ground-based measurements. Water Resour. Res. 2013, 49, 2110–2118. [Google Scholar] [CrossRef]
- Amanambu, A.C.; Obarein, O.A.; Mossa, J.; Li, L.; Ayeni, S.S.; Balogun, O.; Oyebamiji, A.; Ochege, F.U. Groundwater system and climate change: Present status and future considerations. J. Hydrol. 2020, 589, 125163. [Google Scholar] [CrossRef]
- Gurdak, J.J. Climate-induced pumping. Nat. Geosci. 2017, 10, 71. [Google Scholar] [CrossRef]
- Peterson, T.; Western, A. Time-series modelling of groundwater head and its de-composition to historic climate periods. In Proceedings of the 34th World Congress of the International Association for Hydro-Environment Research and Engineering: 33rd Hydrology and Water Resources Symposium and 10th Conference on Hydraulics in Water Engineering, Brisbane, Australia, 26 June–1 July 2011; pp. 1677–1684. [Google Scholar]
- Wada, Y.; van Beek, L.P.H.; van Kempen, C.M.; Reckman, J.W.T.M.; Vasak, S.; Bierkens, M.F.P. Global depletion of groundwater resources. Geophys. Res. Lett. 2010, 37, L20402. [Google Scholar] [CrossRef] [Green Version]
- Wu, W.Y.; Lo, M.H.; Wada, Y.; Famiglietti, J.S.; Reager, J.T.; Yeh, P.J.; Ducharne, A.; Yang, Z.L. Divergent effects of climate change on future groundwater availability in key mid-latitude aquifers. Nat. Commun. 2020, 11, 3710. [Google Scholar] [CrossRef]
- Zhu, Y.; Luo, P.; Zhang, S.; Sun, B. Spatiotemporal Analysis of Hydrological Variations and Their Impacts on Vegetation in Semiarid Areas from Multiple Satellite Data. Remote Sens. 2020, 12, 4177. [Google Scholar] [CrossRef]
- Rodell, M.; Velicogna, I.; Famiglietti, J.S. Satellite-based estimates of groundwater depletion in India. Nature 2009, 460, 999–1002. [Google Scholar] [CrossRef] [Green Version]
- Konikow, L.F.; Kendy, E. Groundwater depletion: A global problem. Hydrogeol. J. 2005, 13, 317–320. [Google Scholar] [CrossRef]
- Fu, G.; Crosbie, R.S.; Barron, O.; Charles, S.P.; Dawes, W.; Shi, X.; Van Niel, T.; Li, C. Attributing variations of temporal and spatial groundwater recharge: A statistical analysis of climatic and non-climatic factors. J. Hydrol. 2019, 568, 816–834. [Google Scholar] [CrossRef]
- Gleeson, T.; Wada, Y.; Bierkens, M.F.P.; van Beek, L.P.H. Water balance of global aquifers revealed by groundwater footprint. Nature 2012, 488, 197–200. [Google Scholar] [CrossRef]
- Tularam, G.; Krishna, M. Long term consequences of groundwater pumping in Australia: A review of impacts around the globe. J. Appl. Sci. Environ. Sanit. 2009, 4, 151–166. [Google Scholar]
- Chen, H.; Guo, S.; Xu, C.-y.; Singh, V.P. Historical temporal trends of hydro-climatic variables and runoff response to climate variability and their relevance in water resource management in the Hanjiang basin. J. Hydrol. 2007, 344, 171–184. [Google Scholar] [CrossRef]
- Helsel, D.R.; Hirsch, R.M.; Ryberg, K.R.; Archfield, S.A.; Gilroy, E.J. Statistical Methods in Water Resources; 4-A3; US Geological Survey: Reston, VA, USA, 2020; p. 484. [Google Scholar]
- Montgomery, D.C.; Peck, E.A.; Vining, G.G. Introduction to Linear Regression Analysis, 5th ed.; John Wiley & Sons Inc.: Hoboken, NJ, USA, 2012. [Google Scholar]
- Snedecor, G.W.; Cochran, W.G. Statistical Methods, 4th ed.; The Iowa State College Press: Ames, IA, USA, 1946. [Google Scholar]
- Libiseller, C.; Grimvall, A. Performance of partial Mann–Kendall tests for trend detection in the presence of covariates. Environmetrics 2002, 13, 71–84. [Google Scholar] [CrossRef]
- Mann, H.B. Nonparametric Tests Against Trend. Econometrica 1945, 13, 245–259. [Google Scholar] [CrossRef]
- Aziz, O.I.A.; Burn, D.H. Trends and variability in the hydrological regime of the Mackenzie River Basin. J. Hydrol. 2006, 319, 282–294. [Google Scholar] [CrossRef]
- Thas, O.; Vooren, L.; Ottoy, J.-P. Selection of Nonparametric Methods for Monotonic Trend Detection in Water Quality. J. Am. Water. Res. Assoc. 1996, 34, 347–357. [Google Scholar] [CrossRef]
- Sen, P.K. Estimates of the regression coefficient based on Kendall’s tau. J. Am. Stat. Assoc. 1968, 63, 1379–1389. [Google Scholar] [CrossRef]
- Kendall, M.G. Rank Correlation Methods; Griffin: Oxford, UK, 1975. [Google Scholar]
- Hirsch, R.M.; Slack, J.R.; Smith, R.A. Techniques of trend analysis for monthly water quality data. Water Resour. Res. 1982, 18, 107–121. [Google Scholar] [CrossRef] [Green Version]
- Bouza-Deaño, R.; Ternero-Rodríguez, M.; Fernández-Espinosa, A.J. Trend study and assessment of surface water quality in the Ebro River (Spain). J. Hydrol. 2008, 361, 227–239. [Google Scholar] [CrossRef]
- Pal, L.; Ojha, C.S.P.; Chandniha, S.K.; Kumar, A. Regional scale analysis of trends in rainfall using nonparametric methods and wavelet transforms over a semi-arid region in India. Int. J. Climatol. 2019, 39, 2737–2764. [Google Scholar] [CrossRef]
- Deb, S.; Jana, K. Nonparametric quantile regression for time series with replicated observations and its application to climate data. arXiv 2021, arXiv:2107.02091. [Google Scholar]
- Xu, J.; Chen, Y.; Li, W.; Ji, M.; Dong, S.; Hong, Y. Wavelet analysis and nonparametric test for climate change in Tarim River Basin of Xinjiang during 1959–2006. Chin. Geogr. Sci. 2009, 19, 306–313. [Google Scholar] [CrossRef] [Green Version]
- Galeati, G. A comparison of parametric and non-parametric methods for runoff forecasting. Hydrol. Sci. J. 1990, 35, 79–94. [Google Scholar] [CrossRef]
- Bui, D.D.; Kawamura, A.; Tong, T.N.; Amaguchi, H.; Nakagawa, N. Spatio-temporal analysis of recent groundwater-level trends in the Red River Delta, Vietnam. Hydrogeol. J. 2012, 20, 1635–1650. [Google Scholar] [CrossRef]
- Kumar, P.; Chandniha, S.K.; Lohani, A.K.; Nema, A.K.; Krishan, G. Trend Analysis of Groundwater Level Using Non-Parametric Tests in Alluvial Aquifers of Uttar Pradesh, India. Curr. World Environ. 2018, 13, 44–54. [Google Scholar] [CrossRef] [Green Version]
- Mirabbasi, R.; Ahmadi, F.; Jhajharia, D. Comparison of parametric and non-parametric methods for trend identification in groundwater levels in Sirjan plain aquifer, Iran. Hydrol. Res. 2020, 51, 1455–1477. [Google Scholar] [CrossRef]
- Şen, Z. Innovative Trend Analysis Methodology. J. Hydrol. Eng. 2012, 17, 1042–1046. [Google Scholar] [CrossRef]
- Serinaldi, F.; Chebana, F.; Kilsby, C.G. Dissecting innovative trend analysis. Stoch. Environ. Res. Risk Assess. 2020, 34, 733–754. [Google Scholar] [CrossRef]
- Thomas, B.F.; Famiglietti, J.S. Identifying Climate-Induced Groundwater Depletion in GRACE Observations. Sci. Rep. 2019, 9, 4124. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Wang, D.; Zhang, R.; Shi, Y. General Hydrogeology; Geology Publishing House: Bath, UK, 1980. [Google Scholar]
- Ferdowsian, R.; Pannell, D.J.; McCarron, C.; Ryder, A.; Crossing, L. Explaining groundwater hydrographs: Separating atypical rainfall events from time trends. Soil. Res. 2001, 39, 861–876. [Google Scholar] [CrossRef] [Green Version]
- Ferdowsian, R.; Ryder, A.; George, R.; Bee, G.; Smart, R. Groundwater level reductions under lucerne depend on the landform and groundwater flow systems (local or intermediate). Soil. Res. 2002, 40, 381–396. [Google Scholar] [CrossRef]
- Ali, R.; McFarlane, D.; Varma, S.; Dawes, W.; Emelyanova, I.; Hodgson, G.; Charles, S. Potential climate change impacts on groundwater resources of south-western Australia. J. Hydrol. 2012, 475, 456–472. [Google Scholar] [CrossRef]
- WAPC. Western Australia Tomorrow: Population Projections for Planning Regions 2004 to 2031 and Local Government Areas 2004 to 2021; Western Australian Planning Commission: Perth, WA, Australia, 2005.
- Tapsuwan, S.; Leviston, Z.; Tucker, D. Community values and attitudes towards land use on the Gnangara Groundwater System: A Sense of Place study in Perth, Western Australia. Landsc. Urban Plan. 2011, 100, 24–34. [Google Scholar] [CrossRef]
- Kong, F.; Song, J.; Crosbie, R.S.; Barron, O.; Schafer, D.; Pigois, J.-P. Groundwater Hydrograph Decomposition With the HydroSight Model. Front. Environ. Sci. 2021, 9, 736400. [Google Scholar] [CrossRef]
- Yesertener, C. Impacts of climate, land and water use on declining groundwater levels in the Gnangara Groundwater Mound, Perth, Australia. Australas. J. Water Resour. 2005, 8, 143–152. [Google Scholar] [CrossRef]
- Davidson, W.A.; Yu, X. Perth Regional Aquifer Modelling System (PRAMS) Model Development: Hydrogeology and Groundwater Modelling, Hydrogeological Record Series HG 20; Department of Water, Government of Western Australia: Perth, Australia, 2008.
- Merz, S.K. Development of Local Area Groundwater Models—Gnangara Mound Lake Bindiar Model Report; Department of Water: Perth, Australia, Government of Western Australia, 2009.
- Department of Water, Government of Western Australia. Gnangara Groundwater Areas Allocation Plan; Report No. 30; Department of Water, Government of Western Australia: Perth, Australia, 2009. [Google Scholar]
- Davidson, W.A. Hydrogeology and Groundwater Resources of the Perth Region, Western Australia; Geological Survey of WA: Perth, Australia, 1995; Volume 142. [Google Scholar]
- Gilbert, R.O. Statistical Methods for Environmental Pollution Monitoring; John Wiley & Sons: Hoboken, NJ, USA, 1987. [Google Scholar]
- Department of Water, Government of Western Australia. Groundwater Level Trends Analysis for the South West Groundwater Areas; Department of Water, Government of Western Australia: Perth, Australia, 2008. [Google Scholar]
- Majidi, R.; Ferdowsian, R.; McCarron, C. HARTT: User-Friendly Software for Hydrograph Analysis to Separate Rainfall and Time Trend. In Proceedings of the Water Challenge: Balancing the Risks: Hydrology and Water Resources Symposium, Melbourne, Australia, 27 January 2002; pp. 314–320. [Google Scholar]
- Dinda, S. Handbook of Research on Climate Change Impact on Health and Environmental Sustainability; IGI Global: Hershey, PA, USA, 2015. [Google Scholar]
- Yesertener, C. Assessment of the Declining Groundwater Levels in the Gnangara Groundwater Mound; Department of Water, Government of Western Australia: Perth, Australia, 2007. [Google Scholar]











Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kong, F.; Xu, W.; Mao, R.; Liang, D. Dynamic Changes in Groundwater Level under Climate Changes in the Gnangara Region, Western Australia. Water 2022, 14, 162. https://doi.org/10.3390/w14020162
Kong F, Xu W, Mao R, Liang D. Dynamic Changes in Groundwater Level under Climate Changes in the Gnangara Region, Western Australia. Water. 2022; 14(2):162. https://doi.org/10.3390/w14020162
Chicago/Turabian StyleKong, Feihe, Wenjin Xu, Ruichen Mao, and Dong Liang. 2022. "Dynamic Changes in Groundwater Level under Climate Changes in the Gnangara Region, Western Australia" Water 14, no. 2: 162. https://doi.org/10.3390/w14020162
APA StyleKong, F., Xu, W., Mao, R., & Liang, D. (2022). Dynamic Changes in Groundwater Level under Climate Changes in the Gnangara Region, Western Australia. Water, 14(2), 162. https://doi.org/10.3390/w14020162






