On Hypsometric Curve and Morphological Analysis of the Collapsed Irrigation Reservoirs
Abstract
:1. Introduction
2. Methodology and Material
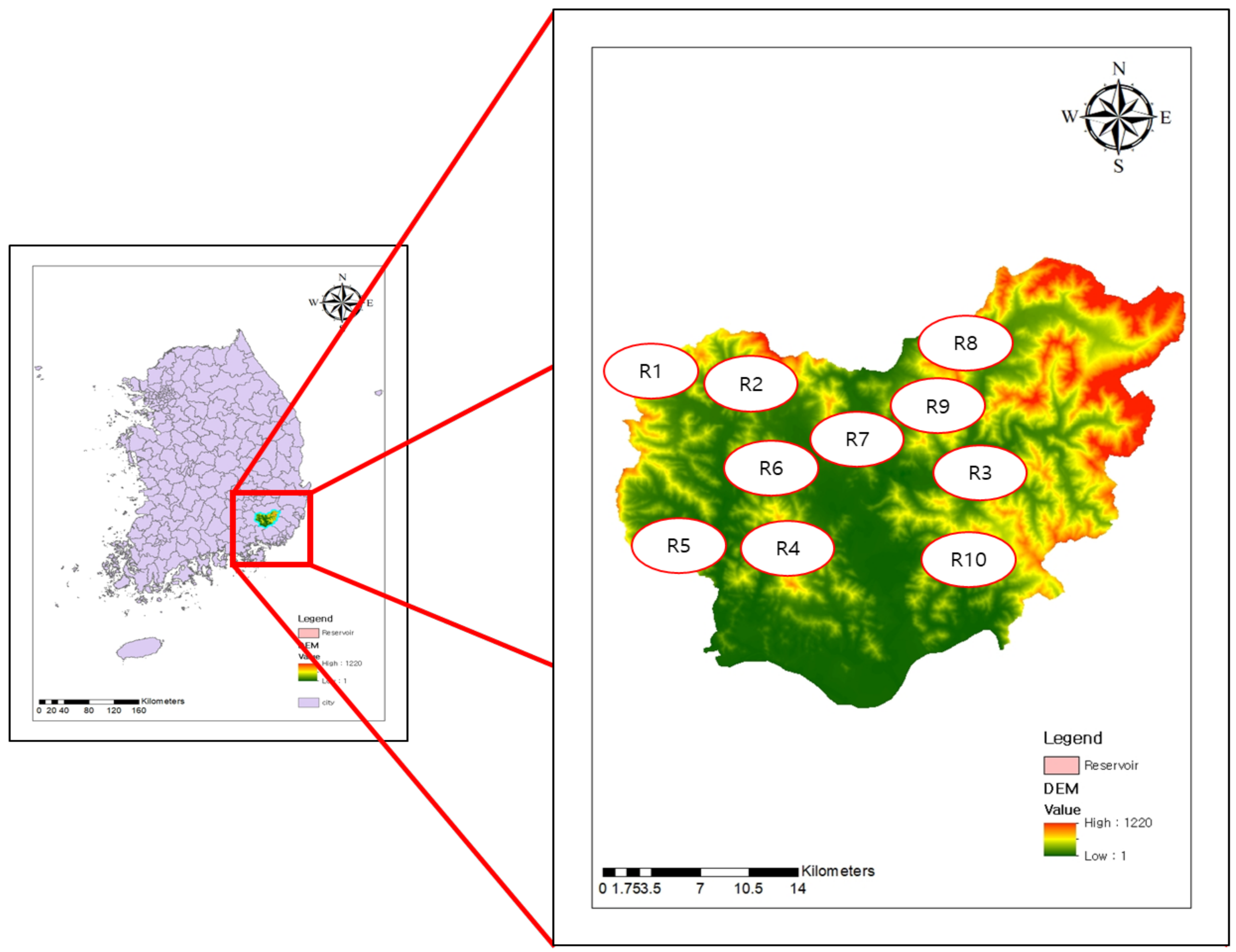
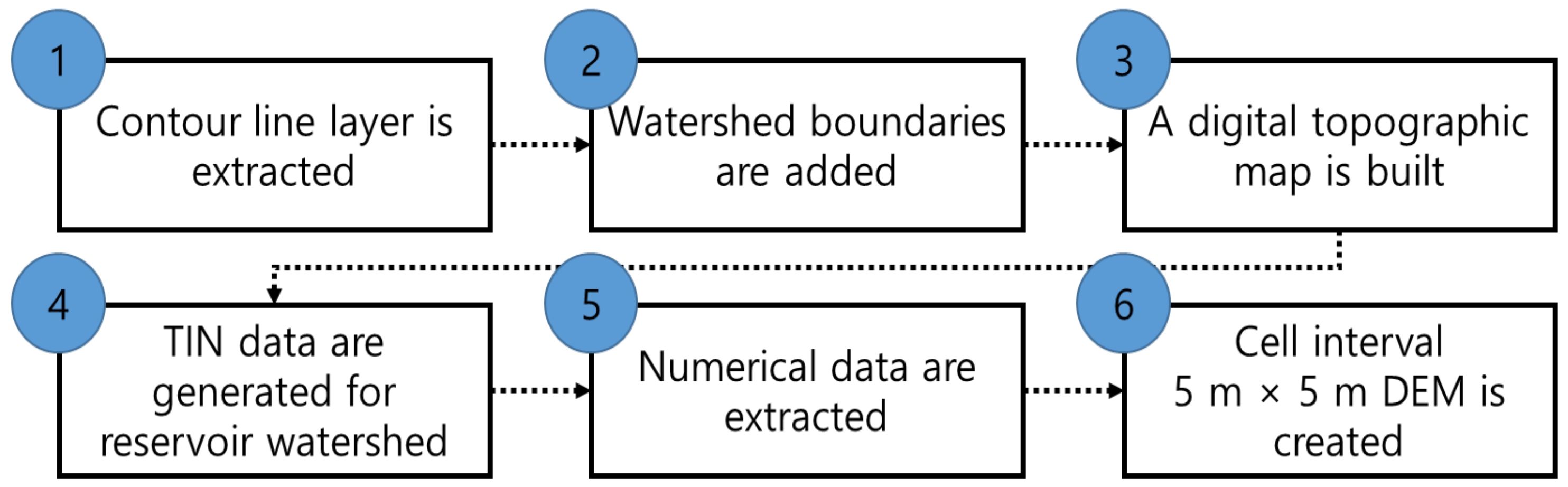
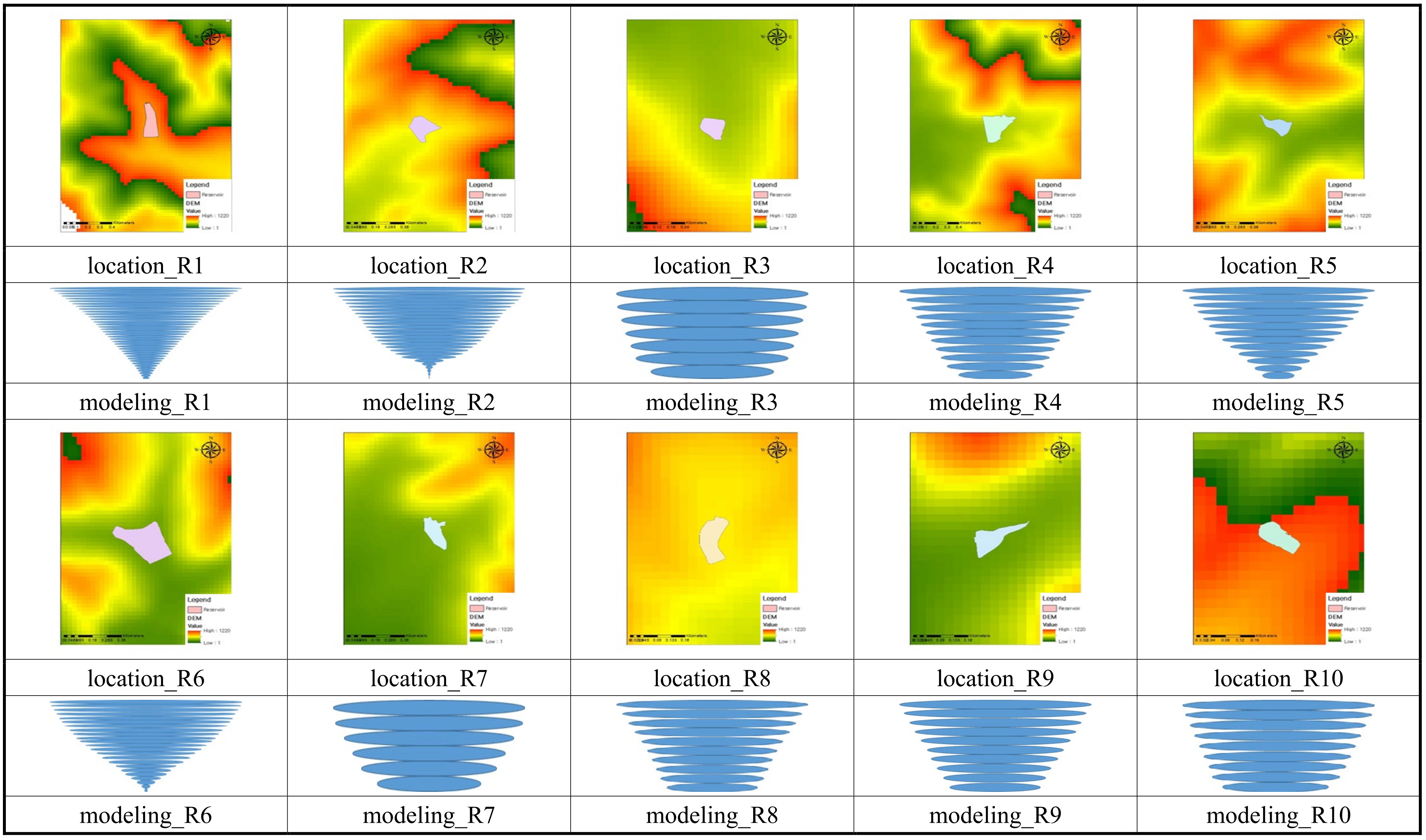
2.1. Study Area
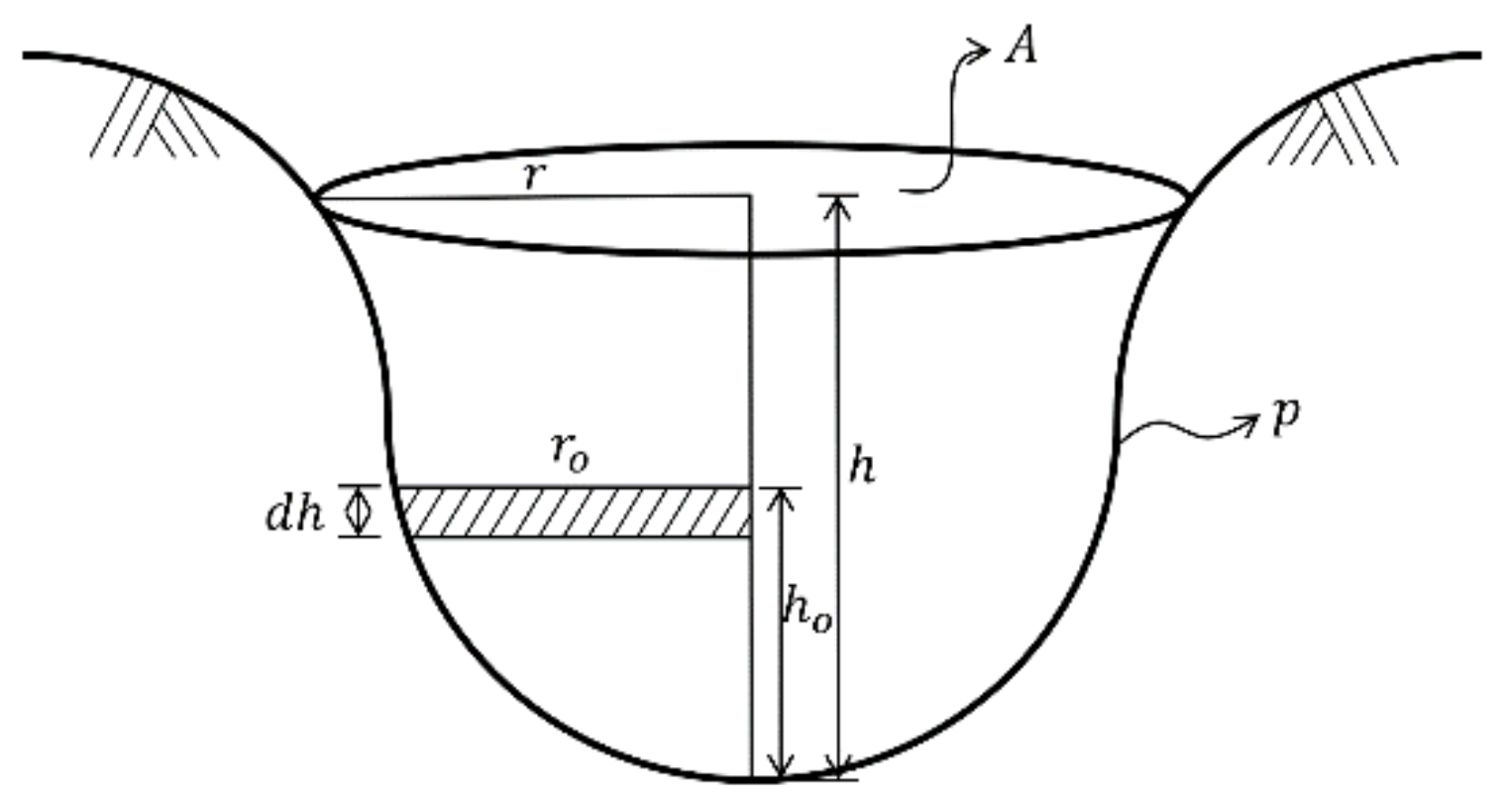
2.2. The Area-Volume Relationship of Reservoirs According to the Depth of Water
2.3. Morphology Index and Equations for the Relationships between Basic Properties
2.3.1. Morphology Index
2.3.2. Full Water Storage Area-Levee Height Relationship
2.3.3. Full Water Storage Area Relationship
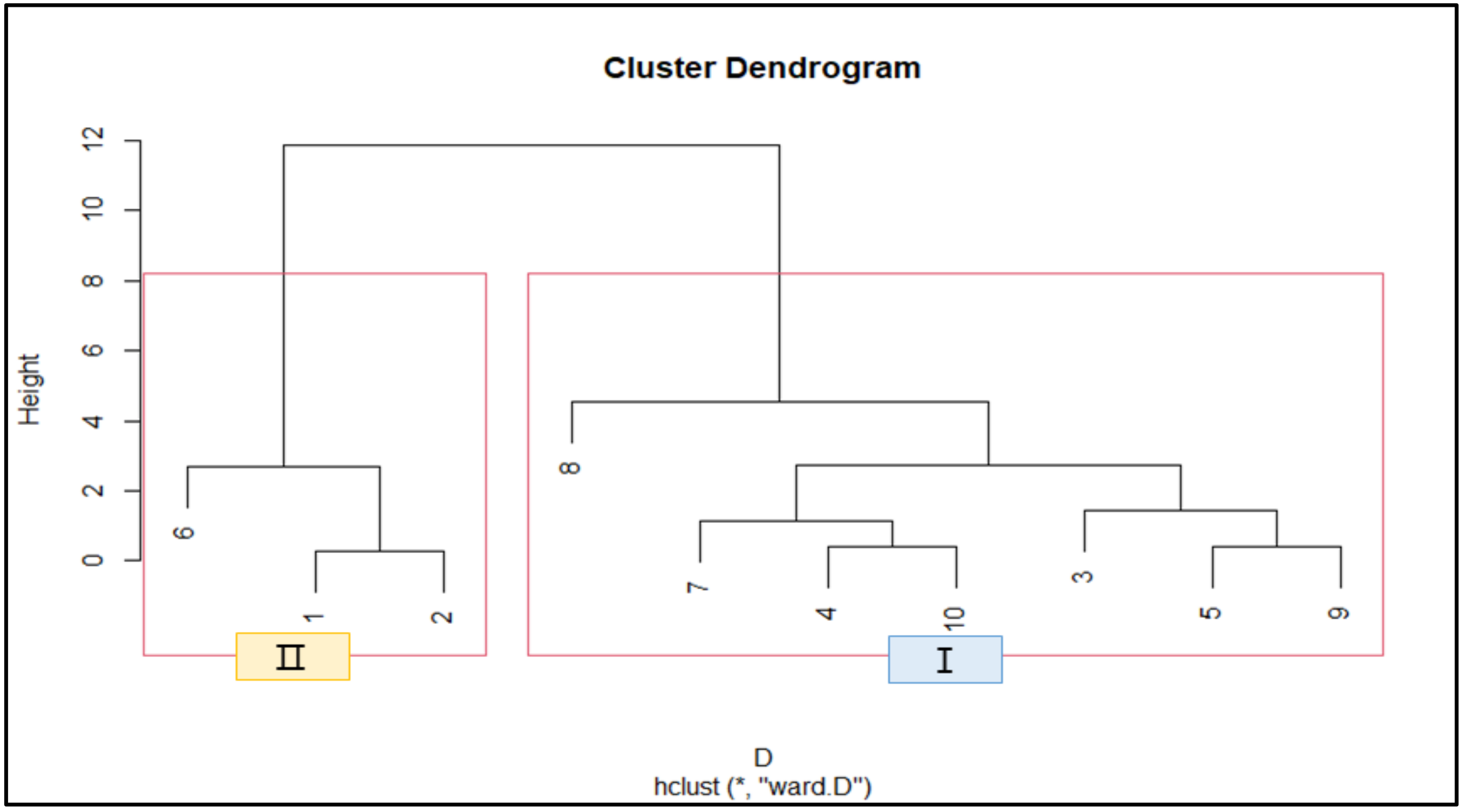
2.4. Cluster Analysis
3. Results
3.1. Data Description
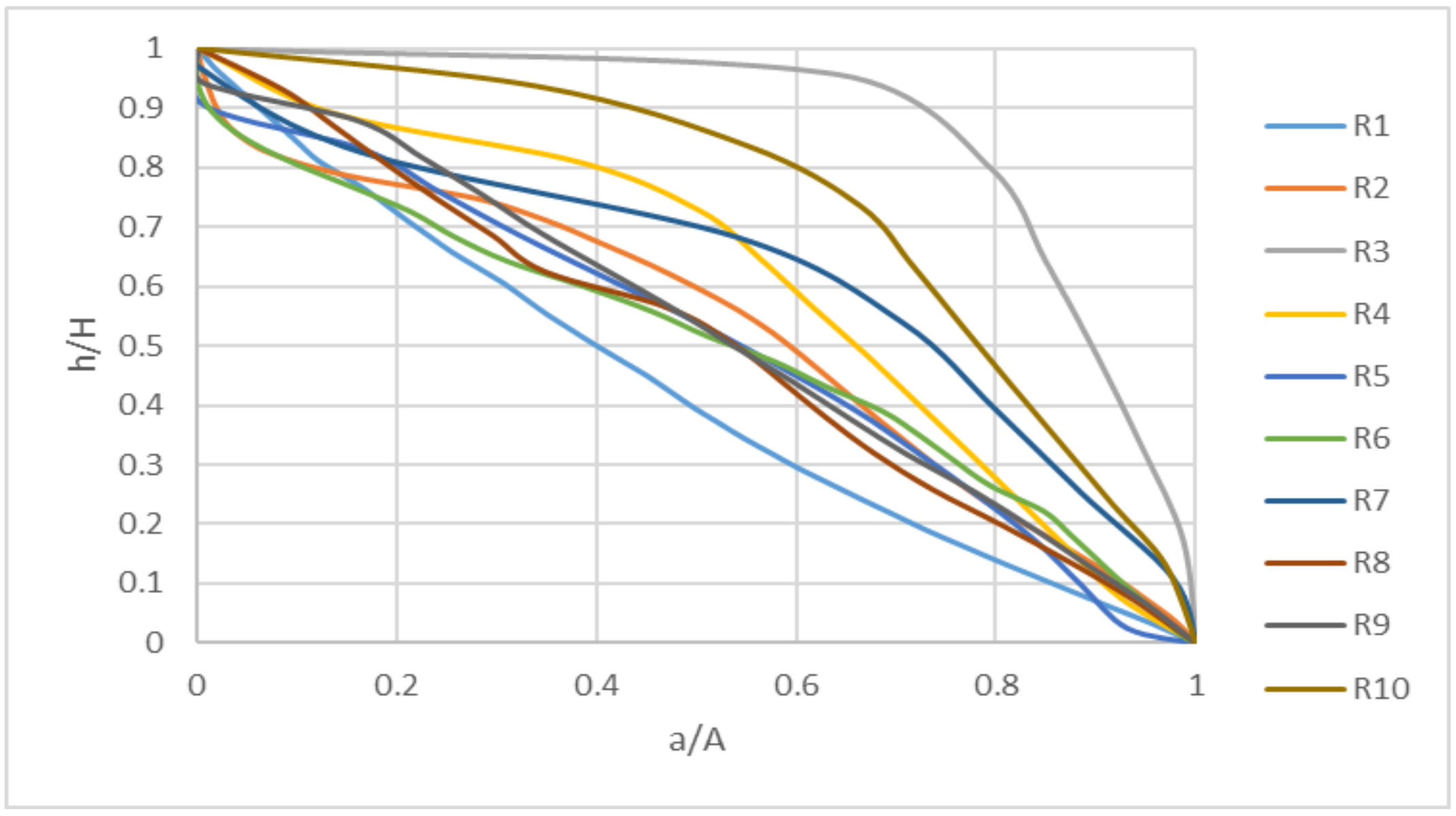
3.2. Estimation of the Area-Volume Relationship of a Reservoir According to the Water Depth
3.3. Analysis of the Morphological Characteristics of the Reservoirs
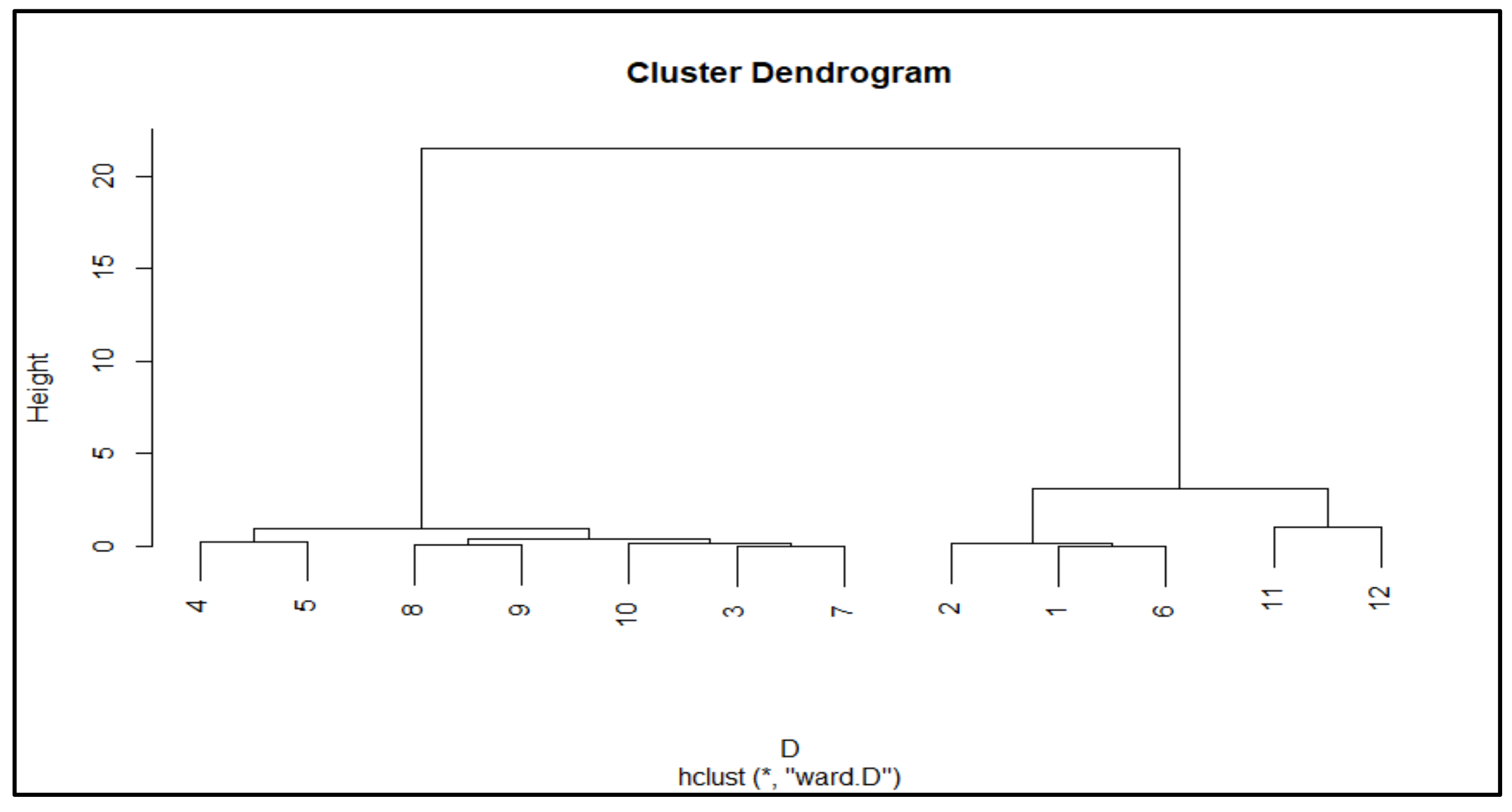
3.4. Analysis of Reservoir Characteristics through Cluster Analysis
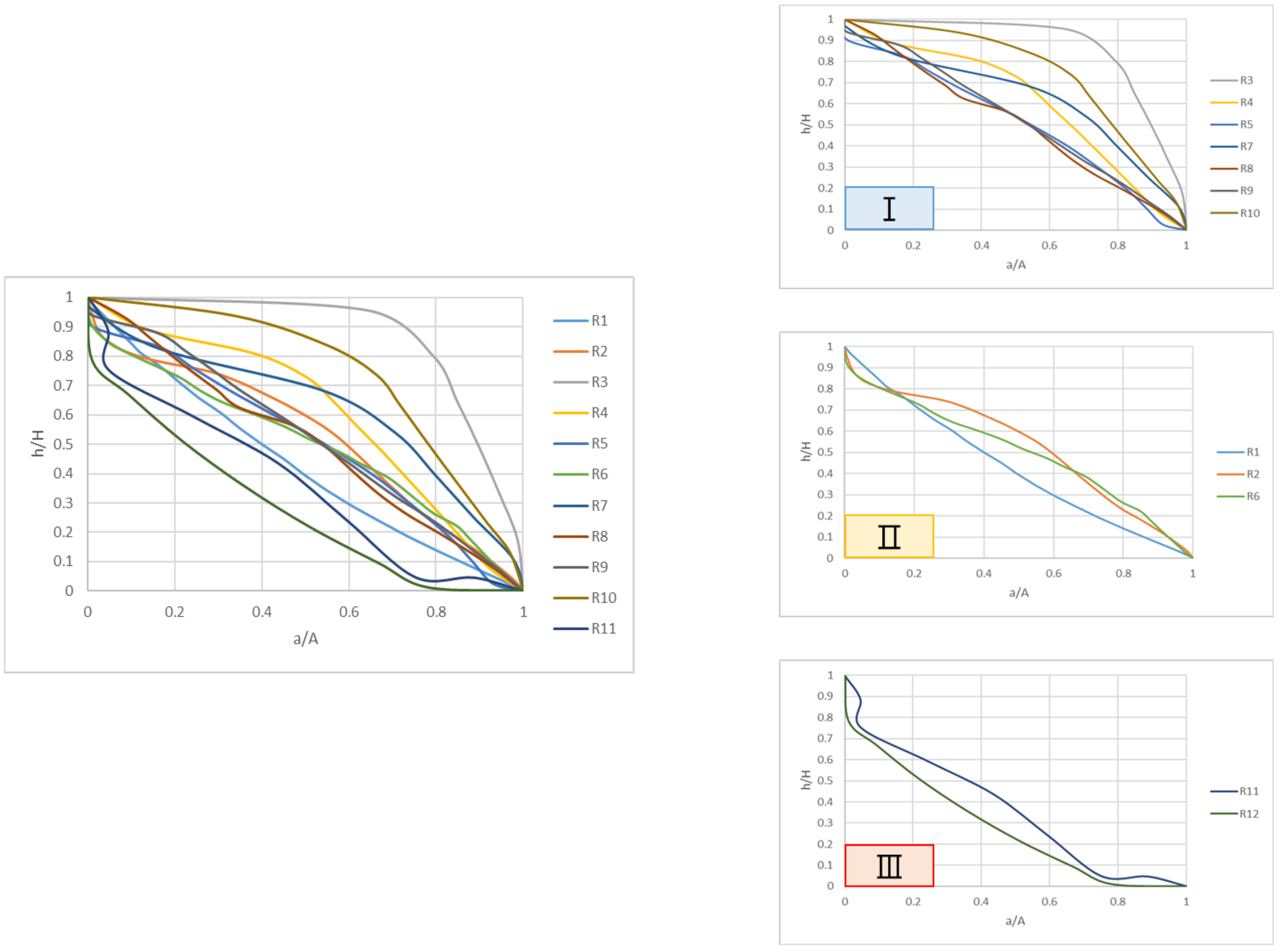
3.5. Morphological Analysis of the Collapsed Reservoirs Using Cluster Analysis and HC
4. Discussions and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Groombridge, B.; Jenkins, M. Freshwater Biodiversity: A Preliminary Global Assessment; WCMC Biodiversity Series Number 8; World Conservation Press: Cambridge, UK, 1998. [Google Scholar]
- Mitsch, W.J.; Gosselink, J.G. Wetlands, 3rd ed.; Wiley: New York, NY, USA, 2000. [Google Scholar]
- Revenga, C.; Brunner, J.; Henninger, N.; Kassem, K.; Payne, R. Pilot Analysis of Global Ecosystems (PAGE): Freshwater Systems; World Resources Institute (WRI): Washington, DC, USA, 2000. [Google Scholar]
- Sanderson, M.G. Global Distribution of Freshwater Wetlands for Use in STOCHEM; Technical Note 32 (HTCN 32); Hadley Centre for Climate Prediction and Research, Met Office: Bracknell, UK, 2001.
- Wetzel, R.G. Limnology: Lake and River Ecosystems, 3rd ed.; Academic Press: San Diego, CA, USA, 2001. [Google Scholar]
- Kim, D.H.; Kim, J.S.; Choi, C.H.; Wang, W.J.; You, Y.H.; Kim, H.S. Estimations of Hazard-Triggering Rainfall and Breach Discharge of Aging Reservoir. J. Korean Soc. Hazard Mitig. 2019, 19, 421–432. [Google Scholar] [CrossRef] [Green Version]
- National Disaster Management Research Institute. Annual Report. 2019. Available online: https://nidm.gov.in/PDF/pubs/AR2020.pdf (accessed on 15 November 2021).
- Kim, D.H.; Kim, J.S.; Wang, W.J.; Lee, J.S.; Jung, J.W.; Kim, H.S. Analysis of Morphological Characteristics of Collapsed Reservoirs in Korea. J. Korean Soc. Hazard Mitig. 2020, 20, 207–216. [Google Scholar] [CrossRef]
- Hong, B.M. Problems and improvement plans for the construction of agricultural reservoirs. Water Future 2004, 37, 29–33. [Google Scholar]
- Yoo, C.S.; Park, H.K. Analysis of Morphological Characteristics of Farm Dams in Korea. J. Korean Geogr. Soc. 2007, 42, 940–954. [Google Scholar]
- Graf, W.L. Dam nation: A geographic census of American dams and their large-scale hydrologic impacts. Water Resour. Res. 1999, 35, 1305–1311. [Google Scholar] [CrossRef]
- McDonald, C.P.; Rover, J.A.; Stets, E.G.; Striegl, R.G. The regional abundance and size distribution of lakes and reservoirs in the United States and implications for estimates of global lake extent. Limnol. Oceanogr. 2012, 57, 597–606. [Google Scholar] [CrossRef]
- Chang, Y.K.; Park, J.Y.; Moon, D.Y.; Kang, I.J. Calculation of Reservoir Capacity by Combination of GPS and Echo Sounder. J. Korean Soc. Geospat. Inf. Sci. 2002, 10, 27–35. [Google Scholar]
- Park, S.K.; Jeong, J.H. Calculation of Sediment Volume of the Agriculture Reservoir Using DGPS Echo-Sounder. J. GIS Assoc. Korea 2005, 13, 297–307. [Google Scholar]
- Choi, B.G.; Lee, H.S. Measuring Water Volume of Reservoir by Echosounding. J. Korea Soc. Geospat. Inf. Syst. 2007, 15, 55–59. [Google Scholar]
- Guerrero, M.; Lamberti, A. Flow Field and Morphology Mapping Using ADCP and Multibeam Techniques: Survey in the Po River. J. Hydraul. Eng. 2011, 137, 1576–1587. [Google Scholar] [CrossRef]
- Ilci, V.; Ozulu, I.M.; Alkan, R.M.; Erol, S.; Uysal, M. Determination of Reservoir Sedimentation with Bathymetric Survey: A Case Study of Obruk Dam Lake. J. Fresenius Environ. Bull. 2019, 28, 2305–2313. [Google Scholar]
- Song, B.G.; Oh, J.Y.; Kim, S.S.; Lee, T.W.; Park, K.H. Analysis of 3D Topographic Information on Reservoir Using UAV and Echo Sounder. J. Korean Soc. Hazard Mitig. 2018, 18, 563–568. [Google Scholar] [CrossRef] [Green Version]
- Langbein, W.B. Topographic Characteristics of Drainage Basin. U.S. Geolological Survey W.S. Paper 968-C. 1947. Available online: https://pubs.usgs.gov/wsp/0968c/report.pdf (accessed on 15 November 2021).
- Hayashi, M.; Kamp, G.V. Simple equations to represent the volume-area depth relations of shallow wetlands in small topographic depression. J. Hydrol. 2000, 237, 74–85. [Google Scholar] [CrossRef]
- Oertel, G.F. Hypsographic, hydro-hysographic and hydrological analysis of coastal bay environments, Great Machipongo Bay, Virginia. J. Coast. Res. 2001, 17, 775–783. [Google Scholar]
- Kim, J.G.; Kim, H.S.; Jeong, S.M. Estimation of Volume-Area-Depth Relationship for Shallow Wetland. J. Korea Water Resour. Assoc. 2002, 35, 231–240. [Google Scholar]
- Nam, W.H.; Kim, T.G.; Hong, E.M.; Hayes, M.J.; Svoboda, M.D. Water Supply Risk Assessment of Agricultural Reservoirs using Irrigation Vulnerability Model and Cluster Analysis. J. Korean Soc. Agric. Eng. 2015, 57, 59–67. [Google Scholar]
- Takeuchi, K. Least marginal environmental impact rule for reservoir development. Hydrol. Sci. J. 1997, 42, 583–597. [Google Scholar] [CrossRef] [Green Version]
- Leonard, J.; Crouzet, P. Lakes and Reservoir in the EEA Area; Topoc Report No. 1/1999; European Environment Agency (EEA): Copenhagen, Denmark, 1999. [Google Scholar]
- Lehner, B.; Doll, P. Development and validation of a global database of lakes, reservoir and wetlands. J. Hydrol. 2004, 296, 1–22. [Google Scholar] [CrossRef]
- Dargahi, B.; Setegn, S.G. Combined 3D hydrodynamic and watershed modelling of Lake Tana, Ethiopia. J. Hydrol. 2011, 398, 44–64. [Google Scholar] [CrossRef]
- Li, Y.; Zhang, Q.; Yao, J.; Werner, A.D.; Li, X. Hydrodynamic and hydrological modeling of the Poyang Lake catchment system in China. J. Hydrol. Eng. 2014, 19, 607–616. [Google Scholar] [CrossRef]
- Zhang, L.; Lu, J.; Chen, X.; Liang, D.; Fu, X.; Sauvage, S.; Sanchez Perez, J.-M. Stream flow simulation and verification in ungauged zones by coupling hydrological and hydrodynamic models: A case study of the Poyang Lake ungauged zone. Hydrol. Earth Syst. Sci. 2017, 21, 5847–5861. [Google Scholar] [CrossRef] [Green Version]
- Lopes, V.A.R.; Fan, F.M.; Pontes, P.R.M.; Siqueira, V.A.; Collischonn, W.; da Motta Marques, D. A first integrated modelling of a river-lagoon large-scale hydrological system for forecasting purposes. J. Hydrol. 2018, 565, 177–196. [Google Scholar] [CrossRef]
- Munar, A.M.; Cavalcanti, J.R.; Bravo, J.M.; Fan, F.M.; da Motta-Marques, D.; Fragoso, C.R. Coupling large-scale hydrological and hydrodynamic modeling: Toward a better comprehension of watershed-shallow lake processes. J. Hydrol. 2018, 564, 424–441. [Google Scholar] [CrossRef]
- Kyoung, M.S.; Kim, S.D.; Kim, B.K.; Kim, H.S. Construction of hydrological drought severity-area-duration curves using cluster analysis. J. Korean Soc. Civ. Eng. 2007, 27, 267–276. [Google Scholar]
- Han, S.M.; Hwang, G.S.; Choe, S.Y.; Park, J.W. A study on classifying algorithm of disaster recovery resources using statistical method. J. Korean Soc. Hazard Mitig. 2014, 14, 49–58. [Google Scholar] [CrossRef] [Green Version]
- Sarp, G.; Duzgun, S.; Toprak, V. Hypsometric properties of the hydrolic basins located on western part of NAFZ. In Proceedings of the 34th International Symposium on Remote Sensing of Environment, The GEOSS Era: Towards Operational Environmental Monitoring, Sydney, Australia, 10–15 April 2011. [Google Scholar]


| h(m) | R1 | R2 | R3 | R4 | R5 | R6 | R7 | R8 | R9 | R10 |
|---|---|---|---|---|---|---|---|---|---|---|
| 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 |
| 0.5 | 1012 | 59 | 4033 | 1488 | 107 | 7 | 18 | 1073 | 53 | 4299 |
| 1.0 | 1542 | 227 | 5000 | 4177 | 2554 | 151 | 3385 | 1704 | 1467 | 6950 |
| 1.5 | 2088 | 433 | 5350 | 5510 | 3767 | 1060 | 11,230 | 2330 | 2071 | 8360 |
| 2.0 | 2634 | 809 | 5670 | 6180 | 5020 | 3053 | 14,660 | 2984 | 2628 | 9020 |
| 2.5 | 3164 | 1646 | 5960 | 6760 | 6390 | 6310 | 16,560 | 3679 | 3151 | 9640 |
| 3.0 | 3694 | 3402 | 6220 | 7360 | 7770 | 10,370 | 18,380 | 4332 | 3752 | 10,270 |
| 3.5 | 4477 | 6260 | 6300 | 7920 | 9030 | 14,330 | 20,290 | 5880 | 4354 | 10,910 |
| 4.0 | 5260 | 7870 | 8480 | 10,200 | 17,230 | 20,760 | 6690 | 4910 | 11,550 | |
| 4.5 | 5905 | 9080 | 9030 | 11,190 | 20,840 | 7270 | 5480 | 12,210 | ||
| 5.0 | 6550 | 10,230 | 9580 | 12,130 | 25,910 | 7830 | 6010 | 12,590 | ||
| 5.5 | 7235 | 11,250 | 10,150 | 12,990 | 30,470 | 8440 | 6560 | |||
| 6.0 | 7920 | 12,180 | 10,970 | 13,690 | 34,170 | 9170 | 7180 | |||
| 6.5 | 8720 | 12,950 | 14,410 | 38,610 | 10,050 | 7730 | ||||
| 7.0 | 9520 | 13,600 | 15,470 | 42,050 | 10,880 | 8240 | ||||
| 7.5 | 10,190 | 14,210 | 45,860 | 11,650 | 8760 | |||||
| 8.0 | 10,860 | 14,810 | 48,400 | 12,460 | 9230 | |||||
| 8.5 | 11,620 | 15,410 | 50,700 | |||||||
| 9.0 | 12,380 | 16,040 | 53,100 | |||||||
| 9.5 | 13,190 | 16,670 | 56,600 | |||||||
| 10.0 | 14,000 | 17,340 | 58,500 | |||||||
| 10.5 | 14,705 | 18,110 | 60,300 | |||||||
| 11.0 | 15,410 | 19,030 | 62,100 | |||||||
| 11.5 | 16,195 | 19,890 | 64,000 | |||||||
| 12.0 | 16,980 | 20,700 | 65,900 | |||||||
| 12.5 | 17,860 | 21,420 | 66,800 | |||||||
| 13.0 | 18,740 | 22,090 | ||||||||
| 13.5 | 19,720 | 22,530 | ||||||||
| 14.0 | 20,700 | |||||||||
| 14.5 | 21,715 | |||||||||
| 15.0 | 22,730 | |||||||||
| 15.5 | 23,845 | |||||||||
| 16.0 | 24,960 | |||||||||
| 16.5 | 26,135 | |||||||||
| 17.0 | 27,310 | |||||||||
| 17.5 | 28,510 | |||||||||
| 18.0 | 29,710 | |||||||||
| 18.5 | 31,070 | |||||||||
| h(m) | R1 | R2 | R3 | R4 | R5 | R6 | R7 | R8 | R9 | R10 |
|---|---|---|---|---|---|---|---|---|---|---|
| 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 |
| 0.5 | 1012 | 59 | 4029 | 1484 | 107 | 7 | 18 | 1030 | 53 | 4084 |
| 1.0 | 1542 | 227 | 4995 | 4164 | 2549 | 151 | 3375 | 1635 | 1466 | 6603 |
| 1.5 | 2088 | 432 | 5345 | 5493 | 3759 | 1059 | 11,196 | 2236 | 2069 | 7942 |
| 2.0 | 2634 | 807 | 5664 | 6161 | 5010 | 3050 | 14,616 | 2864 | 2625 | 8569 |
| 2.5 | 3164 | 1643 | 5954 | 6740 | 6377 | 6304 | 16,510 | 3531 | 3148 | 9158 |
| 3.0 | 3694 | 3395 | 6214 | 7338 | 7754 | 10,360 | 18,325 | 4158 | 3748 | 9757 |
| 3.5 | 4477 | 6247 | 6294 | 7896 | 9012 | 14,316 | 20,229 | 5644 | 4350 | 10,365 |
| 4.0 | 5260 | 7854 | 8455 | 10,180 | 17,213 | 20,698 | 6422 | 4905 | 10,973 | |
| 4.5 | 5905 | 9062 | 9003 | 11,168 | 20,819 | 6979 | 5475 | 11,600 | ||
| 5.0 | 6550 | 10,210 | 9551 | 12,106 | 25,884 | 7516 | 6004 | 11,961 | ||
| 5.5 | 7235 | 11,228 | 10,120 | 12,964 | 30,440 | 8102 | 6553 | |||
| 6.0 | 7920 | 12,156 | 10,937 | 13,663 | 34,136 | 8803 | 7173 | |||
| 6.5 | 8720 | 12,924 | 14,381 | 38,571 | 9648 | 7722 | ||||
| 7.0 | 9520 | 13,573 | 15,439 | 42,008 | 10,444 | 8232 | ||||
| 7.5 | 10,190 | 14,182 | 45,814 | 11,184 | 8751 | |||||
| 8.0 | 10,860 | 14,780 | 48,352 | 11,961 | 9221 | |||||
| 8.5 | 11,620 | 15,379 | 50,649 | |||||||
| 9.0 | 12,380 | 16,008 | 53,047 | |||||||
| 9.5 | 13,190 | 16,637 | 56,543 | |||||||
| 10.0 | 14,000 | 17,305 | 58,442 | |||||||
| 10.5 | 14,705 | 18,074 | 60,240 | |||||||
| 11.0 | 15,410 | 18,992 | 62,038 | |||||||
| 11.5 | 16,195 | 19,850 | 63,936 | |||||||
| 12.0 | 16,980 | 20,659 | 65,834 | |||||||
| 12.5 | 17,860 | 21,377 | 66,733 | |||||||
| 13.0 | 18,740 | 22,046 | ||||||||
| 13.5 | 19,720 | 22,485 | ||||||||
| 14.0 | 20,700 | |||||||||
| 14.5 | 21,715 | |||||||||
| 15.0 | 22,730 | |||||||||
| 15.5 | 23,845 | |||||||||
| 16.0 | 24,960 | |||||||||
| 16.5 | 26,135 | |||||||||
| 17.0 | 27,310 | |||||||||
| 17.5 | 28,510 | |||||||||
| 18.0 | 29,710 | |||||||||
| 18.5 | 31,070 | |||||||||
| h(m) | R1 | R2 | R3 | R4 | R5 | R6 | R7 | R8 | R9 | R10 |
|---|---|---|---|---|---|---|---|---|---|---|
| 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 |
| 0.5 | 843 | 14 | 1109 | 342 | 9 | 1 | 3 | 333 | 14 | 1397 |
| 1.0 | 2081 | 139 | 4742 | 2719 | 891 | 51 | 1497 | 1555 | 790 | 6468 |
| 1.5 | 3866 | 485 | 8021 | 7072 | 3698 | 696 | 10,084 | 3268 | 2724 | 12,631 |
| 2.0 | 6209 | 1223 | 11,296 | 11,456 | 7337 | 3393 | 24,337 | 5633 | 4793 | 18,684 |
| 2.5 | 9074 | 3032 | 14,828 | 15,916 | 12,380 | 10,065 | 37,152 | 8729 | 7339 | 24,725 |
| 3.0 | 12,447 | 7496 | 18,575 | 20,898 | 18,904 | 22,101 | 50,314 | 12,497 | 10,493 | 31,358 |
| 3.5 | 16,873 | 16,764 | 19,969 | 26,434 | 26,628 | 38,903 | 65,352 | 18,484 | 14,348 | 38,654 |
| 4.0 | 22,541 | 28,048 | 32,472 | 35,287 | 57,597 | 71,632 | 25,894 | 18,713 | 46,605 | |
| 4.5 | 28,638 | 37,883 | 39,047 | 44,598 | 78,995 | 32,248 | 23,585 | 55,242 | ||
| 5.0 | 35,061 | 47,985 | 46,153 | 54,452 | 108,694 | 38,656 | 28,955 | 61,628 | ||
| 5.5 | 42,251 | 58,748 | 53,863 | 64,935 | 145,179 | 45,719 | 34,819 | |||
| 6.0 | 50,239 | 69,939 | 64,733 | 75,638 | 182,608 | 53,887 | 41,495 | |||
| 6.5 | 59,322 | 81,296 | 86,689 | 223,799 | 63,618 | 48,756 | ||||
| 7.0 | 69,586 | 92,527 | 102,040 | 268,195 | 74,511 | 56,214 | ||||
| 7.5 | 80,121 | 103,870 | 314,278 | 85,839 | 64,090 | |||||
| 8.0 | 90,831 | 115,645 | 360,545 | 98,851 | 72,050 | |||||
| 8.5 | 102,621 | 127,982 | 403,833 | |||||||
| 9.0 | 115,560 | 141,053 | 448,935 | |||||||
| 9.5 | 129,512 | 154,882 | 501,878 | |||||||
| 10.0 | 144,515 | 169,540 | 555,358 | |||||||
| 10.5 | 159,743 | 185,581 | 602,910 | |||||||
| 11.0 | 175,119 | 203,713 | 651,780 | |||||||
| 11.5 | 191,684 | 223,206 | 703,008 | |||||||
| 12.0 | 209,500 | 242,931 | 756,668 | |||||||
| 12.5 | 228,725 | 262,618 | 791,556 | |||||||
| 13.0 | 249,429 | 282,162 | ||||||||
| 13.5 | 271,720 | 297,392 | ||||||||
| 14.0 | 295,672 | |||||||||
| 14.5 | 320,869 | |||||||||
| 15.0 | 347,338 | |||||||||
| 15.5 | 375,627 | |||||||||
| 16.0 | 405,814 | |||||||||
| 16.5 | 437,629 | |||||||||
| 17.0 | 471,118 | |||||||||
| 17.5 | 506,008 | |||||||||
| 18.0 | 542,319 | |||||||||
| 18.5 | 584,400 | |||||||||
| h(m) | R1 | R2 | R3 | R4 | R5 | R6 | R7 | R8 | R9 | R10 |
|---|---|---|---|---|---|---|---|---|---|---|
| 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 |
| 0.5 | 252 | 15 | 1007 | 371 | 27 | 2 | 4 | 258 | 13 | 1021 |
| 1.0 | 1273 | 143 | 4512 | 2824 | 1328 | 79 | 1696 | 1333 | 759 | 5343 |
| 1.5 | 2714 | 494 | 7755 | 7243 | 4731 | 907 | 10,928 | 2904 | 2651 | 10,908 |
| 2.0 | 4708 | 1240 | 11,009 | 11,655 | 8769 | 4109 | 25,812 | 5101 | 4694 | 16,511 |
| 2.5 | 7226 | 3063 | 14,523 | 16,126 | 14,234 | 11,692 | 38,908 | 7996 | 7217 | 22,159 |
| 3.0 | 10,256 | 7557 | 18,252 | 21,116 | 21,198 | 24,995 | 52,253 | 11,536 | 10,344 | 28,372 |
| 3.5 | 14,256 | 16,875 | 21,888 | 26,660 | 29,341 | 43,182 | 67,469 | 17,156 | 14,171 | 35,212 |
| 4.0 | 19,416 | 28,203 | 32,702 | 38,383 | 63,057 | 81,854 | 24,134 | 18,509 | 42,674 | |
| 4.5 | 25,046 | 38,061 | 39,279 | 48,031 | 85,572 | 30,154 | 23,354 | 50,787 | ||
| 5.0 | 31,044 | 48,178 | 46,385 | 58,183 | 116,758 | 36,240 | 28,696 | 58,900 | ||
| 5.5 | 37,795 | 58,952 | 54,095 | 68,942 | 154,890 | 42,953 | 34,533 | |||
| 6.0 | 45,329 | 70,149 | 63,170 | 79,880 | 193,726 | 50,717 | 41,179 | |||
| 6.5 | 53,918 | 81,509 | 91,142 | 236,298 | 59,966 | 48,409 | ||||
| 7.0 | 63,648 | 92,739 | 104,371 | 282,028 | 70,325 | 55,839 | ||||
| 7.5 | 73,691 | 104,079 | 329,333 | 81,108 | 63,686 | |||||
| 8.0 | 83,947 | 115,848 | 376,663 | 92,582 | 71,888 | |||||
| 8.5 | 95,253 | 128,178 | 420,754 | |||||||
| 9.0 | 107,676 | 141,242 | 466,633 | |||||||
| 9.5 | 121,093 | 155,062 | 520,554 | |||||||
| 10.0 | 135,542 | 169,710 | 574,925 | |||||||
| 10.5 | 150,249 | 185,740 | 623,076 | |||||||
| 11.0 | 165,136 | 203,861 | 672,527 | |||||||
| 11.5 | 181,184 | 223,342 | 724,350 | |||||||
| 12.0 | 198,453 | 243,053 | 778,621 | |||||||
| 12.5 | 217,097 | 262,724 | 828,546 | |||||||
| 13.0 | 237,186 | 282,249 | ||||||||
| 13.5 | 258,826 | 300,583 | ||||||||
| 14.0 | 282,091 | |||||||||
| 14.5 | 306,586 | |||||||||
| 15.0 | 332,337 | |||||||||
| 15.5 | 359,873 | |||||||||
| 16.0 | 389,269 | |||||||||
| 16.5 | 420,269 | |||||||||
| 17.0 | 452,920 | |||||||||
| 17.5 | 486,960 | |||||||||
| 18.0 | 522,408 | |||||||||
| 18.5 | 560,528 | |||||||||
| Classification | Error Rate (Measurement Estimation) | Error Rate (Measurement Estimation) | ||
|---|---|---|---|---|
| R1 | 584,400 | 560,528 | 4.08% | 4.26% |
| R2 | 297,392 | 300,583 | −1.07% | −1.06% |
| R3 | 19,969 | 21,888 | 9.61% | −8.77% |
| R4 | 64,733 | 63,170 | 2.41% | 2.47% |
| R5 | 102,040 | 104,371 | −2.28% | −2.23% |
| R6 | 791,556 | 828,546 | −4.67% | −4.46% |
| R7 | 71,632 | 81,854 | −14.27% | −12.49% |
| R8 | 98,851 | 92,582 | 6.34% | 6.77% |
| R9 | 72,050 | 71,888 | 0.22% | 0.23% |
| R10 | 61,628 | 58,900 | 4.43% | 4.63% |
| Classification | Value (Leonard, 1999) | Value (Lehner, 2004) | Value (Takeuchi, 1997) |
|---|---|---|---|
| R1 | 5.70 | 1.45 | 3.65 |
| R2 | 5.38 | 1.08 | 3.05 |
| R3 | 3.76 | 2.09 | 1.30 |
| R4 | 2.00 | 17.87 | 0.86 |
| R5 | 2.78 | 4.23 | 1.36 |
| R6 | 13.18 | 0.61 | 11.32 |
| R7 | 1.75 | 1.85 | 0.95 |
| R8 | 2.60 | 6.86 | 1.17 |
| R9 | 4.29 | 1.69 | 1.71 |
| R10 | 2.19 | 2.11 | 0.98 |
| Classification | Basin Area (km2) | Useful Capacity (1000 m3) | Full Water Area (m2) | Levee Height (m) | Levee Length (m) | Permissible Area (ha) | Area Irrigated (ha) | Frequency of Drought (Year) |
|---|---|---|---|---|---|---|---|---|
| R1 | 1.5 | 480 | 31,070 | 12.5 | 84 | 35 | 28 | 1 |
| R2 | 0.5 | 280 | 22,530 | 6.2 | 172 | 8.7 | 8.7 | 10 |
| R3 | 0.6 | 28.9 | 6300 | 8.5 | 101 | 10 | 6 | 1 |
| R4 | 1.1 | 35.4 | 10,970 | 18 | 170 | 36.6 | 25 | 1 |
| R5 | 1.7 | 82.1 | 15,470 | 12.5 | 130 | 47 | 12 | 10 |
| R6 | 2.2 | 349 | 66,800 | 10 | 207 | 67.9 | 40.6 | 10 |
| R7 | 1.0 | 80 | 20,760 | 6.3 | 180 | 28.1 | 23.1 | 1 |
| R8 | 0.4 | 55.4 | 12,460 | 14.1 | 140 | 14 | 9 | 10 |
| R9 | 0.4 | 58.2 | 9230 | 10.2 | 112 | 10.4 | 8 | 10 |
| R10 | 0.6 | 47.3 | 12,590 | 7.6 | 241 | 42.2 | 15 | 1 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kim, D.; Kim, J.; Wang, W.; Lee, H.; Kim, H.S. On Hypsometric Curve and Morphological Analysis of the Collapsed Irrigation Reservoirs. Water 2022, 14, 907. https://doi.org/10.3390/w14060907
Kim D, Kim J, Wang W, Lee H, Kim HS. On Hypsometric Curve and Morphological Analysis of the Collapsed Irrigation Reservoirs. Water. 2022; 14(6):907. https://doi.org/10.3390/w14060907
Chicago/Turabian StyleKim, Donghyun, Jongsung Kim, Wonjoon Wang, Haneul Lee, and Hung Soo Kim. 2022. "On Hypsometric Curve and Morphological Analysis of the Collapsed Irrigation Reservoirs" Water 14, no. 6: 907. https://doi.org/10.3390/w14060907
APA StyleKim, D., Kim, J., Wang, W., Lee, H., & Kim, H. S. (2022). On Hypsometric Curve and Morphological Analysis of the Collapsed Irrigation Reservoirs. Water, 14(6), 907. https://doi.org/10.3390/w14060907







