Stability Study of a Double-Row Steel Sheet Pile Cofferdam Structure on Soft Ground
Abstract
1. Introduction
2. Project Overview and Scheme Design
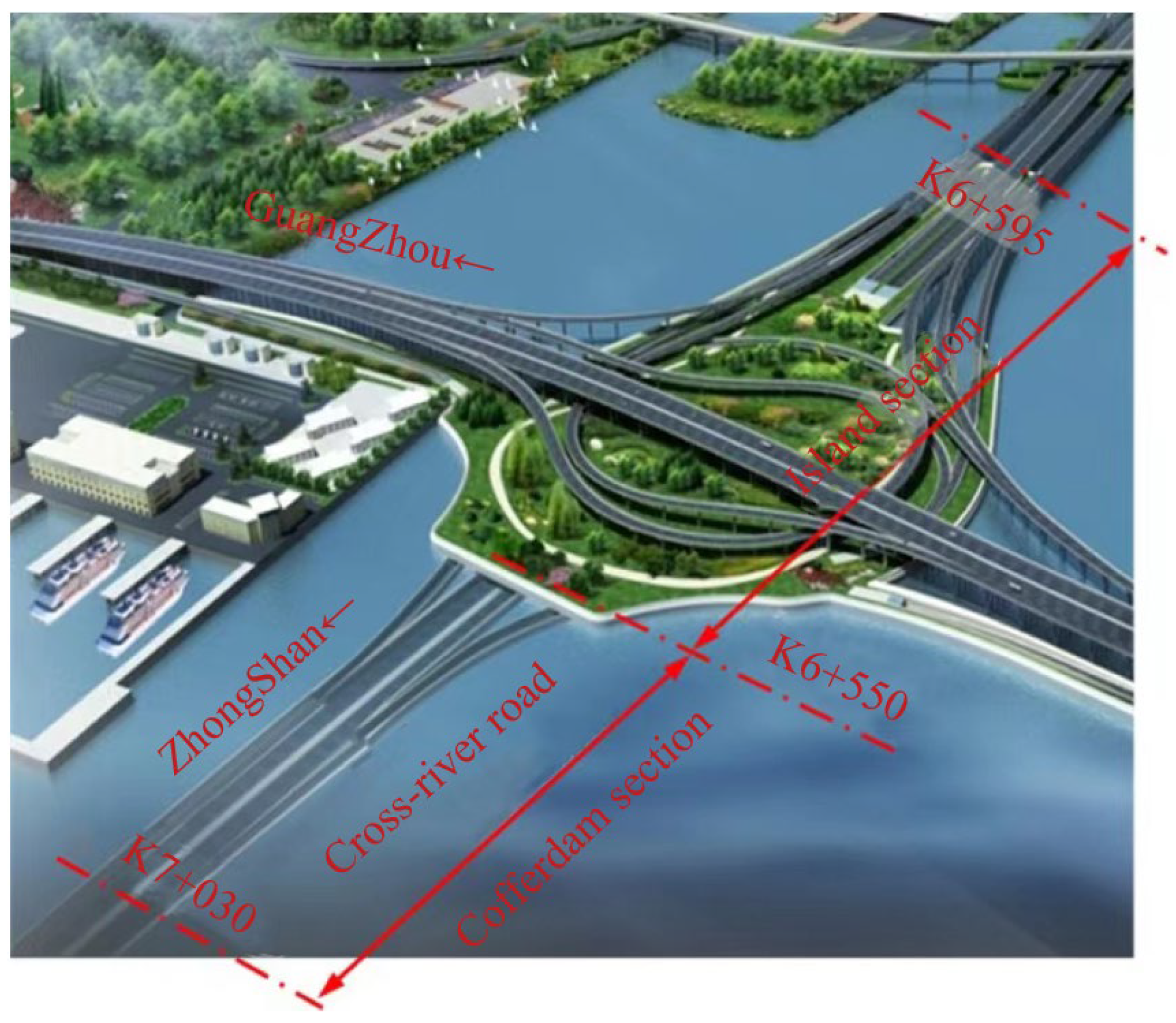
2.1. Project Overview
2.2. Scheme Design
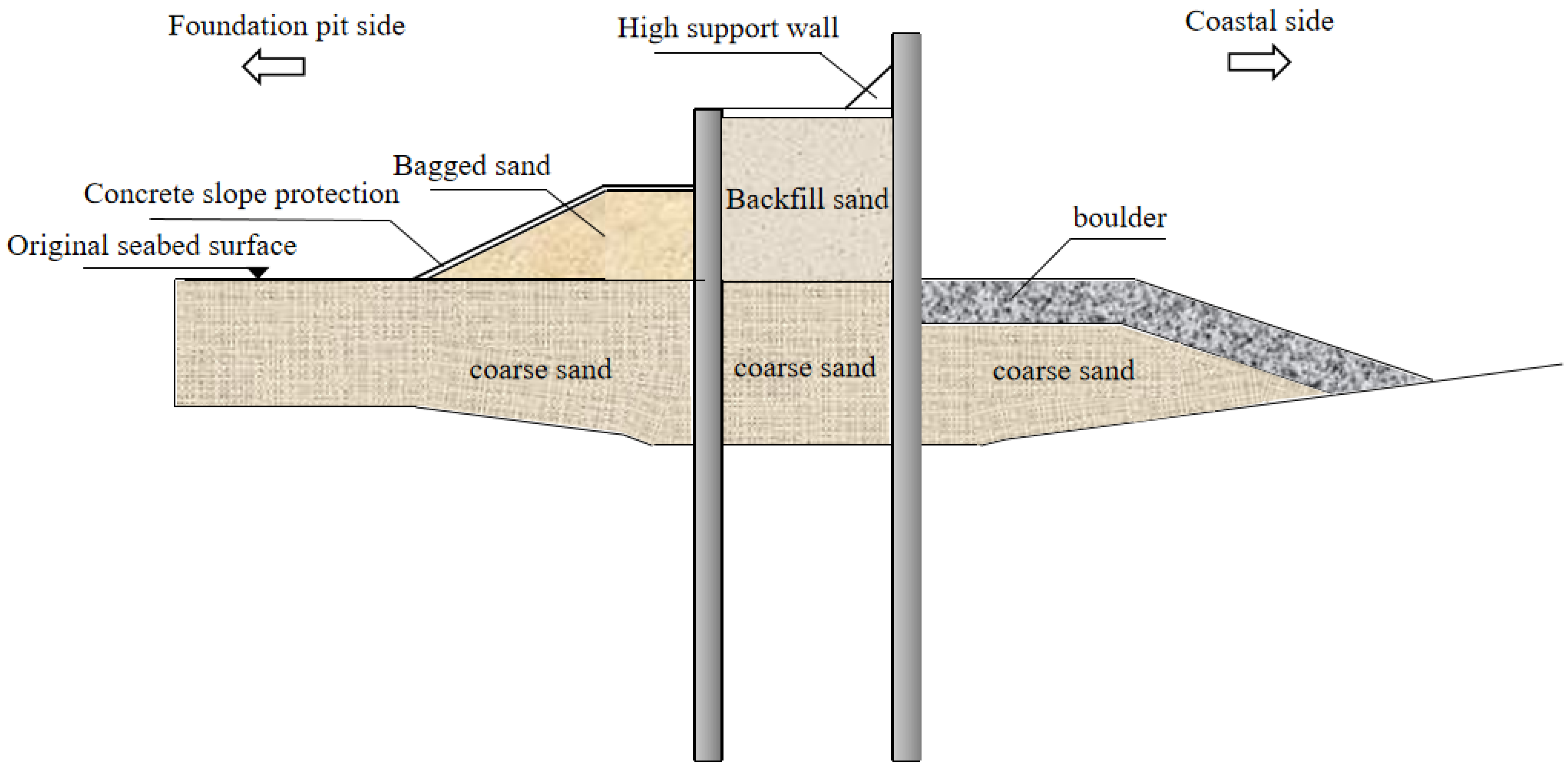
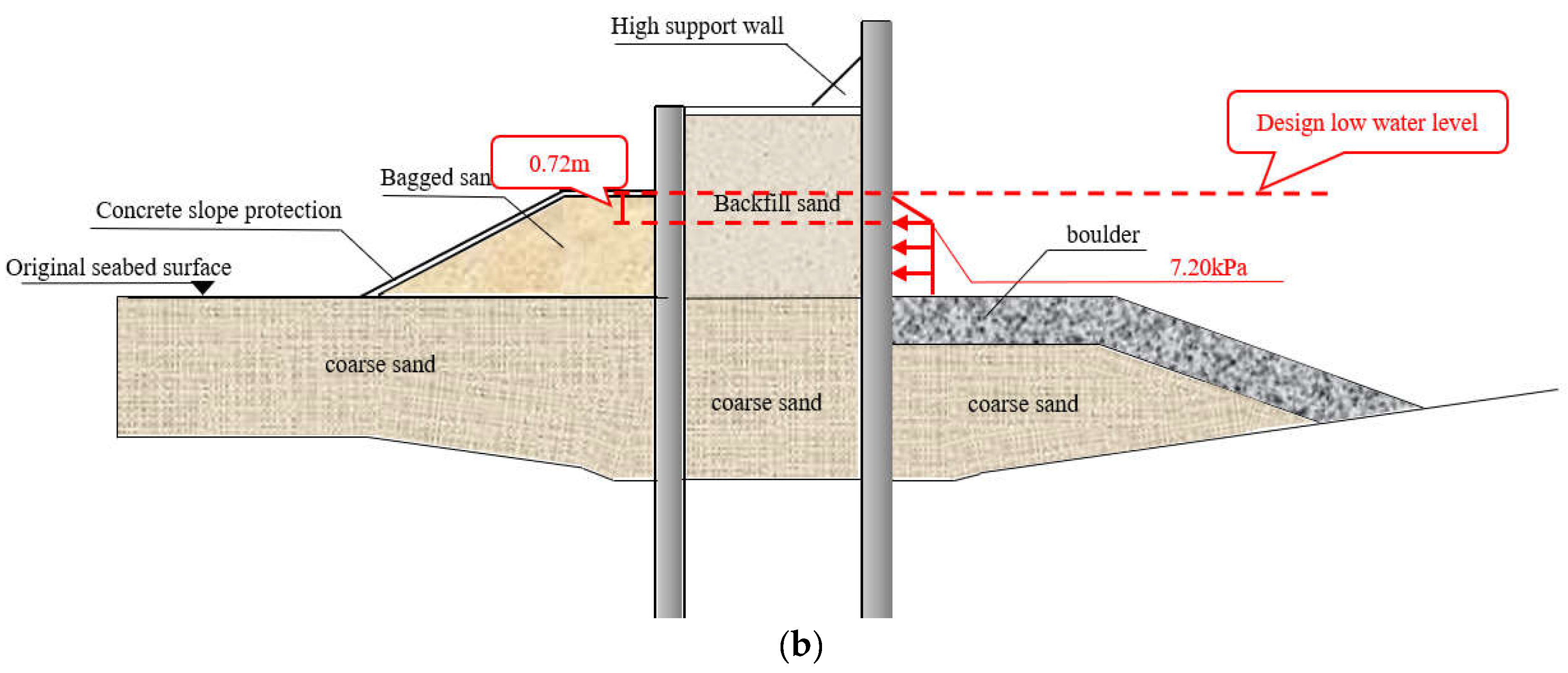
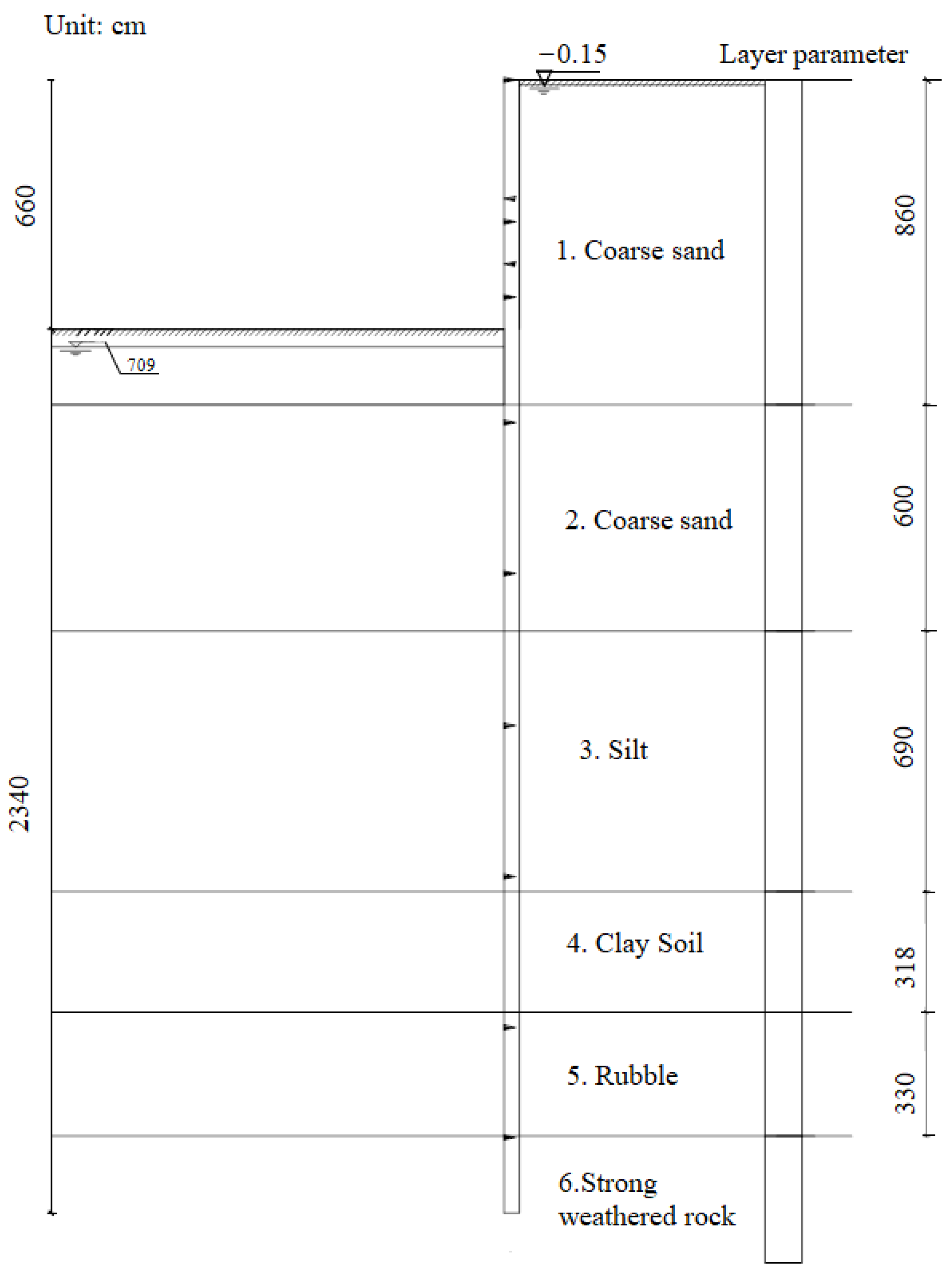
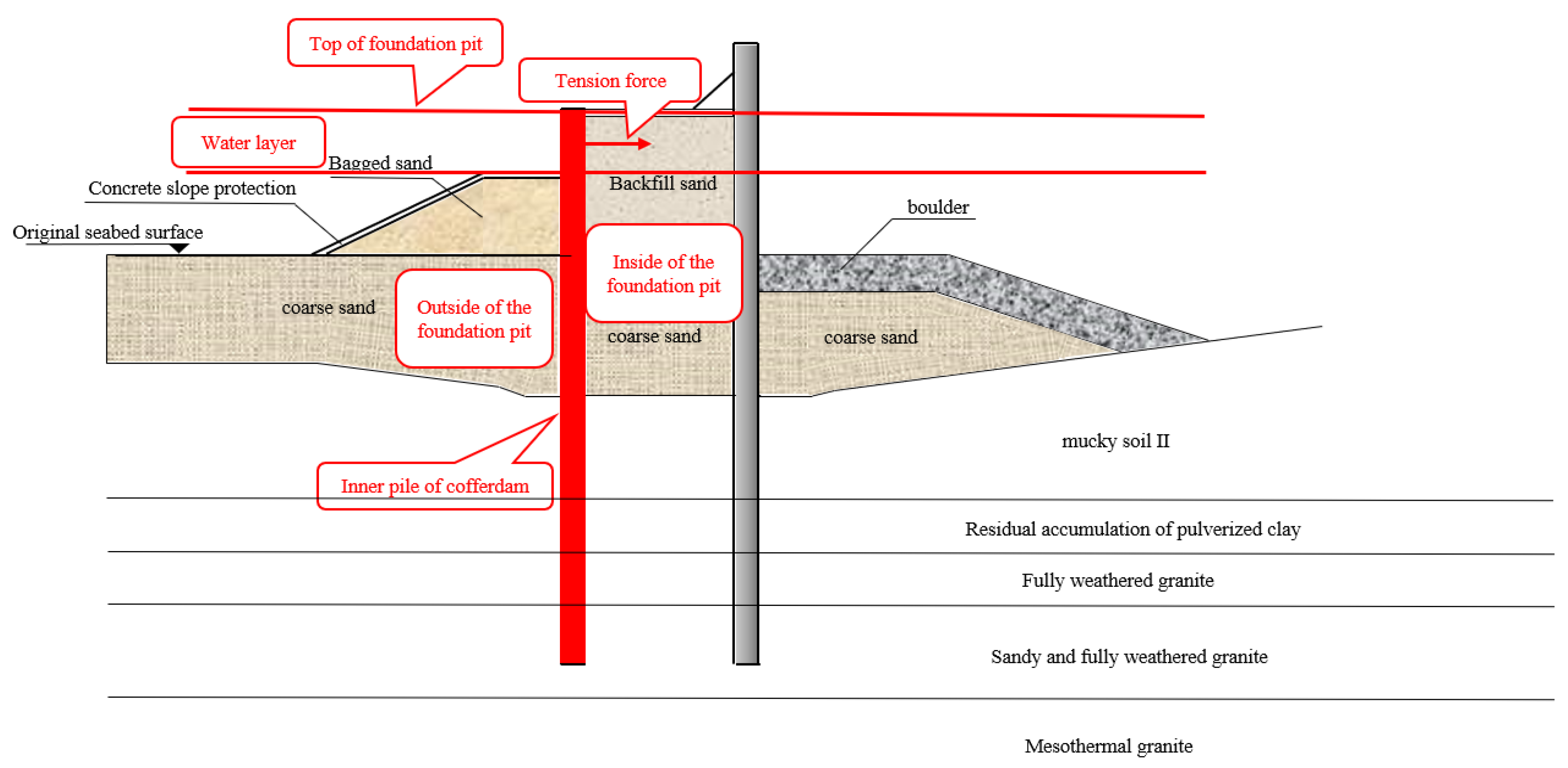
2.2.1. Profile Design
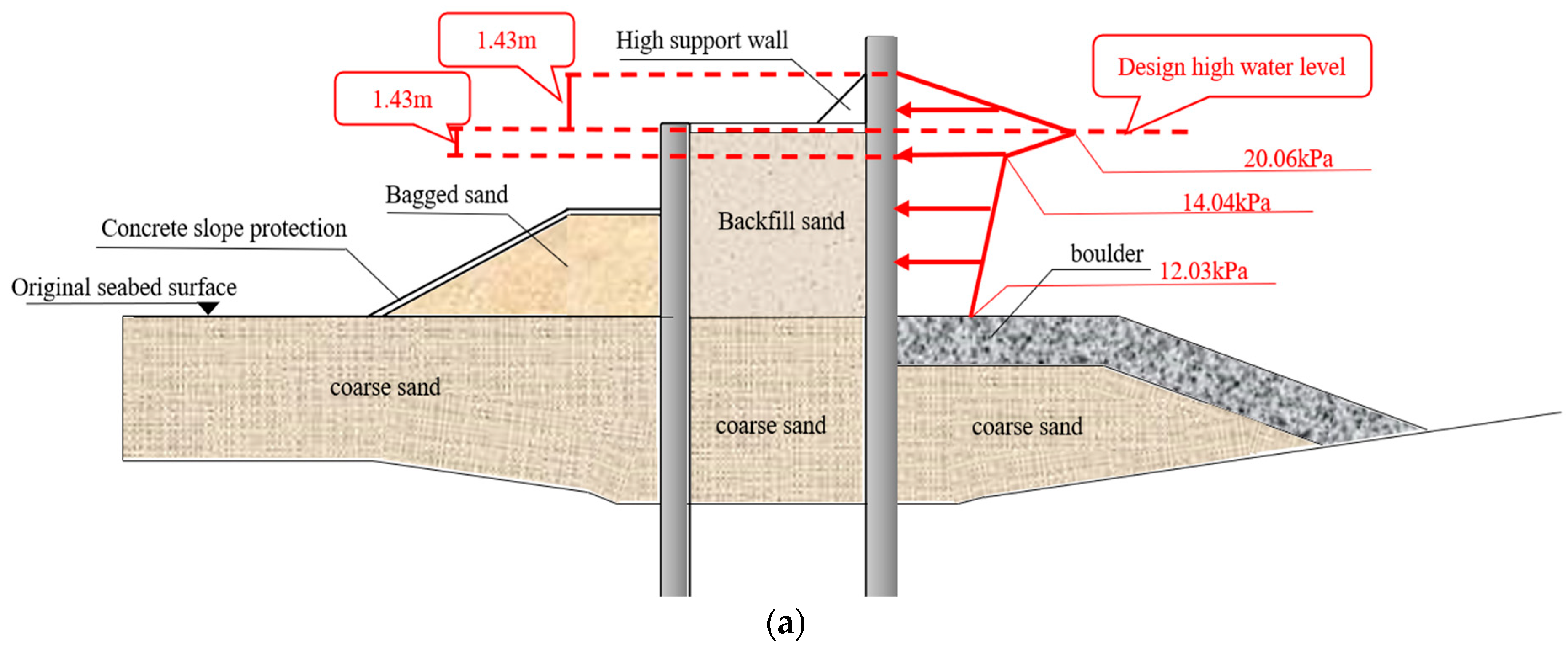
2.2.2. Steel Sheet Pile Section Design and Wave Force Value
3. Structural Stability Analysis
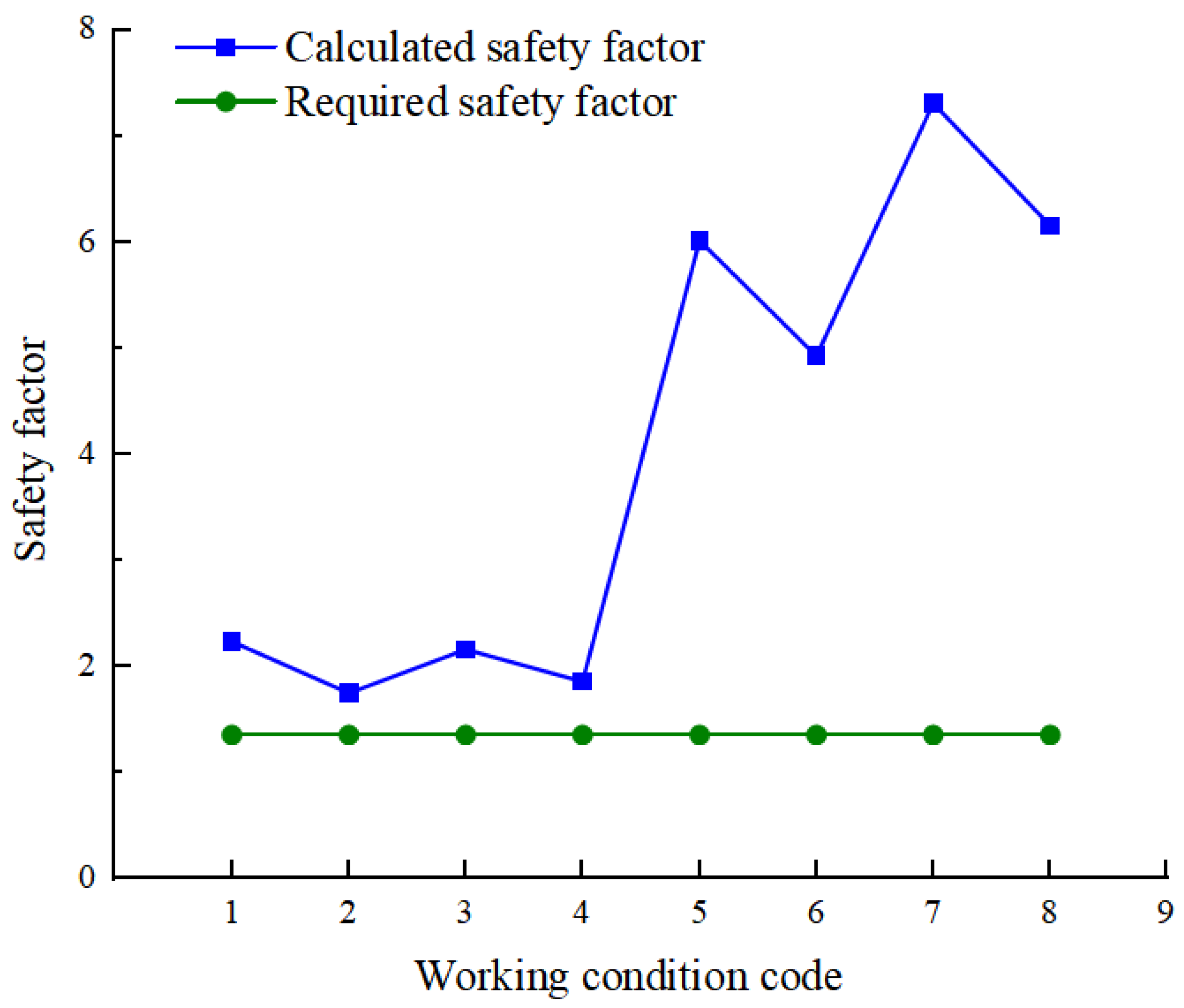
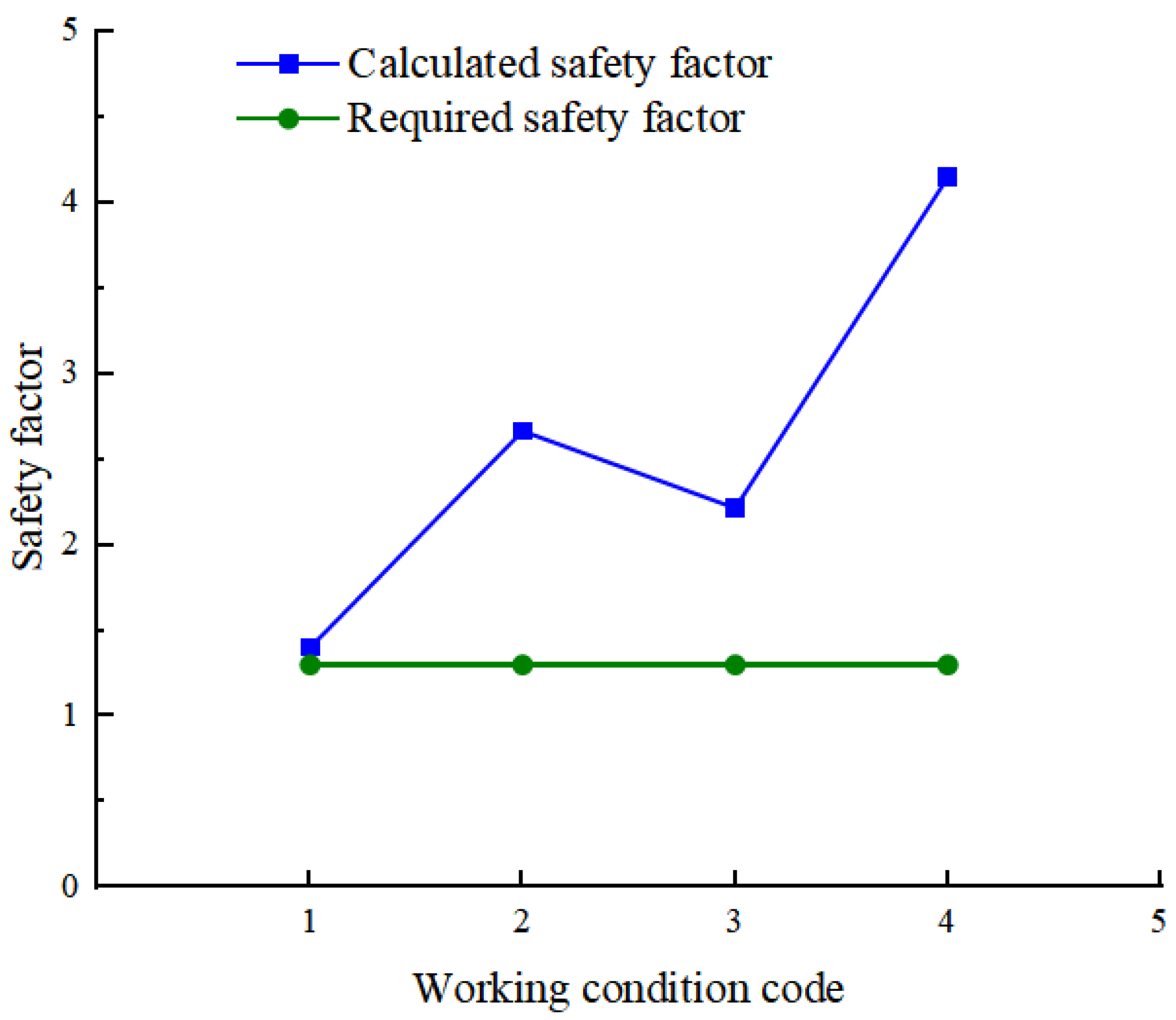
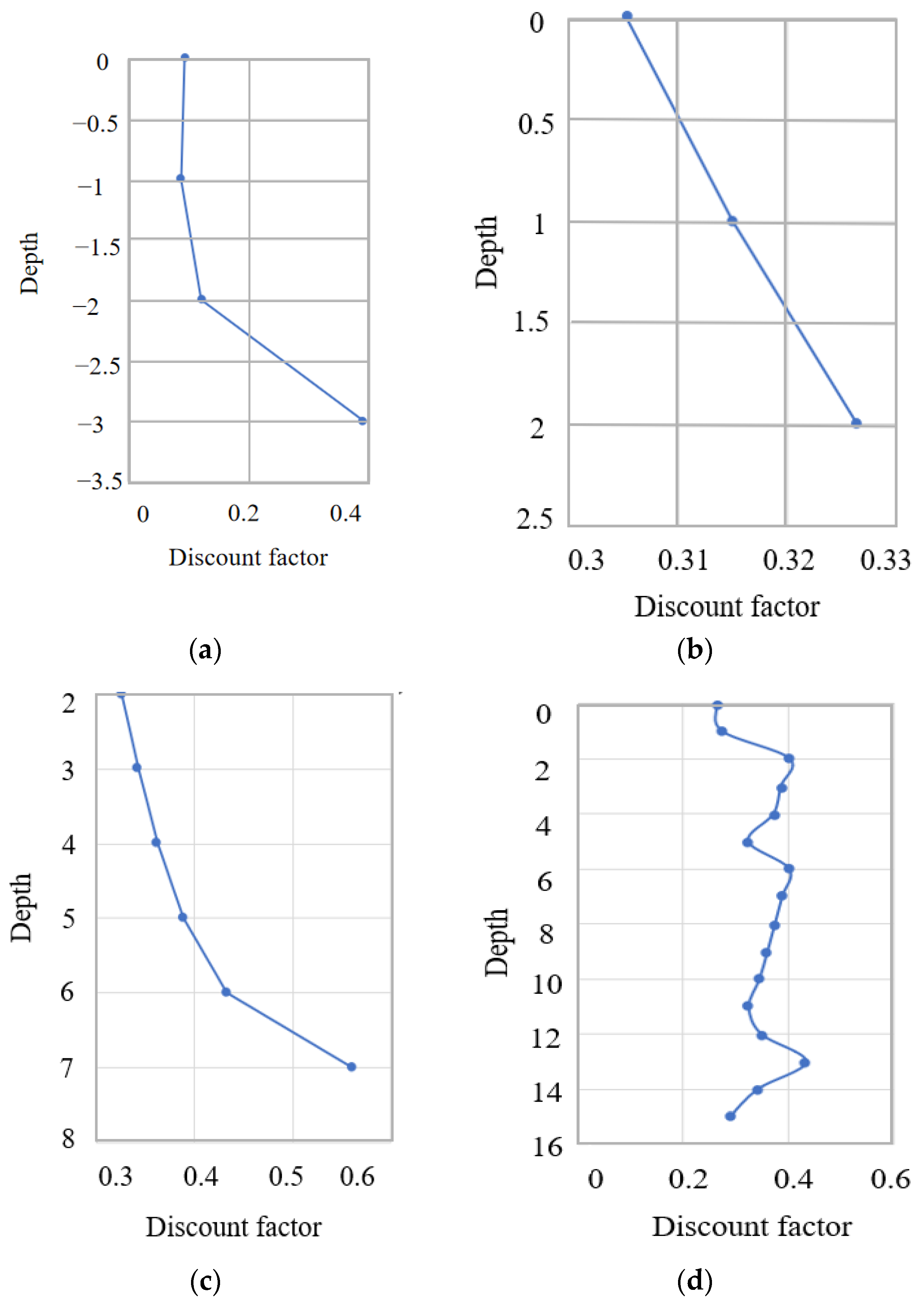
3.1. Overall and Overturning Stability Test
3.1.1. Overall Stability Analysis
3.1.2. Overturning Stability Analysis
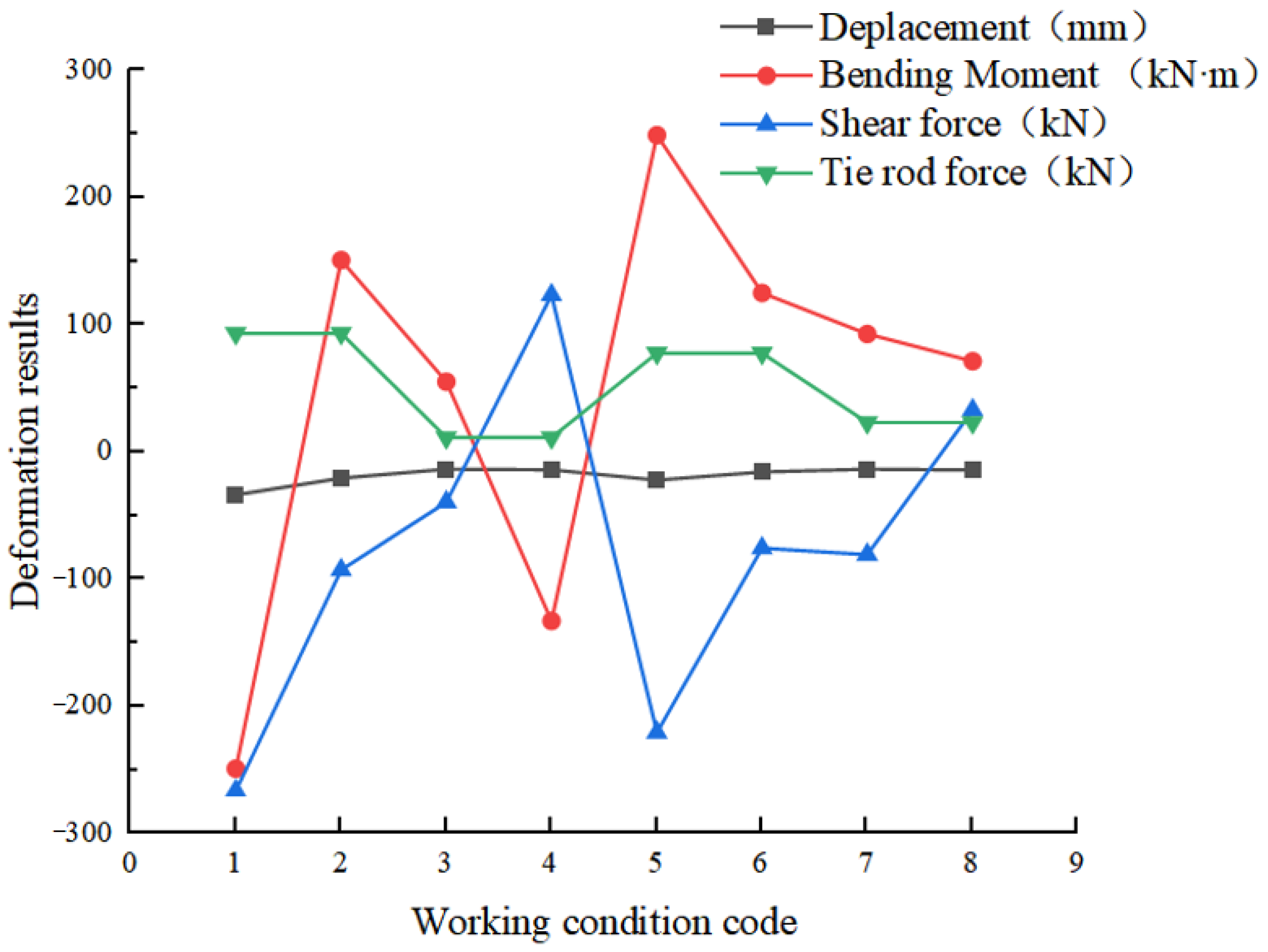
3.2. Internal Force and Displacement Analysis
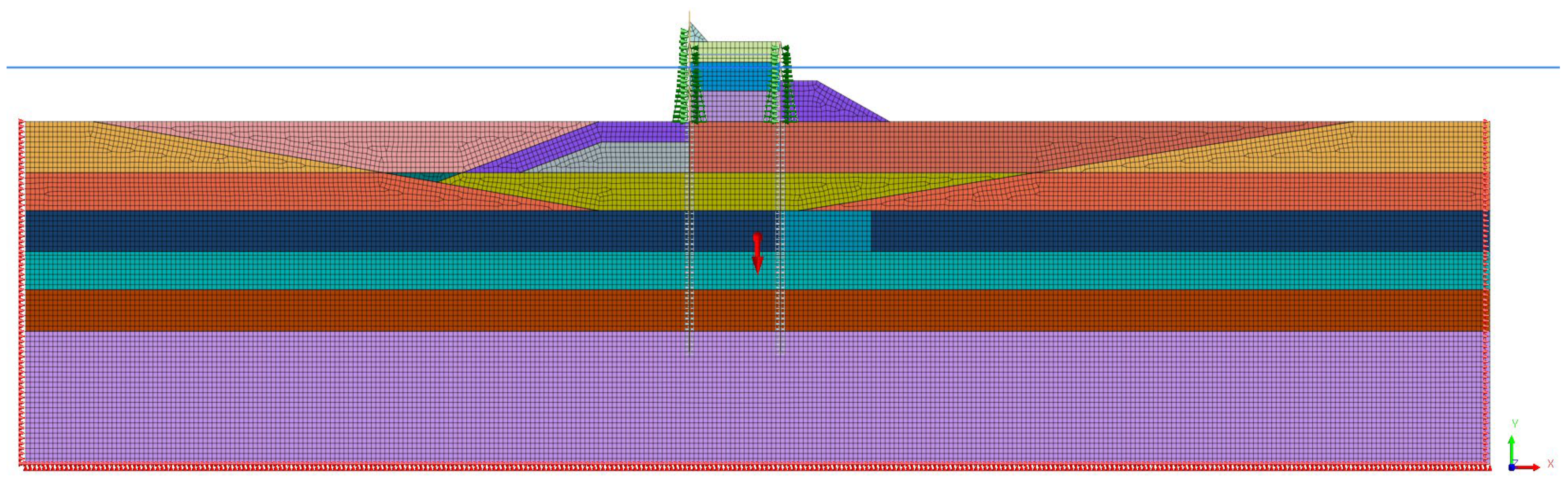
3.3. Planar Finite Element Calculation of Construction Process
4. Conclusions
- (1)
- The calculation results show that the minimum safety factor for all working conditions is 1.744, and the minimum safety factor against overturning for all working conditions is 1.40. Both satisfy the code safety requirement of 1.35.
- (2)
- In the analysis of internal forces and displacements of the cofferdam structure, the calculation results of two typical sections revealed that under the water level drop condition, the maximum displacement on the outside of the right-line section at K6 + 598 is 34 mm. The maximum bending moment is 249.30 kN·m, and the maximum shear force is 266.66 kN. The displacements of the steel sheet pile inside and outside the cofferdam under each condition are minimal, and the internal forces are below the design sheet pile type bearing capacity. Therefore, the cofferdam structure is considered to be safe.
- (3)
- The influence of construction procedures on the internal force deformation of the sheet pile is found to be negligible, according to the calculation results for two typical sections at different steps in the construction process and under different water level conditions.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Yang, Y.; Liu, W.; Hu, A.; Li, F.; Yang, Z. Application of Steel Sheet Pile in Deep Foundation Pit Support of Collapsible Loess Regions. In IOP Conference Series: Earth and Environmental Science; IOP Publishing: Philadelphia, PA, USA, 2020; Volume 474, No. 7. [Google Scholar] [CrossRef]
- Wu, S.; Hao, W.; Yao, Y.; Li, D. Investigation into durability degradation and fracture of cable bolts through laboratorial tests and hydroge-ochemical modelling in underground conditions. Tunn. Undergr. Space Technol. 2023, 138, 105198. [Google Scholar] [CrossRef]
- Zhu, Y.; Li, X.J.; Shi, Z.M.; Peng, M.; Xuan, L.J.; Gao, J.Y.; Cai, S. Dynamic Behavior of Double Steel Sheet Pile Cofferdam under Different Wave Actions. In IOP Conference Series: Earth and Environmental Science; IOP Publishing: Philadelphia, PA, USA, 2021; Volume 861, No. 7. [Google Scholar] [CrossRef]
- Hou, Y.-M.; Wang, J.-H.; Gu, Q.-Y. Deformation performance of double steel sheet piles cofferdam. J. Shanghai Jiaotong Univ. 2009, 43, 1577. [Google Scholar]
- Yan, Z.; Qian-Yan, G.; Jie, J.; Ming, P. Reliability analysis for overall stability of large-span double-row steel sheet-piled dock cofferdam based on Bayesian method. Rock Soil Mech. 2016, 37, 609–615. [Google Scholar]
- Mitobe, Y.; Adityawan, M.B.; Roh, M.; Tanaka, H.; Otsushi, K.; Kurosawa, T. Experimental study on embankment reinforcement by steel sheet pile structure against tsunami overflow. Coast. Eng. J. 2016, 58, 1–18. [Google Scholar] [CrossRef]
- Shen, Y.; Yu, Y.; Ma, F.; Mi, F.; Xiang, Z. Earth pressure evolution of the double-row long-short stabilizing pile system. Environ. Earth Sci. 2017, 76, 1–11. [Google Scholar] [CrossRef]
- Khan, M.R.A.; Takemura, J.; Kusakabe, O. Behavior of double sheet pile wall cofferdam on sand observed in centrifuge tests. Int. J. Phys. Model. Geotech. 2001, 1, 1–16. [Google Scholar] [CrossRef]
- Zhou, Y.; Yao, A.; Li, H.; Zheng, X. Correction of Earth Pressure and Analysis of Deformation for Double-Row Piles in Foundation Excavation in Changchun of China. Adv. Mater. Sci. Eng. 2016, 19, 167–180. [Google Scholar] [CrossRef]
- Tschebotarioff, G.P. Lateral behavior of a double sheet pile wall structure. Soils Found. 1974, 14, 92–93. [Google Scholar]
- De Buhan, P.; Corfdir, A. Limit Design of Axisymmetric Shells with Application to Cellular Cofferdams. J. Eng. Mech. 1996, 122, 921–929. [Google Scholar] [CrossRef]
- Banerjee, S.; Banerjee, M.S.; Member, A. Design Charts for Double-Walled Cofferdams. J. Geotech. Eng. 1993, 119, 214–222. [Google Scholar] [CrossRef]
- Lei, H.; Liu, X.; Song, Y.; Xu, Y. Stability analysis of slope reinforced by double-row stabilizing piles with different locations. Nat. Hazards 2021, 106, 19–42. [Google Scholar] [CrossRef]
- Li, C.; Chen, W.; Song, Y.; Gong, W.; Zhao, Q. Optimal Location of Piles in Stabilizing Slopes Based on a Simplified Double-Row Piles Model. KSCE J. Civ. Eng. 2020, 24, 377–389. [Google Scholar] [CrossRef]
- Zhang, M.-Y.; Ma, J.-X.; Yang, S.-J.; Wang, Y.-H.; Bai, X.-Y.; Sun, S.-X. Experimental Study on Bending Moment of Double-Row Steel Pipe Piles in Foundation Excavation. Adv. Civ. Eng. 2020, 2020, 1–8. [Google Scholar] [CrossRef]
- Zhou, Y.; Luo, L.; Zheng, L. Review of the Deformation Mechanism and Earth Pressure Research on the Double-Row Pile Support Structure. Front. Earth Sci. 2022, 10, 933840. [Google Scholar] [CrossRef]
- Lefas, I.; Georgiannou, V. Analysis of a cofferdam support and design implications. Comput. Struct. 2001, 79, 2461–2469. [Google Scholar] [CrossRef]
- Byfield, M.P.; Crawford, R.J. Oblique bending in U-shaped steel sheet piles. Proc. Inst. Civ. Eng.-Struct. Build. 2003, 156, 255–261. [Google Scholar] [CrossRef]
- Wu, S.; Zhang, Z.; Chen, J.; Yao, Y.; Li, D. Characterisation of stress corrosion durability and time-dependent performance of cable bolts in under-ground mine environments. Eng. Fail. Anal. 2023, 150, 107292. [Google Scholar] [CrossRef]
- Gui, M.-W.; Han, K.-K. An investigation on a failed double-wall cofferdam during construction. Eng. Fail. Anal. 2009, 16, 421–432. [Google Scholar] [CrossRef]
- Zhao, T.; Ding, W.; Wei, L.; Wu, W.; Qiao, Y. The behavior analysis of a cofferdam constructed by double sheet pile wall above muck. In Proceedings of the GeoShanghai 2018 International Conference: Tunnelling and Underground Construction, Shanghai, China, 27–30 May 2018; Springer: Singapore, 2018. [Google Scholar]
- Xue, R.; Bie, S.; Guo, L.; Zhang, P. Stability Analysis for Cofferdams of Pile Wall Frame Structures. KSCE J. Civ. Eng. 2019, 23, 4010–4021. [Google Scholar] [CrossRef]
- Sulovska, M.; Stacho, J.; Kopecky, M. The Stability Analysis of a Cofferdam Using the Numerical Modelling. In IOP Conference Series: Materials Science and Engineering; IOP Publishing: Philadelphia, PA, USA, 2020; Volume 960, No. 2. [Google Scholar]
- Fujiwara, K.; Taenaka, S.; Otsushi, K.; Yashima, A.; Sawada, K.; Ogawa, T.; Takeda, K. Study on levee reinforcement using double sheet-piles with partition walls. Jpn. Geotech. Soc. Spec. Publ. 2017, 5, 11–15. [Google Scholar] [CrossRef]
- Wang, K.; Gao, Y.; Jin, Z.; Zhou, X.; Chen, L.; Zhang, C. Research on stability of steep bank slope and reserved thin-walled rock cofferdam during excavation of intake foundation pit. Eng. Fail. Anal. 2022, 141, 106659. [Google Scholar] [CrossRef]
- Xu, F.; Li, S.-C.; Zhang, Q.-Q.; Li, L.-P.; Wang, K.; Liu, H.-L. Analysis and Design Implications on Stability of Cofferdam Subjected to Water Wave Action. Mar. Georesources Geotechnol. 2014, 34, 181–187. [Google Scholar] [CrossRef]
- Hui, S. Research on the Bearing Capacity of Double Row Steel Sheet Pile Reinforcement Depth of the Based on Soil between Piles. Int. J. Eng. Res. Afr. 2016, 25, 127–132. [Google Scholar] [CrossRef]
- Chen, L.; Jeng, D.-S. Study on the seabed response around a dumbbell cofferdam under combined wave and current loading. Ocean Eng. 2022, 256, 111456. [Google Scholar] [CrossRef]
- Hu, Y.; Huang, J.; Tao, Z.; Zhu, J.; Lv, Q.; Qiao, J.; Cai, W. The Influence of Water-Level Fluctuation on the Instability and Seepage Failure of Dump-Fill Cofferdam. Adv. Civ. Eng. 2022, 2022, 5845340. [Google Scholar] [CrossRef]
- Ti, Z.; Wei, K.; Qin, S.; Mei, D.; Li, Y. Assessment of random wave pressure on the construction cofferdam for sea-crossing bridges under tropical cyclone. Ocean Eng. 2018, 160, 335–345. [Google Scholar] [CrossRef]
- Wang, J.; Jiang, Z.; Li, F.; Chen, W. The prediction of water level based on support vector machine under construction condition of steel sheet pile cofferdam. Concurr. Comput. Pract. Exp. 2020, 33, e6003. [Google Scholar] [CrossRef]
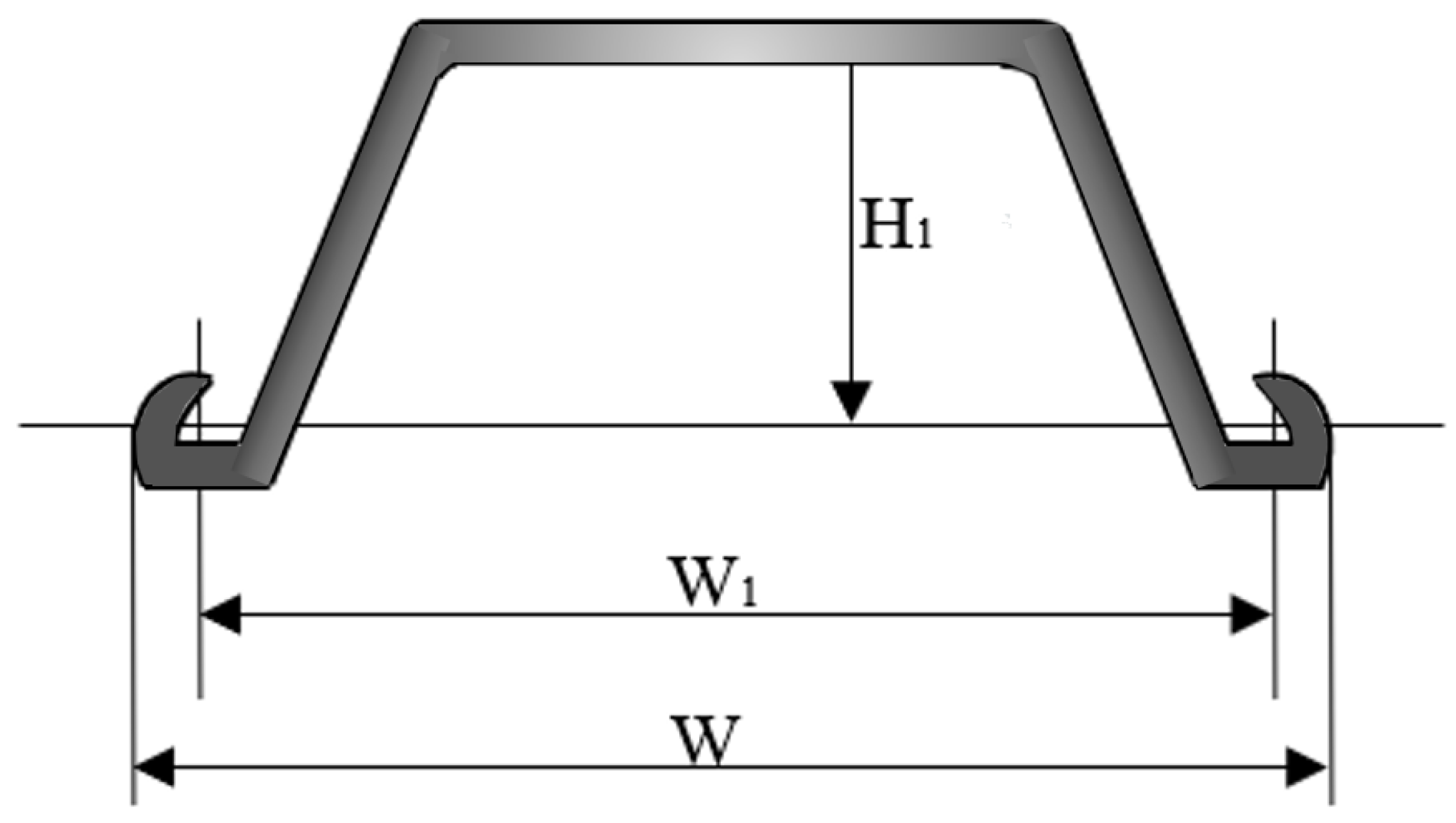



| Model W × H × Tw | Effective Width W1 (mm) | Effective Height H1 (mm) | Board Thickness t (mm) | Cross-Sectional Area per Meter of Sheet (cm2) | Theoretical Weight per Meter of Sheet (kg/m) |
|---|---|---|---|---|---|
| 750 × 225 × 14.5 | 750 | 225 | 14.5 | 188 | 147.2 |
| Steel Type | Design Value of Flexural Strength (MPa) | Design Value of Shear Strength (MPa) | Modulus of Elasticity (MPa) | Other (mm) |
|---|---|---|---|---|
| Q390BZ | 350 | 205 | 2.06 × 105 | Thickness ≤ 16 |
| Q345B | 295 | 170 | 2.06 × 105 | Thickness ≤ 16 |
| Name | Natural Density (g/cm3) | Compression Modulus (MPa) | Poisson’s Ratio | Cohesive Force (kPa) | Internal Friction Angle (°) |
|---|---|---|---|---|---|
| Sludge Ⅰ | 1.49 | 1.53 | 0.4 | 3.1 | 2.0 |
| Sludge Ⅱ | 1.52 | 1.41 | 0.4 | 2.8 | 1.8 |
| Clay | 1.97 | 6.29 | 0.3 | 32.2 | 8.7 |
| Residual silty clay | 1.87 | 9.6 | 0.3 | 15.1 | 25.1 |
| Completely weathered granite | 1.89 | 18 | 0.2 | 18.5 | 27.4 |
| Sandy strongly weathered granite | 1.93 | 26 | 0.2 | 27.5 | 28.6 |
| Fragmented strongly weathered granite | 2.55 | / | 0.2 | 3000 | 30 |
| Moderately weathered granite | 2.62 | 26.2 | 0.2 | 15,000 | 41 |
| Riprap filling | 2.1 | / | 0.2 | 0 | 38 |
| Backfilling with medium coarse sand | 1.9 | / | 0.3 | 0 | 35 |
| Bagging sand | 1.9 | / | 0.3 | 12.3 | 25.96 |
| Mixing pile | 1.8 | / | 0.3 | 20 | 20 |
| Concrete (retaining walls, support beams) | 2.4 | / | 0.15 | / | / |
| Steel (steel tie rods, steel sheet pile cofferdams, steel supports) | 78.5 | / | 0.3 | / | / |
| Attribute | Material Name | Elastic Modulus (MPa) | Poisson’s Ratio | Constitutive | Cohesive Force (kPa) | Internal Friction Angle (°) |
|---|---|---|---|---|---|---|
| Plane Strain (2D) | Sludge Ⅰ | 4.6 | 0.4 | Mohr–Coulomb | 3.1 | 2.0 |
| Plane Strain (2D) | Sludge Ⅱ | 4.2 | 0.4 | Mohr–Coulomb | 2.8 | 1.8 |
| Plane Strain (2D) | Clay | 20 | 0.3 | Mohr–Coulomb | 32.2 | 8.7 |
| Plane Strain (2D) | Residual silty clay | 20 | 0.3 | Mohr–Coulomb | 15.1 | 25.1 |
| Plane Strain (2D) | Completely weathered granite | 50 | 0.2 | Mohr–Coulomb | 18.5 | 27.4 |
| Plane Strain (2D) | Sandy strongly weathered granite | 90 | 0.2 | Mohr–Coulomb | 27.5 | 28.6 |
| Plane Strain (2D) | Fragmented strongly weathered granite | 200 | 0.2 | Mohr–Coulomb | 3000 | 30 |
| Plane Strain (2D) | Moderately weathered granite | 500 | 0.2 | Mohr–Coulomb | 15,000 | 41 |
| Plane Strain (2D) | Riprap filling | 100 | 0.2 | Mohr–Coulomb | 0 | 38 |
| Plane Strain (2D) | Backfilling with medium coarse sand | 30 | 0.3 | Mohr–Coulomb | 0 | 35 |
| Plane Strain (2D) | Bagging sand | 30 | 0.3 | Mohr–Coulomb | 12.3 | 25.96 |
| Plane Strain (2D) | Mixing pile | 30 | 0.3 | Mohr–Coulomb | 20 | 20 |
| Plane Strain (2D) | Concrete (retaining walls, support beams) | 30,000 | 0.15 | Linear Elasticity | / | / |
| Beam/Truss (1D) | Steel (steel tie rods, steel sheet pile cofferdams, steel supports) | 200,000 | 0.3 | Linear Elasticity | / | / |
| Number | Construction Step |
|---|---|
| 1 | Calculation of initial crustal stress |
| 2 | Excavation of foundation trench |
| 3 | Overall replacement of medium-coarse sand to the original seabed surface |
| 4 | Driving inner and outer steel sheet piles |
| 5 | Outer row steel sheet pile outer sand rib soft row, bagged gravel, and bagged soil for roof protection |
| 6 | Install steel tie rods |
| 7 | Synchronous layered backfilling of medium to coarse sand in the weir body to an elevation of −2.0~+1.0 m |
| 8 | Backfilling inside the weir to an elevation of +1.0 m |
| 9 | Continue backfilling the dam body to an elevation of +3.0 m |
| 10 | Construction anti-pressure soil slope, inner soft soil reinforcement |
| 10 (a) | The water level inside the cofferdam is constant, while the water level outside the cofferdam drops sharply by 2.6 m + 10-year wave suction |
| 10 (b) | Design a high tide level on the inner side of the cofferdam, with a sudden drop in water level of 2.6 m and a 10-year wave suction force on the outer side of the cofferdam |
| 11 | The water level on the inner side of the cofferdam drops, the anti-pressure soil slope is protected by concrete bags, and the top of the cofferdam is supported by a retaining wall |
| 11 (a) | Considering 10-year high water level and 10-year wave thrust |
| 11 (b) | Considering 10-year low water level and 10-year wave suction |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jiang, Y.; Guo, F.; Wang, W.; Yang, G.; Yue, J.; Huang, Y. Stability Study of a Double-Row Steel Sheet Pile Cofferdam Structure on Soft Ground. Water 2023, 15, 2643. https://doi.org/10.3390/w15142643
Jiang Y, Guo F, Wang W, Yang G, Yue J, Huang Y. Stability Study of a Double-Row Steel Sheet Pile Cofferdam Structure on Soft Ground. Water. 2023; 15(14):2643. https://doi.org/10.3390/w15142643
Chicago/Turabian StyleJiang, Yan, Fei Guo, Wenlong Wang, Guanghua Yang, Jinchao Yue, and Yibin Huang. 2023. "Stability Study of a Double-Row Steel Sheet Pile Cofferdam Structure on Soft Ground" Water 15, no. 14: 2643. https://doi.org/10.3390/w15142643
APA StyleJiang, Y., Guo, F., Wang, W., Yang, G., Yue, J., & Huang, Y. (2023). Stability Study of a Double-Row Steel Sheet Pile Cofferdam Structure on Soft Ground. Water, 15(14), 2643. https://doi.org/10.3390/w15142643







