Efficient and Low-Cost Water Remediation for Chitosan Derived from Shrimp Waste, an Ecofriendly Material: Kinetics Modeling, Response Surface Methodology Optimization, and Mechanism
Abstract
:1. Introduction
2. Materials and Methods
2.1. Reagent (Adsorbate)
2.2. Preparation of Adsorbent (Chitosan)
2.2.1. Demineralization
2.2.2. Deproteination and Deacetylation
2.3. Characterization of Prepared Chitosan
2.3.1. X-ray Diffraction Analysis (XRD)
2.3.2. ATR Spectroscopy Analysis
2.3.3. pH Zero-Charge Point (pHpzc)
2.4. Adsorption Experiments
2.4.1. Preparation of Dye Solution and Quantification
2.4.2. Batch Kinetics and Equilibrium Studies
2.4.3. Adsorption Tests for Optimization and Modeling
2.5. Response Surface Methodology
2.5.1. Design of Experiment Using Central Composite Design (CCD)
- −
- The vertices of the domain are defined from the combinations of the −1 and +1 levels of the factors. These points represent the experimental treatments of a complete factorial design. When the number of factors becomes important (k > 4), it is possible to perform only a regular or irregular fraction of this set of vertices so that the number of experiments does not increase rapidly. For k = 5 to 7 factors, we realize 2k−1 vertices; for k = 8 and 9 factors, 2k−2 vertices are realized [41].
- −
- The star points are located on each of the axes (two points per axis). The objective here is to estimate the curvatures of the response surface. They are located at a distance α to be determined:
- − The center of the domain (0,0) is subject to repetitions, the number of which is noted n0. In this case, the following relation gives the number of experiments in a centered composite design:
2.5.2. Statistical Background
2.5.3. Analysis of Variance (ANOVA)
2.5.4. Mathematical Modeling
2.5.5. RSM Evolution
2.5.6. Optimization by Desirability of a Response to Be Maximized
3. Results and Discussion
3.1. Characterization of Prepared Chitosan
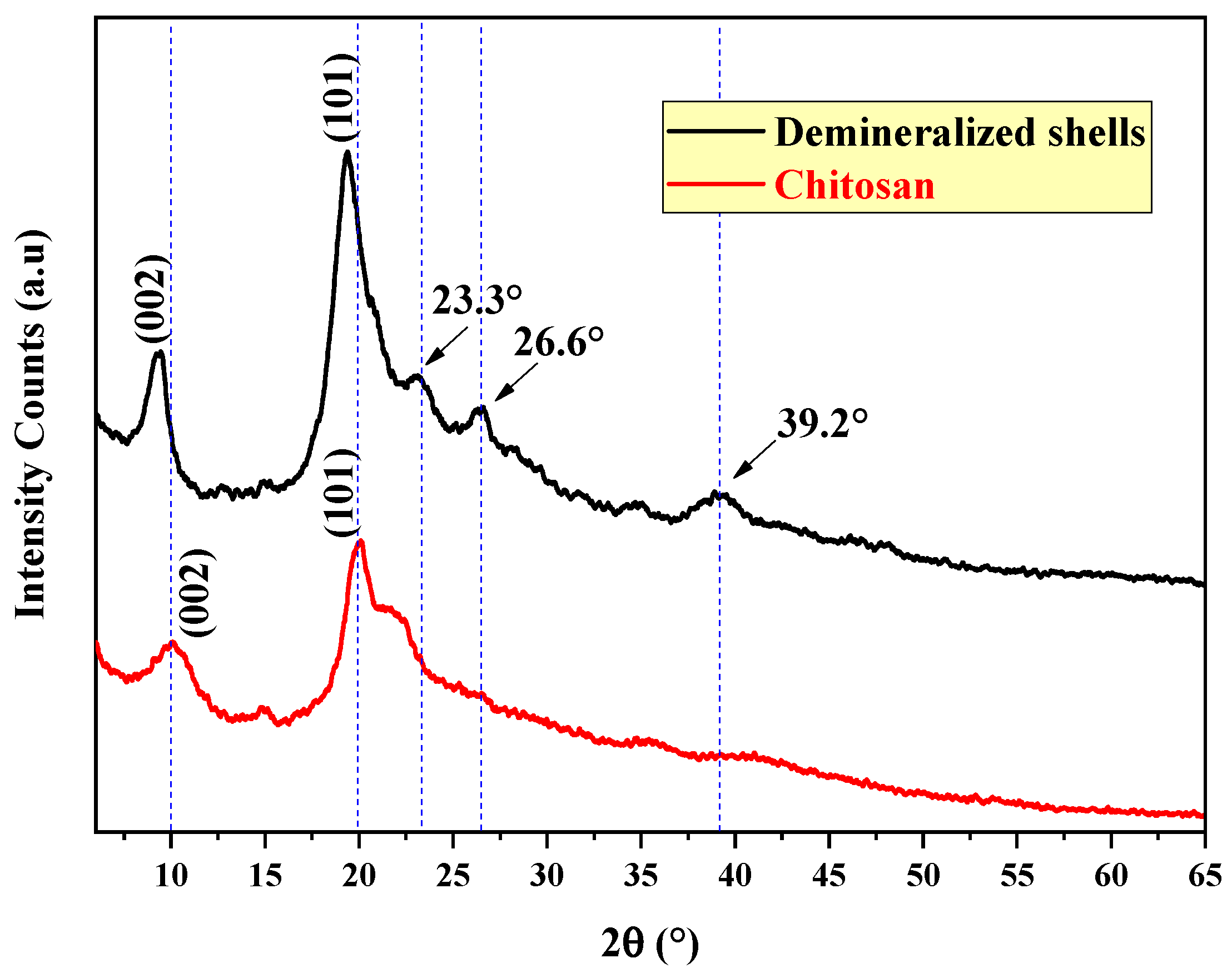
3.1.1. Characterization of Prepared Chitosan via XRD
3.1.2. Characterization of Prepared Chitosan via IR
3.1.3. Point of Zero Charge (pHpzc)
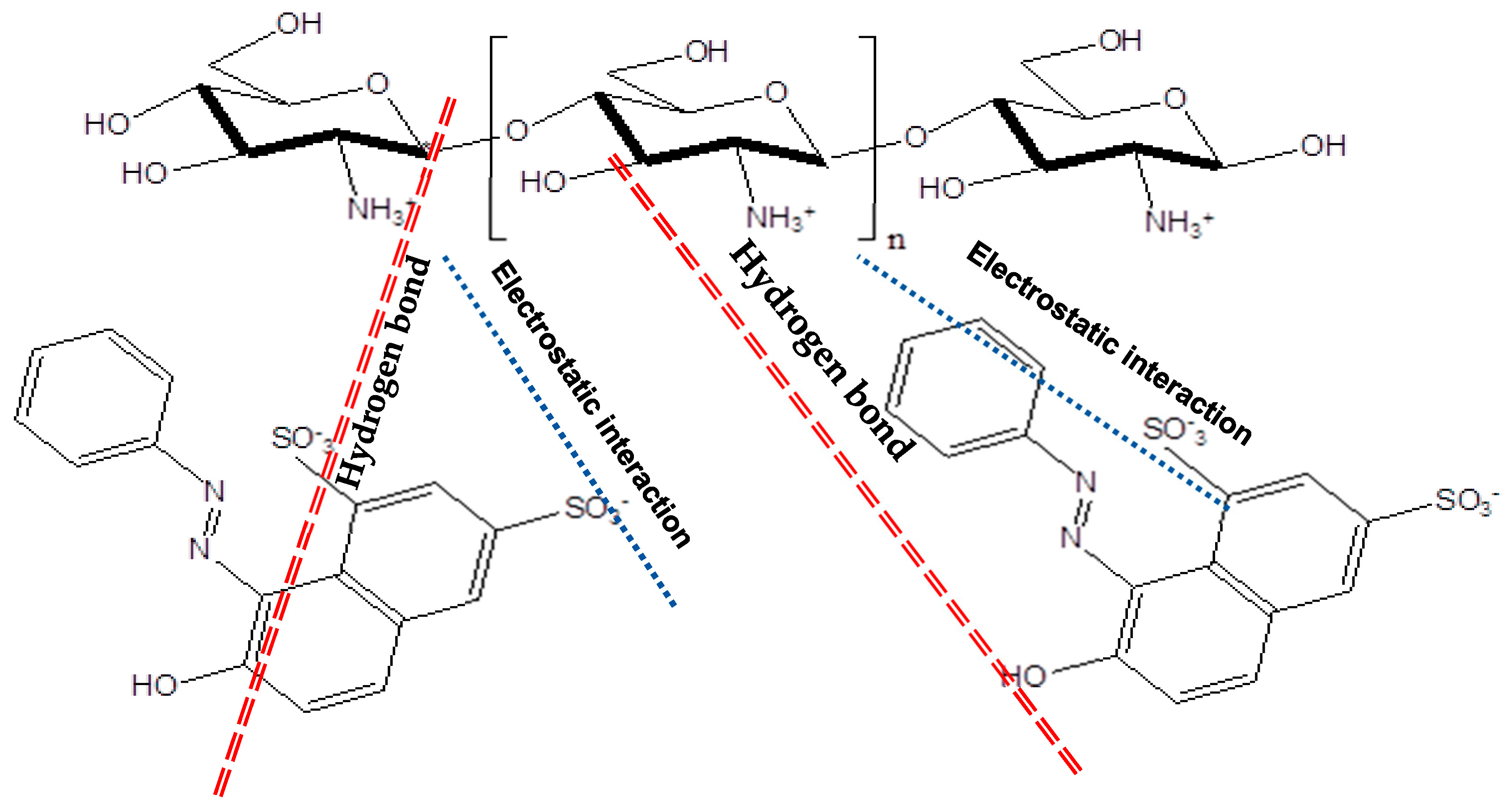
3.2. Adsorption Studies
3.2.1. Equilibrium Studies
3.2.2. Effect of Key Parameters on the OG Adsorption
Effect of OG Concentration and Contact Time
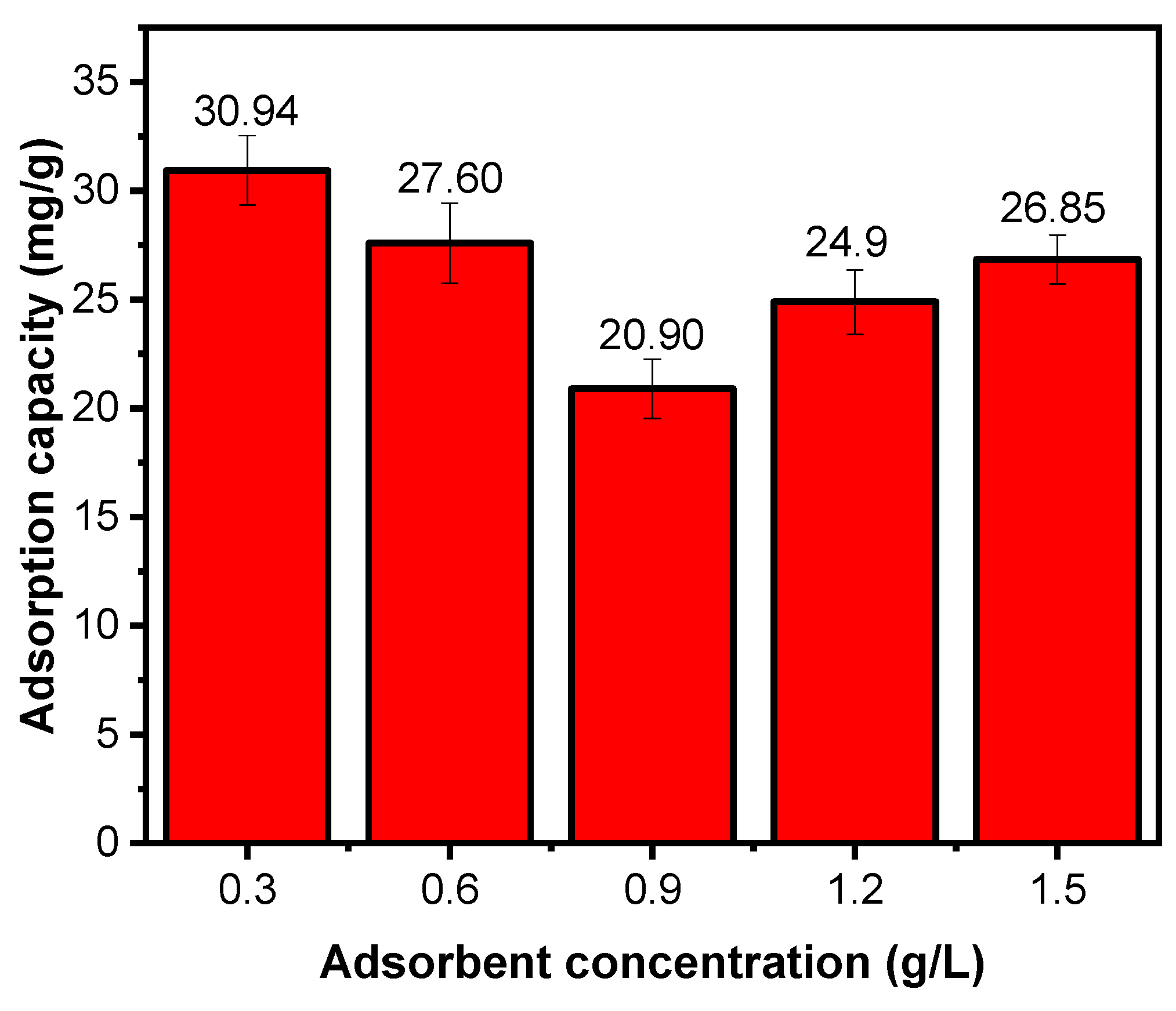
Effect of Chitosan Concentration
Effect of Solution pH
Effect of Temperature on Adsorption Capacity
3.2.3. Adsorption Kinetics Modeling
3.2.4. Adsorption Isotherms
Langmuir Isotherm
Freundlich Isotherm
3.3. Data Analysis via Response Surface Methodology
3.3.1. Analysis of Variance
3.3.2. Mathematical Modeling
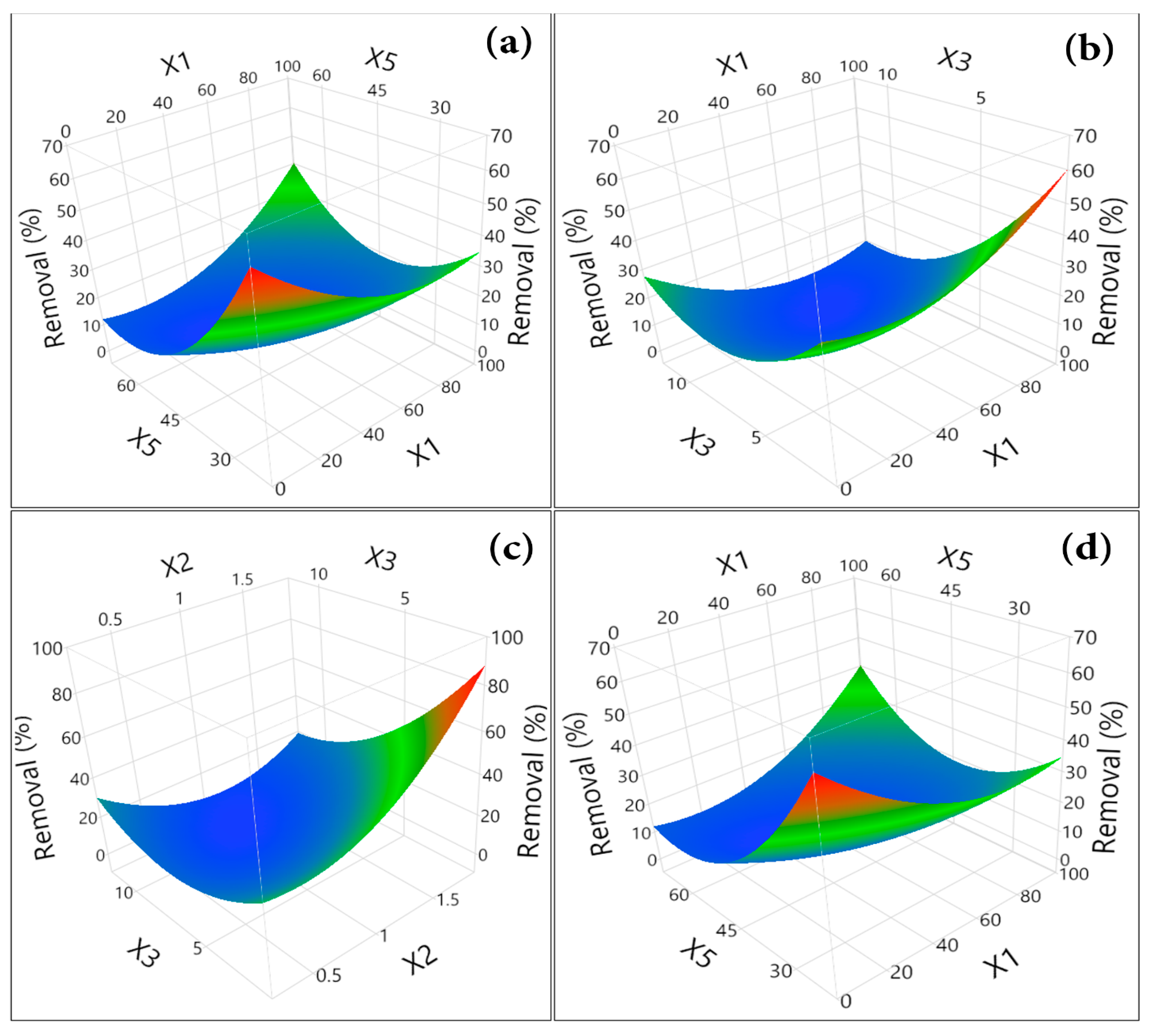
3.3.3. RSM Evolution
3.3.4. Optimization of the Values of the Variables
3.4. Thermodynamic Studies
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Pokhrel, D.; Viraraghavan, T. Treatment of Pulp and Paper Mill Wastewater—A Review. Sci. Total Environ. 2004, 333, 37–58. [Google Scholar] [CrossRef] [PubMed]
- Javadinejad, S.; Dara, R.; Hamed, M.; Akram, M.; Saeed, H.; Jafary, F. Analysis of Gray Water Recycling by Reuse of Industrial Waste Water for Agricultural and Irrigation Purposes. J. Geogr. Res. 2020, 3, 20–24. [Google Scholar] [CrossRef]
- Le, T.T.; Nguyen, T.; Nguyen, Q.; Man, P.; Kim, K.; Nguyen, N.H. Orange G Degradation by Heterogeneous Peroxymonosulfate Activation Based on Magnetic MnFe2O4/α-MnO2 Hybrid. J. Environ. Sci. 2023, 124, 379–396. [Google Scholar] [CrossRef]
- Boudraa, R.; Talantikite-Touati, D.; Souici, A.; Djermoune, A.; Saidani, A.; Fendi, K.; Amrane, A.; Bollinger, J.-C.; Tran, H.N.; Hadadi, A. Optical and Photocatalytic Properties of TiO2–Bi2O3–CuO Supported on Natural Zeolite for Removing Safranin-O Dye from Water and Wastewater. J. Photochem. Photobiol. A Chem. 2023, 443, 114845. [Google Scholar] [CrossRef]
- Patel, H.; Yadav, V.K.; Yadav, K.K.; Choudhary, N.; Kalasariya, H.; Alam, M.M.; Gacem, A.; Amanullah, M.; Ibrahium, H.A.; Park, J.-W. A Recent and Systemic Approach Towards Microbial Biodegradation of Dyes from Textile Industries. Water 2022, 14, 3163. [Google Scholar] [CrossRef]
- Hadadi, A.; Imessaoudene, A.; Bollinger, J.-C.; Assadi, A.A.; Amrane, A.; Mouni, L. Comparison of Four Plant-Based Bio-Coagulants Performances against Alum and Ferric Chloride in the Turbidity Improvement of Bentonite Synthetic Water. Water 2022, 14, 3324. [Google Scholar] [CrossRef]
- Hadadi, A.; Imessaoudene, A.; Bollinger, J.-C.; Cheikh, S.; Assadi, A.A.; Amrane, A.; Kebir, M.; Mouni, L. Parametrical Study for the Effective Removal of Mordant Black 11 from Synthetic Solutions: Moringa Oleifera Seeds’ Extracts Versus Alum. Water 2022, 14, 4109. [Google Scholar] [CrossRef]
- Gupta, V.; Kumar, R.; Nayak, A.; Saleh, T.; Barakat, M. Adsorptive Removal of Dyes from Aqueous Solution onto Carbon Nanotubes: A Review. Adv. Colloid. Interface Sci. 2013, 193, 24–34. [Google Scholar] [CrossRef]
- Lan, D.; Zhu, H.; Zhang, J.; Li, S.; Chen, Q.; Wang, C.; Wu, T.; Xu, M. Adsorptive Removal of Organic Dyes via Porous Materials for Wastewater Treatment in Recent Decades: A Review on Species, Mechanisms and Perspectives. Chemosphere 2022, 293, 133464. [Google Scholar] [CrossRef]
- Imessaoudene, A.; Cheikh, S.; Bollinger, J.-C.; Belkhiri, L.; Tiri, A.; Bouzaza, A.; El Jery, A.; Assadi, A.; Amrane, A.; Mouni, L. Zeolite Waste Characterization and Use as Low-Cost, Ecofriendly, and Sustainable Material for Malachite Green and Methylene Blue Dyes Removal: Box–Behnken Design, Kinetics, and Thermodynamics. Appl. Sci. 2022, 12, 7587. [Google Scholar] [CrossRef]
- Mouni, L.; Belkhiri, L.; Bollinger, J.-C.; Bouzaza, A.; Assadi, A.; Tirri, A.; Dahmoune, F.; Madani, K.; Remini, H. Removal of Methylene Blue from Aqueous Solutions by Adsorption on Kaolin: Kinetic and Equilibrium Studies. Appl. Clay Sci. 2018, 153, 38–45. [Google Scholar] [CrossRef]
- Bouchelkia, N.; Tahraoui, H.; Amrane, A.; Belkacemi, H.; Bollinger, J.-C.; Bouzaza, A.; Zoukel, A.; Zhang, J.; Mouni, L. Jujube Stones Based Highly Efficient Activated Carbon for Methylene Blue Adsorption: Kinetics and Isotherms Modeling, Thermodynamics and Mechanism Study, Optimization via Response Surface Methodology and Machine Learning Approaches. Process Saf. Environ. Prot. 2023, 170, 513–535. [Google Scholar] [CrossRef]
- Chedri Mammar, A.; Mouni, L.; Bollinger, J.-C.; Belkhiri, L.; Bouzaza, A.; Assadi, A.A.; Belkacemi, H. Modeling and Optimization of Process Parameters in Elucidating the Adsorption Mechanism of Gallic Acid on Activated Carbon Prepared from Date Stones. Sep. Sci. Technol. 2020, 55, 3113–3125. [Google Scholar] [CrossRef]
- Rápó, E.; Tonk, S. Factors Affecting Synthetic Dye Adsorption; Desorption Studies: A Review of Results from the Last Five Years (2017–2021). Molecules 2021, 26, 5419. [Google Scholar] [CrossRef] [PubMed]
- Chang, M.-Y.; Juang, R.-S. Adsorption of Tannic Acid, Humic Acid, and Dyes from Water Using the Composite of Chitosan and Activated Clay. J. Colloid. Interface Sci. 2004, 278, 18–25. [Google Scholar] [CrossRef] [PubMed]
- Miretzky, P.; Cirelli, A. Fluoride Removal from Water by Chitosan Derivatives and Composites. J. Fluor. Chem. 2011, 132, 231–240. [Google Scholar] [CrossRef]
- Gul, K.; Sohni, S.; Waqar, M.; Ahmad, F.; Norulaini, N.; Kadir, M. Functionalization of Magnetic Chitosan with Graphene Oxide for Removal of Cationic and Anionic Dyes from Aqueous Solution. Carbohydr. Polym. 2016, 152, 520–531. [Google Scholar] [CrossRef] [PubMed]
- Guo, P.; Anderson, J.; Bozell, J.; Zivanovic, S. The Effect of Solvent Composition on Grafting Gallic Acid onto Chitosan via Carbodiimide. Carbohydr. Polym. 2016, 140, 171–180. [Google Scholar] [CrossRef] [PubMed]
- Zhai, L.; Bai, Z.; Zhu, Y.; Wang, B.; Luo, W. Fabrication of Chitosan Microspheres for Efficient Adsorption of Methyl Orange. Chin. J. Chem. Eng. 2018, 26, 657–666. [Google Scholar] [CrossRef]
- Kumar, M. A Review of Chitin and Chitosan Applications. React. Funct. Polym. 2000, 46, 1–27. [Google Scholar] [CrossRef]
- Xu, Y.; Li, L.; Cao, S.; Zhu, B.; Yao, Z. An Updated Comprehensive Review of Advances on Structural Features, Catalytic Mechanisms, Modification Methods and Applications of Chitosanases. Process Biochem. 2022, 118, 263–273. [Google Scholar] [CrossRef]
- Hahn, T.; Roth, A.; Ji, R.; Schmitt, E.; Zibek, S. Chitosan Production with Larval Exoskeletons Derived from the Insect Protein Production. J. Biotechnol. 2020, 310, 62–67. [Google Scholar] [CrossRef] [PubMed]
- Spranghers, T.; Ottoboni, M.; Klootwijk, C.; Ovyn, A.; Deboosere, S.; Meulenaer, B.; Michiels, J.; Eeckhout, M.; De Clercq, P.; De Smet, S. Nutritional Composition of Black Soldier Fly (Hermetia Illucens) Prepupae Reared on Different Organic Waste Substrates. J. Sci. Food Agric. 2017, 97, 2594–2600. [Google Scholar] [CrossRef] [PubMed]
- Loc, N.; Tuyen, P.; Mai, L.; Phuong, D. Chitosan-Modified Biochar and Unmodified Biochar for Methyl Orange: Adsorption Characteristics and Mechanism Exploration. Toxics 2022, 10, 500. [Google Scholar] [CrossRef]
- Iber, B.; Kasan, N.; Torsabo, D.; Omuwa, J. A Review of Various Sources of Chitin and Chitosan in Nature. J. Renew. Mater. 2021, 10, 42–49. [Google Scholar] [CrossRef]
- Durakovic, B. Design of Experiments Application, Concepts, Examples: State of the Art. Period. Eng. Nat. Sci. 2017, 5, 421–439. [Google Scholar] [CrossRef]
- Osborne, D.M.; Armacost, R.L.; Pet-Edwards, J. State of the Art in Multiple Response Surface Methodology. In Proceedings of the 1997 IEEE International Conference on Systems, Man, and Cybernetics. Computational Cybernetics and Simulation, Orlando, FL, USA, 12–15 October 1997; Volume 4, p. 3838, ISBN 0-7803-4053-1. [Google Scholar]
- Truong, T.; Hausler, R.; Monette, F.; Niquette, P. The Valorization of Industrial Fishery Waste through the Hydrothermal-Chemical Transformation of Chitosan. Rev. Sci. L’Eau 2007, 20, 253–262. [Google Scholar] [CrossRef]
- Boudouaia, N.; Bengharez, Z.; Jellali, S. Preparation and Characterization of Chitosan Extracted from Shrimp Shells Waste and Chitosan Film: Application for Eriochrome Black T Removal from Aqueous Solutions. Appl. Water Sci. 2019, 9, 91. [Google Scholar] [CrossRef]
- Meetani, M.; Rauf, M.; Hisaindee, S.; Khaleel, A.; Alzamly, A.; Ahmad, A. Mechanistic Studies of Photoinduced Degradation of Orange G Using LC/MS. RSC Adv. 2011, 1, 490–497. [Google Scholar] [CrossRef]
- Li, Y.; Yang, Z.; Zhang, H.; Tong, X.; Feng, J. Fabrication of Sewage Sludge-Derived Magnetic Nanocomposites as Heterogeneous Catalyst for Persulfate Activation of Orange G Degradation. Colloids Surf. Physicochem. Eng. Asp. 2017, 529. [Google Scholar] [CrossRef]
- Mondal, M.; Singh, S.; Umareddy, M.; Dasgupta, B. Removal of Orange G from Aqueous Solution by Hematite: Isotherm and Mass Transfer Studies. Korean J. Chem. Eng. 2010, 27, 1811–1815. [Google Scholar] [CrossRef]
- Antonino, R.; Fook, B.; Lima, V.; Farias, R.; Lima, E.; Lima, R.; Covas, C.; Lia Fook, M. Preparation and Characterization of Chitosan Obtained from Shells of Shrimp (Litopenaeus Vannamei Boone). Mar. Drugs 2017, 15, 141. [Google Scholar] [CrossRef]
- Fiol, N.; Villaescusa, I. Determination of sorbent point zero charge: Usefulnessin sorption studies. Environ. Chem. Lett. 2009, 7, 79–84. [Google Scholar] [CrossRef]
- Imessaoudene, A.; Cheikh, S.; Hadadi, A.; Hamri, N.; Bollinger, J.-C.; Amrane, A.; Tahraoui, H.; Manseri, A.; Mouni, L. Adsorption Performance of Zeolite for the Removal of Congo Red Dye: Factorial Design Experiments, Kinetic, and Equilibrium Studies. Separations 2023, 10, 57. [Google Scholar] [CrossRef]
- Hadadi, A.; Imessaoudene, A.; Bollinger, J.-C.; Cheikh, S.; Manseri, A.; Mouni, L. Dual Valorization of Potato Peel (Solanum tuberosum) as a Versatile and Sustainable Agricultural Waste in Both Bioflocculation of Eriochrome Black T and Biosorption of Methylene Blue. J. Polym. Environ. 2023, 1–16. [Google Scholar] [CrossRef]
- Tinsson, W. Plans d’expérience: Constructions et Analyses Statistiques; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2010; Volume 67, ISBN 3-642-11472-5. [Google Scholar]
- Montgomery, D.C. Desing and Analysis of Experiments; Jonh Wiley Sons Quinta: Hoboken, NJ, USA, 2001. [Google Scholar]
- Goupy, J.; Creighton, L. Introduction Aux Plans d’expériences: Avec Applications; Dunod: Paris, France, 2013; ISBN 2-10-059296-3. [Google Scholar]
- Granato, D.; de Araújo Calado, V.M.; Jarvis, B. Observations on the Use of Statistical Methods in Food Science and Technology. Food Res. Int. 2014, 55, 137–149. [Google Scholar] [CrossRef]
- Pillet, M. Les Plans d’expériences Par La Méthode Taguchi; Editions d’Organisation: New York, NY, USA, 2001. [Google Scholar]
- Bhattacharya, S. Central Composite Design for Response Surface Methodology and Its Application in Pharmacy. In Response Surface Methodology in Engineering Science; IntechOpen: London, UK, 2021; pp. 1–19. ISBN 978-1-83968-917-8. [Google Scholar]
- Khoder, K. Optimisation de Composants Hyperfréquences Par La Technique Des Plans à Surfaces de Réponses. Limoges University. 2011. Available online: https://aurore.unilim.fr/ori-oai-search/notice/view/unilim-ori-28713 (accessed on 14 September 2023).
- Molatlhegi, O.; Alagha, L. Adsorption Characteristics of Chitosan Grafted Copolymer on Kaolin. Appl. Clay Sci. 2017, 150, 342–353. [Google Scholar] [CrossRef]
- Kumari, S.; Rath, P. Extraction and Characterization of Chitin and Chitosan from (Labeo Rohit) Fish Scales. Procedia Mater. Sci. 2014, 6, 482–489. [Google Scholar] [CrossRef]
- Povea, M.; Argüelles-Monal, W.; Valerio, J.; Rodríguez, C.; May Pat, A.; Rivero, N.; Peniche, C. Interpenetrated Chitosan-Poly(acrylic acid-co-acrylamide) Hydrogels. Synthesis, Characterization and Sustained Protein Release Studies. Mater. Sci. Appl. 2011, 2, 509–520. [Google Scholar] [CrossRef]
- Al-Ghouti, M.A.; Da’ana, D.A. Guidelines for the Use and Interpretation of Adsorption Isotherm Models: A Review. J. Hazard. Mater. 2020, 393, 122383. [Google Scholar] [CrossRef]
- Lee, B.H.; Lum, N.; Seow, L.; Lim, P.; Tan, L.P. Synthesis and Characterization of Types A and B Gelatin Methacryloyl for Bioink Applications. Materials 2016, 9, 797. [Google Scholar] [CrossRef] [PubMed]
- Dehghani, M.H.; Hassani, A.; Karri, R.; Younesi, B.; Shayeghi, M.; Salari, M.; Zarei, A.; Yousefi, M.; Heidarinejad, Z. Process Optimization and Enhancement of Pesticide Adsorption by Porous Adsorbents by Regression Analysis and Parametric Modelling. Sci. Rep. 2021, 11, 11719. [Google Scholar] [CrossRef]
- Venugopal, V. Seaweed, Microalgae, and Their Polysaccharides: Food Applications. In Marine Polysaccharides: Food Applications; CRC Press: Boca Raton, FL, USA, 2011; pp. 191–236. [Google Scholar]
- Mu’azu, N.D.; Jarrah, N.; Zubair, M.; Manzar, M.S.; Kazeem, T.S.; Qureshi, A.; Haladu, S.A.; Blaisi, N.I.; Essa, M.H.; Al-Harthi, M.A. Mechanistic Aspects of Magnetic MgAlNi Barium-Ferrite Nanocomposites Enhanced Adsorptive Removal of an Anionic Dye from Aqueous Phase. J. Saudi Chem. Soc. 2020, 24, 715–732. [Google Scholar] [CrossRef]
- Cheung, A.; Szeto, Y.S.; Mckay, G. Enhancing the Adsorption Capacities of Acid Dyes by Chitosan Nano Particles. Bioresour. Technol. 2008, 100, 1143–1148. [Google Scholar] [CrossRef]
- Zhou, L.; Jin, J.; Liu, Z.; Liang, X.; Shang, C. Adsorption of Acid Dyes from Aqueous Solutions by the Ethylenediamine-Modified Magnetic Chitosan Nanoparticles. J. Hazard. Mater. 2010, 185, 1045–1052. [Google Scholar] [CrossRef] [PubMed]
- Abualnaja, K.M.; Alprol, A.E.; Abu-Saied, M.A.; Ashour, M.; Mansour, A. Removing of Anionic Dye from Aqueous Solutions by Adsorption Using of Multiwalled Carbon Nanotubes and Poly (Acrylonitrile-Styrene) Impregnated with Activated Carbon. Sustainability 2021, 13, 7077. [Google Scholar] [CrossRef]
- Moussout, H. Critical of Linear and Nonlinear Equations of Pseudo-First Order and Pseudo-Second Order Kinetic Models. Karbala Int. J. Mod. Sci. 2018, 4, 244–254. [Google Scholar] [CrossRef]
- Huang, W.-Y.; Li, D.; Liu, Z.-Q.; Tao, Q.; Zhu, Y.; Yang, J.; Zhang, Y.-M. Kinetics, Isotherm, Thermodynamic, and Adsorption Mechanism Studies of La(OH)3-Modified Exfoliated Vermiculites as Highly Efficient Phosphate Adsorbents. Chem. Eng. J. 2014, 236, 191–201. [Google Scholar] [CrossRef]
- Vitek, R.; Masini, J. Nonlinear Regression for Treating Adsorption Isotherm Data to Characterize New Sorbents: Advantages over Linearization Demonstrated with Simulated and Experimental Data. Heliyon 2023, 9, e15128. [Google Scholar] [CrossRef]
- Tran, H.N.; You, S.-J.; Hosseini-Bandegharaei, A.; Chao, H.-P. Mistakes and Inconsistencies Regarding Adsorption of Contaminants from Aqueous Solutions: A Critical Review. Water Res. 2017, 120, 88–116. [Google Scholar]
- Tran, H.; You, S.-J.; Chao, H.-P. Fast and Efficient Adsorption of Methylene Green 5 on Activated Carbon Prepared from New Chemical Activation Method. J. Environ. Manag. 2017, 188, 322–336. [Google Scholar] [CrossRef] [PubMed]
- Wang, K.; Kou, Y.; Wang, K.; Liang, S.; Guo, G.; Wang, W.; Lu, Y.; Wang, J. Comparing the adsorption of methyl orange and malachite green on similar yet distinct polyamide microplastics: Uncovering hydrogen bond interactions. Chemosphere 2023, 340, 139806. [Google Scholar] [CrossRef] [PubMed]
- Arulkumar, M.; Sathishkumar, P.; Palvannan, T. Optimization of Orange G Dye Adsorption by Activated Carbon of Thespesia Populnea Pods Using Response Surface Methodology. J. Hazard. Mater. 2011, 186, 827–834. [Google Scholar] [CrossRef] [PubMed]
- Dev Vinu, V.; Wilson, B.; Nair, K.; Antony, S.; Krishnan, A. Response surface modeling of Orange-G adsorption onto surface tuned ragi husk. Colloide Interface Sci. Commun. 2021, 41, 100363. [Google Scholar] [CrossRef]
- Atia, A.A.; Donia, A.M.; Al-Amrani, W.A. Adsorption/Desorption Behavior of Acid Orange 10 on Magnetic Silica Modified with Amine Groups. Chem. Eng. J. 2009, 150, 55–62. [Google Scholar] [CrossRef]
- Potgieter, J.H.; Pardesi, C.; Pearson, S. A Kinetic and Thermodynamic Investigation into the Removal of Methyl Orange from Wastewater Utilizing Fly Ash in Different Process Configurations. Environ. Geochem. Health 2021, 43, 2539–2550. [Google Scholar] [CrossRef]
- Victor, R.P.; Fontes, L.L.; Neves, A.A.; de Queiroz, M.E.; Oliveira Miranda, L.D. Removal of orange G dye by manganese oxide nanostructures. J. Braz. Chem. Soc. 2019, 30, 1769–1778. [Google Scholar] [CrossRef]
- Rathee, V.; Awasthi, A.; Sood, D.; Tomar, R.; Tomar, V.; Chandra, R. A new biocompatible ternary layered double hydroxide adsorbent for ultrafast removal of anionic organic dyes. Sci. Rep. 2014, 9, 16225. [Google Scholar] [CrossRef]
- Tran, H.; Lima, E.; Juang, R.-S.; Bollinger, J.-C.; Chao, H.-P. Thermodynamic Parameters of Liquid-Phase Adsorption Process Calculated from Different Equilibrium Constants Related to Adsorption Isotherms: A Comparison Study. J. Environ. Chem. Eng. 2021, 9, 106674. [Google Scholar] [CrossRef]
- Salvestrini, S.; Leone, V.; Iovino, P.; Canzano, S.; Capasso, S. Considerations about the Correct Evaluation of Sorption Thermodynamic Parameters from Equilibrium Isotherms. J. Chem. Thermodyn. 2014, 68, 310–316. [Google Scholar] [CrossRef]





| Dye | Orange G |
|---|---|
| Generic name | Acid Orange 10 |
| Commercial name | Orange G |
| Color index number | 16,230 |
| Color | Dark orange powder |
| Class | Azo dye |
| (λmax) (nm) | 476 |
| Molecular formula | C16H10N2Na2O7S2 |
| Molecular weight (g/mol) | 452.386 |
| Chemical name (IUPAC) | 7-Hydroxy-8-(phenylazo)-1, 3-naphthalenedisulfonic acid disodium salt |
| Molecular structure [32] |  |
| C0 (mg/L) | qe (exp) | qe (calc) | k2 (min−1) | R2 | χ2 |
|---|---|---|---|---|---|
| 10 | 8.742 | 9.088 ± 0.06 | 0.0309 | 0.991 | 0.042 |
| 30 | 18.202 | 17.40 ± 0.08 | 0.0344 | 0.994 | 0.083 |
| 50 | 30.62 | 31.48 ± 0.32 | 0.0038 | 0.986 | 0.927 |
| 70 | 31.75 | 32.29 ± 0.35 | 0.0022 | 0.988 | 0.932 |
| 90 | 32 | 32.66 ± 0.29 | 0.0024 | 0.991 | 0.679 |
| Langmuir | Freundlich | ||||||
|---|---|---|---|---|---|---|---|
| qmax (mg/g) | KL (L/mg) | R2 | χ2 | KF [(mg/g)·(mg/L)1/n] | 1/n | R2 | χ2 |
| 34.631 ± 0.634 | 0. 241 ± 0.023 | 0.998 | 0.379 | 12.459 ± 0.123 | 0.262 ± 0.003 | 0.921 | 3.063 |
| Materials | Adsorption Capacity (mg/g) | Reference |
|---|---|---|
| Polyamide 66 | 8.854 | [60] |
| Activated carbon of Thespesia populnea pods | 9.129 | [61] |
| Formaldehyde-modified Ragi husk | 14.6 | [62] |
| Magnetic silica | 65.89 | [63] |
| Fly ash | 3.9 | [64] |
| Brown birnessite | 4.5 | [65] |
| Ni/Fe/Ti LDH | 11.81 | [66] |
| Shrimp carapace-derived chitosan | 34.63 | This study |
| No | Variable | Name | Variable Level | ||||
|---|---|---|---|---|---|---|---|
| −α (−2) | −1 | 0 | +1 | +α (+2) | |||
| 01 | X1 | C0 (mg/L) | 10 | 30 | 50 | 70 | 90 |
| 02 | X2 | S/L (g/L) | 0.3 | 0.6 | 0.9 | 1.2 | 1.5 |
| 03 | X3 | pH | 1.5 | 4 | 6.5 | 9 | 11.5 |
| 04 | X4 | Time (min) | 30 | 135 | 240 | 345 | 450 |
| 05 | X5 | Temperature (°C) | 25 | 35 | 45 | 55 | 65 |
| Run | Adsorbate Concentration (mg/L) | Adsorbent Concentration (g/L) | pH | Temperature | Time (min) | Removal (%) | ||
|---|---|---|---|---|---|---|---|---|
| Observed | Predicted | Residual | ||||||
| 1 | 50 | 0.9 | 6.5 | 45 | 30 | 16 | 14.12 | 1.87 |
| 2 | 70 | 0.6 | 9 | 55 | 345 | 3.5 | 0.02 | 3.47 |
| 3 | 30 | 0.6 | 4 | 55 | 345 | 12.58 | 10.83 | 1.74 |
| 4 | 30 | 0.6 | 4 | 35 | 135 | 25.08 | 25.71 | −0.63 |
| 5 | 30 | 0.6 | 9 | 35 | 345 | 31.5 | 29.02 | 2.47 |
| 6 | 70 | 1.2 | 4 | 55 | 345 | 18.34 | 17.71 | 0.62 |
| 7 | 50 | 0.9 | 6.5 | 65 | 240 | 7.2 | 10.09 | −2.89 |
| 8 | 50 | 0.9 | 6.5 | 45 | 240 | 8.9 | 7.05 | 1.84 |
| 9 | 30 | 1.2 | 4 | 35 | 345 | 40.75 | 41.11 | −0.36 |
| 10 | 50 | 0.9 | 6.5 | 25 | 240 | 27.85 | 27.84 | 0.00 |
| 11 | 30 | 1.2 | 9 | 55 | 345 | 5.66 | 3.85 | 1.80 |
| 12 | 50 | 0.9 | 6.5 | 45 | 240 | 7.85 | 7.05 | 0.79 |
| 13 | 50 | 0.9 | 6.5 | 45 | 240 | 7.3 | 7.05 | 0.24 |
| 14 | 70 | 1.2 | 4 | 35 | 135 | 40.28 | 42.03 | −1.75 |
| 15 | 50 | 0.9 | 1.5 | 45 | 240 | 36.36 | 34.90 | 1.45 |
| 16 | 10 | 0.9 | 6.5 | 45 | 240 | 13.25 | 14.14 | −0.89 |
| 17 | 70 | 0.6 | 4 | 55 | 135 | 29.35 | 28.98 | 0.36 |
| 18 | 70 | 1.2 | 9 | 35 | 345 | 14.71 | 13.35 | 1.35 |
| 19 | 50 | 1.5 | 6.5 | 45 | 240 | 20.7 | 19.35 | 1.34 |
| 20 | 50 | 0.9 | 11.5 | 45 | 240 | 1.6 | 5.94 | −4.34 |
| 21 | 70 | 0.6 | 4 | 35 | 345 | 22.92 | 21.62 | 1.29 |
| 22 | 90 | 0.9 | 6.5 | 45 | 240 | 12.5 | 14.48 | −1.98 |
| 23 | 50 | 0.3 | 6.5 | 45 | 240 | 9.24 | 13.47 | −4.23 |
| 24 | 70 | 1.2 | 9 | 55 | 135 | 19.46 | 19.04 | 0.41 |
| 25 | 70 | 0.6 | 9 | 35 | 135 | 7.78 | 6.69 | 1.08 |
| 26 | 30 | 1.2 | 9 | 35 | 135 | 4.16 | 4.73 | −0.57 |
| 27 | 30 | 0.6 | 9 | 55 | 135 | 15.66 | 14.11 | 1.54 |
| 28 | 50 | 0.9 | 6.5 | 45 | 450 | 3.75 | 8.50 | 4.75 |
| 29 | 30 | 1.2 | 4 | 55 | 135 | 17.41 | 18.71 | 1.30 |
| Factor | DF | Sum of Squares | F-Value | p-Value |
|---|---|---|---|---|
| pH | 1 | 1258.60 | 82.36 | <0.001 |
| Temperature | 1 | 472.77 | 31.01 | 0.0005 |
| C0 × S/L | 1 | 132.71 | 8.70 | 0.0184 |
| S/L × pH | 1 | 106.60 | 6.99 | 0.0295 |
| pH × T | 1 | 87.79 | 5.75 | 0.0432 |
| C0 × time | 1 | 268.79 | 17.63 | 0.003 |
| T × time | 1 | 345.77 | 22.68 | 0.0014 |
| (S/L) × (S/L) | 1 | 134.37 | 8.81 | 0.0179 |
| pH × pH | 1 | 274.14 | 17.98 | 0.0028 |
| T × T | 1 | 217.73 | 14.28 | 0.0054 |
| T (K) | KL (L/mg) | KL0 | Ln KL0 | ∆G0 (J/mol) | ∆H0 (kJ/mol) | ∆S0 (J/mol·K) |
|---|---|---|---|---|---|---|
| 298.15 | 0.241 | 109,025 | 11.599 | −28.752 | ||
| 308.15 | 0.063 | 28,500.32 | 10.257 | −26.279 | ||
| 318.15 | 0.02305 | 10,427.5 | 9.252 | −24.473 | −92.277 | −213.420 |
| 328.15 | 0.0078 | 3528.611 | 8.168 | −22.286 | ||
| 338.15 | 0.0028 | 1266.681 | 7.144 | −20.084 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Benazouz, K.; Bouchelkia, N.; Imessaoudene, A.; Bollinger, J.-C.; Amrane, A.; Assadi, A.A.; Zeghioud, H.; Mouni, L. Efficient and Low-Cost Water Remediation for Chitosan Derived from Shrimp Waste, an Ecofriendly Material: Kinetics Modeling, Response Surface Methodology Optimization, and Mechanism. Water 2023, 15, 3728. https://doi.org/10.3390/w15213728
Benazouz K, Bouchelkia N, Imessaoudene A, Bollinger J-C, Amrane A, Assadi AA, Zeghioud H, Mouni L. Efficient and Low-Cost Water Remediation for Chitosan Derived from Shrimp Waste, an Ecofriendly Material: Kinetics Modeling, Response Surface Methodology Optimization, and Mechanism. Water. 2023; 15(21):3728. https://doi.org/10.3390/w15213728
Chicago/Turabian StyleBenazouz, Kheira, Nasma Bouchelkia, Ali Imessaoudene, Jean-Claude Bollinger, Abdeltif Amrane, Aymen Amine Assadi, Hicham Zeghioud, and Lotfi Mouni. 2023. "Efficient and Low-Cost Water Remediation for Chitosan Derived from Shrimp Waste, an Ecofriendly Material: Kinetics Modeling, Response Surface Methodology Optimization, and Mechanism" Water 15, no. 21: 3728. https://doi.org/10.3390/w15213728
APA StyleBenazouz, K., Bouchelkia, N., Imessaoudene, A., Bollinger, J.-C., Amrane, A., Assadi, A. A., Zeghioud, H., & Mouni, L. (2023). Efficient and Low-Cost Water Remediation for Chitosan Derived from Shrimp Waste, an Ecofriendly Material: Kinetics Modeling, Response Surface Methodology Optimization, and Mechanism. Water, 15(21), 3728. https://doi.org/10.3390/w15213728










