Comparison of Pressure Pulsation Characteristics of Francis Turbine with Different Draft Tube Arrangement Direction
Abstract
:1. Introduction
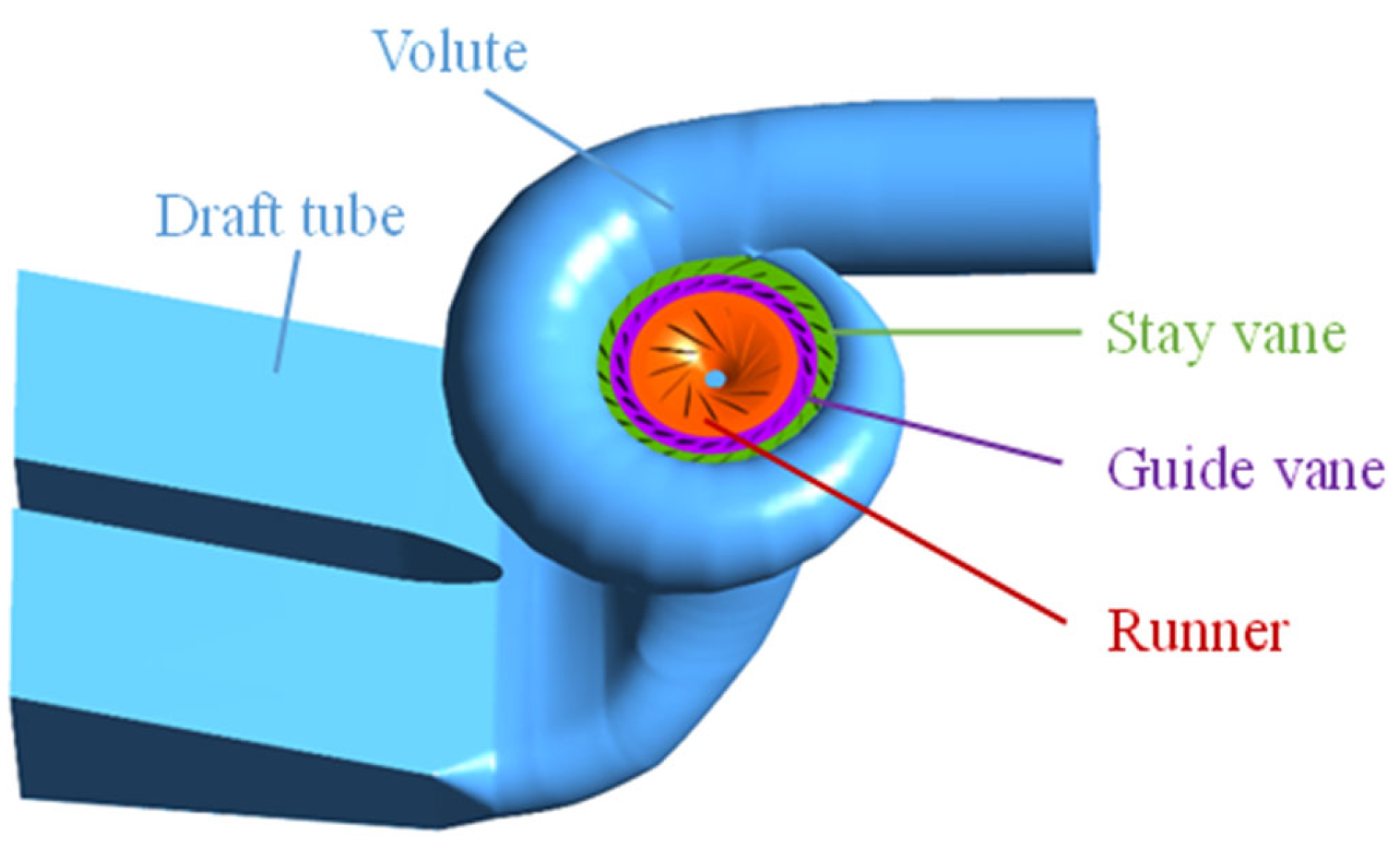
2. Francis Turbine Unit
2.1. Basic Parameters
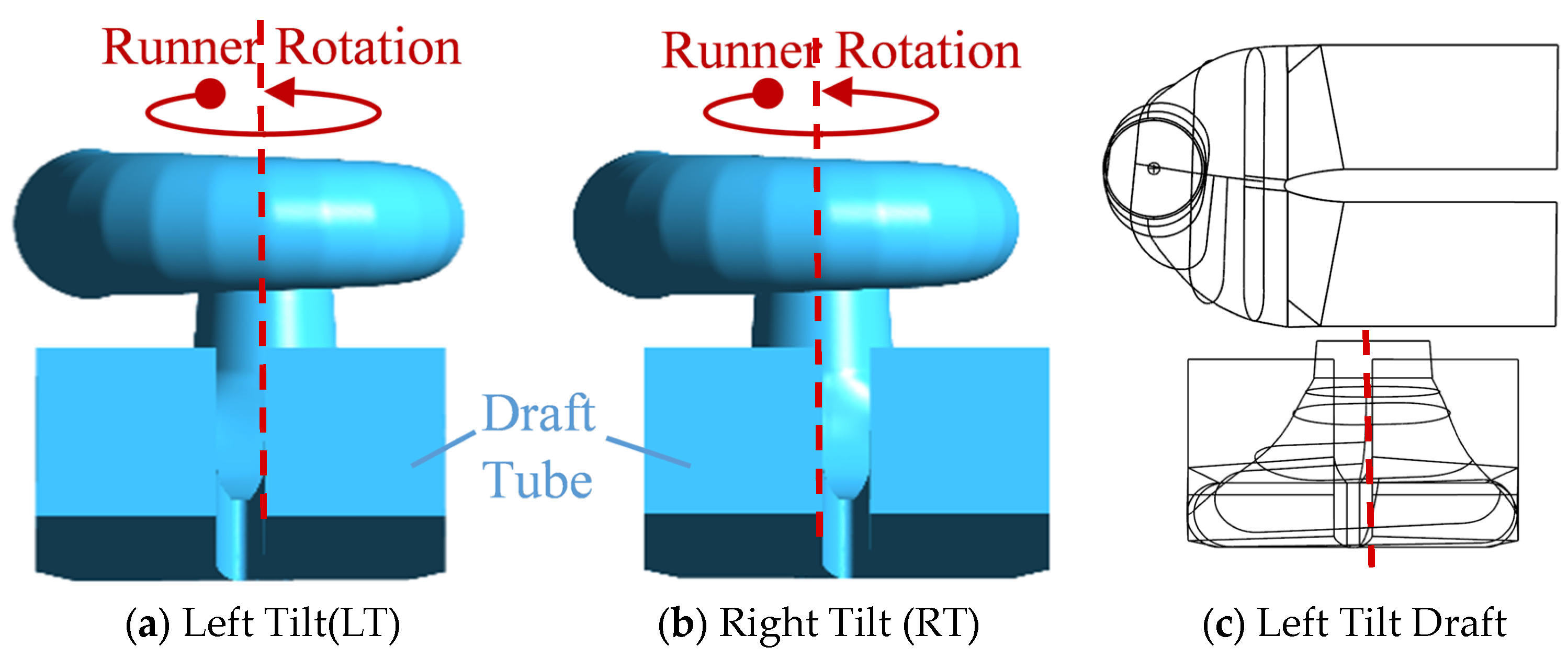
2.2. Asymmetric Arrangement of Draft Tube
3. Numerical Setup
3.1. Governing Equations
3.2. CFD Setup with Monitoring Points
3.3. Determination of Mesh
4. Results of Performance Analysis
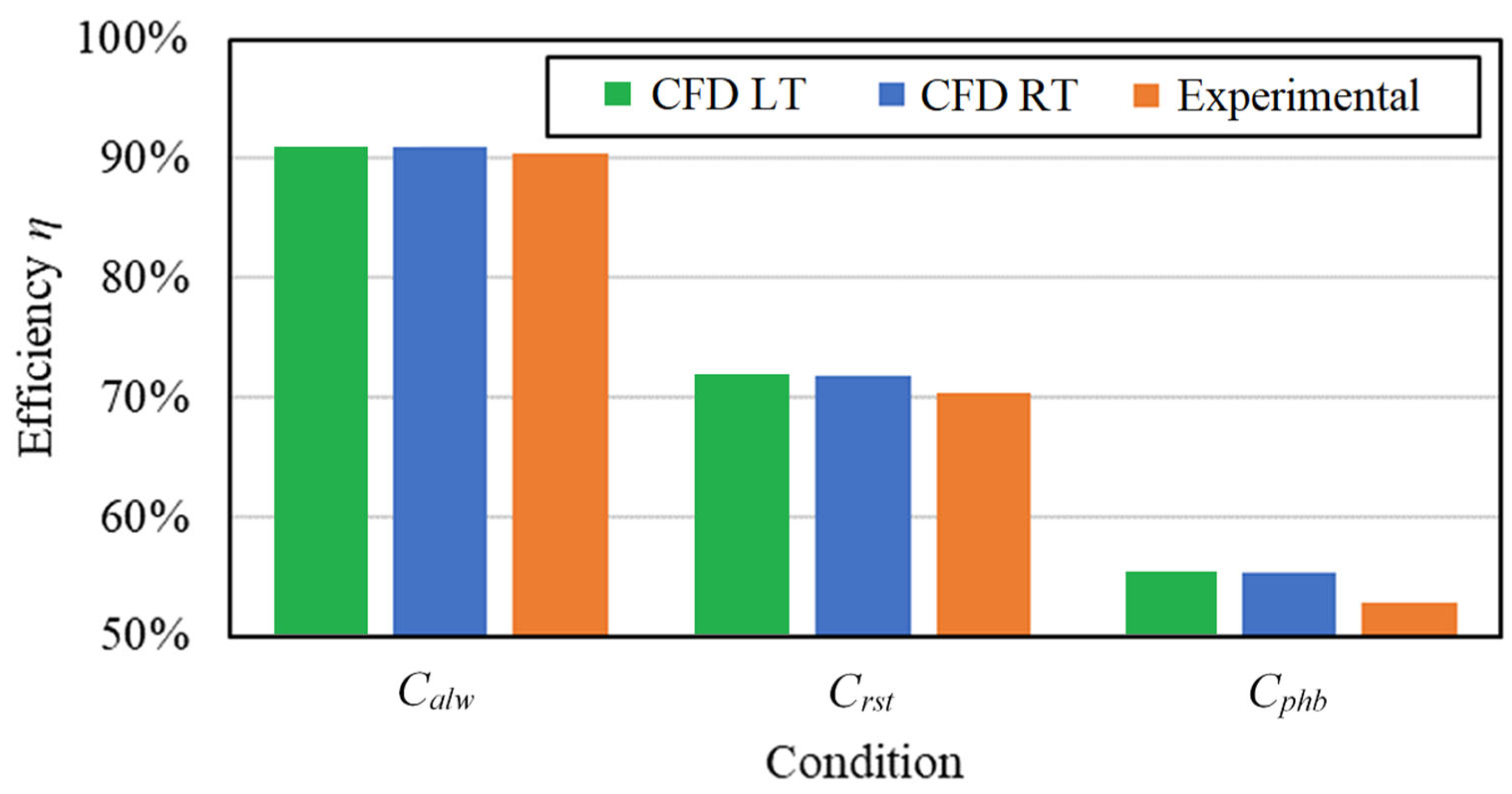
4.1. Efficiency Comparison
4.2. The Internal Flow Pattern
5. Results of Pressure Pulsation Analysis
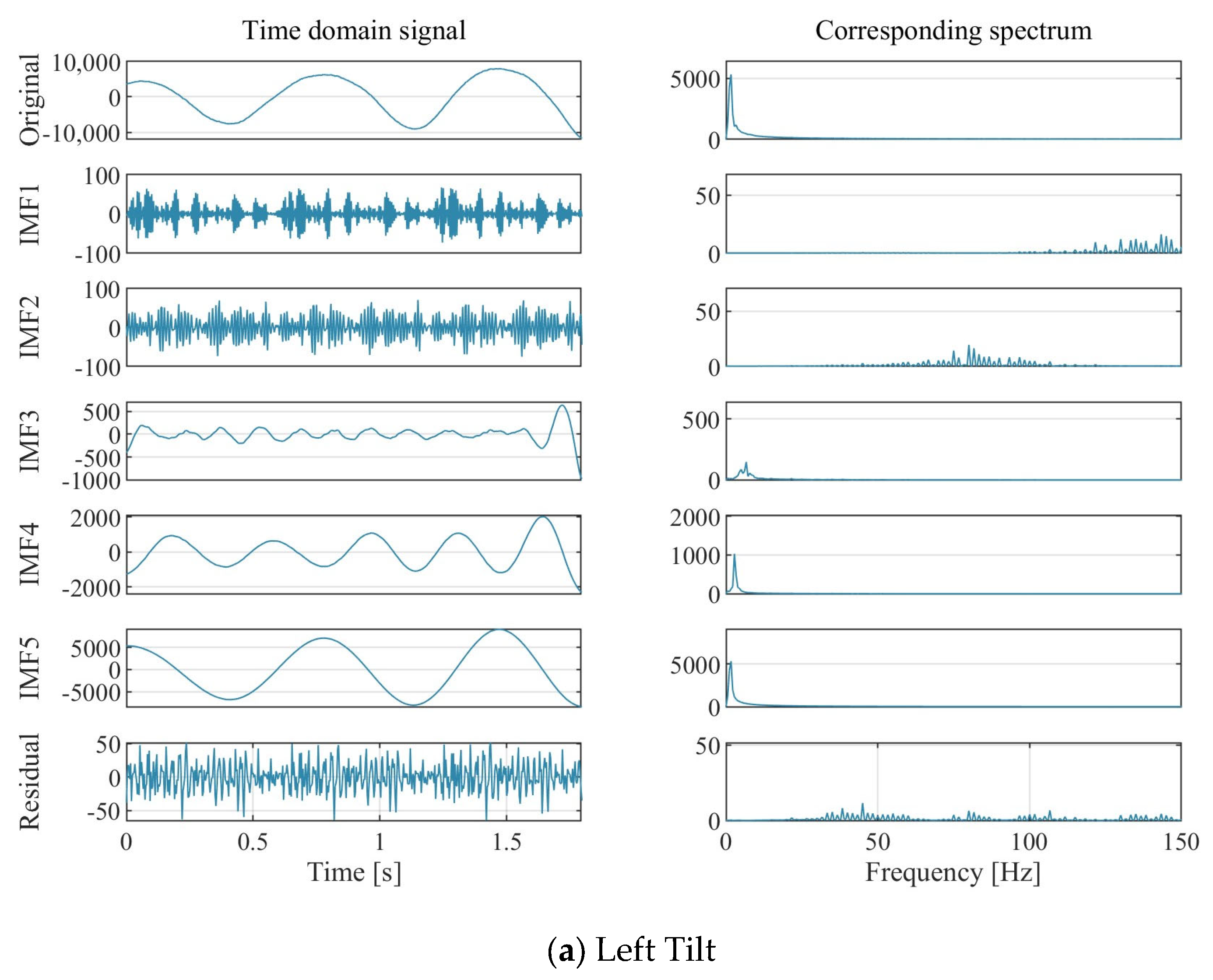
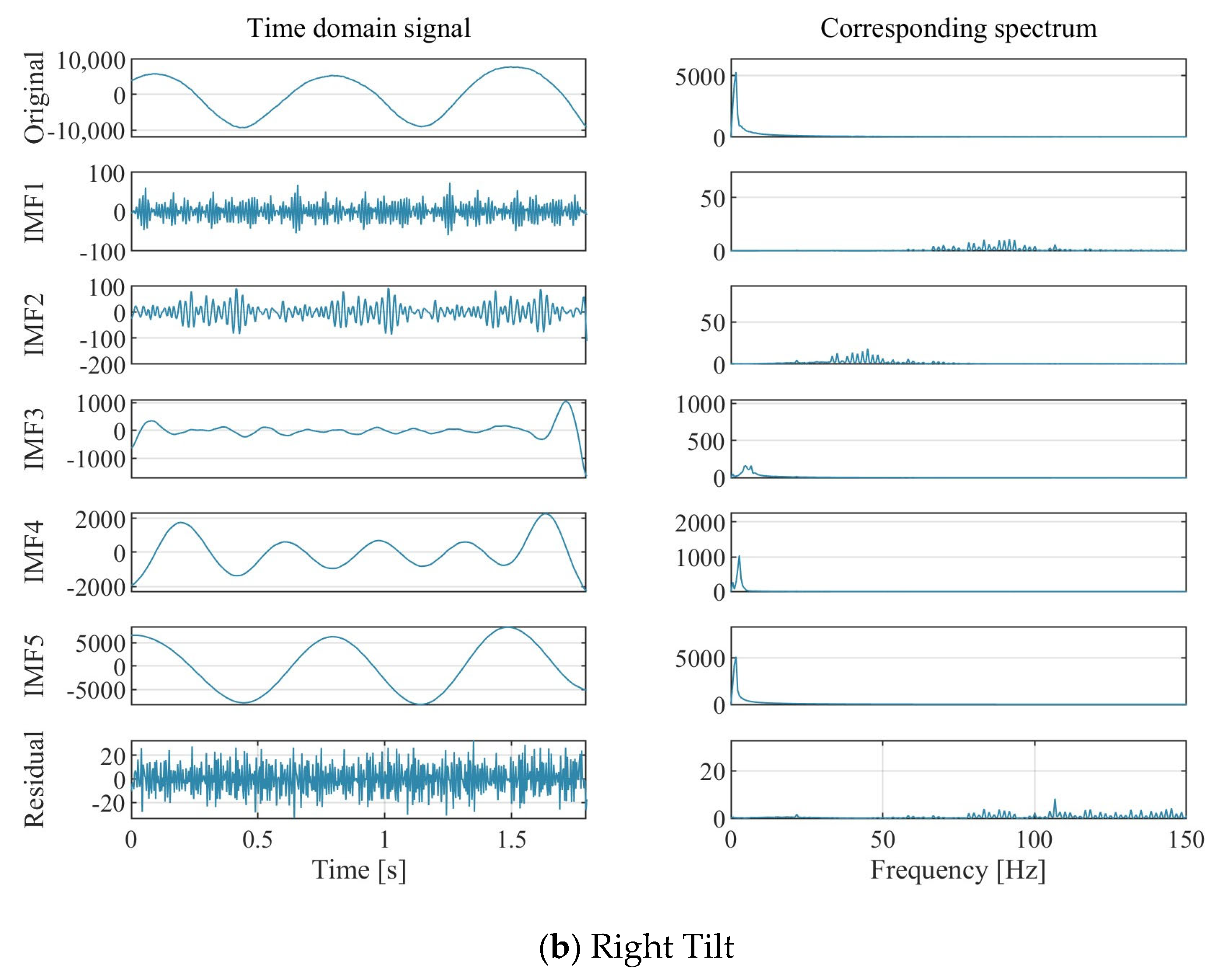
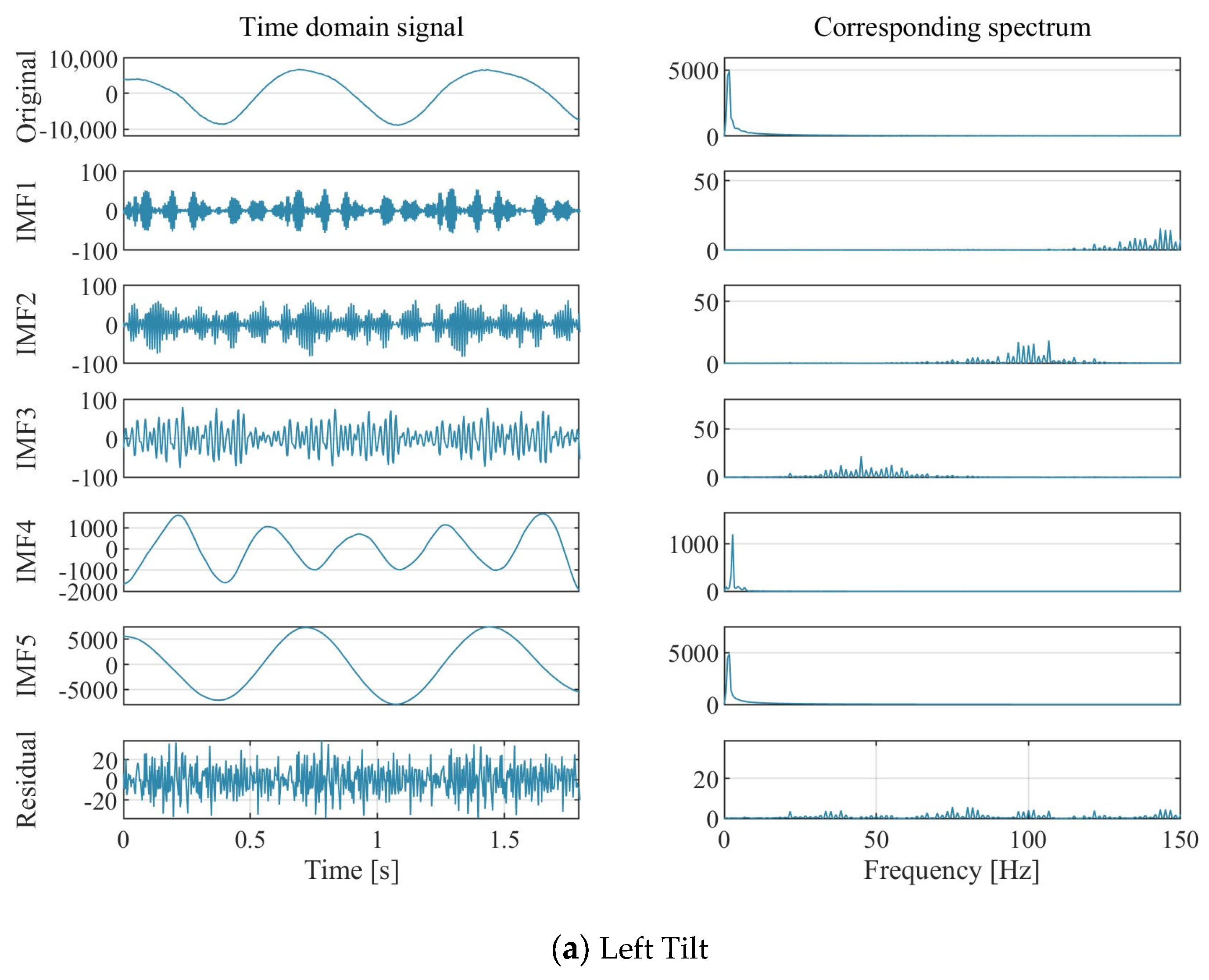
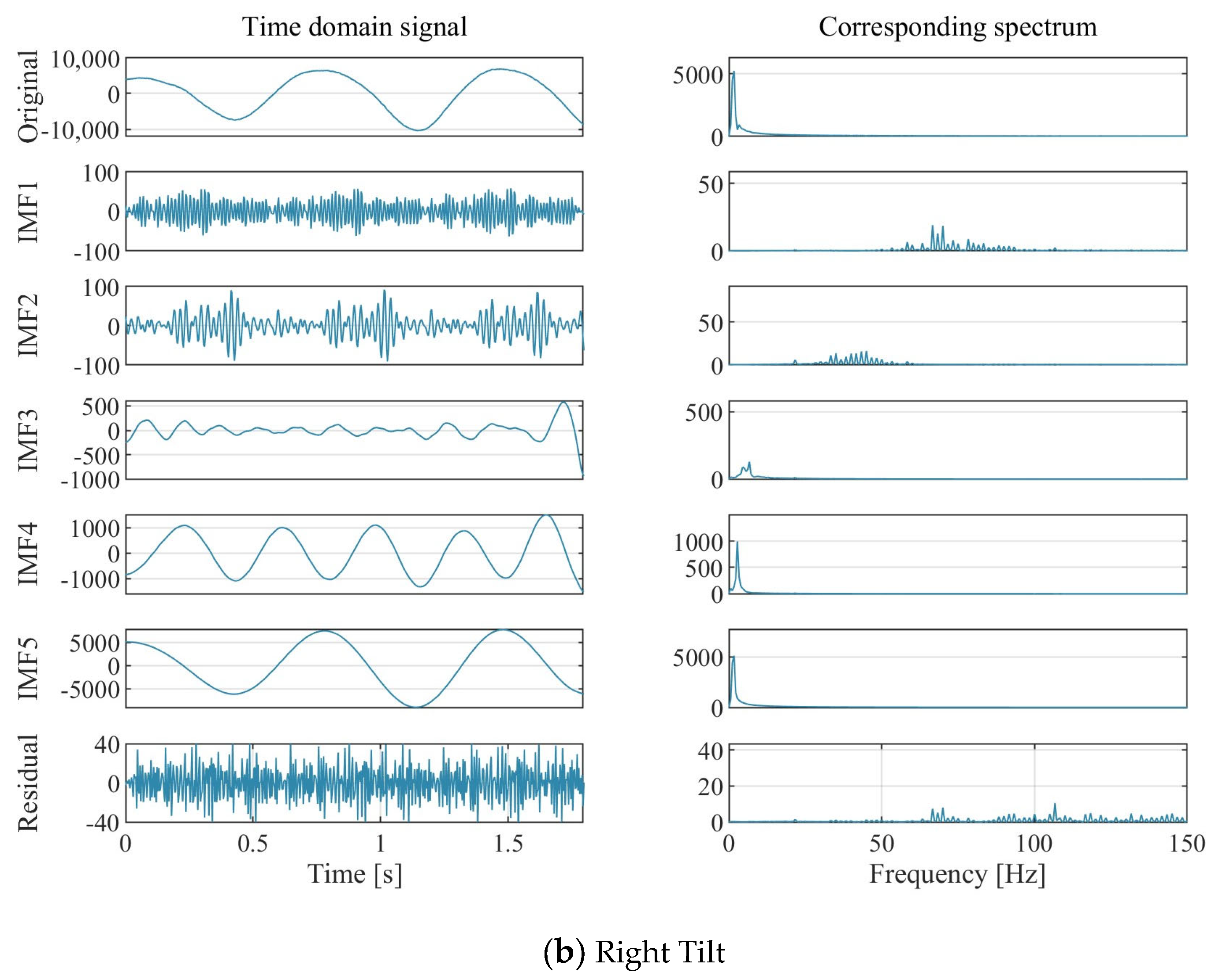
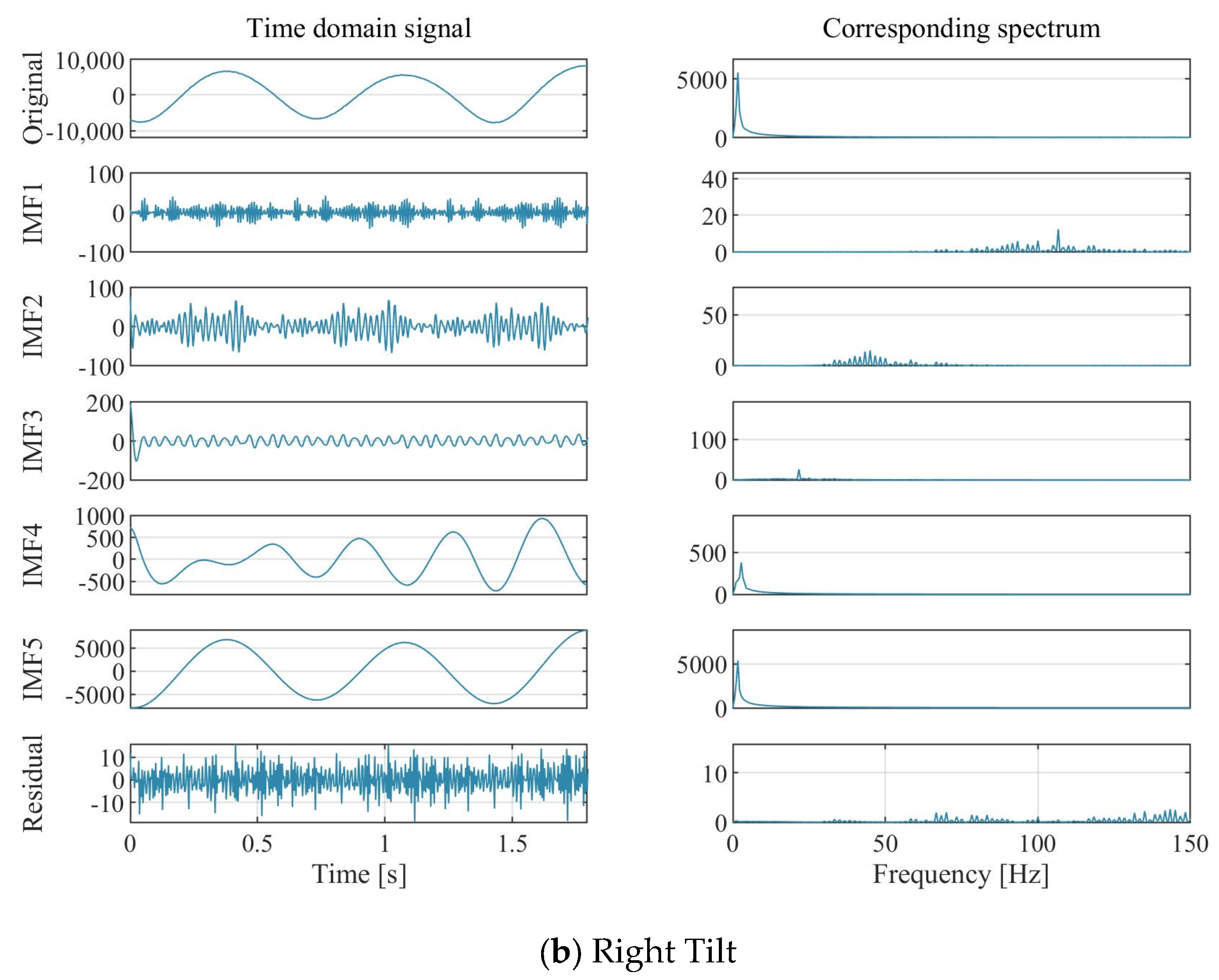
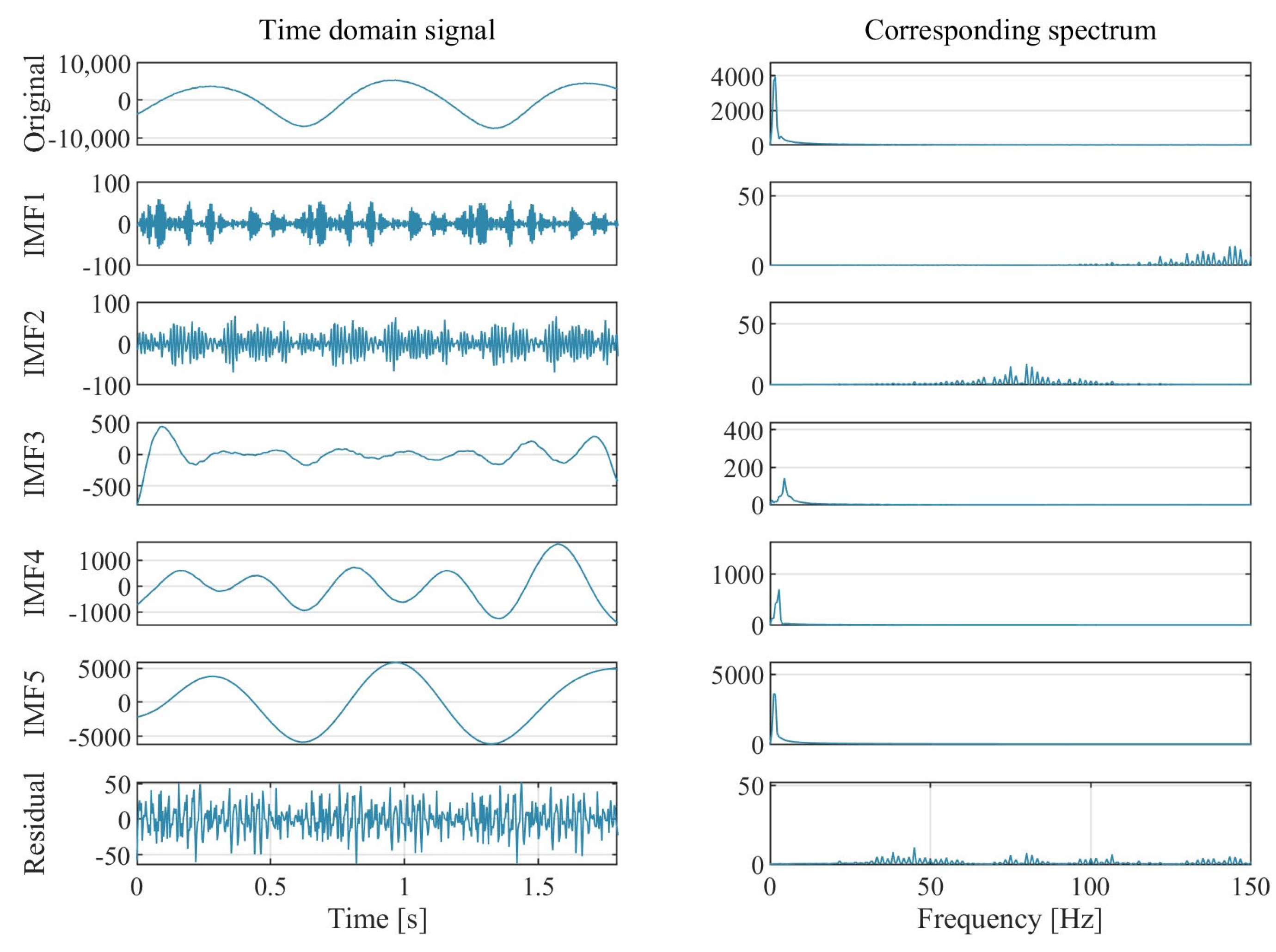
5.1. Analysis of Time Domain and Frequency Domain
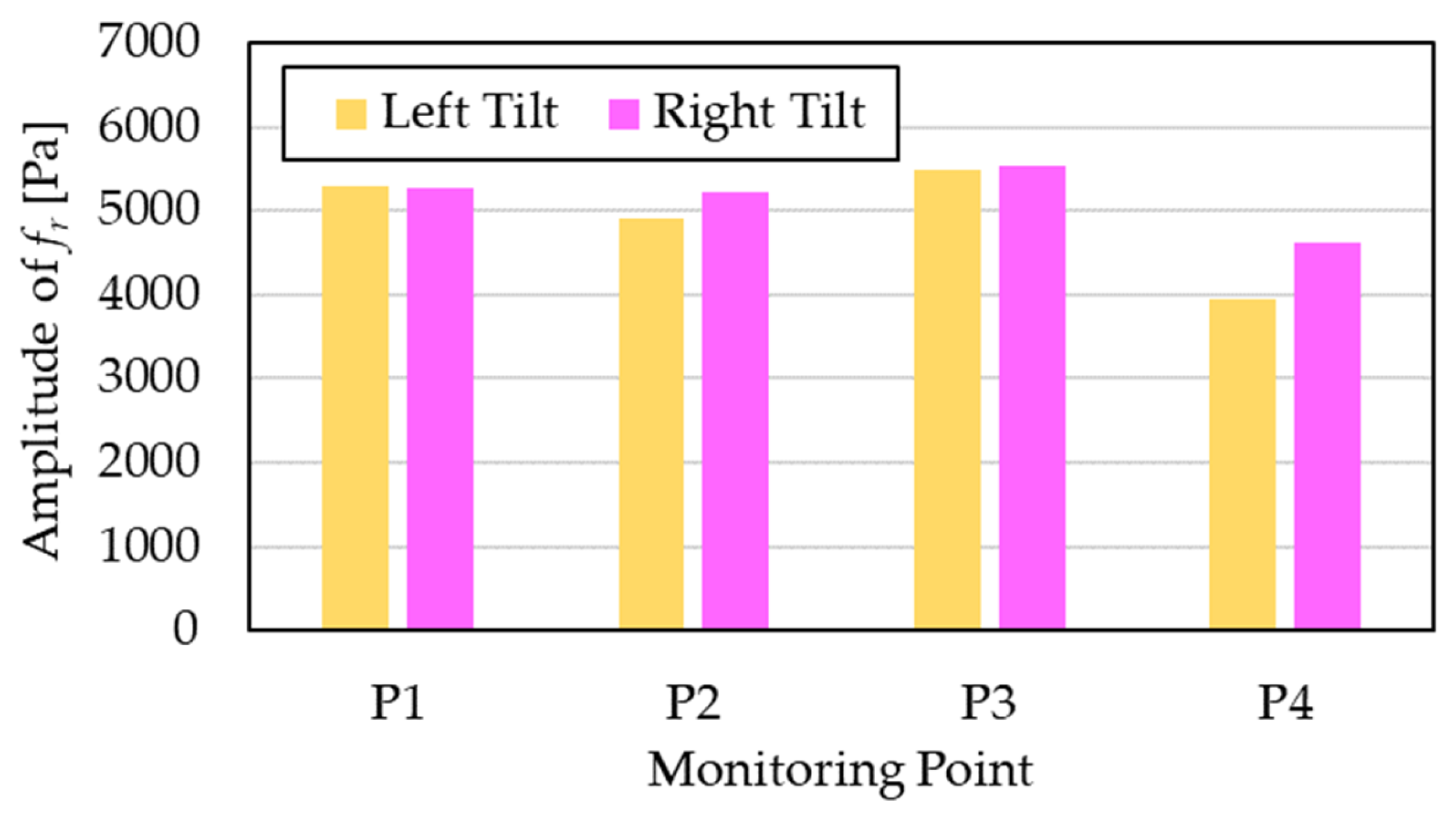
5.2. The Law of Pressure Pulsation
6. Conclusions
- (1)
- Left-tilt arrangement and right-tilt arrangement of the draft tube have little impact on the unit performance of the Francis turbine. By comparing the CFD calculation and analysis results with the prototype measurement results, it can be found that the direction of the asymmetric arrangement of the draft tube has little effect on the efficiency. As long as the cross-sectional area remains unchanged, the variation in total pressure remains unchanged, and the impact of left and right tilts on different working conditions is relatively small, typically less than 1% on efficiency. Therefore, from the perspective of hydraulic performance, the tilt direction of the draft tube can be left or right.
- (2)
- From the internal flow, it can be seen that there are significant differences in the flow inside the unit under different operating conditions. In the allowed region with higher efficiency, the flow smoothness is relatively high, and there are almost no obvious vortices or other forms of adverse flow patterns visible. In restricted and prohibited regions, the velocity distribution will be uneven, and the local velocity may be very high. Flow in the draft tube has also become chaotic, mainly with large-scale strong rotating flow. Judging from the characteristics of the flow direction, both the left-tilt and right-tilt draft tubes are acceptable. The impact of the left-tilt arrangement and right-tilt arrangement is still not significant, and the difference is almost invisible.
- (3)
- Based on the mode decomposition of the pressure fluctuation signal by VMD, it can be seen that different arrangement directions of the draft tube will have some effects on the flow. Overall, the frequency characteristics of the two are relatively similar with no huge differences. However, the dominant mode of the left tilt corresponds to a higher frequency, while the right tilt corresponds to a lower frequency. In addition, in terms of the amplitude of the important runner frequency fr, the value corresponding to the right tilt is slightly higher than that of the left tilt. From a hydrodynamic perspective, the geometric features of flow-passing components that are more adaptable to flow are more acceptable. If the left tilt is used, the fluctuation in the flow field may be more stable due to the same arrangement direction of the draft tube and volute.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Kose, F.; Kaya, M.N. Analysis on meeting the electric energy demand of an active plant with a wind-hydro hybrid power station in Konya, Turkey: Konya water treatment plant. Renew. Energy 2013, 55, 196–201. [Google Scholar] [CrossRef]
- Wu, Y.; Zhang, J.; Yuan, J.; Geng, S.; Zhang, H. Study of decision framework of offshore wind power station site selection based on ELECTRE-III under intuitionistic fuzzy environment: A case of China. Energy Convers. Manag. 2016, 113, 66–81. [Google Scholar] [CrossRef]
- Tao, R.; Lu, J.; Jin, F.; Hu, Z.; Zhu, D.; Luo, Y. Evaluation of the rotor eccentricity added radial force of oscillating water column. Ocean Eng. 2023, 276, 114222. [Google Scholar] [CrossRef]
- Dong, J.; Feng, T.-T.; Yang, Y.-S.; Ma, Y. Macro-site selection of wind/solar hybrid power station based on ELECTRE-II. Renew. Sustain. Energy Rev. 2014, 35, 194–204. [Google Scholar]
- Jafari, B.; Seddiq, M.; Mirsalim, S.M. Impacts of diesel injection timing and syngas fuel composition in a heavy-duty RCCI engine. Energy Convers. Manag. 2021, 247, 114759. [Google Scholar] [CrossRef]
- Ping, X.; Yang, F.; Zhang, H.; Zhang, J.; Xing, C.; Yan, Y.; Yang, A.; Wang, Y. Information theory-based dynamic feature capture and global multi-objective optimization approach for organic Rankine cycle (ORC) considering road environment. Appl. Energy 2023, 348, 121569. [Google Scholar] [CrossRef]
- Jafari, B.; Seddiq, M.; Mirsalim, S.M. Assessment of the impacts of combustion chamber bowl geometry and injection timing on a reactivity controlled compression ignition engine at low and high load conditions. Int. J. Engine Res. 2021, 22, 2852–2868. [Google Scholar] [CrossRef]
- Zhou, M.; Ye, Q.; Liu, Z. Climate impacts on hydro-power development in China. Bot. Mar. 2005, 19, 1–4. [Google Scholar]
- Pereira, J.G.; Vagnoni, E.; Favrel, A.; Landry, C.; Alligne, S.; Nicolet, C.; Avellan, F. Prediction of unstable full load conditions in a Francis turbine prototype. Mech. Syst. Signal Process. 2022, 169, 108666. [Google Scholar] [CrossRef]
- Kalantar, M.; Mousavi, S.M.G. Dynamic behavior of a stand-alone hybrid power generation system of wind turbine, microturbine, solar array and battery storage. Appl. Energy 2010, 87, 3051–3064. [Google Scholar] [CrossRef]
- Zhu, D.; Tao, R.; Xiao, R.; Pan, L. Solving the runner blade crack problem for a Francis hydro-turbine operating under condition-complexity. Renew. Energy 2020, 149, 298–320. [Google Scholar] [CrossRef]
- Li, P.; Xiao, R.; Tao, R. Study of vortex rope based on flow energy dissipation and vortex identification. Renew. Energy 2022, 198, 1065–1081. [Google Scholar] [CrossRef]
- Zhu, D.; Yan, W.; Guang, W.; Wang, Z.; Tao, R. Influence of guide vane opening on the runaway stability of a pump-turbine used for hydropower and ocean power. J. Mar. Sci. Eng. 2023, 11, 1218. [Google Scholar] [CrossRef]
- Tamura, Y.; Tani, K.; Okamoto, N. Experimental and numerical investigation of unsteady behavior of cavitating vortices in draft tube of low specific speed Francis turbine. IOP Conf. Ser. Earth Environ. Sci. 2014, 22, 032011. [Google Scholar] [CrossRef]
- Arthur, F.; Muller, A.; Landry, C.; Yamamoto, K.; Avellan, F. LDV survey of cavitation and resonance effect on the precessing vortex rope dynamics in the draft tube of Francis turbines. Exp. Fluids 2016, 57, 168. [Google Scholar]
- Zhang, J.; Appiah, D.; Zhang, F.; Yuan, S.; Gu, Y.; Asomani, S.N. Experimental and numerical investigations on pressure pulsation in a pump mode operation of a pump as turbine. Energy Sci. Eng. 2019, 7, 1264–1279. [Google Scholar] [CrossRef]
- Bosioc, A.I.; Susan-Resiga, R.; Muntean, S.; Tanasa, C. Unsteady pressure analysis of a swirling flow with vortex rope and axial water injection in a discharge cone. J. Fluids Eng. 2012, 134, 669–679. [Google Scholar] [CrossRef]
- Geng, C.; Li, Y.; Tsujimoto, Y.; Nishi, M.; Luo, X. Pressure oscillations with ultra-low frequency induced by vortical flow inside Francis turbine draft tubes. Sustain. Energy Technol. Assess. 2022, 51, 101908. [Google Scholar] [CrossRef]
- Liu, S.; Shao, Q.; Yang, J.; Wu, Y.; Dai, J. Unsteady turbulent simulation of Three Gorges hydraulic turbine and analysis of pressure in the whole passage. J. Hydroelectr. Eng. 2004, 23, 97–101. [Google Scholar]
- Liao, W.; Ji, J.; Lu, P.; Luo, X. Unsteady flow analysis of francis turbine. Chin. J. Mech. Eng. 2009, 45, 134–140. [Google Scholar] [CrossRef]
- Zhang, Y.; Liu, S.; Wu, Y. Detailed simulation and analysis of pressure pulsation in Francis turbine. J. Hydroelectr. Eng. 2009, 28, 183–186. [Google Scholar]
- Pasche, S.F.; Gallaire, F.; Avellan, F. Origin of the synchronous pressure pulsations in the draft tube of Francis turbines operating at part load conditions. J. Fluids Struct. 2019, 86, 13–33. [Google Scholar] [CrossRef]
- Kim, H.H.; Rakibuzzaman, M.; Kim, K.; Suh, S.-H. Flow and fast fourier transform analyses for tip clearance effect in an operating kaplan turbine. Energies 2019, 12, 264. [Google Scholar] [CrossRef]
- Wu, Y.; Guang, W.; Tao, R.; Liu, J. Dynamic mode structure analysis of the near-wake region of a Savonius-type hydrokinetic turbine. Ocean Eng. 2023, 282, 114965. [Google Scholar] [CrossRef]
- Tian, L.; Wu, J. Fault diagnosis and analysis of submersible sewage pump based on zoom-fft. Mach. Des. Res. 2018, 34, 171–174. [Google Scholar]
- Wang, W.; Tai, G.; Shen, J. Experimental investigation on pressure pulsation characteristics of a mixed-flow pump as turbine at turbine and runaway conditions. J. Energy Storage 2022, 55 Pt C, 105562. [Google Scholar] [CrossRef]
- Tang, Z.; Wang, M.; Ouyang, T.; Fei, C. A wind turbine bearing fault diagnosis method based on fused depth features in time–frequency domain. Energy Rep. 2022, 8, 12727–12739. [Google Scholar] [CrossRef]
- Jin, F.; Tao, R.; Lu, Z.; Xiao, R. A spatially distributed network for tracking the pulsation signal of flow field based on CFD simulation: Method and a case study. Fractal Fract. 2021, 5, 181. [Google Scholar] [CrossRef]
- Lu, Z.; Tao, R.; Jin, F.; Li, P.; Xiao, R.; Liu, W. The Temporal-Spatial Features of Pressure Pulsation in the Diffusers of a Large-Scale Vaned-Voluted Centrifugal Pump. Machines 2021, 9, 266. [Google Scholar] [CrossRef]
- Pope, S.B. Turbulent Flows; Cambridge University Press: Cambridge, UK, 2000. [Google Scholar]
- Terentiev, L. The Turbulence Closure Model Based on Linear Anisotropy Invariant Analysis; VDM Verlag: Saarbrucken, Germany, 2008. [Google Scholar]
- Menter, F.R. Two-Equation Eddy-Viscosity Turbulence Models for Engineering Applications. AIAA J. 1994, 32, 1598–1605. [Google Scholar] [CrossRef]
- Celik, I.B.; Ghia, U.; Roache, P.J.; Freitas, C.J.; Coloman, H.; Raad, P.E. Procedure for estimation and reporting of uncertainty due to discretization in CFD applications. J. Fluids Eng. 2008, 130, 78001. [Google Scholar]



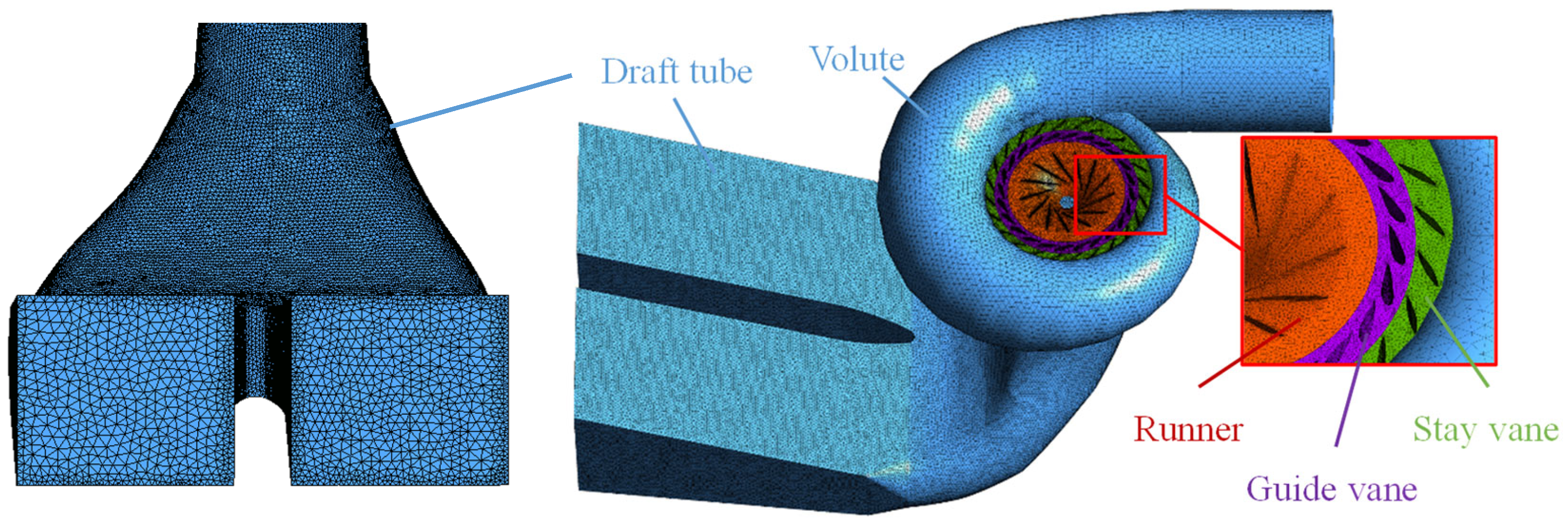




| Component | Mesh Number | Range of y+ | Average of y+ |
|---|---|---|---|
| Volute | 209,260 | 11–230 | 53 |
| Stay vane | 558,230 | 2–165 | 38 |
| Guide vane | 623,824 | 2–165 | 45 |
| Runner | 897,680 | 1.5–211 | 31 |
| Draft tube | 464,692 | 16–253 | 88 |
| Total | 2,753,686 | — | — |
| Left Tilt | Right Tilt | |||||||
|---|---|---|---|---|---|---|---|---|
| P1 | P2 | P3 | P4 | P1 | P2 | P3 | P4 | |
| IMF1 (Hz) | 137.10 | 141.41 | 139.33 | 138.67 | 87.70 | 78.28 | 102.43 | 71.15 |
| IMF2 (Hz) | 81.36 | 99.89 | 93.67 | 78.72 | 42.30 | 42.06 | 45.16 | 40.25 |
| IMF3 (Hz) | 6.24 | 46.85 | 41.29 | 4.67 | 5.51 | 5.78 | 20.28 | 2.58 |
| IMF4 (Hz) | 2.89 | 2.77 | 2.27 | 2.35 | 2.60 | 2.80 | 2.75 | 1.34 |
| IMF5 (Hz) | 1.41 | 1.38 | 1.33 | 1.33 | 1.35 | 1.40 | 1.37 | 0.34 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, T.; Hu, Z.; Liu, X.; Lu, J.; Song, X.; Zhu, D.; Wang, Z. Comparison of Pressure Pulsation Characteristics of Francis Turbine with Different Draft Tube Arrangement Direction. Water 2023, 15, 4028. https://doi.org/10.3390/w15224028
Zhang T, Hu Z, Liu X, Lu J, Song X, Zhu D, Wang Z. Comparison of Pressure Pulsation Characteristics of Francis Turbine with Different Draft Tube Arrangement Direction. Water. 2023; 15(22):4028. https://doi.org/10.3390/w15224028
Chicago/Turabian StyleZhang, Tao, Zilong Hu, Xinjun Liu, Jiahao Lu, Xijie Song, Di Zhu, and Zhengwei Wang. 2023. "Comparison of Pressure Pulsation Characteristics of Francis Turbine with Different Draft Tube Arrangement Direction" Water 15, no. 22: 4028. https://doi.org/10.3390/w15224028








