Analysis of Stress Characteristics of a Vertical Centrifugal Pump Based on Fluid-Structure Interaction
Abstract
:1. Introduction
2. Computational Model and Boundary Conditions
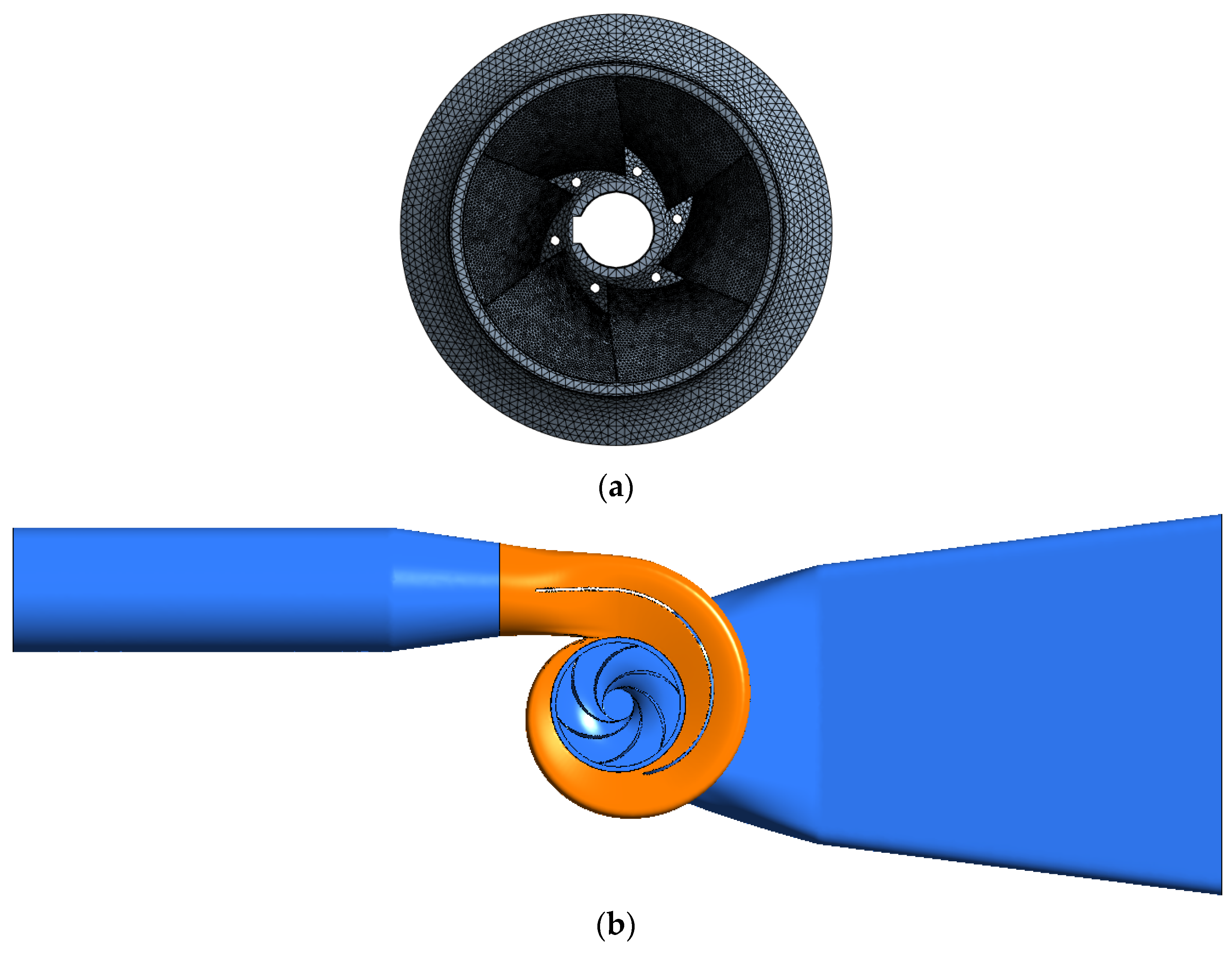
2.1. Computational Models
2.2. Boundary Conditions
2.3. Numerical Model
2.4. Validation
3. Impeller Modal Analysis
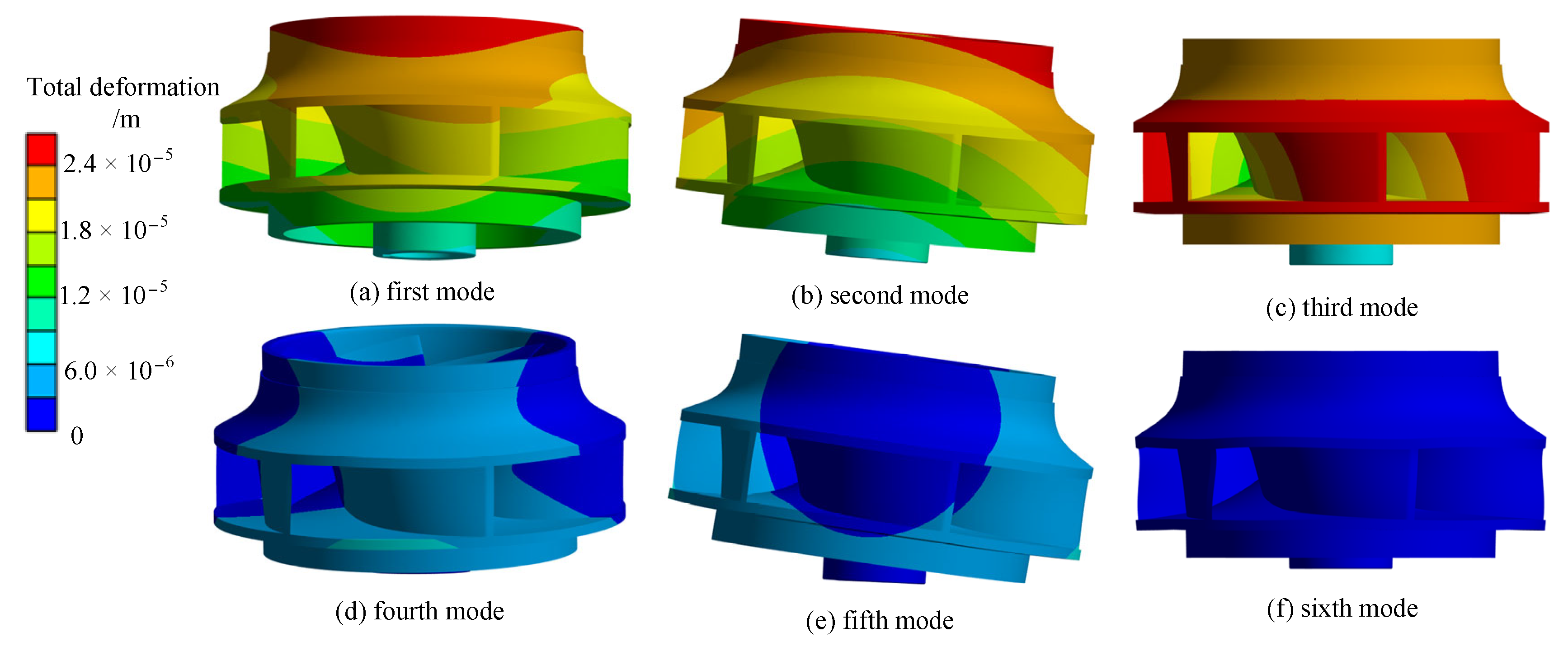
3.1. Modal Analysis of Impeller in Air
3.2. Modal Analysis of Impeller in Water
4. Centrifugal Pump Impeller Full-Condition Stress Characterization
4.1. Computational Modeling and Meshing
4.2. Boundary Conditions for Structural Field Calculations
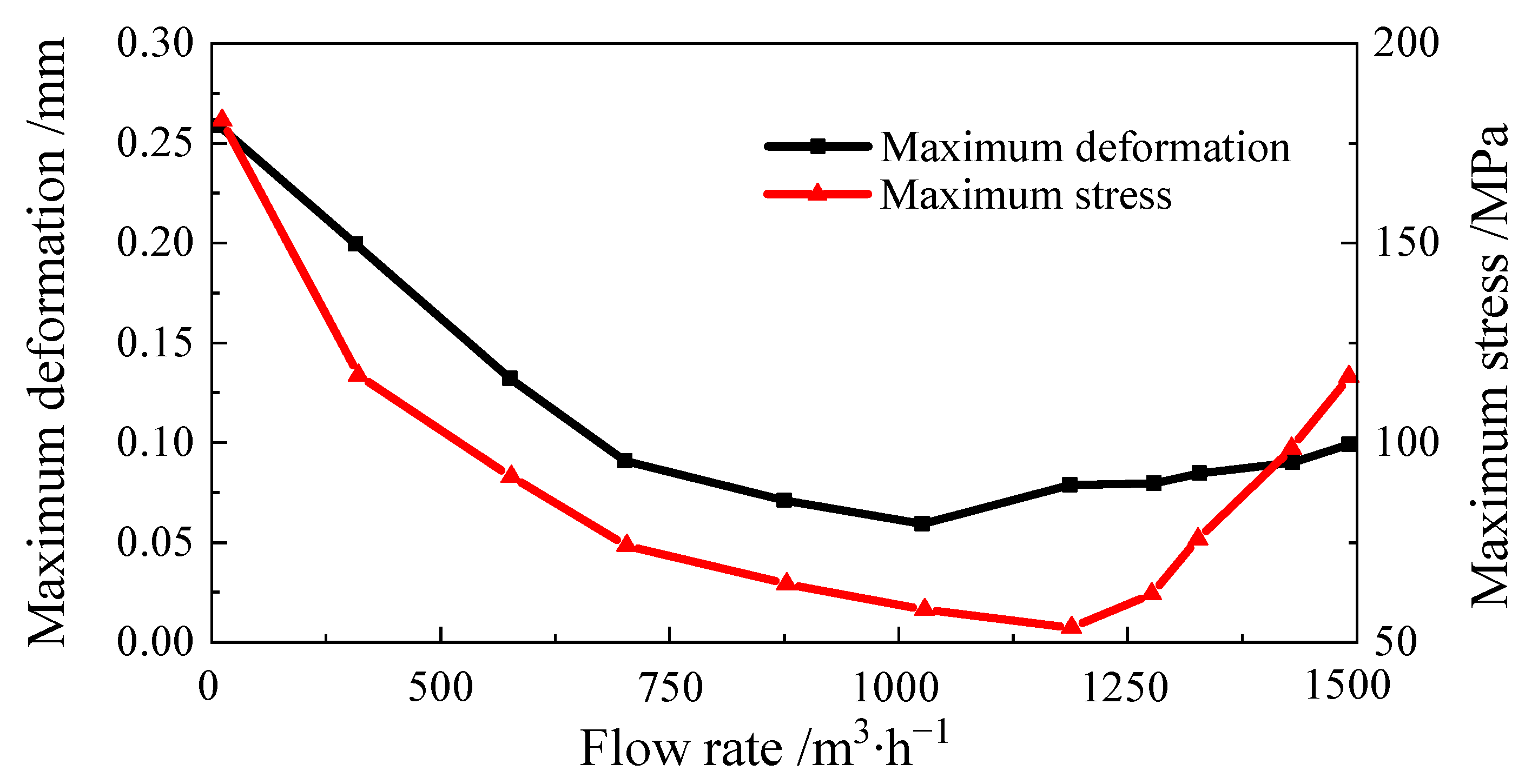
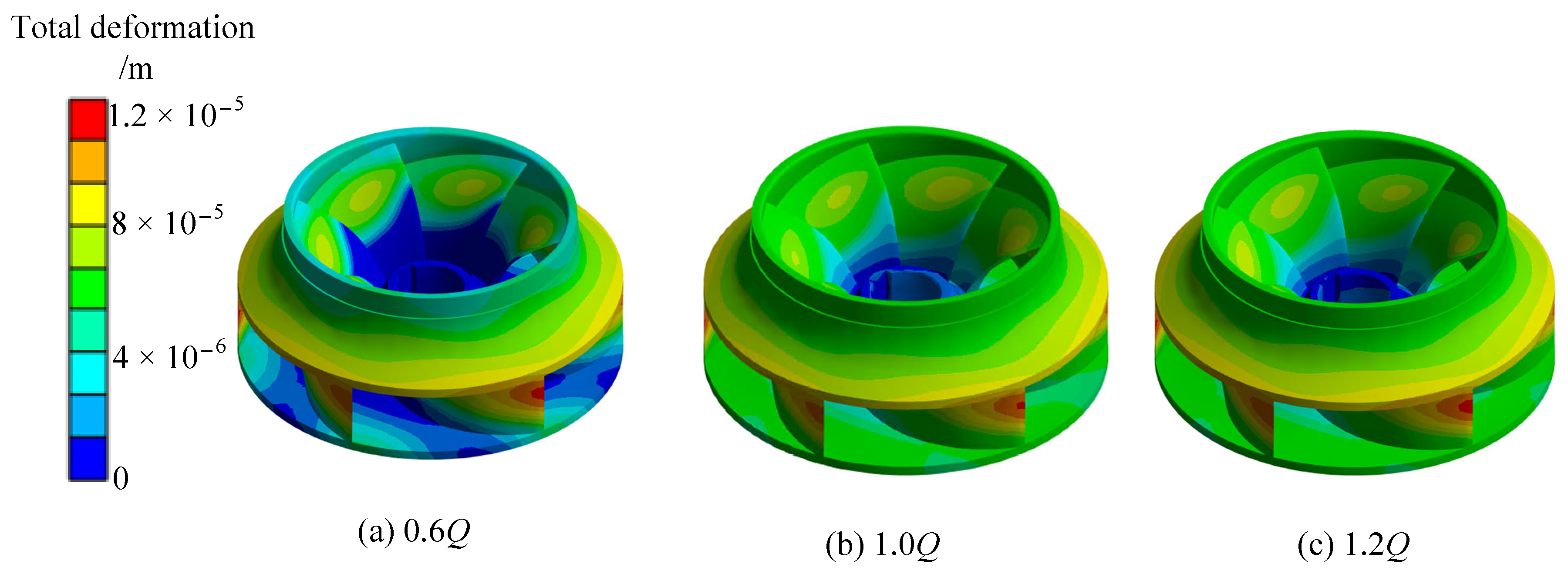
4.3. Calculated Field Results in Structures Analyzed
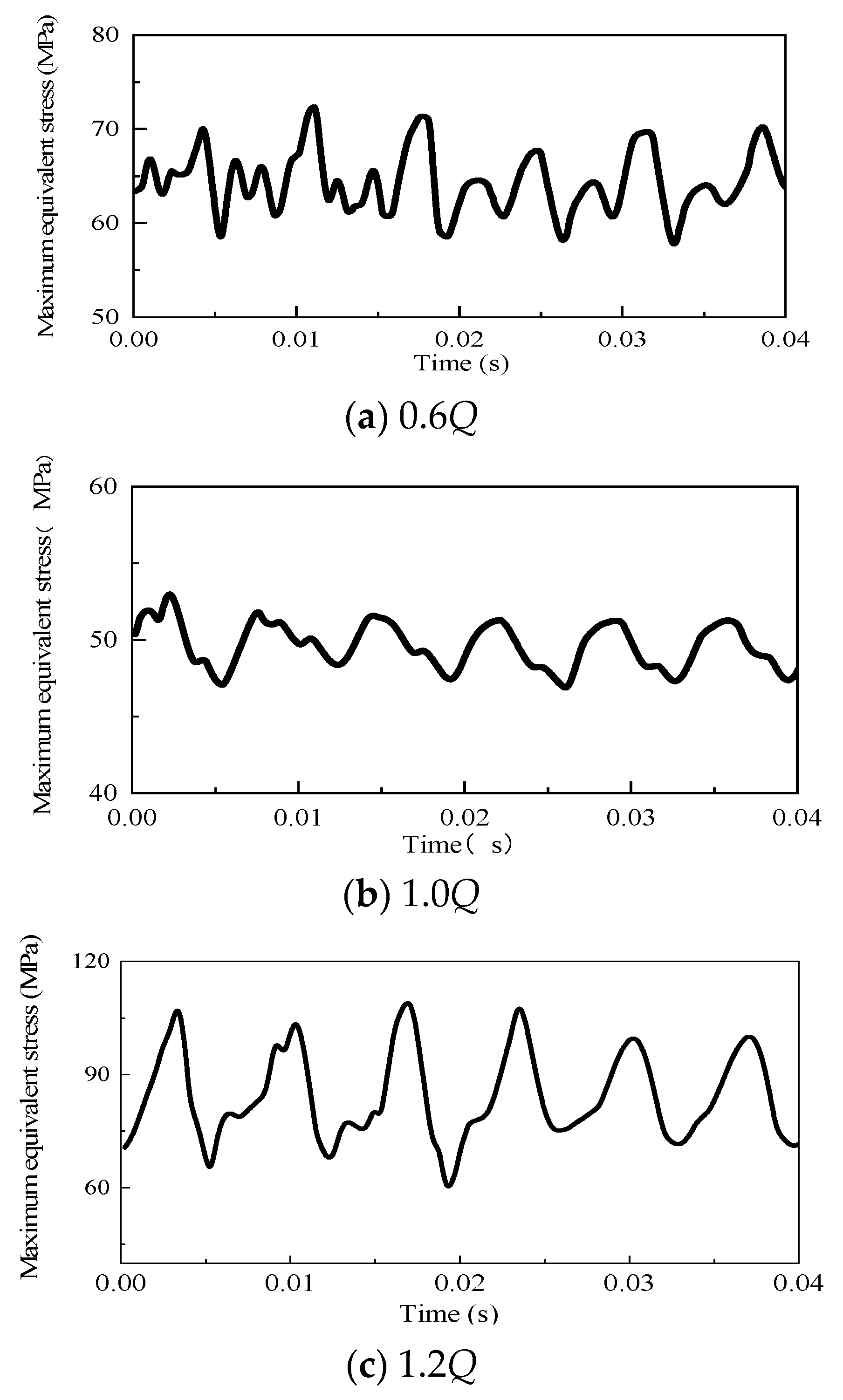
4.4. Stress Characterization of Impeller at Different Moments
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Adams, R. What Constitutes’ High Energy’ In Centrifugal Pumps? In Proceedings of the 30th International Pump Users Symposium, Houston, TX, USA, 23–25 September 2014; Turbomachinery Laboratories, Texas A&M Engineering Experiment Station: College Station, TX, USA, 2014. [Google Scholar]
- Chan, E.Y.Y.; Ho, J.Y.E. Urban water and health issues in Hong Kong. In Urban Drought: Emerging Water Challenges in Asia; Springer Nature: Singapore, 2019; pp. 241–262. [Google Scholar]
- Roy, A.; Palit, P.; Das, S.; Mukhyopadyay, G. Investigation of torsional fatigue failure of a centrifugal pump shaft. Eng. Fail. Anal. 2020, 112, 104511. [Google Scholar] [CrossRef]
- Ye, C.; Tang, Y.; An, D.; Wang, F.; Zheng, Y.; van Esch, B.P.M. Investigation on stall characteristics of marine centrifugal pump considering transition effect. Ocean Eng. 2023, 280, 114823. [Google Scholar] [CrossRef]
- Huang, X.; Fang, T.; Pang, K.; Guo, Q.; Qiu, B.; Lu, J. Air-entrained vortex in open intake: Time–frequency analysis and the interaction with subsurface vortices. Phys. Fluids 2022, 34, 113313. [Google Scholar] [CrossRef]
- Zheng, L.; Chen, X.; Dou, H.-S.; Zhang, W.; Zhu, Z.; Cheng, X. Effects of clearance flow on the characteristics of centrifugal pump under low flow rate. J. Mech. Sci. Technol. 2020, 34, 189–200. [Google Scholar] [CrossRef]
- Hoseini, S.S.; Najafi, G.; Ghobadian, B.; Akbarzadeh, A.H. Impeller shape-optimization of stirred-tank reactor: CFD and fluid structure interaction analyses. Chem. Eng. J. 2021, 413, 127497. [Google Scholar] [CrossRef]
- Hirschhorn, M.; Tchantchaleishvili, V.; Stevens, R.; Rossano, J.; Throckmorton, A. Fluid–structure interaction modeling in cardiovascular medicine—A systematic review 2017–2019. Med. Eng. Phys. 2020, 78, 1–13. [Google Scholar] [CrossRef]
- Cuamatzi-Meléndez, R.; Flores-Cuamatzi, E. Modelling fluid-structure interaction of water recirculating flow to predict damage and/or failure in a jet-pump assembly of a nuclear boiling water reactor. Eng. Struct. 2020, 206, 110155. [Google Scholar] [CrossRef]
- De Donno, R.; Ghidoni, A.; Noventa, G.; Rebay, S. Shape optimization of the ERCOFTAC centrifugal pump impeller using open-source software. Optim. Eng. 2019, 20, 929–953. [Google Scholar] [CrossRef]
- Olimstad, G.; Østby, P.T.K. Failure and redesign of a high-speed pump with respect to rotor-stator interaction. Eng. Fail. Anal. 2019, 104, 704–713. [Google Scholar] [CrossRef]
- Wang, W.; Zhou, L.; Tao, R.; Song, X.; Wang, Z. Numerical simulation of dynamic characteristics of hydrofoil structure under cavitation conditions. Ocean Eng. 2023, 280, 114937. [Google Scholar] [CrossRef]
- Griffith, B.E.; Patankar, N.A. Immersed Methods for Fluid–Structure Interaction. Annu. Rev. Fluid Mech. 2020, 52, 421–448. [Google Scholar] [CrossRef] [PubMed]
- Mo, J.-O.; Lee, Y.-H. Performance Prediction and Flow Characteristics of a Hydraulic Pump for ABS and ESC Systems Using FSI Simulation. Int. J. Automot. Technol. 2020, 21, 1419–1429. [Google Scholar] [CrossRef]
- Ye, C.; Wang, C.; Yan, H.; Wang, F.; Zheng, Y.; van Esch, B.P.M. Investigation on transition characteristics of laminar separation bubble on a hydrofoil. Phys. Fluids 2023, 35, 105154. [Google Scholar] [CrossRef]
- Luo, H.; Zhou, P.; Shu, L.; Mou, J.; Zheng, H.; Jiang, C.; Wang, Y. Energy Performance Curves Prediction of Centrifugal Pumps Based on Constrained PSO-SVR Model. Energies 2022, 15, 3309. [Google Scholar] [CrossRef]
- Krzemianowski, Z.; Steller, J. High specific speed Francis turbine for small hydro purposes-Design methodology based on solving the inverse problem in fluid mechanics and the cavitation test experience. Renew. Energy 2021, 169, 1210–1228. [Google Scholar] [CrossRef]
- Krella, A.; Maurin, A.; Krzemianowski, Z. Degradation of Armco iron caused by cavitation: Part II—Correlation with stress analysis. Eng. Fail. Anal. 2021, 128, 105621. [Google Scholar] [CrossRef]
- He, L.; Zhou, L.; Ahn, S.-H.; Wang, Z.; Nakahara, Y.; Kurosawa, S. Evaluation of gap influence on the dynamic response behavior of pump-turbine runner. Eng. Comput. 2019, 36, 491–508. [Google Scholar] [CrossRef]
- Xia, X.; Zhou, L.; Lv, Y.; Wang, Z. Numerical investigation of two degree-of-freedom galloping oscillation of a cylinder attached with fixed fairing device. Ocean Eng. 2021, 240, 109971. [Google Scholar] [CrossRef]
- Yang, J.; Zhou, L.-J.; Wang, Z.-W.; Jiang, X.-Y.; Zhou, X.-J.; Ding, J.-H.; Han, W.-F. Numerical investigation of the cavitation dynamic parameters in a Francis turbine draft tube with columnar vortex rope. J. Hydrodyn. 2019, 31, 931–939. [Google Scholar] [CrossRef]
- Hübner, B.; Silva, D.R. Advanced simulation of coupled physics in thrust bearings. In IOP Conference Series: Earth and Environmental Science; IOP Publishing: Bristol, UK, 2019; Volume 240, p. 062009. [Google Scholar]
- Hübner, B.; Seidel, U.; Roth, S. Application of fluid-structure coupling to predict the dynamic behavior of turbine components. In IOP Conference Series: Earth and Environmental Science; IOP Publishing: Bristol, UK, 2010; Volume 12, p. 012009. [Google Scholar]
- Trivedi, C.; Cervantes, M.J. Fluid-structure interactions in Francis turbines: A perspective review. Renew. Sustain. Energy Rev. 2017, 68, 87–101. [Google Scholar] [CrossRef]
- Badshah, M.; Badshah, S.; Jan, S. Comparison of computational fluid dynamics and fluid structure interaction models for the performance prediction of tidal current turbines. J. Ocean Eng. Sci. 2020, 5, 164–172. [Google Scholar] [CrossRef]
- Zanetti, G.; Cavazzini, G.; Santolin, A. Effect of the von Karman Shedding Frequency on the Hydrodynamics of a Francis Turbine Operating at Nominal Load. Int. J. Turbomach. Propuls. Power 2023, 8, 27. [Google Scholar] [CrossRef]
- Kan, K.; Zheng, Y.; Fu, S.; Liu, H.; Yang, C.; Zhang, X. Dynamic stress of impeller blade of shaft extension tubular pump device based on bidirectional fluid-structure interaction. J. Mech. Sci. Technol. 2017, 31, 1561–1568. [Google Scholar] [CrossRef]
- Quan, H.; Cheng, J.; Guo, Y.; Kang, L.; Peng, G. Influence of Screw Centrifugal Inducer on Internal Flow Structure of Vortex Pump. J. Fluids Eng. 2020, 142, 091203. [Google Scholar] [CrossRef]
- Presas, A.; Valentin, D.; Zhao, W.; Egusquiza, M.; Valero, C.; Egusquiza, E. On the use of neural networks for dynamic stress prediction in Francis turbines by means of stationary sensors. Renew. Energy 2021, 170, 652–660. [Google Scholar] [CrossRef]
- Valentín, D.; Presas, A.; Valero, C.; Egusquiza, M.; Egusquiza, E.; Gomes, J.; Avellan, F. Transposition of the mechanical behavior from model to prototype of Francis turbines. Renew. Energy 2020, 152, 1011–1023. [Google Scholar] [CrossRef]
- Chen, X.; Lai, X.; Gou, Q.; Song, D. Effect of guide vane profile on the hydraulic performance of moderate low-specific-speed Francis turbine. J. Mech. Sci. Technol. 2023, 37, 1289–1300. [Google Scholar] [CrossRef]
- Menéndez -Blanco, A.; Oro, J.M.F.; Meana-Fernández, A. Unsteady three-dimensional modeling of the Flu-id-Structure Interaction in the check valves of diaphragm volumetric pumps. J. Fluids Struct. 2019, 90, 432–449. [Google Scholar] [CrossRef]
- Li, W.; Ji, L.; Shi, W.; Zhou, L.; Jiang, X.; Zhang, Y. Fluid-structure interaction study of a mixed-flow pump impeller during startup. Eng. Comput. 2018, 35, 18–34. [Google Scholar] [CrossRef]
- Birajdar, R.; Keste, A. Prediction of Flow-Induced Vibrations due to Impeller Hydraulic Unbalance in Vertical Turbine Pumps Using One-Way Fluid-Structure Interaction. J. Vib. Eng. Technol. 2020, 8, 417–430. [Google Scholar] [CrossRef]
- Shi, L.; Zhu, J.; Wang, L.; Chu, S.; Tang, F.; Jin, Y. Comparative Analysis of Strength and Modal Characteristics of a Full Tubular Pump and an Axial Flow Pump Impellers Based on Fluid-Structure Interaction. Energies 2021, 14, 6395. [Google Scholar] [CrossRef]
- Wang, H.; Wang, F.; Wang, B.; Wu, J.; Lu, H.; Wang, C. Partial flow separation in guide-vane region of large-capacity/low-head pumped hydro energy storage system with horizontal shaft. J. Energy Storage 2023, 71, 108173. [Google Scholar] [CrossRef]
- Ye, C.; An, D.; Huang, W.; Heng, Y.; Zheng, Y. Investigation on Stall Characteristics of Centrifugal Pump with Guide Vanes. Water 2023, 15, 21. [Google Scholar] [CrossRef]
- Yan, H.; Heng, Y.; Zheng, Y.; Tao, R.; Ye, C. Investigation on Pressure Fluctuation of the Impellers of a Double-Entry Two-Stage Double Suction Centrifugal Pump. Water 2022, 14, 4065. [Google Scholar] [CrossRef]
- Wang, C.; Wang, F.; Li, C.; Chen, W.; Wang, H.; Lu, L. Investigation on energy conversion instability of pump mode in hydro-pneumatic energy storage system. J. Energy Storage 2022, 53, 105079. [Google Scholar] [CrossRef]
- Zeng, Y.; Yao, Z.; Huang, B.; Wu, Q.; Wang, F. Experimental investigation of the hydrodynamic damping of a vibrating hydrofoil in cavitating flow. Ocean Eng. 2022, 266, 112734. [Google Scholar] [CrossRef]
- Tickoo, S. ANSYS Workbench 2021 R1: A Tutorial Approach; CADCIM Technologies: Schererville, IN, USA, 2021. [Google Scholar]
- Zhou, W.; Zhou, P.; Xiang, C.; Wang, Y.; Mou, J.; Cui, J. A Review of Bionic Structures in Control of Aerodynamic Noise of Centrifugal Fans. Energies 2023, 16, 4331. [Google Scholar] [CrossRef]








| Parameters | Value |
|---|---|
| Modulus of elasticity E (Gpa) | 206 |
| Poisson ratio μ | 0.288 |
| Material density ρ (kg/m3) | 7700 |
| Meshing | 8-node tetrahedral solid unit solid 45 |
| Mode Order | ƒa (Hz) | ƒw (Hz) | η = ƒw/ƒa |
|---|---|---|---|
| 1 | 767.54 | 352.84 | 0.46 |
| 2 | 768.19 | 354.42 | 0.46 |
| 3 | 917.33 | 354.43 | 0.39 |
| 4 | 1105.8 | 477.61 | 0.43 |
| 5 | 1259.2 | 573.66 | 0.46 |
| 6 | 1260.6 | 574.01 | 0.46 |
| 7 | 1696.7 | 591.56 | 0.35 |
| 8 | 1704.7 | 591.56 | 0.35 |
| 9 | 1708.9 | 604.96 | 0.35 |
| 10 | 1747.3 | 695.88 | 0.40 |
| Physical Quantity | Measure Value | Physical Quantity | Measure Value |
|---|---|---|---|
| Density ρ (kg/m3) | 7700 | Tensile strength σb (MPa) | 580 |
| Modulus of elasticity E (GPa) | 206 | Yield limit σs (MPa) | 950 |
| Poisson’s ratio μ | 0.288 | Permissible stress [σ], (MPa) | 560 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, S.; Tu, Y.; Ye, C.; Yan, H.; Dai, J.; Dang, M.; Yang, C.; Zheng, Y.; Li, Y. Analysis of Stress Characteristics of a Vertical Centrifugal Pump Based on Fluid-Structure Interaction. Water 2023, 15, 4269. https://doi.org/10.3390/w15244269
Li S, Tu Y, Ye C, Yan H, Dai J, Dang M, Yang C, Zheng Y, Li Y. Analysis of Stress Characteristics of a Vertical Centrifugal Pump Based on Fluid-Structure Interaction. Water. 2023; 15(24):4269. https://doi.org/10.3390/w15244269
Chicago/Turabian StyleLi, Siwei, Yongsha Tu, Changliang Ye, Hongyeyu Yan, Jin Dai, Mengfan Dang, Chunxia Yang, Yuan Zheng, and Yongbiao Li. 2023. "Analysis of Stress Characteristics of a Vertical Centrifugal Pump Based on Fluid-Structure Interaction" Water 15, no. 24: 4269. https://doi.org/10.3390/w15244269
APA StyleLi, S., Tu, Y., Ye, C., Yan, H., Dai, J., Dang, M., Yang, C., Zheng, Y., & Li, Y. (2023). Analysis of Stress Characteristics of a Vertical Centrifugal Pump Based on Fluid-Structure Interaction. Water, 15(24), 4269. https://doi.org/10.3390/w15244269








