Investigation of Hydraulic Performance and Arrangement Optimization of Non-Prismatic Water Conveyance Tunnel—A Case Study of Project X
Abstract
:1. Introduction
2. Experimental Method
2.1. Project Profile
2.2. Experimental Setup
2.3. Similarity Theory
- Discharge scale:
- Velocity scale:
- Time scale:
2.4. Design of Experiment
3. Numerical Simulation
3.1. Geometric Model
3.2. Governing Equation
4. Results
4.1. Validation of Free Flow
- (1)
- Flow pattern
- (2)
- Flow rate
- (3)
- Water surface profile
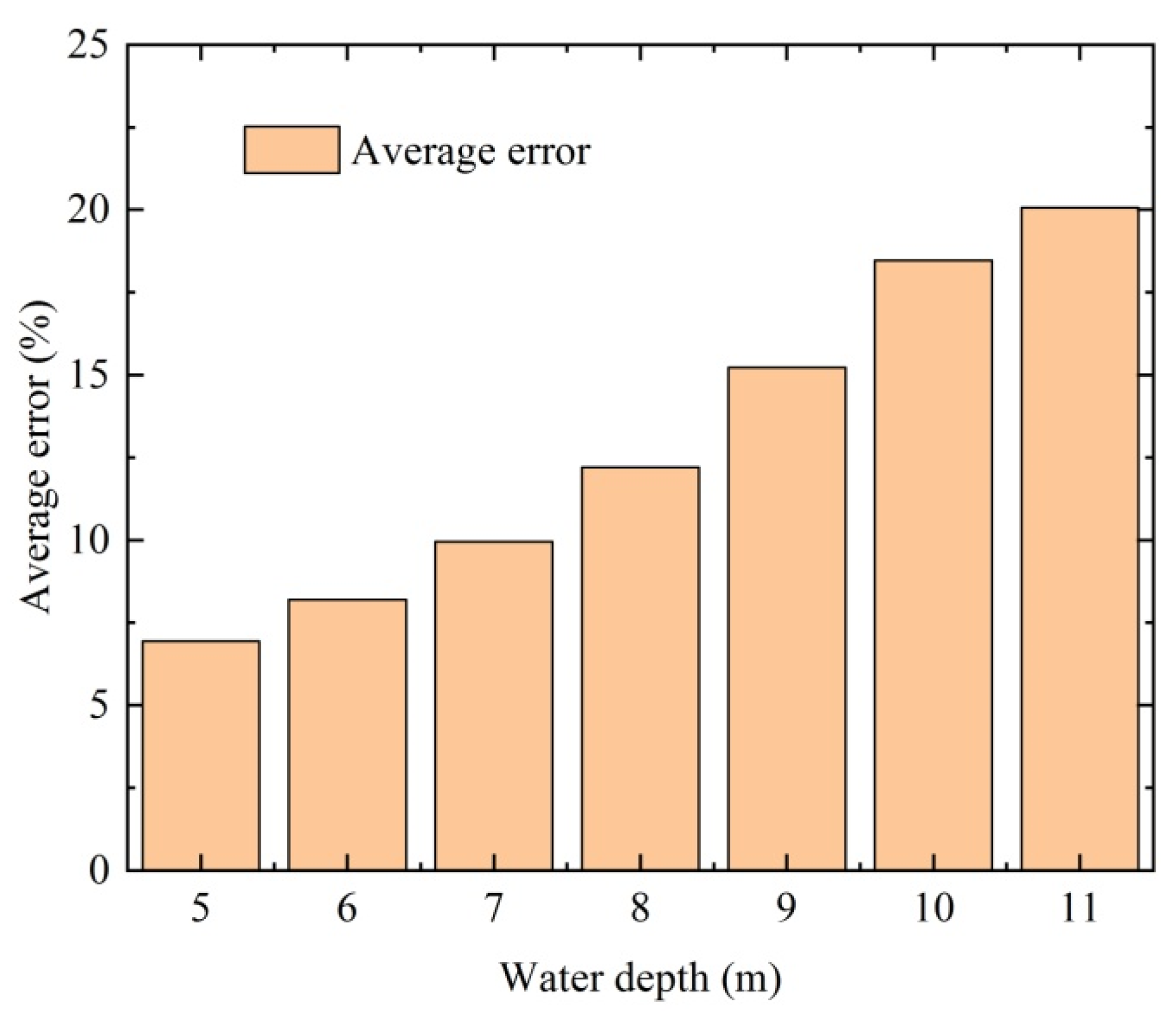
4.2. Hydraulic Discrepancy of Submerged Flow
- (1)
- Flow rate
- (2)
- Velocity along the centerline
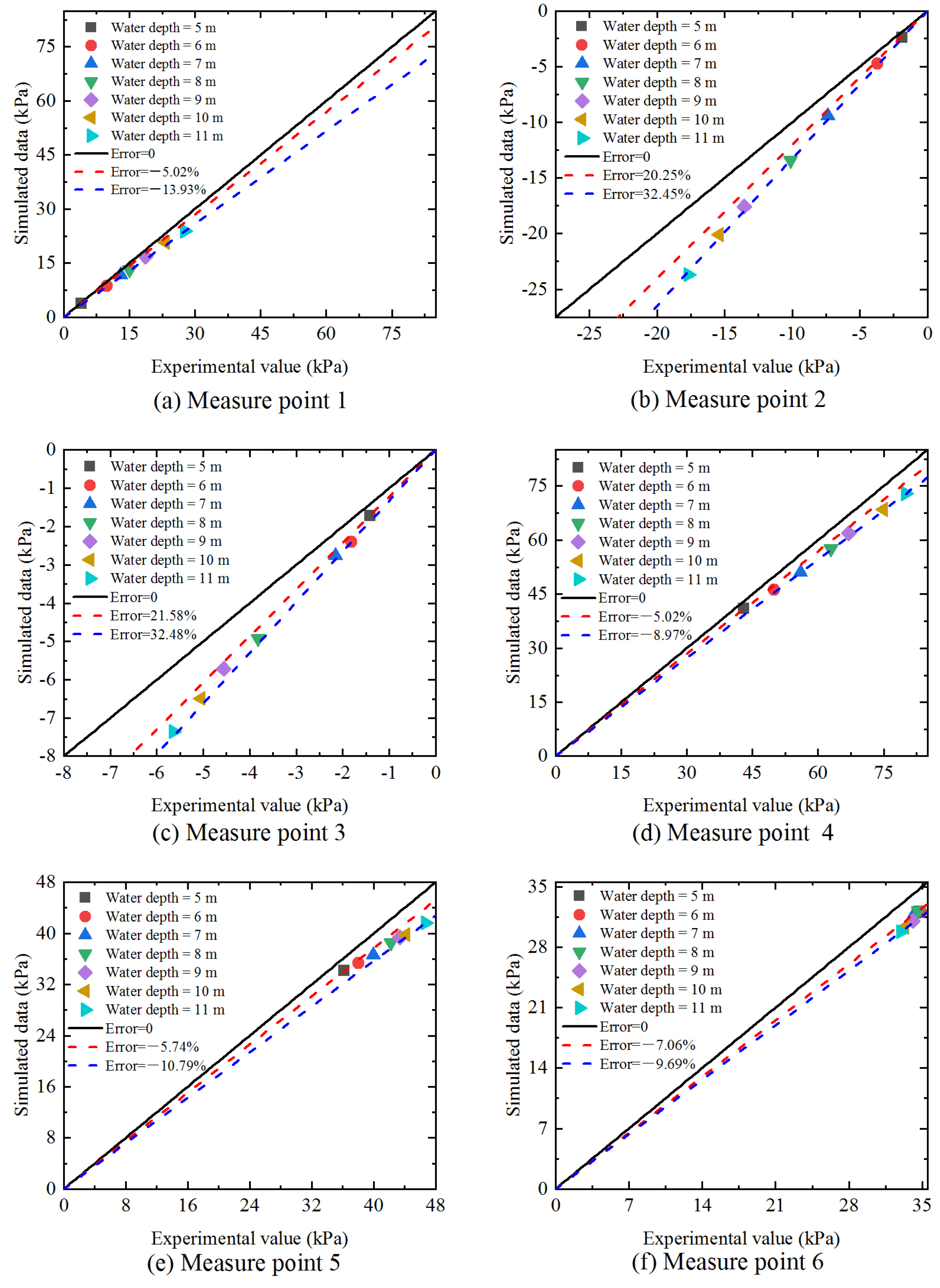
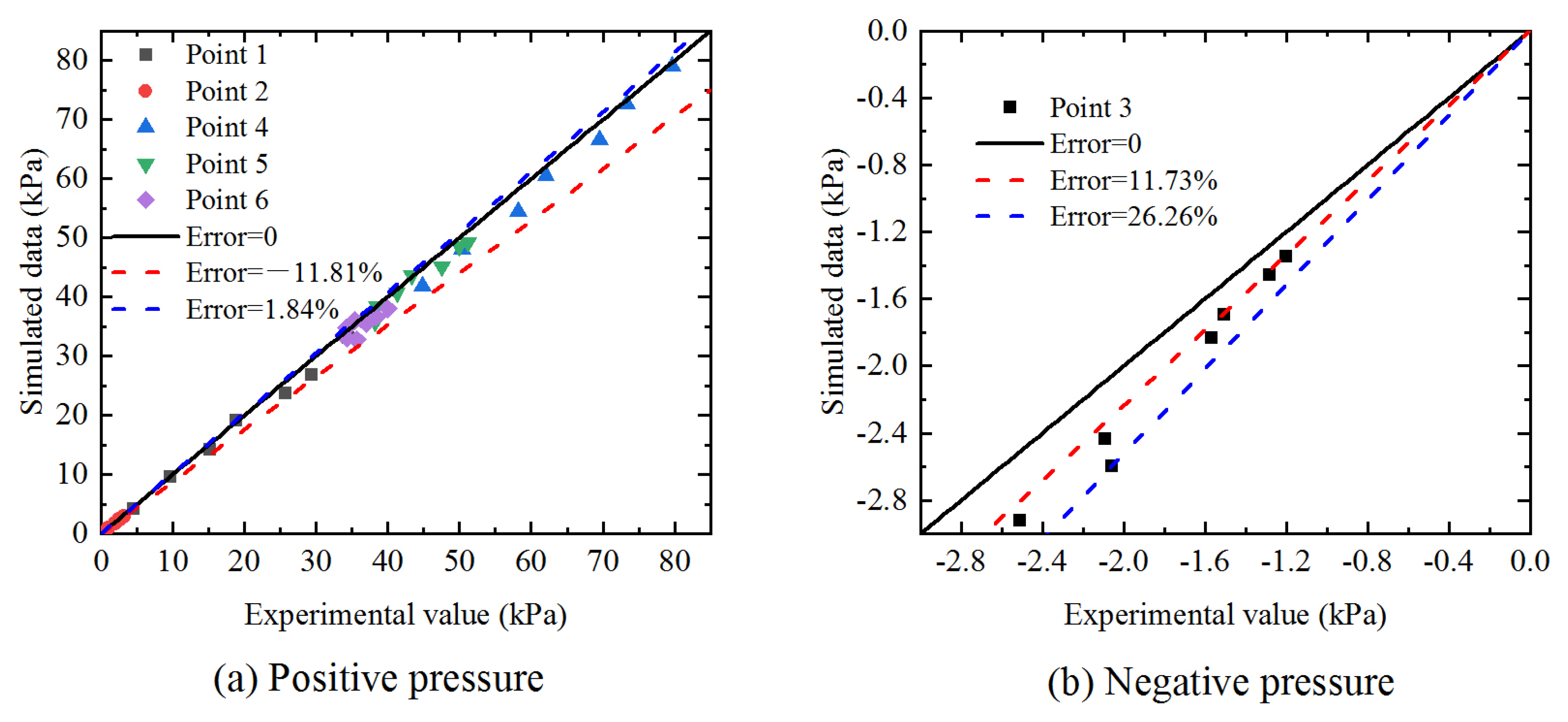
4.3. Hydraulic Performance in Contraction Section of Submerged Flow
- (1)
- Pressure in the contraction section
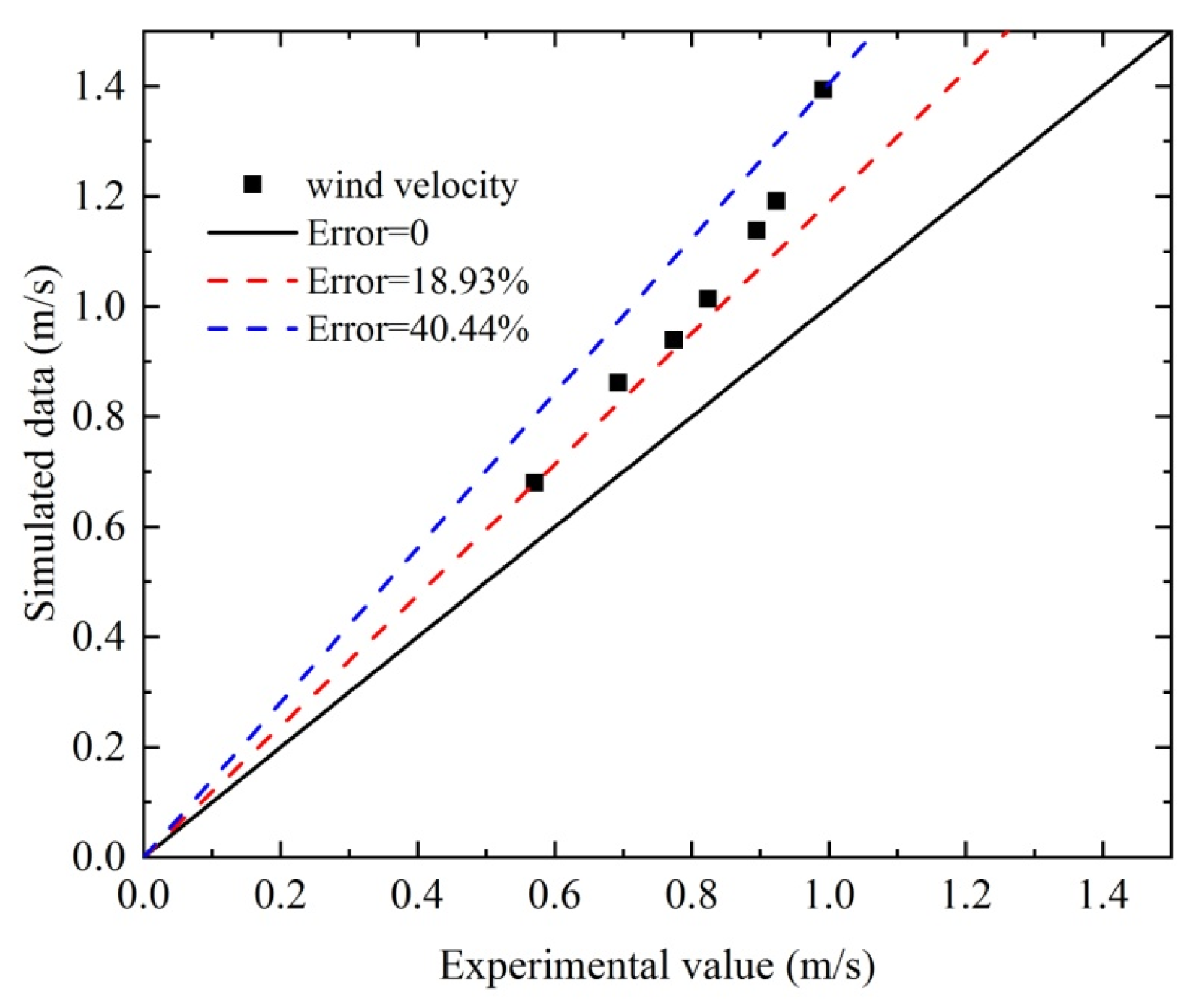
- (2)
- Wind velocity
4.4. Prevention Measures
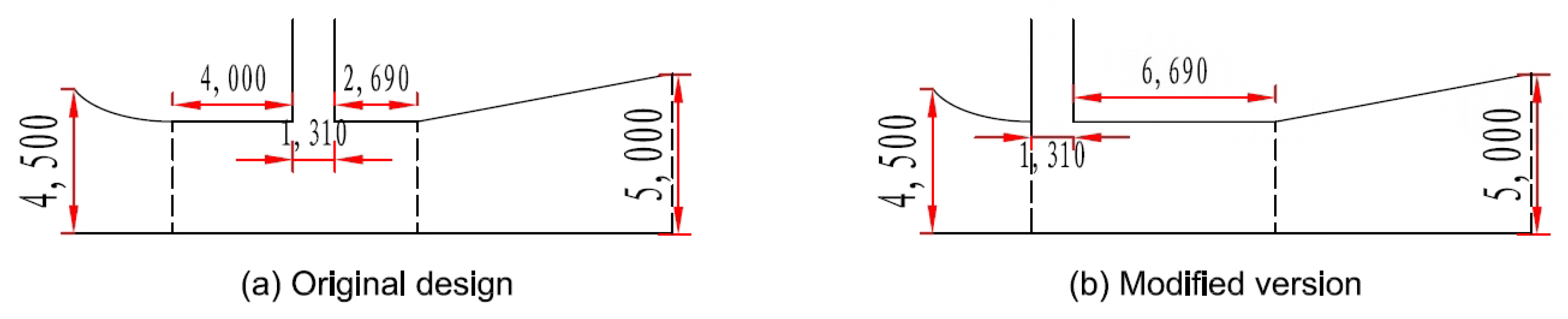
- (1)
- Scheme design
- (2)
- Flow rate
- (3)
- Pressure in contraction section
5. Conclusions
- (1)
- For free flow, the flow characteristics, such as flow pattern, flowrate, and water surface profile, showed good agreement between the experimental results and the simulation predictions; this indicated that the numerical simulation effectively aligned with the experimental scale model test data when assessing the hydraulic performance of a water conveyance tunnel with a contraction section under free flow conditions.
- (2)
- For submerged flow, notable deviations of the flow rate and velocity in non-prismatic tunnels emerged. The primary factor behind this divergence was the development of a vacuum within the distorted cross-sectional area caused by the presence of the contraction section, and the similarity theory proved inadequate for predicting the hydraulic performance during scale model tests.
- (3)
- In order to figure out the applicability of the scale model test and numerical simulation for forecasting the flow state, the hydraulic performances in the contraction section of the submerged flow were analyzed; the results of the pressure and wind velocity verified the inadequacy of the similarity theory in non-prismatic tunnels and indicated the reliability of the simulation method.
- (4)
- The reason for the failure of the scale model test in non-prismatic tunnels is that the vacuum created within the distorted cross-sectional area and the contraction section can be promptly released through the gate shaft. As the vacuum propagates upstream, it gives rise to water entrainment phenomena within the reservoir, which consequently leads to an increase in the flow rate.
- (5)
- To mitigate the adverse effects of negative pressure on both cavitation damage and the hydraulic performance within the tunnel, the placement of the gate shaft was optimized. The vacuum issue was settled in the distorted plan section, and the numerical simulations agreed well with the scale model test. These findings not only validated the accuracy of the CFD analyses, but also substantiated the applicability of the modified design for implementation in water conservancy projects.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Mohaghegh, A.; Wu, J. Effects of hydraulic and geometric parameters on downstream cavity length of discharge tunnel service gate. J. Hydrodyn. 2009, 21, 774–778. [Google Scholar] [CrossRef]
- Li, S.; Zhang, J.M.; Xu, W.L.; Chen, J.G.; Peng, Y. Evolution of pressure and cavitation on side walls affected by lateral divergence angle and opening of radial gate. J. Hydraul. Eng. 2016, 142, 05016003. [Google Scholar] [CrossRef]
- Zhang, J.M.; Xiang, S.; Tang, R.C.; Wang, Q.; Ju, X.M. Calculations and Experiments on Free-Surface-Pressurized Flow in Rectangular Water Conveyance Tunnel with Arch Crown. J. Yangtze River Sci. Res. Inst. 2023, 40, 107. [Google Scholar]
- Sreedhar, B.K.; Albert, S.K.; Pandit, A.B. Cavitation damage: Theory and measurements—A review. Wear 2017, 372, 177–196. [Google Scholar] [CrossRef]
- Liu, B.; Pan, Y.; Ma, F. Pulse pressure loading and erosion pattern of cavitating jet. Eng. Appl. Comput. Fluid Mech. 2020, 14, 136–150. [Google Scholar] [CrossRef]
- Gholizadeh, H.; Behrouj Peely, A.; Karney, B.W.; Malekpour, A. Assessment of groundwater ingress to a partially pressurized water-conveyance tunnel using a conduit-flow process model: A case study in Iran. Hydrogeol. J. 2020, 28, 2573–2585. [Google Scholar] [CrossRef]
- Yang, Y.; He, Y.; Xu, H.; Li, H.; Yin, Z. Determination method of dynamic monitoring indexes for water conveyance tunnels. Adv. Sci. Technol. Water Resour. 2018, 38, 81–85. [Google Scholar]
- Han, Y.C. Horizontal bottomed semi-cubic parabolic channel and best hydraulic section. Flow Meas. Instrum. 2015, 45, 56–61. [Google Scholar] [CrossRef]
- Aminpour, Y.; Farhoudi, J. Similarity of local scour profiles downstream of stepped spillways. Int. J. Civ. Eng. 2017, 15, 763–774. [Google Scholar] [CrossRef]
- Lei, J.; Zhang, J.; Zhang, L. Numerical simulation study on distribution of bubble in flow near aerator based on CFD-PBM coupled model in tunnel. Math. Probl. Eng. 2021, 2021, 6635856. [Google Scholar] [CrossRef]
- Bijankhan, M.; Kouchakzadeh, S. Egg-shaped cross section: Uniform flow direct solution and stability identification. Flow Meas. Instrum. 2011, 22, 511–516. [Google Scholar] [CrossRef]
- Liu, J.L.; Wang, Z.Z.; Leng, C.J.; Zhao, Y.F. Explicit equations for critical depth in open channels with complex compound cross sections. Flow Meas. Instrum. 2012, 24, 13–18. [Google Scholar] [CrossRef]
- Dah-Mardeh, A.; Azizyan, G.; Bejestan, M.S.; Parsaie, A.; Rajaei, S.H. Laboratory Investigation of Scour Downstream of the Stepped Spillway; Springer: Berlin/Heidelberg, Germany, 2023. [Google Scholar]
- Zhang, T.; Hao, R.X.; Zheng, X.Q.; Zhang, Z. Effect of the Area Contraction Ratio on the Hydraulic Characteristics of the Toothed Internal Energy Dissipaters. Water 2019, 11, 1406. [Google Scholar] [CrossRef]
- Zhao, X.; Li, Y.; Zhao, Y.; Zhao, X.; Li, Y.; Zhao, Y.; Li, S.; Yuan, Q.; Jia, W.; Su, X. Experimental Study on Discharge Capacity and Scouring Energy Dissipation of Spillway Tunnel in Qianping Reservoir. Yellow River 2021, 43, 134–136. [Google Scholar]
- Wu, J.; Ai, W. Flows through energy dissipaters with sudden reduction and sudden enlargement forms. J. Hydrodyn. Ser. B 2010, 22, 360–365. [Google Scholar] [CrossRef]
- De Vries, M. Scale Models in Hydraulic Engineering; IHE Delft: Delft, The Netherlands, 1982. [Google Scholar]
- Rydlewicz, W.; Rydlewicz, M.; Pałczyński, T. Experimental investigation of the influence of an orifice plate on the pressure pulsation amplitude in the pulsating flow in a straight pipe. Mech. Syst. Signal Process. 2019, 117, 634–652. [Google Scholar] [CrossRef]
- Heller, V. Scale effects in physical hydraulic engineering models. J. Hydraul. Res. 2011, 49, 293–306. [Google Scholar] [CrossRef]
- Olsen, N.R.B.; Kjellesvig, H.M. Three-dimensional numerical flow modeling for estimation of maximum local scour depth. J. Hydraul. Res. 1998, 36, 579–590. [Google Scholar] [CrossRef]
- Guha, A.; Barron, R.M.; Balachandar, R. Numerical simulation of high-speed turbulent water jets in air. J. Hydraul. Res. 2010, 48, 119–124. [Google Scholar] [CrossRef]
- Lu, L.B.; Tian, Y.; Lei, X.H.; Wang, H.; Qin, T.; Zhang, Z. Numerical analysis of the hydraulic transient process of the water delivery system of cascade pump stations. Water Sci. Technol. Water Supply 2018, 18, 1635–1649. [Google Scholar] [CrossRef]
- Calomino, F.; Alfonsi, G.; Gaudio, R.; D’Ippolito, A.; Lauria, A.; Tafarojnoruz, A.; Artese, S. Experimental and numerical study of free-surface flows in a corrugated pipe. Water 2018, 10, 638. [Google Scholar] [CrossRef]
- Wang, X.; Wang, C.; An, J.; Li, T. Numerical Simulation of Water-Filling Process in Long-Distance Diversion Tunnel with Free Flow. In Proceedings of the 2008 International Symposium on Computer Science and Computational Technology, Shanghai, China, 20–22 December 2008; Volume 1, pp. 499–503. [Google Scholar]
- Mostafazadeh-Fard, S.; Samani, Z. Dissipating Culvert End Design for Erosion Control Using CFD Platform FLOW-3D Numerical Simulation Modeling. J. Pipeline Syst. Eng. Pract. 2023, 14, 04022064. [Google Scholar] [CrossRef]
- Wang, H.; Wang, H.; Zhang, Y. The Effect of Spray-Sprinkler Design Parameters on Energy Consumption Using Computational Fluid Dynamics. J. Irrig. Drain. Eng. 2022, 148, 04022024. [Google Scholar] [CrossRef]
- Formentin, S.M.; Palma, G.; Huerta, J.C.A.; Zanuttigh, B. Statistical assessment of the wave loads at walls through two-phase CFD modeling of the effects of air compressibility. Front. Built Environ. 2023, 9, 1282459. [Google Scholar] [CrossRef]









| Flow Regime | Operating Conditions | |
|---|---|---|
| Upstream Flow Depth in Scaled Model for Experiment (Model Scale Is 1:50) | Upstream Flow Depth in Prototype Model for Simulation | |
| Free flow | 0.01 m | 0.5 m |
| 0.02 m | 1.0 m | |
| 0.03 m | 1.5 m | |
| 0.04 m | 2.0 m | |
| 0.05 m | 2.5 m | |
| 0.06 m | 3.0 m | |
| 0.07 m | 3.5 m | |
| 0.08 m | 4.0 m | |
| Submerged flow | 0. 10 m | 5.0 m |
| 0.12 m | 6.0 m | |
| 0.14 m | 7.0 m | |
| 0.16 m | 8.0 m | |
| 0.18 m | 9.0 m | |
| 0. 20 m | 10.0 m | |
| 0.22 m | 11.0 m | |
| Upstream Flow Depth (m) | Discharge (m3/s) | Relative Deviation | |
|---|---|---|---|
| Experimental Value | Simulation Value | ||
| 0.5 | 1.79 | 1.71 | −4.46% |
| 1 | 4.78 | 4.99 | 4.39% |
| 1.5 | 9.15 | 8.92 | −2.51% |
| 2 | 12.82 | 13.73 | 7.10% |
| 2.5 | 18.21 | 18.67 | 2.52% |
| 3 | 23.32 | 25.15 | 7.85% |
| 3.5 | 30.92 | 31.68 | 2.49% |
| 4 | 36.80 | 38.67 | 5.08% |
| Upstream Flow Depth (m) | Discharge (m3/s) | Relative Deviation | |
|---|---|---|---|
| Experimental Value | Simulation Value | ||
| 5 | 52.3 | 56.06 | 7.19% |
| 6 | 71.19 | 78.28 | 9.96% |
| 7 | 78.58 | 85.56 | 11.43% |
| 8 | 85.35 | 97.84 | 14.63% |
| 9 | 95.12 | 109.69 | 15.32% |
| 10 | 101.77 | 118.98 | 16.91% |
| 11 | 108.43 | 127.89 | 17.94% |
| Flow Regime | Elevation Head | Discharge (m3/s) | Relative Deviation | |
|---|---|---|---|---|
| Experimental Value | Simulation Data | |||
| Free flow | 0.5 | 1.68 | 1.70 | 1.41% |
| 1.0 | 4.77 | 4.93 | 3.42% | |
| 1.5 | 8.76 | 8.93 | 1.94% | |
| 2.0 | 13.52 | 13.08 | −3.24% | |
| 2.5 | 18.57 | 18.73 | 0.88% | |
| 3.0 | 24.93 | 25.25 | 1.27% | |
| 3.5 | 31.42 | 30.31 | −3.53% | |
| 4.0 | 38.29 | 37.31 | −2.56% | |
| Submerged flow | 5.0 | 51.52 | 52.31 | 1.53% |
| 6.0 | 65.53 | 65.95 | 0.63% | |
| 7.0 | 76.32 | 77.67 | 1.77% | |
| 8.0 | 82.21 | 87.88 | 6.90% | |
| 9.0 | 89.07 | 97.17 | 9.10% | |
| 10.0 | 95.63 | 106.24 | 11.09% | |
| 11.0 | 101.98 | 114.28 | 12.06% | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, S.; Yuan, Q.; Zhao, Y.; Cui, H.; Su, X.; Peng, A.; Zhang, Y. Investigation of Hydraulic Performance and Arrangement Optimization of Non-Prismatic Water Conveyance Tunnel—A Case Study of Project X. Water 2024, 16, 311. https://doi.org/10.3390/w16020311
Li S, Yuan Q, Zhao Y, Cui H, Su X, Peng A, Zhang Y. Investigation of Hydraulic Performance and Arrangement Optimization of Non-Prismatic Water Conveyance Tunnel—A Case Study of Project X. Water. 2024; 16(2):311. https://doi.org/10.3390/w16020311
Chicago/Turabian StyleLi, Songping, Qun Yuan, Yuliang Zhao, Hongtao Cui, Xiaoyu Su, Ao Peng, and Yisheng Zhang. 2024. "Investigation of Hydraulic Performance and Arrangement Optimization of Non-Prismatic Water Conveyance Tunnel—A Case Study of Project X" Water 16, no. 2: 311. https://doi.org/10.3390/w16020311
APA StyleLi, S., Yuan, Q., Zhao, Y., Cui, H., Su, X., Peng, A., & Zhang, Y. (2024). Investigation of Hydraulic Performance and Arrangement Optimization of Non-Prismatic Water Conveyance Tunnel—A Case Study of Project X. Water, 16(2), 311. https://doi.org/10.3390/w16020311







